Confinement effect of inert materials on insensitive high explosives
-
摘要: 首先用改进冲击波极曲线理论分析惰性介质对钝感炸药爆轰的约束作用类型。改进冲击波极曲线基于爆轰ZND模型建立在前导冲击波上，并且未反应炸药采用JWL状态方程，惰性介质采用p（ρ，T）形式状态方程。理论考察声速小于炸药CJ爆速且压缩性不同的6种典型惰性介质约束情况。然后用带三项式Lee-Tarver化学反应率的二维Lagrange流体力学方法数值模拟考察约束相互作用。数值考察约束介质的影响因素有：压缩性、厚度、典型双层介质组合约束。从数值结果看出，由介质压缩性的不同给出的约束作用方式共7种：其中6种出现在介质声速小于炸药CJ爆速条件下，可运用冲击波极曲线理论；另外一种出现在介质声速大于炸药CJ爆速条件下，不能使用冲击波极曲线理论。同时，介质厚度、双层介质组合方式也能够影响爆轰前导冲击波阵面形状以及爆轰化学反应流动状态。Abstract: In order to study the confinement effect of inert materials on insensitive high explosives, the improved shock polar curve and phenomenological reaction model were employed.The confinement types were categorized by the improved shock polar theory, which was built on the leading shock wave based on the detonation ZND model, and adopted JWL equation of state in unreacted explosives and p(ρ, T)equation of state in inert material.If the sonic velocity of the confinement material is less than the CJ velocity of an explosive, the shock polar theory can be utilized.In general, there are several types of interactions that give a"match"of the pressure and streamline-deflection across the interface between IHE and confinement material.A two-dimensional Lagrangian hydrodynamic method with three-term Lee-Tarver rate law is used to numerically simulate all types of confinement interactions.The important characters of confinement material include:compressibility, thickness, the representative assembled layers, such as bakelite-iron and iron-beryllium (iron close to the explosive).
-
惰性介质对钝感炸药(IHE)爆轰的约束效应问题, 是爆轰物理学领域非常重要的研究内容。由于钝感炸药爆轰具有爆速更低和化学反应区更宽的特点, 因此钝感炸药爆轰波在惰性介质的约束条件下传播时, 更容易受到约束介质的影响。早期对该问题的研究着重关注钝感炸药驱动惰性介质的做功能力以及被驱动介质获得的速度和位移等物理量, 而对约束作用过程中炸药与惰性介质内部的细致流场图像了解较少。随着精密物理的要求以及数值模拟水平与实验测试技术的提高, 有必要进一步掌握惰性介质对钝感炸药爆轰约束作用的细致流场图像, 以及产生这些细致流场图像的规律与机理。
近年来, 一些研究者从理论、数值模拟与实验方面开展了惰性介质对钝感炸药爆轰的约束效应研究。T.D.Aslam等[1-3]用冲击波极曲线理论把惰性介质约束分为强约束和弱约束等几种类型, 并用二维Euler方法对强约束和弱约束两种类型进行了数值验证。L.G.Hill等[4]设计了一个“三明治”模型来测量有机玻璃和SS304钢对PBX9502炸药约束时的爆轰波阵面形状。C.M.Tarver等[5]和M.L.Garcia等[6]建立了一个三项式的Lee-Tarver化学反应率模型用于研究铜和铝等金属对PBX9502炸药的约束作用。J.W.Banks等[7]和D.S.Stewart等[8]采用多物质流动的高精度二维Euler方法来验证文献[1]中提出的强约束和弱约束2种类型。G.Eden等[9]在实验中用首创的“三明治”模型考察了黄铜和铍对EDC35炸药的约束影响, 在未反应炸药中发现存在预压薄层, 在铍中发现存在弹塑性前驱波和冲击波的双波结构。J.Aveille等[10]在实验中给出铜对TATB基炸药约束作用时的冲击压力, 发现与带程序燃烧模型的二维Lagrange方法的数值模拟结果存在较大的差异。I.A.Balaganskii等[11]在实验中考察了陶瓷材料对TATB基炸药的约束作用, 在陶瓷材料中发现存在由弹塑性波和冲击波组成的双波结构的前驱波。刘尔岩等[12]用数值模拟方法研究了该问题, 孙承纬等[13]用实验方法研究了该问题。数值模拟的二维Lagrange方法使用了较粗的计算网格, 爆轰计算采用程序燃烧模型、Wilkins起爆函数等基于爆轰CJ理论的简单爆轰模型。
本文中以钝感炸药PBX9502为例, 试图进行深入系统的研究来掌握惰性介质对钝感炸药爆轰的约束作用规律与机理。
1. 改进冲击波极曲线理论分析惰性介质对钝感炸药爆轰的约束作用类型
1.1 改进冲击波极曲线理论的建立
传统的冲击波极曲线理论基于爆轰CJ模型。约束相互作用由爆轰产物的反射波极曲线与惰性介质的折射冲击波极曲线决定, 并且爆轰产物的反射波极曲线起始点偏转角为θCJ。
当考虑到钝感炸药的化学反应区较大时, 采用ZND模型更合理, 这时爆轰前导冲击波后流动相对前导冲击波为亚声速。当爆轰波与惰性介质相互作用时, 首先是爆轰前导冲击波在惰性介质中发生折射, 因此, 用建立于前导冲击波上的极曲线理论来分析这种相互作用是更准确的。由于前导冲击波后面的流动是亚声速的, 因此前导冲击波在炸药-惰性介质界面上不会产生任何反射波。这样, 爆轰波与惰性介质相互作用方式由前导冲击波的极曲线与惰性介质的折射冲击波极曲线决定, 并且这2条极曲线的初始点均在坐标原点。
前导冲击波极曲线与未反应炸药状态方程相关, 采用JWL形式状态是准确的。折射冲击波极曲线需要惰性介质的状态方程, 以前多使用多方气体状态方程或线性D-u冲击关系式等简单形式, 为准确这里采用p(ρ, T)形式状态方程[14]。
对JWL状态方程, 冲击波极曲线表达式为
{p=[a(ˉv)−b(ˉv)a(1)/b(1)]/[1−(1−ˉv)b(ˉv)/(2ρ0)]tanθ=√ρ0D2p(1−ˉv)−p2/(ρ0D2−p) 式中: ˉv=vv0,a(ˉv)=A(1−ωR1ˉv)exp(−R1ˉv)+B(1−ωR2ˉv)exp(−R2ˉv),b(ˉv)=ωρ0ˉv, 且ρ0、D是炸药密度和爆轰波速度, 其中A、B、R1、R2、ω是与未反应炸药相关的常数。
p(ρ, T)形式状态方程的表达式为
{p(ρ,T)=pc(ρ)+pn(ρ,T)+pe(ρ,T)E(ρ,T)=Ec(ρ)+En(ρ,T)+Ee(ρ,T) 式中:E(ρ, T)表示比内能, 等式右端3项分别表示冷压(冷能)、原子核热压(原子核热能)、电子热压(电子热能), 各项物理意义及相应表达式见文献[14]。
对p(ρ, T)形式状态方程, 冲击波极曲线表达式为
{E−E0=(p+p0)(v0−v)/2tanθ=±√(ρ0D)2(p−p0)(v0−v)−(p−p0)2/[ρ0D2−(p−p0)]p=p(ρ,T)E=E(ρ,T) 式中:v0、p0、D分别是惰性介质比容、初始压力及爆轰波速度。
本文中建立的改进冲击波极曲线理论与传统的冲击波极曲线理论所计算出的黄铜约束钝感炸药PBX9502的爆轰边缘角分别为78.8°、82.9°[13], 可以看出本文的理论结果很接近实验结果78.5°[15], 可以认为本文的改进冲击波极曲线理论是合理的。
1.2 惰性介质对钝感炸药爆轰的约束作用类型的理论分析
惰性介质对钝感炸药爆轰的约束作用类型, 首先可分为具有定常流动结构的约束和非定常流动结构的约束两大类。在惰性介质声速小于炸药CJ爆速条件下(PBX9502的CJ爆速约为7.655 km/s), 炸药-惰性介质界面附近的流动具有定常结构, 这种约束作用类型为具有定常流动结构的约束, 这时的约束作用可以采用冲击波极曲线理论来分析。
经理论分析, 具有定常流动结构的约束作用再细分为如图 1所示的6种类型, 典型的约束介质分别为钢、酚醛树脂、有机玻璃、泡沫塑料、氢化锂、氘化锂, 图中“Ce”点为未反应炸药前导冲击波的声速状态, “Cm”点为惰性约束介质折射冲击波的声速状态。
图 1 具有定常流动结构的约束作用极曲线图Figure 1. The polar curves of the confinement interactions with steady flow structures从图 1(a)所示情况看出, 两曲线交于炸药极曲线的强支, 因此叫做“强约束”, 同时, 交点也位于惰性介质极曲线的弱支, 惰性介质中出现超声速流动。从图 1(b)所示情况看出, 两曲线交于从炸药前导冲击波极曲线上声速点发出的稀疏曲线, 因此叫做“弱约束”, 同时, 交点也位于惰性介质极曲线的弱支, 惰性介质中会出现超声速流动。从图 1(c)所示情况看出, 惰性介质极曲线分别与炸药极曲线的强支和从炸药前导冲击波极曲线上声速点发出的稀疏曲线相交, 同时, 这2个交点也分别位于惰性介质极曲线的强支和弱支。从图 1(d)所示情况看出, 惰性介质极曲线分别与炸药极曲线的强支、弱支以及从炸药前导冲击波极曲线上声速点发出的稀疏曲线相交, 同时, 这3个交点有2个位于惰性介质极曲线的强支, 另1个位于其弱支。从图 1(e)所示情况看出, 两曲线交于炸药极曲线的弱支, 同时, 交点位于惰性介质极曲线的强支。从图 1(f)所示情况看出, 两曲线无交点。
2. 唯象化学反应率方法数值模拟惰性介质对钝感炸药爆轰的约束作用
2.1 三项式的Lee-Tarver化学反应率数值模拟方法
本文中使用的化学反应率表达式为
dF dt=I(η−1−a)n(1−F)yH(FI−F)+G1(1−F)y1Fx1Pz1H(FG1−F)+G2(1−F)y2Fx2Pz2H(F−FG2) 式中:F是反应道, H(*)表示单位阶梯函数, 其余各常数项物理意义见文献[5-6]。
数值模拟采用显式有限差分的二维Lagrange流体力学程序[16], 炸药采用JWL状态方程, 惰性介质采用p(ρ, T)形式状态方程。计算模型如图 2所示, 炸药和惰性介质长7.0 cm, 炸药厚2.0 cm, 上边界为固壁, 右端平面起爆; 网格划分为200 cells/cm。
2.2 数值模拟惰性介质对钝感炸药爆轰的约束作用
在惰性介质声速大于CJ爆速条件下, 炸药-惰性介质界面附近的流动具有非定常结构, 这种约束作用类型为具有非定常流动结构的约束, 介质中的应力波将运动到爆轰波的前面形成前驱波, 这时冲击波极曲线理论失效。同时, 为了进一步给出爆轰化学反应区内部以及惰性介质内部的流动状态, 使用数值模拟是必要的。考察惰性介质如下3个方面的重要性质对钝感炸药爆轰结构的影响:压缩性的不同、厚度的不同、典型双层介质组合约束。
2.2.1压缩性的影响
压缩性包括介质声速小于炸药CJ爆速以及介质声速大于炸药CJ爆速2种情况。在介质声速小于CJ爆速条件下, 炸药-介质界面附近的流动具有定常结构, 这里选择的约束介质与极曲线理论分析相对应, 仍为钢、酚醛树脂、有机玻璃、泡沫塑料、氢化锂、氘化锂等6种; 在介质声速大于CJ爆速条件下, 炸药-介质界面附近的流动具有非定常结构, 典型的约束介质选为铍(铍标准状态声速约为7.95 km/s)。这里惰性介质厚0.5 cm。
具有定常结构的6种约束作用的数值模拟结果如图 3所示, 图中给出了压力等值线, 炸药区同时给出了前导冲击波阵面、声速线以及化学反应结束线。从图 3(a)所示“强约束”情况看出, 炸药前导冲击波阵面整体向后弯曲, 但炸药-惰性介质附近的弯曲程度较小, 化学反应区较薄, 而达到声速状态的距离更长, 并且惰性介质内部出现交替的压缩和膨胀过程。从图 3(b)所示“弱约束”情况看出, 炸药前导冲击波阵面整体向后弯曲, 但界面附近的弯曲程度较大, 化学反应区较厚, 声速线与前导冲击波阵面在界面上相交, 并且惰性介质内部出现中心稀疏波。从图 3(c)所示“一强一弱约束”情况看出, 炸药前导冲击波阵面整体向后弯曲, 流场结构与“弱约束”类似。从图 3(d)所示“三弱解约束”情况看出, 炸药区内部流动状态与“弱约束”情况类似, 但惰性介质内部出现亚声速区。从图 3(e)所示“一超声速弱解约束”情况看出, 炸药前导冲击波阵面整体向后弯曲, 但界面附近的前导冲击波阵面局部向前弯曲, 化学反应区较厚, 声速线与前导冲击波在界面上相交, 且惰性介质内部出现亚声速区。从图 3(f)所示“无解约束”情况看出, 炸药前导冲击波阵面和声速线整体向前弯曲, 惰性介质内部出现局部亚声速区。
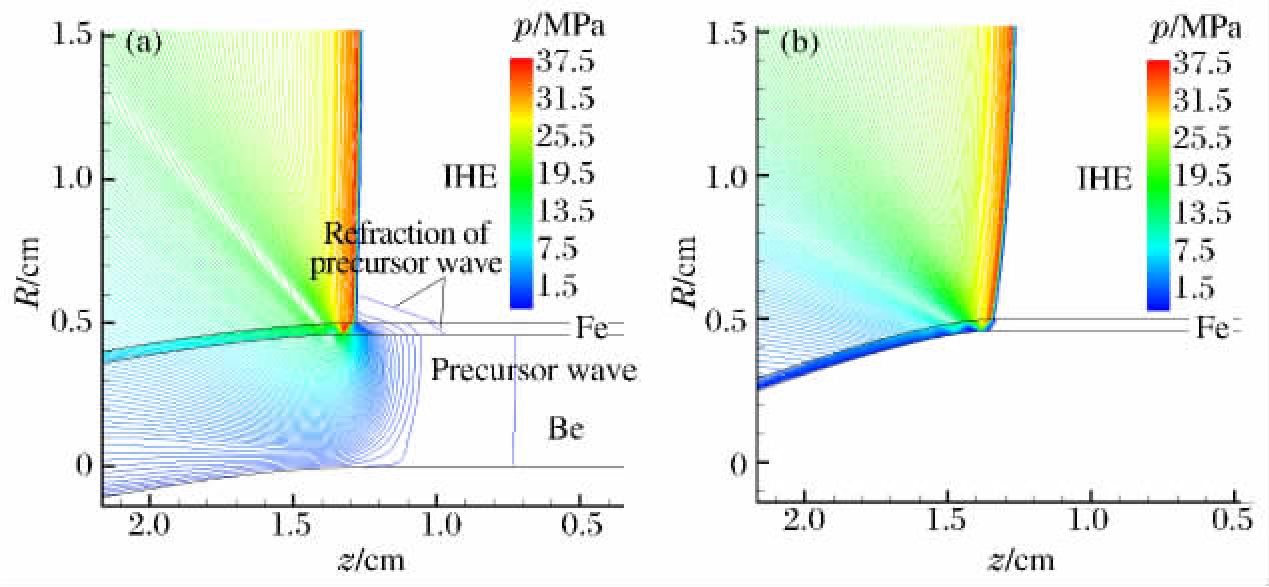
图 3 具有定常流动结构的约束作用的流场图Figure 3. The flow fields of the confinement interactions with steady flow structures具有非定常结构的约束作用的数值模拟结果如图 4所示, 图中给出了压力等值线, 炸药区同时给出前导冲击波阵面、声速线以及化学反应结束线。计算时铍采用Johnson-Cook本构模型。可以看出, 炸药前导冲击波阵面整体向前弯曲, 在炸药界面附近爆轰流动先达到声速状态然后达到化学反应结束状态, 而离炸药界面一定距离处爆轰流动先达到化学反应结束状态然后达到声速状态, 声速线与化学反应结束线在炸药区内部相交; 铍介质内的应力波比炸药爆轰波传播得更快而成为前驱波, 并且随时间增长, 铍介质内的应力波与炸药爆轰波的距离越来越大, 流动呈现非定常性质; 铍介质内的前驱波在传播过程中向炸药折射应力波, 从而使炸药前导冲击波阵面之前的未反应炸药被预先压缩。
图 4 具有非定常流动结构的约束作用爆轰流场图Figure 4. The flow field of the confinement interaction with unsteady flow structure特别地, 铍介质内的应力波结构如图 5所示。这是一个双波结构:第1个应力波为弹性波, 波速约为13.53 km/s; 第2个应力波为冲击波, 波速约为8.56 km/s。显然, 这2个应力波均为前驱波, 相应地2个前驱应力波会在未反应炸药内产生2个折射应力波。
2.2.2介质厚度的影响
这里给出钢厚度为0.5、0.02 cm条件下, 炸药区流动结构的模拟结果, 如图 6所示。从数值结果看出, 当作为约束介质的钢更薄时, 炸药前导冲击波阵面更弯曲, 声速线和化学反应结束线也更弯曲, 这是因为薄约束介质自由面处的扰动会传进爆轰化学反应区, 使得化学反应变慢, 进而影响炸药-钢界面附近的流动状态。图 7给出钢厚度对炸药-钢界面附近的爆轰波法向速度的影响, 可以看出当约束介质更薄时, 爆轰波法向速度变慢, 并且厚度越小影响越显著。
图 7 钢厚度对爆轰波法向速度的影响Figure 7. Effect of the thickness of steel on normal speed of detonation2.2.3典型双层介质组合约束的影响
本文中考察了双层组合介质酚醛树脂-钢、钢-铍的约束影响, 这里仅给出钢-铍约束情况的结果。钢厚度为0.04 cm, 铍厚度为0.46 cm, 铍同样使用Johnson-Cook本构模型, 且钢靠近炸药。与仅单层0.04 cm厚的钢约束情况相比较, 结果如图 8所示, 可以看出, 钢-铍的组合约束条件下, 炸药前导冲击波阵面、声速线、化学反应结束线的弯曲度均变小, 因此钢-铍的双层组合约束比单层钢的约束更强。特别地, 双层组合介质钢-铍约束条件下, 由于铍的声速大, 铍介质中产生的前驱应力波会使钢介质中也出现前驱波, 钢中前驱波向炸药折射应力波从而预先压缩未反应炸药, 如图 9(a)所示; 仅单层钢约束时, 钢中不出现前驱波, 如图 9(b)所示。显然, 铍介质的扰动会通过钢介质传到爆轰化学反应区内影响化学反应的快慢, 使得钢-铍双层组合介质与单层钢介质对爆轰反应流动的约束影响更大。
3. 结论
(1) 建立了分析惰性介质对钝感炸药爆轰的约束作用的改进冲击波极曲线理论。
(2) 改进冲击波极曲线理论与唯象化学反应率数值模拟相结合, 由介质压缩性的不同给出约束作用方式共7种:其中6种出现在惰性介质的声速小于炸药CJ爆速的条件下, 它们具有定常结构, 可运用冲击波极曲线理论; 另外1种出现在惰性介质的声速大于炸药CJ爆速的条件下, 它具有非定常结构, 不能使用冲击波极曲线理论。
(3) 约束介质的厚度对爆轰状态有影响:当介质更薄时, 爆轰反应区内化学反应变慢, 并且厚度越小影响越显著。双层介质组合方式对爆轰状态也有影响:如果靠近炸药的介质较薄, 炸药的反应流动状态既会受到靠近炸药的约束介质压缩性的影响, 也会受到外层介质压缩性的影响, 文中给出的酚醛树脂-钢与钢-铍约束均比单层酚醛树脂与钢约束更强。
(4) 对铍等声速大于炸药CJ爆速的惰性介质, 约束作用过程中通常会出现双波结构的前驱应力波:弹性波在前, 冲击波在后, 此前驱应力波会预先压缩未反应炸药。
-
图 1 具有定常流动结构的约束作用极曲线图
Figure 1. The polar curves of the confinement interactions with steady flow structures
图 3 具有定常流动结构的约束作用的流场图
Figure 3. The flow fields of the confinement interactions with steady flow structures
图 4 具有非定常流动结构的约束作用爆轰流场图
Figure 4. The flow field of the confinement interaction with unsteady flow structure
图 7 钢厚度对爆轰波法向速度的影响
Figure 7. Effect of the thickness of steel on normal speed of detonation
-
[1] Aslam T D, Bdzil J B. Numerical and theoretical investigation on detonation-inert confinement interactions[C]//The 12th Symposium(International)on Detonation. San Diego, California, 2002: 483-488. [2] Aslam T D, Bdzil J B. Analysis of the LANL detonation-confinement test[C]//Proceedings of the Conference on Shock Compression of Condensed Matter. Portland, OR, 2003: 831-834. [3] Aslam T D, Bdzil J B. Numerical and theoretical investigation on detonation confinement sandwich tests[C]//The 13th Symposium(International)on Detonation. Norfolk, VA, 2006: 6-10. [4] Hill L G, Aslam T D. The LANL detonation-confinement test: Prototype development and sample results[C]//Proceedings of the Conference on Shock Compression of Condensed Matter. Portland, OR, 2003: 847-850. [5] Tarver C M, McGuire E M. Reactive flow modeling of the interaction of TATB detonation waves with inert materials[C]//The 12th Symposium(International)on Detonation. San Diego, California, 2002: 641-649. [6] Garcia M L, Tarver C M. Three-dimensional ignition and growth reactive flow modeling of prism failure tests on PBX9502[R]. UCRL-CONF-222376, 2006. [7] Banks J W, Schwendeman D W, Kapila A K, et al. A study of detonation propagation and diffraction with compliant confinement[R]. UCRL-JRNL-233735, 2007. [8] Stewart D S, Yoo S, Wescott B L. High-order numerical simulation and modelling of the interaction of energetic and inert materials[J]. Combustion Theory and Modelling, 2007, 11(2): 305-332. doi: 10.1080/13647830600876629a [9] Eden G, Belcher R A. The effects of inert walls on the velocity of detonation in EDC35[C]//The 9th Symposium(International)on Detonation. Portland, Oregon, 1989: 831-841. [10] Aveille J, Carion N. Experimental and numerical study of oblique interactions of detonation waves with explosives/solid material[C]//The 9th Symposium(International)on Detonation. Portland, Oregon, 1989: 842-851. [11] Balaganskii I A, Agureikin V A. Effect of an inert high-modulus ceramic wall on detonation propagation in solid explosive charges[J]. Combustion, Explosive and Shock Waves, 1994, 30(5): 674-681. doi: 10.1007/BF00755836 [12] 刘尔岩, 王元书, 刘邦弟.爆轰波与金属的斜相互作用[J].爆炸与冲击, 2002, 22(3): 203-209. doi: 10.3321/j.issn:1001-1455.2002.03.003Liu Er-yan, Wang Yuan-shu, Liu Bang-di. Interaction between detonation and metals[J]. Explosion and Shock Waves, 2002, 22(3): 203-209. doi: 10.3321/j.issn:1001-1455.2002.03.003 [13] 孙承纬, 卫玉章, 周之奎.应用爆轰物理[M].北京: 国防工业出版社, 2000. [14] 经福谦.实验物态方程导引[M]. 2版.北京: 科学出版社, 1999. [15] Bdzil J B. Steady-state two-dimensional detonation[J]. Journal of Fluid Mechanics, 1981, 108(2): 195-206. http://adsabs.harvard.edu/abs/1981JFM...108..195B [16] 李德元, 徐国荣, 水鸿寿, 等.二维非定常流体力学数值方法[M].北京: 科学出版社, 1987. 期刊类型引用(1)
1. 于明, 孙宇涛, 刘全. 爆轰波在炸药-金属界面上的折射分析. 物理学报. 2015(11): 282-291 . 
百度学术其他类型引用(0)
-
下载:
下载:
百度学术
计量
- 文章访问数: 3237
- HTML全文浏览量: 452
- PDF下载量: 392
- 被引次数: 1