Constitutive model of transparent aviation polyurethane at high strain rates
-
摘要: 采用低阻抗分离式霍普金森压杆对航空透明聚氨酯进行了高应变率下的动态力学性能测试,得到的应力应变曲线表现出了显著的非线性黏弹性特征。基于本构理论和实验数据,构建了航空透明聚氨酯的松弛时间应变相关的超黏弹性本构形式。该本构模型由2部分组成:一部分表征准静态下的超弹性行为,另一部分描述非线性应变率的相关特性。利用超黏弹性本构模型对不同应变率下航空透明聚氨酯的动态应力应变曲线进行拟合,拟合曲线与实验曲线一致性良好。Abstract: The uniaxial compressive properties of aviation polyurethane were investigated experimentally by using a modified aluminum split Hopkinson pressure bar apparatus. The obtained stress-strain curves presented distinct non-linear viscoelastic characteristic.Based on the constitutive theory and the experimental data, a hyper-viscoelastic constitutive model that incorporated a strain-dependent relaxation time was proposed to describe the large compressive deformation response of incompressible aviation polyurethane at high strain rates. The proposed model was made up of two parallel mechanical elements-one component to characterize quasi-static hyperelastic behavior, and the other to define rate-sensitivity and strain history dependence. The predictions of the mechanical behavior using a hyper-viscoelastic constitutive model based on strain energy functions and hereditary approach had a good agreement with experimental results.
-
Key words:
- solid mechanics /
- constitutive model /
- SHPB /
- aviation polyurethane /
- high strain rate /
- hyper-viscoelastic
-
分离式霍普金森杆实验技术广泛应用于材料的动态力学性能测试,经典SHPB系统的撞击杆一般长200~800 mm,对于通常采用的钢杆或铝杆,其对应的加载时间约为80~320 μs,既难以实现材料在不太高的应变率下的高压缩应变,也难以实现一定应变下的中应变率加载。而延长有效加载时间,最直接的方法就是采用超长杆系统,简单地将撞击杆、入射杆和透射杆加长,即可获得更长的加载时间。Song等[1]采用撞击杆长为2.5 m、总长27.4 m的超长SHPB系统,结合泡沫整形器,获得了3 ms以上的加载时长,但加载应力较低,只适用于软材料的动态力学性能测试。超长杆系统最大的优势就是沿用传统的测量技术和数据处理方法,简洁方便,但对于实验场地和杆件加工有较高的要求,推广较为困难。Zhao等[2]提出了采用液压装置取代撞击杆的“慢杆”霍普金森压杆装置,原则上可以实现从准静态到中高应变率材料的动态性能测试,但从已有的研究[2-3]看,该技术仅限于软材料,且存在应变率不够稳定的问题,加上其结构相对复杂,因此应用较少。
直撞式霍普金森压杆(direct-impact Hopkinson bar, DHB)技术最早由Dharan等[4]提出, 后由Wulf[5]、Gorham等[6]、Shioiri等[7]予以改进, 该技术的提出基于对高应变率(104 s−1量级)冲击压缩实验的需要,它解决了常规压杆的最大加载速度受限于压杆屈服强度的问题。DHB也可用于实现试件在中高应变率下的大变形,如Zhao等[8]、Liu等[9]、陈浩等[10]给出了大变形直撞式压杆的一般设计方法。Gilat等[11]利用液压装置对试件直接加载,其稳定压缩加载时间约为6 ms,但其透射杆长达40 m。Whittington等[12]提出了一种折叠型压杆结构来增加压杆的有效长度,从而实现较长时的加载及测试,可视为超长杆的变型,该结构可以节省空间,但折叠联结更为困难,且易产生应力波反射干扰。利用入射杆中的反射波对试件多次加载是另一种实现材料大变形的方法,Lindholm[13]最早使用该方法测试了铝合金,Xia等[14]结合Lindholm提出的方法实现了精准可控的多脉冲加载。巫绪涛等[15]利用反射波再加载测试了泡沫铝,由于应力波过长,因此采用了两片法处理数据。虽然该方法能够延长加载时间,但是其多次加载之间出现了完全卸载,很难说是真正的延长了加载时间。
本文中提出一种直撞式二次加载霍普金森压杆(direct-impact double-loading Hopkinson bar, DDHB)实验技术,该技术仅需在直撞式压杆系统的透射杆末端加设一大质量钢块,利用其在实验中形成的近似刚性壁反射应力波来实现试件的二次加载。应力波的波形重叠问题则采用二点法[16-17]波分离技术来解决,并通过数值模拟和实验验证该技术的可行性和准确性。
1. 直撞式二次加载技术
1.1 实验原理
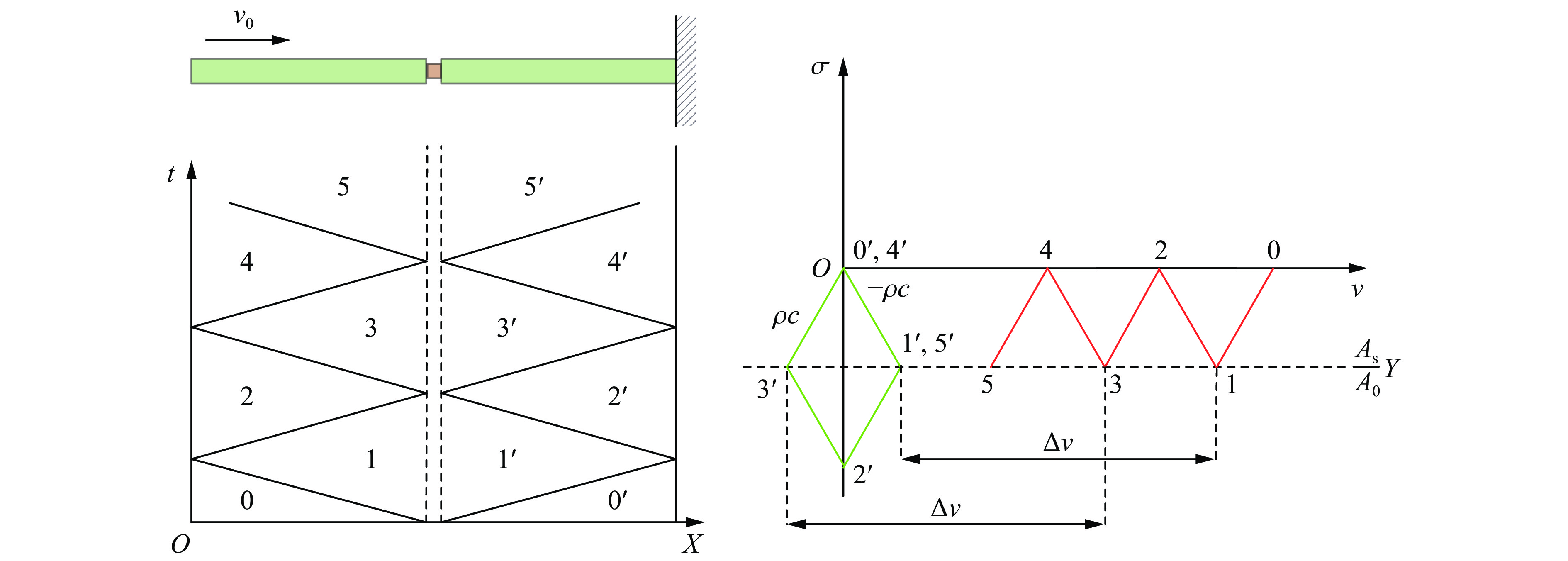
在透射杆末端设置一个大质量钢块,由一维应力波传播理论可知[18],当应力波传播到杆-质量块界面时将形成应力波反射,由于钢块的广义波阻抗远大于压杆的广义波阻抗,可将质量块视为准刚性壁,因此反射波与入射波同号,幅值近似相同,即可形成对试件的二次加载。DDHB系统如图1所示。
假定试件为理想刚塑性材料,屈服强度为Y,长度为ls,截面积为As;撞击杆与透射杆的长度均为l,弹性模量为E,截面积为A0,密度为ρ,弹性波速为c,撞击速度为v0。由一维应力波理论可得撞击过程中的应力波传播轨迹(X-t图)、质点速度和应力状态(σ-v图),如图2所示。
撞击杆撞击试件时,两者相互作用产生的压缩波对试件形成第一次加载。左行压缩波(区域1)在撞击杆中传播到自由端后反射形成右行拉伸卸载波,右行压缩波(区域1′)在透射杆中传播到刚性壁后反射形成左行压缩加载波,右行拉伸卸载波与左行压缩加载波同时到达试件两端面,形成第二次加载。第一次加载试件两端分别处于区域1、1′的状态,而第二次加载试件两端分别处于区域3、3′的状态,由图2中σ-v图可知,两次加载的速度差均为
Δ v,即两次加载的工程应变率相等:˙ε0=(v0−2YAsρcA0)/ls0≤t<4T (1) 式中:
T=l/c 。直撞式二次加载可实现4l/c 的有效加载历时,一个1.5 m+1.5 m的DDHB系统可实现1.2 ms的加载;考虑一般实验室条件,将撞击杆和透射杆加长至6 m+6 m,则DDHB系统可实现高达4.8 ms的加载。如果试件为一般的弹塑性材料,通过数值分析可知,有效加载时间仍能达到4T,但加载应变率相对于刚塑性材料有一定的下降,且在第二次加载起始处应变率小幅度突降,如图3所示。造成该现象的主要原因是,应力波在试件中传播需要一定的时间,等长的撞击杆与透射杆会导致二次加载时两端应力波不能同时到达试件。同时,从图中可以看出,采用相同长度的杆件,SHPB系统的加载时长为T,DHB系统的加载时长为2T,而DDHB系统的加载时长为4T。
当撞击杆初速度较大(
v0>4YAs/ρcA0 )时,DDHB系统还可以4T为一个周期形成多次加载,每个周期的加载应变率均下降Δ˙ε ,即:˙εn=ε0−nΔ˙ε=(v0−(2+4n)YAsρcA0)/ls (2) 式中:
n=[t/(4T)] ,Δ˙ε=4YAs/(ρcA0ls) 。若针对软材料(Y较小)、小尺寸试件(As较小),提高撞击杆速度(v0较大),使得Δ˙ε≪˙ε0 ,可近似实现对试件的多次有效加载,加载时间可高达4nT。需要说明的是,对于应变硬化显著的弹塑性材料,直撞式霍普金森压杆技术由于无法采用整形器整形,从而较难实现恒应变率加载,因此材料在加载过程中的应变率下降较显著,通过式(1)可以简单估算工程应变率的下降量。1.2 波反演技术
由于采用了等长的撞击杆和透射杆来提高加载时间,应力波将会在杆件中出现重叠。因此采用两点法波分离技术[16-17]分离杆中叠加的左行和右行应力波,应力波分离技术参见文献[19]。如图4所示,以撞击端为原点,并以撞击时刻为时间原点,当杆的左端受到撞击时产生右行波。当
t≤tA 时,点A处应变片所记录的信号εA(t) 就是点A处的右行波εr,A(t) ,其中tA=(2L−lA)/c ,lA为点A到原点的距离,L为杆的长度;当t>tA 时,εA(t) 是t时刻点A处的右行波εr,A(t) 与反射回来的左行波εl,A(t) 的叠加,即:εA(t)=εr,A(t)+εl,A(t) (3) 由于杆中的弹性波均为简单波,因此点A处的左行波
εl,A(t) 与点B处的左行波εl,B(t) 传播Δt 后的波形一致,即:εl,A(t)=εl,B(t−Δt) (4) 式中:
Δt=(lB−lA)/c ,lB为点B到原点的距离。由叠加关系可知,点B处应变片所记录的信号εB(t−Δt)=εl,B(t−Δt)+εr,B(t−Δt) ,而点B的右行波εr,B(t) 与点A的右行波εr,A(t) 之间的关系为:εr,B(t)=εr,A(t−Δt) 。点A的右行波εr,A(t) [9]为:εr,A(t)={εA(t)t<tAεA(t)−εB(t−Δt)+εr,A(t−2Δt)t≥tA (5) 同理,点B的左行波
εl,B (t)为:εl,B(t)={0t<tBεB(t)−εA(t−Δt)+εl,B(t−2Δt)t≥tB (6) 式中:
tB=(2L−lB)/c 。值得注意的是,当t≤tB 时,左行波未到达点B,因此εl,B (t)=0。1.3 质量块尺寸对二次加载的影响
应力波在相同材质的变截面杆中将发生透反射,其反射系数[18]为:
F=1−n1+n (7) 式中:n=(ρ0c0A)1/(ρ0c0A)2,ρ0c0A为广义波阻抗,下标1和2表示变截面的前后截面。当杆的直径为16 mm时,可计算得到反射系数为98%时质量块的直径D为160 mm。为了弄清楚质量块长度对反射的影响,建立有限元模型,分析质量块长度分别为其直径的0.5、1.0、1.5、2.0、3.0倍时的反射系数。如图5所示,当质量快长度大于240 mm时,继续增加杆长对反射系数的影响不明显,因此选取直径为杆径10倍、长度为杆径20倍的质量块即可较好地实现准固壁反射。
1.4 试件受力及变形计算
为了计算试件的应力与应变,必须获得透射杆左端面的应变信号
ε0(t) 以及质点速度v2,而ε0(t) 是左端面的右行波εr,0(t) 与左行波εl,0(t) 的简单叠加,其中εr,0(t) 与εr,A(t) 传播lA/c 前的波形一致,εl,0(t) 与εl,B(t) 传播lB/c 后的波形一致,即:ε0(t)=εr,A(t+lAc)+εl,B(t−lBc) (8) 而透射杆左端面的质点速度v2可由下式[2]确定:
v2=c(εr,A(t+lAc)−εl,B(t−lBc)) (9) 沿用经典霍普金森杆应力平衡假定,结合初始碰撞速度v0和透射杆信号,能够获得撞击杆端面质点速度。当2NT≤t≤2(N+1)T,试件左端面的质点速度可由下式[8]确定:
v1=v0−1ρcA0(F(t)+N∑i=12F(t−2iT)) (10) 式中:
F(t)=ε0(t)EA0 ,N=[t/(2T)],[a]代表对数a取整。从而可获得试件的应力、应变以及应变率的表达式:
˙ε=v1−v2ls,ε=∫˙εdt,σ=F(t)As (11) 2. 数值模拟
采用Abaqus/Explicit有限元软件建立DDHB以及超长SHPB模型。DDHB系统中透射杆长度为1 500 mm,质量块为
∅ 186 mm×500 mm的圆柱钢块;超长SHPB系统中撞击杆长度为3028 mm,入射杆长度为7000 mm,透射杆长度为4 000 mm。杆件均采用∅ 16 mm的高强钢,杨氏模量E=206 GPa,泊松比μ=0.295,密度ρ=7 800 kg/m3。试件选用无氧铜材料,尺寸为∅ 6 mm×8 mm的圆柱体,采用Johnson-Cook本构模型描述其弹塑性力学行为,材料参数取自文献[20]。杆和质量块的网格尺寸为1 mm,单元总量分别为12 000和46 500,试件的网格尺寸为0.5 mm,单元总量为96,所有的网格类型均为CAX4R。当试件为弹塑性材料时,第二次加载起始处应变率有小幅度下降,因此需要适当延长撞击杆来使应力波同时达到试件两端。固定DDHB透射杆长度为1 500 mm,通过改变撞击杆长度,分析二次加载中的应变率突降,如图6所示。当撞击杆与透射杆等长时,应变率在第二次加载起始阶段具有明显的下降,而当撞击杆过长(如1 530 mm)时,又有明显的上升。而当撞击杆为1 514~1 520 mm时,二次加载的连贯性较好,下降或者上升均不明显,如图6(a)所示。撞击杆和透射杆长度的精准匹配依赖于被测材料的长度和波速,基本原则是撞击杆略长于透射杆即可,但即使撞击杆和透射杆的长度略微有些不匹配,对于应变率不是特别敏感的材料,对材料力学性能的测试结果也几乎没有影响,如图6(b)所示。
对比分析DDHB系统与超长SHPB系统加载性能。当撞击杆长为1 514 mm、初始速度为6 m/s时,DDHB系统与超长SHPB系统的加载应变率历史基本一致,试件中的平均应力-应变曲线也完全相同,如图7所示。计算结果表明,DDHB系统完全可以实现与超长SHPB系统相同的加载时长,只是在二次加载时,由于大质量块尚不能完全等效为固壁端,加载应变率略低于超长SHPB系统,如图7(a)所示;但其应力-应变曲线的差异完全可以忽略,如图7(b)所示。
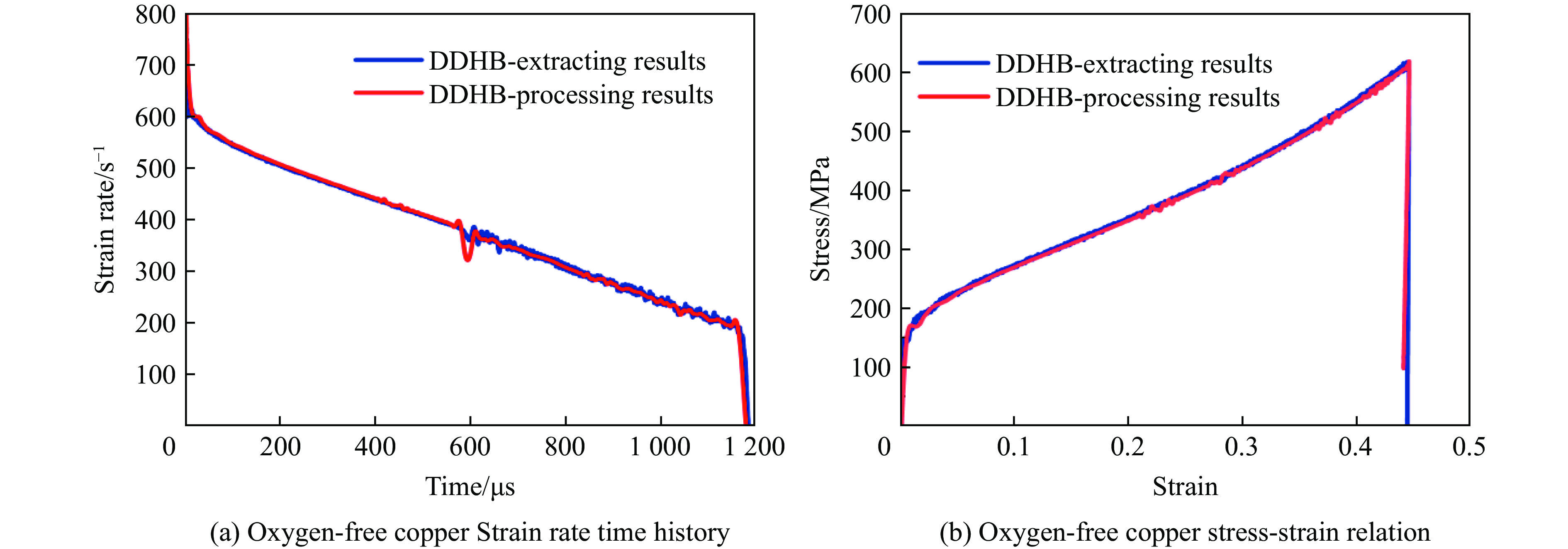
提取无氧铜模型DDHB系统中透射杆上距离撞击端50、1 150 mm位置处的轴向应变信号,等效实验中应变片的采集情况,通过波反演技术和数据处理可到试件的加载应变率时程曲线和应力-应变关系,如图8所示。通过杆上两点应变信号获得的结果与试件直接提取的结果基本一致,但二次加载初期时,通过应变数据处理获得的应变率出现小幅回落现象,如图8(a)所示,该现象主要与波形几何弥散有关。
3. 实验验证
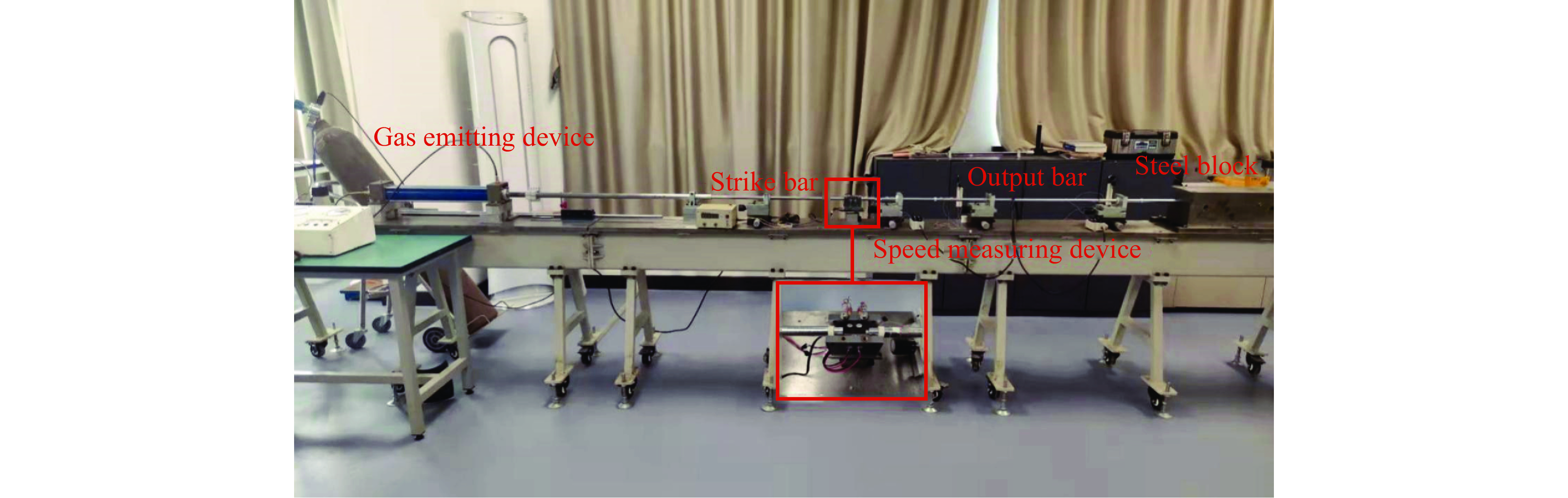
如图9所示,实验采用
∅ 16 mm的DDHB系统,撞击杆长度为1 500 mm,透射杆长度略小于撞击杆,等效固定端的质量块为165 mm×200 mm×425 mm的方形钢块。杆件和质量块的材料均为高强度钢,杨氏模量E=206 GPa、泊松比μ=0.295、密度ρ=7 800 kg/m3,试件为∅ 6.0 mm×8.2 mm的铝合金。测速装置紧靠试件,以获得尽可能准确的撞击速度。图10(a)为撞击杆速度为5.81 m/s时透射杆的两个(位置A、B)应变片上测得的波形;利用式(5)~(6)可分离得到位置A上的右行波和位置B上的左行波(位置A更靠近撞击端),如图10(b)所示;结合式(8)~(10)可获得试件两端面的速度以及试件受到的载荷,如图10(c)~(d)所示。
利用式(11)即可得到相应的应力-应变曲线和应变率曲线,实验结果采取了100 kHz的低通滤波进行光滑。如图11所示,当撞击杆速度为5.81 m/s时,铝合金试件的平均应变率约为500 s−1,试件的最大压缩应变约为60%;当撞击杆速度为1.62 m/s时,铝合金的平均应变率约为80 s−1,试件的最大压缩应变将近10%。诚然,在二次加载初期,应变率的连贯性尚有不足,但是对于材料在一定应变率加载范围内的应力-应变关系的获得没有显著影响。
4. 结 论
提出了一种直撞式二次加载霍普金森压杆实验技术,采用略长于透射杆的撞击杆,通过准刚性壁应力波反射实现二次加载,从而在一个整体长度仅4 m的压杆系统中产生了1.2 ms的加载时长,并且DDHB系统对测试材料的强度没有限制。
数值模拟结果表明,DDHB系统能有效地实现试件的二次加载,与超长SHPB压杆系统获得的数值结果一致;通过波分离技术计算得到的试件应力-应变关系与试件内部直接提取结果也相同;DDHB系统中撞击杆略长于透射杆,能有效降低二次加载初期的应变率不连贯性。利用DDHB实验技术对铝合金进行动态加载,在应变率约80 s−1加载下最大压缩应变达到了10%,而在应变率约500 s−1加载下最大压缩应变达到了60 %。
-
表 1 由实验数据拟合确定的模型参量
Table 1. Parameters in proposed stress-strain equations
C10/MPa C01/MPa C11/MPa A1/μs A2 A3/MPa A4/MPa A5/MPa -1.182 -0.069 -0.173 7 0.4 600 -185 45 -
[1] Roland C M, Twigg J N, Vu Y, et al. High strain rate mechanical behavior of polyurea[J]. Polymer, 2007, 48(2): 574-578. [2] Yi J, Boyce M C, Lee G F, et al. Large deformation rate-dependent stress-strain behavior of polyurea and polyurethanes[J]. Polymer, 2006, 47(1): 319-329. https://www.sciencedirect.com/science/article/pii/S0032386105015740 [3] Sarva S S, Deschanel S, Boyce M C, et al. Stress-strain behavior of a polyurea and a polyurethane from low to high strain rates[J]. Polymer, 2007, 48(8): 2208-2213. [4] Shim J, Mohr D. Using split Hopkinson pressure bars to perform large strain compression tests on polyurea at low, intermediate and high strain rates[J]. International Journal of Impact Engineering, 2009, 36(9): 1116-1127. [5] Amirkhizi A V, Isaacs J, Mcgee J, et al. An experimentally-based viscoelastic constitutive model for polyurea, including pressure and temperature effects[J]. Philosophical Magazine, 2006, 86(36): 5847-5866. [6] Li C, Lua J. A hyper-viscoelastic constitutive model for polyurea[J]. Materials Letters, 2009, 63(11): 877-880. https://www.sciencedirect.com/science/article/pii/S0167577X09000482 [7] Yang L M, Shim V, Lim C T. A visco-hyperelastic approach to modelling the constitutive behaviour of rubber[J]. International Journal of Impact Engineering, 2000, 24(6): 545-560. [8] Pouriayevali H, Guo Y B, Shim V. A constitutive description of Elastomer behaviour at high strain rates-A strain-dependent relaxation time approach[J]. International Journal of Impact Engineering, 2012, 47: 71-78. https://www.sciencedirect.com/science/article/pii/S0734743X12000760 [9] Chen W, Zhang B, Forrestal M J. A split Hopkinson bar technique for low-impedance materials[J]. Experimental Mechanics, 1999, 39(2): 81-85. [10] 林玉亮, 卢芳云, 卢力.高应变率下硅橡胶的本构行为研究[J].高压物理学报, 2007, 21(3): 289-294.Lin Yu-liang, Lu Fang-yun, Lu Li. Constitutive behaviors of a silicone rubber at high strain rates[J]. Chinese Journal of High Pressure Physics, 2007, 21(3): 289-294. [11] 王礼立.冲击动力学进展[M].合肥: 中国科学技术大学出版社, 1992. [12] Rivlin R S. Collected papers of R. S. Rivlin[M]. Berlin: Springer, 1997. [13] Hoo Fatt M S, Xin O. Integral-based constitutive equation for rubber at high strain rates[J]. International Journal of Solids and Structures, 2007, 44(20): 6491-6506. 期刊类型引用(1)
1. 徐沛栋,倪萍,杨宝,蒋震宇,刘逸平,刘泽佳,周立成,汤立群. 用于软材料的中应变率LSHPB系统及应用. 爆炸与冲击. 2025(03): 3-12 .  本站查看
本站查看其他类型引用(1)
-








 下载:
下载:

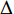






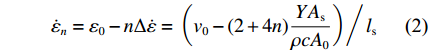






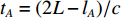



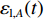










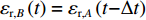
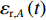
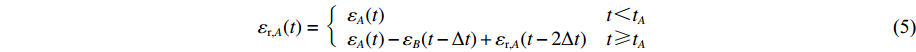











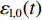




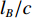




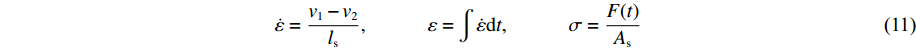












 下载:
下载: