Reinforcing design of openings of a spherical explosive containment vessel
-
摘要: 参考压力容器设计标准,针对某实验用球形爆炸容器进行了开孔补强设计,对容器开孔处等效应变随接管壁厚、补强圈尺寸的变化规律进行了数值计算,确定了“5/3倍球壳厚度的接管”配合“与球壳等厚度、张角10°的补强圈”的开孔补强设计方案。并对数值计算结果和补强设计进行了实验验证。Abstract: Reinforcing design for the opening on a certain experiment-used spherical explosive containment vessel was carried out referring to the pressure vessel code GB150-2011. The relationships between the effective plastic strain around the opening and the geometrical parameters of the nozzle and the reinforcing ring were investigated by numerical simulation. And a reinforcing scheme was proposed which included a nozzle with the wall thickness equal to 5/3 times of the wall thickness of the main spherical shell and a 10°-reinforcing ring with the same thickness to the main spherical shell wall. Experiments were performed to validate the reliability of the simulation results and the rationality of the reinforcing scheme.
-
生物体经过亿万年的进化,经历了自然选择和优胜劣汰,进化出独特的多尺度和多层级微观结构,并且具有优异的综合性能,如骨骼、贝壳、竹子、树干等[1-3]。从天然生物材料独特的宏微观结构获得灵感,对人工合成材料的设计有重要的指导和启示意义。
贝壳珍珠层是一种具有高强度和高韧性的天然材料,这种优异的性能主要来源于文石片与有机基质层自下而上堆砌而形成的多尺度、多层级的“砖泥”结构[4-5]。珍珠层文石片与有机基质的交替排列是其高强度、高韧性的关键,其强韧化机制主要包括裂纹偏转[6]、文石片拔出[7]和有机质桥接[8],许多学者开展了对其“砖泥”结构的研究。Yin等[9]制备出模仿珍珠质“砖和砂浆”排列的夹层玻璃,在保持透光率和高强度基本不变的同时,其抗冲击能力比夹胶玻璃和钢化玻璃提高了2~3倍。Nguyen-Van等[10]设计了不同类型的仿贝壳珍珠联锁波状层合板,并通过数值模拟研究其在爆炸载荷下的破坏模式,发现纤维/乙烯酯的波状联锁层合板的抗爆性能要远优于平面复合材料板。Jia等[11]通过层压的方法生产出类贝壳珍珠层的氧化石墨烯基块体,研究结果表明,不同载荷下结构的界面载荷传递和失效模式将发生改变,随着压力的增加,复合材料由渐进破坏转变为灾难性破坏。Tan等[12]研究了基于生物灵感的类珍珠层陶瓷聚合物复合材料在循环压缩下的疲劳行为和损伤机制,研究发现仿生结构的破坏体现出阶梯状断裂行为,能够有效的提高其断裂韧性。武晓东等[13]建立了一种仿贝壳珍珠层的铝/乙烯基复合材料的三维Voronoi有限元模型,并研究其在冲击载荷下的动态响应。结果表明,与规则图形相比,Voronoi模型有着更好的力学性能和能量吸收。
近20年来,增材制造技术取得了快速的发展,这种技术可以快速而精密地制造出任意形状的零件,解决许多过去难以制造的复杂结构零件的成型,同时能更好的契合多材料结构制备需求。Liu等[14]受红鲍鱼联锁片剂的启发,利用多材料3D打印构建了联锁和非联锁类珠晶复合材料试样,并进行拉伸和三点弯断裂试验,发现联锁比非联锁珍珠状复合材料的刚度、强度和韧性分别提高了55%、143%和176%。马骁勇等[15]利用光固化3D打印技术制备了仿贝壳复合材料,采用准静态拉伸试验和有限元模拟相结合的方法研究了文石片长宽比与材料弹性模量间的关系,研究发现材料微观排布对宏观力学性能及破坏模式有很大的影响。刘英志等[16]构建了仿贝壳砖泥结构有限元模型,研究了其在落锤冲击载荷下的动态响应,研究结果表明三层仿贝壳砖泥结构具有最大比吸能,且冲击速度、锤头类型均对其力学性能有很大的影响。Gu等[17]通过增材制造模仿了三维海螺壳结构,并通过落锤实验和有限元方法研究其抗冲击性能,结果表明,与单层结构和单一刚性结构相比,添加第二层交叉层状结构可以分别提高70%和85%的冲击性能。Jia等[18]利用3D打印制造了仿生架构梁,通过动态三点弯曲试验发现其动态性能远远超过其组成成分,同时依据实验结果设计了多种仿生混合梁,进一步提高了柔性、强度和韧性等。Ko等[19]通过3D打印设计并制造了Voronoi图类似珍珠的层状复合材料,并进行了三点弯曲试验,结果表明结构的吸能比单一材料结构大11%。对于仿贝壳砖泥结构,多数研究集中在静态拉压和动态三点弯,而对于其在高应变率和爆炸载荷下的动态响应研究较少。
本文基于天然贝壳珍珠层砖泥排列的结构特点,构建仿贝壳砖泥结构的有限元模型,利用LS-DYNA有限元软件模拟其在爆炸载荷下的动态力学响应,通过爆炸实验验证有限元模型的有效性,重点研究堆叠层数、爆炸冲量对仿贝壳砖泥结构的影响,并且探讨其增韧机理。
1. 几何模型和材料参数
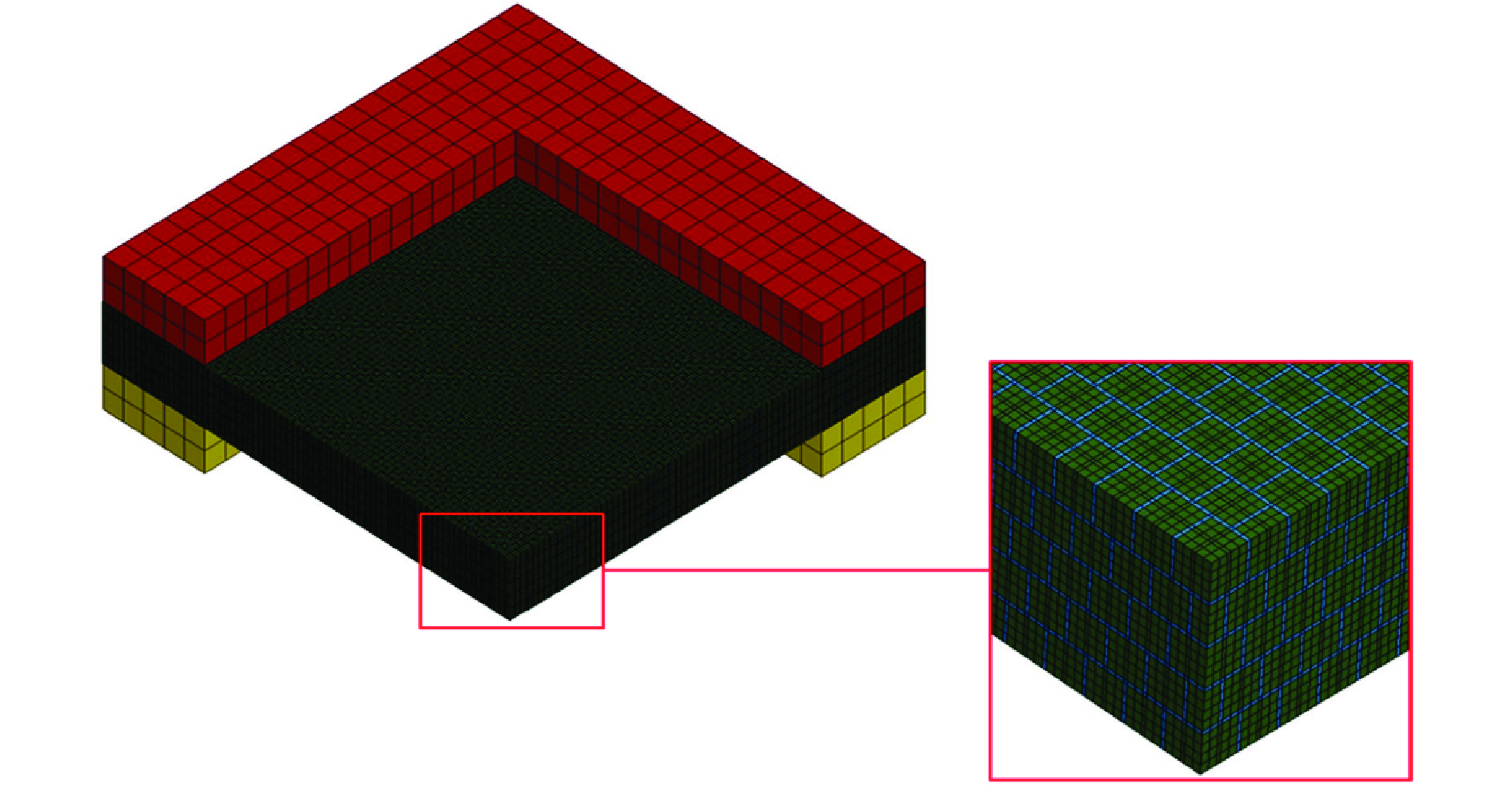
本文所构建的仿贝壳砖泥结构,与刘英志等[16]所构建的结构类似:首先,建立单层砖、泥的单胞模型,如图1所示,其中绿色为仿贝壳砖泥结构对应的“砖”结构,其大小为1 mm正方体,紫色为仿贝壳砖泥结构对应的“泥”结构,其厚度为0.1 mm;随后将其组装在一起得到单层仿贝壳砖泥结构的胞元尺寸为2.2 mm×2.2 mm×1.0 mm。对于多层仿贝壳砖泥结构来说,相邻每层之间均为对中排布,相隔两层结构相同,并且层与层间均排布0.1 mm的泥材料。以三层单胞模型为例,其胞元尺寸为2.2 mm×2.2 mm×3.2 mm。将单胞模型沿面内两方向阵列得到仿贝壳砖泥结构的整体模型,其平面尺寸为100 mm×100 mm,如图2所示。本文研究1~5层的仿贝壳砖泥结构在动态载荷下的力学响应,探索层数对仿贝壳砖泥结构的影响。
天然贝壳珍珠层是一种多材料结构,其结构是由体积分数较大的无机相碳酸钙(砖材料)及体积分数较小的有机基质(泥材料)组成,其中无机相碳酸钙是一种强度高但非常脆的材料,而有机质是一种可塑性好的材料。VeroWhite Plus弹性模量较大,而且具有较大的承载能力用以替代生物材料的无机相,以弹性模量较小且可变形性能力好的Tango Plus材料替代有机基质[16-17]。
2. 实验及有限元验证
2.1 实验结构及装置
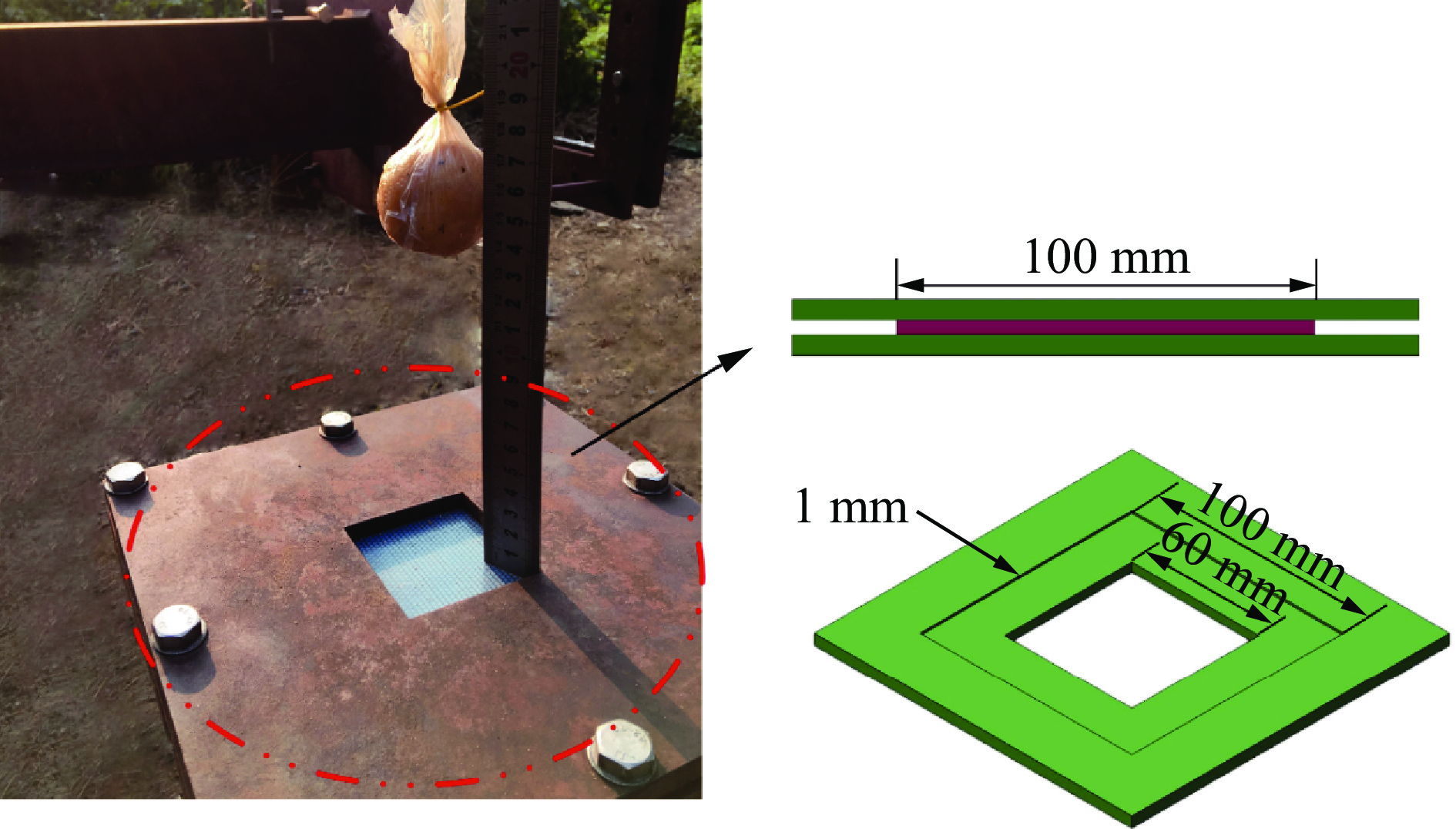
使用3D打印机打印了堆叠层数为5的仿贝壳砖泥结构(如图3和图4所示),尺寸为100 mm×100 mm×5.4 mm。本文研究了其在爆炸载荷下的力学响应,爆炸实验装置如图4所示,炸药为球形硝酸铵炸药,炸药中心距离试件中心150 mm,试样被两块盖板夹持在中间,夹持的宽度为20 mm,上下盖板内测均有1 mm的凹槽,这使得试件可以更好地被固定,而后通过8个螺栓将上下盖板及试件紧固在固定支架上,实验过程中试件的受载面积为60 mm×60 mm。
2.2 有限元模型
采用有限元软件LS-DYNA建立仿贝壳砖泥结构进行有限元分析,为了节约模拟计算时间,采用1/4模型进行计算。仿贝壳结构有限元模型尺寸为40 mm×40 mm×5.4 mm,夹持板的宽度为10 mm,厚度为4 mm,模型的有效作用载荷尺寸与实验中一致,为30 mm×30 mm。图5为有限元模型,其中硬质和软质材料采用C3D8实体单元,网格大小为0.25 mm,值得注意的是,层间软材料厚度为0.1 mm,在保持其面外厚度为0.1 mm的同时,将其面内尺寸设置为0.25 mm;对于上下夹持板也采用C3D8实体单元,网格大小为2 mm。分别采用MAT 89 (PLASTICITY-POLYMER)和MAT 269 (BERGSTROM-BOYCE-RUBBER)来模拟生物材料中硬、软质材料,并且分别采用失效应力、失效应变来控制其失效;选用MAT 20 (RIGID)来模拟夹持夹板[16]。有限元模拟中,采用LOAD-BLAST-ENHANCED施加爆炸载荷,有限元中TNT的药量为实验中硝酸铵乳化炸药药量乘以0.693[20]。对上下夹持板与软硬材料整体间采用AUTOMATIC-SURFACE-TO-SURFACE接触,其中静摩擦因数为0.2,动摩擦因数为0.1。软硬材料之间采用TIEBREAK-SURFACE-TO-SURFACE接触,其失效参数为45 MPa。软硬材料整体设置了AUTOMATIC-SINGLE-SURFACE和ERODING-SINGLE-SURFACE接触来保证计算的收敛性。
2.3 模型验证
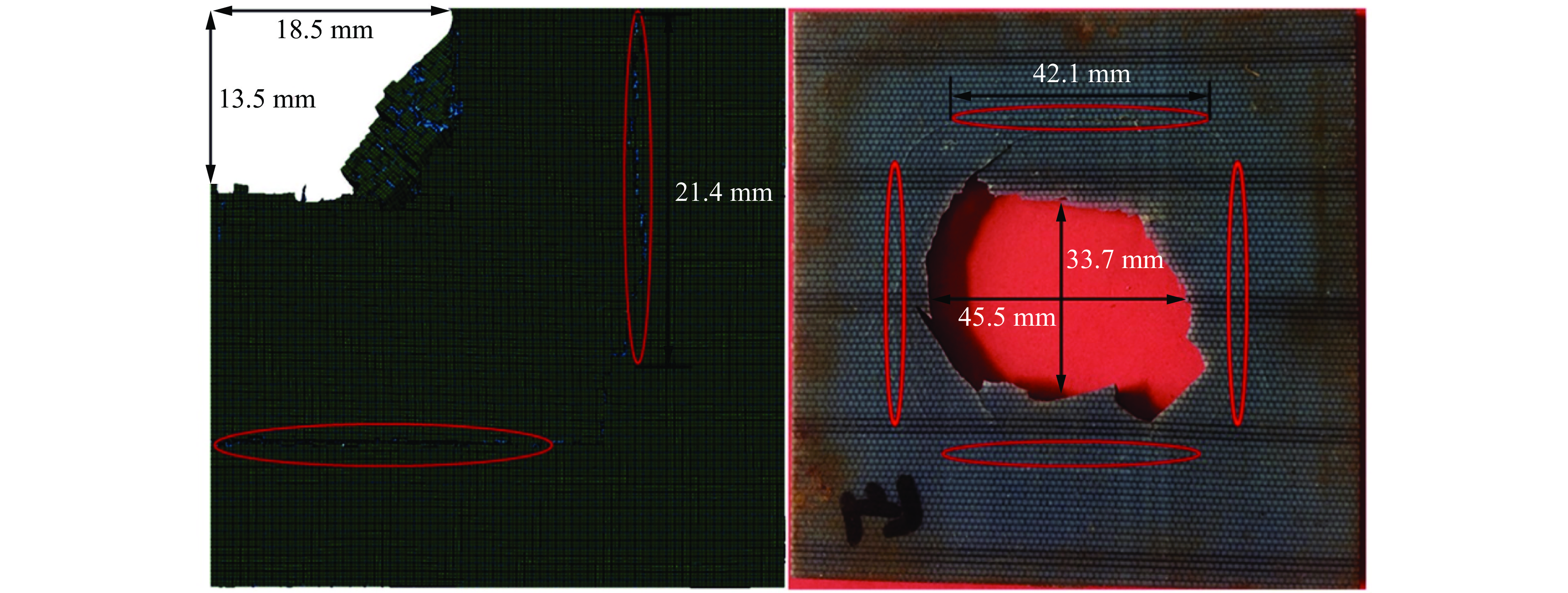
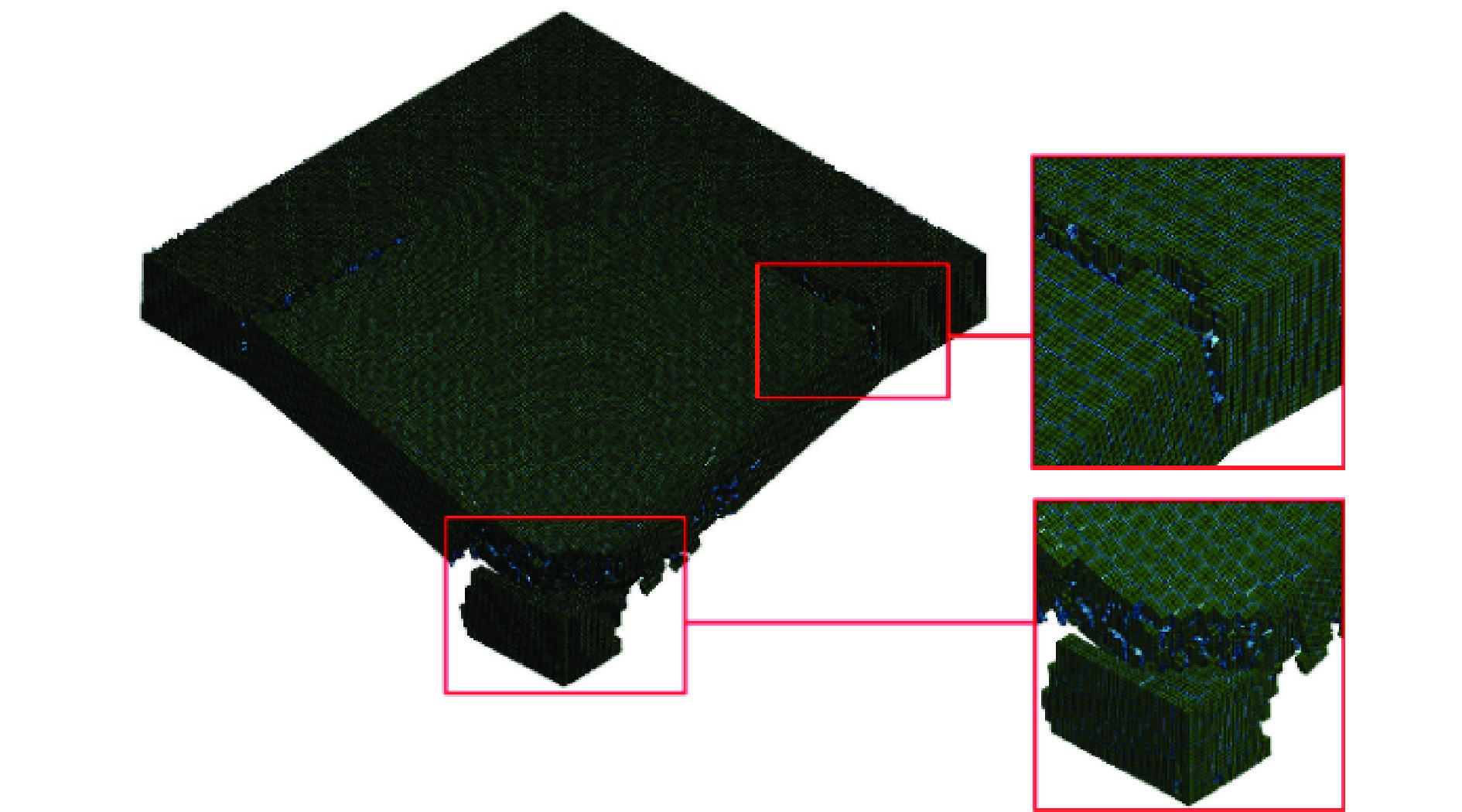
图6为TNT炸药药量为14 g下(实验中硝酸铵乳化炸药药量为20 g)实验结果与有限元模拟结果图。从实验结果来看,仿贝壳砖泥结构在中心处断裂,发生了灾难性破坏,使得中心处发生掉落;而且试件夹持端四周均存在剪切破坏。在有限元数值模拟中,在试件中心处发生灾难性的掉落破坏,在夹持端发生明显的剪切破坏。在实验中,结构中心处掉落尺寸约为45.5 mm×33.7 mm,剪切端破坏长度为42.1 mm;在1/4有限元模拟中,结构中心处掉落尺寸为18.5 mm×13.5 mm,且夹持板夹持处存在剪切裂纹,长度为21.4 mm,从而说明有限元结果的有效性。
图7给出了五层仿贝壳砖泥结构模型在TNT炸药药量为14 g情况下的能量曲线,伪应变能(Hourglass Energy)只占总能量的3.6%,而外力做功大于系统的内能与系统动能之和,这是由于在有限元模拟过程中材料加入破坏准则使得网格删除而导致能量的损耗。
3. 结果与讨论
3.1 破坏模式
冲量作为冲击波的基本参数,超压(Δp)-时间(t)关系曲线所包含的面积称为冲击波比冲量
im ,根据萨多夫斯基[21]理论:im={∫τ0Δp(t)dt=A∗∗3√W2/R¯R>0.515W/R2¯R<0.25 (1) 式中:A**为常数,34≤A**≤36;W为炸药质量,kg;R为炸药与试件的距离,m;
¯R=R/3√W 。为了研究仿贝壳砖泥结构在不同药量下破坏模式,分别对五层仿贝壳砖泥结构在TNT炸药药量为3.5、7、8.75、10.5、14 g,冲量分别为0.019、0.030、0.035、0.039、0.047 N·s时进行数值模拟,通过数值模拟结果发现,不同药量下仿贝壳砖泥结构的破坏模式可以分为如下5种,分别为:
Ⅰ,结构整体无损伤,仅发生塑性形变,如图8所示;
Ⅱ,结构前表面无明显破坏,后表面发生破坏,如图9所示;
Ⅲ,结构发生掉落型贯穿破坏,夹持端无剪切破坏,如图10所示;
Ⅳ,结构发生小块掉落型贯穿破坏,夹持端发生剪切破坏,如图11所示;
Ⅴ,结构发生大块掉落型贯穿破坏,夹持端发生剪切破坏,如图12所示。
研究发现,随着爆炸冲量的增加,结构破坏模式也随之改变。当冲量小于0.019 N·s,结构无破坏,仅发生一定的形变,随着炸药药量不断地增加,结构开始发生破坏,当冲量大于0.030 N·s,结构会发生一定的损伤,其承载能力会降低。当冲量大于0.047 N·s,结构发生毁坏性损伤,造成其承载力的消失。
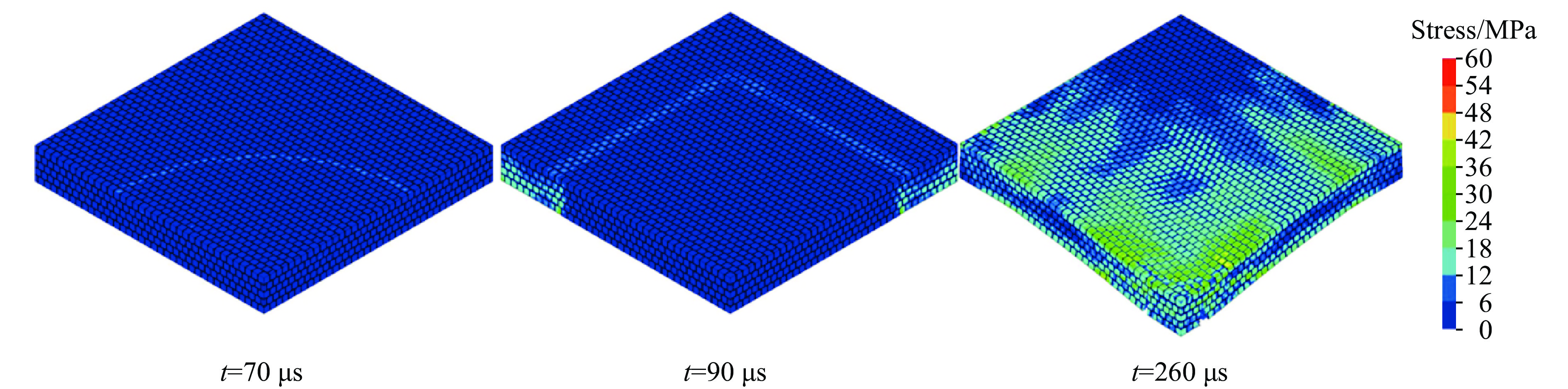
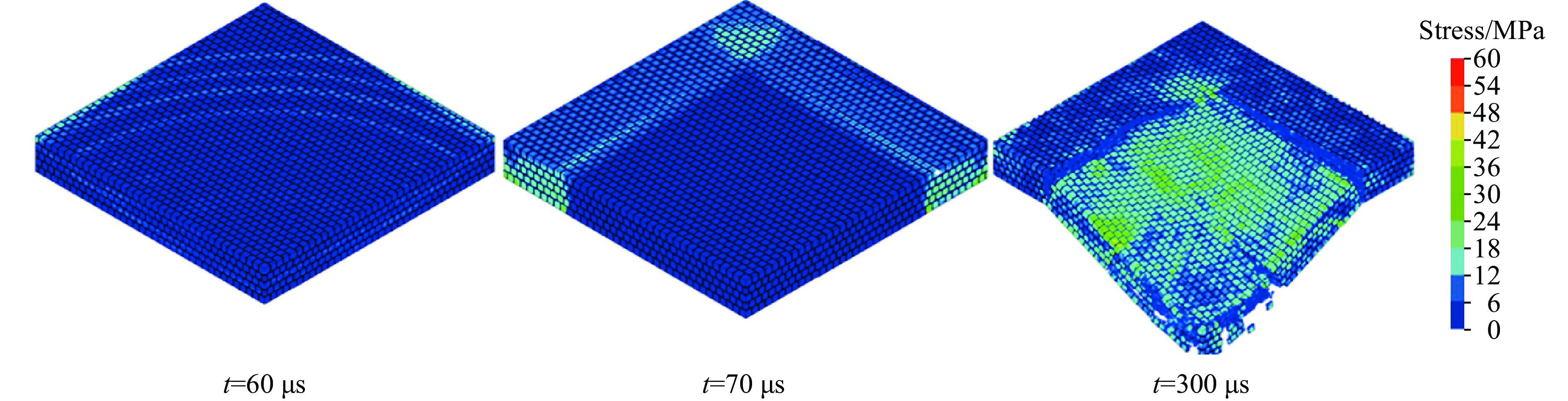
图13和图14分别显示了冲量为0.030 N·s以及0.047 N·s时五层砖混结构的应力云图。如图13所示,在t=70 μs时,爆炸冲击波传递至面板中心,为典型的球形波。t=90 μs时,应力波传递至夹持板固定处,由于试件的厚度相比于试件的长度不能忽略,因此试件后面板应力波到达夹持处时间要迟于前面板。当t=260 μs时,后面板中心处硬材料应力值达到破坏阈值,结构在后面板中心处发生拉伸破坏,而结构在夹持端并未达到破坏极限。随着冲量继续增加,当冲量达到0.047 N·s时,如图14所示,t=60 μs时,爆炸冲击波传递至面板中心,t=70 μs时,应力波传递至夹持板固定处,t=300 μs时,试件中心以及夹持端处硬材料应力值达到破坏阈值,因此在试件的中心处发生破坏,夹持端发生剪切破坏。
3.2 层数影响
为研究层数对仿贝壳砖泥结构的影响,分别对1~5层的仿贝壳砖泥结构在冲量0.019 N·s下进行了有限元模拟。从图15中可以发现,层数对破坏模式的影响很大,单层试件破坏模式为Ⅴ型:结构发生大块掉落型贯穿破坏,夹持端发生剪切破坏;双层试件破坏模式为Ⅳ型:结构发生小块掉落型贯穿破坏,夹持端发生剪切破坏;三层试件的破坏模式为Ⅲ型:结构发生掉落型贯穿破坏,夹持端无剪切破坏,四层试件的破坏模式为Ⅱ型:结构前表面无明显破坏,后表面发生破坏;五层试件的破坏模式为Ⅰ型:结构整体无损伤,仅发生塑性形变。可以发现在相同的冲量下,随着堆叠层数的增加,结构的破坏模式由开始的灾难性破坏到只发生一定变形,结构的抗爆性能随着层数的增加有显著的提升;同时发现,在一定炸药药量下,随着堆叠层数的增加,结构的后面板挠度逐渐减小。
为了比较不同层数的仿贝壳砖泥结构在不同药量下的动态响应结果,分别对不同层数的仿贝壳砖泥结构在冲量为0.019、0.030、0.035、0.039和0.047 N·s下进行数值模拟,并分析其破坏模式。其结果如表1所示,
表 1 不同层级结构在不同药量下的动态响应Table 1. Dynamic response of structure with different layers to different explosive charges冲量/(N·s) 1 layer 2 layers 3 layers 4 layers 5 layers 0.019 Ⅴ Ⅳ Ⅲ Ⅱ Ⅰ 0.030 Ⅴ Ⅴ Ⅳ Ⅲ Ⅱ 0.035 − Ⅴ Ⅴ Ⅳ Ⅲ 0.039 − − Ⅴ Ⅴ Ⅳ 0.047 − − − Ⅴ Ⅴ 根据不同层数的仿贝壳砖泥结构在不同炸药药量下的动态响应结果来看,当结构的破坏模式到达Ⅴ型时,认为结构不能承载。在同一冲量下,随着层数的增加,结构的破坏模式发生改变, 例如:在冲量为0.019 N·s时,1~5层结构的破坏模式分别呈现Ⅴ型、Ⅳ型、Ⅲ型、Ⅱ型、Ⅰ型。在同一层数下,随着冲量的增加,结构的破坏模式增加,例如:当结构为5层时,随着冲量由0.019 N·s增加到0.047 N·s时,结构的破坏模式从开始的Ⅰ型破坏,逐步演变为Ⅴ型破坏。而且当层数增加时,结构的损伤阈值增加,例如:单层结构在冲量达到0.019 N·s时不能承载,三层结构在冲量达到0.035 N·s时不能承载,五层结构在冲量达到0.047 N·s时不能承载。值得注意的是,随着药量或冲量的增加,其破坏模式均是夹持端发生更严重的剪切破坏,结构中心发生大块掉落型贯穿破坏,不会出现其他的破坏模式。
3.3 增韧机制
贝壳是组织高度分级的复合结构,它独特的结构有利于引导裂纹沿结构单元界面在三维方向上扩展来消散裂纹能量,在保持硬度和强度的同时,具有非常好的韧性。
(1) 裂纹偏转
裂纹偏转是贝壳珍珠层中最常见的一种裂纹扩展现象,裂纹首先沿着无机相组织间的有机层扩展一段距离,然后发生偏转,或者转移到相邻其他有机层中扩展,或者穿过无机相,再转移到另一有机层中扩展。这种裂纹的频繁偏转必然造成扩展途径的延长,从而吸收更多的能量,并且当裂纹从一个利于裂纹发展的方向转向另一个不利于裂纹发展的方向扩展时,将导致扩展阻力的明显增加[22]。
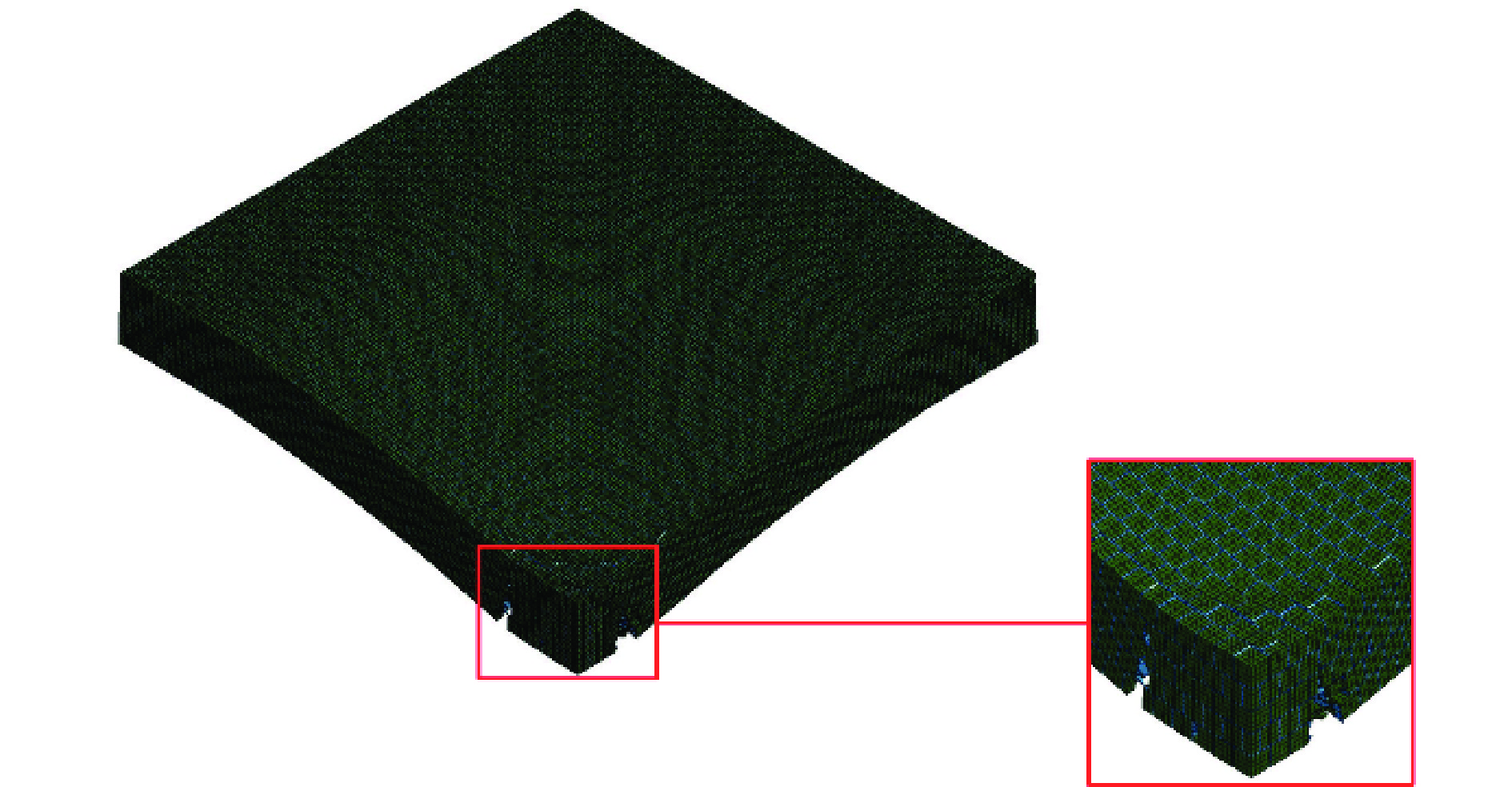
如图16所示,五层结构在冲量0.039 N·s下的破坏模式图,裂纹偏转主要发生在软硬材料的结合面,在面内方向和面外方向,裂纹先是硬材料的界面扩展,在到达硬材料胞元的端面时,裂纹突然发生90°转向,沿着硬材料胞元另一个垂直方向上的界面继续扩展,在遇到邻近的硬材料胞元时,裂纹继续转向,在结构表面形成锯齿形的扩展路径。
(2) 微裂纹
微裂纹的增韧机理为通过微裂纹的形成来分散主裂纹尖端能量从而了提高材料韧性。即微裂纹在扩展和形成新的微裂纹过程中消耗部分能量,而达到宏观增韧效果。对于仿贝壳砖泥结构,可以发现微裂纹主要集中在后面板。如图17所示,五层结构在冲量0.047 N·s下的结构损伤,由于珍珠质独特的“砖和砂浆”结构,软硬材料结合的界面比较薄弱,在外力的作用下,这些界面会开裂,形成主裂纹而产生破坏,而在主裂纹周围萌生了许多微裂纹,这些微裂纹耗散了更多的能量,使得仿贝壳砖泥结构拥有了很好的韧性。
4. 结 论
受贝壳生物材料优异力学性能的启发,构建了仿贝壳砖泥结构的有限元模型,利用LS-DYNA有限元软件模拟了其在爆炸载荷下的动态力学响应,而后将有限元数值模拟结果与爆炸试验结果进行了对比,验证了有限元模型的有效性。在此基础上,对于仿贝壳砖泥结构进行了不同冲量和不同层数下的爆炸数值模拟,主要结论如下。
(1) 对于五层仿贝壳砖泥结构在不同药量下的数值模拟结果可以发现,试件的破坏主要由试件中心掉落和四周夹持端试件剪切破坏组成。结构的破坏模式可以分为5种情况:Ⅰ,结构整体无损伤;Ⅱ,结构前表面无明显破坏,后表面发生破坏;Ⅲ,结构发生掉落型贯穿破坏,夹持端无剪切破坏;Ⅳ,结构发生小块掉落型贯穿破坏,夹持端发生剪切破坏;Ⅴ,结构发生大块掉落型贯穿破坏,夹持端发生剪切破坏。
(2) 详细的分析了层数对的仿贝壳砖泥结构在动态载荷下力学响应的影响。随着层数的增加,在同一冲量为0.019 N·s时,试件的破坏模式由单层的V型大块掉落型贯穿破坏,逐渐降低为五层的Ⅰ型仅发生一定塑性变形。五层砖泥结构的破坏阈值为0.047 N·s, 当冲量超过0.047 N·s时,试件发生灾难性破坏。
(3) 研究发现材料微观结构对仿生材料的宏观力学性能有很大的影响,仿贝壳砖泥结构的增韧机制主要有裂纹偏转和微裂纹。但是由于本文的局限性,缺乏对这两种增韧机理的定量描述,这也是下一步研究的重点。
-
表 1 连接处等效应变随补强圈尺寸变化情况
Table 1. The effective strain of the junction varied with the geometrical size of the reinforcing ring
δH/H εj (εj, s-εj)/εj εj (εj, s-εj)/εj εj (εj, s-εj)/εj φs=5° φs=10° φs=15° 0.5 0.081 -43% 0.067 -53% 0.057 -60% 1.0 0.043 -70% 0.022 -85% 0.020 -86% 1.5 0.020 -86% 0.008 -94% 0.009 -94% 表 2 厚壁接管配合补强圈计算结果(φs=10°)
Table 2. Simulation results of the model for the thick-walled nozzle with a reinforcing ring (φs=10°)
δH εr, max εn, max h/H=1 h/H=5/3 h/H=1 h/H=5/3 1.0H 0.022 0.012 0.057 0.021 1.5H 0.008 0.003 0.011 0.005 -
[1] Weiβ E, Rudolph J. Finite element analyses concerning the fatigue strength of nozzle-to-spherical shell intersections[J]. International Journal of Pressure Vessels and Piping, 1995, 64(2): 101-109. doi: 10.1016/0308-0161(94)00074-S [2] Weiβ E, Rauth M, Rudolph J. Fatigue behavior of oblique nozzles on cylindrical shells submitted to internal pressure and axial forces[J]. International Journal of Pressure Vessels and Piping, 1998, 75(6): 473-481. doi: 10.1016/S0308-0161(98)00050-7 [3] Dekker C J, Stikvoort W J. Pressure stress intensity at nozzles on cylindrical vessels: A comparison of calculation methods[J]. International Journal of Pressure Vessels and Piping, 1997, 74(2): 121-128. doi: 10.1016/S0308-0161(97)00106-3 [4] Dekker C J, Brink H J. Nozzles on spheres with outward weld area under internal pressure analysed by FEM and thin shell theory[J]. International Journal of Pressure Vessels and Piping, 2000, 77(7): 399-415. doi: 10.1016/S0308-0161(00)00043-0 [5] Dekker C J, Bos H J. Nozzles: On external loads and internal pressure[J]. International Journal of Pressure Vessels and Piping, 1997, 72(1): 1-18. doi: 10.1016/S0308-0161(97)00014-8 [6] Liu J S, Parks G T, Clarkson P J. Shape optimisation of axisymmetric cylindrical nozzles in spherical pressure vessels subject to stress constraints[J]. International Journal of Pressure Vessels and Piping, 2001, 78(1): 1-9. doi: 10.1016/S0308-0161(00)00065-X [7] 国家质量监督检验总局.压力容器: GB150.1-150.4-2011[S].北京: 中国标准出版社, 2011. [8] 张德志.柱形爆炸容器载荷与塑性结构响应研究[D].西安: 西北核技术研究所, 2012. 期刊类型引用(3)
1. 张保勇,张义宇,陶金,王亚军,刘传海,韩永辉,孙曼. 三明治结构复合材料对甲烷-空气混合气体爆炸传播规律的影响. 兵工学报. 2025(01): 128-137 .  百度学术
百度学术2. 黄斌,范启东,梁剑烽. 薄壁仿生护具微冲击响应智能预测方法仿真. 计算机仿真. 2024(10): 438-442 .  百度学术
百度学术3. 陈鑫康,李志洋,雷建银,刘志芳. 爆炸载荷下仿贝壳Voronoi砖泥结构的动态响应. 高压物理学报. 2024(06): 102-110 .  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:




















 下载:
下载:








 百度学术
百度学术