Determination of safety coefficient for predicting blasting vibration velocity
-
摘要: 基于爆破振动速度预测公式回归分析的基本原理,提出根据建筑物不同安全等级应选择一定的可靠性指标,并推导了预测爆破振动速度公式中所含安全保证系数的计算过程。为简化计算过程,又进一步提出了基于监测数据量、回归分析相关系数以及可靠性指标的关于安全保证系数计算的经验公式。经过大量监测数据验证了该经验公式的计算精度和可靠性均能满足工程实际需要,从而修正了萨氏公式预测精确度不够的缺陷,可为重要建筑物周边的安全爆破设计提供参考。Abstract: The basic principle of regression analysis was considered for the prediction formula of blasting vibration velocity. On the basis of the considered principle, an opinion was put forward that certain reliability indexes should be chosen according to the safety levels of buildings. And the calculation process was deduced for the safety assurance coefficient included in the prediction formula of blasting vibration velocity. To simplify the calculation process, an empirical calculation formula was proposed for the safety assurance coefficient based on the parameters consisting of amount of monitoring data, correlation coefficient of regression analysis, and reliability index. The accuracy and reliability of the empirical calculation formula was verified by the monitoring data in actual projects. The investigated results show that the empirical calculation formula can meet the practical needs and it can make up the shortcoming that the accuracy of Sadov's formula is not enough. So the empirical calculation formula proposed can provide a reference for the design of safety blasting surrounding important buildings.
-
分离式霍普金森压杆(split Hopkinson pressure bar,SHPB)是最常用的材料动态力学性能测试系统之一,自1914年被Hopkinson提出以来,得到了持续的优化和发展[1]。目前,应用最广泛的是Kolsky等在20世纪40年代改进的分离式霍普金森压杆[1]。此外,基于压杆技术,20世纪70年代前后还发展出了分离式霍普金森拉杆和分离式霍普金森扭杆技术。传统的分离式霍普金森压杆通过撞击杆撞击入射杆产生压缩应力波。撞击杆通常由高压气体或电磁力驱动,并在炮管内完成加速过程[2]。分离式霍普金森扭杆一般通过突然释放杆中储存的扭转变形能来产生动态扭矩[3],释放方式包括小型爆炸[4]、剪断钢丝[5]、释放机械爪[6]等,但这些传统装置通常存在时间控制精度不高的问题。
随着航空航天、生物医学和军事等领域的快速发展,相关材料在动态复杂应力状态下的力学响应逐渐受到关注[7-9]。然而,由于传统的压缩和扭转荷载产生方式在时间上难以精确控制,加上压缩波与扭转波的波速不同,直接结合压缩和扭转的传统加载方式难以使两列应力波同时到达试件[10]。因此,动态复合加载技术仍存在优化空间和诸多挑战。
目前,确保动态复合加载同步性的主要策略有两种:一是调整杆或试件的设计,使压缩应力波在杆和试件的接触端面被分解成两种不同的应力波;二是革新应力波的产生方式。调整试件设计是指将传统的圆柱形试件改变成特殊形状,使试件在单轴荷载作用下局部或全域处于多轴应力状态。例如,帽子型试件在受压缩荷载时,会在变截面处同时出现压缩应力和剪切应力[11],与之类似的还有剪切-压缩试件(shear-compression specimen,SCS)[12]。斜圆柱试件在动态压缩荷载与端面摩擦力的共同作用下,会同时产生压缩变形和剪切变形[13]。然而,由于不规则的形状有可能导致试件出现非均匀变形,实验结果准确性不够理想。再例如,将入射杆和透射杆的端面从平面改为斜面,只要试件与端面间有足够的摩擦,试件将会同时受到压缩和剪切荷载[14-15]。崔云霄等[16]、赵鹏铎等[17]将入射杆的末端改进为与轴向成45°角的楔形面,利用光通量法对试件进行剪切应变测量,所提出的压剪杆系统受到了广泛关注和应用。这类方法通常需要足够的摩擦力,且可能会引入干扰的弯曲波。
对于革新应力波的产生方式,Nie等[18]、Liu等[19]提出了一种创新的方法,即利用电磁场直接产生压缩应力波,而扭转应变能则通过电磁场控制夹具释放而获得。由于电磁控制精度能达到微秒量级,远高于动态加载持续时间的毫秒量级,因此该方法能够保证压缩与扭转荷载的同步性,可满足动态复合加载实验的要求,且具有较高的控制精度,可以适用于多种动态复合加载[20],但电磁SHPB的设备相对复杂,成本也较高。Johnson[21]提出借助各向异性晶体,使压缩波分解成准纵波和准横波再传播到试件上,但这种方法受到晶体性质的限制,试件受到的准纵波与准横波的比值不易控制,且不易根据被测试件调整晶体的刚度以达到最好的匹配效果。因此,即使解决了复合加载同步性难的问题,现有的动态复合加载实验技术可能还存在弯曲波干扰、设备复杂、实验成本较高,以及纵波和横波比值不易控制等局限。
自压扭耦合超材料首次被提出以来[22],众多学者致力于其结构设计[23-24]和力学性能的研究[25],并指出该超材料在能量吸收[26-27]、能量收集[28]、减振隔振[29-30]、单向传动[31]等方面具有广阔的应用前景。Ren等[32]提出利用压扭超材料将压缩应力波转换成压缩-剪切复合应力波,实现对试件的同步压缩和扭转加载。相较于各向异性晶体,这种超材料的应力波转换效果可通过简单地改变几何参数来调节,并可根据被测材料的特性灵活选择基体材料,实现刚度匹配。不仅如此,在实际实施中,此方案仅需在入射杆端面粘接一个压扭超材料即可,无需其他复杂改造,操作简单,从而易于推广。
本文在课题组前期工作[32]的基础上,利用有限元模型深入探讨了压扭超材料的几何参数对其承载能力和压扭效应的影响,进而估算在应用此实验技术时,被测试件可承受的压缩荷载、扭转荷载以及压扭系数的范围,旨在为该实验技术的推广应用提供必要的参数参考。此外,运用该实验技术对金属钛、304不锈钢和316L不锈钢等3种材料进行动态压扭实验,验证实验技术的可靠性。这一结合超材料和传统实验设备的新思路,以期为实现更多复杂的动态加载实验开辟新的方向。
1. 基于压扭超材料的动态压缩-扭转加载实验技术
压扭超材料由上、下两圆板和中间数根以手性对称方式排布的斜圆柱杆构成。因其独特构型,在受到压缩荷载时会自发产生扭转变形,从而能将输入的压缩应力转换为同步的压缩应力和剪切应力输出。基于此特性,将其与一维SHPB结合,实现材料的动态压缩-扭转复合加载。此动态复合加载实验技术操作简单,将超材料和被测试件依次粘接于入射杆与透射杆之间即可。当子弹撞击入射杆产生的压缩应力波传播到压扭超材料后,便被转化为压缩与剪切复合应力波,并作用于被测试件,使试件同时受到压缩和扭转荷载,如图1所示。透射杆表面的正应力和切应力可通过应变花获得。透射杆横截面内正应力均匀分布,而任意点的切应力与该点到圆心的距离成正比,通过透射杆表面的正应力与切应力可计算得到加载的轴力与扭矩。由于试件与透射杆牢固粘贴,因此可认为透射杆受到的压缩和扭转荷载与试件上的相同。以薄壁圆柱试件为例,其轴力
F 与扭矩T 可分别表示为:F=πR2tσt=π(R2o−r2o)σo (1) T=πR3t2τt=∫Arτ(r)dA (2) 式中:
σt 和τt 分别为透射杆表面测得的正应力和切应力,Rt 为透射杆半径,A 和τ 分别为试件的截面积和切应力。原本τ 应是半径r 的函数,但由于薄壁圆柱的外半径Ro 与内半径ro 非常接近,因此可认为τ 在整个试件中均匀分布,将其值记为τo ,则式(2)变为:T=πR3t2τt=2π(R3o−r3o)3τo (3) 由式(1)和(3)可知,对于薄壁圆柱试件,其正应力
σo 和切应力τo 可分别表示为:σo=σtR2tR2o−r2o (4) τo=3R3tτt4(R3o−r3o) (5) 试件的应变可通过高速摄影机和三维数字图像相关性技术(3D digital image correlation, 3D DIC)获得。此外,通过调整超材料的几何参数,可改变应力波的转换效果,因此,只需更换超材料即可简单实现对试件所受压缩荷载和扭转荷载比值的控制。
2. 压扭超材料的设计及其影响
压扭超材料的压扭效应和承载能力是实验方案中的两大关键要素。压扭效应影响被测试件所受剪切应力与压缩应力的比值,而承载能力则决定超材料能施加给试件的最大荷载。此两要素均可通过调整超材料的几何参数进行调控,但调控范围受到几何协调性和基体材料力学性能等因素的制约。设定基体材料为Ti6Al4V钛合金,探讨超材料的压扭系数及承载能力范围。
2.1 压扭超材料的几何限制
超材料由上、下两圆板和中间的斜圆柱杆构成,圆板不影响结构的压扭耦合效应,为实验方便,通常使圆盘直径与杆直径相同;与耦合机制相关的主要几何参量包括斜杆圆心连成的圆的直径
D∗ (以下简称有效直径)、斜杆数量n 、斜杆长度l 、斜杆直径d ,以及斜杆轴线与下底板形成的夹角θ (斜杆倾斜角度),其构型如图2(a)所示。为确保斜杆间不相互碰触,这些几何参量间必须满足一定关系。任取一根斜杆作为研究对象,标记其轴线与上、下薄板的交点分别为
A1 和A2 ,与之相邻的另一斜杆与上、下薄板的交点分别为B1 和B2 。将A2 和B2 两点投影至上薄板,得到点A′2 和B′2 。以上薄板的圆心O 为坐标原点建立直角坐标系,以→OA1 为x 轴正方向,以竖直向上为z轴正方向。标记→OA1 与→OA′2 的夹角为α ,→OA1 与→OB1 的夹角为β ,有效直径为D∗ ,如图2(a)所示,则有:α=2arcsin(lcosθD∗),β=360∘n (6) 令:
→A1A2=(D∗2(cosα−cos0),D∗2(sinα−sin0),lsinθ) (7) →B1B2=(D∗2(cos(α+β)−cosβ),D∗2(sin(α+β)−sinβ),lsinθ) (8) →A1B1=(D∗2(cosβ−cos0),D∗2(sinβ−sin0),0) (9) 则,两根斜杆间的距离
s 表达式为:s=|→A1B1,→A1A2,→B1B2|‖→A1A2×→B1B2‖ (10) 因为斜杆不能相互接触,则必须有:
s>d (11) 联合式(6)~(11),可得各几何参量间的相互约束关系。图2(b)展示了
D∗ =16 mm,n =8时符合要求的几何参量分布。2.2 压扭超材料几何参数对压扭效应和承载能力的影响
2.2.1 压扭超材料准静态压缩有限元模型
为探究压扭超材料性能与其几何参数之间的变化规律,通过Solidworks构建了超材料的几何模型,导入Abaqus进行力学性能的计算。如图3所示,超材料的上、下圆盘通过“Tie”方式分别连接于两块刚体板,下刚体板完全固定,上刚体板除轴向位移外其余自由度完全固定,由此通过上刚体板对超材料施加准静态轴向压缩位移。超材料采用C3D8R六面体单元。基材参数设置为:密度4.4 g/cm3,弹性模量120 GPa,屈服强度970 MPa,泊松比0.33。需要注意的是,本节仅关注超材料性能随几何参数变化的规律,基材的材料参数对整体规律无影响。
图4(a)展示了一组准静态压缩加载条件下的有限元模型计算结果。图中横坐标表示超材料的压缩位移,即超材料上、下两块刚性板之间的相对轴向位移。红线描述了超材料受到的扭转荷载与压缩位移的关系,而黑线是轴向荷载-压缩位移曲线。在到达屈服点前,扭转荷载和轴向荷载均呈线性上升趋势;一旦超过屈服点,则出现下降趋势。屈服点对应的荷载即为超材料的承载极限。为了更精准地识别屈服点,计算了荷载-位移曲线的二次导数,并认为屈服发生在该曲线的第1个极值点处(图4(b))。
超材料的压扭效应可通过无量纲的压扭系数
λ 来衡量,其定义如下:λ=2TFD∗ (12) 在线弹性阶段,扭矩
T 与轴向荷载F 的比值保持恒定,且有效直径D∗ 也是常数,所以λ 是一个固定值。2.2.2 压扭超材料几何参数对性能影响讨论
斜杆数量
n 、长度l 、直径d 、倾斜角度θ 以及有效直径D∗ 均对超材料的压扭系数和承载极限有显著影响,由于D∗ 通常会受到SHPB的杆件直径以及实际制作工艺的限制,可变化范围较小,因此不作过多分析,可根据实际情况设定D∗ =16 mm。采用有限元模型对n 、l 、d 和θ 等参数的影响进行评估。如图5所示,随着斜杆数量
n 的增加,超材料的扭转承载极限T 和轴向承载极限F 均呈上升趋势,而压扭系数λ 则几乎不受影响。虽然增大n 值可在不改变压扭系数的前提下提升承载能力,但过多斜杆会导致实际生产困难,同时考虑到2.1节中的几何制约,且斜杆不宜太细以免容易发生屈曲,因此,在D∗ 不超过20 mm且d≥ 2 mm的条件下,斜杆数量一般不超过8根。在后续讨论中,默认斜杆数量为8。讨论斜杆倾斜角度
θ 时,将l 和d 分别初步设置为20和2 mm。根据几何约束条件,此时θ 的变化范围为45°~90°。从图6中可以看出,随着θ 的增加,F 逐渐增大,而T 和λ 均呈先上升后下降的趋势,分别大约在θ =55°和θ =50°时达到峰值。这表明倾斜角度与超材料扭转效果之间并非单调关系。接着,选取λ 达到最大值时的对应参数,即θ =50°和l =20 mm,并在1~2.4 mm范围内调节d 。图7中可以看出,T 和F 均随d 的增大而增大,而λ 略微下降,且下降速率随d 的增大而有所增加。这说明增大斜杆直径可以显著提高超材料的承载能力,但在一定范围内对扭转效果的影响相对较小。最后,将θ 和d 分别设定为50°和2 mm,根据几何约束条件,l 的可调节范围大致为4~22 mm。图8中可以看出,F 随l 的增大而略有上升;T 则先上升,然后在l =10~16 mm时基本保持稳定,随后下降;λ 先增加后减小,在l =10 mm时达到最大值。为进一步验证λ 的变化规律,改变θ ,使其分别等于25°、30°和40°,同时保持d =2 mm不变,计算结果如图9所示。可以发现,λ 的变化规律未变,且最大值仍在l =10 mm时出现。因此,本文中将l 设定为10 mm,可使超材料同时具有较高的承载极限和压扭系数。2.3 SHPB系统建模与约束强度分析
2.3.1 压扭超材料和SHPB的有限元模型
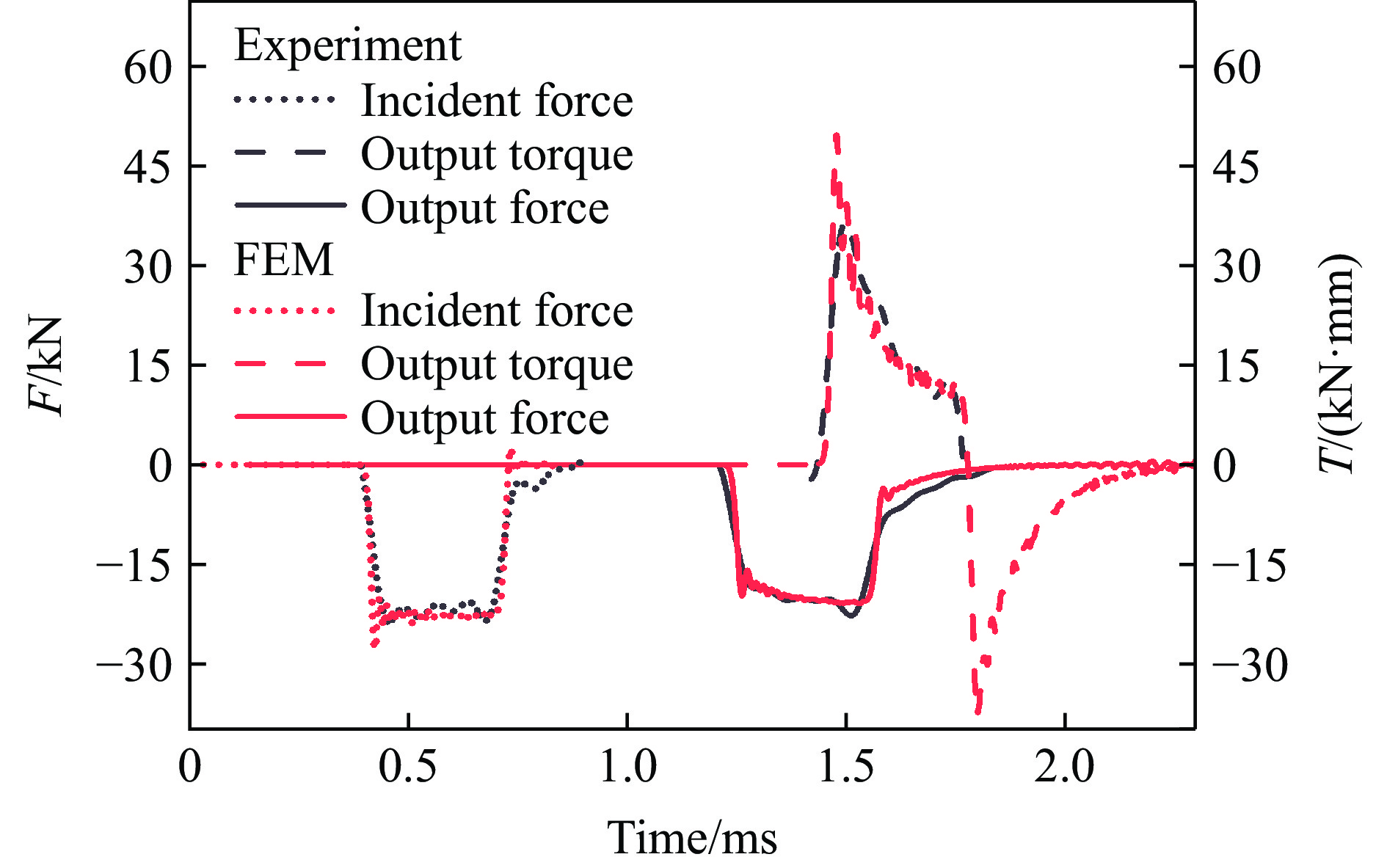
为了更准确地分析压扭超材料在冲击实验中的力学行为,建立包含SHPB系统和超材料的有限元模型(finite element model, FEM),如图10所示。在模型中,入射杆和超材料,超材料和透射杆之间均采用“Tie”接触连接方式。SHPB系统由撞击杆、入射杆和透射杆组成,长度分别为0.8、5.0和3.0 m。对撞击杆施加初速度,使其与入射杆碰撞产生输入应力波。压杆系统材料属性选用7075铝合金,具体参数列于表1。压扭超材料的基体材料为Ti6Al4V钛合金,其准静态拉伸实验的应力-应变曲线如图11中黑线所示,绿线为采用理想弹塑性模型拟合的结果,相关参数列于表1。此处未考虑钛合金的应变率效应,可能会低估超材料的承载能力。然而,考虑到超材料的打印缺陷,其实际承载能力往往低于理想状态,因此,钛合金应变率效应对评估超材料实际承载力的影响可能并不显著,故在此暂不作考虑。
表 1 有限元模型采用的材料参数Table 1. Material parameters used in the finite element model材料 密度/
(g∙cm−3)弹性模量/
GPa屈服应力/
MPa泊松比 7075铝合金 2.85 71 0.33 Ti6Al4V钛合金 4.40 120 910 0.33 2.3.2 透射杆的约束强度
根据前文的分析,在超材料几何限制范围内,通过增大斜杆直径,可在对扭转效果影响较小的基础上,显著提高超材料的承载能力,因此,将超材料斜杆直径
d 设计为3.5 mm,并在直径D 为20 mm的SHPB系统上进行实验和有限元分析,所用有限元模型与图10中的一致。在实验和有限元分析中,撞击杆在接触入射杆前的初速度均为10.3 m/s。实验中,入射杆上用于测量压缩信号的应变片组距离入射杆末端2600 mm,透射杆上用于测量压缩信号和剪切信号的应变片组分别距离透射杆前端750和950 mm。由于纵波与横波在同一介质中的传播速度不同,实验获取的原始数据中透射杆的压缩与剪切信号起始点存在一定时间间隔,为便于观察,本文中在有限元模型中提取了与实验应变片位置一致的相关数据进行对比和分析。有限元模型中轴力F 和扭矩T 均根据压杆系统中的单元应力计算得到,将有限元模型计算结果与实验结果进行对比,可发现有限元模型与实验之间吻合很好,如图12所示,说明有限元模型的有效性。然而,同时也可观察到,图12中的扭矩出现了三角波峰,与入射的梯形波相差较大。为探究这一现象,将有限元模型中的斜杆直径
d 减小至3.0、2.5和2.0 mm,同时保持其他几何参量不变,撞击杆初速度均为3 m/s。计算得到透射杆输出结果如图13(a)所示,可见扭矩的三角波形随d 的减小逐渐变得平缓。基于这一现象,推测三角波峰的出现与透射杆提供的扭转约束有关。随着d 的增加,超材料产生的扭矩相应增大,而直径只有20 mm的透射杆可能无法提供足够的扭转约束,导致超材料产生的扭矩被削减,形成三角波。为了验证这一猜想,保持d =3.5 mm不变,在有限元模型中改变SHPB的直径D ,分别设为20、30、40和50 mm,撞击杆初速度保持为3 m/s。透射杆输出结果如图13(b)所示,随着透射杆直径D 的增大,扭矩峰值也在不断升高,且波形逐渐由三角波变为梯形波。这一结果证实了上述猜测,即扭矩的三角波问题是由透射杆惯性约束不足所致。为确保增强透射杆约束以消除三角波的方法在实验中的可行性,采用直径为50 mm的SHPB进行实验验证。实验中,超材料的斜杆直径
d 为3.5 mm。有限元模拟中撞击杆的初速度与实验一致,为2.66 m/s。实验结果如图14所示,与图12中所示的实验结果相比,扭矩的三角波问题得到了明显改善。此外,相应的有限元模拟结果与实验结果吻合较好,再次验证了有限元模拟的可靠性。在实验和有限元模拟计算结果讨论中,为确保透射杆提供的惯性约束力足够,从透射杆上获得的剪切应力波形为梯形波,将全部采用直径
D 为50 mm的SHPB系统。3. 动态压缩-扭转加载实验
选取屈服强度不同的3种材料进行实验。通过测量这些材料在动态压缩-扭转加载下的力学性能,进一步验证动态压缩-扭转实验技术的可靠性及其适用材料的多样性。
3.1 实验系统
图15(a)展示了一维SHPB系统。杆件材料为7075铝合金,撞击杆、入射杆和透射杆长度分别为0.8、5.0和3.0 m,直径均为50 mm。压杆系统中用于测量试件应力的应变片位置与2.3.2节所描述的一致,应变采集仪的采样频率为800 kHz。另外配有2台高速摄影机用于记录加载过程中试件的变形情况,采集频率为150 000 Hz,图像分辨率为
1280 ×288。试件的压缩应变和扭转应变均使用3D DIC技术计算,所用软件为Vic-3D9。应力与应变数据进行关联时,先分别以应变-时间曲线和应力-时间曲线的起跳点为对应数据的初始值,然后对应力-时间数据进行插值处理,使其时间间隔与应变-时间的相同,从而应力与应变可一一对应。压扭超材料采用3D打印技术制备,基体材料为Ti6Al4V钛合金。入射杆与超材料、超材料与试件、试件与透射杆间分别进行粘接。选用金属钛、304不锈钢和316L不锈钢进行了实验测试,采用的待测试件如图15(b)所示,试件整体呈轮轴型,由上、下两块中空圆盘和中间的薄壁圆筒组成。薄壁圆筒的设计旨在确保试件的切应力分布均匀;两端外扩的圆盘设计则是为了增大粘胶面积,确保实验过程中试件的端面不与超材料或透射杆的端面发生相对位移。试件上、下中空圆盘的外径和内径均为50和13 mm,厚度均为1.5 mm,薄壁圆筒的外径和内径则分别为16和13 mm,高度为11 mm。
实验采用的压扭超材料斜杆数量
n =8,斜杆长度l =10 mm,有效直径D∗ =16 mm。为确保超材料有足够的承载力,且承载力随斜杆直径的增大而显著增大,选用较大的斜杆直径d =3.5 mm。3.2 金属钛的压缩-扭转实验
金属钛因其硬度高、质量轻,耐腐蚀性强,以及良好的可塑性和生物相容性,被广泛应用于航空航天、医疗器械和人工骨骼等多个领域。
根据2.1和2.2节中关于压扭超材料几何参数的分析结果,压扭超材料斜杆倾斜角度大约可在40°~90°范围内变化,选用50°、60°和70°。实验过程中利用高速摄像机拍摄加载过程中试件的形变,并通过DIC技术获取试件表面应变云图,以此评估试件压缩与剪切应变的均匀性。图16~17为试件表面的压缩应变与剪切应变云图,其中图16中的试件处于弹性应变范围,而图17中的试件处于塑性应变范围。图16~17中可以看出,在计算域内,大部分区域的压缩应变和剪切应变均较为均匀,未出现屈曲、鼓形变形等非均匀形变。因此,可认为薄壁圆筒试件处于均匀的压缩和扭转复合应力状态。
子弹的撞击速度约为4.2 m/s,通过DIC计算得到加载压缩应变率约为120 s−1(图18(a))。剪切应变率在被测试件弹性范围内可达200 s−1以上,但在加载的中后期降至约110 s−1(图18(b))。相应地,von-Mises应变率范围为100~200 s−1(图18(c))。虽然剪切应变率在加载后期有所下降,但材料屈服前以及屈服后一段时间内仍可保持相对稳定,因此认为利用该实验技术所获取的材料屈服点是可靠的。
在超材料斜杆倾斜角度为60°和70°条件下,各自进行了两组重复实验。以倾斜角度为70°的实验为例,将两次加载所得的金属钛的正应力-应变曲线和切应力-应变曲线进行对比,如图19(a)所示,可观察到两条曲线重合度较高,验证了该实验技术具有良好的可重复性。
改变超材料斜杆的倾斜角,可以改变作用在试件上的压力与剪力比值,将不同倾斜角度条件下获得的金属钛的von-Mises应力-应变曲线绘于图19(b)中,可观察到这些曲线高度重合,表明压缩与扭转荷载的比值对钛的von-Mises应力-应变曲线影响较小。进一步地,以von-Mises应力-应变曲线二阶导的极值点作为屈服点,将5组实验中测得的钛的屈服点对应的正应力
σs 、切应力τs 及von-Mises屈服应力σy 汇总于表2,可知各组实验的von-Mises屈服应力均接近485 MPa,与预期相符。这些实验结果验证了此实验技术的可靠性和准确性。表 2 钛的动态压缩-扭转实验结果Table 2. Dynamic compression-torsion experiment results of titanium试件编号 θ/(°) σs/MPa τs/MPa σy/MPa 1 70 453.58 117.01 496.80 2 70 429.49 126.49 482.15 3 60 374.31 174.72 481.34 4 60 382.43 180.40 493.84 5 50 346.23 198.34 487.74 3.3 304不锈钢的压缩-扭转实验
304不锈钢作为一种常见的不锈钢材料,因其卓越的耐蚀性和耐热性,在多个领域应用广泛。对304不锈钢圆柱形试件进行准静态单轴压缩实验,加载应变率为10−3 s−1,获得的应力-应变曲线如图20所示,屈服应力约为215 MPa。
在对轮轴型试件进行的动态压缩-扭转复合加载实验中,通过DIC计算得到加载压缩应变率为102 s−1。所用压扭超材料的斜杆倾斜角度
θ 分别为60°和70°。图21(a)展示了304不锈钢试件在冲击荷载作用下的正应力-应变曲线和切应力-应变曲线。可以发现,屈服点对应的切应力与正应力的比值(剪压比)会因超材料斜杆倾斜角度的不同而发生变化,且随着斜杆倾斜角度的增大,该比值呈现下降趋势。图21(b)展示了2组实验中被测材料的von-Mises应力-应变曲线,并标出了屈服点。屈服点对应的正应力、切应力和von-Mises应力被汇总于表3。结果显示,在两种不同的压缩-扭转荷载作用下,304不锈钢的von-Mises应力-应变曲线非常接近,屈服应力也几乎一致,约为412 MPa。这一数值明显高于准静态压缩加载条件下的屈服应力,表明304不锈钢材料具有显著的应变率效应。表 3 304不锈钢的动态压缩-扭转实验结果Table 3. Dynamic compression-torsion experiment results of 304 stainless steel试件编号 θ/(°) σs/MPa τs/MPa σy/MPa 1 70 350.08 125.87 412.42 2 60 305.43 160.12 412.56 3.4 316L不锈钢的压缩-扭转实验
316L不锈钢同样是一种在日常生活中常见的金属材料,并凭借其独特的元素配比,相较于304不锈钢,展现出更为卓越的抗腐蚀能力,被广泛应用于耐腐蚀性要求严苛的领域。制作与金属钛和304不锈钢试件几何尺寸相同的316L轮轴型试件,并进行动态实验,子弹撞击速度约为4.5 m/s。实验所用超材料斜杆倾斜角度
θ 分别为50°、60°和70°。通过对超材料在3种不同斜杆倾斜角度条件下的316L不锈钢试件的正应力-应变曲线和切应力-应变曲线进行对比分析可知(图22(a)),随着倾斜角度的增大,超材料压扭系数
λ 减小,被测试件屈服点的正应力逐渐增大,而切应力则逐渐减小。此外,图22(b)展示了316L不锈钢试件在不同斜杆倾斜角度下的von-Mises应力-应变曲线,这些曲线展现了较高的重合度,表明压扭系数λ 对316L不锈钢的von-Mises应力-应变影响较小。表4则列出了316L不锈钢的von-Mises屈服强度数据,显示其可达575 MPa以上,说明本文中提出的实验技术对强度较高的材料也适用。表 4 316L不锈钢的动态压缩-扭转实验结果Table 4. Dynamic compression-torsion experiment results of 316L stainless steel试件编号 θ/(°) σs/MPa τs/MPa σy/MPa 1 70 471.19 172.86 558.27 2 60 440.02 221.21 583.45 3 50 402.85 237.92 576.28 3.5 问题与讨论
以上实验结果验证了动态压剪复合加载技术在测量材料屈服强度等关键参数方面的有效性与可靠性。然而,作为一项处于创新阶段的实验技术,仍然存在一定的优化空间与值得深入分析讨论的内容。
首先,由于压扭超材料的存在,难以测量试件的反射波,从而不能像传统方法一样通过入射波、透射波和反射波的关系来推算试件的两端是否应力平衡。根据应变与应力的相关性,通过观察试件的应变云图来评估试件上的应力分布,这种做法的准确度容易受到DIC计算精度的限制。
由于应变的测量完全依赖DIC计算,DIC的计算精度对实验结果存在明显影响。实验中应变观测区域主要位于试件的中间位置,观测区域投影的大小约为4.5 mm×7.5 mm。此投影在宽度上偏小,这是因为在实际实验中,当SHPB杆径相较于试件外径较大时,容易遮挡相机对试件表面带有散斑的圆柱形区域的拍摄。此问题在未来工作中可以通过适当减小压杆杆径或完善试件构型设计来解决,例如增大圆柱试件的直径等。另外,本次实验中所用透射杆直径为50 mm,长度为3 m,具有较大惯性,加载中几乎不发生刚体位移,又由于被测试件的压缩与剪切应变均不超过0.05,处于小变形范围内,所以应变观测区域的移动量及其对应变测量结果的影响程度均较小,计算结果较为稳定。但若在某些实验中,应变观测区域移动量较大,则有可能影响其DIC计算精度,必要时需要对其应变测量方法进行改善。
其次,现有应力、应变结果的关联方法可能存在人为偏差,偏差原因主要在于应力与应变起始点的选择。在未来工作中,可尝试通过在动态应变仪与高速摄影机之间设置同步触发装置的方式来提高数据起始点的准确性。此外,两者的关联精度也会受到高速摄影机帧率的影响,帧率越高,应力-应变曲线弹性段的数据点就越充分,从而对关联精度也会有一定改善。
再者,图18(b)中试件的剪切应变率在试件屈服后一段时间出现了明显下降,而图18(a)中的压缩应变率则相对稳定,直至卸载压缩应变率才出现大幅度下降。造成这种现象的主要原因可能在于,当试件屈服进入塑性段后,轴向压力的增幅和增速将下降,由于力平衡,压扭超材料的轴向压力的增速也必然下降,且压扭超材料的扭矩与压力的比值是恒定值,所以压扭超材料的扭转变形速率,同时也是试件的扭转变形速率也随之下降;而对于压缩应变率而言,加载过程中输入的压缩波(由子弹撞击入射杆产生)在试件屈服后仍持续输入,因此可维持相对稳定。
对于应变率敏感材料,剪切应变率的下降会带来切应力的降低。当试件在进入屈服一段时间后,其剪切应力曲线呈下降趋势,可能就是这一原因造成。对比图18(b)中的剪切应变率曲线与图19(a)中的剪切应力曲线,应变率和应力下降的转折点大致相同,而参考文献[32]中的铝是非应变率敏感材料,其剪切应力曲线并未出现降低现象,这些现象可在较大程度上证实这一猜想的合理性。由于剪切应变率下降出现在加载中后期,对材料屈服强度的测量精度并无影响,因此不影响这一实验技术的可靠性。
另外,虽然根据我们对高速摄影得到的试件变形图像的观察和对图像质量的评估,认为在本文的实验中出现测量畸变的可能性较小。但若实验条件发生改变,存在冲击过程中发生测量畸变的可能。因此,实验中必须保证被测试件结构设计合理且图像质量较高,提高实验结果的可靠性。
4. 适用范围讨论
在316L不锈钢的动态压扭实验中发现,此实验技术可适用于强度较高的材料。本节将结合有限元进一步讨论此实验技术的适用范围。
由于超材料作为应力波转化的功能部件,要求超材料在冲击应力下不发生塑性变形且可提供的剪压比范围尽可能大,因此该实验技术的适用范围取决于超材料在弹性范围内的承载能力和压扭系数。
在有限元模型中将压扭超材料固定于入射杆与透射杆之间,与2.3节中的类似,但撞击杆、入射杆和透射杆的直径变为50 mm。超材料在冲击过程中受到的轴力
F 和扭矩T 可通过透射杆上的应力计算得到。同样以超材料荷载-位移曲线的二次导数的极小值点作为屈服点,该点对应的轴力和扭矩为超材料在弹性范围内所能承受的极限载荷。根据2.2.2节中的讨论结果,当斜杆长度
l =10 mm时,超材料可呈现较优的扭转效果,且承载能力也较强,因此,下面固定斜杆长度l =10 mm不变,主要通过调节斜杆直径d 和斜杆倾斜角度θ 来确定超材料的承载范围和压扭系数范围。虽然从图7(b)已可获知,随着斜杆直径d 的增大,压扭系数λ 呈轻微下降趋势,但图7(b)中d 的变化范围较小,λ 随d 的变化规律有必要做进一步分析。令d =2.0, 3.0, 3.5 mm,同时保持斜杆数量n 和长度l 分别为8和10 mm不变,根据2.1节中提及的几何限制条件,选定θ 的变化范围分别为25°~90°、40°~90°和50°~90°。图23展示了超材料输出的扭矩
T 和轴力F 的关系。图中各点横、纵坐标分别表示压扭超材料屈服点对应的轴力与扭矩。不同颜色曲线的右侧边界线表示不同斜杆直径下超材料的承载极限;左侧边界线斜率与超材料压扭系数极限正相关;曲线围成的阴影区域代表了压扭超材料在弹性范围内所能提供的扭矩和轴向荷载的范围。可以看出,随着d 的增加,超材料的承载极限也相应提高,对被测试件可提供的载荷增大;但左边界线的斜率逐渐减小,说明随d 增大,超材料能提供的T/F 的最大值减小,即被测试件所受剪压比范围减小。当d =2.0 mm时,超材料可提供的最大压扭系数λ =1.07,此时对应的扭矩和轴向荷载分别为T =62.17 kN∙mm和F =7.27 kN(图23中A点)。当d =3.5 mm时,超材料可提供的最大扭矩为T =211.40 kN∙mm,此时对应的轴向荷载F 和压扭系数λ 分别为48.66 kN和0.54(图23中B点)。为了更直观地展示此动态压剪复合加载实验技术的可测材料范围,根据图23中所示的超材料能提供的压力和扭矩范围,以及力平衡原理,计算出对应的被测试件的正应力和切应力的范围。例如,对于外径16 mm、内径13 mm的轮轴型待测试件,该实验技术能测试的材料应力范围如图24中阴影部分所示。图中的散点为表2~4以及文献[32]中实验测得的各种材料屈服点所对应的正应力和切应力数据,这些材料的屈服强度在数十至数百兆帕范围,各数据点均落于阴影区域内,且与边界线仍有一定距离,说明该技术还可用于测试更高强度材料的力学性能。
图24中虚线表示应力为1 GPa对应的von-Mises屈服面,可以看出,该屈服面处于阴影区域内,为该实验技术的可测试范围,因此对于外径16 mm、内径13 mm的轮轴型试件,预测该实验技术能测试的材料强度可达约1 GPa。并且由图24中B点可知,该实验技术能施加给材料的切应力为425 MPa,此时对应的正应力约为712 MPa,剪压比为0.59;由图24中A点可知,施加给试件的切应力与正应力的比值可达1.18,此时切应力为125 MPa,正应力为106 MPa。
需要注意的是,图24所示的适用范围是基于特定的被测试件构型、特定的压扭超材料几何参数和基材提出的,若相关参数有所调整,则对应的适用范围也随之改变。然而,这并不影响说明该技术在多种材料测试中的普遍适用性。另外,对于存在2种塑性流动率的泡沫金属,单轴压缩不足以表征材料的力学行为,但复合压剪加载则可以通过一次加载得到材料在当前应变率下的力学行为,因此,本技术对泡沫类材料的研究具有重要意义[33]。不仅如此,若使用聚合物作为超材料的基材,还可尝试用于测试强度在1 MPa以下的软材料。总之,动态压剪同步复合加载技术在材料测试方面具有巨大的应用潜力。
5. 结 论
本文中介绍了一种创新的动态压剪复合加载实验技术,结合了压扭超材料和一维SHPB,探讨了超材料的几何参数、透射杆的约束强度以及此实验技术的适用范围等,主要结论归纳如下。
(1)提出利用压扭超材料进行应力波转化,在一维SHPB上实现动态压剪同步复合加载的新实验技术。该技术具有确保荷载同步性、被测试件所受剪压比可控、易操作及低成本等优点。当扭转波幅值较大导致剪切应力呈现为三角波时,可通过增大透射杆直径以增强其惯性约束来解决。
(2)对金属钛、304不锈钢和316L不锈钢开展了动态压剪复合加载实验,测得了其在复杂应力状态下的屈服应力,通过这3种材料的实验结果验证了该实验技术的可行性。
(3)通过研究压扭超材料的几何参数对其承载能力和压扭耦合效应的影响,讨论了该实验技术的适用范围,预测该实验技术可测试的材料强度可达约1 GPa,剪压比可达1.18。
本文中提出的结合压扭超材料实现动态复合加载的思想并不局限于SHPB,亦可应用于其他动态加载和准静态加载装置。同时,这一思想并不局限于压缩-扭转加载,其他类型的力学超材料[34-35]有望实现更多样化的加载方式。总之,利用超材料实现应力波转换这一思路,可为实现更多复杂的动态加载实验技术开辟新的研究方向。
-
表 1 油库方向监测数据
Table 1. Data monitored along the direction from the quarry to the oil depot
测点编号 R/m Q/kg qmax/kg vh, t/(cm·s-1) vh, l/(cm·s-1) vv/(cm·s-1) 1
2335
3406 420 350 0.48
0.460.41
0.460.64
0.573
4
5
6
7339
387
431
465
4952 872 288 0.31
0.24
0.22
0.17
0.310.38
0.29
0.20
0.16
0.090.40
0.22
0.33
0.22
0.228
9
10297
307
2422 040 264 0.40
0.32
0.910.22
0.27
0.290.84
0.49
1.0111
12
13288
300
2322 408 276 0.51
0.45
0.880.43
0.40
0.760.59
0.36
0.9914
15
16364
363
3272 832 386 0.55
0.52
0.560.25
0.31
0.520.69
0.51
0.7317
18275
2873 168 280 0.70
0.530.46
0.370.79
0.5919
20401
4107 000 387 0.39
0.250.38
0.310.55
0.3721 181 2 848 183 0.54 0.51 0.62 22
23173
1963 720 186 0.63
0.430.61
0.410.64
0.5924
25
26
27
28309
324
321
347
3644 928 264 0.48
0.30
0.30
0.28
0.260.34
0.32
0.23
0.26
0.210.44
0.31
0.52
0.30
0.31表 2 计算结果
Table 2. Calculation results
方向 K α r γs, r γs, e 1-β=95% 1-β=99% 1-β=95% 1-β=99% 水平纵向 130.4 1.45 0.77 1.75 2.15 1.87 2.34 水平横向 152.9 1.59 0.79 1.73 2.12 1.85 2.29 垂直向 179.4 1.53 0.74 1.90 2.39 1.99 2.54 -
[1] 刘殿书.爆破工程[M].北京: 科学出版社, 2011: 98-133. [2] 吕涛, 石永强, 黄诚, 等.非线性回归分析法求解爆破震动速度衰减公式参数[J].岩土力学, 2007, 28(9): 1871-1878. http://www.cqvip.com/Main/Detail.aspx?id=25430136Lü Tao, Shi Yong-qiang, Huang Cheng, et al. Study on attenuation parameters of blasting vibration by nonlinear regression analysis[J]. Rock and Soil Mechanics, 2007, 28(9): 1871-1878. http://www.cqvip.com/Main/Detail.aspx?id=25430136 [3] 言志信, 王永和, 江平, 等.爆破地震测试及建筑结构安全标准研究[J].岩石力学与工程学报. 2003, 22(11): 1907-1911.Yan Zhi-xin, Wang Yong-he, Jiang Ping, et al. Study on measurement of blast-induced seism and building safety criteria[J]. Journal of Rock Mechanics and Engineering, 2003, 22(11): 1907-1911. [4] 中国工程爆破协会. GB6722-2011, 爆破安全规程[S].北京: 中国标准出版社, 2011. [5] 杨佑发, 崔波.爆破震动速度峰值预测[J].振与冲击, 2009, 28(10): 195-198. http://www.cqvip.com/Main/Detail.aspx?id=31994360Yang You-fa, Cui Bo. Prediction for vibration intensity due to blasting induced ground motions[J]. Journal of Vibration and Shock, 2009, 28(10): 195-198. http://www.cqvip.com/Main/Detail.aspx?id=31994360 [6] 盛骤.概率论与数理统计[M].北京: 高等教育出版社, 2008: 40-60. [7] Kim D S, Lee J S. Propagation and attenuation characteristics of various ground vibrations[J]. Soil Dynamics and Earthquake Engineering, 2000, 19(2): 115-126. doi: 10.1016/S0267-7261(00)00002-6 [8] 杜汉清.爆破振动衰减规律的现场试验研究[J].爆破, 2007, 24(3): 107-109. http://www.cqvip.com/Main/Detail.aspx?id=25457763Du Han-qing. Experimentally study on the attenuation law of blasting vibration[J]. Blasting, 2007, 24(3): 107-109. http://www.cqvip.com/Main/Detail.aspx?id=25457763 [9] 毕卫国, 石崇.爆破振动速度衰减公式的优化选择[J].岩土力学, 2004, 25(增刊): 99-102. http://www.cnki.com.cn/Article/CJFDTotal-YTLX2004S100N.htmBi Wei-guo, Shi Chong. Optimization selection of blasting vibration velocity attenuation formulas[J]. Rock and Soil Mechanics, 2004, 25(Suppl): 99-102. http://www.cnki.com.cn/Article/CJFDTotal-YTLX2004S100N.htm -










 下载:
下载:













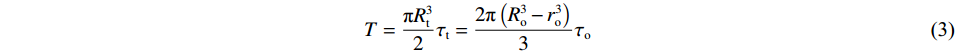







































































































































































 下载:
下载: