Theoretical and numerical study on detonation wave Mach reflection in high explosive charge with waveshaper
-
摘要: 基于三波理论和Whitham方法对带隔板装药爆轰波相互作用后发生的正规反射和非正规反射进行了理论分析,给出了爆轰波发生马赫反射时临界入射角和马赫杆增长角等参数的变化规律,提出了马赫杆高度的计算模型。基于凝聚炸药爆轰Jones-Wilkins-Lee(JWL)模型和冲击起爆的Lee-Tarver模型,利用有限元计算软件对带隔板装药爆轰波的传播过程进行了数值模拟。结果表明,发生马赫反射后,随着爆轰波的传播,马赫杆的高度不断增加。数值模拟结果与理论计算结果吻合较好,说明本文中采用的理论模型和数值模拟方法能够较准确地描述带隔板装药爆轰波马赫反射的传播过程。Abstract: On the basis of the three-wave theory and Whitham's method, the flow fields associated with regular reflection and Mach reflection in high explosives with waveshapers were investigated, and the relevant theoretical model for deriving the detonation configuration was proposed. The calculated results of pressure, flow velocity and triple point growth angle of Mach stem were presented and the Mach stem height was also determined based on the modified Whitham's method. The finite element code was used to numerically simulate the detonation processes of the high explosives with waveshapers. The shock initiation of the cylindrical charge was described by the Jones-Wilkins-Lee(JWL) and Lee-Travel models. The calculated results show that the Mach stem height increases with the propagation of detonation wave. The numerical results are consistent with the predictions based on the presented model, which shows that the analytical model provides reasonably accurate predictions of the Mach reflection process.
-
在常规武器系统中,聚能装药战斗部扮演着越来越重要的角色,提高炸药能量利用率是战斗部设计者追求的目标之一。带隔板装药不仅结构简单而且可以在不增加装药量以及不改变装药长径比的条件下,提高其对药型罩的压垮做功能力。典型的带隔板装药结构如图 1所示,根据惠更斯原理[1],爆轰波经过隔板的绕射后将以O′点开始传播,以较小的入射角驱动药型罩形成聚能侵彻体。这类带隔板装药在驱动药型罩形成高速杆式射流、大长径比爆炸成型弹丸以及利用起爆控制技术形成多模毁伤元等方面应用非常广泛。
早在20世纪70年代,汤明钧等[2]利用高速摄影测定了带隔板破甲弹中装药端面上爆轰波的爆速和爆压,得出马赫波的波速达到正常爆速的1.9倍,爆压达到正常CJ爆压的3倍。杨全中等[3]对带隔板装药爆轰波的相互作用进行了研究,并利用Whitham方法[4]计算了爆轰波传播过程中的波形和碰撞临界角等参数。B.B.Dunne等[5]指出了发生马赫反射的临界入射角取决于爆轰产物的多方指数,并给出了马赫杆压力等参数的计算方法。L.M.Hull等[6]计算了球面爆轰波的马赫反射参数,通过实验给出了PBX9501和PBXN-9等炸药的马赫反射参数。张俊秀等[7]进行了爆轰波马赫反射的实验研究,并根据修改的Whitham方法[4]计算了马赫反射的压力、爆速和增长角等参数。Zhang Xianfeng等[8]、张先锋等[9]分别利用理论计算和数值模拟分析了夹层装药爆轰波的传播过程,并基于质量守恒给出了爆轰波传播过程中马赫杆高度的计算模型。
本文中,基于三波理论[3]和Whitham方法[4],对带隔板装药爆轰波相互作用的正规反射和马赫反射进行理论分析,并给出马赫杆高度的计算模型。在此基础上,利用AUTODYN有限元计算程序中的Lee-Tarver模型[10]对带隔板装药爆轰波传播过程进行数值模拟, 以期研究成果可为描述带隔板装药驱动典型介质提供理论基础和数据支持。
1. 带隔板装药爆轰波马赫反射理论模型
1.1 带隔板装药爆轰波相互作用
2个等强度爆轰波的正规斜碰撞,可视为其中一侧爆轰波对刚性壁面的正规斜碰撞,当入射角φⅠ较小时,流入界面I和界面R的粒子流的偏转角相等。入射角不断增大,达到马赫反射的临界入射角φⅠ, c时,偏转角θ2小于θ1。粒子流的不断积累迫使碰撞点P移动到Q点,便形成了图 2所示的马赫反射。基于F.Grasso等[11]关于爆轰波反射的描述,爆轰前反应区用带下标1的参数表示,反射波前反应区用带下标2的参数表示,超压爆轰反应区用带下标3的参数表示。
假设炸药完全反应且满足CJ条件,区域(1)中的爆轰参数可由炸药的CJ参数、初始密度ρ0、多方指数γ和入射角获得。粒子流通过区域(1)发生偏转流入区域(2),偏转角和反射角满足下列关系:
tanθ1=[Ma21sin2(φⅡ+ε)−1]tan−1(φⅡ+ε)Ma21[(γ+1)/2−sin2(φⅡ+ε)]+1 (1) 式中Ma1=√1+(γ+1)2/(γtanφⅠ)2为马赫数; 发生正规反射时,ε=θ1;发生马赫反射时,ε=θ1-θ2。
发生正规反射和非正规反射时, 式(1)中ε的表达式是不同的,若用发生正规反射时ε=θ1求解发生非正规反射时式子(1)的表达式,在0<φⅡ+ε<π/2范围内必定无解。根据此条件可以获得发生马赫反射的临界入射角,不同炸药临界入射角的理论计算值和实验结果如表 1所示, 表中DCJ为炸药CJ爆轰速度。通过比较发现,两者比较接近,从而可以验证马赫反射临界入射角计算的正确性。通过计算还发现,发生马赫反射时的临界入射角与炸药多方指数成一一对应关系,随着多方指数的增大,发生马赫反射的临界入射角不断减小,如图 3所示,此结论与B.B.Dunne[5]给出的结果一致。
表 1 不同炸药装药中发生马赫反射时临界入射角的计算值与实验结果Table 1. Calculated and experimental results of critical incident angles corresponding to Mach reflection in different explosive charges大量理论和实验研究发现[5, 11-12],马赫杆是一个弯曲的曲面,交于对称轴P点,相切于爆轰波(I)Q点。在P点, 偏转角α=θ1-θ2=0°; 在Q点, α=θ1-θ2;马赫杆与对称轴切线的夹角从π/2变化到φⅠ。由于碰撞点P处的压力和密度等都远大于CJ值,难以准确获取,本文中借助炸药爆轰产物的状态方程p=p(E, v)来求得此处的压力, 其中p为压力,E为炸药能量,v为比容。由H.M.Sternberg等[12]的定义,产生马赫反射时爆轰产物中将从三波点产生一条滑动线。此直线通过的地方,压力是连续的,但速度和密度非连续,因此,在p3=p2处存在一个三波点。
1.2 马赫杆高度模型
基于Whitham方法[4, 13]获取马赫反射的增长角和高度等参数,并将马赫波简化为平面波。将爆轰波的每一元素沿每个微元射线管的传播当作固壁管道中的激波传播来处理,在二维情况下,爆轰波的位置和与其垂直的射线位置如图 4所示。爆轰波的传播过程可以看成是在变截面流管中的传播过程,当截面发生微小变化时,流动符合等熵流性质,得到马赫反射的A(z)关系式为:
Af(z)=A0f(1)=const (2) 式中:z=p/pCJ为爆轰波强度,且:
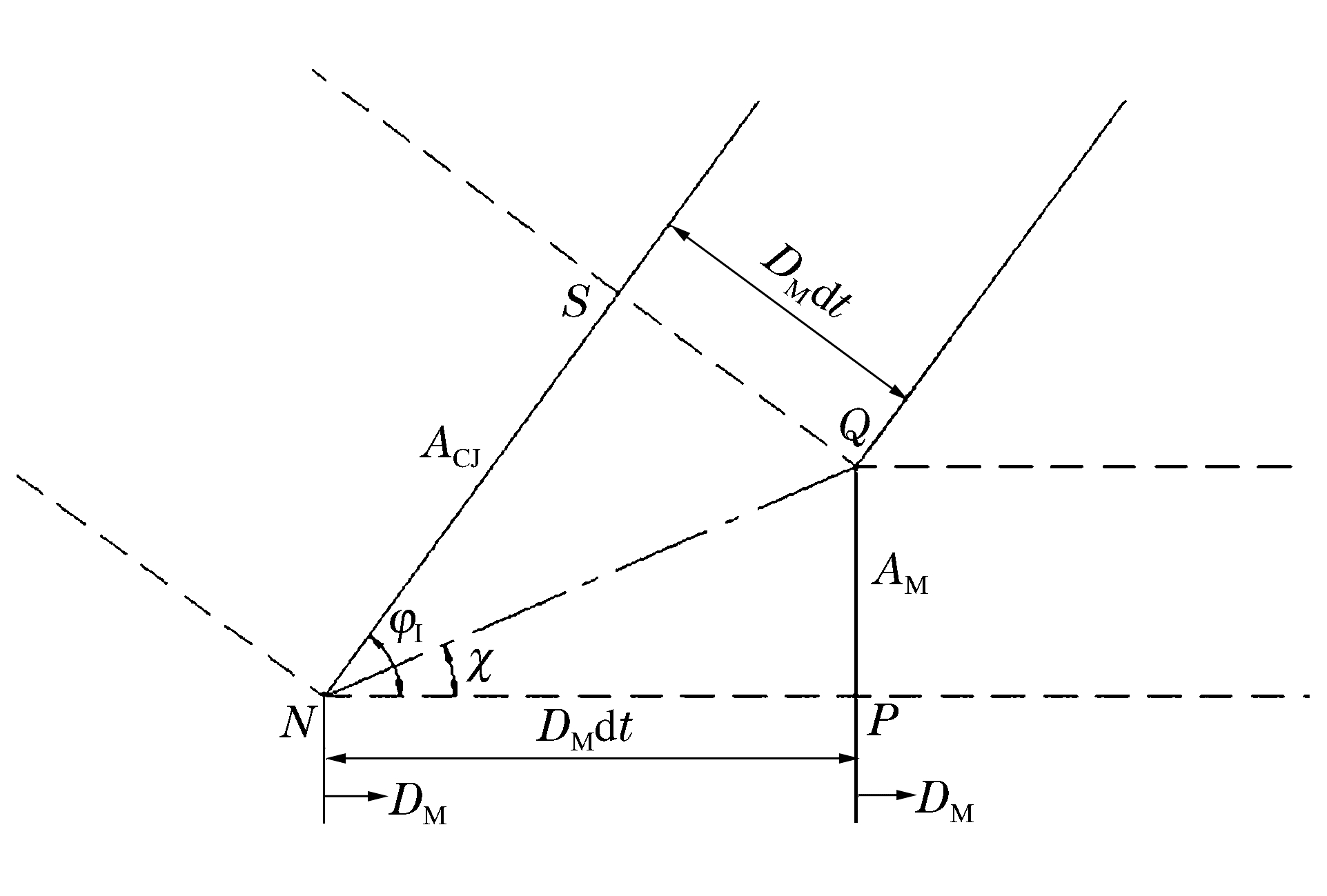
f(z)=z1/γ(2z−1)1/2B(z)C(z);C(z)=exp{γ−1/2sin−1[(3−γ)z−2(γ+1)z]} B(z)={[2(γ−1)(2z−1)]1/2+2[(γ−1)z+1]1/2}[2γ/(γ−1)]1/2 基于上述讨论,如图 4所示, 假设Δt时刻后,由爆轰波NS变成马赫波PQ,φⅠ为爆轰波入射角,χ为三波点增长角,N和Q点为不同时刻的三波点。设NS管道截面积为ACJ,PQ处管道截面积为AM,根据几何关系知:
tanφⅠ=(DCJDM+AMACJ){[1−(DCJDM)2][1−(AMACJ)2]}−1/2 (3) cosχsin(φⅠ−χ)=DMDCJ (4) sinχcos(φⅠ−χ)=AMACJ (5) 由式(5)知:
AMACJ=f(1)f(z) (6) 参考B.D.Lambourn等[14]的研究,将爆速DM和DCJ定义为:
DMDCJ=z(2z−1)1/2 (7) 上述计算中未考虑爆轰波后稀疏波的影响,计算结果误差较大,对Whitham方法[4, 13]中的A(z)关系式进行修正:
ACJAM=(2z−1)12k(z) (8) 式中:k(z)是慢变函数,一般取常数, 通常由实验确定。
根据张俊秀等[7]提供的入射角和爆速参数计算方法,通过方程(2)~(8),得到马赫波参数DM、z和χ的计算结果如表 2所示。利用Whitham方法[4, 13]计算获得的马赫波速度和三波点增长角与实验结果[7]吻合较好,从而验证此方法是可行的。
φⅠ/(°) DCJ/(km·s-1) DM, exp/(km·s-1) DM, cal/(km·s-1) DM,exp−DM,calDM,exp/% z χexp/(°) χcal/(°) χexp−χcalχexp/% 46.5 4.14 5.89 5.86 0.5 3.42 1.5 1.55 -3.2 48.5 4.20 5.77 5.79 -0.3 3.22 2.27 2.13 6.6 49.5 4.48 6.07 6.11 -0.7 3.13 2.80 2.45 14.3 同一装药中炸药的CJ爆速为常数,三波点增长角只与入射角和k(z)有关。根据带隔板装药爆轰波传播的特点,将入射角离散化,网格点(n, n)上的值可由网格点(n-1, n-1)上的值求得,马赫杆的高度可以定义为:
{Hn=Rtanχn−1tanφn−1−Ln−1tanφn−1+Hn−11+tanχn−1tanφn−1Ln=Ln−1tanχn−1tanφn−1+(R−Hn−1)tanφn−11+tanχn−1tanφn−1 (9) 式中:R为隔板半径;Hn和Ln分别为马赫杆的高度和传播距离,n=1, 2, 3, …。
当n=1时,H0=1, L1=Rtanφ0。L.M.Hull[5]也认为,当入射角为临界入射角时,马赫杆高度为零或非常小。结合表 1的数据和方程(3)~(9),可以确定马赫杆高度。
爆轰波绕射过隔板后,爆轰波不断向轴线方向汇聚,入射角不断增大。当入射角小于临界入射角时,发生正规反射,马赫杆高度为零。当入射角大于临界入射角时,发生马赫反射,马赫杆高度随着入射角的增大而增大,相同炸药中,随着k(z)的变化,马赫杆高度计算结果如图 5所示。马赫杆在不断增大的过程中,马赫杆上的压力和速度迅速降低,当马赫杆上的压力和速度降低到CJ值时,马赫杆消失,此时,马赫反射变成正常的CJ爆轰。
2. 带隔板装药爆轰波传播及马赫反射过程数值模拟
2.1 有限元计算方案
根据前述对带隔板装药爆轰波传播过程的分析,在保证隔板外侧爆轰波可以正常传播的条件下,隔板直径越大,调整爆轰波形状的能力越强,在装药结构中爆轰波马赫杆的形状就越明显。带隔板装药爆轰波的传播过程是一种多物质相互作用的大变形运动过程,因此本文中采用多物质耦合的Euler算法描述带隔板装药爆轰波的传播过程。图 6所示为装药的有限元模型。
2.2 材料模型及参数
凝聚炸药爆轰产物状态方程描述炸药爆轰达到CJ状态后爆轰产物中压力、温度和体积等物理量之间的关系,不仅体现炸药本身的做功能力,而且是研究爆炸力学各种问题的基础。本文中分别采用点火与增长模型的Lee-Tarver状态方程[10]和JWL状态方程[15]来描述爆轰波绕射过隔板后在炸药中的传播过程。炸药参数主要来自文献[10, 15]及AUTODYN中内置的参数。
为了获取环形爆轰波形并保证透射波不起爆主装药,隔板厚度应足够大,这里隔板厚度选20 mm。隔板直径选42 mm,药柱直径选50 mm,使得隔板外侧装药厚度大于爆轰波正常传播时装药的临界直径。隔板材料为环氧树脂,其状态方程为Puff状态方程[16],主要参数分别为:ρ0=1.985 g/cm3,A1=17.89 MPa,A2=201.2 MPa,A3=-6.053 MPa,Grüneisen系数为1.72,膨胀系数为0.25。
2.3 数值模拟结果与分析
针对上述讨论,对主装药分别采用JWL[15]和Lee-Travel[10]2种不同的状态方程进行描述,爆轰波波形的模拟结果如图 7所示。基于JWL模型[15]所形成的爆轰波的波阵面存在多段线组合形式,如图 7(a)所示。产生该结果的主要原因是:由于JWL模型为程序起爆模式,而炸药微元的爆轰采用预先设定时间起爆,JWL模型在描述带隔板装药爆轰(特别是隔板直径较大)时存在局限性,较难精确描述炸药微元的超压爆轰行为。基于炸药冲击起爆点火与增长的Lee-Travel模型[10]形成的爆轰波形较光滑,如图 7(b)所示。通过对比发现,Lee-Travel模型[10]能更真实地描述带隔板装药爆轰波的传播过程。
对带隔板装药中的主副装药分别采用JWL[15]和Lee-Travel[10]2种不同的状态方程进行描述,爆轰波传播过程中不同时刻的波形如图 8所示。装药起爆后,爆轰波开始以球面波向前传播,经过隔板绕射后变成汇聚爆轰波。汇聚爆轰波在装药轴线处发生相互碰撞反射,当t=86 μs时,爆轰波已经发生马赫反射,随着爆轰波的传播, 马赫杆的高度不断增加,如图 8(b)和(c)所示。随着爆轰波的传播,马赫杆的高度不断增加,但其压力和速度迅速降低,当马赫杆上的压力和爆轰速度等参数降低到CJ值时,马赫反射消失,马赫反射变成正常爆轰, 如图 8(d)所示。
为了进一步了解带隔板装药爆轰波传播过程中发生马赫发射后参数的变化,在装药轴线以及距轴线2.5、5.0、7.5和10.0 mm处分别设有一系列高斯点。以TNT炸药为例,通过设置高斯点可以获取爆轰产物的参数变化,当马赫反射发生后,随着爆轰波的传播,不同位置处压力和密度的变化见图 9~10。发生马赫反射时,装药轴线处(即马赫杆碰撞点处)的压力和密度均达到最大值,其中最大压力与CJ压力的比值达到4.3,最大密度与CJ密度的比为2.05,与Zhang Xianfeng等[8]对TNT装药发生马赫反射时最大压力和密度的理论计算值接近。随着爆轰波的传播,马赫杆碰撞点的压力和密度逐渐降低。通过对距轴线不同距离处设置高斯点发现,压力和密度先增大后减小,这说明马赫杆存在一定高度且是不断变化的。
为了获取带隔板装药中马赫杆高度的变化,分别对带隔板装药中的Comp B、TNT和PBX9404炸药爆轰波的传播过程进行了数值模拟。随着爆轰波的传播,马赫杆高度的精确捕捉越来越难,其数值模拟结果和理论计算结果如图 11~13所示。
当入射角较小时,马赫杆的高度为零,随着入射角不断增大并达到临界入射角时,马赫反射发生。此时,马赫杆碰撞点的压力和密度均达到最大值,随爆轰波的传播,马赫杆高度不断增加,马赫杆上的压力和密度不断减小。当马赫杆上的压力和密度等降到CJ值时,马赫反射消失,变成正常爆轰。
3. 结论
通过对带隔板装药爆轰波马赫反射过程的理论分析以及数值模拟,得到的主要结论如下:
(1) 基于三波理论和修正的Whitham方法,得到了马赫反射临界入射角和三波点增长角等参数,最终获得了马赫杆高度的计算模型。计算结果表明:爆轰波相互作用发生马赫反射时,马赫杆的高度为零;随着爆轰波的传播,马赫杆的高度不断增加,马赫杆上的压力和速度降低,当其降低到CJ值时,马赫反射消失。
(2) 基于点火增长模型的Lee-Travel模型,带隔板装药爆轰波发生马赫反射后马赫杆高度的数值模拟结果与理论计算结果吻合较好,这说明利用Lee-Travel模型可以较准确地描述带隔板装药爆轰波的传播过程。
(3) 带隔板装药技术是一种利用爆轰波相互作用产生超压爆轰的装药技术。马赫杆高度模型的建立不仅丰富和完善了带隔板装药爆轰波马赫反射的传播规律,而且可为描述带隔板装药驱动药型罩的过程提供理论基础。
-
表 1 不同炸药装药中发生马赫反射时临界入射角的计算值与实验结果
Table 1. Calculated and experimental results of critical incident angles corresponding to Mach reflection in different explosive charges
φⅠ/(°) DCJ/(km·s-1) DM, exp/(km·s-1) DM, cal/(km·s-1) DM,exp−DM,calDM,exp/% z χexp/(°) χcal/(°) χexp−χcalχexp/% 46.5 4.14 5.89 5.86 0.5 3.42 1.5 1.55 -3.2 48.5 4.20 5.77 5.79 -0.3 3.22 2.27 2.13 6.6 49.5 4.48 6.07 6.11 -0.7 3.13 2.80 2.45 14.3 -
[1] Dowker J S. Quantum mechanics on group space and Huygens' principle[J]. Annals of Physics, 1971, 62(2):361-382. doi: 10.1016/0003-4916(71)90096-0 [2] 汤明钧, 杨全中, 阎秀华.破甲弹中爆轰波相互作用的初步研究[J].南京理工大学学报:自然科学版, 1979(1):145-156. http://www.cnki.com.cn/Article/CJFDTOTAL-NJLG197901008.htmTang Mingjun, Yang Quanzhong, Yan Xiuhua. Preliminary study of detonation wave interaction in HEAT[J]. Journal of Nanjing University of Science Technology:Nature Science, 1979(1):145-156. http://www.cnki.com.cn/Article/CJFDTOTAL-NJLG197901008.htm [3] 杨全中, 范宝春, 杨崇惠.带隔板装药中爆轰波的相互作用[J].南京理工大学学报:然科学版, 1982(2):23-30. http://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ201604003.htmYang Quanzhong, Fan Baochun, Yang Chonghui. Interaction of the detonation waves in the explosive charge with the inserter[J]. Journal of Nanjing University of Science Technology:Nature Science, 1982(2):23-30. http://www.cnki.com.cn/Article/CJFDTOTAL-BZCJ201604003.htm [4] Whitham G B. A new approach to problems of shock dynamics:Part Ⅰ:Two-dimension problem[J]. Journal of Fluid Mechanics, 1957, 2(2):145-171. doi: 10.1017/S002211205700004X [5] Dunne B B. Mach reflection of detonation waves in condensed high explosives:Ⅰ[J]. Physics of Fluids, 1961, 4(7):918-933. doi: 10.1063/1.1706425 [6] Hull L M. Mach reflection of spherical detonation waves[C]//Short J M. Proceedings of the 10th International Symposium on Detonationm. Boston: Plenum Publishing Corporation, 1993: 12-16. [7] 张俊秀, 恽寿榕.凝聚炸药中爆轰波马赫反射的实验研究[J].爆炸与冲击, 1986, 6(3):208-213. http://www.bzycj.cn/article/id/11076Zhang Junxiu, Yun Shourong. Experimental investigations of the Mach relection in a condensed explosive[J]. Explosion and Shock Waves, 1986, 6(3):208-213. http://www.bzycj.cn/article/id/11076 [8] Zhang Xianfeng, Huang Zhengxiang, Qiao Liang. Detonation wave propagation in double-layer cylindrical high explosive charge[J]. Propellants, Explosives, Pyrotechnics, 2011, 36(3):210-218. doi: 10.1002/prep.v36.3 [9] 张先锋, 丁建宝, 赵晓宁.夹层装药作用过程的数字模拟[J].爆炸与冲击, 2009, 29(6):617-624. doi: 10.3321/j.issn:1001-1455.2009.06.011Zhang Xianfeng, Ding Jianbao, Zhao Xiaoning. Numberical simulation of duble layer shaped charge[J]. Explosion and Shock Waves, 2009, 29(6):617-624. doi: 10.3321/j.issn:1001-1455.2009.06.011 [10] Murphy M J, Lee E L, Weston A M. Modeling shock initiation in composition B[C]//Short J M. Proceedings of the 10th International Symposium on Detonationm. Boston: Plenum Publishing Corporation, 1992: 965-970. [11] Grasso F, Paoli R. An analytical study of mach reflection in nonequilibrium steady flows[J]. Physics of Fluids, 1999, 11(10):3150-3167. doi: 10.1063/1.870172 [12] Sternberg H M, Piacesi D. Interaction of oblique detonation waves with iron[J]. Physics of Fluids, 1966, 9(7):1307-1315. doi: 10.1063/1.1761845 [13] Whithan G B. Liner and nonlinear waves[M]. New York:John Wiley & Sons Press, 1974:263-274. [14] Lambourn B D, Wright P W.Mach interaction of two plane detonation waves[C]//Proceeding of the Fourth International Symposium on Detonation. 1965: 142-152. [15] Lee E, Finger M, Collins W. JWL equation of state coefficients for high explosives[R]. Livermore: Lawrence Livermore Laboratory, 1973. [16] Davison L, Horie Y, Shahinpoor M. High-pressure shock compression of solids[M]. Springer Science & Business Media, 2012:123-143. 期刊类型引用(6)
1. 李昱锦,缪玉松,李斌,黄飞飞,葛帅,杨涛,吴佳怡. 对称双线性起爆下爆轰波的轴向传播特性. 爆破器材. 2025(01): 7-13 .  百度学术
百度学术2. 张朝平,张先锋,谈梦婷,侯先苇,熊玮,刘闯,顾晓辉. 聚能杆式射流侵彻混凝土和岩石靶体试验与数值模拟. 含能材料. 2023(08): 773-785 .  百度学术
百度学术3. 李瑞,李伟兵,靳洪忠,王桂林,洪晓文,朱建军,李文彬,王晓鸣. 基于Jones-Wilkins-Lee状态方程的爆轰波相互作用参数理论分析. 兵工学报. 2019(03): 516-521 .  百度学术
百度学术4. 潘建,张建军,侯云辉,冯雪磊. 带隔板装药EFP成型数值模拟与实验研究. 振动与冲击. 2019(18): 89-94+121 .  百度学术
百度学术5. 潘建,张建军,侯云辉,刘燕宁. 带隔板装药爆轰波驱动药型罩数值模拟研究. 弹箭与制导学报. 2019(04): 149-154 .  百度学术
百度学术6. 成乐乐,赵太勇,陈智刚,印立魁,王维占,郭光全,雷文星. 分层装药对杆式射流的影响分析. 爆破器材. 2018(04): 35-39 .  百度学术
百度学术其他类型引用(5)
-








 下载:
下载:













 下载:
下载:











 百度学术
百度学术











