High order spectral volume method for multi-component flows
-
摘要: 针对高维及多物理耦合计算耗费大等困难,设计适合多介质流动模拟的模板紧致、易于并行、高阶精度、计算耗费小的谱体积方法。该方法是求解双曲型守恒率谱体积方法的直接推广,针对多介质流动物质界面捕捉的困难,利用拟守恒格式的思想避免物质界面处的非物理振荡。数值模拟结果表明,本方法具有高阶精度、高分辨率,且节约计算量,并且可以有效避免物质界面处非物理振荡。Abstract: Numerical simulation of multi-material flows has been an important issues in CFD, and most CFD production codes used for multi-material flow simulation is of either first or second order accuracy, too inefficient and costly with its grid refinement for high accuracy required problems. In this paper, a high-order, efficient, compact method, called the spectral volume method, was developed for the simulation of the multi-material flow as an extension of the spectral volume method for the conservation laws. It has been pointed out that the conservative spectral volume method for the multi-material flow will cause oscillation, and the reason for this has been analyzed. So the idea of quasi-conservative scheme was borrowed to prevent the spurious oscillations in the vicinity of a material contact discontinuity. Several numerical experiments proved that there is no oscillation near the material interface and the result also demonstrates the accuracy, the efficiency and the high performance of the scheme for the multi-material flow simulation.
-
Key words:
- fluid mechanics /
- spectral volume method /
- high order /
- stiffened gas /
- multi-component flows /
- compact stencil
-
当前,随着海洋战略地位的日益突出,水下枪炮研究逐渐成为热点。膛口流场现象的复杂性和极高的时空演变特性会对弹丸的飞行产生初始扰动,进而影响到射击精度。因此,中间弹道学的研究受到了广泛关注。不同于空气中发射,水下发射时弹丸及火药燃气受到的水阻力更大,膛口流场现象更复杂。因此,有必要对水下枪炮发射膛口流场发展过程进行深入研究。
针对枪炮空气中发射时的膛口流场已经开展了大量的实验、理论分析和数值模拟研究。为了能够较清楚地认识膛口流场的结构,Steward等[1]、Moumen等[2]和郭则庆等[3]对枪炮膛口流场进行了不同的可视化实验研究。随着计算机和动网格技术的发展,含初始流场和运动弹丸的膛口流场以及弹丸在膛口流场中的受力情况开始受到关注。膛口初始流场对火药燃气流场的发展及弹丸运动有一定的影响,李子杰等[4]基于有限体积法,对有、无初始流场两种条件下的膛口流场进行了数值模拟,分析了初始流场对膛口流场的影响。陈川琳等[5]利用实验和数值模拟相结合的方法分析了弹头在膛口流场中的受力和运动规律。
相较于空气中发射,枪炮水下发射时的情形更为复杂,因此学者们从不同的方面对枪炮水下发射过程进行了研究。水下发射时,燃气从炮口中喷出,在液体中高速扩展形成燃气射流。Harby等[6]、甘晓松等[7]和Xue等[8]对水下燃气射流进行了实验和数值模拟研究,分析了射流气液边界的不稳定性及燃气扩展过程中出现的颈缩、断裂等现象。水下炮密封式发射内弹道特性虽与常规内弹道有许多相似之处,但仍存在一定的差异[9],通过对水下炮内弹道的研究,可以更好地掌握弹丸水下运动规律。超空泡射弹入水后形成超空泡,利用超空泡的水中减阻特性,降低了弹丸衰减速度,实现水中高速航行。易文俊等[10]、施红辉等[11]、刘富强等[12]、黄海龙等[13]及Gao等[14]对水下超空泡射弹特性进行了数值模拟。针对水下膛口流场方面的研究,则主要体现在密封式发射和全淹没式发射方式。张欣尉等[15]、张京辉等[16]分别利用密封式发射和全淹没式发射方式对12.7 mm水下枪在不同水深条件下的膛口流场进行了数值模拟,发现水深与膛口流场特性存在一定的规律性。
前人所研究的重点多为空气中膛口流场、水下燃气射流场及水下枪膛口流场特性,对于水下火炮发射膛口流场特性的研究尚未见报道。本文中,利用30 mm口径火炮研究水下炮密封式发射膛口流场特性及演变规律,通过对火炮在空气中和水下发射时的膛口流场特性进行对比分析,讨论不同介质对火炮膛口流场演化特性的影响规律。
1. 数理模型
1.1 物理模型
水下发射膛口流场是一个较复杂的流场,为了能够对其进行有效的数值模拟,根据火炮水下密封式发射的特点,对所研究模型进行如下假设:
(1)火药颗粒膛内燃烧遵循几何燃烧定律,药粒具有均一的理化性质,形状和尺寸一致,且遵循燃烧速度定律。
(2)弹丸沿x轴正向移动,不考虑重力的影响,将膛口燃气射流与水的相互作用近似看作二维轴对称非稳态过程问题进行处理。
(3)膛口燃气射流视为可压缩理想气体,满足理想气体状态方程,且忽略膛口燃气多组分化学反应的影响;将水视为不可压缩相,密度取 998.2 kg/m3。
(4)因水下密封式发射时,身管内有少量气体,弹丸出膛后不会直接与水接触,且膛口流场作用时间短暂,因此不考虑膛口附近水的空化及相变。
1.2 数学模型[15-16]
本文中,各模型方程式如下。
(1)连续性方程为:
∂(αqρq)∂t+∇⋅(αqρqv)=0 (1) 式中:
q=1,2 分别表示气体相和液体相;ρq 为对应气体相和液体相的密度;αq 为对应气体相和液体相的体积分数,且α1+α2=1 ;t 为时间;v为速度矢量。(2)动量守恒方程为:
∂∂t(ρv)+∇⋅(ρvv)=−∇p+∇⋅[μ(∇v+∇vT)] (2) ρ=n∑q=1αqρq (3) 式中:
ρ 为气液混合密度,且ρ=α1ρ1+(1−α1)ρ2 ;p为流场中的流体压力;μ 为黏度系数。(3)能量守恒方程为:
∂∂t(ρE)+∇⋅[v(ρE+p)]=∇⋅(ke∇T) (4) 式中: E为平均能量,E=(
α1ρ1E1+α2ρ2E2 )/(α1ρ1+α2ρ2 );T为平均温度,T= (α1ρ1T1+α2ρ2T2 )/(α1ρ1+α2ρ2 );ke 为有效热传导率。(4)理想气体状态方程为:
p=ρRT (5) 式中:R为火药燃气常数。
(5)湍流方程:
采用的湍流模型为标准k-
ε 模型,该模型的优点是可以忽略分子黏性的影响,具有较高的稳定性、经济性和计算精度:∂∂t(ρκ)+∂∂xi(ρκui)=∂∂xj[(μ+μtσκ)∂κ∂xj]−ρ¯u′iu′j∂uj∂xi−ρε (6) ∂∂t(ρε)+∂∂xi(ρεui)=∂∂xj[(μ+μtσε)∂ε∂xj]−Cε2ρε2κ+Cε1εκρ¯u′iu′j∂uj∂xi (7) μt=Cμκ2/ε (8) 式中:
κ 为湍流脉动动能;ε 为湍流耗散率;i和j为自由指标,ui 和uj 为速度矢量;¯u′iu′j 为雷诺应力;常数σκ =1.0和σε =1.3分别为湍流脉动动能和湍流耗散率对应的普朗特数;μt 为湍流黏性系数;经验系数Cε1 =1.44,Cε2 =1.92,Cμ =0.08。本文中,数值模拟计算需要耦合以下内弹道方程组[15]。
(1)火药形状函数为:
ψ=χZ(1+λZ+μcZ2) (9) 式中:
ψ 为药室内已燃火药的百分数,χ 、λ 和μc 分别为火药形状特征量,Z 为已燃相对厚度。(2)火药燃速方程为:
dZdt=u1pnae (10) 式中:u1为燃速常数; n为燃速指数,由实验确定;e为药粒半厚度;pa为膛内燃气平均压力。
(3)弹丸运动方程为:
∫A0(pb−ph)dA=φmdvpdt (11) 式中:
pb 和ph 分别为弹丸底部压力和弹丸头部压力,A 为弹丸的横截面积,m 为弹丸的质量,φ 为次要功系数,vp为弹丸速度。(4)内弹道基本方程组为:
Ap(lψ+l)θ=fωψθ−φ2mv2p−∫x0∫A0phdAdl (12) lψ=l0[1−Δρp(1−ψ)−αΔψ] (13) 式中:
l0 为药室容积缩径长;lψ 为药室自由容积缩径长;Δ 为火药装填密度;ω 为火药装药质量;α 为火药气体的余容;ρp 为火药密度;l 为弹丸运动距离;比热比θ=k−1 ,k 为绝热指数;f 为火药力。(5)弹丸速度与行程关系式为:
dldt=vp (14) 将式(9)~(14)构成的内弹道方程组编写用户自定义函数(UDF)和FLUENT程序进行耦合计算。
1.3 数值模拟
数值模拟基于压力的隐式算法求解,多相流采用VOF模型,利用PRESTO!插值格式进行压力项离散,利用压力隐式算子分裂(PISO)算法求解压力-密度的耦合,动量和能量的离散均采用一阶迎风格式,为了保证计算的稳定性,计算过程中时间步长控制在0.1 μs以内。
2. 计算模型及边界条件
2.1 计算模型及网格划分
对膛口流场进行数值模拟时,很难生成单块高质量网格,因此采用网格分区划分进行处理。将整个计算域划分为3个区,即药室Ⅰ区、身管Ⅱ区和膛口流场Ⅲ区。炮膛身管内径为30 mm,弹丸内弹道行程为1.9 m,膛口流场计算域为长1.3 m、半径0.35 m的圆柱形区域。为了更好地捕捉膛口流场波系结构,对膛口流场区采用渐变网格的方式进行了局部加密,膛口附近计算域的网格比较密集,最小网格尺寸为0.5 mm×0.5 mm,流场边界域的网格比较稀疏;在弹头附近采用三角形网格以更好地捕捉其形状,药室区和身管区采用均匀大小的结构网格,计算网格总数为240 000。图1(a)为计算模型示意图,图1(b)为计算网格示意图。
2.2 初始及边界条件
设定药室为压力入口边界条件,身管、膛口为固壁边界条件,弹丸设定为刚体运动,从膛底开始按内弹道方程组计算弹丸运动速度;膛口流场区域外边界为压力出口边界。初始时刻,药室和身管充满气体,膛口外部区域中充满液体介质水,计算初值与环境参数相同,即初始压力为101 325 Pa,初始温度为300 K。
2.3 网格无关性验证
网格无关性验证的目的是验证网格密度变化对计算结果的影响,即通过不断改变网格疏密来观察计算结果的变化,当其波动幅度在允许范围内时,就可以认为计算值与网格无关。
为了保证数值模拟的效率与结果的精确性,对计算网格模型膛口周围流场区域进行了不同尺寸的网格加密,得到了3组不同密度的计算网格数,分别为200 000、240 000及270 000。以膛口到弹底轴向燃气压力分布为参考值,如图2所示,与采用240 000网格数计算时膛口到弹底轴向燃气压力分布相比,采用200 000和270 000网格数进行计算时的平均误差分别为12.5%和4.2%,其波动幅度在允许范围内,因此本文中采用240 000网格数进行数值模拟。
3. 膛口流场数值模拟结果与分析
3.1 数值模型的实验验证
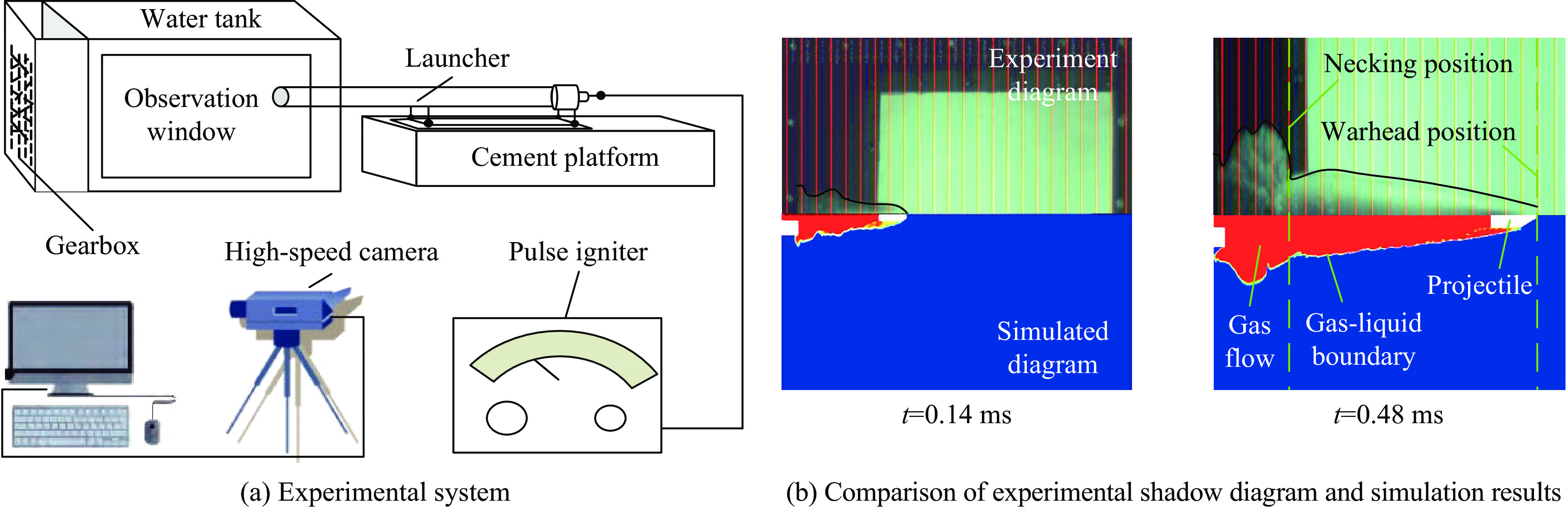
为了研究水下发射膛口流场演变特性并验证数值模型的有效性,通过搭建可视化水下发射实验测试系统,对弹道枪水下密封式发射进行了可视化实验,图3(a)为实验系统。密封式发射时,为了保证身管中充满空气,使用密封膜片将膛口密封,当膛内燃气达到一定压力时膜片打开。实验采用高速摄像机观察和记录多相流场的演化过程,得到了不同时刻的实验阴影图。通过采用与实验相同(弹体质量为45 g、水深0.5 m)的条件工况进行数值模拟,得到相应时刻的模拟相图与实验阴影图对比,如图3(b)所示。图3(b)中上半部分为实验阴影图,下半部分为采用本文中数值模拟方法得到的相图。由图3(b)可知,数值模拟相图中燃气的物质边界扩展尺度和位置与实验阴影图吻合较好。为了进一步说明数值模型的有效性,图4给出了不同时刻射流头部的最大轴向位移对比,由图4可知,数值模拟结果与实验测量结果吻合较好,最大偏差为4.2%。由此可知,利用本文中的数值模型和计算方法进行水下发射膛口流场模拟是可行的。
3.2 计算结果与分析
对30 mm火炮在水下密封式发射时的膛口流场分布进行数值模拟,密封片破膛压力取0.2 MPa,并与在空气中发射时的膛口流场进行比较,将弹丸出膛口瞬间看作t=0时刻。表1为两种发射环境下的部分内弹道及膛口参数,通过内弹道方程组(式(9)~(14))求解所得,表1中
x 为身管长度,pm 为膛内最大压力,v0 为弹丸出膛口时的速度(弹丸初速),p0 为燃气在膛口处的压力,T0 为燃气在膛口处的温度。图5给出了两种发射环境下的燃气射流膛口压力在不同时刻的变化曲线。由表1中可以看出,由于两种发射环境下的膛内阻力基本相同,水下密封式发射时膛内最大压力较空气中发射只升高了7 MPa,而弹丸初速却比空气中发射降低了32 m/s,这是由于密封片使膛口处压力升高和弹丸出膛口时受到水的阻力共同使得弹丸初速降低,此时弹前燃气在炮口处聚集,导致膛口压力和温度显著升高,分别升高了54.8%和10.6%。由图5可知,弹丸出膛口后,两种发射环境下的燃气射流膛口压力均随时间呈衰减趋势,在50 μs内压力衰减迅速,然而由于水对燃气扩展的阻碍较大,气体在水中的膨胀速度比在空气中慢,燃气聚集使得炮口气体压力始终较高。表 1 内弹道及膛口参数Table 1. Interior ballistics and muzzle parameters发射环境 x/m v0/(m·s−1) pm/MPa p0/MPa T0/K 空气中 1.94 985 317 62 2 152 水下 1.94 953 324 96 2 380 为了研究膛口压力场的演变特性,图6、图7分别给出了两种环境下不同时刻的压力分布和纹影图,上半部为压力云图,下半部为纹影图。图8给出了200 μs时刻燃气压力沿轴向的分布曲线。弹丸出膛口后,高温高压的火药燃气迅速喷出扩展,当射流滞止压力与环境压力之比大于3~4时, 流场结构中会出现瓶状正激波结构, 称为马赫盘。由图6可知,当弹丸运动30 μs时,燃气还未追上弹丸,炮口处燃气呈球状扩展。当弹丸运动70 μs时,火药燃气轴向迅速膨胀且已经包围弹丸;弹丸运动240 μs时,弹丸追赶初始冲击波,初始冲击波是弹前激波在膛口外绕射形成的球形冲击波,火药燃气扩展受冲击波影响压力升高;随着弹丸运动350 μs,弹丸已完全摆脱火药燃气的包围,形成完整的膛口流场。由图7可以发现,水下发射膛口压力场与空气中有所不同。弹丸运动30 μs时,火药燃气主要向弹丸侧前方(径向)膨胀且激波核心区较小,这是由于燃气同时受到弹丸和水的阻力,扩展不够充分。随着弹丸在水下不断运动,燃气逐渐由径向转为轴向膨胀,膛口处的燃气压力衰减比空气中更迅速。在水下运动过程中,由于高密度的水,弹丸头部产生的压力远高于膛口核心区的燃气压力,在空气运动过程中,受初始燃气流场的影响,被压缩的低密度空气在弹丸头部产生的压力极低,尽管弹丸被燃气包围后弹前压力有所升高,但仍远低于膛口核心区的燃气压力,从纹影图中可以更加清晰地看出两种介质中的流场波系结构。结合图8可以看出,在200 μs时,水下发射时燃气压力先沿轴向快速下降,穿越马赫盘后有较大幅度的上升,然后波动变化。由于此时空气中发射时马赫盘尚未形成,燃气压力迅速下降,之后基本保持不变。可见,介质密度的巨大差异导致膛口压力场的时空分布存在显著差别。
为进一步了解水下发射膛口燃气压力变化,图9给出了水下不同时刻膛口的轴向压力分布曲线,由图9可知,70 μs时,由于燃气速度大于弹丸速度而形成的弹底激波所致,燃气压力会有突跃,此时马赫盘尚未形成;140 μs时,马赫盘开始形成,燃气压力上升幅度最大;随着弹丸不断运动,燃气压力波动逐渐减小,趋于平缓。由此可见,膛口激波结构是一个生长-衰减-稳定的过程。
弹丸出膛口时,膛内燃气压力远高于外部环境压力,属于高度欠膨胀射流。为了更直观地了解膛口燃气高度欠膨胀射流的结构特征,图10给出了空气中发射和水下密封式发射时膛口燃气射流结构流谱图[17],并给出了气液边界线。其中A区为核心激波自由膨胀区,火药燃气主要在该区域内膨胀,压力剧降,速度激增,该区域为超音速气流,Ma>1。B区为相交激波与反射边界之间的超音速区域。大部分燃气在扩展过程中穿过马赫盘进入亚声速区C,该区域燃气经过马赫盘后聚集,压力陡增,速度降为亚声速,Ma<1。有少部分的燃气经过两次斜激波后(入射激波和反射激波)进入D区,D区的燃气压力虽与C区相同,但由于经过两次不同的压缩过程使得速度增高,为超音速气流。由图10可以看出,空气中发射时膛口马赫盘完全形成后呈圆弧状结构,而水下发射时马赫盘结构呈梯形状。由于火炮在水下发射时,燃气在扩展过程中受到高密度水(约为空气密度的800倍)的挤压,射流前端高压区的存在使气体产生回流现象,该回流对射流主通道具有剪切作用,挤压与回流导致气流在垂直于炮口轴线方向上产生不稳定性,使得燃气射流扩展过程中出现颈缩现象,激波核心区受颈缩作用,马赫盘形状结构呈梯形状,导致空气中发射和水中发射时的马赫盘结构不同。
为了更好地了解马赫盘的形成过程及特性,图11~12分别给出了水下发射和空气中发射时不同时刻的马赫数分布和纹影图,其中上半部为马赫数云图,下半部为纹影图。图13给出了70与200 μs时刻马赫数沿轴线的分布曲线。由图11可以看出,70 μs时,由于受到弹丸和水的阻力作用,燃气主要为径向膨胀,炮口两侧马赫数较高。随着燃气的喷射和膨胀,气体射流形成主轴激波结构,直到140 μs时马赫盘初步生成。随着弹丸不断运动,燃气射流充分发展,激波面积增大,马赫盘向垂直轴线方向变化,直径逐渐增大,在240 μs时,入射激波、反射激波及马赫盘在接触面交汇于一点,形成三波点结构。由图12可知,70 μs时,火药燃气流场逐步吞没初始流场,在炮口后形成球状激波结构;随着弹丸运动和燃气不断喷出,在320 μs时马赫盘开始初步生成,三波点结构也已形成。当弹丸运动到480 μs,激波结构完全生成,马赫盘呈碗状结构。由图13(a)可知,两种环境发射时马赫数均先沿轴线增大后减小,由图13(b)可知,200 μs、水下发射时,马赫数沿轴线增大后呈断崖式衰减,结合图10的膛口流场流谱图可知,燃气在穿越马赫盘后进入亚声速区,速度骤降,与水下发射不同,空气中发射时的马赫盘还未形成。
对比可知:水下发射时膛口附近会有气液夹带,而空气中发射时低密度的空气对射流尾翼没有大的影响;水下发射时火药燃气射流受气液界面的相互作用影响,更快形成马赫盘结构,而在空气中,火药燃气膨胀过程中受阻较小,燃气射流较长时间与弹底作用形成弹底激波,阻碍马赫盘的形成;水下发射时的激波核心区面积明显小于空气中发射时的激波核心区面积,且弹丸头部不存在冠状冲击波。
两种环境下的马赫盘轴向位移随时间变化曲线如图14所示,为了直观地看出马赫盘距离膛口位置随时间变化的规律,经过计算得出,马赫盘距离膛口位置随时间变化呈指数增长,拟合公式为:
x(t)=x0+x1e−t/t1 (15) 式中:
x(t) 为马赫盘距膛口位移(mm),膛口为坐标原点;x0 为初始系数,x0=113mm ;x1 为增速系数,x1=−80mm ;t1 为时间增长因子,t1=180 。而空气中发射时马赫盘距离膛口位置随时间的变化呈线性增长,拟合公式为:
x(t)=x′0+x′1t (16) 式中:
x(t) 为马赫盘距膛口位移(mm);膛口为坐标原点;x′0=152.2mm ;x′1 为线性增长因子,x′1= 0.35m/s 。为了进一步研究不同介质中的弹丸速度衰减规律,图15给出了两种介质中弹丸速度随时间变化的曲线,弹头出膛口记为零时刻,从图15中可以看出,水下发射时,当弹头与水接触后,弹丸速度开始迅速衰减,直到弹丸全部出膛后一直呈线性衰减,而在空气中发射时,弹丸刚飞出膛口后,弹丸在火药燃气作用下先加速运动,当弹丸摆脱燃气流作用后,在空气阻力作用下,弹丸速度又开始缓慢衰减。
4. 结 论
利用30 mm火炮建立了水下密封式发射数值模型,模拟了火炮水下发射时的膛口流场演变过程,通过对火炮在空气中和水下发射时的膛口流场特性进行对比分析,发现两种不同介质环境下的膛口流场特性存在较大的不同。
(1)水下密封式发射时,弹丸在膛内所受的阻力与空气中发射时基本相同,水下发射时的膛内最大压力只比空气中发射时高7 MPa,弹丸出膛口时受到水的阻力较大,弹丸初速比空气中发射时降低了32 m/s,弹丸初速的降低使得膛口压力和温度比空气中发射时分别升高54.8%和10.6%。
(2)弹丸出膛口后,两种发射环境下的燃气射流膛口压力均随时间呈衰减趋势,水下发射时燃气膨胀受水的阻碍,燃气压力始终高于空气中发射;弹丸入水后,弹丸头部产生的压力远高于膛口核心区的燃气压力,而弹丸在空气中飞行时,弹丸头部产生的压力却远低于膛口核心区的燃气压力。
(3)水下密封式发射时,膛口附近会有气液夹带,而空气中发射时,低密度的空气对射流尾翼没有较大的影响;火药燃气射流受气液界面的相互作用影响,在140 μs时初步形成马赫盘结构,而空气中发射时马赫盘结构形成较晚,约在320 μs时形成;水下发射时的激波核心区面积明显小于空气中发射时的激波核心区面积。水下密封式发射时,马赫盘距离膛口轴向位移随时间变化呈指数增长;而空气中发射时,马赫盘距离膛口位置随时间变化呈线性增长。
本文中在计算和分析时暂未考虑弹体高速运动在水中的冲击波效应及空穴效应,在后续的工作中将会进一步研究冲击波效应及空穴效应对膛口流场演化过程的影响规律。
-
表 1 格式的数值精度
Table 1. Numerical accuracy of present schemes
N Lerr1 order Lerr1 order Lerr1 order Lerr1 order k=2 k=3 k=4 k=4 10 4.80×10-3 - 3.91×10-4 - 7.22×10-6 - 4.69×10-7 - 20 1.17×10-4 2.04 6.77×10-5 2.53 4.34×10-7 4.06 1.93×10-8 4.60 40 2.93×10-5 2.00 9.64×10-6 2.81 2.47×10-8 4.14 6.79×10-10 4.83 80 7.34×10-5 2.00 1.26×10-6 2.94 1.56×10-9 3.98 2.21×10-11 4.94 160 1.84×10-5 2.00 1.59×10-7 2.99 9.77×10-11 4.00 6.85×10-13 5.01 -
[1] Abgrall R. How to prevent pressure oscillations in multicomponent flow calculation: A quasi conservative approach[J]. Journal of Computational Physics, 1996, 125(1):150-160. doi: 10.1006/jcph.1996.0085 [2] Abgrall R, Karni S. Computations of compressible multifluids[J]. Journal of Computational Physics, 2001, 169(2):594-623. doi: 10.1006/jcph.2000.6685 [3] Shyue K M. An effcient shock-capturing algorithm for compressible multicomponent problems[J]. Journal of Computational Physics, 1998, 142(1):208-242. http://cn.bing.com/academic/profile?id=0353bed7b41acec082faa9e0749fd595&encoded=0&v=paper_preview&mkt=zh-cn [4] Shyue K M. A fluid-mixture type algorithm for compressible multicomponent flow with Mie-Gruneisen equation of state[J]. Journal of Computational Physics, 2001, 171(2):678-707. doi: 10.1006/jcph.2001.6801 [5] Chen Y B, Jiang S. A non-oscillatory kinetic scheme for multicomponent flows with the equation of state for a stiffned gas[J]. Journal of Computational Mathematics, 2011, 29(6):661-683. doi: 10.4208/jcm [6] Johnsen E, Colonius T. Implementation of WENO schemes in compressible multicomponent flow problems[J]. Journal of Computational Physics, 2006, 219(2):715-732. http://www.sciencedirect.com/science/article/pii/S0021999106002014 [7] Zhu J, Qiu J X, Liu T G, et al. RKDG methods with WENO type limiters and conservative interfacial procedure for one-dimensional compressible multi-medium flow simulations[J]. Applied Numerical Mathematics, 2011, 61(4):554-580. doi: 10.1016/j.apnum.2010.12.002 [8] Wang Z J. Spectral (finite) volume method for conservation laws on unstructured grids: Basic formulation[J]. Journal of Computational Physics, 2002, 178(1):210-251. doi: 10.1006/jcph.2002.7041 [9] Wang Z J, Liu Y. Spectral (finite) volume method for conservation laws on unstructured grids Ⅲ: One dimensional systems and partition optimization[J]. Journal of Scientific Computing, 2004, 20(1):137-157. http://www.sciencedirect.com/science/article/pii/S0021999103005035 [10] Karni S. Multicomponent flow calculations by a consistent primitive algorithm[J]. Journal of Computational Physics, 1994, 112(1):31-43. http://dl.acm.org/citation.cfm?id=182760 期刊类型引用(7)
1. 胡可镇,卓长飞,纪元,蒋显松. 武装直升机机载空地导弹发射过程燃气冲击效应研究. 兵工自动化. 2025(01): 82-89 .  百度学术
百度学术2. 康越,马天,王俊龙,张逸之,张文博,韩笑,栗志杰. 不同海拔高度炮口冲击波动态演化特性数值模拟研究. 爆炸与冲击. 2024(12): 57-73 .  本站查看
本站查看3. 柏雯斌,余永刚,张欣尉. 水下火炮气幕式发射膛口流场演化特性的数值分析. 兵工学报. 2024(12): 4383-4394 .  百度学术
百度学术4. 纪元,卓长飞,孙皓天,蒋显松,蔡雨娇. 极端载弹下直升机载火箭发射燃气冲击效应研究. 兵工自动化. 2023(02): 75-82 .  百度学术
百度学术5. 廖国柔,马国鹭,张浩,陈万华,宗建宇,李中杨. 一级气体炮制退器的流场分析及其效率测试. 高压物理学报. 2023(02): 24-31 .  百度学术
百度学术6. 刘越,赵子杰,戴琪,王安华,张辉. 航行体水下高膛压与高速发射载荷特性. 兵工学报. 2023(04): 1126-1138 .  百度学术
百度学术7. 王雨舒,吕续舰. 水下枪炮发射问题研究综述. 装备环境工程. 2022(05): 1-13 .  百度学术
百度学术其他类型引用(4)
-









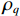





















































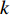



 下载:
下载:






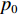



























 下载:
下载:




 百度学术
百度学术