运用极限荷载法研究钢筋混凝土板低速侵彻效应

王德荣; 苏杭; 程怡豪; 冯淑芳

doi:10.11883/1001-1455(2017)05-0837-07

运用极限荷载法研究钢筋混凝土板低速侵彻效应

doi: 10.11883/1001-1455(2017)05-0837-07

1.
中国人民解放军陆军工程大学爆炸冲击防灾减灾国家重点实验室，江苏南京 210007
2.
北部战区陆军第二工程科研设计所，辽宁沈阳 110162

基金项目:

国家自然科学基金项目 51409258

长江学者与创新团队发展计划项目 IRT13071

详细信息

作者简介:
王德荣(1968—)，男，博士，副教授, wdrjb@163.com

中图分类号: O347
计量
- 文章访问数: 4484
- HTML全文浏览量: 1349
- PDF下载量: 252
- 被引次数: 1
出版历程
- 收稿日期: 2016-01-11
- 修回日期: 2016-08-08
- 刊出日期: 2017-09-25

Response of reinforced concrete slabs to low-velocity projectile impact investigated using upper bound method

1.
State Key Laboratory of Disaster Prevention and Mitigation of Explosive and Impact, The Army Engineering University of PLA, Nanjing 210007, Jiangsu, China
2.
The Second Institute of Engineering Research and Design, Northern Theater Army, Shenyang 110162, Liaoning, China

摘要

摘要: 基于不可压缩刚塑性材料模型和滑移线场理论，获得了单一容许速度场条件下刚性弹低速侵彻半无限介质的阻力函数。在此基础上，基于多速度容许场得到了刚性弹侵彻有限厚度靶的三阶段阻力曲线，并提出了震塌与贯穿的临界条件，通过与实验结果、UMIST公式及古比雪夫的对比，验证了本文方法在钢筋混凝土板低速撞击问题中的适用性,分析了弹头形状、冲击因子和钢筋阻力系数等参数对临界震塌(贯穿)厚度的影响。
- 刚塑性极限分析 /
- 低速侵彻 /
- 混凝土 /
- 震塌 /
- 贯穿
Abstract: Based on the incompressible-rigid-plastic material assumption and the slip line field theory, the resistance function of a rigid projectile penetrating a semi-infinite target at a low velocity was obtained with a single admissible velocity field. A three-stage resistance curve of a rigid projectile impacting on a thin target was analyzed under multiple velocity fields, where the critical conditions for scabbing or perforation were calculated. The methods and formulae for local effects on reinforced concrete slab under low-velocity impact were further verified using comparative analysis of the results from the experiments, the UMIST formulae, the Kuibyshev formulae, and the present paper's calculations. The relationships between the normalized critical scabbing/perforation thickness, and the nose-shape factor, the impact factor and the reinforcement factor were examined to present potential guide to experimental studies.
- rigid-plasticity limit analysis /
- low velocity penetration /
- concrete /
- scabbing /
- perforation

HTML全文

研究撞击和侵彻问题时，需要确定物体间的相互作用力，其中极限荷载法是研究低速侵彻阻力的重要方法。R.Hill^[1]、M.Ravid等^[2]、A.Amini等^[3]、J.Tirosh等^[4]、陈士林等^[5]、王明洋等^[6]、冯淑芳等^[7]、咸玉席等^[8]均开展过这方面的研究，利用刚塑性不可压缩介质模型，用基于机动容许速度场的极限分析法的上限定理求解了弹体低速侵彻的阻力上限。一般认为低速撞击条件下结构整体响应的贡献显著，但Q.M.Li等^[9]的研究显示，当结构厚度为冲击体直径2倍以上时仍以局部效应为主。

本文中，主要考虑平头弹和锥形头弹两种弹头形式，假设混凝土介质满足刚塑性、不可压缩性和弹靶间无摩擦假定，将极限震塌(贯穿)厚度计算公式进行简化，通过与Y.S.Tai^[10]和A.N.Dancygier等^[11-12]实验结果及UMIST公式、古比雪夫公式的对比和参数分析来验证公式的可靠性，分析冲击因子、钢筋阻力系数和弹头形状之间的关系。

1. 低速撞击条件下半无限介质阻力的上限解

先考虑锥角2β、直径2a的刚性弹体以单位初速度v₀侵彻半无限靶的情形。弹体头部尚未完全没入靶体的情形，已经由R.Hill^[1]和L.M.卡恰诺夫^[13]进行了分析，这里主要考虑弹头已经完全没入靶体之后的过程。

如图 1所示，BB′=2a=d，∠BAB′=2β，弹体变截面点至靶体初始自由面的距离为y。假定的速度场如下：(1)刚体ABC以速度v₁沿着AC运动，且令∠BAC=π/4，∠ACB=γ；运动至BC后，改变方向沿着弧CD运动，而v₁在B处分解为v₂和v₃。(2)在圆心角为β而半径为z的扇形CBD中，点的运动轨迹沿着弧CD且都具有速度v₃；运动至BD后，刚体BDEF则以速度v₄沿着DE运动，且BD⊥DE，v₃=v₄，∠DEG=δ。ABC和BDEF均为刚性，因而能量全部耗散在各区域的相互错动和扇形CBD的内变形上，据此得到上限阻力：

图 1 锥形头弹侵彻半无限靶的容许速度场

Figure 1. Admissible velocity field of semi-infinite target under penetration of cone-nosed projectile

下载: 全尺寸图片幻灯片

$p_{\rm{i}}^{{\rm{up}}} \cdot {v_0} \cdot a = {\tau _{\rm{s}}}\left( {{v_1} \cdot AC + {v_2} \cdot BC + {v_3} \cdot CD + {v_3} \cdot \beta \cdot BC + {v_4} \cdot ED} \right)$

(1)

式中：p_i^up为作用在弹体截面上的阻力上限，τ_s为满足Mises准则的剪切应力。利用各速度场之间的几何关系，并令A=a/2sinβ，z=A/sinγ，可以得到：

${v_1} = \sqrt 2 {v_0}{\rm{sin}}\beta , {\rm{ }}\;\;\;\;\;\;\;{v_2} = {v_1}{\rm{cos}}\gamma , {\rm{ }}\;\;\;\;\;\;{v_3} = {v_4} = {v_1}{\rm{sin}}\gamma$

(2)

$\begin{array}{l} {\rm{ }}BC = BD = z, {\rm{ }}AC = \sqrt {{z^2} - {A^2}} + A, {\rm{ }}CD = z\beta , {\rm{ }}\\ ED = z\frac{{A - \sqrt {{z^2} - {A^2}} }}{{A + \sqrt {{z^2} - {A^2}} }} + y\frac{{\sqrt 2 z}}{{A + \sqrt {{z^2} - {A^2}} }} \end{array}$

(3)

将式(2)~(3)代入式(1)，得：

$\frac{{p_{\rm{i}}^{{\rm{up}}}a}}{{{\tau _{\rm{s}}}\sqrt 2 {\rm{sin}}\beta }} = 2\sqrt {{z^2} - {A^2}} + A + 2A\beta + A\frac{{A - \sqrt {{z^2} - {A^2}} }}{{A + \sqrt {{z^2} + {A^2}} }} + \sqrt 2 A\frac{y}{{A + \sqrt {{z^2} + {A^2}} }}$

(4)

将式(4)对z求极值，得p_i^up的极小值：

$\frac{{p_{\rm{i}}^{{\rm{up}}}}}{{{\tau _{\rm{s}}}}} = 2\beta - 2 + 4\sqrt {1 + \frac{y}{a}\;\frac{1}{{{\rm{sin}}\beta }}} = 2\beta - 2 + 4\sqrt {1 + \left( {\frac{h}{a} - \frac{1}{{{\rm{tan}}\beta }}} \right){\rm{sin}}\beta }$

(5)

当顶角为90°(β=π/4)，此时：

$\begin{array}{l} h = y + a, {\rm{ }}\\ \frac{{p_{\rm{i}}^{{\rm{up}}}}}{{{\tau _{\rm{s}}}}} = \frac{{\rm{ \mathsf{ π} }}}{2} - 2 + 4\sqrt {1 + \sqrt 2 \frac{y}{a}} \end{array}$

(6a)

咸玉席等^[8]根据实验结果，假定平头弹侵彻过程中弹头前部存在一个顶角90°的刚性死区，从而将平头弹的侵彻问题转化为顶角90°的锥形弹侵彻问题，其中静阻力项表达式(忽略重力)为：

$\frac{{p_{\rm{i}}^{{\rm{up}}}}}{{{\tau _{\rm{s}}}}} = \left( {\frac{3}{2}{\rm{ \mathsf{ π} }} + 1} \right) + \frac{{\tau _{\rm{s}}^{\rm{e}}}}{{{\tau _{\rm{s}}}}}\;\frac{y}{{\sqrt 2 a}}$

(6b)

式中：τ_s^e为破碎混凝土的残余抗剪强度。由于对速度场分布的假定存在差异，因此式(6a)和式(6b)形式不同。若假定τ_s^e=τ_s并在y/a=0~30的条件下，比较式(6a)和式(6b)的数值变化(见图 2)，则可发现当1.6 < y/a < 26时，阻力上限随着侵彻深度增加而增加，式(6a)稍大于式(6b)但差值不超过后者的20%，此时速度场假定带来的差异对结果的影响在数值上很小。

图 2 平头弹条件下阻力上限

Figure 2. Upper bound of penetration resistance for flat-nosed projectile

下载: 全尺寸图片幻灯片

2. 低速撞击条件下混凝土板的临界震塌和贯穿条件

采用与构造半无限靶速度场相似的方法，冯淑芳等^[7]利用构造容许速度场的方法，得到不同条件下的阻力上限曲线(见图 3)，曲线1、2、3分别对应无限厚度、有限厚度第一速度场和有限厚度第二速度场。点A₁处弹体同时满足半无限靶和震塌条件下的速度场；点B₁处弹体同时满足震塌和贯穿条件下的速度场。由于各个速度场所对应的阻力均为真实阻力的上限值，可在各个交点所划分的区域内的阻力上限值通过比较，取到O→A₁→B₁→F₁(见图 3中实线)。沿着O→A₁→B₁→F₁，即表征了侵彻阻力上限随着侵彻深度变化和背部自由面参与侵彻过程而变化的过程。

图 3 不同条件下侵彻阻力上限曲线

Figure 3. Upper bound of penetration resistance curves under different conditions

下载: 全尺寸图片幻灯片

对于平头弹，在板厚和弹体直径之比满足一定条件时，则会出现图 3(b)中的情形，即曲线1整个位于曲线2和3上面，使A₁实际上不存在，侵彻阻力曲线为O→B₁→F₁，这意味着开始时靶体背部的自由面效应(震塌)就影响着侵彻过程，直至在B₁贯穿发生。计算表明，这个现象发生的条件为^[14]：L/2a≤1.82，其中L为靶板厚度。结合文献[7]，进一步得到有限厚度板在平头和锥形两种弹头局部冲击下的临界震塌与贯穿厚度计算公式。

对平头弹，有：

$\left\{ \begin{array}{l} \frac{{{h_{\rm{s}}}}}{{2a}} = \frac{{{h_{\rm{p}}}}}{{2a}} = - \frac{{0.24\mu + 0.5}}{{.48\mu + 1.6}}0 + {\frac{{\left[ {I + {{\left( {0.24\mu + 2.3} \right)}^2}} \right]}}{{0.48\mu + 1.6}}^{1/2}}\;\;\;\;\;\;\;\frac{L}{{2a}}<1.82\\ \frac{{{h_{\rm{s}}}}}{{2a}} = \frac{{{{\left( {I + 15.3} \right)}^{1/2}} - 0.12\mu - 2.5}}{{0.24\mu + 0.78}}, \;\frac{{{h_{\rm{p}}}}}{{2a}}\; = \frac{{{{\left( {I + 15.3} \right)}^{1/2}} - 2.4}}{{0.41\mu + 1.34}}\;\;\;\;\;\frac{L}{{2a}} \ge 1.82 \end{array} \right.{\rm{ }}$

(7)

对锥形弹，有：

$\frac{{{h_{\rm{s}}}}}{{2a}} = \frac{{{{\left( {I + 2.4} \right)}^{1/2}} - 0.23\mu - 1.57}}{{0.45\mu + 0.4}}, \;\;\;\;\;\;\;{\rm{ }}\frac{{{h_{\rm{p}}}}}{{2a}} = \frac{{{{\left( {I + 2.4} \right)}^{1/2}} - 1.25}}{{1.2\mu + 1}}$

(8)

式中：h_s和h_p分别为临界震塌厚度和临界贯穿厚度，I为冲击因子，μ为量纲一的钢筋抗力系数。I和μ的表达式分别为：

$I = \frac{{{M_{\rm{p}}}v_0^2}}{{{\tau _{\rm{s}}}{{\left( {2a} \right)}^3}}}, \;\;\;\;\;\;\;\;{\rm{ }}\mu = \frac{{{f_{\rm{s}}}{A_{\rm{s}}}}}{{{\tau _{\rm{s}}}s\left( {2a} \right)}}$

式中：M_p为弹体质量，f_s为钢筋的抗拉强度，A_s为直径d_r的钢筋截面积，s为平行钢筋的轴线间距，τ_s为混凝土的抗剪强度。

本文计算结果与文献[10-12]的比较见表 1~3。由于震塌的临界状态很难判断，这里只比较临界贯穿速度，即弹道极限v_BL。在文献[10]中：M_p= 0.3 kg，2a=25 mm，L=50 mm。在普通混凝土侵彻实验^[11]中：M_p= 120 g，2a=25 mm，β=20°，采用两种厚度的混凝土靶(L=5 cm和L=6 cm)。在高性能混凝土侵彻实验^[12]中：M_p=1.5 kg，2a=25 mm，β=20°，L=0.2 m。其他参数(混凝土轴压强度f_c、混凝土抗拉强度f_t、混凝土抗剪强度τ_s、钢筋抗拉强度f_s、配筋情况、与弹道极限对应的冲击因子I_BL、钢筋抗力系数μ等)和v_BL的实验结果和预测结果见表 1~3。

表 1 本文计算结果与实验^[10]的比较

Table 1. Comparison between experimental results^[10] and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	实验	本文计算
T-1	2	25.0	2.6	4.0	10.5	27.0~35.7	47
T-2	2	25.2	3.1	4.4	10.5	41.7~56.8	49
T-3	2	161.9	7.3	17.2	10.5	34.7~58.5	97
T-4	2	175.3	13.8	24.6	10.5	76.0~104.0	116

下载: 导出CSV

| 显示表格

表 2 本文计算结果与普通混凝土实验^[11]的比较

Table 2. Comparison between experimental results ^[11] of normal strength concrete and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	φ/mm	@/mm	f_s/MPa	μ	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	配筋		f_s/MPa	μ	实验	本文计算
D-1-1	2.0	35.0	3.0	5.12	15.9	2.5	34	382	0.43	165	103
D-1-2	2.0	35.0	3.0	5.12	15.4	3.0	25	183	0.40	222	101
D-1-3	2.4	35.0	3.0	5.12	20.8	3.0	25	183	0.40	232	117
D-1-4	2.4	35.0	3.0	5.12	127.1	5.0	27	473	2.68	236	291
D-1-5	2.0	34.0	3.4	5.38	53.6	3.25	21	600	1.76	210	193
D-1-6	2.0	34.0	3.4	5.38	34.8	2.5	20	650	1.19	162	156

下载: 导出CSV

| 显示表格

表 3 本文计算结果与高性能混凝土实验^[12]的比较

Table 3. Comparison between experimental results^[12] of high performance concrete and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	φ/mm	@/mm	f_s/MPa	μ	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	配筋		f_s/MPa	μ	实验	本文计算
D-2-1	4	40.0	4.0	6.3	66.5	8	100	400	0.64	204~245	187
D-2-2	4	108.0	10.8	17.1	38.3	8	100	400	0.24	273~276	233
D-2-3	4	102.0	10.2	16.1	39.1	8	100	400	0.25	281~289	229
D-2-4	4	104.0	10.4	16.4	38.9	8	100	400	0.24	287~291	231
D-2-5	4	113.0	11.3	17.9	37.7	8	100	400	0.22	262~289	237
D-2-6	4	106.0	10.6	16.8	38.6	8	100	400	0.24	291~307	232
D-2-7	4	101.0	10.1	16.0	39.3	8	100	400	0.25	286~292	229
D-2-8	4	93.0	9.3	14.7	40.7	8	100	400	0.27	292~313	223
D-2-9	4	94.0	9.4	14.9	40.5	8	100	400	0.27	313~314	224
D-2-10	4	102.0	10.2	16.1	39.2	8	100	400	0.25	287~289	229
D-2-11	4	103.0	10.3	16.3	39.0	8	100	400	0.25	287~292	230

下载: 导出CSV

| 显示表格

低速撞击下混凝土介质中静水压水平较低，因此将τ_s近似地取为Mohr-Coulomb准则下的黏聚力c，并采用以下公式^[15]：

${\tau _{\rm{s}}} \approx c = \frac{{\sqrt {{f_{\rm{c}}}{f_{\rm{t}}}} }}{2}$

(9)

对于未给出f_t的情况，将f_t近似取为0.1f_c。从表 1~3可见，本文中对T1~4和D-1-3~4的预测较准确，对D-2-1~8的弹道极限的预测稍有低估，但误差在20%之内，误差较大的情况主要体现在D-1-1~3，这可能与钢筋作用机理的复杂性有关。

为了进一步评价本文计算方法的预测效果，图 4还给出了式(7)~(8)与UMIST公式^[16]和古比雪夫公式^[7]关于临界震塌\贯穿速度的对比结果。其中f_c =25 MPa，M_p=500 kg，2a=400 mm。通过比较发现：(1)对于素混凝土而言(图 4(a)~(b))，本文中对临界速度的预测总体上稍高于UMIST公式和古比雪夫公式，而古比雪夫公式稍高于UMIST公式；(2)当考虑钢筋((图 4(c)~(d))时，配布f_s =300 MPa的Ø20 mm@100 mm钢筋)，本文的预测数值要显著高于UMIST公式。这在很大程度上归因于，本文中建议的钢筋阻力系数μ既考虑了f_s的影响又考虑了d_r和s的影响，而UMIST公式在形式上只考虑了d_r和s的影响。

图 4 本文公式与UMIST公式、古比雪夫公式的比较

Figure 4. Comparison between UMIST formulae, Kuibyshev formulae and this paper's formule

下载: 全尺寸图片幻灯片

综上，对于素混凝土而言，式(7)~(8)和实验结果及经典公式的吻合程度较高；当考虑钢筋时，式(7)~(8)与实验结果及UMIST公式的吻合程度下降。由于钢筋混凝土材料的复杂性，钢筋阻力的作用模式可能不是单一的，它对侵彻阻力的贡献仍需要开展更多研究。

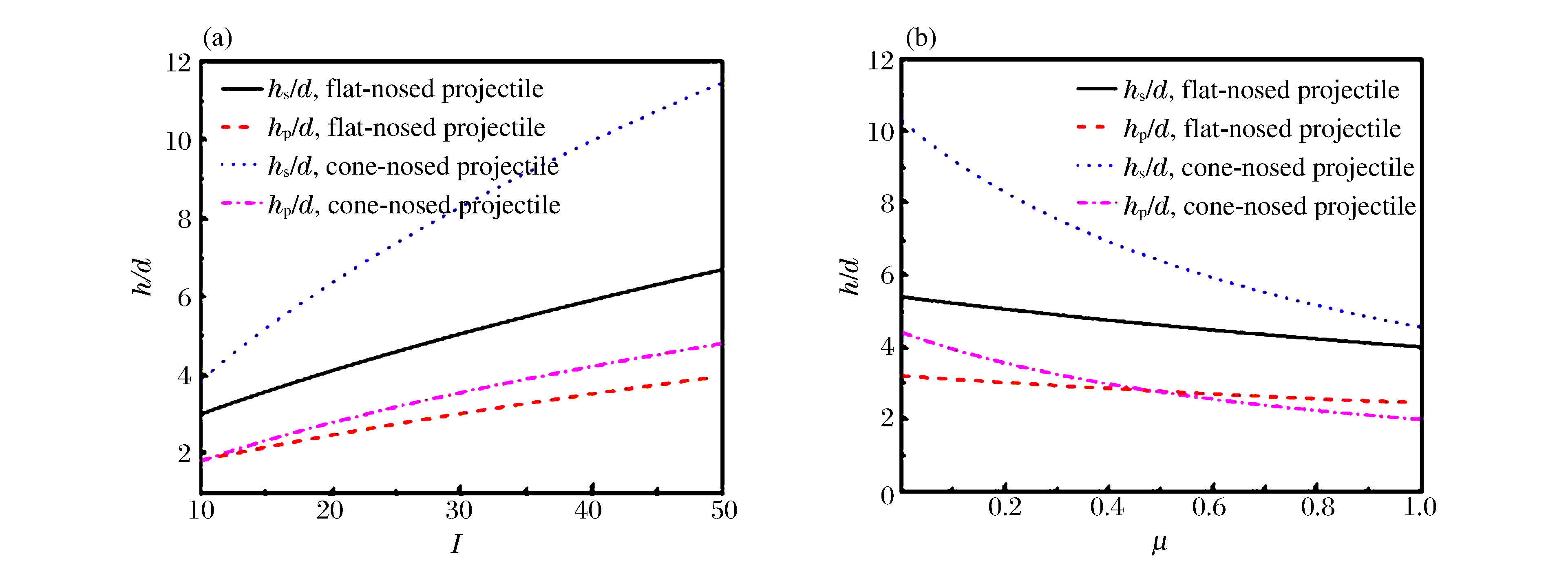
为了进一步观察因子I和μ的影响，分别绘制两种弹头形式下h_s/2a和h_p/2a相对I和μ的参数曲线。在图 5(a)中，μ=0.2，I=10~50；在图 5(b)中，I=30，μ=0~1.0。结果显示：(1)在上述参数范围内，h_s/2a和h_p/2a分别伴随着I和μ的增加而单调递增和单调减小；(2)在相同参数条件下，锥形弹的h_s/2a和h_p/2a大体高于平头弹，但h_p/2a的差值较小；(3)当冲击因子较小而钢筋阻力系数较大时，还可能出现锥形弹的临界贯穿厚度小于平头弹的情况，这和平头弹条件下的动力冲塞规律基本符合。可见，式(7)~(8)基本正确地反映了薄板低速侵彻下的主要规律，也把握了控制震塌和贯穿的关键因素。

图 5 临界震塌和贯穿厚度随冲击因子与钢筋阻力系数的变化

Figure 5. Normalized critical scabbing or perforation thickness versus impact factor and reinforcement factor

下载: 全尺寸图片幻灯片

3. 结论

利用极限分析理论和滑移线场理论，获得了锥形头弹和平头弹低速侵彻混凝土靶和钢筋混凝土靶的阻力上限计算方法。结果表明，低速撞击条件下混凝土板的归一化临界震塌与贯穿厚度可以归结为冲击因子、钢筋抗力系数和弹头形状等3个量纲一参数的函数。当不考虑钢筋时，理论方法与实验结果、UMIST公式、古比雪夫公式的结果吻合较好；当考虑钢筋时，吻合程度下降，这可能与侵彻过程中弹体-混凝土-钢筋三者相互作用的复杂性有关。但从参数分析的结果看，本文方法基本正确地归纳了控制震塌和贯穿机制的量纲一参数，可以为进一步的实验研究提供参考。

图 1 锥形头弹侵彻半无限靶的容许速度场

Figure 1. Admissible velocity field of semi-infinite target under penetration of cone-nosed projectile

下载: 全尺寸图片幻灯片

图 2 平头弹条件下阻力上限

Figure 2. Upper bound of penetration resistance for flat-nosed projectile

下载: 全尺寸图片幻灯片

图 3 不同条件下侵彻阻力上限曲线

Figure 3. Upper bound of penetration resistance curves under different conditions

下载: 全尺寸图片幻灯片

图 4 本文公式与UMIST公式、古比雪夫公式的比较

Figure 4. Comparison between UMIST formulae, Kuibyshev formulae and this paper's formule

下载: 全尺寸图片幻灯片

图 5 临界震塌和贯穿厚度随冲击因子与钢筋阻力系数的变化

Figure 5. Normalized critical scabbing or perforation thickness versus impact factor and reinforcement factor

下载: 全尺寸图片幻灯片

表 1 本文计算结果与实验^[10]的比较

Table 1. Comparison between experimental results^[10] and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	实验	本文计算
T-1	2	25.0	2.6	4.0	10.5	27.0~35.7	47
T-2	2	25.2	3.1	4.4	10.5	41.7~56.8	49
T-3	2	161.9	7.3	17.2	10.5	34.7~58.5	97
T-4	2	175.3	13.8	24.6	10.5	76.0~104.0	116

下载: 导出CSV

表 2 本文计算结果与普通混凝土实验^[11]的比较

Table 2. Comparison between experimental results ^[11] of normal strength concrete and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	φ/mm	@/mm	f_s/MPa	μ	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	配筋		f_s/MPa	μ	实验	本文计算
D-1-1	2.0	35.0	3.0	5.12	15.9	2.5	34	382	0.43	165	103
D-1-2	2.0	35.0	3.0	5.12	15.4	3.0	25	183	0.40	222	101
D-1-3	2.4	35.0	3.0	5.12	20.8	3.0	25	183	0.40	232	117
D-1-4	2.4	35.0	3.0	5.12	127.1	5.0	27	473	2.68	236	291
D-1-5	2.0	34.0	3.4	5.38	53.6	3.25	21	600	1.76	210	193
D-1-6	2.0	34.0	3.4	5.38	34.8	2.5	20	650	1.19	162	156

下载: 导出CSV

表 3 本文计算结果与高性能混凝土实验^[12]的比较

Table 3. Comparison between experimental results^[12] of high performance concrete and present method

实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	φ/mm	@/mm	f_s/MPa	μ	v_BL/(m·s^-1)
实验	L/2a	f_c /MPa	f_t/MPa	τ_s/MPa	I_BL	配筋		f_s/MPa	μ	实验	本文计算
D-2-1	4	40.0	4.0	6.3	66.5	8	100	400	0.64	204~245	187
D-2-2	4	108.0	10.8	17.1	38.3	8	100	400	0.24	273~276	233
D-2-3	4	102.0	10.2	16.1	39.1	8	100	400	0.25	281~289	229
D-2-4	4	104.0	10.4	16.4	38.9	8	100	400	0.24	287~291	231
D-2-5	4	113.0	11.3	17.9	37.7	8	100	400	0.22	262~289	237
D-2-6	4	106.0	10.6	16.8	38.6	8	100	400	0.24	291~307	232
D-2-7	4	101.0	10.1	16.0	39.3	8	100	400	0.25	286~292	229
D-2-8	4	93.0	9.3	14.7	40.7	8	100	400	0.27	292~313	223
D-2-9	4	94.0	9.4	14.9	40.5	8	100	400	0.27	313~314	224
D-2-10	4	102.0	10.2	16.1	39.2	8	100	400	0.25	287~289	229
D-2-11	4	103.0	10.3	16.3	39.0	8	100	400	0.25	287~292	230

下载: 导出CSV

参考文献(16)

[1]	Hill R. The mathematical theory of plasticity[M]. Oxford: Clarendon Press, 1950.
[2]	Ravid M, Bodner S R. Dynamic perforation of visco-plastic plates by rigid projectiles[J]. International Journal of Engineering Science, 1983, 21(6):577-591. doi: 10.1016/0020-7225(83)90105-2
[3]	Amini A, Anderson J. Modeling of projectile penetration into geologic targets based on energy tracking and momentum impulse principles[C]//Proceedings of the Sixth International Symposium on Interaction of Nonnuclear Munitions with Structures. Germany, 1993.
[4]	Tirosh J, Tylis A, Davidi G. Foreign object damage: Penetration of rigid projectiles into solids[J]. Mechanics of Materials, 2009, 41(5):535-544. doi: 10.1016/j.mechmat.2009.01.024
[5]	陈士林, 王明洋, 潘越峰.锥形端部弹体在岩石(混凝土)介质层中侵彻实用计算方法[J].爆炸与冲击, 2004, 24(1):7-15. http://www.bzycj.cn/article/id/9912 Chen Shilin, Wang Mingyang, Pan Yuefeng. The method of calculation for penetration of conical nosed projectile in rock (concrete) layers[J]. Explosion and Shock Waves, 2004, 24(1):7-15. http://www.bzycj.cn/article/id/9912
[6]	王明洋, 施翠英, 陈士林.事故型撞击混凝土板的临界震塌与贯穿厚度计算方法[J].工程力学, 2009, 26(11):238-246. http://www.cnki.com.cn/Article/CJFDTotal-GCLX200911042.htm Wang Mingyang, Shi Cuiying, Chen Shilin. Method of calculating critical spalling and penetration thickness of concrete slab of block under accident impact[J]. Engineering Mechanics, 2009, 26(11):238-246. http://www.cnki.com.cn/Article/CJFDTotal-GCLX200911042.htm
[7]	冯淑芳, 王明洋, 任广昊, 等.深地下坑道衬砌结构抗局部冲击计算方法研究[J].岩石力学与工程学报, 2011, 30(6):1150-1156. http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb201106008 Feng Shufang, Wang Mingyang, Ren Guanghao, et al. Research on calculation method for local impact of deep tunnel lining structure[J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(6):1150-1156. http://d.old.wanfangdata.com.cn/Periodical/yslxygcxb201106008
[8]	咸玉席, 文鹤鸣.平头弹侵彻半无限混凝土靶的工程模型[J].防护工程, 2012, 34(2):35-38. http://cdmd.cnki.com.cn/Article/CDMD-10358-1014432193.htm Xian Yuxi, Wen Heming. An engineering model for the penetration of flat-nosed projectiles into semi-infinite concrete targets[J]. Protective Engineering, 2012, 34(2):35-38. http://cdmd.cnki.com.cn/Article/CDMD-10358-1014432193.htm
[9]	Li Q M, Ye Z Q, Ma G W, et al. Influence of overall structural response on perforation of concrete targets[J]. International Journal of Impact Engineering, 2007, 34(5):926-941. doi: 10.1016/j.ijimpeng.2006.03.005
[10]	Tai Y S. Flat ended projectile penetrating ultra-high strength concrete plate target[J]. Theoretical and Applied Fracture Mechanics, 2009, 51(2):117-128. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0214989790/
[11]	Dancygier A N. Effect of reinforcement ratio on the resistance of reinforced concrete to hard projectile impact[J]. Nuclear Engineering and Design, 1997, 172(1):233-245. http://cn.bing.com/academic/profile?id=723b6881b18fa7ba33bf200ca91ae81f&encoded=0&v=paper_preview&mkt=zh-cn
[12]	Dancygier A N, Yankelevsky D Z, Jaegermann C. Response of high performance concrete plates to impact of non-deforming projectiles[J]. International Journal of Impact Engineering, 2007, 34(11):1768-1779. doi: 10.1016/j.ijimpeng.2006.09.094
[13]	卡恰诺夫L M.塑性理论基础(第二版)[M].周承倜, 译.北京: 人民教育出版社, 1982: 332-385.
[14]	冯淑芳.深埋地下结构抗爆动力计算方法研究[D].南京: 解放军理工大学, 2011.
[15]	徐秉业, 刘信声.应用弹塑性力学[M].北京:清华大学出版社, 1995:510-512.
[16]	Wen H M, Xian Y U. A unified approach for concrete impact[J]. International Journal of Impact Engineering, 2015, 77:84-96. doi: 10.1016/j.ijimpeng.2014.11.015