由于具有比强度高和耐腐蚀能力强等优点，2A16-T4等铝合金材料在飞机结构中得到大量应用。而飞机在使用过程中不可避免受到飞鸟、跑道上碎石、冰雹以及轮胎碎片等外来物的撞击，为提高飞机的安全性并尽可能减轻飞机重量，就需要了解材料在不同应变率下的动态力学特性，获得材料准确的动态本构关系。

随着霍普金森实验技术的发展，对铝合金动态力学性能的研究也不断深入。研究表明，铝合金的塑性流动应力具有明显的应变率敏感性，而热激活位错运动是塑性流动行为的机理^[1]。D.L.Holt等^[2]发现纯铝在室温下表现出一定的应变率敏感性。王洪欣等^[3]发现3004铝在应变率0.000 5~2 000 s^-1范围内有明显的应变率强化效应; 然而，也有学者发现铝合金的应变率敏感性并不明显。张伟等^[4-5]通过电子万能试验机、扭转试验机、霍普金森拉杆等装置，发现2A12和7A04铝合金的应变强化效应和温度软化效应显著，而应变率强化效应不明显。张正礼^[6]结合电子万能试验机和Hopkinson杆实验，也发现2024铝合金是应变率不敏感材料; 此外，还有学者发现铝合金的应变率敏感性与应变率范围和温度有关。朱耀^[7]通过实验发现，AA7055铝合金在常温下的应变率敏感性不明显，而在高温下应变率敏感性较强。谢灿军等^[8]发现，7075-T6铝合金在应变率500 s^-1范围内应变率敏感性不明显，而当应变率超过1 000 s^-1后出现了明显的应变率强化效应，并基于实验结果拟合了修正的Johnson-Cook本构方程。杨超等^[9]也发现，5083H111铝合金材料在低应变率情况下存在应变率软化效应，在中低应变率范围内存在应变率软化再强化特性，在高应变率情况下又表现出明显的应变率强化效应。

上述研究表明，尽管在准静态(10^-4~10^-2 s^-1)和高应变率(10²~10⁴ s^-1)范围内对铝合金的动态力学性能进行了大量研究，但关于应变率效应实验结果的规律并不一致。而基于实验数据的经验型Johnson-Cook本构模型及其修正形式也被广泛应用于材料动态本构模型的构建中，但研究主要集中在准静态和高应变率区间，由此得到的材料本构模型不具备完备性，且到目前为止还未见关于2A16-T4铝合金动态力学性能的研究报道。因此，很有必要对较宽应变率范围下2A16-T4铝合金的动态力学性能进行研究，了解它在不同应变率范围内的应变率敏感特性，构建较完备的动态本构模型。

1.   动态力学性能实验

1.1   准静态拉伸

准静态拉伸实验平台为INSTRON 8801电子万能试验机(见图 1)，拉伸载荷通过试验机自带的载荷传感器测得，传感器量程为100 kN，拉伸应变通过接触式引伸计测得，夹头加载速度为2 mm/min。2A16-T4铝合金准静态真实应力应变曲线如图 2所示，在塑性段应变硬化效应较强，试件在准静态拉伸载荷作用下发生拉伸破坏，未出现明显的颈缩现象(见图 3)。

图  1  电子万能试验机

Figure  1.  INSTRON 8801 test system

下载: 全尺寸图片 幻灯片

图  2  真实应力应变曲线

Figure  2.  True stress-strain curve

下载: 全尺寸图片 幻灯片

图  3  准静态下试件破坏结果

Figure  3.  Failure results in quasi-static state

下载: 全尺寸图片 幻灯片

1.2   中应变率拉伸

相比材料的准静态及高应变率力学性能实验，材料的中应变率力学性能实验最难实现。主要是因为，在这个速率范围内的加载会引起加载链的共振问题，使实验过程中的关键参数不易采集。而传统的气锤、落锤等装置都具有在冲击过程中速度逐渐变低、应变率不恒定的缺点。相比于这些装置，高速液压伺服材料试验机具有横速率加载、实验重复性好及加载稳定等优点。因此，采用INSTRON VHS 160高速液压伺服试验机(见图 4)作为材料中应变率力学性能的测试平台。

图  4  高速液压伺服试验机

Figure  4.  INSTRON VHS 160 test system

下载: 全尺寸图片 幻灯片

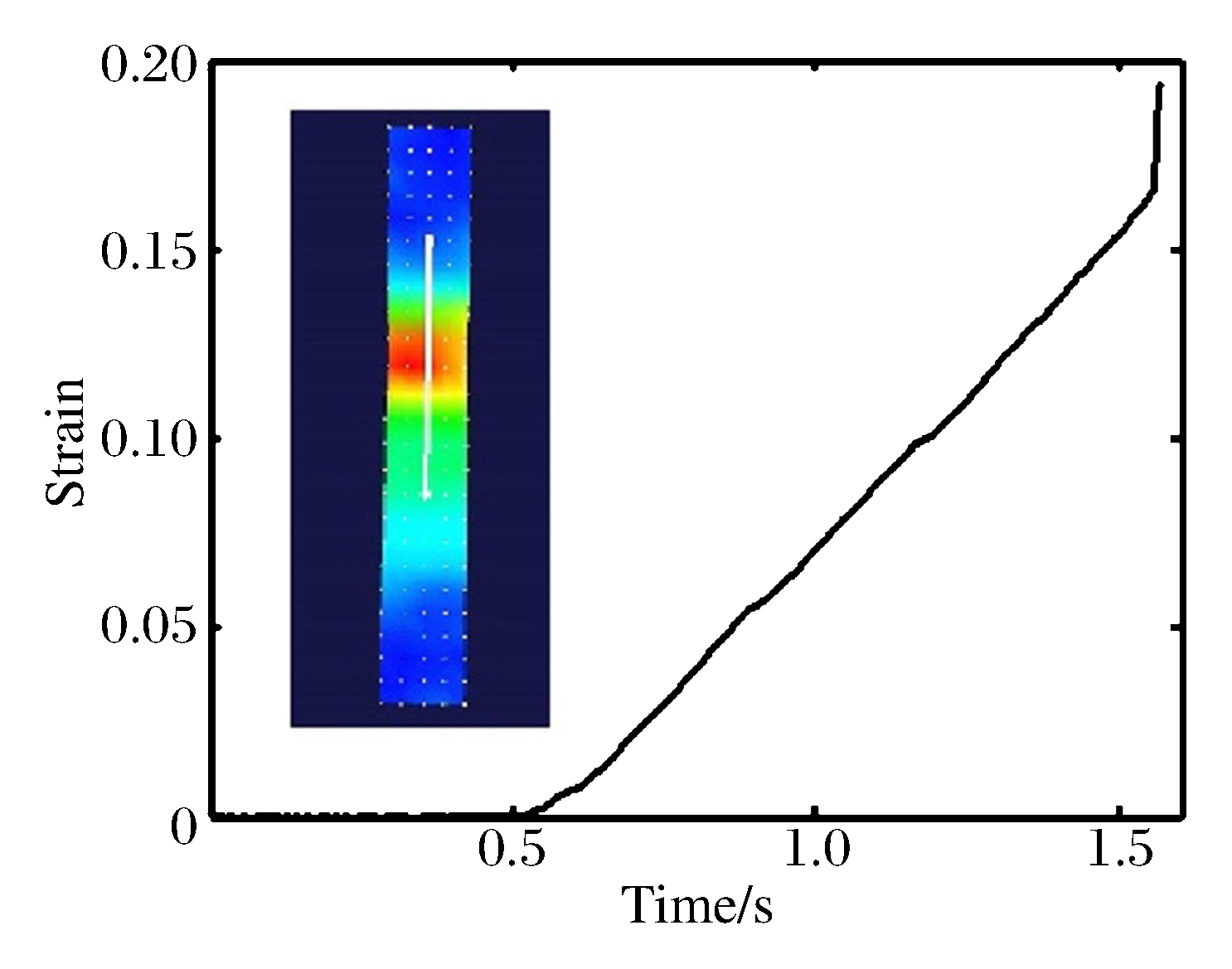
对于应变的测试，传统的引伸计和应变片测试方法受测量方式和量程的限制而无法使用，而基于高速摄像技术的非接触测试和分析系统(见图 5)可用来测试高速拉伸过程中试件表面的应变场。通过在试件的标距段喷涂散斑，利用高速摄像机实时采集目标区域散斑图像的变形情况，结合非接触分析软件计算试件的位移场，进而得到试件表面的应变场(见图 6)和动态拉伸应变数据(见图 7)。

图  5  非接触分析系统

Figure  5.  DIC system

下载: 全尺寸图片 幻灯片

图  6  试件表面应变场

Figure  6.  Strain field of specimen's surface

下载: 全尺寸图片 幻灯片

图  7  动态拉伸应变结果

Figure  7.  Strain result of dynamic tensile process

下载: 全尺寸图片 幻灯片

对于拉伸载荷的测量，较低应变率下可通过压电传感器直接得到，但应变率高于10 s^-1时，试验机系统的共振效应会导致测试结果发生很大幅度的振荡而失真^[10]，此时压电传感器的测量结果已无法反映材料的真实力学特性。为解决这个问题，在试件非标距段两侧的对等位置沿拉伸方向粘贴应变片，利用应变片间接测量试件的载荷数据，可提高测试结果的准确性^[11-12] (见图 8)。

图  8  载荷间接测试方法

Figure  8.  Indirect loading measurement

下载: 全尺寸图片 幻灯片

高速摄像机和数采系统同时触发，采样率相同，可以获得同步的载荷与应变信号，经数据处理得到2A16-T4铝合金中应变率下的真实塑性应力应变曲线，如图 9所示。可知在应变率10^-4~10² s^-1范围内应变率敏感性较弱，随着应变率的增加，材料的流动应力和动态屈服强度增加很小，流动应力仅强化3%左右。但应变硬化效应较强，随着塑性应变的增加，材料的流动应力明显提高。

图  9  真实塑性应力应变曲线

Figure  9.  True plastic stress-strain curves

下载: 全尺寸图片 幻灯片

为研究应变率和应变对材料流动应力的影响，取不同应变率下参考塑性应变为0.03、0.08和0.12时的流动应力进行分析，如图 10所示(准静态下塑性应变0.12的流动应力值为外插结果)。可以看出，流动应力均随应变率的增大而缓慢增加，表现出较弱的应变率强化效应。在相同应变率下，流动应力均随应变的增大而增大，表现出较强的应变硬化效应。当塑性应变为0.03、0.08、0.12时，应变率强化效应分别为5.8%、3.0%、2.5%;当应变率为0.000 4、0.032、0.065、10、130、350 s^-1时，应变硬化效应分别为15.8%、15.0%、15.0%、14.8%、13.8%、13.2%。可以看出，材料的应变率强化效应随塑性应变的增大而减小(用0.032 s^-1和350 s^-1计算)，材料的应变硬化效应随应变率的增大而减小(用应变0.03和0.08计算)。在高速拉伸载荷作用下，材料的应变硬化和热软化同时进行，一方面，它具有一定的应变硬化作用，另一方面，较高应变率变形时载荷作用时间极短，试件内由塑性变形能转化的热量无法耗散，导致的试件温升使试件产生一定的热软化，且应变率越高热软化效应越强，所以材料的应变硬化效应随应变率的增大呈现出减小的趋势(见图 11)。

图  10  流动应力与应变率的关系

Figure  10.  Relation of flow stress with strain rates

下载: 全尺寸图片 幻灯片

图  11  试件拉伸破坏结果

Figure  11.  Failure result of specimen

下载: 全尺寸图片 幻灯片

1.3   高应变率压缩

高应变率压缩实验平台如图 12所示，由发射装置、输入杆和输出杆组成。采用应变片测量输入杆中的入射、反射脉冲和输出杆中的透射脉冲，根据测量数据结合一维应力波理论和均匀性假设，计算试件的应力、应变和应变率^[13]。试件尺寸为$\varnothing$ 8 mm×8 mm，共进行3种应变率(1 000、2 000、3 000 s^-1)下的动态压缩实验。

图  12  SHPB装置

Figure  12.  SHPB setup

下载: 全尺寸图片 幻灯片

2A16-T4铝合金真实塑性应力应变曲线如图 13所示，可以看出，它在应变率10^-4~10³ s^-1范围内表现出明显的应变率强化效应，随着应变率的增加，流动应力强化50%左右。这与它在中应变率范围内的应变率敏感特性明显不同，表明2A16-T4铝合金在常温下的应变率敏感特性与应变率范围有关。在应变率350 s^-1以内，应变率敏感性较弱，而当应变率超过1 000 s^-1以后，材料的应变率敏感性明显增强。该特性与文献[8]中对7075-T6铝合金动态力学特性的研究结果相似。此外，2A16-T4铝合金在高应变率范围内的应变硬化效应依然很明显。

图  13  真实塑性应力应变曲线

Figure  13.  True plastic stress-strain curves

下载: 全尺寸图片 幻灯片

为研究应变率和应变对材料流动应力的影响，取不同应变率下参考塑性应变为0.03、0.08和0.12时的流动应力进行分析，如图 14所示。可以看出，流动应力均随应变率的增大而迅速增加，表现出较强的应变率强化效应。在相同应变率下，流动应力均随应变的增大而增大，表现出一定的应变硬化效应。当塑性应变为0.03、0.08、0.12时，应变率强化效应分别为18.3%、16.1%、11.7%;当应变率为0.000 4、1 000、2 000、3 000 s^-1时，应变硬化效应分别为15.8%、12.2%、11.9%、10.1%。可以看出，材料的应变率强化效应随塑性应变的增大而减小(用应变率1 000 s^-1和3 000 s^-1计算)，应变硬化效应随应变率的增大而减小(用应变0.03和0.08计算)，变化规律与中应变率范围下的结果相同。

图  14  流动应力与应变率的关系

Figure  14.  Flow stress vs strain rate

下载: 全尺寸图片 幻灯片

2.   Johnson-Cook本构关系拟合

Johnson-Cook模型^[14]用于描述材料流动应力与应变、应变率及温度之间的关系。它采用连乘的形式将应变效应、应变率效应和温度效应综合处理，具体形式为：

式中：σ为等效应力; B、n为应变硬化系数; A为参考应变率和参考温度下的屈服应力; ε为等效塑性应变; C为应变率敏感系数; $\dot{\varepsilon }_{\text{e}}^{*}$为量纲一应变率，$\dot{\varepsilon }_{\text{e}}^{*}={{{\dot{\varepsilon }}}_{\text{e}}}/{{{\dot{\varepsilon }}}^{0}}, {{{\dot{\varepsilon }}}^{0}}$为参考应变率; m为温度敏感性系数; ${{T}^{*}}=(T-{{T}_{\text{r}}})/({{T}_{\text{m}}}-{{T}_{\text{r}}})$为量纲一温度，T_m为材料熔点，T_r为参考温度。

2.1   Johnson-Cook本构模型的修正

由实验结果可知，2A16-T4铝合金在中应变率范围下应变率强化效应较弱，而在高应变率范围下应变率强化效应较强，流动应力与对数应变率近似成指数关系(见15)。考虑到标准Johnson-Cook模型将材料流动应力与对数应变率描述为线性形式，因此，需对应变率项进行修正。

W.J.Kang等^[15]、G.R.Cowper等^[16]和H.Huh等^[17]针对Johnson-Cook模型的应变率项进行了修正，分别为：

采用新的应变率项后对实验结果的拟合效果更好。考虑2A16-T4铝合金在低、中、高应变率范围内的应变率敏感特性，选择式(2)作为应变率项的修正形式。

2.2   本构模型参数的确定

实验均在室温下进行，参数拟合时不考虑温度项m，只有5个待定材料参数A、B、n、C和P。

选取参考应变率4×10^-4 s^-1的实验数据，拟合参数A、B、n，在室温、参考应变率下，式(1)简化为：

根据参考应变率下的应力应变曲线结合matlab软件，拟合得参数A=289 MPa、B=500 MPa、n=0.52。

通常认为金属材料的力学性能具有较好的各向同性，因此在结果分析中没有区分拉伸与压缩。利用室温不同应变率下的应力应变数据拟合参数C、P。室温下Johnson-Cook本构模型可简化为：

式中：σ₀为参考应变率下的屈服应力; σ为不同应变率下的屈服应力。

为研究材料中应变率力学特性对应变率敏感参量的影响，对以下3种情况进行分析：(1)只考虑准静态和中应变率实验数据; (2)只考虑准静态和高应变率实验数据; (3)综合考虑准静态、中应变率和高应变率实验数据。

目前，应变率敏感参数C大多通过对不同应变率下的屈服应力进行拟合得到，而2A16-T4铝合金的应变率强化效应与应变有关，因此依次取2A16-T4铝合金塑性应变0.03、0.08、0.12时的流动应力与应变率关系(见图 15)进行分析。分析结果见表 1。可以看出，3种情况下的拟合结果之间有较大的区别，但各自的拟合结果与实验结果吻合很好，如图 16所示。

图  15  材料流动应力与应变率关系

Figure  15.  Relation of flow stress with strain rates

下载: 全尺寸图片 幻灯片

表  1  2A16-T4铝合金应变率参数拟合结果

Table  1.  Fitted results of strain rate sensitive parameters

情况	C	P		C	P		C	P	${\bar{C}}$	${\bar{P}}$
	ε=0.03			ε=0.08			ε=0.12
1	2.3×10-3	1.29		1.80×10-3	1.04		1.20×10-3	0.95	1.77×10-3	1.093
2	4.9×10-9	6.69		4.75×10-9	6.60		4.72×10-9	6.55	4.79×10-9	6.630
3	1.9×10-11	8.71		1.82×10-11	8.63		1.78×10-11	8.58	1.83×10-11	8.660

下载: 导出CSV 

| 显示表格

图  16  2A16-T4铝合金应变率敏感性拟合结果与实验结果对比

Figure  16.  Comparison of fitted results of Johnson-Cook model with experiment results for 2A16-T4

下载: 全尺寸图片 幻灯片

2.3   材料中应变率力学性能数据对率相关参数的影响

由于材料中应变率力学性能实验技术普及程度不高，对材料动态力学性能的研究主要集中在准静态和高应变率范围内。此时，只能利用高应变率实验数据得到的Johnson-Cook本构模型，通过内插或外推方法得到其他应变率下的结果。

而实际情况中，材料在不同应变率范围内的应变率敏感性不一定相同，利用内插或外推法可能会与实际结果产生一定的误差。

图 17为利用高应变率实验结果拟合的Johnson-Cook本构模型外推得到的材料中低应变率力学特性与真实结果的对比，可以看出，外推法对较低应变率数据预测结果较好，但对较高应变率的预测值偏高(预测值偏差大小与所选本构方程有关)。因此，为得到能覆盖材料由准静态到高应变率全范围力学特性的本构模型参数，必需综合考虑材料准静态、低、中、高应变率实验数据。由此得到的材料修正Johnson-Cook本构模型参数分别为A=289 MPa、B=500 MPa、n=0.52、C=1.83×10^-11、P=8.66，修正本构模型拟合结果与实验结果的对比如图 18所示。

图  17  外推结果与实验结果的对比

Figure  17.  Comparison of extrapolated results with experimental results

下载: 全尺寸图片 幻灯片

图  18  拟合结果与实验结果的对比

Figure  18.  Comparison of fitted results with experimental results

下载: 全尺寸图片 幻灯片

可以看出，所有应变率下拟合结果与实验结果均吻合很好，证明了本文所得修正Johnson-Cook本构模型预测2A16-T4铝合金动态力学行为的准确性。

3.   结论

通过实验方法研究了较宽应变率范围下2A16-T4铝合金的动态力学特性，基于实验结果拟合得到修正Johnson-Cook本构模型，并分析了材料中应变率力学特性对率相关参量的影响。主要结论如下：

(1) 2A16-T4铝合金在常温下的应变率敏感性与应变率范围有关，在应变率10^-4~10² s^-1范围内率敏感性较弱，而在10²~10³ s^-1范围内率敏感性很强，且应变率强化效应随应变的增大而减小;

(2) 2A16-T4铝合金在应变率10^-4~10³ s^-1范围内具有较强的应变硬化效应，且应变硬化效应随应变率的增大而减小;

(3) 2A16-T4铝合金的流动应力与应变率和应变相耦合，但在中应变率范围内主要受应变的影响，而在高应变率范围内同时受应变率和应变的影响;

(4) 基于实验结果拟合得到修正的Johnson-Cook本构模型的预测结果与实验数据吻合很好，且考虑材料中应变率力学特性可提高应变率相关参量的准确性。