Assessment on single degree of freedom modelin steel column analysis of anti-detonation
-
摘要: 为评估单自由度(SDOF)模型在结构抗爆设计中的适用性,分别采用SDOF模型和通用有限元软件ANSYS/LS-DYNA对简支钢柱承受爆炸荷载时的动力响应进行模拟;对比二者计算结果,并以有限元模拟为准,分析SDOF模型的适用范围。研究表明:可按照自由振动阶段SDOF模型位移结果的振幅大小,将其位移响应划分为有限变形阶段、临界阶段、失稳破坏阶段,有限变形阶段SDOF模型与有限元结果基本一致;截面高宽比、翼缘宽厚比对钢柱动力破坏形式有重要影响,高宽比越大、翼缘的宽厚比越小,越容易发生平面外弯扭失稳;在SDOF模型中通过假定塑性铰分布长度计算塑性阶段应变及应变率,采用随时间变化的应变率计算Cowper-Symonds本构关系中的应力放大系数是可行的。Abstract: For the evaluation of the applicability of the single degree of freedom (SDOF) model in the structural antiknock design, the dynamic response of the simply supported steel column under explosion load was simulated using both the SDOF model and the ANSYS/LS-DYNA in this paper. By the comparison of the two calculation results, the scope of application of the SDOF model was analyzed according to the finite element simulation. The results show that the displacements calculated using the SDOF model can be divided into three different phases including the finite deformation, in which the SDOF model agrees well with the DYNA simulation, the critical deformation, and the buckling failure deformation, according to the amplitude size in the free vibration. The ratio of the cross section's depth to its width and that of the flange's width to its thickness have significant effect on the dynamic failure forms of the steel column, namely the bigger the ratio of the depth to the width and the smaller the ratio of the width to the thickness, the more prone it is for the buckling to suffer out-of-plane bending and twisting. In the SDOF model, it is feasible to calculate the strain and the strain rate in the plastic deformation phase by assuming the plastic hinge distribution length and the stress-magnified coefficient in the Cowper-Symonds constitutive relation by using the time-dependent strain rate.
-
Key words:
- blast load /
- steel column /
- single degree of freedom model /
- finite element /
- strain rate
-
弹药通常为带壳体的约束装药,约束装药点火后的灾变反应演化过程十分复杂,涉及炸药本征燃烧特性、产物气体驱动炸药裂纹扩展、壳体发生力学变形和失效解体加速运动等过程,决定了弹药最终的反应烈度。弹药在意外刺激下的安全性评估结果是弹药不敏感等级划定的重要依据,建立约束装药灾变反应演化模型对实现弹药安全性量化评估具有重要意义。
针对约束装药点火后的反应演化行为,在实验方面,Dickson等[1]观测到,约束装药点火后,火焰从中心孔射出,初始裂缝由孔中气体压力产生的环向应力导致,随后内部压力继续驱动裂纹扩展,促使形成多条裂纹,大大增加了反应表面积,提高了反应速率。Parker等[2]认为,在加热的炸药中,裂纹内的燃烧可能会扩展形成连通的孔隙。Holmes等[3-4]分别对不同约束强度下的PBX-9501炸药开展了激光点火试验,结果表明,约束装药最终的反应演化程度受到壳体约束的影响,其对产物气体的约束作用是促进炸药基体裂纹扩展的主控因素之一。Wang等[5]采用不同强度的金属约束圆环控制装药的约束强度,观测了激光与装药中心电热丝点火加载下,炸药燃烧产生高温高压气体驱动装药裂纹的形成和扩展过程,发现强约束下裂纹扩展和基体破碎是加剧反应烈度的关键机制。姚奎光等[6]通过测量内部压力和壳体膨胀速度,研究了炸药力学性能及机械约束强度对炸药反应演化行为的影响,结果表证明,机械约束条件对装药反应演化存在显著影响,壳体膨胀速度可达量级差异。Tringe等[7]采用脉冲X射线技术对圆柱形约束装药点火实验进行了观测,通过位移推算出径向侧壁和轴向端盖的运动速度,但并未获得内部压力的分布历史,结果表明,炸药消耗情况与壳体膨胀速度存在关联。Smilowitz[8]通过X摄影技术获得了约束装药烤燃试验的动态图像,并利用固体密度损失的测量值推测出裂纹的传播速度,认为壳体的运动速度是装药裂纹扩展的重要表征量。
上述研究结果表明,装药内部压力和壳体膨胀运动速度是表征装药反应演化行为的关键参量,反映了约束装药反应的能量释放和做功特性,而考虑壳体惯性约束效应影响下壳体膨胀速度与内部压力的耦合关系是准确描述约束装药反应演化行为的前提。近年来,Hill[9]基于Dickson等[1]和Parker等[2]的研究,考虑炸药装药内孔隙分布规律,建立了受约束炸药燃烧裂纹网络模型,初步实现了炸药装药反应演化过程的计算,但未给出与实验结果的对比,模型的有效性有待验证,且模型考虑因素单一,与实际工程要求差距较大。段卓平等[10-11]和白志玲等[12]分别建立了强约束固体炸药燃烧裂纹网络演化和调控模型,以及热刺激约束熔铸炸药装药燃烧气泡云反应演化模型,可较好地反映炸药本征燃烧特征、装药结构尺寸、空隙体积、壳体厚度等对反应演化的影响,但上述模型仅考虑了壳体的弹塑性变形直至失效破坏,未考虑壳体的运动,即未考虑壳体进入完全屈服后和壳体破裂后的运动惯性约束,预测装药反应演化行为和量化表征反应烈度时误差较大。
本文中,在段卓平等[10-11]和白志玲等[12]的模型的基础上,建立考虑壳体运动惯性约束效应的装药燃烧裂纹网络反应演化模型,描述壳体变形运动与装药反应压力演化的耦合作用过程,通过与典型实验结果进行对比,验证模型的合理性,以期为弹药安全性设计与评估提供理论依据。
1. 模型构建
圆筒约束装药点火后生成的气体产物形成局部高压,驱动裂纹扩展成网状,燃烧比表面积增加,加速反应进行,壳体在内部不断升高的压力作用下发生变形、运动直至破裂,最终形成破片并对外输出高压气体,约束装药壳体破裂后,由于惯性效应,内部压力衰减和装药反应将维持一定的时间,同时推动破片做加速运动[13-15]。为方便描述,将圆柱段壳体变形分为3个阶段:(1) 准静态弹塑性变形阶段;(2) 完全屈服运动阶段;(3) 壳体破裂后运动的惯性约束运动阶段,不考虑端盖变形,仅根据端盖处是否达到剪切强度判断破坏的发生情况。需要指出的是,本文中提及的裂纹和裂纹扩展泛指固体炸药装药的初始孔洞、裂纹以及在各种刺激载荷作用下产生的宏细观孔洞、裂纹等。
1.1 圆柱段壳体弹塑性阶段
圆柱段壳体弹塑性阶段[11]从装药点火时刻开始,点火产生的内部压力使炸药基体局部破碎,出现随机分布的裂纹,随后的燃烧反应演化过程中,圆柱段壳体在内部压力作用下发生弹塑性变形,直至壳体达到完全屈服状态[16]。该阶段的圆柱段壳体变形较小,因此,忽略壳体运动,不考虑惯性约束效应。为研究装药反应演化过程,便于试验测试,壳体与炸药装药之间往往预留一定的空气隙,该体积统称为初始空隙体积。因此,约束装药体系总体积与炸药体积、初始空隙体积及裂纹扩展体积的关系满足:
V=Ve+Va+Vf (1) 式中:V为约束装药体系总体积,Ve为炸药体积,Va为初始空隙体积,Vf为裂纹扩展体积。裂纹处理为类裂缝空间,由装药体系的裂纹网络总表面积(S)和裂纹宽度(δ)决定,即Vf=Sδ。初始时刻,炸药内部有裂纹缺陷但初始裂纹宽度δ0=0 mm。因此,装药初始体积V0满足:
V0=Ve0+Va (2) 式中:Ve0为炸药初始体积,假设燃烧反应演化过程中,初始空隙体积保持不变,即Va保持不变。
联立式(1)~(2)得
V−V0V0=Ve−Ve0Ve0Ve0V0+VfV0 (3) 壳体内部空间体积的相对变化,即为整个系统的体应变εv=(V–V0)/V0。假设壳体广义等效刚度
I 满足I=p/εv,p为压力,采用理想弹塑性本构模型描述圆筒壳体材料变形,并考虑薄壳与厚壳等情况,壳体弹塑性变形过程中广义等效刚度I 的表达式参考文献[11],这里仅给出厚壁圆柱壳体的广义等效刚度I [11]的表达式:I = σs√3(1−r2R2o+2lnrRo)(1−2μ)σsE(K2−1)(1−r2R2o+2lnrRi)(r22R2i−12)+σsr2ER2o[(1−2μ)r2R2i+(1+μ)K2]+(1−2μ)σs2E(K2−1)(1−r2R2o+2lnrRi) (4) 式中:Ri、Ro分别为圆柱段壳体的初始内半径和初始外半径,r为弹塑性半径,σs为圆柱段壳体的静态屈服强度,K为壳体初始内半径与外半径之比,μ为圆柱段壳体的泊松比,E为弹性模量。
炸药的体应变为εve=(Ve–Ve0)/Ve0,满足–B=p/εve,
B 为炸药体积模量,代入式(3)得:pI=−pBVe0V0+VfV0 (5) 定义系统的广义刚度为M,满足:
1M=1I+1BVe0V0 (6) 由式(5)~(6)可得裂纹扩展体积:
Vf = pV0M (7) 约束装药燃烧裂纹扩展如图1所示,圆柱形约束装药中心发生一定强度的点火,引发炸药基体表面形成燃烧区,产生的高温高压气体驱动装药发生局部破碎形成裂纹,燃烧比表面积增加,导致产物气体增多,进一步驱动裂纹扩展成网状,为燃烧和气体产物提供了更多通道,如此“正反馈”使得燃烧机制由层流燃烧转为自增强燃烧,促进了反应高速进行。
炸药燃烧速率满足Vielle定律[17]:
˙mq=αpβ (8) 式中:
˙mq 为单位燃烧表面积产物的质量流,α为燃烧相关系数,β为燃烧相关指数。炸药装药体系含有足够多的裂纹时,燃烧裂纹分叉扩展成网,表征燃烧裂纹网络的关键参量即燃烧裂纹网络表面积S与压力p满足以下关系[10-11]。
裂纹和裂纹扩展具有明显的不规则性和随机性,可采用Weibull分布描述燃烧裂纹网络特征,建立点火后燃烧裂纹网络细观结构与宏观性能的关系。点火后,某条裂纹在压力p下发生扩展的概率密度函数f(p)可定义为:
f(p)={nPr(p−p0Pr)n−1exp[−(p−p0Pr)n]p≥pi0p<pi (9) 式中:n为形状参数,一般认为是材料常数;Pr为尺度参数;p0为初始压力;pi为点火阈值压力。根据最弱环理论,在压力p作用下,炸药装药燃烧裂纹网络发生扩展演化的概率为:
Pf(p)=1−exp[−(p−piPr)n] (10) 于是,燃烧裂纹网络面积S与压力p的关系表达式为:
S(p)=Si+(ρe0Ve0Smax−Si){1−exp[−(p−piPr)n]} (11) 式中:Si与点火强度有关,可通过典型实验标定;Smax为燃烧饱和比表面积;ρe0为炸药初始密度。
由式(8)和(11)得到产物气体质量增加速率:
dmgdt=S(p)˙mq=αpβS(p) (12) 式中:mg为产物气体的质量,即已反应的炸药质量。采用理想气体状态方程描述产物气体,则存在以下关系:
p = ρRpTpMg=mgRpTp(Va+pV0/M)Mg (13) 式中:Rp为普适气体常数,Tp为产物气体温度,Mg为产物气体的摩尔质量。
整理得到壳体弹塑性阶段的固体炸药装药非冲击点火反应演化过程中压力p关于时间t的表达式:
pb=∫tb0Va+2V0ξ/MαξβS(ξ)Zdξ (14) 式中:Z=RpTp/Mg。圆柱段壳体达到完全屈服状态时,准静态弹塑性阶段结束,此刻系统体积为Vb,炸药体积为Veb,内部压力为pb,时间为tb,圆筒内半径为Rb,圆柱高度为Lb。
1.2 圆柱段壳体完全屈服运动阶段
第一阶段结束后,圆柱段壳体处于完全屈服状态,假设圆柱段壳体在内部压力作用下以恒定屈服应力状态向外加速膨胀,直至壳体发生断裂破裂,断裂应变大小由材料性质决定。圆柱形装药壳体受内压作用发生破坏,由于壳体具体结构及材料参数、点火后装药网络燃烧反应特性不同,导致圆柱段壳体与端盖发生破坏的时刻不一致,因此,需要分别对2处结构是否发生破坏及顺序进行判断。
轴向端盖破坏判据假设端盖厚度与大小在装药反应过程中不发生变化,顶部端盖达到轴向剪切强度[τ]时沿轴向发生剪切破坏。对端盖进行受力分析:
πpR2i−2πτRiHz=0 (15) 式中:Hz为圆筒端盖厚度,τ为剪切应力。端盖受均布压力和剪切力作用如图2所示。
圆柱段壳体破坏判据基于以下假设:(1) 圆柱段壳体材料不可压缩,即密度不变;(2) 圆柱段壳体为薄壁圆筒,不考虑圆柱壳体的伸长作用,壳体环向屈服应力σθ沿厚度均匀分布;(3) 气体压力动态均匀分布于圆筒内壁,变形过程中壳体应力处于材料流动应力(屈服应力)状态,圆柱段仅受到环向屈服应力σθ约束。
考虑圆柱段壳体在变形过程中半径扩大,壁厚不断变薄,假设圆柱段壳体长度不变,则总截面不变,有:
R2o(t)−R2i(t)=R2o−R2i (16) Hr=Ro−Ri (17) Hr(t)=Ro(t)−Ri(t) (18) 式中:Ro(t)、Ri(t)分别为圆筒变形过程中的实时外半径和内半径,其中
Ri(t)=Ri+∫ttbvrdt ;Hr为初始圆筒壁厚;Hr(t)为实时壁厚;vr为圆筒径向运动速度(这里不考虑内、外壁运动差)。对于图3中的薄壳圆柱,由于不考虑其z轴方向的长度变化,因此,不考虑轴向应力σz和厚度方向的剪切应力τzr,建立圆柱段壳体运动控制方程如下:
pRi(t)dθdz−2σθsindθ2Hr(t)dz=12[R2o(t)−R2i(t)]ρsdθdzdvrdt (19) 式中:ρs为材料密度。环向应变的表达式为:
εθ=Ri(t)−RiRi (20) 圆柱段壳体达到断裂应变时壳体发生破裂,即
εθ>εc ,εc 为材料的断裂应变,由壳体材料的性质决定。需要指出的是:若轴向端盖约束较弱,则需要在圆柱段壳体准静态弹塑性阶段内首先对轴向端盖是否发生破坏进行判断,如果端盖发生破坏,由于此时约束装药处于反应初期,内部压力较小,可能出现反应淬灭停止或圆柱段壳体无法变形至完全屈服阶段,此时约束装药反应状态和壳体变形将停留在第一阶段;如果反应继续进行,需要对圆柱段壳体能否变形直至完全屈服阶段进行计算。
若轴向端盖约束较强,在圆柱段壳体准静态弹塑性阶段内,轴向端盖和圆柱段壳体均不发生破坏,则圆柱段壳体将进入完全屈服阶段,壳体破裂状态在时序上大致分为2种情况:若端盖剪切应力达到剪切强度时,圆柱段壳体的环向应变小于断裂应变,则端盖结构先于圆柱段壳体发生破坏,随后圆柱段筒身将继续膨胀或停止或破裂;若圆柱段壳体的环向应变大于断裂应变时,端盖剪切应力未达到剪切强度,则圆柱段壳体结构先发生破坏形成破片,随后端盖受到产物气体的作用在轴向发生运动。壳体的几种破坏状态如图4所示。
壳体破裂属于何种状态是由壳体膨胀时的环向应变和端盖的剪切大小决定的,通过两者达到断裂应变的时间先后确定计算流程以及启动破裂后的惯性运动约束阶段计算。本文中以“在圆柱段壳体完全屈服阶段,端盖先发生破坏,随后圆柱段壳体继续膨胀直至破裂”这种破坏状态为例简要描述分析建模过程。
以圆柱段壳体弹塑性阶段结束时刻tb作为圆柱段壳体完全屈服阶段的起始时刻,此阶段系统体应变的表达式为:
εⅡv=π(Rb+∫ttbvrdt)2(Lb+∫ttbvzdt)−πR2bLbπR2bLb (21) 壳体完全屈服阶段运动过程的时间为tb~t,壳体的广义等效刚度依旧满足
IⅡ=p/εⅡv ,重新定义此阶段系统的广义变形等效刚度为MⅡ ,得到壳体完全屈服阶段的裂纹扩展体积:VⅡf=pVbMⅡ (22) 采用理想气体状态方程描述产物气体,则存在以下关系:
p=ρRpTpMg=mgRpTp(Va+Vf+VⅡf)Mg (23) 整理得到壳体完全屈服阶段固体炸药装药点火后反应演化过程中压力p关于时间t的表达式:
pc−pb=∫tctbVa+Vf+VⅡ−Vb+2VebBξαξβS(ξ)Z−ξdVⅡdtdξ (24) 式中:
VⅡ 为壳体完全屈服阶段的系统体积,其中dVⅡdt=π[Rb(2Lbvr+Rbvz+2vr∫tctbvzdt+2vz∫tctbvrdt)+2vrLb∫tctbvrdt+2vr∫tctbvrdt∫tctbvzdt+vz(∫tctbvrdt)2]。 已有研究表明,弹体达到断裂应变时壳体发生破裂,破裂应变由材料性质决定[18],此时完全屈服阶段结束,形成终态破片,此刻系统体积为Vc,炸药体积为Vec,壳体内部压力为pc,时间为tc,圆筒内半径为Rc,圆柱高度为Lc。
1.3 壳体破裂后惯性运动约束阶段
壳体破裂后,内外压差导致高压气体产物通过裂缝向外泄漏,壳体破碎形成的破片在向外高速运动的同时仍对装药存在约束作用,在惯性约束运动阶段,装药持续反应的增压效应与壳体运动及泄压导致的降压效应相互耦合作用,共同决定了装药的反应演化进程。如图5所示,假设圆柱段壳体沿z方向形成长条形破片,壳体应力消失,运动方程只考虑惯性约束力,气体产物驱动破片做加速运动,破片之间力的作用可以忽略,破片尺寸不再发生变化。
根据牛顿第二定律,对惯性运动约束效应阶段圆筒侧壁建立运动控制方程:
2πpRcLc=2πRcLcρcHrdvrdt (25) 端盖运动满足:
πpR2c=πR2cρcHzdvzdt (26) 以壳体破碎时刻tc为惯性运动约束效应的起始时刻,此阶段系统体应变的表达式为:
εⅢv=π(Rc+∫ttcvrdt)2(Lc+∫ttcvzdt)−πR2cLcπR2cLc (27) 定义系统广义等效惯性约束刚度为
MⅢ ,得到壳体破裂后惯性运动约束阶段的裂纹扩展体积为:VⅢf=pVcMⅢ (28) 考虑壳体破裂后的高压“泄漏”效果如图6所示,裂缝由多个随机宏观裂缝组成,泄露效果等同于随半径扩大而增大的裂缝泄漏。
裂缝的泄压面积为:
Ag=2π(Rc+∫ttcvrdt)(Lc+∫ttcvzdt)−2πRcLc+π(Rc+∫ttcvrdt)2−πR2c (29) 对于不考虑散热流动,随壳体一起运动的理想气体近似为准定常流动,由能量守恒定律可知,壳体内任一点与泄压处上的点存在以下关系[19]:
Hg1+v2g12=Hg2+v2g22 (30) 式中:Hg1为壳体内的焓,Hg2为壳体泄压到大气压时的焓,vg1为壳体破裂后“壳体内部”的产物气体径向膨胀速度,vg2为气体泄漏速度。气体径向膨胀速度比泄压处气体流速小得多,因此,可以忽略。Hg1=pγ/(ργ−ρ),Hg2=pg2γ/(ρg2γ−ρg2),其中γ为气体等熵指数,ρ为产物气体密度,pg2为大气压强,取1.013×105 Pa,ρg2为泄压至大气压时的密度。
假设产物气体等熵膨胀,根据多方方程:
pg2p=(ρg2ρ)γ (31) 结合式(30),得到产物气体泄漏速度vg2为:
vg2={2γγ−1pVⅡ[1−(pg2p)γ−1γ]}12 (32) 综上,产物气体泄漏质量流率为:
˙mout=Agp√2γMgRpTp(γ−1)[(pg2p)2γ−(pg2p)γ+1γ] (33) 惯性约束效应阶段的产物气体质量增加速率为:
dmgdt=S(p)˙mq−˙mout=αpβS(p)−˙mout (34) 当压力开始下降时,炸药裂纹S(p)不再随压力变化而变化。
采用理想气体状态方程描述产物气体,则存在以下关系:
p = ρRpTpMg=mgRpTp(Va+Vf+VⅡf+VⅢf)Mg (35) 整理得到壳体破裂后惯性运动约束阶段固体炸药装药非冲击点火反应演化过程中的p-t表达式:
p−pc=∫ttc(Va+Vf+VⅡf+VⅢ−Vc+2VecBξ){αξβS(ξ)−ξAe√2γMgRpTp(γ−1)[(pg2ξ)2γ−(pg2ξ)γ+1γ]}Z−ξdVⅢdtdξ (36) 式中:
VⅢ 为壳体破裂后惯性运动约束阶段的系统体积,其中dVⅢdt=π[Rc(2Lcvr+Rcvz+2vr∫ttcvzdt+2vz∫ttcvrdt)+2vrLc∫ttcvrdt+2vr∫ttcvrdt∫ttcvzdt+vz(∫ttcvrdt)2]。 反应演化过程中,装药反应度的表达式为:
λ=∫t0S(p)˙mgdtρe0Ve0 (37) 反应速率
˙λ 为反应度的导数。2. 模型验证与分析
为确定反应演化模型参数并验证模型的合理性,建立典型约束装药点火及反应演化测量实验系统,如图7所示。装药为PBX8701,装药尺寸为
∅ 50 mm×50 mm,密度为1.60 g/cm3,一端中心开有20 mm的深孔,采用点火具和孔内预填黑火药实现装药中心点火;约束壳体由壁厚为20 mm的环向筒体和厚度可调节的顶端约束体组成,通过调整约束体底部厚度改变壳体约束强度,设计了2种约束体底部厚度,分别为1和3 mm。筒体侧壁安装QSY压力传感器用于测量内部压力-时间曲线,采用多通道光子多普勒测速系统(photon Doppler velocimetry,PDV)测量筒体侧壁膨胀运动速度-时间曲线。将实验数据和模型计算压力以及筒体侧壁运动速度结果汇总,分别如图8~9所示。计算用8701炸药的热力学参数及壳体参数如表1所示。结果表明,计算结果与实验数据均符合较好,初步验证了本文中模型的合理性。
表 1 计算所需PBX8701炸药的热力学参数及壳体参数Table 1. Thermodynamic parameters of PBX8701 and physical parameters of wall采用本模型计算得到点火至壳体破裂过程的炸药反应度曲线,如图10所示。壳体破碎时刻,1和3 mm约束厚度装药反应度分别为33.74%和46.96%。可以看出,底部尺寸越薄的结构约束强度越小,装药点火后自增强燃烧速率增长越慢,最终反应度越低,装药释放总能量也越小。
需要解释的是,实验选用的QSY压力传感器的有效量程为0~1 GPa,实际装药反应压力峰值超过传感器的最大量程,压力-时间曲线均出现了截顶现象,因此,无法对压力峰值进行定量对比。此外,由于壳体破碎的随机性,筒体侧壁不同测点的运动速度存在一定差异。
针对约束体厚度为1和3 mm的装药反应演化过程,对比实验测量与理论模型计算得到的内部压力和壳体膨胀速度,如图11~12和表2所示,由于惯性约束效应,壳体破裂后存在约束作用,导致内部装药继续反应,压力持续上升,壳体碎片在高压气体的推动下做加速运动,壳体的运动惯性约束效应对点火后炸药反应的后续成长影响显著,本文中模型有效弥补了理论计算[11]精度不足的问题,可为后续约束装药安全性评估提供基础。导致模型产生误差的原因是,目前模型采用的理想弹塑性本构模型未考虑圆柱壳体在z轴方向的伸长变化等因素,后续工作将进一步完善模型及壳体变形过程。
表 2 约束体厚度为1和3 mm时实验与理论模型壳体膨胀速度的对比Table 2. Comparison between the experimental data and calculating results of wall velocity of the steel case with 1 and 3 mm wall thickness约束体厚度/mm 壳体膨胀速度/(m·s−1) 误差/% 精度提高/% 实验峰值 模型峰值 无惯性 1 202.5/177.1 197.8 91.6 4.0 47.52 3 243.6 234.2 140.1 3.9 38.63 关注装药尺寸对装药点火反应增长过程的影响,以约束体厚度1 mm为例,如图13所示,在几何相似条件下(取外半径与内半径之比K=1.78),装药直径越大,炸药点火后经历的早期高温产物气体流动和后续炸药表面燃烧导致裂纹增压扩展过程的时间越长,炸药燃烧反应初期越缓慢,压力成长越缓慢,壳体最终破坏后压力峰值越高,但壳体变形响应时间相应延后,速度峰值变大。
壳体厚度对装药点火反应增长过程的影响如图14所示。从图14可以看出,壳体越厚,结构约束越强,壳体变形越小,装药反应过程压力成长越快,但质量也越大,壳体膨胀速度成长越缓慢,速度峰值反而越低。
3. 结 论
建立了考虑壳体惯性约束效应的装药反应演化模型来描述装药反应演化、壳体膨胀与破裂后运动的耦合作用,反映出惯性约束效应在装药反应演化过程中不可忽视的影响,表明内部压力与壳体速度的变化本质是能量释放与产物气体对外做功耦合的结果,完善了机械约束下装药点火后反应演化行为的数学表征,得到以下主要结论:
(1) 惯性约束效应对壳体解体后装药反应演化过程十分重要,忽略惯性约束效应将导致模型计算得到的内部压力、壳体速度、反应度和反应速率偏低;
(2) 通过壳体膨胀速度变化等信息,模型可以反演弹内压力变化,是研究能量释放特性与做功传递关系的重要参量,同时模型可得到终态时装药的反应度与反应速率。
-
表 1 柱中点挠度
Table 1. Mid-span displacements
pp0 ymax/mm Δymax
/mmymin/mm Δymin
/mmymax/mm Δymax
/mmymin/mm Δymin
/mmymax/mm Δymax
/mmymin/mm Δymin
/mmSDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA HM150×100 HW150×150 HN200×100 0.5 11 11 0 -11 -11 0 10 11 -1 -10 -11 1 7 8 -1 -7 -8 1 1.0 22 22 0 -26 -23 -3 21 22 -1 -23 -22 -1 15 16 -1 -18 -16 -2 2.0 46 48 -2 -7 -19 12 44 46 -2 -7 -19 12 32 34 -2 -8 -18 10 3.0 82 87 -5 39 27 12 77 81 -4 33 21 12 55 58 -3 18 7 11 4.0 139 149 -10 111 108 3 127 137 -10 94 87 7 89 93 -4 56 46 10 4.5 185 * 170 * 5.0 * * * * * * 220 240 -20 214 231 -17 141 142 -1 116 103 13 5.1 * * * * * * * * * * * * 6.0 * * * * * * * * * * * * 215 * 200 * 表 2 柱中点截面边缘压应变
Table 2. Mid-span compression strains
pp0 εmax/10-4 Δεmax
/10-4εmin/10-4 Δεmin
/10-4εmax/10-4 Δεmax
/10-4εmin/10-4 Δεmin
/10-4εmax/10-4 Δεmax
/10-4εmin/10-4 Δεmin
/10-4SDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA SDOF DYNA HM150×100 HW150×150 HN200×100 0.5 9 13 -4 -9 -5 -4 8 12 -4 -8 -4 -4 8 12 -4 -8 -4 -4 1.0 18 22 -4 -20 -14 -6 18 21 -3 -17 -13 -4 17 21 -4 -18 -13 -5 2.0 67 69 -2 25 20 5 63 69 -6 22 19 3 50 60 -10 8 5 3 3.0 184 181 3 152 137 15 168 178 -10 134 134 0 127 147 -20 89 96 -7 4.0 374 377 -3 351 350 1 339 362 -23 313 326 -13 239 263 -24 205 216 -11 5.0 * * * * * * 655 1069 -414 650 1060 -410 406 444 -38 380 405 -25 表 3 柱中点截面最大应变率
Table 3. Mid-span maximum strain rates
pp0 ˙εmax/s-1 Δ˙εmax/s-1 ˙εmax/s-1 Δ˙εmax/s-1 ˙εmax/s-1 Δ˙εmax/s-1 SDOF DYNA SDOF DYNA SDOF DYNA HM150×100 HW150×150 HN200×100 1.0 1.21 0.02 1.19 1.84 0.02 1.82 1.06 0.01 1.05 2.0 3.08 3.16 -0.08 2.94 3.00 -0.06 2.81 2.80 0.01 3.0 6.22 7.00 -0.78 6.11 7.23 -1.12 5.59 7.91 -2.32 4.0 8.53 9.63 -1.10 8.34 10.2 -1.86 7.57 12.00 -4.43 5.0 10.85 11.9 -1.05 10.70 12.6 -1.90 9.84 14.10 -4.26 -
[1] 中华人民共和国住房和城乡建设部.GB 50009-2012建筑结构荷载规范[S].北京: 中国建筑工业出版社, 2012. https://www.hanspub.org/reference/ReferencePapers.aspx?ReferenceID=157206 [2] Nassr A A, Razaqpur A G. Single and multi degree of freedom analysis of steel beams under blast loading[J]. Nuclear Engineering and Design, 2012, 242:63-77. doi: 10.1016/j.nucengdes.2011.10.020 [3] LS-DYNA keyword user's manual: Version 971[Z]. Livermore, California: Livermore Software Technology Corporation, 2007. [4] Nassr A A, Razaqpur A G. Strength and stability of steel beam columns under blast load[J].International Journal of Impact Engineering, 2013, 55(5):34-48. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=258376df12a28550b207823b7b9302fb [5] 席丰, 张云.脉冲载荷作用下钢梁动力响应及反常行为的应变率效应[J].爆炸与冲击, 2012, 32(1):34-42. doi: 10.3969/j.issn.1001-1455.2012.01.006Xi Feng, Zhang Yun. The effects of strain rate on the dynamic response and abnormal Behavior of steel beams under pulse loading[J]. Explosion and Shock Waves, 2012, 32(1):34-42. doi: 10.3969/j.issn.1001-1455.2012.01.006 [6] 刘锋, 席丰.子弹撞击作用下固支浅圆拱的弹塑性动力响应[J].爆炸与冲击, 2005, 25(4):361-367. doi: 10.3321/j.issn:1001-1455.2005.04.013Liu Feng, Xi Feng. Elastic-plastic dynamic response of a fully clamped shallow arch subjected to projectile impact[J]. Explosion and Shock Waves, 2005, 25(4):361-367. doi: 10.3321/j.issn:1001-1455.2005.04.013 [7] 谭浩强.C程序设计(第四版)[M].北京:清华大学出版社, 2010. [8] Nassr A A, Razaqpur A G. Dynamic response of steel columns subjected to blast loading[J]. Journal of Structural Engineering, 2014, 140(7):165-180. http://d.old.wanfangdata.com.cn/Periodical/bpqc201804005 [9] Nassr A A, Razaqpur A G. Experimental performance of steel beams under blast loading[J]. Journal of Performance of Constructed Facilities, 2012, 26(26):600-619. http://www.wanfangdata.com.cn/details/detail.do?_type=perio&id=dbc670001ec88ef3091f049baebd1566 [10] 陈骥.钢结构稳定理论与设计(第六版)[M].北京:科学出版社, 2014. [11] 余同希, 斯壮W J.塑性结构的动力学模型[M].北京:北京大学出版社, 2002. [12] 陈绍蕃, 顾强.钢结构(第二版)[M].北京:中国建筑工业出版社, 2007. -



















 下载:
下载:





































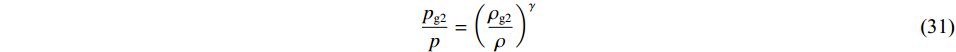






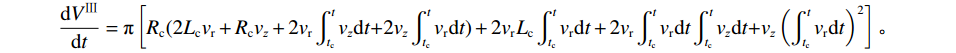












 下载:
下载: