Dynamic shear behavior and failure mechanism of Ti-6Al-4V at high strain rates
-
摘要: 采用基于霍普金森压杆的新型加载技术对Ti-6Al-4V材料的动态剪切特性及失效机理进行了测试研究。获得了Ti-6Al-4V材料在超过104 s-1应变率下的剪应力-剪应变曲线及失效参数。研究发现,材料的流动应力存在明显的应变率强化效应;随着应变率的增加,材料的失效应力逐渐增大,而失效应变逐渐减小。采用ABAQUS/Explicit对加载过程进行了数值模拟。结果显示,剪切区材料基本处于平面剪切状态,应力应变场分布较为均匀,计算得到的剪应力-剪应变曲线与实验结果吻合较好。经断口分析可知,随着应变率的升高,Ti-6Al-4V的失效机理存在由韧窝、拉伸韧窝至台阶及河流花样的演化过程,材料的失效模式主要表现为韧性断裂。Abstract: Dynamic shear properties and failure mechanism of Ti-6Al-4V were studied at strain rates in excess of 104 s-1, with a new loading method based on the split Hopkinson pressure bar (SHPB) technique. The shear stress-shear strain curves and failure parameters of Ti-6Al-4V were acquired in a wide range of high shear strain rates. It is found that the flow stress of the material shows an obvious strain rate hardening effect. With the increase of strain rates, the failure stress of the material increases gradually, while the failure strain decreases. The loading process was modeled by ABAQUS/Explicit software. The results show that the shear zone material is substantially in the state of plane shear. The tested stress-strain curves have good agreement with the simulated results. The fracture surface examination shows that with the increase of strain rate, the failure of Ti-6Al-4V is closely related to the different behaviors of dimples, and it indicates an evolution process from dimples and tensile dimples to steps and river patterns. The fracture analyses show that the failure mode of the material is mainly ductile fracture.
-
Key words:
- dynamic shear /
- double shear specimen /
- high strain rate /
- micro-mechanism
-
水下隧道经过近百年的发展,在世界范围内得到了广泛的应用,已经成为跨水交通的重要方式之一。相较于轮渡和大桥,水下隧道不受台风、浓雾等恶劣天气的影响,有稳定的通行能力,且不对水上航运造成影响,仅在20世纪全球范围内就已通过盾构技术开挖了100多条水底隧道[1]。在现代战争中,水工结构已经成为爆炸攻击的重点目标,例如在俄乌战争中北溪一号管道[2]、克里米亚大桥[3]和卡霍夫卡大坝[4]均遭受到了严重的爆炸袭击,水工结构一旦损毁,将会导致巨大的伤亡和重大的经济损失。因此,对水下隧道结构开展抗爆研究非常必要。
目前,学者们对水下隧道的抗爆性能已经开展了相关研究。Xu等[5]以港珠澳大桥沉管隧道管节为原型,通过缩尺模型试验研究了车辆在隧道内部发生爆炸后隧道结构的毁伤效应,以炸药到隧道顶板的比例距离进行分类,将爆炸破坏划分为4个等级。Kristoffersen等[6]基于挪威海岸的一个悬浮隧道工程方案,建立了圆形和矩形截面两种隧道管节的有限元模型,对隧道内部车辆爆炸的工况进行了研究,发现圆形横截面的隧道具有更好的抗爆性能。Yang等[7-8]基于数值模拟的方法,评估了水下沉管隧道在爆炸荷载作用下的损伤特征,提出了基于药量和爆炸距离的经验关系来预测隧道损伤水平的方法,并确定了CFRP(carbon fiber reinforced polymer)布最佳厚度用以加固沉管隧道的方案。De等[9-10]通过离心机模型试验和数值模拟相结合的方法,研究了不同比例爆距对水底沉埋隧道的影响,并以此评估了0.9 t炸药对直径5.5 m水底隧道的影响。虽然学者们对爆炸作用下水下隧道结构进行了一定的研究,但由于在爆炸荷载作用下水下隧道结构受力复杂,隧道结构上的荷载分布规律和动力响应尚不明确,需要进一步开展相关试验研究。
本文中,以水下隧道管节为研究对象,浇筑1/10缩尺模型,进行3次水下爆炸试验,研究水中近场非接触爆炸下隧道管节的荷载分布特征和结构的动力响应规律,以期为水下隧道的抗爆设计提供参考。
1. 试 验
1.1 试验设计
参考文献[11],结合沉管隧道设计规范[12],根据浮重比要求,适当调整了截面尺寸,同时,兼顾施工可行性和试验条件,设计并制作了1/10缩尺钢筋混凝土隧道模型,如图1所示。模型试件截面整体近似圆形,外径为1 200 mm,内径为900 mm,圆壳段壁厚150 mm。模型底部为凹形平台,平台厚200 mm,长2 400 mm,高100 mm,以增强试件的抗剪能力。
模型试件采用C45混凝土浇筑,混凝土保护层厚度为20 mm。纵筋和箍筋均采用HRB335
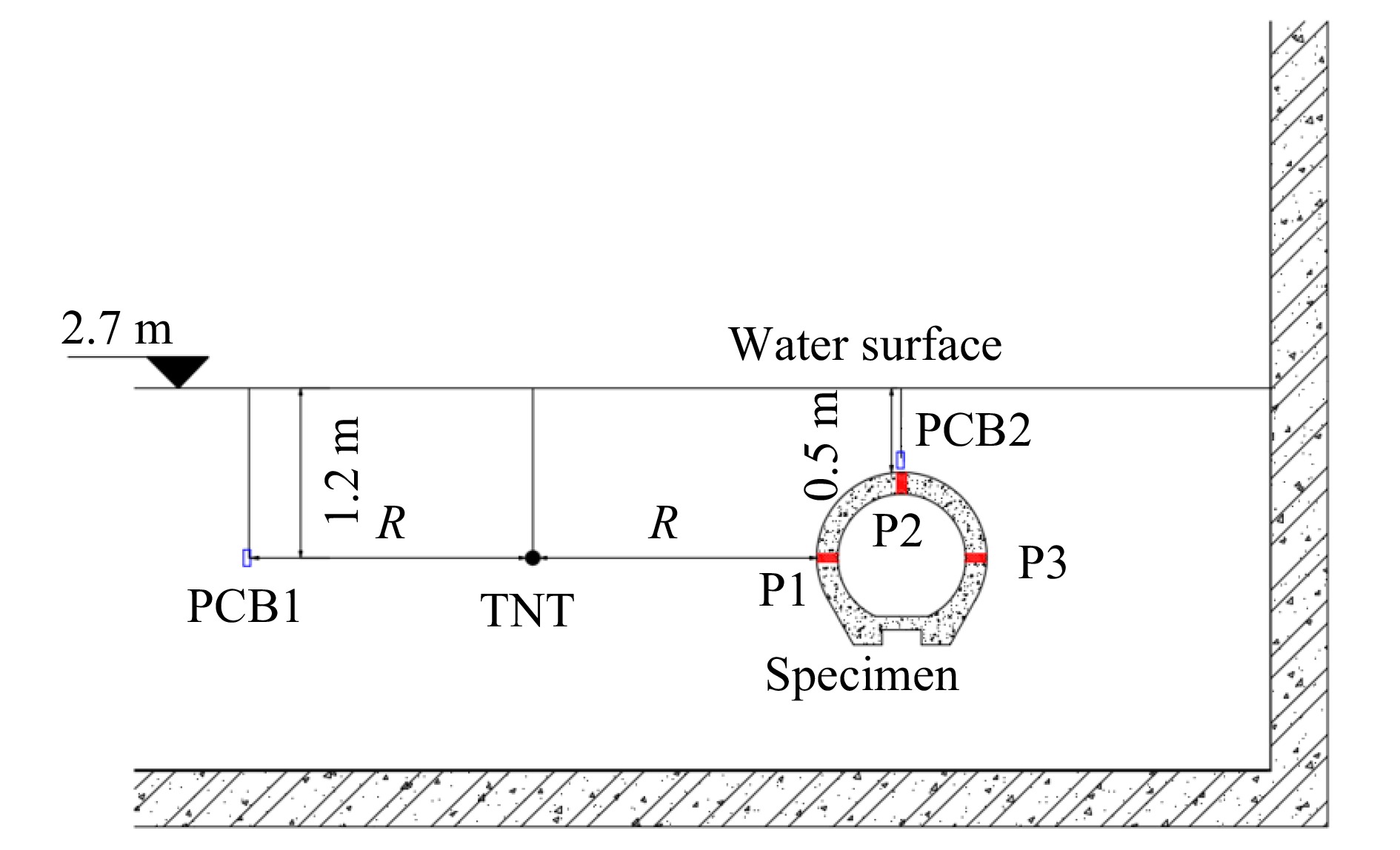
∅ 6钢筋。纵向钢筋双层布置:内侧30根,间距120 mm;外侧32根,间距126 mm。箍筋也双层布置,纵向间距100 mm,两层箍筋之间间距82 mm。同时,在两层钢筋网之间的箍筋上布置若干架立钢筋,以保证两层钢筋的整体性。为了保证试件内部不被水填充,在试件两端端口处用钢板将其密封。具体做法:通过法兰、橡胶垫和螺栓将钢板与试件连接,并在端口处涂抹耐油硅酮密封胶以保证试件内部不进水。试验在圆形爆炸试验水池中进行,如图2所示。水池直径为10 m,深10 m。在水池周围和底部设置10 mm厚的钢板,以蓄水。试验时,首先将试件水平放置在池底预先浇筑的混凝土基座上,试件底部距池底0.9 m,两端距池壁3.2 m,背爆面距池壁2 m,以减少池底和池壁反射波对试验结果的影响;然后,安装测试压力传感器和位移传感器;最后,注水入池至2.7 m深。炸药采用TNT集团装药,用绳子吊入水中,放置在试件正前面。共进行3次有效试验,试验工况如表1所示,W为装药质量,R为爆心距。
表 1 试验工况Table 1. Test conditions工况 W/kg R/m (R∙W −1/3)/(m∙kg−1/3) 1 0.2 3 5.13 2 0.4 2 2.71 3 0.4 1 1.36 1.2 仪器布置
压力传感器布置位置及安装方式如图3所示。在试件的迎爆面、管节顶面和背爆面分别安装CYG4100壁面压力传感器P1、P2和P3,其量程分别为60、60和30 MPa,以测量试件不同位置处的反射压力和绕射压力。同时,为了测量同等位置处的自由场压力,分别在与炸药距传感器P1和P2同等位置的自由水域放置压力传感器PCB1和PCB2。
位移传感器选用西安杰诚JW2C型,安装位置见图4。在试件迎爆面内侧安装3支量程50 mm的位移传感器,以测量试件的局部变形。试件中间截面放置位移传感器D2,左右两侧间距50 cm处分别放置位移传感器D1和D3。在试件背爆面中心处安装位移传感器D4,以测量试件水平方向的整体位移。试件底部中心点安装位移传感器D5,以测量试件竖直方向的整体位移。外部位移传感器D4和D5为回弹式,量程均为100 mm。数据采集使用DH5960数据采集仪,数据采样频率设为1 MHz,采样长度为2.5 s,采样延时为0.5 s,触发方式为信号触发。
2. 试验结果分析
2.1 压力
荷载分布特征是研究结构动力响应和毁伤效应的基础,通过分析试验测得的压力时程数据,讨论圆形隧道的水下爆炸荷载分布特征。
2.1.1 自由场压力
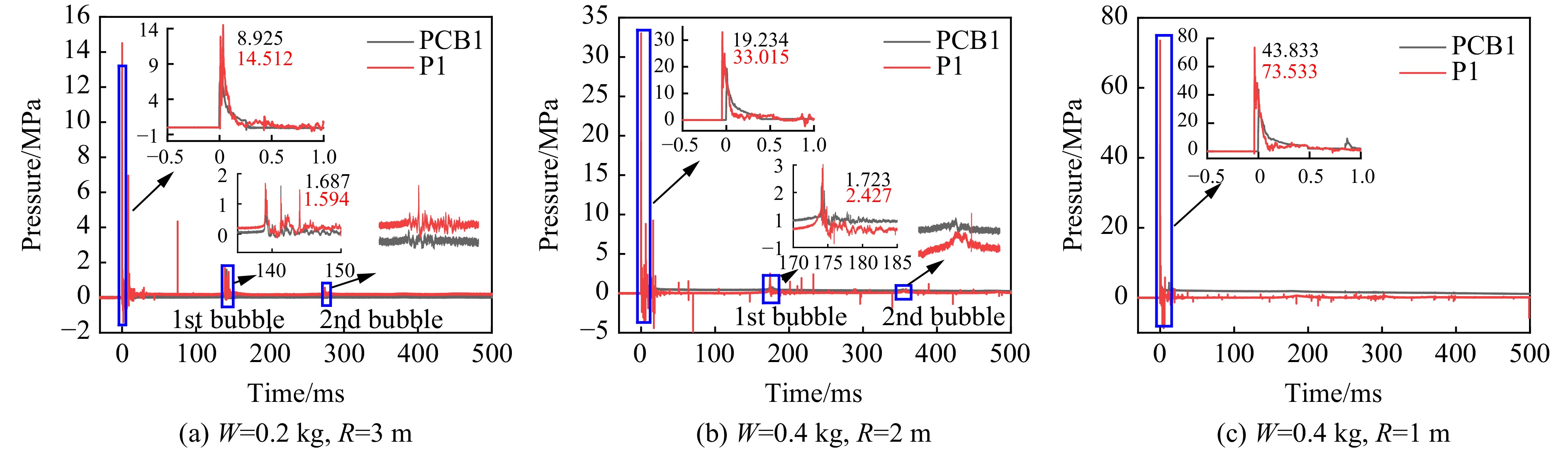
炸药爆炸后,产生的冲击波迅速在水中传播。冲击波过后,爆炸产物在水中形成的气泡在水中脉动。图5为不同工况下压力传感器PCB1和PCB2测得的水下爆炸自由场压力时程曲线。由图5可见,水下爆炸产生的冲击波具有峰值压力大、作用时间短等特点。冲击波到达测点时,压力几乎瞬间增大到峰值,然后呈指数形式衰减至零。气泡脉动压力则相对较低,仅为冲击波峰值压力的1/5左右,甚至更低。
冲击波在到达不同介质的界面时会形成反射波和透射波,两种介质的波阻抗比决定了反射波和透射波的强弱。由于空气的波阻抗远小于水的波阻抗,水中爆炸产生的冲击波在水面反射形成拉伸波。水中爆炸产生的冲击波传递到自由场压力传感器上,随后冲击波在水面反射形成的拉伸波也传播到测点,拉伸波与冲击波叠加导致冲击波压力急剧下降,即水面截断效应。从图5可以观测到明显的水面截断效应。传感器PCB2放置位置离水面更近,因此其截断效应更明显,Zhang等[13]在试验中也观测到了此现象。
对于水下爆炸冲击波压力,Zamyshlyayev等[14]基于Cole公式[15],改进了水下爆炸自由场冲击波峰值压力:
pm={4.41×107(3√WR)1.56≤Rr<125.24×107(3√WR)1.1312≤Rr<240 (1) 式中:
pm 为冲击波峰值压力,MPa;炸药质量W 的单位为kg;爆心距测点的垂直距离R 的单位为m;r 为等效球形装药的药包半径,m。将试验中测得的冲击波峰值压力与理论公式的计算值进行对比,结果如图6所示。可以看出,理论曲线和试验测点吻合较好。试验中所测得的冲击波峰值压力略高于理论计算结果,这是由于式(1)是基于球形装药,而试验中的装药为长方体,在计算时将其等效为球形装药的尺寸,因此有一定的误差。总体上,各工况下的相对误差均在20%以内,误差较小,说明可用式(1)对自由场冲击波峰值压力进行计算。
2.1.2 迎爆面压力
将压力传感P1测得的迎爆面压力时程曲线与压力传感PCB1测得的自由场压力时程曲线进行对比,如图7所示。可以看出,迎爆面压力时程曲线与自由场压力时程曲线吻合较好,二者冲击波的上升段和下降段几乎重合,冲击波均在传播到测点时骤然上升,攀升至峰值压力,随后呈指数形式迅速衰减,二者冲击波压力的持续时间几乎一致,但自由场的冲击波峰值压力明显小于迎爆面冲击波峰值压力。同时可以看出,对于爆炸产生的气泡脉动压力而言,两者几乎一致,无明显区别。自由场中的冲击波峰值压力与试件表面的反射波峰值压力对比如表2所示。可以看出,在同种爆炸工况下,试件表面迎爆面的压力峰值为自由场中的1.626~1.716倍。这是由于,冲击波在传播到隧道管壁时会发生反射,反射波和入射波在隧道迎爆面叠加,导致压力升高。这会对结构造成强烈的冲击,如果压力过高可能会导致隧道管壁产生局部压碎破坏。
表 2 不同工况下迎爆面反射波峰值压力与自由场峰值压力的对比Table 2. Comparison between the peak reflected pressure on the blast face and the peak pressure in the free field under different test conditions工况 反射波峰值压力/MPa 自由场峰值压力/MPa 两者的比 1 14.512 8.925 1.626 2 33.015 19.234 1.716 3 73.533 43.833 1.678 2.1.3 顶面压力
压力传感P2测得的试件顶面绕射波压力时程曲线与压力传感PCB2测得的临近位置的自由场压力时程曲线进行对比,如图8所示。可以看出,冲击波与试件表面的绕射波几乎同时到达,其上升段曲线也一致,但隧道表面的绕射波压力明显小于自由场中的冲击波压力,且绕射波压力时程曲线中并无明显的截断效应。在冲击波到达测点前,隧道表面受到一明显的小绕射波,该绕射波峰值压力较小,不到第2次绕射波峰值压力的50%。
自由场中的冲击波峰值压力和试件顶面的绕射波峰值压力如表3所示,可以看出,在同种爆炸工况下,试件顶面的绕射波峰值压力约为自由场中冲击波峰值压力的54.3%~65.2%。
表 3 顶面绕射波峰值压力与自由场峰值压力的对比Table 3. Comparison between the peak diffraction wave pressure and the peak free-field pressure at the top surface工况 绕射波峰值压力/MPa 自由场峰值压力/MPa 两者的比 1 5.171 7.933 0.652 2 7.626 13.774 0.554 3 11.608 21.378 0.543 2.1.4 背爆面压力
压力传感P3所测得的背爆面冲击波绕射压力时程曲线如图9所示,可以看出,其曲线特征与自由场冲击波压力时程曲线明显不同,主要体现在压力峰值较小,且压力并非瞬间到达峰值,上升段和下降段均比较平缓,冲击波作用时间相对较长。自由场中的冲击波峰值压力与试件背爆面的绕射波峰值压力的对比如表4所示,可以看出,在同种爆炸工况下,试件背爆面绕射波峰值压力为自由场中冲击波峰值压力的25.5%~31.3%。
表 4 不同工况下测得的背爆面绕射波峰值压力与自由场理论峰值压力的对比Table 4. Comparison between the test peak diffraction wave pressure and the theoretical peak free-field pressure on the back blast surface under different test conditions工况 绕射波峰值压力
测试值/MPa自由场峰值压力
理论值/MPa两者的比 1 1.769 5.647 0.313 2 2.542 9.968 0.255 3 4.158 15.223 0.273 总体上,对比同种工况下不同测点的峰值压力,不难发现,结构迎爆面测点的峰值压力最大,顶面的峰值压力次之,背爆面的峰值压力最小,且其与同等爆距下自由场峰值压力之间的比值也逐渐减小。这是由于,水中爆炸作用下冲击波在圆柱壳结构表面形成的压力荷载极其复杂。压力传感器P1在迎爆面测得的压力时程曲线为入射波和反射波的相互叠加,其峰值压力远大于自由场峰值压力;而冲击波并不能直接作用于测点P2和P3,在测点P2和P3所测得的主要为绕射波压力,测点P3相较于测点P2,冲击波绕过的外凸面范围更大,其压力的衰减也就更多,在测点P3所测得的压力与自由场压力的比值更小。
2.2 冲量
结构在爆炸荷载作用下的动力响应和损伤程度不仅受到冲击波超压的影响,而且受冲量的显著影响[16]。冲量是压力场持续作用在目标物上的时间积累量,对试验测得的压力时程曲线进行积分,即可得到爆炸作用下结构单位面积的冲量时程曲线。
Zamyshlyaev等[14]提出了可用于计算爆炸深度小于100 m的自由场水下爆炸冲量的公式:
I={pmθ(1−e−t/θ)0≤t<θpmθ[0.632+0.368ln(t/θ)]θ≤t<10θ (2) θ={0.45r(R/r)0.45×10−3R/r≤303.5(r/c)√lg(R/r)−0.930<R/r<240 (3) 式中:
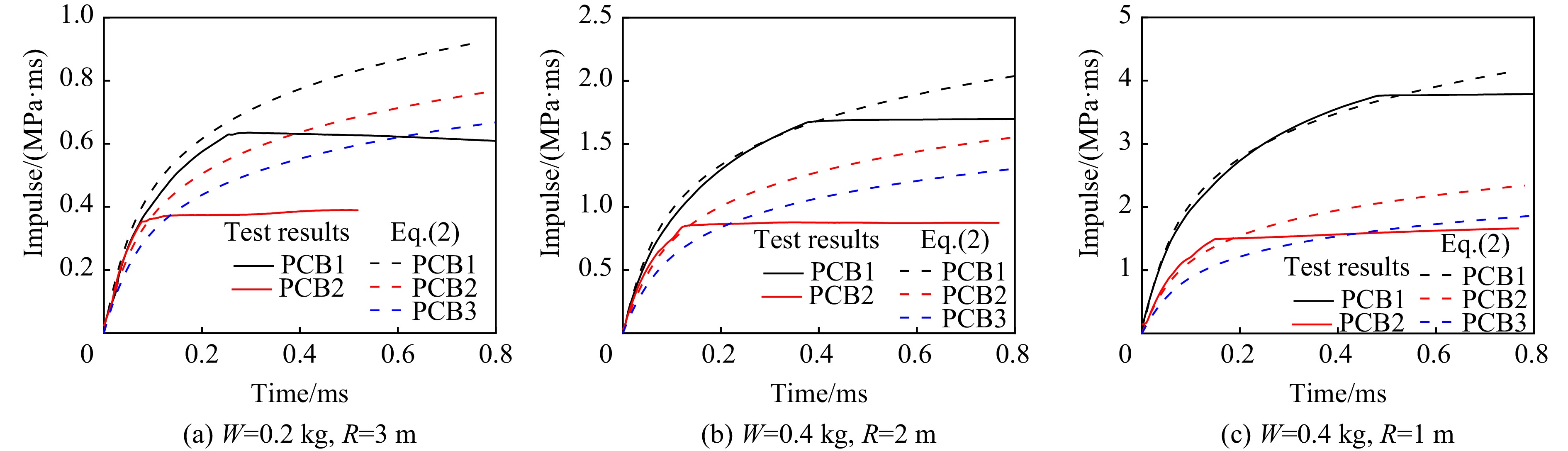
I 为冲量;t 为冲击波超压峰值后的时间;θ 为时间常数,表示从冲击波峰值压力pm 衰减至pm/e 所需要的时间;c 为水中的声速,取1500 m/s。利用式(2)仅能计算冲击波阶段的冲量,无法预估后续气泡脉动产生的冲量。对测得的自由场压力时程曲线(见图5)中的冲击波阶段进行积分,即得到不同工况下自由场的冲量时程曲线,如图10所示,并将其与式(2)的计算结果进行比较。从整体上看,自由场冲量时程测量结果与理论公式的计算结果前部分吻合良好,后部分差异较大。这是由于,试验中水较浅,传感器放置位置距水面较近,产生了明显的水面截断效应,冲击波未衰减结束其时程曲线便急剧下降,导致冲量时程曲线后半段突然变为水平直线。此外,背爆面没有安装压力传感器,仅有理论公式计算曲线,见图10中PCB3指示的曲线。随着时间的增长,冲量在增大,但显然前期增速快,后期减慢。
水下爆炸过程并非仅有冲击波的作用,气泡脉动的影响也不可忽视。为了研究气泡脉动所产生的冲量,对试验测得的冲击波压力时程曲线进行积分,即可得到整个水下爆炸过程中冲量的时程曲线。由于试验所用压力传感器均为压电式传感器,受环境影响,通常会产生零点漂移现象[17],用带有零漂的信号直接计算冲量会带来很大的误差,甚至得出完全错误的结论。因此,在对压力时程曲线积分前,要先对其使用多项式最小二乘法或滑动平均法进行修正[18]。图11为试件上不同测点的冲击波压力时程曲线和冲量时程曲线,可以看出:(1)在爆炸作用下的冲量时程曲线呈明显的阶梯状,每一次的气泡脉动都伴随着相应的冲量增加,但气泡脉动阶段所引起的冲量增加较小,冲量增加阶段主要在冲击波阶段;(2)试件背爆面受到的冲击波压力较低,但由于其作用时间较长,在试件背爆面也产生了较大的冲量,在分析时不能忽略试件背爆面的冲量。
2.3 位移
将测得的位移时程曲线进行傅里叶低通滤波,截止频率为1000 Hz,得到如图12所示的位移时程曲线(传感器压缩为负向位移,传感器伸长为正向位移),将其与测得的冲击波压力时程曲线结合分析,并标记冲击波、气泡脉动发生时间。
图12为工况1下测得的位移时程曲线。位移传感器D1距结构试件中心截面50 cm,其在冲击波到达结构试件表面时迅速变形,产生0.52 mm的变形位移,随后回弹。当气泡脉动产生的压力到达结构时,位移传感器D1再次运动,并产生整个过程中的最大变形达0.68 mm,接着在历经若干次振动之后,静止于原点。在工况1的爆炸作用下位移传感器D1产生的变形为弹性变形,并未对其造成损伤。位移传感器D2正对结构中心截面位置,其在冲击波到达结构表面时迅速变形,产生0.76 mm的变形位移,随后回弹。在位移传感器D2尚未回弹至原点时,气泡脉动产生的荷载已传播到结构表面,使得位移传感器D2再次运动,并产生1.01 mm的最大位移。在此之后,位移传感器D2继续回弹,但未回归原点,而是产生了约0.40 mm的残余位移,表明试件迎爆面中心截面位置附近产生了较小的塑性变形。
位移传感器D4放置在试件背爆面中心截面位置,其测量的是试件水平方向的整体位移。在冲击波开始阶段,位移传感器D4测量的位移曲线与位移传感器D1和D2测量的位移曲线相似。当冲击波到达结构表面时,位移传感器D4的位移瞬间达到峰值2.80 mm,随后回弹。当气泡脉动产生后,试件发生较大幅度的无规则运动,并在这一阶段形成最大负向位移4.02 mm和最大正向位移3.26 mm。在1 s左右,试件开始有规律地水平振动,并逐渐趋于稳定。
位移传感器D5放置在试件中心截面的下方,测量结构的竖向位移。在冲击波开始阶段,试件先向下运动3.85 mm,随后向上回弹2.72 mm。在试件再次向下运动的过程中,气泡脉动与试件相互作用,使试件上浮11.88 mm。气泡脉动作用结束后,试件由于自身重力作用,缓慢下沉,归于原位。
隧道结构的位移变形大致分为3个阶段。第1阶段,结构急速变形。由冲击波作用引起的结构运动变形,其变形速度极快,几乎在瞬时达到变形最大值,然后回弹,冲击波阶段引起的位移时程曲线呈V形。第2阶段,结构大幅振动。发生在第1次气泡脉动之后,此时冲击波造成的结构运动变形并未完全结束,所以第2阶段结构的运动变形往往是气泡脉动在冲击波造成的运动变形的基础上叠加而成的,位移时程曲线的最大值通常在这一阶段产生。第3阶段,结构颤动。在冲击波和气泡脉动之后,随着时间的推进,隧道振动振幅减小,隧道结构逐渐趋于稳定。可以看出,迎爆面的局部变形明显比结构的整体运动更早地达到稳定阶段。
上述分析也表明,对于裸露在水中的隧道管节,在爆炸作用下,结构整体浮动引起的失稳比结构的局部变形更严重,而结构的整体浮动则主要发生在结构大幅振动阶段。
3. 结 论
通过开展缩尺模型试验,研究了水下爆炸过程中圆形混凝土隧道管节表面的荷载分布特征和动力响应,得到的主要结论如下。
(1)总体上集团装药水下爆炸所产生的冲击波峰值压力的试验值和Cole公式的理论曲线吻合良好,试验值略高于理论值,误差在20%以内。水下爆炸的冲击波在浅水区域会产生明显的水面截断效应,该效应会使近水面的冲量减小。在水面截断效应产生之前,Zamyshlyaev冲量公式的计算结果与集团装药水下爆炸所产生的冲量测试结果吻合良好。
(2)水中爆炸作用下,隧道结构迎爆面峰值压力为自由场峰值压力的1.626~1.716倍,顶面绕射波峰值压力为自由场峰值压力的54.3%~65.2%。结构背爆面绕射压力时程曲线的上升段和下降段均相对平缓,冲击波作用时间相对较长,但峰值压力小,仅为同等爆距下自由场冲击波峰值压力的25.5%~31.3%。
(3)水中爆炸作用下的冲量时程曲线呈明显的阶梯状,每一次的气泡脉动都伴随着相应的冲量增加,但气泡脉动阶段所引起的冲量增加较小,冲量增加阶段主要在冲击波阶段。结构背爆面受到的冲击波压力较小,但由于其作用时间较长,在管节背面也产生了较大的冲量。
(4)水下爆炸过程中隧道管节的运动过程主要分3个阶段,分别为冲击波诱导的结构急速变形阶段、气泡脉动诱导的结构大幅振动阶段和结构颤振动阶段。隧道结构在气泡脉动诱导的结构大幅振动阶段产生最大位移。
-
表 1 数值模拟的主要材料参数
Table 1. Material parameters in FE simulation
部位 材料 ρ/(g·cm-3) E/GPa ν λ/(W·m-1·K-1) 入射杆 18Ni钢 8.0 190 0.3 - 试样 Ti-6Al-4V 4.43 114 0.33 6.7 透射杆 7075铝合金 2.7 70 0.3 - -
[1] BAI Y, DODD B. Adiabatic shear localization: Occurrence, theories and applications[J]. Oxford University Press, 1992. [2] LIAO S C, DUFFY J. Adiabatic shear bands in a Ti-6Al-4V titanium alloy[J]. Journal of the Mechanics and Physics of Solids, 1998, 46(11):2201-2231. doi: 10.1016/S0022-5096(98)00044-1 [3] RITTEL D, WANG Z G. Thermo-mechanical aspects of adiabatic shear failure of AM50 and Ti-6Al-4V alloys[J]. Mechanics of Materials, 2008, 40(8):629-635. doi: 10.1016/j.mechmat.2008.03.002 [4] PEIRS J, VERLEYSEN P, DEGRIECK J, et al. The use of hat-shaped specimens to study the high strain rate shear behaviour of Ti-6Al-4V[J]. International Journal of Impact Engineering, 2010, 37(6):703-714. doi: 10.1016/j.ijimpeng.2009.08.002 [5] CHICHILI D R, RAMESH K T, HEMKER K J. Adiabatic shear localization in α-titanium:Experiments, modeling and microstructural evolution[J]. Journal of the Mechanics and Physics of Solids, 2004, 52(8):1889-1909. doi: 10.1016/j.jmps.2004.02.013 [6] RITTEL D, LEE S, RAVICHANDRAN G. A shear-compression specimen for large strain testing[J]. Experimental Mechanics, 2002, 42(1):58-64. doi: 10.1007/BF02411052 [7] DOROGOY A, RITTEL D, GODINGER A. A shear-tension specimen for large strain testing[J]. Experimental Mechanics, 2016, 56(3):437-449. doi: 10.1007/s11340-015-0106-1 [8] 林艺生, 傅学金, 杨月诚.30CrMnSiA绝热剪切带显微观察与分析[J].兵器材料科学与工程, 2010, 33(6):59-61. doi: 10.3969/j.issn.1004-244X.2010.06.018LIN Yisheng, FU Xuejin, YANG Yuecheng. Microstructure observation and analysis of adiabatic shear band in 30CrMnSiA steel[J]. Ordnance Material Science and Engineering, 2010, 33(6):59-61. doi: 10.3969/j.issn.1004-244X.2010.06.018 [9] MEYERS M A, CHEN Y J, MARQUIS F D S, et al. High-strain, high-strain-rate behavior of tantalum[J]. Metallurgical and Materials Transactions:A, 1995, 26(10):2493-2501. doi: 10.1007/BF02669407 [10] 魏志刚, 李永池, 李剑荣, 等.冲击载荷作用下钨合金材料绝热剪切带形成机理[J].金属学报, 2000, 36(12):1263-1268. doi: 10.3321/j.issn:0412-1961.2000.12.008WEI Zhigang, LI Yongchi, LI Jianrong, et al. Formation mechanism of adiabatic shear band in tungsten heavy alloys[J]. Acta Metallurgica Sinica, 2000, 36(12):1263-1268. doi: 10.3321/j.issn:0412-1961.2000.12.008 [11] ROGERS H C, SHASTRY C V. Shock waves and high-strain-rate phenomena in metals[M]. Plenum Press, 1981:683. [12] FERGUSON W G, HAUSER F E, DORN J E. Dislocation damping in zinc single crystals[J]. British Journal of Applied Physics, 1967, 18(18):411-417. http://adsabs.harvard.edu/abs/1967BJAP...18..411F [13] 刘新芹, 谭成文, 张静, 等.应力状态对Ti-6Al-4V绝热剪切敏感性的影响[J].稀有金属材料与工程, 2008, 37(9):1522-1525. doi: 10.3321/j.issn:1002-185X.2008.09.004LIU Xinqin, TAN Chengwen, ZHANG Jing, et al. Influence of stress-state on adiabatic shear sensitivity of Ti-6Al-4V[J]. Rare Metal Materials and Engineering, 2008, 37(9):1522-1525. doi: 10.3321/j.issn:1002-185X.2008.09.004 [14] ZHANG Jing, TAN Chengwen, REN Yu, et al. Adiabatic shear fracture in Ti-6Al-4V alloy[J]. Transactions of Nonferrous Metals Society of China, 2011, 21(11):2396-2401. doi: 10.1016/S1003-6326(11)61026-1 [15] 苏冠龙, 龚煦, 李玉龙, 等.TC4在动态载荷下的剪切行为研究[J].爆炸与冲击, 2015, 35(4):527-535. http://www.bzycj.cn/CN/abstract/abstract9496.shtmlSU Guanlong, GONG Xu, LI Yulong, et al. Shear behavior of TC4 alloy under dynamic loading[J]. Explosion and Shock Waves, 2015, 35(4):527-535. http://www.bzycj.cn/CN/abstract/abstract9496.shtml [16] LANDAU P, VENKERT A, RITTEL D. Microstructural aspects of adiabatic shear failure in annealed Ti6AL4V[J]. Metallurgical and Materials Transactions:A, 2010, 41(2):389-396. doi: 10.1007/s11661-009-0098-5 [17] GUO Yazhou, LI Yulong. A novel approach to testing the dynamic shear response of Ti-6Al-4V[J]. Acta Mechanica Solida Sinica, 2012, 25(3):299-311. doi: 10.1016/S0894-9166(12)60027-5 [18] LONGÈRE P, DRAGON A. Dynamic vs. quasi-static shear failure of high strength metallic alloys:Experimental issues[J]. Mechanics of Materials, 2015, 80:203-218. doi: 10.1016/j.mechmat.2014.05.001 [19] 许泽建, 丁晓燕, 张炜琪, 等.一种用于材料高应变率剪切性能测试的新型加载技术[J].力学学报, 2016, 48(3):654-659. http://d.old.wanfangdata.com.cn/Periodical/lxxb201603015XU Zejian, DING Xiaoyan, ZHANG Weiqi, et al. A new loading technique for measuring shearing properties of materials under high strain rates[J]. Chinese Journal of Theoretical and Applied Mechanics, 2016, 48(3):654-659. http://d.old.wanfangdata.com.cn/Periodical/lxxb201603015 [20] XU Zejian, DING Xiaoyan, ZHANG Weiqi, et al. A novel method in dynamic shear testing of bulk materials using the traditional SHPB technique[J]. International Journal of Impact Engineering, 2017, 101:90-104. doi: 10.1016/j.ijimpeng.2016.11.012 [21] NEMAT-NASSER S. Hopkinson techniques for dynamic recovery experiments[J]. Proceedings of the Royal Society:A, 1991, 435:371-391. doi: 10.1098/rspa.1991.0150 [22] SEO S, MIN O, YANG H. Constitutive equation for Ti-6Al-4V at high temperatures measured using the SHPB technique[J]. International Journal of Impact Engineering, 2005, 31(6):735-754. doi: 10.1016/j.ijimpeng.2004.04.010 [23] 钟群鹏, 赵子华.断口学[M].北京:高等教育出版社, 2006. 期刊类型引用(1)
1. 马治权. 基于隧道开挖过程中拱顶下沉和周边收敛数据的对比分析. 价值工程. 2024(23): 95-98 .  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:













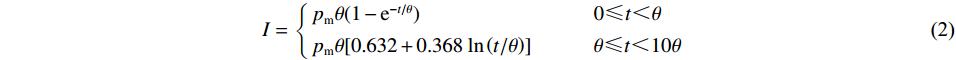











 下载:
下载:











 百度学术
百度学术