The robust non-fragile H∞ control for the combustion process in rocket motor
-
摘要: 针对某液体火箭发动机燃烧室的燃烧过程,设计了鲁棒非脆弱 H∞ 状态反馈控制器。首先,基于一种新型的时滞分割法和交互式凸组合技术,借助于构造一个包含四重积分项的Lyapunov-Krasovskii泛函(LKF),并利用新的积分不等式方法给出了LMI形式的时滞相关有界实判据;其次,在有界实判据的基础上,采用矩阵的合同变换以及变量替代技巧将燃烧过程非线性矩阵不等式线性化,通过求解线性矩阵不等式得到相应的非脆弱H∞控制器的可行解。模拟结果验证了本文设计方法的有效性。
-
关键词:
- 非脆弱 /
- 交互式凸组合技术 /
- Lyapunov-Krasovskii泛函(LKF) /
- 火箭发动机 /
- 四重积分
Abstract: In this study we dealt with the robust non-fragile H∞ controller for the combustion process in liquid propellant rocket motor chambers. In developing a less conservative H∞ performance analysis criterion, we introduced a Lyapunov-Krasovskii functional comprising quadruple-integral term. Then, based on a new delay-partitioning method, the reciprocally convex combination technique and the integral inequality approach (IIA), we formulated the bounded real criterion in terms of linear matrix inequalities (LMIs). Furthermore, based on this bounded real criterion, we translated the nonlinear matrix inequality into the linear matrix inequality by using the matrix congruent transformation and the variable substitution technique, and obtained the parameter expression of non-fragile H∞ controller by solving the feasible linear matrix inequality. The numerical examples we provided showed the effectiveness of the proposed theoretical results. -
钙质砂是富含碳酸钙或其他难溶碳酸盐类物质的一种海洋沉积物,普遍分布于我国南沙群岛珊瑚礁礁坪和潟湖表层。20世纪70年代中期,一系列岛礁工程地质性质的现场测试和室内试验中发现钙质砂的土力学性质与陆相沉积的石英砂有很大差异,主要表现为高孔隙率、高内摩擦角、低强度值和颗粒易破碎等[1]。作为岛礁上的主要岩土类介质之一,钙质砂的基本物理/力学性质、地基/桩基工程特性和动力学特性也越来越受到人们的关注[2]。对钙质砂的研究主要集中在静力学性质[3-5]和颗粒破碎[6-7]等方面,动力学研究主要是针对循环荷载的作用[8-10]以及钙质砂场地的液化特性[11-12]等。但从军事防御和灾害预防的角度,岛礁工程在爆炸、冲击和地震等荷载作用下的响应与钙质砂在高应变率作用下的动态力学特性密切相关,而国内外对钙质砂的动态力学特性的研究很少[13]。
分离式霍普金森压杆(SHPB)被认为是102~104 s−1应变率范围内研究材料动态力学特性最有效的实验技术,近年来广泛被用来研究砂土的冲击力学性能。Song等[14]利用SHPB试验技术研究了约束条件对干燥石英砂动态力学特性的影响,Brogov等[15]利用SHPB试验技术研究了细颗粒软土在应变率为103~104 s−1范围内的一维压缩特性,郑文等[16]利用SHPB试验技术研究了预压条件对干燥石英砂一维压缩特性的影响,发现应变率对干燥石英砂的压缩性能影响不大。虽然SHPB试验技术已广泛应用于动态加载下陆相沉积砂土的力学特性研究,但针对钙质砂动态力学特性的研究未见报道。
本文中,参考李英雷等[17]测量吉帕量级及以下低体积模量材料压力-体应变关系的被动围压SHPB试验方法,利用
∅∅ 100 mm SHPB装置进行了不同预压力的钙质砂在高应变率下的动态一维压缩试验,并将结果与钙质砂的静态一维压缩试验进行对比,分析预压力对钙质砂力学特性的影响以及钙质砂的静态容变关系和高压下的动态容变关系。1. 试验准备
1.1 钙质砂原样
试验所使用的钙质砂取自南海岛礁,主要成分是珊瑚和贝类的破碎沉积物。钙质砂原样含大量海水及许多大直径的珊瑚和贝类等,为控制钙质砂的均匀性,试验前先将原样自然风干,然后对原样进行筛选,保留以上粒径为0.075~2 mm的成分,经过筛分的钙质砂如图1所示,含水率为0.56%。根据《土工试验方法标准》(GB/T50123-1999)中颗粒分析试验的筛分法测得筛选后钙质砂的颗分曲线见图2。根据该规范进行钙质砂的相对密度试验,测得其最大和最小干密度分别为1.317和1.136 g/cm3。
1.2 一维压缩原理
钙质砂为散体介质,制备钙质砂试样需要对其约束成型。本文使用厚壁圆筒约束钙质砂,形成钙质砂试样在一维压缩下的应力应变状态,如图3所示,主应力σ1、σ2、σ3和主应变ε1、ε2、ε3分别为:
σ1=σx,σ2=σ3=σr,ε1=εx,ε2=ε3≈0 (1) 式中:σx和εx分别是由实验得到的钙质砂试样的轴向应力和应变,σr是对应的径向应力。
钙质砂试样在动态一维压缩下的轴向应力可由SHPB试验中透射杆上的应变数据换算得到,静态一维压缩下的轴向应力可由静态一维压缩试验中轴向力数据换算得到,但钙质砂试样的径向应力不能直接测得。为了在平面应变条件下得到一维压缩状态下钙质砂试样的径向应力,Ravi-Chandar等[18]使用弹性理论中无限长厚壁圆筒的计算方法,求得筒壁内压pi与外表面应变满足以下关系:
pi=[(ro/ri)2−1]Ecεh/2(2) 式中:Ec为厚壁圆筒的弹性模量,νc为泊松比,εh为厚壁圆筒外壁对应钙质砂试样中心位置的应变计测得的环向应变,ro和ri分别为厚壁圆筒的外径和内径。假设约束试样的厚壁圆筒处于弹性状态,则其环向应变εθ与厚壁圆筒外表面环向应变εh的关系如下[19]:
εθ=0.5εh[(1−νc)+(1+νc)(ro/ri)2] (3) 但是在试验过程中厚壁圆筒实际上是有限长的,根据Forquin等[20]的分析,处于被动围压受力状态的试样还会导致厚壁圆筒不均匀凸出变形,因此需要通过数值模拟对平均压力和体应变的测量结果进行参数修正,李英雷等[17]也采用数值模拟对金属材料的准一维应变试验进行了修正。本文中为了得到钙质砂试样中的径向应力σr,选用高强度钢制厚壁圆筒,在确保试样准一维应变和厚壁圆筒弹性变形的前提下,根据式(2)计算内筒壁压力pi,然后通过数值模拟的方法考虑该计算值与实际内筒壁压力之间折算系数k,得到钙质砂试样中的径向应力为:
σr=pi/k (4) 假设侧向压力系数
ξ =σr /σx,则试件中的最大剪应力τ和平均应力p可写成仅与轴向应力σx和侧向压力系数ξ 相关的形式:{τ=(σx−σr)/2=σx(1−ξ)/2p=(σx+2σr)/3=σx(1+2ξ)/3 (5) 1.3 钙质砂装样
本次试验制备钙质砂试样时,使用厚壁圆筒(confining ring)加上、下两个钢垫块的形式约束钙质砂试样成型,如图4所示。厚壁圆筒内径为100 mm,外径150 mm,高110 mm,使用45号钢制作并淬火。钢垫块两端平整,外径为
∅∅ 100 mm,公差为0.1 mm,高60 mm,可沿着厚壁圆筒内壁轴向自由滑动。钢垫块均使用35CrMnSiA制作,与所用100 mm SHPB装置入射杆及透射杆的材料相同。限位环(limit ring)高20 mm,内径为100 mm,主要作用是为下面的钢垫块预留一定高度,方便预压后进行SHPB试验。钙质砂试样的装样直径与厚壁圆筒的内径相同,为了进行对比,本文所有试验中钙质砂试样的初始装样密度均为1.263 g/cm3。依据图4进行装样。先在平台上依次放置限位环、下钢垫块以及厚壁圆筒,钢垫块与厚壁圆筒之间紧密接触并涂抹凡士林进行润滑,然后称取定量钙质砂利用“砂雨法”[21]进行装样,抹平钙质砂试样表面并放置上钢垫块,用橡胶锤轻敲厚壁圆筒及上面的钢垫块,边敲击边用游标卡尺测量上面的钢垫块表面的高度hs,通过多点测量控制钙质砂试样的高度,进而控制钙质砂试样的装样密度,并调整上、下两块钢垫块表面平行。
2. 试验过程
2.1 静态一维压缩试验
静态一维压缩试验和SHPB试验中的预压试验都在HUT106D微机控制电液伺服万能试验机上进行,如图5所示。为了进行对比,所有工况的加载速率均为3 mm/min,换算得钙质砂试样的应变率为2×10−3 s−1。该试验机采集到的原始数据为轴向力F(t)和位移L(t),根据钙质砂试样的横截面积As和初始高度Ls换算出钙质砂试样中的轴向应力σx和应变εx分别为:
σx(t)=F(t)/As,εx(t)=L(t)/Ls (6) 静态一维压缩过程中,钙质砂试样对应厚壁圆筒外侧的环向应变εh由该位置处的环向应变计测得,但是由于钙质砂高孔隙率、低强度值和颗粒易破碎的基本力学特性,所以钙质砂试样的可压缩性很强,这导致厚壁圆筒外部的环向应变很小。为提高应变计的信噪比,在静态和动态一维压缩试验中,厚壁圆筒外侧布置的环向应变计均使用中航电测的SB3.8-120-P-2高灵敏度半导体应变计,应变极限为6×10−3。应变采集仪为DH5922动态信号测试分析系统。静态一维压缩试验包括单调加载试验和加卸载试验,考虑到HUT106D微机控制电液伺服万能试验机的加载能力,单调加载试验的轴向峰值压力为120 MPa,加卸载试验进行了峰值压力依次为10、20、30、60、120 MPa的重复加载。
2.2 SHPB试验
SHPB试验基本过程为:SHPB系统初始静止,当子弹杆以某一速度撞击入射杆时,会在入射杆中产生一个入射脉冲εi(t),当入射脉冲到达入射杆与钙质砂试样的界面时,一部分脉冲作用在试样并透过透射杆产生一个透射脉冲εt(t),另一部分脉冲εr(t)反射回入射杆。根据一维弹性波假设和均匀性假设,可得到试样的轴向平均应力σx(t)、应变εx(t)和应变率
˙εx(t) :{σx(t)=[A/(2As)]E[εi(t)+εr(t)+εt(t)]εx(t)=(c/Ls)∫t0[εi(t)−εr(t)−εt(t)]dt˙εx(t)=(c/Ls)[εi(t)−εr(t)−εt(t)] (7) 式中:E和c分别为杆的杨氏模量和弹性波速,c=(E/ρ)1/2,A为杆的横截面积。
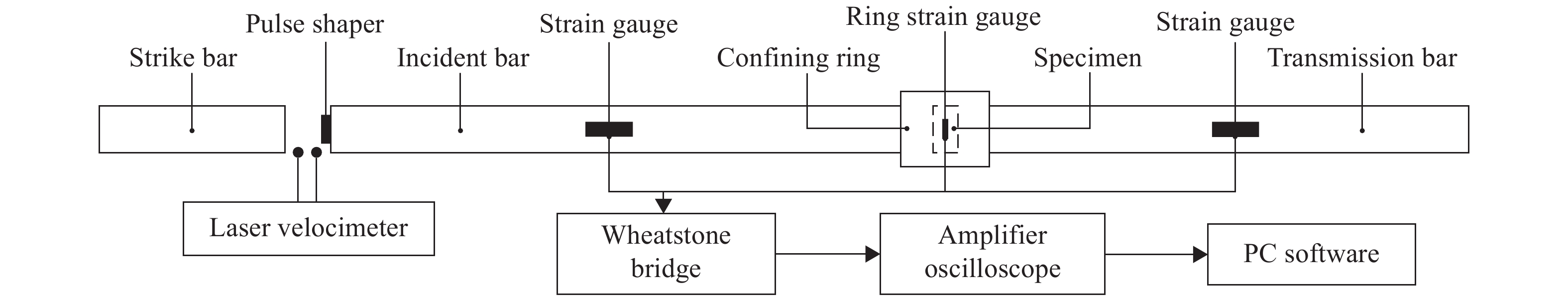
本次试验所用钙质砂含有较大颗粒的成分,为保证试样均匀性,动态一维压缩试验使用陆军工程大学爆炸冲击防灾减灾国家重点实验室的
∅∅ 100 mm SHPB系统。此外还采用了波形整形技术,如图6所示,通过延长入射脉冲的上升沿时间实现钙质砂试样的动态应力平衡。该∅∅ 100 mm SHPB系统的子弹杆、入射杆和透射杆分别长800、4 000和2 800 mm,均使用35CrMnSiA制作,密度ρ=7.85 g/cm3,杨氏模量E=218 GPa,一维应力弹性波速c=5 282 m·s−1。由于钙质砂的声阻抗远低于SHPB试验系统入射杆和透射杆的声阻抗,因此透射杆中的透射脉冲很小,为了提高信噪比,入射杆和透射杆也使用SB3.8-120-P-2高灵敏度半导体应变计并分别置于各自中点处。在进行SHPB试验前分别对钙质砂试样进行0、10、20和30 MPa的预压,经预压后的钙质砂试样再次测量高度,然后通过预留螺栓锁紧上、下钢垫块,待将钙质砂试样置于入射杆和透射杆之间之后再松开螺栓,并将上、下钢垫块分别对齐入射杆和透射杆,如图7所示。SHPB试验工况如表1所示,每个工况均至少进行一次有效重复试验。表 1 SHPB试验工况表Table 1. SHPB test table工况 质量/g 初始长度/mm 预压力/MPa 压后长度/mm 压后密度/(g·cm−3) 子弹速度/(m·s−1) 应变率/s−1 1 125 12.6 0 12.6 1.263 7.1 778 2 125 12.6 0 12.6 1.263 7.13 797 3 250 25.2 20 19.7 1.616 15.61 513 4 250 25.2 20 19.7 1.616 15.59 506 5 250 25.2 10 21.3 1.494 15.71 569 6 250 25.2 10 21.3 1.494 15.68 551 7 250 25.2 20 19.4 1.641 21.55 647 8 250 25.2 20 19.4 1.641 21.52 626 9 250 25.2 30 18.4 1.73 21.41 590 10 250 25.2 30 18.4 1.73 21.38 561 3. 试验结果分析
图8为工况10的原始波形中SHPB试验得到的原始数据为入射杆、透射杆和环向的应变时程数据。该工况是应变测量值最大的工况,其最大值接近2×10−3,而所使用应变计的极限应变为6×10−3,因此应变数据有效。图9给出了预压值分别为0、10、20和30 MPa工况下的钙质砂应力平衡状态。
3.1 预压对钙质砂压缩特性的影响
静态一维压缩试验得到单调加载和加卸载条件下钙质砂的应力应变关系,如图10所示。加卸载条件下的再次加载相当于经过预压后的加载,每次加载对应初始应变均不同,但是当再次加载超过上次加载的峰值压力后,钙质砂的应力应变关系与单调加载的试验结果完全重合。这说明在静态一维压缩条件下,当再次加载应力超过钙质砂的预压力后,钙质砂的应力应变关系与其受预压力的过程无关。
假设动态一维压缩下钙质砂也有上述特征,通过不同预压力下钙质砂的SHPB试验得到了钙质砂在高应力和高应变率条件下的应力应变关系,如图11所示,应变率范围为500~800 s−1。观察各个试验工况发现当应力超过约2倍预压力的时候,钙质砂进入较为一致的应力应变趋势,且预压力值相邻的工况之间的应力应变关系具有一定的重复性,说明钙质砂在高应力作用下的应力应变关系与前面经历的较低应力的静态加卸载过程无关。
3.2 侧压力系数
试验中厚壁圆筒使用45钢制作并淬火,屈服强度为355 MPa,而试验工况中最大的轴向应力约为200 MPa,因此可认为厚壁圆筒处于弹性状态。45钢的泊松比νc=0.3,则根据式(3)可由厚壁圆筒外表面测得的环向应变εh计算得到钙质砂试样的环向应变ε
,以工况10为例,计算得到εθ=0.34×10−3,而该工况中钙质砂试样的轴向应变εx=0.41,将其余所有工况均进行如上相同的计算并对比,发现钙质砂的轴向应变比环向应变至少大3个数量级,因此可以认为本次试验中钙质砂试样处于准一维应变状态。 在厚壁圆筒的弹性假设和试样准一维应变状态的条件下,本文中使用ANSYS中的弹性模型对厚壁圆筒进行建模。厚壁圆筒材料为45钢,体积模量为151 GPa,剪切模量为82 GPa。设置厚壁圆筒为自由边界,圆筒内壁施加不同厚度的径向均布荷载模拟试样实时厚度h。结合本次试验中钙质砂试样的厚度,模拟试验工况中取h为1.2~2.6 cm,间隔0.2 cm进行数值计算,并根据数值计算点拟合得到折算系数
k 与试样实时厚度h 的关系:k=−0.0248h2+0.2466h−0.0587 (8) 根据式(2)、(4)和(8),结合SHPB试验中各个试验工况下钙质砂试样的实时厚度h可计算钙质砂试样的径向应力σr,将其与对应工况中钙质砂试样的轴向透射应力
σ∗x 进行对比,就可得到该工况下侧压力系数ξ,以工况10的对比为例,如图12所示。根据该方法,SHPB试验的每个试验工况都可以得到一个侧压力系数ξ,将各个试验工况的侧压力系数与动态加载峰值压力的关系进行统计,如图13所示。Bragov等[22]总结大量砂土的试验数据认为在一定压力范围内,砂土的平均应力与最大剪应力的比值可认为是一定值,则结合式(3)可知侧压力系数也可以取为一定值。因此由图13的拟合结果可知本次SHPB试验的侧压力系数可取ξ1=0.495。静态一维压缩试验侧压力系数的计算方法与SHPB试验相同,经计算得到ξ2=0.38。
3.3 钙质砂静-动态压缩特性对比
对于岩土类材料,温度变化对其压力影响不大,这类介质一般称为正压性介质,因此其压力-密度关系式(也可换算为压力与体应变的关系式)即为其完整的物态方程。Tait物态方程最早由苏格兰物理学家Tait提出并用于描述液体的压力密度关系,后续学者研究发现该物态方程也适合于描述许多固体材料,其压力与体应变的一般关系形式为[23-26]:
p=p0+K0[(1−εV)−n−1]/n (9) 式中:
p0 为标准大气压,εV 为体应变,K0 是体积模量,n为材料常数。根据图10和11,结合式(3)和侧压力系数ξ1=0.495及ξ2=0.38,可以分别计算得到钙质砂在动态和静态一维压缩下的平均应力和体积应变的关系如图14所示。
结合式(9)对动态和静态一维压缩下的钙质砂的平均压力和体应变关系进行拟合,分别得到动态和静态的物态方程:
{p1=0.101+6.42[(1−εV)−5.85−1],R21=0.9952p2=0.101+5.38[(1−εV)−3.846−1],R22=0.9979(10) 对于静态一维压缩试验,在试验结果的压力范围内基本符合Tait物态方程;对于动态一维压缩,在低压范围内由于土骨架“来不及”变形,因此显示出了“屈服”的特征,土骨架屈服后,其受力变形特征又显示出“硬化”特征,此时采用Tait物态方程可以完全描述其容变关系。由图14可以看出,在相同变形的条件下,动态一维压缩下钙质砂的平均应力明显高于静态一维压缩。对于密实固体而言,一般认为其畸变律是应变率相关,而容变律是与应变率无关的[27]。但是钙质砂是高孔隙率、易破碎的颗粒材料,在强荷载作用下,钙质砂的变形主要来自于颗粒的破碎以及颗粒的滑移、滚转而导致的结构重组,而这些现象都是与颗粒运动变形速度相关的,因此钙质砂的体积压缩过程表现出应变率效应。
4. 结 论
本文进行了钙质砂的SHPB试验和静态一维压缩试验研究发现:(1)在动态或静态一维压缩条件下,当再次加载超过一定值之后可以认为钙质砂的应力应变关系与初始受压状态无关;(2)Tait物态方程可以描述钙质砂的静态容变关系及高压下的动态容变关系;(3)钙质砂动、静态容变关系对比表明钙质砂的体积压缩过程存在应变率效应。
-
表 1 不同的H
∞ 性能指标ρ,模拟给出的MADB值hMTable 1. The maximum allowable delay bound hM for a given ρ
表 2 不同的MADB值hM,仿真给出的MAPI值ρ
Table 2. The minimum allowable performance index ρ for a given hM
-
[1] CROCCO L. Aspects of combustion stability in liquid propellant rocket motors, part I: fundamentals-low frequency instability with monopropellants [J]. Journal of the American Rocket Society, 1951, 21(2): 163–178. doi: 10.2514/8.4393 [2] 钱学森, 宋健. 工程控制论[M]. 北京: 科学出版社, 1980: 343−365. [3] ZHANG Jin, PENG Chen, ZHENG Min. Improved results for linear discrete-time systems with an interval time-varying input delay [J]. International Journal of Systems Science, 2015, 47(2): 492–499. doi: 10.1080/00207721.2014.891674 [4] ZHANG X M, HAN Q L. A delay decomposition approach to delay-dependent stability for linear systems with time-varying delays [J]. International Journal of Robust and Nonlinear Control, 2009, 19(17): 1922–1930. doi: 10.1002/rnc.1413 [5] LEE W I, PARK P G. Second-order reciprocally convex approach to stability of systems with interval time-varying delays [J]. Applied Mathematics and Computation, 2014, 229(1): 245–253. doi: 10.1016/j.amc.2013.12.025 [6] 张合新, 惠俊军, 周鑫, 等. 基于时滞分割法的区间变时滞不确定系统鲁棒稳定新判据 [J]. 控制与决策, 2014, 29(5): 907–912 doi: 10.13195/j.kzyjc.2013.0120ZHANG Hexin, HUI Junjun, ZHOU Xin, et al. New robust stability criteria for uncertain systems with interval time-varying delay based on delay-partitioning approach [J]. Control and Decision, 2014, 29(5): 907–912 doi: 10.13195/j.kzyjc.2013.0120 [7] FARNAM A, REZA M E. Improved linear matrix inequality approach to stability analysis of linear systems with interval time-varying delays [J]. Journal of Computational and Applied Mathematics, 2016, 294(1): 49–56. doi: 10.1016/j.cam.2015.07.031 [8] 聂万胜, 丰松江. 液体火箭发动机燃烧动力学模型与数值计算[M]. 北京: 国防工业出版社, 2011: 25−31. [9] 李涛, 张合新, 孟飞. 火箭发动机燃烧过程无记忆鲁棒镇定的积分不等式方法 [J]. 宇航学报, 2010, 31(12): 2788–2793 doi: 10.3873/j.issn.1000-1328.2010.12.025LI Tao, ZHANG Hexin, MENG Fei. Integral inequality approach to memoryless robust stabilization of combustion process in rocket motor [J]. Journal of Astronautics, 2010, 31(12): 2788–2793 doi: 10.3873/j.issn.1000-1328.2010.12.025 [10] 惠俊军, 张合新, 周鑫, 等. 基于时滞分割方法的火箭发动机燃烧过程有记忆反馈控制 [J]. 航空学报, 2014, 35(4): 948–956HUI Junjun, ZHANG Hexin, ZHOU Xin, et al. Delay-decomposition approach to memory state feedback controller for stabilization of combustion process in rocket motor [J]. Acta Aeronautica ET Astronautica Sinica, 2014, 35(4): 948–956 [11] SHEN Yi, LIU Hao. Robust control system design for missiles based on theory of time-delay and uncertainty [J]. Acta Aeronautica Astronautica Sinica, 2011, 32(3): 473–479. doi: 10.7527/S1000-6893.2010.21379 [12] XIAO L, SAN Y, ZHU Y. Delay-dependent robust stabilization by states feedback for linear systems with uncertainties [J]. Systems Engineering and Electronics, 2013, 35(4): 802–806. [13] LIU P L. State feedback stabilization of time-varying delay uncertain systems: A delay decomposition approach [J]. Linear Algebra and its Applications, 2013, 438(5): 2188–2209. doi: 10.1016/j.laa.2012.10.008 [14] LI C F, WANG Z S, WANG Y J, et al. Robust H∞ delay control for glide vehicles via LMI [J]. Systems Engineering and Electronics, 2011, 33(9): 2060–2065. [15] SUN J, LIU G P, CHEN J. Delay-dependent stability and stabilization of neutral time-delay systems [J]. International Journal of Robust and Nonlinear Control, 2009, 19(1): 1364–1375. doi: 10.1002/rnc.1384 [16] KEEL L H, BHATTACHARYYA S P. Robust, fragile, or optimal [J]. IEEE trans on automatic control, 1997, 42(8): 1098–1105. doi: 10.1109/9.618239 [17] WU M, XIAO S P, ZHANG X M, et al. Non-fragile delay-dependent H∞ control for linear neutral systems [J]. Systems Engineering and Electronics, 2008, 30(9): 1768–1773. [18] ZHANG J H, SHI P, YANG H J. Non-fragile robust stabilization and H∞ control for uncertain stochastic nonlinear time-delay systems [J]. Chaos, Solitons Fractals, 2009, 42(5): 3187–3196. doi: 10.1016/j.chaos.2009.04.049 [19] HUI J J, ZHANG H X, KONG X Y. Delay-dependent non-fragile H∞ control for linear systems with interval time-varying delay [J]. International Journal of Automation and Computing, 2015, 12(1): 109–116. doi: 10.1007/s11633-014-0851-0 [20] RAMAKRISHNAN K, RAY G. Robust stability criteria for uncertain linear systems with interval time-varying delay [J]. Journal of Control Theory and Applications, 2011, 9(4): 559–566. doi: 10.1007/s11768-011-9131-5 [21] KIM J H. Delay-dependent robust and non-fragile guaranteed cost control for uncertain singular systems with time-varying state and input delays [J]. International Journal of Control, Automation, and Systems, 2009, 7(3): 357–364. doi: 10.1007/s12555-009-0304-7 期刊类型引用(1)
1. 付兴建,郭宏梅. 小脑神经网络用于不确定时滞系统的鲁棒非脆弱控制. 西安科技大学学报. 2020(03): 477-483 .  百度学术
百度学术其他类型引用(4)
-









 下载:
下载:

































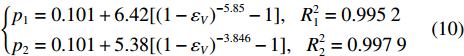

 下载:
下载:








 百度学术
百度学术