工程爆破过程所诱发的振动效应不仅影响工程本身的质量以及后续的施工和使用安全性，而且不可避免地对周围建筑物、临近边坡和施工平台产生负面影响^[1-2]。Singh等^[3]、Lu等^[4]通过研究指出振动波诱发边坡自身和周围建筑物结构动力响应与地表质点峰值振动速度、振动波传播历程以及振动频率等因素有关；同时闫鸿浩等^[5]、史秀志等^[6]、Mcgarr等^[7]通过大量边坡爆破工程实例研究发现，爆区周围环境设施因振动造成的破坏与地表质点峰值速度有着密切的相关性；因此，振动波传播过程诱发的地表质点峰值速度可作为降振研究的主要指标之一。目前对于爆破振动引起地表质点峰值速度的半经验公式，运用最广泛的是萨道夫斯基模型^[8-9]：

式中：v为质点峰值振动速度，R为测点与爆源之间距离，Q为最大段药量，K、α分别为与爆区地形、地质条件、爆破方案等相关的系数和衰减指数。顾毅成^[10]总结指出，通常规定用于数据采集的监测点具有一定控制范围及置信率，比例距离$\bar R = {\left( {{\sqrt[{\rm{3}}]{Q}}/{R}} \right)^\alpha }$在0.01～0.2范围内，超出该范围经验数据不再符合理论依据；而衰减指数α随监测点与爆源之间距离的增大逐渐减小，在爆源附近接近3，随测距增大逐渐减小趋近于1。

通过大量工程实例验证，运用萨氏公式预测地表质点峰值振动速度时，只有在同一高程的平整地形下具有较高精度；如存在较大高程差以及地表起伏明显的情况下，实测数据与预测数据之间存在较大误差。因此，陈均等^[11]、Mcgarr等^[12]、陈明等^[13]对高程影响振动峰值速度进行研究，并指出随高差正向增大振动加强，反之降低，具体数学模型为：

式中：H为测点与爆源之间的高程差，β为高程影响指数。公式(2)中虽考虑了高程对峰值振动速率的影响，但未说明各系数的相互变化关系以及这些参数之间的相互影响，且没有进行无量纲处理。

朱传统等^[14]通过试验研究指出，高程放大效应并非完全随着高程差的增大而增强，还与质点距爆源的水平距离以及岩性因素等有关，且给出如下半经验公式：

杨珊等^[15]、Yan等^[16]考虑到振动波传播过程中的高程放大效应，对萨氏公式进行修正，增加了高程影响因子，给出质点振动峰值速度模型如下：

式中：L为测点与爆心之间的水平距离。

1.   基于无量纲化改进高程影响的振动峰值速度模型

1.1   无量纲化振动速度模型

边坡抛掷爆破过程中测点与爆源之间的距离、高程差、岩体介质的物理和力学参数、炸药性质、爆破工艺参数等都会引起振动波在介质中传播的衰减或者出现高程放大效应^[17-18]。因此，可将引起振动波峰值速度变化的物理量总结为14个，具体如下。工艺参数：最小抵抗线，W；漏斗半径，r；距爆源距离，R；药包半径，r₀。介质参数：介质密度，ρ₀；振动波波速，c；地表振动频率，f；地表振动位移，μ；极限抗拉强度，σ_t；极限抗压强度，σ_c；极限抗剪强度，σ_τ。炸药参数：炸药密度，ρ₁；爆轰波速度，D；最大段装药量，Q。

依据量纲分析π定理，取c、R、Q为独立量纲，将影响振动波传播峰值速度的函数模型用15个独立参量组合成的12个无因次数组π_i之间的函数关系来表示：

因此，地表质点峰值速度函数关系式可表示为：

从式（6）可以看出，众多参数影响爆破振动质点峰值速度的传播，由无量纲分析法组建的参变函数也十分复杂，要构建包含所有影响参数的振动峰值速度模型十分困难；在实际工程中，同一工程同一地区往往要进行多次爆破，才能得出爆破振动速度的计算经验公式。在这种情况下，不同次爆破中使用的炸药种类、装药密度和岩体特性变化不大, 因此式（6）可简化为：

由无量纲理论可知不同无因次量π的乘积和商比仍为无因次量，取π₁、π₂进行商比运算得${{{\textit{π}} }_{12}}{=}{{{{\textit{π}} }_{2}}}/{{{{\textit{π}} }_{1}}={r}/{W}\;}\;$，代入式（7）组合得：

岩土爆破理论中将${{{\textit{π}}}_{12}}={v}/{W}\;$称为爆破作用指数，该物理量决定爆后岩体块度、爆破漏斗大小以及抛掷距离；在炸药种类不变的条件下，装药半径和装药量之间存在三次方函数关系式（${{r}_{0}}\cong \sqrt[3]{Q}$），刘殿中^[19]指出爆破作用指数和最小抵抗线反映装药量的多少（$Q=K{{W}^{3}}(0.6\;{{n}^{3}}+0.4)$，标准抛掷爆破单位体积炸药消耗量）；因此峰值速度可用2个物理量表示为：

式中：n为爆破作用指数。

将式(9)按照指数函数泰勒展开化简可得：

1.2   边坡抛掷爆破振动峰值速度模型

设边坡抛掷爆破台阶面监测点距炮孔孔底垂直深度为H、堵塞长度为H₁、装药长度为H₂，将装药段划分为无数个微元体，每个微元体长度为dY，则每个微元体装药量${\rm{d}}Q = \rho {}_1{\rm{d}}Y$，具体药包起爆示意图见图1。药包起爆后，每个药包微元体使得地表质点A产生的峰值振动速度为：

图  1  装药结构平面图

Figure  1.  Plane diagram of loaded constitution

下载: 全尺寸图片 幻灯片

炸药起爆后引起的岩体介质应力波传播速度远小于爆轰波传播速度，炮孔内各微元体药包先后引起地表质点振动速度十分复杂。因此，构建地表质点振动峰值速度模型时，假设各微元体药包对地表质点造成的速度时间步长和方向的差异可忽略，该情况下整个炮孔内炸药引起的地表质点振动峰值速度表示为：

对式(12)积分计算可得边坡抛掷爆破引起的地表质点振动峰值速度模型为：

2.   工程实例

2.1   工程概况及测振试验说明

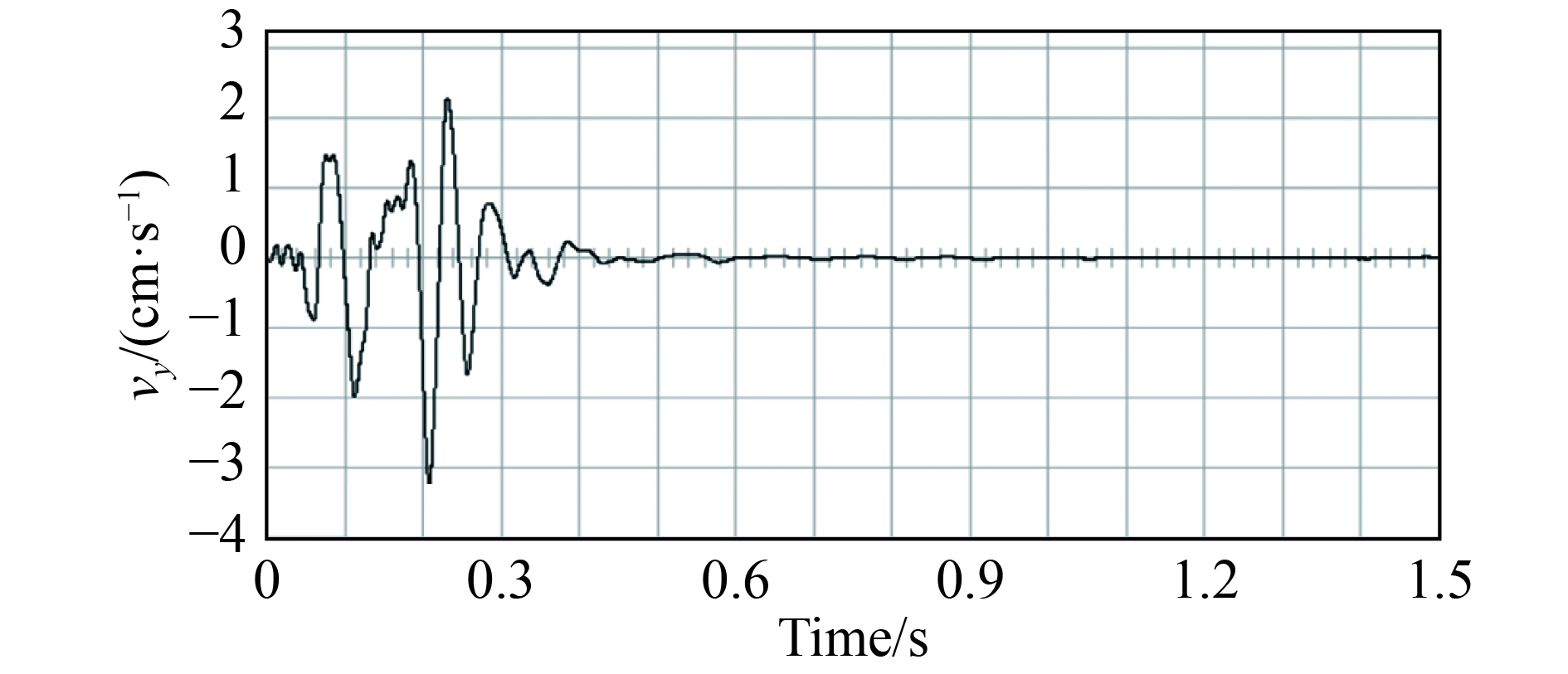
某施工平台临近高陡边坡，紧接最终边帮的交接位置，岩层较为破碎，受前期爆破振动的影响，台阶稳定性较差。为保证安全生产、确保最终边帮的稳固以及提高生产效益和经济利益，现对距爆源不同距离监测点的振动波传播规律以及坡面和坡体振动波诱发的高程放大效应进行研究。此次施工采用爆破测振仪对爆区周围地表质点进行测振试验，在3处不同位置布置监测，分别记为A、B、C，其中A组和C组分别布置于不同高度的边坡坡体，B组布置于和A组相同高程的同一边坡坡面处，且每组安装4个监测点，监测点布置平面图如图2所示。具体试验参数见表1，表1中${v_{{{x}},\max }}$、${y_{{{y}},\max }}$和${v_{{\textit z},\max }}$分别为监测点水平(x轴)、垂直(y轴)和z轴方向的地表振动速度。监测点C4实测振动波速度传播规律如图3～5所示。

图  2  监测点布置平面图

Figure  2.  The plane drawing of monitoring points layout

下载: 全尺寸图片 幻灯片

表  1  试验参数

Table  1.  Test parameters

测点编号	Q/kg	L/m	H/m	vx, max/(cm·s−1)	vy, max/(cm·s−1)	vz, max/(cm·s−1)	H2/m	H1/m
A1	575	291.8	71	0.179 2	0.233 4	0.153 6	5	2
A2	575	301.4	88	0.158 0	0.201 2	0.147 4	5	2
A3	575	311.2	105	0.136 7	0.177 1	0.135 1	5	2
A4	575	320.9	122	0.110 3	0.152 4	0.128 7	5	2
B1	575	279.4	71	1.973 6	2.177 6	1.740 2	5	2
B2	575	288.6	88	1.692 2	1.746 2	1.583 7	5	2
B3	575	298.1	105	1.313 5	1.452 1	1.395 2	5	2
B4	575	307.7	122	1.009 7	1.282 2	1.316 7	5	2
C1	975	176.19	22	2.583 8	2.207 7	1.913 2	9	4
C2	975	178.28	37	2.671 5	2.508 3	2.084 6	9	4
C3	975	176.26	52	2.781 4	2.835 1	2.194 6	9	4
C4	975	177.42	67	2.943 3	3.221 7	2.284 6	9	4

下载: 导出CSV 

| 显示表格

图  3  监测点C4水平方向地表振动速度

Figure  3.  Surface vibration velocity in horizontal direction at the measured point C4

下载: 全尺寸图片 幻灯片

图  4  监测点C4垂直方向地表振动速度

Figure  4.  Surface vibration velocity in vertical direction at the measured point C4

下载: 全尺寸图片 幻灯片

图  5  监测点C4 z轴方向地表振动速度

Figure  5.  Surface vibration velocity in z direction at the measured point C4

下载: 全尺寸图片 幻灯片

对表1中A、B组各监测点实测数据分析可知，当监测点与爆源之间的水平距离和高差分别以相同步长增量增大时（水平增量约为10 m，垂直增量约为17 m）：(1)地表监测点三轴振动速度都呈递减趋势，说明随引爆距离的增大地表质点振动效应呈衰减趋势；(2)B组测点振动速度衰减程度明显高于A组测点，说明爆破振动速度主要沿边坡面衰减，而在坡体内衰减不够明显。实测三轴振动速度数据随测点距炮孔水平距离增大的变化趋势如图6～7所示。

图  6  地表x、y向峰值振速随测点距炮孔水平距离的变化

Figure  6.  Peak vibration velocities in x and y directions of the surface varying with horizontal distances of measuring points away from blasting holes

下载: 全尺寸图片 幻灯片

图  7  地表z、y向峰值振速随测点距炮孔水平距离的变化

Figure  7.  Peak vibration velocities in z and y directions of the surface varying with horizontal distances of measuring points away from blasting holes

下载: 全尺寸图片 幻灯片

通过对表1中C组各测点实测数据分析可知，当监测点与爆源之间水平距离约一致时，随高程差值正向增大（步长增量相同）：(1)地表质点三轴方向振动速度都有增大趋势，出现高程放大效应；但增大程度不一致，高程放大效应主要由垂直方向（y轴）振动速度所决定，与文献[17]研究所得结论一致。(2)由于C组监测点布置越来越靠近坡顶角，因此出现质点振动速度放大现象部分原因是尖端放大效应所致，但根据整体放大趋势可判断主要原因是出现了高程放大效应。实测三轴振动速度数据随测点高程变化趋势如图8～9所示。

图  8  地表x、y方向峰值振速随测点高程差的变化

Figure  8.  Peak vibration velocities in x and y directions of the surface varying with elevation differences of measuring points

下载: 全尺寸图片 幻灯片

图  9  地表z、y方向峰值振速随测点高程差的变化

Figure  9.  Peak vibration velocities in z and y directions of the surface varying with elevation differences of measuring points

下载: 全尺寸图片 幻灯片

2.2   实测数据、振动速度拟合结果分析

通过对本文实测数据变化规律分析发现，地表质点振动大小、高程放大效应以及振动波衰减性能等主要由振动波垂直方向（y轴）的速度所决定；因此将各测点实测垂直方向峰值振动速度数值分别代入萨道夫斯基公式（1）、萨道夫斯基修正公式（2）～（4）以及本文通过无量纲分析所得的边坡抛掷爆破诱发地表质点峰值振动速度公式（13）进行非线性回归法拟合运算。由于振动波在坡面和坡体内传播过程中数值差异性较大，如果将坡面和坡体数据综合在一起进行拟合分析，得到的拟合结果势必误差较大；因此，为保证最终结论的准确性，对坡面和坡体实测数据分别进行非线性回归拟合计算，最终拟合结果以及实测值与拟合预测值之间的误差见表2，表2中$v_{{{y}},\max }^{(i)}$为拟合值，${\varepsilon ^{(i)}}$为相对误差值，${\varepsilon ^{(i)}} = \left.{{\left| {{v_{{{y}},\max }} - v_{{{y}},\max }^{(i)}} \right|}}\right/{{{v_{{{y}},\max }}}}$，上标i对应正文的公式编号。

表  2  实测与预测峰值速度对比表

Table  2.  The peak velocity contrast table of measured values and predictive values

测点编号	vy, max/ (cm·s−1)	公式（1）			公式（2）			公式（3）			公式（4）			公式（13）
		$v_{{y},\max }^{(1)}/$ (cm·s−1)	${\varepsilon ^{(1)}}/$ %		$v_{{{y}},\max }^{(2)}/$ (cm·s−1)	${\varepsilon ^{(2)}}/$ %		$v_{{{y}},\max }^{(3)}/$ (cm·s−1)	${\varepsilon ^{(3)}}/$ %		$v_{{{y}},\max }^{(4)}/$ (cm·s−1)	${\varepsilon ^{(4)}}/$ %		$v_{{{y}},\max }^{(13)}/$ (cm·s−1)	${\varepsilon ^{(13)}}/$ %
A1	0.233 4	0.299 1	28		0.259 9	10		0.322 1	11		0.292 7	46		0.255 2	10
A2	0.191 2	0.248 7	30		0.237 8	24		0.229 2	20		0.240 0	26		0.175 6	8
A3	0.157 1	0.214 1	36		0.221 5	41		0.173 4	15		0.208 9	33		0.129 5	18
A4	0.132 4	0.172 8	31		0.291 5	49		0.130 1	2		0.190 7	44		0.092 2	30
B1	2.177 6	1.458 9	33		1.418 5	35		2.655 9	22		1.546 1	29		1.869 8	14
B2	1.846 2	1.253 4	32		1.229 9	33		1.510 5	40		0.992 6	46		1.769 4	4
B3	1.552 1	1.077 9	31		2.216 4	37		1.184 1	24		1.034 9	32		1.470 3	5
B4	1.382 2	0.944 5	32		0.943 1	32		0.946 8	32		0.706 3	49		1.451 3	5
C1	2.207 7	3.047 3	62		2.896 0	31		2.902 4	31		3.404 8	54		2.633 6	2
C2	2.508 3	2.958 7	18		3.013 9	20		2.672 9	21		3.366 7	34		2.574 7	3
C3	2.835 1	2.924 6	3		3.129 3	10		2.571 3	9		3.383 2	19		2.516 1	11
C4	3.221 7	2.811 2	13		3.315 9	3		2.374 5	26		3.269 1	15		2.438 6	24

下载: 导出CSV 

| 显示表格

对公式（1）进行非线性回归拟合结果为：坡体，k=24.17，α=1.161，误差值分布在3%～62%范围内，平均误差为27.63%；坡面，k=67.67，α=1.071，误差值分布在31%～33%范围内，平均误差为32%。

对公式（2）进行非线性回归拟合结果为：坡体，k=3.616，α=1.161，β=0.370，误差值分布在3%～49%范围内，平均误差为23.5%；坡面，k=53.48，α=1.241，β=0.195，误差值分布在32%～35%范围内，平均误差为34.25%。

对公式（3）进行非线性回归拟合结果为：坡体，k=292.71，α=1.743，β=0.286，误差值分布在2%～31%范围内，平均误差为16.88%；坡面，k=181.67，α=1.388，β=0.104，误差值分布在22%～40%范围内，平均误差为29.58%。

对公式（4）进行非线性回归拟合结果为：坡体，k=22.81，α=1.225，β=0.747，误差值分布在15%～54%范围内，平均误差为33.88%；坡面，k=274.4，α=1.516，β=0.922，误差值分布在29%～49%范围内，平均误差为39%。

如果将本文无量纲分析理论推导出的普通标准爆破峰值速度公式（10）中的爆破作用指数n取1，该公式将转换成萨道夫斯基模型，所以借鉴萨道夫斯基模型，将公式（13）中爆破作用指数n取1，这样既使得本文中给出的模型公式更加简化，也不至于对分析结果有太大的影响；因此爆破作用指数n=1，则β将从公式中消去。为研究方便减少计算工作量，假设公式（13）中爆破作用指数按标准抛掷爆破n=1取值。对公式（13）进行非线性回归拟合结果为：坡体，k=139.31，α=1.492，误差值分布在2%～30%范围内，平均误差为13.25%；坡面，k=980.12，α=2.502，误差值分布在4%～14%范围内，平均误差为7%。

3.   结　论

(1)当监测点与爆源之间的距离正向增大时，地表质点振动效应呈衰减趋势，爆破振动速度主要沿边坡面衰减，而在坡体内衰减不够明显；当监测点与爆源之间的水平距离约一致，且其随高差正向增大时，高程放大效应表现明显，该效应主要由垂直方向振动速度所决定。

(2)经无量纲理论π定理分析给出同一地质条件下边坡抛掷爆破所引起的地表质点峰值振动速度与炸药性能、测点与爆源之间的距离、炮孔装药深度、堵塞长度、爆破作用指数等因素有关，其计算模型可表示为：

(3)采用非线性回归拟合法将实测峰值速度数据分别代入萨氏公式(1)、3个常用萨氏修正公式(2)～(4)以及本文中通过无量纲理论推导出的速度公式(13)进行非线性回归运算，得到坡表面和坡体内实测值与各峰值速度公式预测值之间平均误差分别为32%、34.25%、29.58%、39%、7%和27.63%、23.5%、16.88%、33.889%、13.25%。

(4)本文中所得峰值速度模型(13)可运用于地表起伏明显或存在较大高程差地形条件的边坡抛掷爆破工程中，如若施工环境为平整地形或要求对起伏地形峰值速度寻求简化预判，可将公式(13)中爆破作用指数取1。