Temporal and spatial distribution models of explosive cloud diffusionand their characteristics
-
摘要: 针对爆炸烟云扩散的时空分布问题,在严格限定实验条件的前提下,设计并开展了不同当量、不同地面条件、不同炸药装置壳体厚度的3组TNT爆炸烟云扩散外场实验,获得了不同实验条件下烟云扩散时空分布数据,运用计算流体力学(computational fluid dynamics, CFD)对爆炸烟云的扩散过程进行了数值模拟。通过分析实验和数值模拟获取的烟云时空分布数据,得到了烟云扩散形态演变过程及浮力流场信息,确定了厚水泥地面条件和硬质地面条件下不同的爆炸烟云扩散高度分布模型。研究结果表明:采用实验与数值模拟相结合的方法来表征烟云时空分布模型是科学可行的,爆炸烟云高度随时间呈1/2次幂函数增长,烟云宽度短时间内几乎呈线性扩展,烟云温度随时间呈反比例函数衰减,爆轰地面条件和炸药装置壳体厚度对烟云扩散高度均有不同程度的影响。Abstract: In order to solve the problem of temporal and spatial distribution of explosive clouds, three groups of TNT explosion cloud field experiments were designed and carried out under the premise of strictly restricting the explosion conditions. The experiments obtained the spatiotemporal distribution of cloud under different experimental conditions. The diffusion process of the explosive cloud was simulated by computational fluid dynamics (CFD). By analyzing the space-time distribution data from the cloud experiments and simulations, the evolution process of the cloud and the information of the buoyancy flow field were obtained, and the diffusion height distribution models of different clouds were determined under the conditions of thick cement floor and hard ground. The results show that the combination of experiment and simulation can be used to characterize the spatial and temporal distribution model of the cloud. The cloud height of the explosion increases by 1/2 power function with time. The cloud width increases linearly with time in a short time. The temperature of the cloud attenuates in inverse proportion to time. The ground conditions for the detonation, and the shell thickness of the explosive device can affect the diffusion height of the explosive cloud in different degrees.
-
炸药爆炸后的烟云扩散是常见的物理现象[1]。对炸药爆炸的研究,往往偏重于炸药的性能、威力和毁伤效应,大多只关注炸药爆轰参数及火球膨胀尺度。事实上,深入开展爆炸烟云扩散规律的研究不仅可以反演炸药爆炸当量、威力、爆炸火球大小等信息,实现战场爆炸或事故爆炸侦测,而且还能推测炸药所驱动的污染物的扩散信息,预测烟云高度、体积以及污染物含量等源项参数。总之,开展烟云时空分布规律的研究对战场爆炸侦测、脏弹袭击与核武器化学爆炸事故源项反演、后果评价以及常规爆炸事故污染物扩散评估等都有重要意义。
郑毅等[2]采用气象模式对中尺度烟云扩散进行了数值模拟,没有对小尺度烟云进行讨论,也没有建立扩散模型。李晓丽等[3]开展了爆炸烟云实验和变密度法数值模拟,但实验测得的烟云边界模糊且时间过短(仅15 s),数值计算模型过于简化。王善强等[4]分析了脏弹烟云扩散特性,认为经验公式适用范围有限。Makhviladze等[5] 、Kansa等[6] 、Kanarska等[7]及Mishra等[8]都开展过浮力烟团相关理论研究,大多属纯理论计算,没有建立具体的通用模型。近年由于脏弹袭击风险增大,Thielen等[9]、Sharon等[10]及Leblel等[11]进行了少量的爆炸实验以寻找烟云扩散相关规律,并根据实验结果提出了一些拟合模型,有关这些实验的具体细节报道较少,实验过程没有严格限定烟云扩散条件,也没有通过扩散机理分析和理论模型计算加以讨论。目前爆炸烟云污染评估都是直接采用Church经验公式H=93M0.25来计算烟云的最终高度,但Church公式不能描述烟云在扩散过程中的时空分布[12]。事实上Church公式主要由53 kg TNT以上炸药爆炸及核爆炸的实验结果拟合得到,没有考虑爆炸条件本身的差异和外在气象因素的影响,拟合的幂函数系数过大、幂次过小,后期大量实验显示该公式对小于100 kg TNT的小尺度爆炸烟云爆高计算误差较大[9-10]。
综上所述,目前通过实验或数值模拟开展的烟云扩散规律的研究各存利弊,因此本文中拟开展多组不同条件下爆炸烟云实验,并结合数值模拟对爆炸烟云扩散模型及其特性进行系统研究,分析烟云的扩散机理,寻找扩散参数的变化规律,以期建立不同条件下爆炸烟云扩散通用模型。
1. 爆炸烟云扩散时空分布理论与实验
1.1 烟云扩散模型的物理描述
根据SCM(source characterization model) [13],高能炸药的爆炸过程可以划分为爆轰、浮力烟团上升、大气散布3个阶段,具体如图1所示。
第1阶段是炸药爆轰阶段。高温、高压的爆轰产物瞬间迅速向周围膨胀,使得爆轰瞬间所达到的温度、压力不断下降,当压力下降到空气压力p0时,由于惯性效应,爆轰产物还要过度膨胀,直到速度基本为零[1],此时爆炸产物密度远低于空气密度。该过程非常复杂,与炸药种类、装置构型、地表状况、起爆方式等因素相关,对该过程开展精准研究非常困难[10]。烟云膨胀到最大时刻将形成一个固定形态与体积、低密度、高温、气体产物为主(含一定颗粒物)、有较小翻滚上升速度的初始烟团,本文拟采用实验与经验公式计算相结合的方法确定初始烟云参数。
第2阶段是爆炸烟云扩散上升的过程,该阶段烟云扩散速度先升高后降低,扩散过程是一个复杂的流场动力学问题。此烟云抬升过程包含2个亚阶段:(1)烟云克服重力和上升阻力进行热抬升加速运动的阶段,持续几秒到十几秒,这一阶段往往还伴随少量后续燃烧化学反应;(2)热抬升结束后的减速阶段,该过程持续几十秒到上百秒。整个第2阶段空气不断进入烟团,烟团密度增大、温度降低,此时影响烟云时空分布的因素除烟云本身的体积、形态、密度、温度、粒子携带外,外在因素主要是风和大气稳定度。烟云扩散的理想状态如图2所示,烟云最终将趋于稳定状态后不再上升。
第3阶段为大气扩散阶段,烟云稳定后将在大气风场作用下沉降至下游区域。
1.2 爆炸烟云扩散实验过程
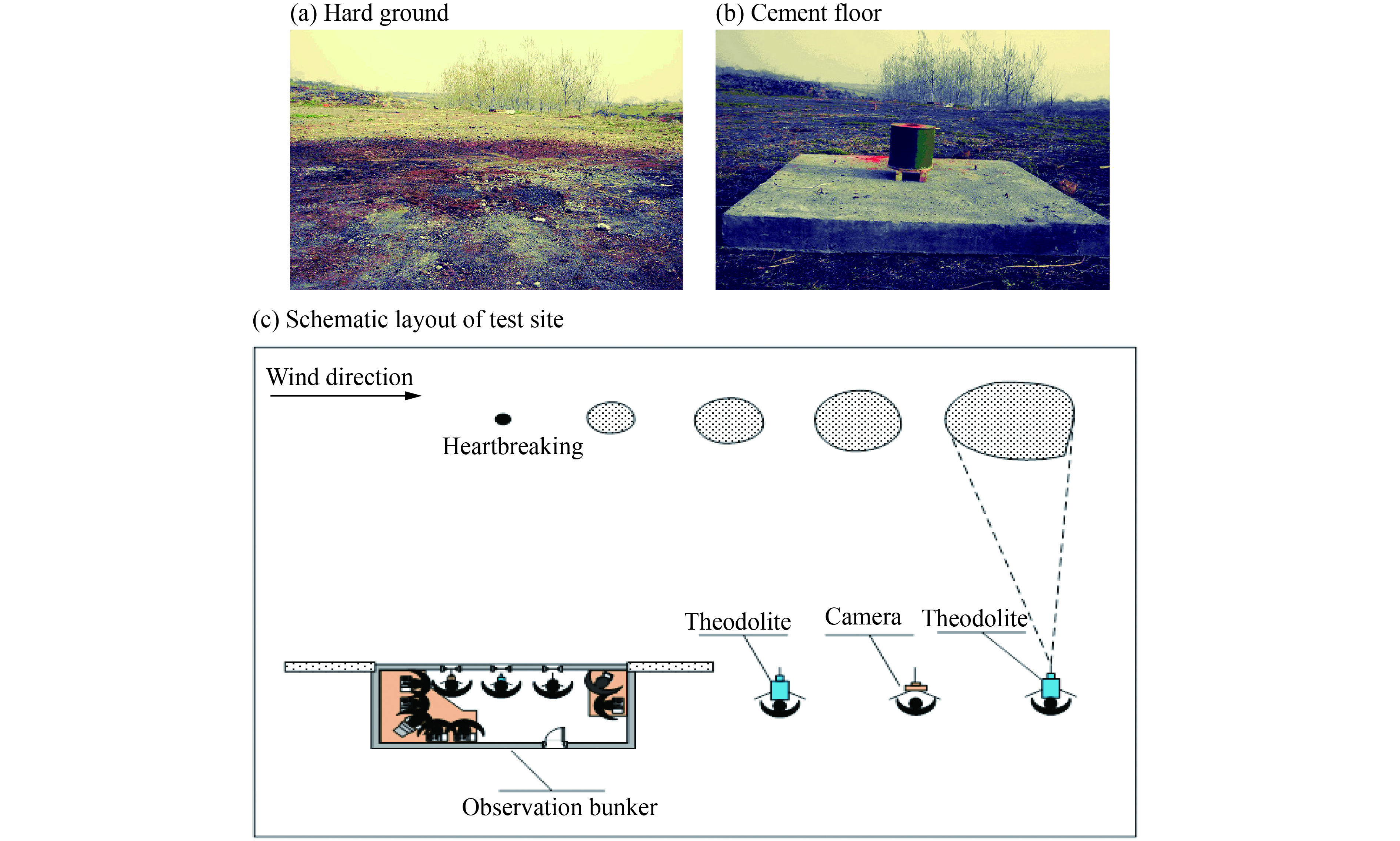
本实验属于大型外场爆炸烟云扩散实验,先后开展了不同TNT当量M(1、16、62 kg)、不同地面条件(硬质地面和厚水泥地面)、不同壳体束缚(裸装药和厚壳体)的重复实验,具体实验概况如图3所示。实验条件的变化是爆炸烟云扩散实验成败的关键[10],因此,本实验中均严格限定实验条件,以便能够得到可靠的实验数据,具体实验分组情况见表1,表1中T0为空气温度,φ为空气的相对湿度。
表 1 实验分组Table 1. Experiment groups组号 M/kg 地面条件 水平风速 炸药装置 大气稳定性 T0/℃ φ/% 1 1 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 16 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 62 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 2 16 硬质地面 无风 裸装药 基本稳定 25±3 40~60 62 硬质地面 无风 裸装药 基本稳定 25±3 40~60 3 62 厚水泥地面 无风 厚铁壳 基本稳定 25±3 40~60 首先设计了1、16、62 kg级的TNT炸药化学爆炸装置,为尽可能长时间记录烟云,在实验爆炸装置里均匀布置了少量可示踪烟云的发烟剂;然后选取实验条件理想的时刻起爆装置,同时记录爆炸烟团的初始形态及其演变过程中的动态图片和视频;最后根据视场标定结果,使用便携式计算机及其安装的专业像素分析计算软件对实验所得视频及图片信息进行计算,得到了爆炸烟云扩散的时空分布数据。
2. 爆炸烟云扩散理论方程与仿真模拟方法
2.1 烟云扩散流体动力学方程
由烟云浮力扩散机理及SCM[13]可知,炸药爆炸后生成大量高温低密度烟云,烟云主要受重力、上升阻力和大气密度差带来的浮力等影响,巨大的浮力是烟云运动的动力来源,烟云在浮力作用下快速上升,同时空气进入、密度变大、温度降低,爆炸烟云在扩散过程中遵循质量、动量和能量守恒方程。质量守恒方程一般形式:
∂ρ∂t+∂∂xi(ρui)=Sm (1) 式中:ρ为密度;ui为i方向上的速度;Sm可以为其他的自定义源项,本文中定义爆炸后的低密度烟团为流场源项。在惯性(非加速)坐标系中各方向遵守动量守恒方程,以i方向为例:
∂∂t(ρui)+∂∂xj(ρuiuj)=−∂p∂xi+∂τij∂xj+ρgi+Fi (2) 式中:p为静压,tij为应力张量,gi和Fi分别为i方向上的重力体积力和外部体积力;Fi包含了其他的模型相关源项,如多孔介质和自定义源项。烟团热量的交换遵循能量守恒传递方程:
∂∂t(ρE)+∂∂xi[ui(ρE+p)]=∂∂xi(keff∂T∂xi)−∑j′hj′Jj′+uj(τij)eff+Sh (3) 式中:E为流体微团的总能量,包含内能、动能和势能;hj′为组分j′的焓;keff为有效热传导系数;Jj′为组分j′的扩散通量。方程(3)等号右边的前3项分别描述热传导、组分扩散和黏性耗散带来的能量输运,Sh包括了化学反应热以及其他体积热源项。
2.2 烟云扩散时空分布建模方法与计算
根据烟云扩散机理可选用计算流体力学(computational fluid dynamics, CFD)方法对模型简化后进行计算。本文将初始烟团简化为椭球形、大小规整、密度温度均匀、有一定初始速度、无粒子携带,不考虑抛洒、后续燃烧化学反应的理想烟团模型[14-15],段中山等[16]对简化模型的计算结果进行了实验验证,证明该简化方法虽然不能完全仿真复杂烟云的扩散过程,但表征烟云基本时空分布、获取流场基本信息等比较可靠。计算在ANSYS/Fluent软件中编定高温低密度源项,实验测量和理论公式相结合可得半径r=1.93M0.32/(T/3 600)1/3,M为炸药当量,T为爆温(2 588 ℃),由于受地面影响火球近似呈半椭球形态,确定1、16、62 kg TNT的椭球烟团水平直径约为4.17、10.12、15.61 m,垂直高度约3.13、7.59、11.71 m。假设火球膨胀后的压力与空气压力基本相当或略低于空气压力,由ρ1=ρ0(T0/T),其中ρ1为烟团密度,ρ0、T0分别为空气密度和温度,可得烟团密度为0.08~0.12 kg/m3,烟团的组成按照TNT化学爆炸式中气体产物体积分数设定,考虑到温度迅速下降,将初始烟团均值温度设为727 ℃,且烟团具有约5 m/s的初始翻滚上升速度[17-20]。
本文中网格划分采用Gambit软件完成,网格从中间到两边、从下到上采取1.02倍递增的步进划分方式,生成的网格中间和下部网格密集以便更好地关注烟云扩散区域;设置左边为inflow、右边为outflow、底部边界选取为wall;计算中合理设置混合物耦合计算模型、湍流模式、可压缩流动、随时间变化的非定常流;重力加速度为9.8 m/s2,空气温度为25 ℃;对x向和y向速度、能量、各物相体积分数及动能能量等进行收敛设定和残差监视,残差线下行且收敛较容易,能达到计算精度要求[21]。
3. 研究结果及讨论
3.1 爆炸烟云扩散机理
烟云扩散的基本情况如图4~5所示,实验结果和模拟结果均显示烟团横截面呈蘑菇云形态。图4整体较好地记录了烟云的运动过程,但无法得到流场的具体信息。图5模拟结果则显示,对于一个最初是椭球或是圆球的烟团,由于反向水平密度梯度作用而产生了大尺度、反旋转、束状涡流,它明显地改变了气团由传统的高斯型浓度分布所描述的形状,变成由2个反漩涡环流组成的涡流结构,涡环围绕其涡核由内向外翻卷,涡环直径逐渐变大并且非常稳定地上升。CFD方法获得的烟云形态仿真结果与实验观测现象吻合,证明了烟云扩散机理是低密度高温烟团在空气浮力驱动下上升和扩散。
3.2 爆炸烟云扩散的时空分布
3.2.1 烟云顶部高度的变化
第1组实验主要为获取大气稳定、无风、厚水泥地面、裸装药条件下理想烟云的扩散模型。在有限实验条件下,烟云扩散的不确定性因素多,难以保证实验条件无干扰和实验数据可靠,所以采取实验与理论计算相互验证的方法来开展研究。图6显示烟云高度随时间变化的实验结果与理论计算数据相关性强、变化规律基本一致。理论计算结果比实验值略高,这是因为爆炸后的真实烟团扩散非常复杂,伴有抛洒和后续燃烧化学反应,还可能存在较小的风场干扰,而简化后的理想模型初始条件的设置上也存在可进一步优化的地方,所以两者存在一定的偏差。结果表明:(1)实验中获取的烟云扩散时空分布数据整体规律性较强,数据可靠;(2)基于低密度高温烟团扩散机理的建模计算方法能较好地表征烟云高度的变化。
从图6可见,烟云扩散呈现先加速、后减速、最后几乎不再上升的物理过程。进一步观察烟云高度随时间的变化曲线,发现烟云高度几乎都是呈现幂函数规律上升,符合浮力加速、空气阻力和重力减速的物理模型。采用Matlab幂函数数学模型H(t)=atb对烟云高度变化规律进行拟合,拟合结果和拟合度R如表2所示。拟合结果显示b=0.5±0.05相对固定,而a值随炸药量的增加而增大。令t=1 s,a=H(1),H(1)也就是爆炸火球成型且翻滚总共1 s时的烟云高度。火球膨胀到最大所需时间不到1 s,也就是说火球形成大小和1 s上升高度共同决定H(1),a的下限值为2r=3.86M0.32/ (T/3 600) 1/3,但是烟云在1 s时刻由于抛洒和翻滚均大于2r,实验和计算都显示a=(6.1±1)M(0.32±0.03)。由于爆炸当量的不同,烟云到达稳定状态的时间肯定也不同,Church在描述烟云最大高度时只假设烟云2 min到顶部,没有具体时间模型[12]。实验烟云后期视野模糊难以通过使用完全确定烟云上升的最终高度和时间,结合仿真计算共同确定1、16、62 kg TNT爆炸烟云抬升至稳定状态所需的时间tmax分别为30、70、110 s,抬升时间tmax与当量M的拟合关系为:tmax=(28± 1.1)M(0.33±0.04)。
表 2 烟云高度拟合参数Table 2. Parameters of cloud height fitM/kg a b R 1 6.15 0.518 0.983 16 14.92 0.502 0.995 62 25.56 0.486 0.987 综上所述,大气稳定、无风、厚水泥地面、裸装药条件下,理想烟云扩散高度随时间变化的模型为:H(t)= (6.1±1)M(0.32±0.03)t(0.5±0.05),在tmax= (28±1.1)M(0.33±0.04)时刻烟云到达顶高。通过烟云高度分布模型和最大上升时间确定1、16、62 kg TNT爆炸烟云的最终高度约36、125、240 m。Church实验在钢板上进行,8 m/s风速下53.52 kg TNT爆炸烟云高度约220 m,实验结果符合上述拟合模型[12]。
3.2.2 烟云宽度和温度的变化
烟云的宽度是计算烟云扩散体积变化的重要依据,Church只给出了烟云宽度是其高度的0.4倍的模糊结论[12],本文实验则显示烟云宽度的变化与爆炸条件关系较大,爆炸环境的差异性使得烟云宽度差距较大,但可以确定的是烟云宽度与其高度不是简单的比例关系。62 kg TNT爆炸实验中得到的烟云宽度变化如图7所示,对该烟云宽度进行D=atb+c拟合,得到D=4.5t0.77+4.57,b值较接近1,烟云宽度随时间近乎呈线性规律增大,这与李晓丽等[3]在烟云扩散实验中观察到的一致。烟云温度云图也非常复杂,选取烟云中最高温度的变化值拟合后如图8所示,发现温度随时间快速单调递减,直到无限逼近常温,温度变化近似Tmax=a/t+b反比例函数规律,a的取值与炸药爆炸后初始烟云温度相关,b的取值围绕环境温度变化。由反函数性质可以发现,烟云热抬升时间总是在几秒到十几秒,该结果与已获得的红外热成像实验结果[16]相符。
3.3 硬质地面和厚壳体束缚条件下烟云高度模型及特性
第2组实验为泥土硬质地面爆炸烟云扩散实验,第1、2组实验结果的对比如图9所示,硬质地面爆炸烟云高度比水泥地面烟云高度低(5~20)%,证明硬质地面成坑与土壤颗粒物夹带影响烟云上升,同时说明建立烟云扩散模型必须严格限定实验条件。泥土硬质地面爆炸形成土坑要消耗一定的爆炸能量,使得同等TNT当量下炸药驱动能变小;且土坑中的泥土进入烟云,导致烟云整体密度变大、体积变小,这些原因使得烟云的上升高度降低。该条件下的实验数据也显示出烟云的扩散高度随时间呈指数规律上升,可通过调整模型的a值和b值以获得地面成坑条件下的烟云扩散模型,实验数据拟合模型为H(t)=(6.3±1)M(0.29±0.03)t(0.5±0.05)。Thielen等[9]开展的爆炸烟云实验的实验条件未见报道,但是从实验结果分析来看,应该为硬质地面条件,而Sharon等[10]报道的图片显示其开展的爆炸烟云实验均在泥土地面上进行,其实验烟云高度与水泥地模型计算高度相比要低,两者实验条件和实验数据拟合均符合本文第2组实验的拟合模型。
针对爆炸装置壳体对爆炸烟云高度的影响,仅开展了1组裸装药和厚壳体束缚装置TNT爆炸烟云扩散对比实验。结果显示,采用厚壳体束缚爆炸装置时,同等时刻爆炸烟云高度整体偏低。这是因为炸药爆炸在抛撒壳体中会损失部分能量,同时壳体束缚会导致初始烟云体积变小、密度变大。具体壳体厚度与烟云扩散高度的定量关系还需设计更多实验加以确定。炸药种类、壳体束缚、风场变化、地面成坑等对烟云扩散都有影响,模型在不同条件下使用时,参数a、b的值应随之调整。本文中在不同实验条件下构建的爆炸烟云高度模型在计算当量低于100 kg TNT的爆炸烟云扩散高度时有一定的通用性。
4. 结 语
(1)本文中开展的烟云时空分布实验和烟云流体动力学仿真计算均得到了烟云流场从椭球形到蘑菇云形态的演变过程,仿真结果更清晰地显示烟云流场由2个反漩涡环流结构组成,实验结果和仿真结果证明烟云扩散过程是一个高温低密度浮力涡环运动的物理过程。
(2)实验得到大气稳定、无风、厚水泥地面、裸装药条件下烟云扩散高度H随时间t变化的模型为:H(t)=(6.1±1)M(0.32±0.03)t(0.5±0.05),在时间tmax=(28±1.1)M(0.33±0.04)时烟云趋于稳定,1、16、62 kg TNT爆炸烟云的扩散高度分别在30、70、110 s左右不再上升,最终烟云高度约为36、125、240 m。
(3)根据实验结果认为Church公式描述的烟云宽度是其高度的0.4倍的结论不够准确,讨论了烟云宽度随时间变化的拟合规律,结果显示烟云宽度几乎呈线性增大;发现烟云温度随时间快速单调递减至常温,烟云中温度变化近似反比例函数规律。
(4)实验初步讨论了不同地面条件和不同壳体束缚下的烟云扩散高度。第2组实验显示硬质地面条件会降低烟云上升高度(5~20)%,该条件下高度变化修正模型为H(t)= (6.3±1)M(0.29±0.03)t(0.5±0.05),第3组实验则显示厚壳体装置烟云扩散高度略低于裸装药烟云扩散高度。
针对爆炸烟云扩散的研究目前较少,但对污染评估和爆炸侦测却非常重要。下一步还将建立和修正不同爆炸装置、不同地面高度、不同粒子携带、不同大气环境下的烟云扩散模型,开发爆炸烟云扩散源项计算程序,以期为科学解释和预测爆炸烟云这一物理现象提供参考。
-
表 1 实验分组
Table 1. Experiment groups
组号 M/kg 地面条件 水平风速 炸药装置 大气稳定性 T0/℃ φ/% 1 1 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 16 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 62 厚水泥地面 无风 裸装药 基本稳定 25±3 40~60 2 16 硬质地面 无风 裸装药 基本稳定 25±3 40~60 62 硬质地面 无风 裸装药 基本稳定 25±3 40~60 3 62 厚水泥地面 无风 厚铁壳 基本稳定 25±3 40~60 表 2 烟云高度拟合参数
Table 2. Parameters of cloud height fit
M/kg a b R 1 6.15 0.518 0.983 16 14.92 0.502 0.995 62 25.56 0.486 0.987 -
[1] 黄寅生. 炸药理论[M]. 北京: 兵器工业出版社, 2009: 6−30. [2] 郑毅. 瞬时热源(爆炸烟云)浮力涡环研究[D]. 北京: 清华大学, 2008: 12−40. [3] 李晓丽, 郑毅, 刘伟, 等. 爆炸烟云运动的试验与数值模拟研究初探 [J]. 核电子学与探测技术, 2011, 31(2): 131–135. doi: 10.3969/j.issn.0258-0934.2011.02.002LI Xiaoli, ZHENG Yi, LIU Wei, et al. A preliminary study on the experiment and numerical simulation of explosive smoke [J]. Nuclear Electronics and Detection Technology, 2011, 31(2): 131–135. doi: 10.3969/j.issn.0258-0934.2011.02.002 [4] 王善强, 毛用泽. " 脏弹”爆炸初始烟云特征分析 [J]. 防化研究, 2007(4): 21–24. [5] MAKHVILADZE G M, ROBERTS J P, YAKUSH S E. Modeling of atmospheric pollution by explosions [J]. Environmental Software, 1995, 10(2): 117–127. DOI: 10.1016/0266-9838(94)00005-r. [6] KANSA E J. A time dependant buoyant puff model for explosion sources: UCRL-ID-128733 [R]. USA: Lawrence Livermore National Laboratory, 1997. [7] KANARSKA Y, LOMOV I, GLENN L, et al. Numerical simulation of cloud rise phenomena associated with nuclear bursts [J]. Annals of Nuclear Energy, 2009, 36(10): 1475–1483. doi: 10.1016/j.anucene.2009.08.009 [8] MISHRA K B, WEHRSTEDT K D, KREBS H. Boiling liquid expanding vapour explosion (BLEVE) of peroxy-fuels: experiments and computational fluid dynamics (CFD) simulation [J]. Energy Procedia, 2015, 66(2): 149–152. [9] THIELEN H, SCHRODL E. Blast experiments for the derivation of initial cloud dimensions after a " dirty bomb” event: Koln- 50667 [R]. Germany, 2004. [10] SHARON A, HALEVY I, SATTINGER D, et al. Cloud rise model for radiological dispersal devices events [J]. Atmospheric Environment, 2012, 54: 603–610. doi: 10.1016/j.atmosenv.2012.02.050 [11] LEBLEL L, BOURGOUIN P, CHOUHAN S, et al. The sensitivity of atmospheric dispersion calculations in near-field applications: modeling of the full-scale RDD experiments with operational models in Canada: part I [J]. Health Physics, 2016, 110(5): 499–517. [12] CHURCH H W. Cloud rise from high explosive detonations: TID-4500 [R]. USA: Sandia National Laboratories (SNL), 1969. [13] BROWN R C, KOLB C E, Conant J A, et al. Source characterization model (SCM): a predictive capability for the source terms of residual energetic material from burning and/or detonation activities: ART-RR-1384 [R]. 2004. [14] 袁涛, 罗永锋, 尚爱国, 等. 爆炸烟云中粒子运动的数值模拟 [J]. 爆炸与冲击, 2013, 33(5): 556–560.YUAN Tao, LUO Yongfeng, SHANG Aiguo, et al. Numerical study on particle motion in explosive buoyant [J]. Explosion and Shock Waves, 2013, 33(5): 556–560. [15] 陈福振, 强洪夫, 苗刚, 等. 燃料抛撒成雾及其燃烧爆炸的光滑离散颗粒流体动力学方法数值模拟研究 [J]. 物理学报, 2015, 64(11): 110202–110202.CHEN Fuzhen, QIANG Hongfu, MIAO Gang, et al. Numerical simulation of smooth discrete particle hydrodynamic method for fuel dispersion and its burning and explosion [J]. Chinese Journal of Physics, 2015, 64(11): 110202–110202. [16] 段中山, 罗永锋, 袁伟, 等. 爆炸烟团扩散的实验与仿真研究 [J]. 高压物理学报, 2013, 27(2): 305–311.DUAN Zhongshan, LUO Yongfeng, YUAN Wei, et al. Experiment and simulation of explosive cloud diffusion [J]. Journal of High Pressure Physics, 2013, 27(2): 305–311. [17] WU C, LUKASZEWICZ M, SCHEBELLA K, et al. Experimental and numerical investigation of confined explosion in a blast chamber [J]. Journal of Loss Prevention in the Process Industries, 2013, 26(4): 737–750. DOI: 10.1016/j.jlp.2013.02.001. [18] ABDUL-KARIM N, BLACKMAN C S, GILL P P, et al. The spatial distribution patterns of condensed phase post-blast explosive residues formed during detonation [J]. Journal of Hazardous Materials, 2016, 316(4): 204–213. DOI: 10.1016/j.jhazmat.2016.04.081. [19] KWAK H Y, KANG K M, KO I, et al. Fire-ball expansion and subsequent shock wave propagation from explosives detonation [J]. International Journal of Thermal Sciences, 2012, 59(9): 9–16. DOI: 10.1016/j.ijthermalsci.2012.04.022. [20] 王瑞金, 张凯, 王刚. Fluent技术基础与应用实例[M]. 北京: 清华大学出版社, 2007: 5−60. [21] FEDINA E, FUREBY C. Investigating ground effects on mixing and afterburning during a TNT explosion [J]. Shock Waves, 2013, 23(3): 251–261. DOI: 10.1007/s00193-012-0420-9. 期刊类型引用(4)
1. 霍勇刚,姚弘毅,蔡幸福,姜仁强,路志勇. 化学爆炸条件下钚气溶胶扩散数值模拟. 火箭军工程大学学报. 2024(02): 25-31 .  百度学术
百度学术2. 郝海彤,刘伟,郑毅,刘强,张凯,赵锋. 基于OpenFOAM的爆炸烟云扩散模拟研究. 核电子学与探测技术. 2022(04): 739-744 .  百度学术
百度学术3. 张延泽,肖凯涛,宋伟伟,秦健,迟卉,黄瑞源. 防暴弹室内爆炸扩散试验及刺激剂浓度预测. 爆破. 2021(04): 163-172 .  百度学术
百度学术4. 段中山,龚朋彬,袁伟,过惠平,罗永锋,罗昆升. 不同风场下TNT炸药爆炸烟云的扩散模型及特性. 爆炸与冲击. 2020(05): 115-123 .  本站查看
本站查看其他类型引用(2)
-








 下载:
下载:












 下载:
下载:








 百度学术
百度学术