Equivalent static load dynamical coefficient for exponential air blast loading with transition
-
摘要: 为对比抗爆设计规范采用的线性荷载计算模式,建立了考虑跃迁的指数型衰减荷载表达式,通过爆炸荷载等效单自由度微分方程,求解了关于跃迁时长、超压峰值、指数型形状调整参数、结构自振频率与荷载作用时长的等效静载抗力动力系数表达式。根据跃迁时长与形状调整参数,分析了四种典型计算工况,结果表明:现行结构抗爆设计规范等冲量线性衰减荷载可设计范围明显偏小,动力系数在延性比β<3.0下偏保守,而β≥3.0,wt+>1.4δ时偏不安全,最大偏低17.4%;跃迁时长比值越大,动力系数越大,跃迁时长比为1%~2%时,对动力系数影响程度为0.4~0.7%,指数型荷载形状调整参数对柔度特别大的结构动力系数无影响,对其它结构动力系数增大或减少影响程度不一。Abstract: Compared to linear attenuation load calculation model, exponential attenuation load with transition expression was given to build equivalent SDOF differential equations under air blast loading. The expressions, which had relationship to transition duration, overpressure peak, exponential load adjusting parameter, natural frequency and overpressure duration, were derived to solve equivalent static load dynamical coefficients. Changed with transition duration and load adjusting parameters, four typical calculation conditions results were calculated. The results show that the linear attenuation blast load’s application range is limited. When the ductility ratio β<3.0, the dynamical coefficients from the linear attenuation blast load behaves greater value and safer characteristic. When the ductility ratio β≥3.0 and wt+>1.4δ, it will be lower 17.4% than the value from exponential attenuation load with transition. For the much transition duration ratio, dynamical coefficients will be greater. In the range of 1%−2% for the transition duration ratio, the effect on dynamical coefficients is greater at 0.4−0.7% and can be ignored. Exponential load adjusting parameter has no effect on structural design with great flexibility while it would lead to increase or decrease the dynamical coefficients for the structure with the general flexibility.
-
对防护结构及建筑结构而言,炸药空爆荷载具有超压峰值高、作用时间短、扩散速度快等特点,相比其它设计荷载,为典型的动力荷载。在结构设计阶段,若能将此爆炸荷载等效为静载计算,可避免繁琐的非线性有限元分析,易为结构工程师所接受和使用。因此,多数规范中[1-7]爆炸荷载均按考虑动力系数的静载进行结构计算。入射超压从零跃迁至正超压峰值时长占冲击波正超压作用时长比值很低,故规范常省略此跃迁升压阶段,为简化计算,并进一步将入射超压简化为线性衰减荷载。
爆炸荷载等效静载理论研究方面研究较多。Biggs[8]对线性衰减类动力荷载进行等效静载处理,并用于爆炸荷载近似计算。伍俊等[9]采用等效单自由度与有限元方法对防爆墙结构进行对比分析,模型中爆炸荷载为未考虑跃迁升压的线性衰减荷载模式,他指出等效单自由度静载方法结构设计具有良好精度。颜海春等[10]采用爆炸荷载等效静载对人防工程封堵梁内力分析,但未指出结构延性比与荷载动力系数之间的详细关系。杨涛春等[11]采用爆炸荷载线性衰减函数对钢混组合梁进行了等效静载与有限元分析对比计算,但计算未涉及结构自振频率、延性比。Baker等[12]指出空爆冲击波呈指数型衰减,等效冲量计算按此计算较准确。杨科之[13-14]研究了直线衰减荷载作用下动力系数与延性比的关系,延性比较大时动力系数可计算范围受限。陈俊杰[15]研究了爆炸荷载冲量简化分析方法,所采用的荷载形式为线性衰减荷载模式。Chen等[16]采用等效荷载分析了地下拱结构-土体爆炸耦合效应,指出了冲击波等效单自由度的有效及方便性。Shi等[17]指出采用指数型函数进行爆炸荷载冲量及等效静载时会更准确,但未考虑跃迁段的影响。Gantes等[18]计算了指数型爆炸荷载作用单自由度结构的弹塑性位移响应解,但尚未明确与划分荷载作用时间与结构进入塑性阶段时间关系,且未对结构延性比、动力系数分析。Louca等 [19]分析爆炸作用时指出正超压衰减段采用指数型函数拟合会更接近冲击波实测结果。
设计延性比与荷载作用时长有关,线性衰减荷载正超压等效作用时长小于爆炸冲击波正超压作用真实时长,这对动力系数影响如何?同时,忽略升压过程对结构的动响应尤其对结构弹性响应,继而对结构塑性阶段响应及延性比、动力系数的影响程度如何,国内外学者鲜有研究。
因此,本文考虑超压从零跃迁升压至超压峰值,以指数形式从超压峰值衰减至零的分段荷载函数进行等效单自由度体系下结构弹塑性动力系数分析,并与设计规范中采用的未考虑升压阶段的等冲量线性衰减荷载动力系数计算结果对比,为基于延性比的抗爆结构设计提供理论支持。参考笔者前期所做的TNT、B炸药空爆冲击波衰减波形测试工作及已出版文献[20],常规炸药等化学爆炸跃迁升压至超压峰值时长所占正超压作用时长比一般为3%以下,且接近直线升压,因此本文考虑的升压为直线跃迁升压模式。
1. 理想弹塑性体系动力微分方程
1.1 等效单自由度微分方程
常规炸药等化学空爆冲击波正超压作用时长很小,小于结构出现最大动位移反应时间[5]。根据结构动力学等效单自由度理论及达朗贝尔原理,其等效单自由度体系弹性阶段微分方程:
kM−Lm¨W(t)+1lKW(t)=Δp(t) (1) 式中:kM-L为弹性等效质量-等效荷载系数比,m为结构单位长度质量,W(t)为结构动位移,l为结构长度,K为结构等效弹簧刚度,Δp(t)为爆炸荷载时程函数。
设在tT时刻,结构弹性位移达到最大值WT,速度为vT,之后结构进入塑性振动。结构塑性阶段微分方程为:
km−lm¨W(t)+qm=Δp(t) (2) 式中:km-l为塑性等效质量-等效荷载系数比,qm为结构塑性阶段结构抗力。
图1给出了3种荷载模式的时程曲线。作为等效时长计算对比的基准量,不考虑跃迁段的指数型衰减荷载所对应的函数为:
Δp(t)=Δpmf(t)={Δpm(1−t/t+)e−at/t+t≤t+0t>t+(3) 式中:Δpm为超压峰值,f(t)为荷载归一化函数,t+为冲击波正超压作用时长,a为荷载曲线形状调整参数。
作为动力系数对比的基准量,不考虑正超压跃迁段,采用等冲量线性衰减荷载模式时,对应的函数为:
Δp(t)=Δpmf(t)={Δpm(1−t/tI)t≤tI0t>tI (4) 式中:tI为等冲量线性衰减荷载等效作用时长。
增加跃迁时间而不改变正超压作用时长的指数型衰减荷载模式,对应函数为:
Δp(t)=Δpmf(t)={Δpm(t/t0)t≤t0Δpm(1−t−t0t+−t0)e−at−t0t+−t0t0<t≤t+0t>t+ (5) 式中:t0为荷载从0跃迁至超压峰值跃迁时长。
由式(1)及杜哈梅积分可知,在弹性阶段:
W(t)=Wcmω∫t0f(τ)sinω(t−τ)dτ (6) V(t)=˙W(t)=Wcmω2∫t0f(τ)cosω(t−τ)dτ (7) 式中:Wcm为超压峰值为静载数值时对应的结构位移,ω为振动角频率。
在tT时刻弹性响应结束准备进入塑性响应,此时对应结构最大弹性位移及速度:
WT=W(tT)=Wcmω∫tT0f(τ)sinω(tT−τ)dτ (8) VT=˙W(tT)=Wcmω2∫tT0f(τ)cosω(tT−τ)dτ (9) 且可知塑性阶段结构任一时刻t对应的结构速度及位移为
V(t)=VT+1mkm−l[ΔPm∫ttTf(τ)dτ−qm(t−tT)] (10) W(t)=WT+(t−tT)VT+1mkm−l[∫ttT∫τtTf(τ)dτdτ−12qm(t−tT)2] (11) 由于结构动荷载等效为基于理想弹塑阶段内的等效静载,其动力系数表达式为
Kh=WTWcm=qmΔpm (12) 1.2 冲量及等效时长分析
由冲量定义及图1所示,未考虑跃迁的指数型衰减荷载所对应冲量函数表达式
I=∫t+0Δpm(1−t/t+)e−at/t+dt=Δpmt+[1a+1a2(e−a−1)] (13) 荷载曲线形状调整参数a数值越大,指数型衰减荷载所对应的曲线越陡峭,负超压峰值及作用时长越小。为较好拟合测试曲线,其数值取值范围可取1.27≤a≤1.61[11],为满足建筑结构抗爆设计一般特点,本文取端部值即1.27与1.61为后续分析参数值。
结合图1荷载曲线所包围的面积变化可知:t0时刻之前,考虑跃迁后的指数型衰减荷载所对应的冲量数值小于未考虑跃迁的指数型衰减荷载冲量数值,t0时刻之后该冲量又偏大。因此需计算其冲量:
I=∫t00Δpm(t/t0)+∫t+t0Δpm(1−t−t0t+−t0)e−at−t0t+−t0dt=12Δpmt0+Δpm(t+−t0)[1a+(e−a−1)a2] (14) 将式(13)~(14)做函数差值对a进行求导分析,可知对于任意的t0>0,当a>0时,公式(14)对应的数值为大,即考虑跃迁后,冲量随之增大,对结构抗爆设计偏不利。
以式(13)为基准,未考虑跃迁的等冲量线性衰减荷载,其等效时长为:
ti=2IΔpm=2t+[1a+1a2(e−a−1)] (15) 即指数型衰减荷载真实作用时长t+与等冲量线性衰减荷载等效时长ti的比值δ分别为1.464、1.6。
2. 结构体系塑性阶段划分
常规炸药空爆冲击波作用时长t+很短,经等冲量换算后的线性衰减荷载等效时长tI更短,结构完成弹塑性最大变形时间tm>t+及tm>tI,即此时对应结构外荷载为零。结构完成弹性变形进入塑性变形时刻tT存在两种可能性:t0<t+<tT<tm,即较晚进入塑性阶段及t0<tT<t+<tm,即较早进入塑性阶段。
为精简篇幅,本文只给出考虑跃迁的指数型衰减荷载动力系数求解过程及公式,等冲量线性衰减荷载求法简单,公式不再单独求解,而以其计算结果的相对差值比例在本文工况示例计算给出。
2.1 较晚进入塑性阶段
若令θ0=ωt0,θT=ωtT,θ+=ωt+,由公式(7)计算考虑跃迁的指数型衰减荷载等效静载动力系数:
Kh=ω[∫t00τt0sinω(tT−τ)dτ+∫t+t0[(1−τ−t0t+−t0)e−aτ−t0t+−t0]sinω(tT−τ)dτ]=sin(θT−θ0)−sinθTθ0+cos(θT−θ0)+e−asin(θT−θ+)a2(θ+−θ0)−(θ+−θ0)3((θ+−θ0)2+a2)2+e−acos(θT−θ+)−2a(θ+−θ0)2((θ+−θ0)2+a2)2+sin(θT−θ0)(a+1)(θ+−θ0)3+(a3−a2)(θ+−θ0)((θ+−θ0)2+a2)2+cos(θT−θ0)(2a−a2)(θ+−θ0)2−(θ+−θ0)4((θ+−θ0)2+a2)2 (16) 若定义A=Kh
B=−sinθ0θ0+cosθ0+(sinθ0−e−asinθ+)a2(θ+−θ0)−(θ+−θ0)3[(θ+−θ0)2+a2]2−2a(cosθ0−e−acosθ+)(θ+−θ0)2[(θ+−θ0)2+a2]2−a(θ+−θ0)sinθ0+(θ+−θ0)2cosθ0(θ+−θ0)2+a2 C=cosθ0−1θ0+sinθ0+(e−acosθ+−cosθ0)a2(θ+−θ0)−(θ+−θ0)3((θ+−θ0)2+a2)2+2a(sinθ0−e−asinθ+)(θ+−θ0)2((θ+−α0)2+a2)2+a(θ+−θ0)cosθ0−(θ+−θ0)2sinθ0(θ+−θ0)2+a2 因θT为中间变量,所以需将其求出,求解后其表达式为
cosθT=AB±C√B2+C2−A2B2+C2 (17) 由式(8)可得考虑跃迁的指数型衰减荷载弹性阶段结束时对应的振动速度与位移比值为
VTWT=WcmWT⋅ω2[∫t00τt0cosω(tT−τ)dτ+∫t+t0t+−τt+−t0e−aτ−t0t+−t0cosω(tT−τ)dτ]=ω(B2+C2ACcosθT−BC) (18) 由式(9)及塑性阶段v(tm)=0可导出
VTWT=ΔpmKhm⋅km−l⋅WT(tm−tT) (19) 由式(10)及塑性阶段v(tm)=0可导出
tm−tT=ωm⋅km−lWTΔpmKh(B2+C2ACcosθT−BC) (20) 由式(11)可知
β=WmWT=1+(tm−tT)VTWT−ΔpmKh2mkm−lWT(tm−tT)2=1+ΔpmKh2mkm−lWT(tm−tT)2 (21) 式中,Wm为结构进入塑性阶段结构最大位移。又因为
ΔpmWT=ΔpmlmlmWT=KWcmmMWT=KMkM−LWcmWTmkM−L=ω2mkM−LKh (22) 将式(20)、(22)代入式(21)后可知
β=1+km−l2kM−L(B2+C2ACcosθT−BC)2=1+km−l2kM−L(AB±C√B2+C2−A2AC−BC)2 (23) 2.2 较早进入塑性阶段
若令θm=ωtm,由前述定义t0<tT<t+<tm及动力系数公式可知,考虑跃迁的指数型衰减荷载等效静载动力系数为:
Kh=ω[∫t00τt0sinω(tT−τ)dτ+∫tTt0(1−τ−t0t+−t0)e−aτ−t0t+−t0sinω(tT−τ)dτ]=sin(θT−θ0)−sinθTθ0+e−aθT−θ0θ+−θ0[(θ+−θ0)2(θ+−θ0)2+a2⋅(θ+−θT)(θ+−θ0)−2a(θ+−θ0)2((θ+−θ0)2+a2)2]+cos(θT−θ0)(a2+2a)(θ+−θ0)2+a4((θ+−θ0)2+a2)2+sin(θT−θ0)(a+1)(θ+−θ0)3+(a3−a2)(θ+−θ0)((θ+−θ0)2+a2)2 (24) 由式(7)可得考虑跃迁的指数型衰减荷载对应的弹性结束时对应的振动速度为
VT=Wcm⋅ω2[∫t00τt0cosω(tT−τ)dτ+∫tTt0(1−τ−t0t+−t0)e−aτ−t0t+−t0cosω(tT−τ)dτ]=Wcmω{cos(θT−θ0)−cosθTθ0+e−a(θT−θ0)(θ+−θ0)[(θ+−θ0)a2−(θ+−θ0)3((θ+−θ0)2+a2)2−a(θ+−θ0)(θ+−θ0)2+a2(θ+−θTθ+−θ0)]+cos(θT−θ0)(a+1)(θ+−θ0)3+(a3−a2)(θ+−θ0)((θ+−θ0)2+a2)2−sin(θT−θ0)(a2+2a)(θ+−θ0)2+a4((θ+−θ0)2+a2)2} (25) 由式(10)及塑性阶段v(tm)=0可得速度的另一解为
VT=−Δpmmkm−l[1a(t+−tTt+−t0)e−atT−t0t+−t0+e−a−e−atT−t0t+−t0a2(t+−t0)−Kh(tm−tT)] (26) θm=km−lKhkM−LVTWcmω+1Kh[(θ+−θT)ae−a(θT−θ0)(θ+−θ0)+(θ+−θ0)a2(e−a−e−a(θT−θ0)(θ+−θ0))]+θT (27) 代入式(11)后可得
β=WmWT=1+(tm−tT)VTWT+1mkm−lWT[Δpm∫t+tT∫τtT[(t+−τt+−t0)e−aτ−t0t+−t0]dτdτ−qm2(tm−tT)2]=1+kM−Lkm−lKh{e−a(θT−θ0)(θ+−θ0)[(θ+−θT)(θ+−θm)a+(θ+−θ0)(θm+θT−2θ+)a2+2a3(θ+−θ0)2]−e−a[(θ+−θ0)(θm−θT)a2+2a3(θ+−θ0)2]+Kh2(θm−θT)2} (28) 3. 弹塑性阶段动力系数计算算例
3.1 工况分组及计算结果
根据跃迁时长占正超压总时长的比值(t0/t+)、爆炸荷载正超压作用时间t+与等冲量线性衰减荷载等效时间tI比值δ确定了4种典型计算工况。为相互对比,以等冲量线性衰减荷载结构荷载参数θI=ωtI为0.2~2.8,0.2为步长,共计14项作为基本计算数据,而ωt+(即θ+)按作用时长与等效时长换算后再计算为对比数据。为能涵盖工程结构设计时所对应的延性比,延性比β为1~5作为计算范围。工况分组如表1所示。
表 1 工况分组Table 1. Calculation cases工况 t0/t+ δ a θI 工况1 0.01 1.464 1.27 0.2~2.8 工况2 0.01 1.6 1.61 0.2~2.8 工况3 0.02 1.464 1.27 0.2~2.8 工况4 0.02 1.6 1.61 0.2~2.8 由式(23)及(28)可知,动力系数Kh不为显式函数,由θT、θ+、θm定义及延性比β的范围作为控制条件,分别赋初值后迭代求解并汇总。工况1计算结果及与线性衰减荷载的差异比值见表2。 表中β为1.0数据列为结构动力系数公式退化至弹性状态时求解结果,红色间断线左侧数据为考虑跃迁升压的指数型衰减荷载模式下结构较晚进入塑性阶段所对应的公式计算得出,红色间断线包围的右侧数据为考虑跃迁升压的指数型衰减荷载模式下结构较早进入塑性阶段所对应公式计算得出。表中蓝线左上方(右下方)数据为等冲量线性衰减荷载模式下结构较晚进入塑性阶(较早进入塑性阶段)段所对应计算公式得出。工况1~工况4之间相对差值及与直线型衰减荷载差异如图2所示。
表 2 工况1动力系数计算KhTable 2. Dynamical coefficient Kh for calculation case 1θ+ Kh β=1.0 β=1.2 β=1.6 β=2 β=3 β=5 0.292 8 0.100 (1.0%) 0.085 (1.2%) 0.067 (0.0%) 0.057 (0.0%) 0.044 (0.0%) 0.033 (0.0%) 0.585 6 0.199 (0.0%) 0.168 (0.0%) 0.134 (0.7%) 0.114 (0.0%) 0.088 (0.0%) 0.065 (0.3%) 0.878 4 0.296 (−0.3%) 0.25 (0.0%) 0.199 (0.0%) 0.17 (0.0%) 0.129 (−2.4%) 0.090 (−7.3%) 1.171 2 0.389 (−0.8%) 0.328 (−0.9%) 0.261 (−0.9%) 0.220 (−2.3%) 0.162 (−6.6%) 0.110 (−9.2%) 1.464 0 0.478 (−1.7%) 0.403 (−1.7%) 0.316 (−3.1%) 0.265 (−5.1%) 0.192 (−7.0%) 0.125 (−8.3%) 1.756 8 0.562 (−2.5%) 0.472 (−2.9%) 0.368 (−4.7%) 0.307 (−5.1%) 0.221 (−5.1%) 0.138 (−4.4%) 2.049 6 0.640 (−3.4%) 0.537 (−3.8%) 0.420 (−4.1%) 0.350 (−3.4%) 0.251 (−1.4%) 0.149 (3.2%) 2.342 4 0.711 (−4.6%) 0.599 (−4.7%) 0.474 (−2.0%) 0.397 (−0.4%) 0.283 (3.0%) 0.161 2.635 2 0.774 (−6.1%) 0.652 (−5.6%) 0.519 (−1.9%) 0.446 (2.9%) 0.319 (7.9%) 0.176 2.928 0 0.830 (−7.6%) 0.700 (−6.7%) 0.557 (−2.9%) 0.476 (1.6%) 0.359 (12.8%) 0.198 3.220 8 0.877 (−9.4%) 0.740 (−8.5%) 0.589 (−5.1%) 0.504 (−0.6%) 0.404 (17.4%) 0.229 3.513 6 0.917 0.773 0.615 0.526 0.407 0.267 3.806 4 0.948 0.799 0.636 0.544 0.421 0.308 4.099 2 0.972 0.819 0.652 0.558 0.431 0.354 由表1、图2、各计算工况与规范指定的等冲量线性衰减荷载计算结果可知,延性比β<1.6时,等冲量线性衰减荷载动力系数偏大;β>1.6时,线性衰减荷载计算数值先偏大后偏小。线性衰减荷载的ωt+对应的设计范围明显偏小;β≤3.0时,考虑跃迁的指数衰减荷载计算结果数值大小依次递减顺序为工况3、1、4、2,其中工况3与工况1之间的差异最大值为1.2%,最小为0,平均为0.4%,差异极小;工况4与工况2之间的差异最大值为1.5%,最小为0,平均为0.7%,差异很小,略大于工况3与工况1之间的差异,即跃迁时长比对动力系数的影响很小,β=5.0时,跃迁时长比对动力系数的影响可忽略,指数形状调整参数影响效果明显。
结合图2,以工况1与工况2之差异为典型对比,取规范采用的等冲量线性衰减荷载为基准量,分析指数形状调整参数a对动力系数的计算结果影响(η),见图3所示。
设计参数θ+<0.8δ时,工况1和工况2之间的差异逐渐增大,数值上仍很小,差异数值最大为2%,0.8δ<θ+<1.4δ时,除β=5的差异约为5.0%以外,其它延性比对应的工况1和工况2之间的差异先减小后增加,数值上仍很小,差异约仍小于2%;1.4δ<θ+<2.2δ时,工况1和工况2之间的差异逐渐增大,趋于稳定数值,平均约为3.8%,说明形状调整参数a对计算结果的影响与设计参数θ+紧密相关。
3.2 结果分析
考虑跃迁升压的指数型衰减荷载等效静载动力系数,其求解结果可分为弹性状态、较晚进入塑性状态、较早进入塑性状态3种类型,3种物理含义对应的间数据能很好衔接;等冲量线性衰减荷载等效静载动力系数也有此3种状态,但当θ+>1.4δ时,弹性状态数据直接与较早进入塑性状态的数据进行衔接,缺少较晚进入塑性状态的过渡。在同一爆炸荷载作用下,若结构设计的柔度越大,即θ+数值越小,对应的等效静载动力系数越小,在θ+<0.4δ时,4种工况对应的动力系数均大于由等冲量线性衰减荷载计算数值,最大数值为1.7%,误差较小;当0.6δ≤θ+≤1.2δ时,其动力系数均小于等冲量线性衰减荷载计算结果,最大误差为10%,当θ+>2.2δ时,或当设计延性比β=5且θ+>1.4δ时,等冲量线性衰减荷载动力系数无解而考虑跃迁的指数型衰减荷载有解。
特别地,4种典型工况中,均当延性比β=3且θ+>1.4δ时,考虑跃迁后的指数型衰减荷载等效静载动力系数均大于等冲量线性衰减荷载等效静载动力系数数值,且大于幅度较高,最大误差为17.7%,即此区间按规范所规定的等冲量线性衰减荷载进行等效静载设计时,偏不安全。
相同跃迁时长比值下,当θ+<0.4δ时,即柔度特别大的结构,形状调整参数a对应的动力系数计算数值误差基本为0,即形状调整参数a在此范围对计算结果不敏感;较晚进入塑性状态范围内,随着形状调整参数a的提高,动力系数计算数值变小;较早进入塑性状态数据,随形状调整参数a的提高,动力系数计算数值增大。
相同的指数荷载形状调整参数a下,随着跃迁时长比值的增加,所有工况的动力系数均增加,增加幅度较小且有限,最大值1.5%,即不考虑跃迁会导致计算结果偏小,但误差可忽略。
4. 结 论
以考虑升压跃迁的指数型衰减荷载为研究对象,考查了与等冲量线性衰减荷载等效静载动力系数的差别,并分析了跃迁时长比、指数形状调整参数对动力系数的影响,得到以下主要结论:
(1) 与等冲量线性衰减荷载相比,考虑跃迁的指数型衰减荷载动力系数弹性状态、较晚及较早进入塑性状态衔接更为合理,可进行设计的延性比β与结构荷载参数θ+范围更广,较小β及结构荷载参数θ+对应的动力系数较小,对应为当前现行结构设计规范设计偏安全;较大β及θ+对应的动力系数偏大,且幅值较高,若此范围区间仍采用规范类线性衰减荷载进行抗爆设计,偏不安全;
(2) 相同跃迁时长比值下,指数荷载形状调整参数a越大,对较早进入塑性状态动力系数提高越大,对θ+<0.4δ对应的较晚进入塑性状态计算结果基本不产生影响,对θ+>2.5对应的较晚进入塑性状态计算数值为降低作用;
(3) 相同指数荷载形状调整参数a下,跃迁时长比值增加会导致动力系数结果提高,但幅度有限,最大值1.5%,平均值依据不同工况在0.4%~0.7%范围,可忽略其影响。
-
表 1 工况分组
Table 1. Calculation cases
工况 t0/t+ δ a θI 工况1 0.01 1.464 1.27 0.2~2.8 工况2 0.01 1.6 1.61 0.2~2.8 工况3 0.02 1.464 1.27 0.2~2.8 工况4 0.02 1.6 1.61 0.2~2.8 表 2 工况1动力系数计算Kh
Table 2. Dynamical coefficient Kh for calculation case 1
θ+ Kh β=1.0 β=1.2 β=1.6 β=2 β=3 β=5 0.292 8 0.100 (1.0%) 0.085 (1.2%) 0.067 (0.0%) 0.057 (0.0%) 0.044 (0.0%) 0.033 (0.0%) 0.585 6 0.199 (0.0%) 0.168 (0.0%) 0.134 (0.7%) 0.114 (0.0%) 0.088 (0.0%) 0.065 (0.3%) 0.878 4 0.296 (−0.3%) 0.25 (0.0%) 0.199 (0.0%) 0.17 (0.0%) 0.129 (−2.4%) 0.090 (−7.3%) 1.171 2 0.389 (−0.8%) 0.328 (−0.9%) 0.261 (−0.9%) 0.220 (−2.3%) 0.162 (−6.6%) 0.110 (−9.2%) 1.464 0 0.478 (−1.7%) 0.403 (−1.7%) 0.316 (−3.1%) 0.265 (−5.1%) 0.192 (−7.0%) 0.125 (−8.3%) 1.756 8 0.562 (−2.5%) 0.472 (−2.9%) 0.368 (−4.7%) 0.307 (−5.1%) 0.221 (−5.1%) 0.138 (−4.4%) 2.049 6 0.640 (−3.4%) 0.537 (−3.8%) 0.420 (−4.1%) 0.350 (−3.4%) 0.251 (−1.4%) 0.149 (3.2%) 2.342 4 0.711 (−4.6%) 0.599 (−4.7%) 0.474 (−2.0%) 0.397 (−0.4%) 0.283 (3.0%) 0.161 2.635 2 0.774 (−6.1%) 0.652 (−5.6%) 0.519 (−1.9%) 0.446 (2.9%) 0.319 (7.9%) 0.176 2.928 0 0.830 (−7.6%) 0.700 (−6.7%) 0.557 (−2.9%) 0.476 (1.6%) 0.359 (12.8%) 0.198 3.220 8 0.877 (−9.4%) 0.740 (−8.5%) 0.589 (−5.1%) 0.504 (−0.6%) 0.404 (17.4%) 0.229 3.513 6 0.917 0.773 0.615 0.526 0.407 0.267 3.806 4 0.948 0.799 0.636 0.544 0.421 0.308 4.099 2 0.972 0.819 0.652 0.558 0.431 0.354 -
[1] 中华人民共和国住房和城乡建设部. 建筑结构荷载规范: GB50009-2012 [S]. 北京: 中国建筑工业出版社, 2012. [2] 中华人民共和国建设部. 人民防空地下室设计规范: GB50038-2005 [S]. 北京: 中国计划出版社, 2005. [3] 中华人民共和国住房和城乡建设部. 石油化工控制室抗爆设计规范: GB50009-2012 [S]. 北京: 中国计划出版社, 2012. [4] 中华人民共和国住房和城乡建设部. 抗爆间室结构设计规范: GB50907-2013 [S]. 北京: 中国计划出版社, 2013. [5] 北京市规划委员会. 平战结合人民防空工程设计规范: DB11/994-2013 [S]. 北京: 中国建筑工业出版社, 2013. [6] U.S. ARMY CORPS OF ENGINEERS. Structures to Resist the Effects of Accidental Explosions : TM5-1300 [S]. USA: US Army, 1990. [7] American Society of Civil Engineering. Design of Blast Resistant Buildings in Petrochemical Facilities: ASCE-41088 [S]. USA: ASCE, 2010. [8] BIGGS J M. Introduction to structural dynamics[M]. New York: McGraw-Hill, 1964. [9] 伍俊, 刘晶波, 杜义欣. 汽车炸弹爆炸下装配式防爆墙弹塑性动力计算与数值分析 [J]. 防灾减灾工程学报, 2007, 27(4): 394–400 doi: 10.3969/j.issn.1672-2132.2007.04.004WU Jun, LIU Jingbo, DU Yixin. Elastic-plastic dynamic calculation and numerical analysis of assembling blast resistant wall under effect of vehicle bombs [J]. Journal of Disaster Prevention & Mitigation Engineering, 2007, 27(4): 394–400 doi: 10.3969/j.issn.1672-2132.2007.04.004 [10] 颜海春, 方秦, 张亚栋, 等. 化爆作用下人防工程口部封堵梁的计算与分析 [J]. 解放军理工大学学报: 自然科学版, 2001, 2(3): 78–81 doi: 10.3969/j.issn.1009-3443.2001.03.018YAN Haichun, FANG Qin, ZHANG Yadong, et al. Calculation and analysis of beam for blocking entrance in civil air-defense engineering under conventional weapon explosion [J]. Journal of PLA University of Science & Technology, 2001, 2(3): 78–81 doi: 10.3969/j.issn.1009-3443.2001.03.018 [11] 杨涛春, 李国强, 王开强. 接触爆炸荷载下钢—混凝土组合梁简化设计方法研究 [J]. 四川建筑科学研究, 2009, 35(6): 1–4 doi: 10.3969/j.issn.1008-1933.2009.06.001YANG Taochun, LI Guoqiang, WANG Kaiqiang. Research on the simplified design method for steel-concrete composite beam subjected to contact blast loading [J]. Sichuan Building Science, 2009, 35(6): 1–4 doi: 10.3969/j.issn.1008-1933.2009.06.001 [12] BAKER W E. Explosion hazards and evaluation[M]. Amsterdam: Elsevier Scientific Pub. Co. 1983. [13] 杨科之, 王年桥. 化爆条件下地面结构等效静载计算方法 [J]. 防护工程, 2001, 23(2): 1–7YANG Kezhi, WANG Nianqiao. Equivalent static load calculation method for ground structures under chemical explosion [J]. Protective Engineering, 2001, 23(2): 1–7 [14] 杨科之, 杨秀敏, 王年桥. 内爆荷载作用下结构等效静载计算方法 [J]. 解放军理工大学学报: 自然科学版, 2002, 3(4): 31–33 doi: 10.3969/j.issn.1009-3443.2002.04.008YANG Kezhi, YANG Xiumin, WANG Nianqiao. Equivalent Static Load Calculation Method of Structure Subjected to Internal Explosion [J]. Journal of PLA University of Science & Technology, 2002, 3(4): 31–33 doi: 10.3969/j.issn.1009-3443.2002.04.008 [15] 陈俊杰, 高康华, 孙敖. 爆炸条件下结构超压-冲量曲线简化计算研究 [J]. 振动与冲击, 2016, 35(13): 224–232 doi: 10.13465/j.cnki.jvs.2016.13.036CHEN Junjie, GAO Kanghua, SUN Ao. Simplified calculation method for pressure-impulse curve under blast load [J]. Journal of Vibration and Shock, 2016, 35(13): 224–232 doi: 10.13465/j.cnki.jvs.2016.13.036 [16] CHEN H L, JIN F N, FAN H L. Elastic responses of underground circular arches considering dynamic soil-structure interaction: A theoretical analysis [J]. Acta Mechanica Sinica, 2013, 29(1): 110–122. doi: 10.1007/s10409-013-0012-7 [17] SHI Y, HAO H, LI Z X. Numerical derivation of pressure–impulse diagrams for prediction of RC column damage to blast loads [J]. International Journal of Impact Engineering, 2008, 35(11): 1213–1227. doi: 10.1016/j.ijimpeng.2007.09.001 [18] GANTES C J, PNEVMATIKOS N G. Elastic–plastic response spectra for exponential blast loading [J]. International Journal of Impact Engineering, 2004, 30(3): 323–343. doi: 10.1016/S0734-743X(03)00077-0 [19] LOUCA L A, PUNJANI M, HARDING J E. Non-linear analysis of blast walls and stiffened panels subjected to hydrocarbon explosions [J]. Journal of Constructional Steel Research, 1996, 37(2): 93–113. doi: 10.1016/0143-974X(95)00026-R [20] 刘亚玲, 耿少波, 刘玉存, 等. 钢箱梁抗爆试验中冲击波超压测试方法研究 [J]. 中北大学学报学报: 自然科学版, 2018, 39(5): 609–614, 620 doi: 10.3969/j.issn.1673-3193.2018.05.021LIU Yaling, GENG Shaobo, LIU Yucun, et al. Research on Shock Wave Overpressure Measurement Method in the Anti-Explosion Test of Steel Box Girder [J]. Journal of North University of China (Natural Science Edition), 2018, 39(5): 609–614, 620 doi: 10.3969/j.issn.1673-3193.2018.05.021 期刊类型引用(3)
1. 王滢,赵彩清,王海萍. 隧道内爆炸荷载作用下衬砌及周围非饱和土的动力响应解答. 岩土力学. 2024(04): 1026-1038 .  百度学术
百度学术2. 耿少波,罗干,陈佳龙,赵洲. 阻尼对空爆荷载等效静载动力系数的影响. 爆炸与冲击. 2022(02): 53-61 .  本站查看
本站查看3. 耿少波,陈佳龙,赵洲,牛艳伟. 基于双折线抗力模型的空爆荷载梁式构件振动位移研究. 爆炸与冲击. 2022(10): 139-151 .  本站查看
本站查看其他类型引用(5)
-










 下载:
下载:









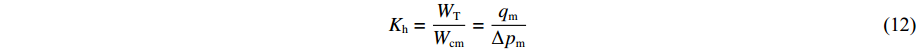
















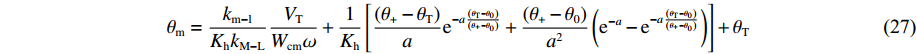




 下载:
下载:


 百度学术
百度学术

