Reliability analysis and design optimization of a shear pin constrained by mechanical boundaries
-
摘要: 剪切销是火工装置关键部件,其可靠性不仅表现为点火作用下可靠剪断,还表现为受力学环境激励不发生断裂。本文中以多项式混沌展开方法为基础,建立了力学环境约束下的剪切销分析模型,结合序贯优化与可靠性分析方法,提出了剪切销可靠性优化设计的思路。以某型火工作动装置为应用实例,依据实用的力学环境,进行了剪切销可靠性分析及优化设计,揭示了设计参数与力学环境之间的关系,并获得了影响可靠性的关键参数。最后,开展了优化后的火工作动装置实验测试,结果佐证了优化设计的有效性。Abstract: The shear pin is a key component of an explosive-actuated device. It must be cut by explosive force and guarantee regular work under mechanical environment in reliability analysis. The mechanical model of the shear pin constrained by the mechanical boundary is built based on the polynomial chaos expansion (PCE) method. Then, the sequential optimization and reliability assessment (SORA) method is adopted to promote a reliability-based design optimization (RBDO) for the shear pin. An explosive-actuated device is selected as an application example of reliability analysis and design, which is based on the idea proposed in this paper. Through the parametric sensitivity analysis of the shear pin, the relationship between designing parameters and mechanical environments is revealed and the effective factors on its reliability are obtained. At last, the explosive-actuated device is manufactured with optimal parameters and works normally under mechanical environment. It is proved that the promoted idea is accurate and useful for the reliability design and optimization of the shear pin under mechanical environments.
-
钻地武器打击会造成建筑结构严重破坏甚至倒塌,而准确预测作用于建筑结构的爆炸荷载是武器毁伤效能和结构抗爆性能评估,以及结构加固与设计的基础。已有规范[1-5]针对球形装药中心起爆的空中爆炸或半球形装药平面中心起爆的地面爆炸工况,给出了爆炸入射和反射冲击波峰值超压随比例距离Z(Z=R/W1/3,R为爆炸中心到目标的距离,W为等效TNT装药质量)的变化规律。此外,诸多学者基于爆炸试验和数值模拟提出了中心起爆的球形装药空中爆炸冲击波入射峰值超压的预测公式[6-12]。与球形装药相比,柱形装药的爆炸荷载还受装药形状(长径比L/D,L和D分别为柱形装药的长度和直径)、起爆方式(起爆点位置和数量)和方位角等因素影响[13-15]。因此,已有针对球形和半球形装药的爆炸荷载预测公式不适用于武器战斗部等柱形弹药爆炸的入射和反射冲击波荷载的计算。
Stoner等[6]基于爆炸试验提出了柱形装药空中爆炸的无量纲入射冲击波峰值超压的计算公式,得出:柱形装药爆炸初始阶段爆炸波接近于柱形,当传播距离超过约20倍装药直径时逐渐演化为球形波。Simoens等[15]指出,柱形装药长径比增大会引起入射冲击波峰值超压和最大冲量在径向增大而在轴向降低。Anastacio等[16]和Knock等[17-19]开展了一系列柱形装药的空中爆炸试验,提出了考虑长径比的径向和轴向入射冲击波峰值超压和最大冲量的计算公式。Gao等[20]基于数值模拟探讨了1≤L/D≤8的柱形TNT装药空中爆炸的入射冲击波峰值超压和最大冲量的分布规律,提出了考虑方位角和长径比影响的入射峰值超压和最大冲量计算公式。Wu等[21]基于爆炸试验和数值模拟研究了柱形装药空中爆炸作用于钢筋混凝土板上的反射冲击波峰值超压和最大冲量分布规律,提出了L/D=1的柱形装药轴向正反射冲击波峰值超压和最大冲量的计算公式。
柱形装药常见的起爆方式有中心、单端和双端起爆。Simoens等[15]基于爆炸试验发现中心起爆时装药两端入射冲击波峰值超压和最大冲量呈对称分布,而单端起爆会造成起爆点相对一侧的峰值超压增大,但对最大冲量分布的影响较小。Hu等[22]认为双端起爆会产生自马赫反射效应,从而增强装药端面和侧面冲击波的相互作用并使其形状更加复杂。Sherkar等[23]通过数值模拟发现起爆方式会影响入射冲击波的形状和峰值超压分布,单端起爆中起爆点同侧的峰值超压较相对一侧小,而当Z≥3 m/kg1/3时,可忽略起爆方式对超压的影响。Xiao等[24]基于数值模拟发现单端起爆会产生最大的爆炸荷载,其入射冲击波峰值超压和最大冲量约为等质量球形装药中心起爆的4.5和4倍,3种起爆方式按其峰值超压大小排列的顺序为:单端、中心和双端。Tham[25]发现双端延时起爆会降低装药径向爆轰产物的膨胀速度并改变其分布的对称性,与单端起爆的峰值超压分布特征相似。
上述工作表明,对柱形装药空中爆炸荷载的研究主要还存在以下不足:(1)现有规范[1-5]和研究[6-12]多基于球形或半球形装药假设,而爆炸荷载受装药形状、起爆方式和方位角等影响,基于球形装药中心起爆提出的计算公式预测柱形装药的爆炸荷载会引起较大误差;(2)现有工作较多针对柱形装药爆炸入射冲击波超压荷载,对于考虑比例距离、长径比、起爆方式、入射角和反射面相对位置等多种因素影响的柱形装药空中爆炸作用于结构的反射冲击波荷载的研究较少;(3)现有针对柱形装药空中爆炸提出的冲击波入射荷载计算方法[20]考虑的比例距离(0.3 m/kg1/3≤Z≤10 m/kg1/3)和长径比(1≤L/D≤8)范围较小,且缺乏充分的试验验证,其结论适用范围有限。
本文中首先开展柱形TNT装药单端起爆的空中爆炸试验,基于有限元软件AUTODYN对本文中试验、Shi等[13]开展的球/柱形装药爆炸试验和UFC 3-340-02规范[4]中球形装药空中爆炸工况进行数值模拟。通过对比数值模拟与试验的入射/反射冲击波超压时程曲线以及规范的入射峰值超压-比例距离关系,对采用的材料模型参数和网格尺寸的适用性进行充分验证。进一步基于验证的有限元分析方法,开展考虑比例距离、长径比、起爆方式、方位角和入射角及反射面相对位置等多种因素影响的共48组柱形装药空中爆炸冲击波自由场传播和960组冲击波刚性反射工况的数值模拟。基于计算结果对柱形装药空中爆炸入射冲击波峰值超压和最大冲量及其形状因子的分布特征、反射冲击波超压和反射系数的变化规律以及峰值超压/最大冲量临界比例距离的判定准则和确定方法进行探究。最终提出柱形装药空中爆炸入射和反射冲击波峰值超压和持时的计算方法,并开展试验对比验证,以期为柱形装药空中爆炸冲击波入射和反射超压荷载的快速计算提供参考。
1. 柱形装药爆炸试验和数值模拟
为验证有限元分析方法的可靠性,首先开展柱形TNT装药空中爆炸试验,获取3组比例距离下冲击波入射/反射超压时程曲线。进一步基于AUTODYN软件对本文中试验、Shi等[13]试验和UFC 3-340-02规范[4]工况进行数值模拟,对采用的材料模型和参数以及网格尺寸的适用性进行充分验证。
1.1 爆炸试验
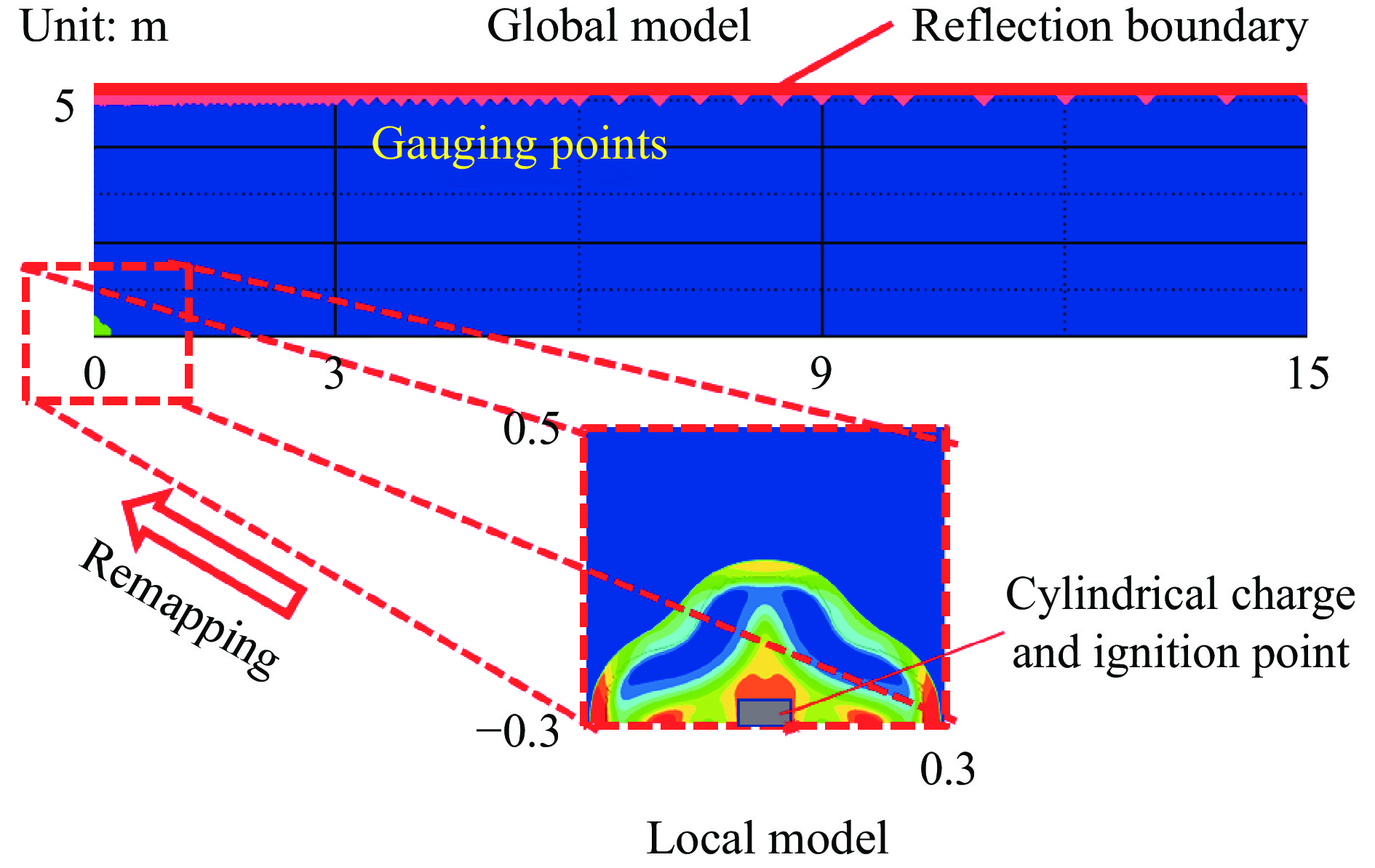
图1给出了爆炸试验设置和传感器布置,其中柱形TNT炸药长度L=224 mm,直径D=120 mm,质量W=4 kg,起爆点位于装药一端中心。在装药中心径向距离R处布置反射超压传感器Pr2,并在其两侧水平距离各0.5 m处等高布置反射超压传感器Pr1和Pr3。共开展3次爆炸试验,R分别为0.95、1.27和1.59 m。每次试验中,在装药径向距离为4.85 m处,以及起爆点相对一侧距离为4.96和7.75 m处分别等高布置笔式自由场超压传感器Pi1、Pi2和Pi3。
1.2 数值模拟
图2给出了对爆炸试验进行数值模拟的有限元模型。基于AUTODYN软件和重映射策略建模,包括局部和全域模型。局部模型尺寸为0.6 m×0.5 m,通过填充建立柱形TNT炸药,起爆点位于装药一端(靠近Pr1),可计算得到冲击波产生和传播初始阶段的高精度结果。全域模型为含测点的整体空气域模型,分为模拟冲击波入射和反射的入射模型和反射模型,测点按实际位置布置(Pi1~Pi3和Pr1~Pr3),尺寸分别为8.0 m×5.0 m(入射)和2.0 m×1.59 m(反射),目的是高效求解冲击波的传播和反射过程。计算过程中,首先计算局部模型,然后将求解结果作为初始条件映射到全域模型中计算得到最终结果。模型为2D轴对称模型并采用Euler, 2D multi-material求解器求解,分别通过Fill unused和Flow out命令模拟刚性反射和自由边界。
空气采用AIR材料模型和理想气体状态方程:
p1=(γ−1)ρe0 (1) 式中:p1为压力;γ为空气常数,取1.4;密度ρ=1.225 kg/m3;比内能e0=206.8 kJ/kg。炸药采用TNT材料模型和Jones-Wilkins-Lee状态方程:
p2=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωEV (2) 式中:p2和V分别为爆轰产物压力和相对体积;E是单位体积初始内能,取6.0 GJ/m3,A=373.77 GPa、B=3.7471 GPa、R1=4.15、R2=0.9和ω=0.35为材料常数。
为平衡计算精度和效率,首先进行网格敏感性分析。局部和全域模型分别依次采用0.8~3.0 mm和8~25 mm网格尺寸计算,在Z=0.3,1.0 m/kg1/3处布置测点获取不同网格尺寸下的入射冲击波压力时程曲线,如图3所示。可以看出,当网格尺寸分别小于1和10 mm时,计算结果收敛。因此分别取1和10 mm作为局部和全域模型的网格尺寸进行有限元建模和计算。
1.3 结果对比
1.3.1 试验
图4分别给出了试验中Pi1、Pi2和Pi3等测点处的入射冲击波超压时程曲线,以及比例距离分别为0.6、0.8和1.0 m/kg1/3时Pr3测点处的反射冲击波超压时程曲线的试验和数值模拟结果。可以看出,数值模拟和试验结果吻合较好,说明建立的有限元模型以及采用的材料模型和参数、网格尺寸等可以较好地预测柱形装药空中爆炸冲击波的入射和反射荷载。
1.3.2 Shi等[13]试验
Shi等[13]开展了一系列1 kg球形和柱形TNT装药(L/D=1,垂直放置)的爆炸试验。装药悬吊于空中,起爆点均位于顶部中心,下方0.8 m固定一块1.50 m×1.20 m×0.025 m的钢板,板上布置反射超压传感器Pr1~Pr5:正下方布置Pr2,Pr2两侧沿钢板短边方向各0.2 m处分别布置Pr1和Pr3,Pr2一侧沿钢板长边方向0.4 m和0.776 m处分别布置Pr4和Pr5。此外,距装药1.31 m处等高布置自由场超压传感器Pi6。试验设置和有限元模型如图5所示,其中有限元模型材料参数和网格尺寸同1.2节。图6给出了球形装药典型测点处的入射(Pi6)和反射(Pr5)超压时程曲线,以及柱形装药在Pr1处反射超压时程曲线的试验和数值模拟结果对比。可以看出,计算结果与试验结果吻合很好,验证了采用的有限元分析方法对预测球形和柱形装药爆炸荷载的适用性。
1.3.3 UFC 3-340-02规范[4]
UFC 3-340-02规范[4]给出了球形TNT装药中心起爆入射峰值超压与比例距离的关系。对其建模分析,如图7所示,采用1 kg球形装药中心起爆空中爆炸,材料模型参数和网格尺寸同1.2节,并在不同方位角和距离处布置测点记录冲击波压力时程曲线。计算结果与规范对比如图8所示,二者吻合良好,再次验证了采用的有限元分析方法的适用性。
2. 柱形装药空中爆炸有限元模型
采用有限元分析方法,分别对柱形装药空中爆炸冲击波自由场传播和遇刚性墙反射两类工况进行有限元建模。考虑比例距离Z、装药长径比L/D、起爆方式和方位角α,以及入射角θ和刚性墙相对位置的影响,分别建立48组空中爆炸的自由场模型和960组刚性反射场模型。
2.1 空中爆炸的自由场模型
柱形装药空中自由场爆炸共考虑12组装药长径比(0.25≤L/D≤10)和3种起爆方式(中心、单端和双端起爆),装药质量为1 kg,如图9(a)~(b)所示。规定装药轴向为0°/180°方向,装药径向为90°/270°方向,空气域中任一点的方位角α定义为该点到装药中心的连线与装药轴线0°方向的夹角,如图9(c)所示。图10给出了相应的有限元模型,其中局部和全域模型尺寸分别为0.6 m×0.5 m和15 m×15 m,材料参数和网格尺寸同1.2节,并在不同方位角和距离处布置测点记录冲击波压力时程曲线。为提高计算效率,根据装药形状和冲击波传播特征分别对中心起爆和双端起爆建立2D的1/4轴对称模型,对单端起爆分别建立起爆点位于0°和180°方向的2D的1/2轴对称局部模型。
2.2 空中爆炸的反射场模型
对柱形装药空中爆炸冲击波在轴向和径向遇刚性墙发生反射的工况进行有限元建模,装药长径比和起爆方式同图9。图11分别给出了3种起爆方式下柱形装药轴向和径向冲击波反射示意图。对于柱形装药轴向和径向垂直于刚性墙,在比例距离Z为1~5 m/kg1/3范围内设置刚性墙并布置测点记录冲击波反射超压时程曲线。规定刚性墙上任一点的入射角θ为冲击波入射方向(即装药中心指向墙面该点方向)与墙面法线方向构成的锐角,此处到装药在刚性墙的投影点的距离为投影距离Dp。对中心和双端起爆工况建立1/4轴对称模型,即在装药轴向和径向一侧建立刚性墙,如图11(a)所示。对单端起爆工况,在起爆点一侧(0°方向)和相对一侧(180°方向)分别设置刚性墙,如图11(b)~(c)所示。单端起爆径向反射工况中,规定起爆点同侧的入射角为θ,相对一侧的入射角为−θ。此外,需按照上述建模策略建立无刚性墙的有限元模型以获取入射波超压时程曲线。有限元模型材料参数、网格尺寸和反射边界同1.2节。以L/D=1的柱形装药中心起爆为例,图12给出了Z=5 m/kg1/3时径向反射场的有限元模型和测点布置。
3. 柱形装药空中爆炸入射冲击波荷载
基于2.1节48组柱形装药空中爆炸冲击波自由场传播有限元模型的计算结果,对冲击波在理想空气中的传播规律进行分析,包括入射冲击波的特征、峰值超压pso、最大冲量Iso和峰值超压/最大冲量形状因子Sp和SI分布,提出基于Sp和SI的临界比例距离的判定准则和确定方法,以及柱形装药空中爆炸pso、Iso和等效正相持时td,e的计算方法。
3.1 入射冲击波特征
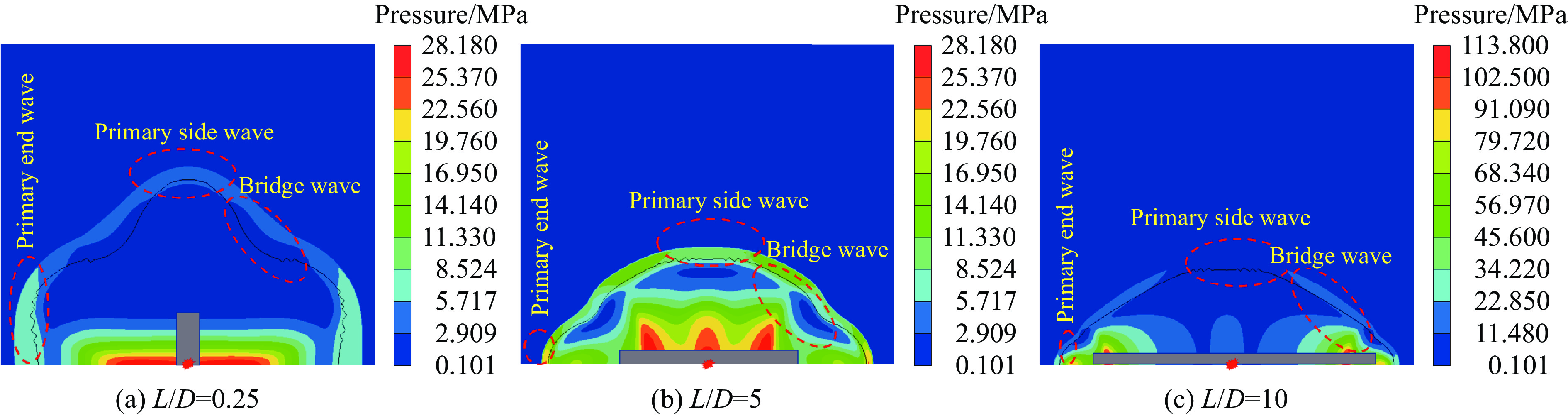
柱形装药的入射冲击波传播特征与装药形状密切相关。如图13(a)所示,以L/D=1装药中心起爆为例,根据装药方向将周围空气域分为轴向区和径向区,二者之间称为过渡区。装药爆炸时在端部和侧面方向分别产生端部主波和侧面主波,二者相遇形成为桥波。不同方位角的冲击波强度不同,端部和侧面主波压力高于桥波,原因在于装药端面和侧面是主要的能量释放部位,对应区域压力较高。图13给出了L/D=1的柱形装药在3种起爆方式下的冲击波压力云图。可以看出:中心和双端起爆压力云图呈现明显对称性,后者爆轰波从两端面同时产生并在径向相遇合成增强的侧面主波,波形较前者更加尖锐;而单端起爆波阵面不对称,在起爆点一侧端部主波强度弱于相对一侧,且侧面主波靠近起爆点的部分也弱于另一侧。图14给出了典型长径比的柱形装药在中心起爆下的冲击波压力云图,当L/D=0.25时,轴向区和过渡区范围较大,端部主波波阵面近似为平面波,随着L/D增大,装药侧面与端面面积之比增大,二者释放的爆炸能量之比也随之增大,在压力云图上表现为轴向区减小和径向区扩大。图15进一步给出了L/D=1的柱形装药中心起爆在不同时刻的冲击波压力云图。可以看出:入射波在空气中自由传播的初始阶段波阵面形状近似为椭球形,波阵面压力分布不均,随着距离增大波阵面逐渐演变为球形,压力值也逐渐趋于一致。
3.2 入射冲击波峰值超压
3.2.1 峰值超压分布特征
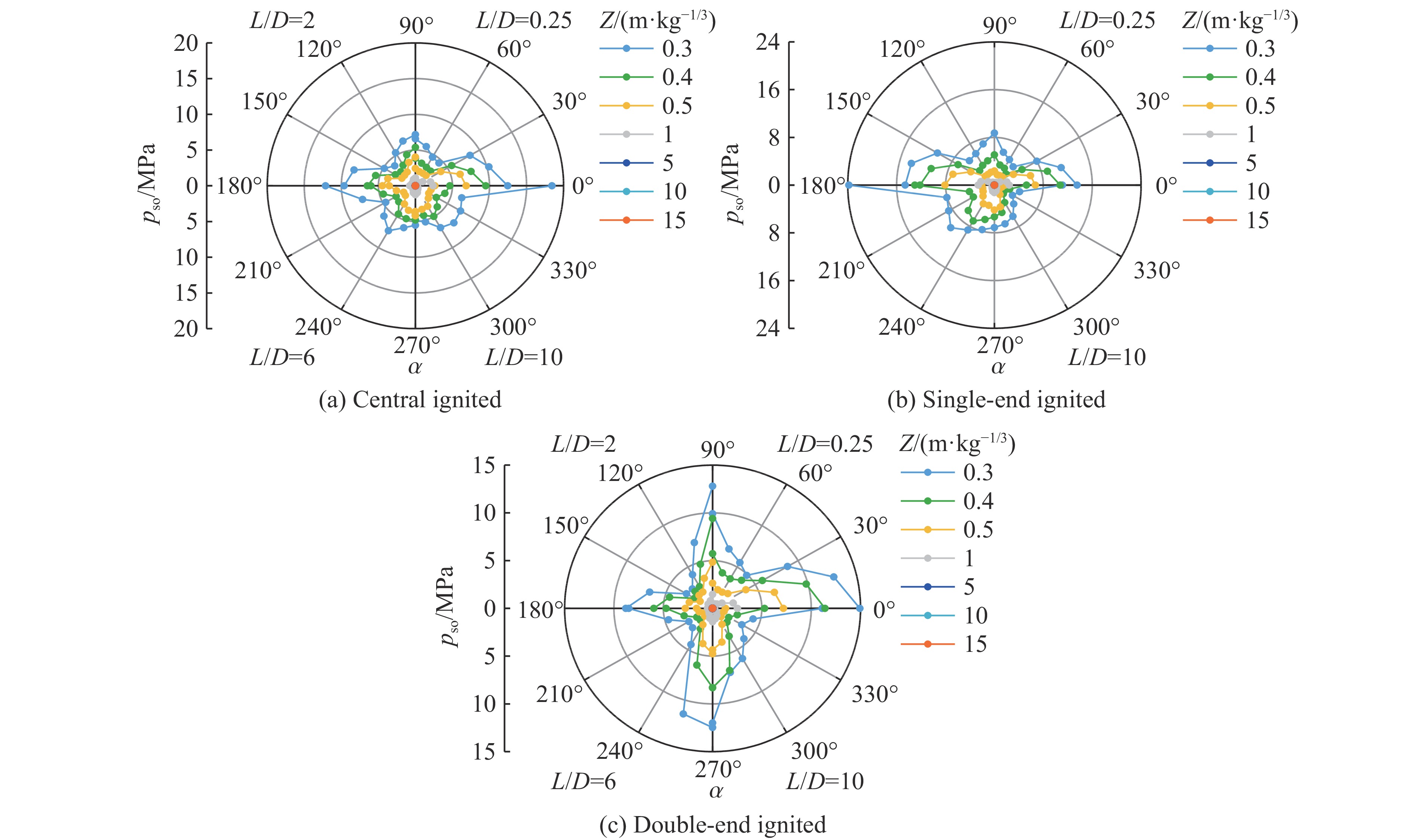
以长径比L/D=0.25的柱形装药中心起爆为例,图16(a)第一象限给出了各方位角入射峰值超压pso的分布,其中水平轴为装药中心轴。可以看出:不同各方位角的pso在比例距离Z较小时差异较大,轴向区和径向区pso明显大于过渡区;随着Z增大,等压线逐渐趋近于圆形,标志着各方位角的pso趋于一致,即柱形装药的冲击波随Z增大逐渐演变为球形波。
不同长径比柱形装药中心起爆的入射峰值超压分布存在较大的差异。图16(a)还给出了其他典型长径比柱形装药中心起爆的入射峰值超压分布,可以看出:在0.25≤L/D≤10范围内,随着L/D不断增大,轴向pso先减小后增大,位于轴向区其他方向的pso也存在相同趋势;径向的pso随L/D增大先增大后减小,但减小的幅值较低,且径向区范围不断增大。上述变化的原因在于,随着L/D的增大,端面与侧面的表面积和爆轰产物分布的变化引起了炸药能量释放路径的变化,从而造成不同方位角的pso变化。此外,随着L/D增大,pso的分布差异逐渐减小,如7≤L/D≤10时,pso值和分区差异均较小。原因在于,随着L/D增大,柱形装药的侧面面积远大于端面面积,径向区和过渡区远大于轴向区范围,而端面直径变化几乎可以忽略,因此爆轰产物和初始爆轰波的变化不大。
此外,起爆方式的改变也会引起入射峰值超压的分布差异。图16(b)、(c)分别给出了典型长径比柱形装药单端和双端起爆下pso的分布情况。可以看出:对于单端起爆,以L/D=0.25装药为例,在较小比例距离处,由于起爆点的不对称性,180°方向(起爆点相对一侧)pso明显高于0°方向(起爆点一侧);而单端起爆中径向的pso则低于中心起爆,且随着L/D的增大,0°方向pso不断减小而180°方向pso不断增大,不对称性愈加明显;对于双端起爆,径向和轴向pso明显高于中心起爆,而过渡区的超压值则小于后者,超压分布曲线呈现十字形,随着L/D不断增大,十字形横轴缩小而纵轴增大,差异逐渐减小。
3.2.2 峰值超压形状因子分布特征
由上述分析可知,柱形装药爆炸入射波波阵面随比例距离Z的增大逐渐趋近于球面波。为定量分析两种形状装药爆炸冲击波超压的关系,Simones等[26]提出了峰值超压形状因子Sp,定义为:
Sp=pso/psopsps (3) 式中:pso和ps分别代表等质量的柱形和球形装药在同一距离处的入射峰值超压。
基于48组自由场模型的计算结果和UFC 3-340-02规范[4]中相应的球形装药中心起爆的冲击波超压值,计算得到柱形装药的Sp。图17分别给出了典型长径比柱形装药在3种起爆方式下Sp的分布。可以看出,当Z较小时,轴向区Sp较大而径向区Sp较小,随着Z增大,Sp逐渐趋近于定值。
以L/D=0.25装药为例,图18(a)进一步给出了中心起爆方式下柱形装药的峰值超压形状因子随比例距离的变化曲线。可以看出,当Z较小时各方位角的Sp变化较大,当Z超过某一值后Sp趋于1,即装药形状对入射峰值超压的影响可以忽略,该比例距离称为峰值超压临界比例距离Zc,p。Sherkar等[23]认为当Sp≤1.1时可忽略装药形状对超压的影响,因此Sp=1.1时所对应的Z为Zc,p,此为Zc,p的第一判定准则。
由于比例距离相等时柱形装药在各方位角的Sp不一致,保守估计选取最大的Sp(即Sp,max)进行比较。图18(b)给出了中心起爆方式下不同长径比柱形装药爆炸冲击波在不同方位角处Sp,max与Z的关系。可以发现:存在较大比例距离处Sp>1.1的情况。根据《爆破安全规程》[5]和相关研究[27],认为2种形状装药在同一比例距离处pso的差值小于2 kPa时,对结构影响的差距可忽略,即二者等效,此为Zc,p的第二判定准则。
根据上述判定准则,可分别求得不同L/D柱形装药在3种起爆方式下的Zc,p,如图19所示。可以看出:当L/D<1时,3种起爆方式的Zc,p均随L/D的增大而减小;当L/D≥1时,中心起爆和单端起爆的Zc,p变化趋势相同,随L/D的增大逐渐递减并趋于平缓,双端起爆则相反。
3.2.3 峰值超压计算公式
Knock等[28]提出了柱形装药轴向入射峰值超压经验计算公式:
pso=A(L/LDD)1/3Z3+B(L/LDD)2/9Z2+C(L/LDD)1/9Z (4) 式中:入射峰值超压pso单位为kPa,A、B和C为拟合参数。式(4)中未考虑柱形装药起爆方式和方位角α的影响。基于48组自由场模型计算结果,可进一步拟合得到综合考虑L/D、Z、α和起爆方式等因素影响的系数值。篇幅所限,表1仅给出3种起爆方式下L/D≥1工况部分角度的系数取值,比例距离适用范围为0.3 m/kg1/3≤Z≤15 m/kg1/3。
表 1L/D≥1 柱形装药入射峰值超压拟合公式系数Table 1. Fitted formula coefficients for peak incident overpressure of central ignited cylindrical charges withL/D≥1 起爆方式 α/(°) 0.3 m/kg1/3≤Z≤1 m/kg1/3 1 m/kg1/3<Z≤15 m/kg1/3 A B C R2 A B C R2 中心起爆 0 173 9 399 0.943 7 −211 390 31 0.612 6 30 −33 503 −166 0.904 5 −200 424 40 0.902 7 60 −103 958 −491 0.988 3 −202 644 −11 0.969 0 90 −242 1 352 −141 0.926 4 281 501 −11 0.986 3 单端起爆 0 −56 1 013 −900 0.884 5 −491 619 22 0.616 4 30 −39 365 −96 0.800 2 −284 546 1 0.880 1 60 60 −131 693 0.940 7 −114 509 9 0.961 8 90 −225 1 438 −244 0.941 8 306 512 −19 0.976 8 120 −205 1 732 −1 360 0.977 9 −238 721 −16 0.969 6 150 −27 580 −261 0.943 1 −171 431 43 0.915 8 180 77 1 246 −1 057 0.909 9 −531 574 34 0.775 3 双端起爆 0 −25 865 −796 0.875 3 −887 1 096 −56 0.733 9 30 −29 304 −24 0.793 5 −313 593 4 0.914 4 60 29 22 602 0.954 3 −69 505 18 0.968 2 90 −255 2 054 −1 068 0.923 7 187 694 −74 0.932 8 3.3 入射冲击波最大冲量
3.3.1 最大冲量分布特征
定义入射冲击波最大冲量Iso为正相超压-时间(p-t)曲线围成的面积,即:
Iso=∫p(t)dt (5) 以L/D=0.25装药中心起爆为例,图20(a)给出了柱形装药中心起爆入射冲击波Iso随比例距离Z和方位角α的分布。可以看出:与超压分布类似,当比例距离较小时,不同方位角的Iso差异较大,并随Z的增大逐渐减小,Iso曲线演变为圆形。不同的是,Iso曲线出现了穿透现象,即在某些方位角(如α=0°),当Z1>Z2时,Iso,1>Iso,2,表明柱形装药的Iso并非随Z增大单调衰减。其原因在于:冲击波在空气中自由传播,波阵面以超声速传播,而正压区尾部以声速传播,造成正压区不断拉长,即正压区持时增大,虽然空气压力持续减小,但最大冲量仍可能增大;柱形装药在特定的方位角会出现由于装药形状引起的二次冲击波波峰,总冲量增大,这与Knock等[19]的试验结论一致。
图20(b)~(c)进一步给出了典型长径比柱形装药在单端和双端起爆下的最大冲量分布。可以看出:以柱形装药中心起爆为例,当L/D较小时,最大冲量Iso在轴向区较大,随着L/D增大,Iso峰值向径向区转移;与中心起爆不同,单端起爆下起爆点一侧的Iso明显低于相对一侧,Iso分布随L/D的变化趋势与中心起爆相同;双端起爆下随着L/D增大,径向区的Iso的增大程度明显高于中心起爆工况,而过渡区的Iso衰减更加明显。其原因在于双端起爆下爆轰波从两端面同时产生,其在径向合成的侧面主波压力幅值更高,因而径向区冲量更大,而过渡区压力也因此迅速衰减。
3.3.2 最大冲量形状因子分布特征
与峰值超压类似,为评价装药形状对入射冲击波最大冲量的影响,定义最大冲量形状因子SI为:
SI=Iso/IsoIsIs (6) 式中:Iso和Is分别代表等质量的柱形和球形装药在同一比例距离处的最大冲量。
图21给出了典型长径比柱形装药在3种起爆方式下SI的分布情况。以L/D=0.25装药中心起爆为例,可以看出:SI在比例距离Z较小时分布极不规则,在装药表面积较大的端面突出,随着Z增大逐渐趋向于圆形。随着L/D增大,轴向区SI逐渐减小而径向区SI逐渐增大,与Iso的变化趋势一致;单端和双端起爆的情况基本类似,但前者在Z较大时SI趋向于卵状,起爆点一侧SI较小,而双端起爆在比例距离较大时SI趋于扁平的椭圆状,其中长轴为装药轴向。此外,3种起爆方式下随着Z变大,SI趋向于某个小于1的值,说明柱形装药的最大冲量Iso在一定比例距离范围内将持续低于等质量球形装药的Iso。
与峰值超压形状因子Sp相同,当各方位角中最大的SI不超过1.1时,可忽略装药形状对最大冲量的影响,对应的Z为最大冲量临界比例距离Zc,I。图22~23分别给出了柱形装药在3种起爆方式下的SI-Z、SI,max-Z和Zc,I-L/D曲线。可以看出:3种起爆方式下Zc,I均随L/D增大不断减小并趋于定值,说明随着L/D的增大,装药形状引起的冲量差异逐渐减小。进一步可拟合得到Zc,I与L/D的关系式,为:
Zc,I=ke(a−L/D)/c+b (7) 式中:k、a、b和c分别为拟合系数,取值见表2所示。
表 2 最大冲量临界比例距离拟合公式系数Table 2. Coefficients of fitted formula for the maximal impulse critical scaled distance起爆方式 拟合公式系数 k a b c R2 中心起爆 2.13 0.25 1.14 0.32 0.945 0 单端起爆 2.48 0.24 1.09 0.61 0.981 2 双端起爆 2.60 0.23 0.97 0.46 0.927 8 3.3.3 最大冲量计算公式
由3.3.1节可知,在轴向区±15°范围内,入射最大冲量Iso沿比例距离Z的变化较复杂,难以进行准确拟合。本节针对不同起爆方式下柱形装药其余方位角的Iso随Z'的变化规律进行拟合,得到:
Iso=iso3√W={3∑i=1[(Ai,1+Ai,2(L/D)i/9+4∑j=1(Ai,j,1+Ai,j,2(L/D)i/9)sin(jα))/Z′i]3√W3∑i=1[(Ai,1+Ai,2(L/D)i/9+4∑j=1(Ai,j,1+Ai,j,2(L/D)i/9)cos(jα))/Z′i]3√W (8) 式中:iso为比冲量,
Z′=R/RWW2/233 为名义比例距离,Ai,1、Ai,2、Ai,j,1和Ai,j,2为拟合系数,取值见表3,适用范围分别为0.3 m/kg2/3≤Z′ <0.8 m/kg2/3和0.8 m/kg2/3≤Z′ ≤15 m/kg2/3,篇幅所限仅给出前者系数取值。表 3 最大冲量拟合公式系数Table 3. Coefficients of the fitted formula for the maximal impulse起爆方式 公式拟合系数 A1,1 A1,2 A1,1,1 A1,1,2 A1,2,1 A1,2,2 A1,3,1 A1,3,2 A1,4,1 A1,4,2 中心 2 088 −2 044 1 222 −1 043 −4 422 4 503 2 583 −2 715 −971 1 101 单端 341 −110 276 −257 186 −163 247 −236 −27 105 双端 640 −384 1 304 −783 −2077 1 352 1 026 −620 85 −190 起爆方式 A2,1 A2,2 A2,1,1 A2,1,2 A2,2,1 A2,2,2 A2,3,1 A2,3,2 A2,4,1 A2,4,2 中心 −543 568 −550 459 1 321 −1 436 −677 824 173 −293 单端 −135 21 −127 101 −43 22 −106 95 −40 −23 双端 −88 −123 −516 225 528 16 −154 −126 −174 250 起爆方式 A3,1 A3,2 A3,1,1 A3,1,2 A3,2,1 A3,2,2 A3,3,1 A3,3,2 A3,4,1 A3,4,2 中心 45 −50 60 −49 −110 134 42 −73 3 21 单端 18 −1 17 −12 7 −2 13 −11 11 2 双端 15 28 57 −16 −63 −33 12 34 25 −38 3.4 入射冲击波持时
图24给出了TNT炸药空中爆炸理想入射(蓝实线)和反射(红实线)冲击波超压时程曲线。忽略负压的影响[29],入射和反射冲击波超压时程曲线可近似简化为无升压时间的突加三角形线性衰减荷载(浅色实线)。对于同一位置,认为入射和反射冲击波超压正相持时td相等[4],基于等冲量原则可得:
td,e=2Iso/Isopsopso (9) 式中:td,e为入射(反射)冲击波等效正相持时。不同爆炸比例距离、长径比、起爆方式和方位角的柱形装药入射冲击波峰值超压pso和最大冲量Iso可分别由式(4)和(8)计算得到。
4. 柱形装药空中爆炸反射冲击波荷载
对2.2节中960组柱形装药空中爆炸反射场有限元模型的计算结果进行分析,讨论柱形装药长径比L/D、起爆方式、入射角度θ、装药相对于刚性墙的方向(轴/径向)以及垂直比例距离Z对反射冲击波峰值超压pr和超压反射系数μ的影响。
4.1 反射冲击波峰值超压
基于图11所示的爆炸工况,典型轴向反射峰值超压-投影距离(pr-Dp)曲线如图25所示。以长径比L/D=1柱形装药中心起爆为例(图25(a)),可以看出:正反射超压(Dp=0,θ=0°)随比例距离Z增大不断减小,与入射峰值超压的衰减规律一致。3种起爆方式的反射峰值超压pr均与投影距离Dp呈负相关关系:当Z较小时,pr在墙面上随投影距离Dp增大呈现逐渐减小的趋势;随着Z增大,pr随Dp的增大呈现先增大后减小的趋势。如图26所示,不同长径比的pr随Dp增大整体保持负相关关系,随着长径比增大,pr随Dp的增大出现先增后减的趋势。原因在于:轴向工况装药端面正对墙面,L/D较大时装药端面面积较小,能量释放较少,而侧面面积较大,能量释放较多;随着Dp增大,装药侧面取代端面成为墙面承受的爆炸能量的主要释放源,因此出现pr先增大后减小的趋势。
4.2 冲击波超压反射系数
为辅助结构设计,定量分析柱形装药反射与入射峰值超压的关系,定义峰值超压反射系数μ为:
μ = pr/prpsopso (10) 式中:pso和pr分别为同一位置处的入射和反射冲击波峰值超压。
图27给出了典型长径比柱形装药中心起爆的超压反射系数-入射角(μ-θ)的关系,图中深色和浅色实线分别代表轴向和径向,图例的超压值代表垂直比例距离为Z处的正入射峰值超压pso(θ=0°)。可以发现:pso(θ=0°)越大,μ的初始值(θ=0°)越大,并随入射角θ的增大而递减;随着Z逐渐增大,pso和pr减小,μ的初始值也减小,μ随θ增大而下降的趋势也变缓;L/D和Z较小时,轴向反射系数μ衰减较径向快,随着L/D增大,轴向的μ值衰减趋缓而径向的μ值衰减加剧,随着Z的增大,轴向和径向的μ值衰减均趋于平缓。需要指出的是:μ下降过程中出现的小阶跃可能由冲击波在刚性墙上产生的马赫波或柱形装药的二次波峰引起。此外,θ=90°代表无限远处,此时pso=pr,即μ=1。
由于单端起爆的不对称性,需要对轴向和径向分别进行阐述。图28(a)给出了L/D=0.25柱形装药单端起爆时轴向反射系数与入射角(μ-θ)的关系曲线,其中深色和浅色实线分别代表起爆点同侧(0°)和相对一侧(180°)。可以看出:等垂直比例距离Z处起爆点同侧(0°)的正入射峰值超压pso(θ=0°)大于相对一侧(180°),对应的反射系数初始值μ(θ=0°)规律相同;随着θ增大,两侧的μ值均大体呈现递减并发生小阶跃的趋势。图28(b)给出了径向的μ-θ关系,其中横坐标沿用2.2节中关于入射角±θ的规定。可以看出,起爆点位置不对称造成μ值在起爆点两侧的不对称,即μ(−θ)>μ(θ)。图28(c)进一步给出了双端起爆时轴/径向反射系数与入射角(μ-θ)的关系曲线,其变化趋势与中心起爆基本相同。
5. 柱形装药爆炸荷载计算方法与验证
基于上述工作,可以建立柱形TNT装药空中爆炸的爆炸荷载计算方法。首先,输入柱形装药的长径比L/D、装药质量W、目标距离R和起爆方式,根据式(7)和图19、23得到峰值超压和最大冲量的临界比例距离Zc,p和Zc,I,然后判断目标位置的比例距离Z是否在临界比例距离Zc,p和Zc,I之外。一般情况下Z同时满足大于Zc,p和Zc,I即可认为装药形状无影响,此时通过UFC 3-340-02规范[4]中关于球形装药爆炸荷载公式进行计算,否则需按照柱形装药进行计算。
当Z在临界比例距离内时,输入目标位置相对于装药的方位角α,通过式(4)、(8)和(9)以及表1和表3计算得到入射冲击波荷载,包括入射峰值超压pso、最大冲量Iso和等效正相持时td,e。然后输入入射角θ,基于数值计算得到的反射系数μ,结合式(10)计算得到反射峰值超压pr。此时,得到了作用于结构任一点处的突加三角形荷载的反射峰值超压pr和等效正相持时td,e,可直接用于结构计算与设计。上述方法适用范围为:0.25≤L/D≤10,0.3 m/kg1/3≤Z≤15 m/kg1/3(入射)和1 m/kg1/3≤Z≤5 m/kg1/3(反射),目标物迎爆面垂直和平行于装药轴向,以及中心、单端和双端起爆等3种起爆方式。
基于文献[16,19,21,28,30-33]中共360余组柱形装药空中爆炸试验数据,对上述计算方法进行验证。 Knock等[19,28]开展了一系列0.1 kg≤W≤5 kg、4≤L/D≤6的柱形PE4装药,以及0.14 kg≤W≤0.42 kg、5≤L/D≤6.8的柱形B炸药单端起爆的空中爆炸试验,获取了装药轴向和径向的入射峰值超压pso;Wisotski等[30]开展了0.7 kg≤W≤3.7 kg、0.25≤L/D≤10的柱形B炸药单端起爆的空中爆炸试验,获取了装药径向的pso;Plooster[31]开展了3.63 kg≤W≤7.26 kg、0.25≤L/D≤6的柱形Pentolite炸药单端起爆的空中爆炸试验,并测得装药径向的pso;Su等[32]开展了一系列W=4 kg、L/D=1.87的柱形TNT装药单端起爆空中爆炸试验并测得其轴向和径向的pso。上述试验中,pso的试验结果与本文中的预测结果对比如图29(a)所示,可以看出提出的计算方法对pso的预测准确性较好。
Anastacio等[16]开展了0.25 kg≤W≤0.45 kg、0.27≤L/D≤5.75的柱形PE4装药双端起爆的空中爆炸试验,在装药径向1~3.5 m范围内布置了压力传感器,得到了一系列入射冲击波最大冲量试验数据,相关的对比结果如图29(b)所示,可以看出预测结果与试验数据吻合很好。
Wu等[21]开展了0.24 kg≤W≤8.0 kg、L/D=1的柱形B炸药单端起爆的空中爆炸试验,获取了比例距离在0.75~3 m/kg1/3范围内的pr和td;Tian等[33]开展了W=4 kg、L/D=1.87的柱形TNT装药单端起爆的空中爆炸试验,获取了Z=1 m/kg1/3处的pr和td。Su等[32]的试验也获得了一系列pr和td。图29(c)~(d)分别给出了上述试验中的pr与相应的计算结果pr,c,以及等效持时td,e与计算等效持时td,ec的对比,可以看出数据基本在±25%误差区间内。考虑到爆炸波测试受海拔、天气和空气温湿度等多种环境因素影响,上述误差对于结构抗爆设计可以接受。需要指出的是,上述计算中炸药量均取等效TNT当量,其中PE4、B炸药、Pentolite炸药和TNT炸药的超压(冲量)等效当量换算系数分别取1.34(1.30)、1.11(0.98)和1.42(1.00)[1, 28, 34]。
6. 结 论
本文中首先开展柱形TNT装药单端起爆的空中爆炸试验和数值模拟,通过与试验和UFC 3-340-02规范进行对比,验证了有限元分析方法的适用性。进一步基于1000余组柱形装药空中爆炸自由场和反射场工况的数值仿真,对柱形装药空中爆炸的冲击波场分布、冲击波传播规律、入射和反射超压荷载进行了研究,主要结论有:
(1)通过分析长径比L/D、起爆方式和比例距离Z对不同方位角α的自由场入射峰值超压pso和最大冲量Iso的影响,得出L/D的增大会引起轴向pso先减小后增大;而径向pso则先增大后减小;其他方位角pso的变化介于二者之间,但其值均小于轴向和径向值;Iso变化规律与之类似;1 kg装药工况下,单端起爆中起爆点相对一侧0.3 m/kg1/3处的pso和Iso约为同侧的1.53倍和1.23倍,双端起爆径向0.3 m/kg1/3处的pso和Iso约为中心起爆的2.47倍和1.68倍。
(2)通过分析装药L/D、起爆方式和Z等对不同α的入射冲击波峰值超压和最大冲量形状因子分布特征的影响,提出了峰值超压和最大冲量临界比例距离的判定准则与确定方法。阐明了3种起爆方式下不同L/D柱形装药空中爆炸反射冲击波峰值超压随刚性墙比例距离Z和投影距离Dp的变化规律,以及轴向和径向冲击波超压反射系数与Z和Dp的关系。
(3)提出了柱形装药空中爆炸入射冲击波荷载(入射峰值超压、最大冲量和等效正相持时)和反射冲击波荷载(反射峰值超压和等效正相持时)的计算方法,并得到试验验证。
-
表 1 火工作动装置工作环境
Table 1. Work situation of the pyrotechnic pin
表 2 影响火工作动装置可靠性的因素
Table 2. Influence factors on the pyrotechnic pin reliability
序号 参数符号 参数名称 参数均值 参数标准差 1 ppow 火药工作产生压强 10 MPa 2.5 MPa 2 S1 火药压力作用面积 500 mm2 5 mm2 3 d 剪切销截面直径 2.5 mm 0.1 mm 4 E 7075铝合金弹性模量 70 GPa 0.005 GPa 5 σb 7075铝合金极限强度 429 MPa 5 MPa 6 σfatigue 7075铝合金真实断裂强度 627 MPa 5 MPa 7 εfatigue 7075铝合金真实断裂延性 0.29 0.000 5 8 μ 泊松比 0.33 0.000 5 9 l 剪切面长度 3.5 mm 0.1 mm 10 ξ 损耗因子 0.005 0.001 11 mcylinderpin 圆柱销质量 15 g 0.1 g 12 acylinderpin 圆柱销加速度 200 m/s2 10 m/s2 13 tvib 振动时长 10 h 1 h 14 σmean 平均预紧力 400 MPa 5 MPa 15 aimpact 起落冲击(发射冲击)加速度幅值 200(30 000) m/s2 100 m/s2 16 timpact 起落冲击(发射冲击)时长 11(3) ms 1 ms 表 3 变量对不同工作环境的不确定性灵敏度
Table 3. Uncertainty sensitivity of variables in different mechanic environments
参数 点火工作 产品发射 载机加速 载机起落 挂载飞行 综合影响系数 τ1 σ1 σ2 τ2 τ3 σ3 σ4 τ4 σ5 ppow 0.260 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.029 S1 0.302 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.034 d 0.289 0.226 0.199 0.178 0.378 0.233 0.236 0.258 0.254 0.250 E 0.000 0.106 0.092 0.128 0.000 0.110 0.064 0.072 0.063 0.070 σb 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.062 0.007 σfatigue 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.046 0.005 εfatigue 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.057 0.006 μ 0.000 0.000 0.000 0.107 0.000 0.000 0.000 0.071 0.000 0.020 l 0.000 0.000 0.126 0.099 0.000 0.000 0.135 0.043 0.000 0.045 ξ 0.000 0.019 0.012 0.010 0.000 0.016 0.019 0.017 0.034 0.014 mcylinderpin 0.000 0.112 0.115 0.090 0.202 0.121 0.065 0.065 0.060 0.092 acylinderpin 0.000 0.000 0.000 0.000 0.203 0.000 0.000 0.000 0.000 0.023 tvib 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.121 0.014 σmean 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.000 0.136 0.015 aimpact 0.000 0.173 0.156 0.130 0.000 0.158 0.173 0.159 0.000 0.106 timpact 0.000 0.197 0.157 0.133 0.000 0.178 0.152 0.141 0.000 0.107 注:τ1、τ2、τ3、τ4为剪切应力,σ1、σ3为弯曲应力,σ2、σ4为拉伸应力,σ5为疲劳破坏应力。 表 4 设计变量初值、取值范围和优化值
Table 4. Initial values, ranges and optimal values of design variables
设计变量 单位 初值 优化值 取值范围 ppow MPa 10.00 13.51 [8, 15] d mm 2.50 1.49 [1, 2] l mm 3.50 2.62 [1, 3] σmean MPa 400 300 [300, 400] -
[1] 荣吉利, 宋乾强, 张涛. 一种预测航天火工装置可靠性的小样本方法 [J]. 宇航学报, 2015, 36(3): 360–364. DOI: 10.3873/j.issn.1000-1328.2015.03.016.RONG Jili, SONG Qianqiang, ZHANG Tao. A small sample method for predicting reliability of space pyrotechnic devices [J]. Journal of Astronautics, 2015, 36(3): 360–364. DOI: 10.3873/j.issn.1000-1328.2015.03.016. [2] 荣吉利, 宋乾强, 张涛, 等. 冗余航天火工装置可靠性评估方法及应用 [J]. 宇航学报, 2013, 34(7): 1021–1026. DOI: 10.3873/j.issn.1000-1328.2013.07.019.RONG Jili, SONG Qianqiang, ZHANG Tao. Reliability assessment method for redundant aerospace pyrotechnic devices and its application [J]. Journal of Astronautics, 2013, 34(7): 1021–1026. DOI: 10.3873/j.issn.1000-1328.2013.07.019. [3] 董海平, 蔡瑞娇, 穆慧娜. 火工品可靠性计量计数评估方法的有效性研究 [J]. 含能材料, 2008, 16(5): 553–555. DOI: 10.3969/j.issn.1006-9941.2008.05.020.DONG Haiping, CAI Ruijiao, MU Huina. Validity of variables attributes assessment method for reliability of initiating explosive devices [J]. Chinese Journal of Energetic Materials, 2008, 16(5): 553–555. DOI: 10.3969/j.issn.1006-9941.2008.05.020. [4] 董海平, 赵霞, 蔡瑞娇. 基于信息等值的火工品可靠性评估小样本方法 [J]. 兵工学报, 2011, 32(5): 554–558.DONG Haiping, ZHAO Xia, CAI Ruijiao. An assessment method of reliability of initiating devices based on information measure equivalency [J]. Acta Armamentarii, 2011, 32(5): 554–558. [5] 董海平, 蔡瑞娇, 穆慧娜, 等. 火工品可靠性的感度参数设计法 [J]. 爆炸与冲击, 2009, 29(6): 613–616. DOI: 10.11883/1001-1455(2009)06-0613-04 .DONG Haiping, CAI Ruijiao, MU Huina, et al. A sensitivity parameter design method for reliability of explosive initiators [J]. Explosion and Shock Waves, 2009, 29(6): 613–616. DOI: 10.11883/1001-1455(2009)06-0613-04 . [6] 伊枭剑, 董海平, 翟志强, 等. 基于应力-强度干涉模型的火工品可靠性设计方法 [J]. 北京理工大学学报, 2014, 34(10): 1007–1011.YI Xiaojian, DONG Haiping, ZHAI Zhiqiang, et al. Reliability design for initiating devices based on stress-strength interference model [J]. Transactions of Beijing Institute of Technology, 2014, 34(10): 1007–1011. [7] OUCH R, UKRITCHON B, PIPATPONGSA T, et al. Finite element analyses of the stability of a soil block reinforced by shear pins [J]. Geomechanics and Engineering, 2017, 12(6): 1021–1046. [8] 孙洁, 郭崇星, 吴瑞德, 等. 拔销器作用过程的仿真研究 [J]. 火工品, 2017(1): 10–13. doi: 10.3969/j.issn.1003-1480.2017.01.004SUN Jie, GUO Chongxing, WU Ruide, et al. Simulation research on the function process of the pin puller [J]. Initiators and Pyrotechnics, 2017(1): 10–13. doi: 10.3969/j.issn.1003-1480.2017.01.004 [9] 高滨. 火工作动装置设计参数的敏感性分析 [J]. 航天返回与遥感, 2006, 27(3): 57–60. doi: 10.3969/j.issn.1009-8518.2006.03.011GAO Bin. Design parameter sensitivity analysis of pyrotechnically actuated devices [J]. Space Craft Recovery and Remote Sensing, 2006, 27(3): 57–60. doi: 10.3969/j.issn.1009-8518.2006.03.011 [10] COLANTUONO T, GROSSONI I, ALLEN P, et al. The optimal strength of shear pins: requirements in point run-throughs [J]. Journal of Rail and Rapid Transit, 2016, 232(2): 2–19. DOI: 10.1177/0954409716682657. [11] SALTELLI A, RATTO M, ANDRES T, et al. Global sensitivity analysis: the primer [M]. Chichester: John Wiley and Sons, 2008. [12] HELTON J C, JOHNSON J D, SALLABERRY C J, et al. Survey of sampling-based methods for uncertainty and sensitivity analysis [J]. Reliability Engineering and System Safety, 2006, 91(10/11): 1175–1209. [13] IMAN R L, HELTON J C. An investigation of uncertainty and sensitivity analysis techniques for computer models [J]. Risk Analysis, 2010, 8(1): 71–90. [14] WIENER N. The homogeneous chaos [J]. American Journal of Mathematics, 1938, 60(4): 897–936. doi: 10.2307/2371268 [15] DU Xiaoping, GUO Jia, BEERAM H. Sequential optimization and reliability assessment for multidisciplinary systems design [J]. Structural and Multidisciplinary Optimization, 2008, 35(2): 117–130. DOI: 10.1007/s00158-007-0121-7. [16] 崔卫东, 关云翔, 惠蕾. 拔销器的小型化设计 [J]. 火工品, 2006(2): 17–19. doi: 10.3969/j.issn.1003-1480.2006.02.005CUI Weidong, GUAN Yunxiang, HUI Lei. Design of the small-sized piston actuator [J]. Initiators and Pyrotechnics, 2006(2): 17–19. doi: 10.3969/j.issn.1003-1480.2006.02.005 [17] 汪凤泉. 电子设备振动与冲击手册 [M]. 北京: 科学出版社, 1998. [18] 苟文选. 材料力学 [M]. 北京: 科学出版社, 2005. [19] 徐鹏. 金属材料应变寿命曲线估算的新方法[D]. 南京: 南京航空航天大学, 2012.XU Peng. A new method for the estimation of strain-life curve of metals [D]. Nanjing: Nanjing University of Aeronautics and Astronautics, 2012. [20] 陈小前, 姚雯, 欧阳琦. 飞行器不确定性多学科设计优化理论与应用 [M]. 北京: 科学出版社, 2013. [21] 王若冰, 谷良贤, 龚春林. 随机-区间混合不确定性分层序列化多学科可靠性分析方法 [J]. 西北工业大学学报, 2016(1): 139–146. DOI: 10.3969/j.issn.1000-2758.2016.01.021.WANG Ruobing, GU Liangxian, GONG Chunlin. A stratified sequencing multi-disciplinary reliability analysis method under random and interval uncertainty [J]. Journal of Northwestern Polytechnical University, 2016(1): 139–146. DOI: 10.3969/j.issn.1000-2758.2016.01.021. [22] 施荣明、朱广荣、吴飒, 等. 军用装备实验室环境试验方法: 第16部分: 振动试验: GJB 150. 16A-2009 [S]. 北京: 总装备部军标出版发行部, 2009: 42; 53. 期刊类型引用(1)
1. 王明涛,程月华,吴昊. 柱形装药空中运动爆炸冲击波荷载计算模型. 爆炸与冲击. 2024(07): 107-125 .  本站查看
本站查看其他类型引用(0)
-








 下载:
下载:






























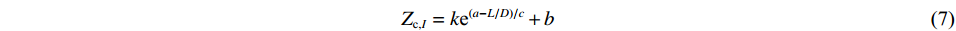
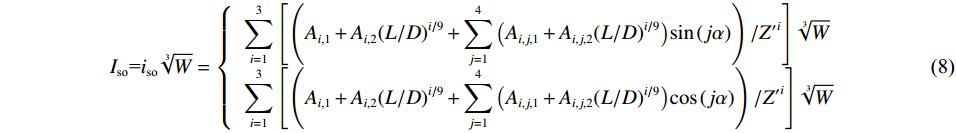




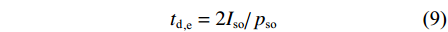


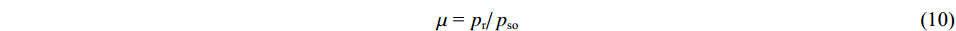




 下载:
下载: