Calculation methods for peak pressure on borehole wall of contour blasting
-
摘要: 爆破孔壁压力峰值是进行非流固耦合爆破动力响应分析的重要参数。针对轮廓爆破孔壁压力峰值的计算方法问题,理论分析了爆炸冲击波与弹性壁面的相互作用,推导了空气冲击波与弹性壁碰撞后压力增大倍数的理论解,并采用流固耦合动力有限元数值分析方法,研究了3种岩体介质、2种轮廓爆破常用炸药、5种常用不耦合系数、2种轴向装药系数工况下轮廓爆破的冲击波碰撞压力增大倍数和炮孔壁压力峰值。结果表明:轮廓爆破时,爆炸冲击波与孔壁碰撞后压力增大倍数并不是常值,与炸药特性、孔壁介质条件、不耦合装药系数等因素相关,孔壁压力峰值也与上述因素密切相关。基于模拟的孔壁压力峰值数据的统计分析,并结合理论推导成果及常用爆破孔壁压力峰值计算形式,提出了一种新的轮廓爆破孔壁压力峰值计算方法。Abstract: The peak pressure on the borehole wall is an important parameter for non-fluid solid coupling dynamic analysis of blasting. Aiming at the method for calculating the borehole peak pressure of the contour blasting, the interaction between explosion shock wave and elastic wall is theoretically analyzed, and the theoretical solution of pressure increase multiple after the collision of air shock wave and elastic wall is derived. The fluid solid coupling dynamic finite element numerical analysis method is used to study the blast air shock wave increase factor and borehole peak pressure under three kinds of rock mass media, two kinds of commonly used explosives for contour blasting, five common decoupling coefficients and two kinds of axial charging coefficient conditions. The results show that: the explosion shock wave increase factor of contour blasting is not a constant, and it is related to explosives characteristics, borehole medium conditions, decouple coefficient and other factors, correspondingly, the borehole peak pressure is also related to the above factors. Based on the statistical analysis results of borehole peak pressure simulation, combined with the theoretical deduction results and the commonly used calculation formula for the peak pressure on borehole wall, a new method for calculating borehole peak pressure in the contour blasting is proposed.
-
轮廓爆破是岩体开挖成型及爆破损伤控制的主要手段,被广泛应用于水利水电、交通及矿山等工程建设领域。作用在爆破孔孔壁的爆炸荷载压力峰值,直接影响到岩体轮廓成型质量及其保留岩体的损伤破坏范围,是进行轮廓爆破参数设计及非流固耦合爆破振动响应数值模拟分析的关键参数之一,受到了爆破工程技术研究人员的高度关注。
传统的轮廓爆破均采用不耦合装药结构,众多研究者分析了不耦合装药条件下爆破荷载峰值。朱瑞赓等[1]、费鸿禄等[2]较早研究了不耦合装药炮孔内的峰值压力计算方法;王志亮等[3]运用数值模拟对水不耦合炮孔的孔壁压力进行了研究,揭示了炮孔中压力的变化规律;凌伟明[4]利用试验方法研究了炮孔壁的峰值压力;王伟等[5]根据爆轰产物中反射波方程和介质中的冲击波方程求解出岩体中爆炸冲击波参数,并与弹性波理论透射公式结果对比,发现两者间的差别很大;刘云川等[6]指出了现有孔壁压力峰值计算方法可能存在的不足,并按照能量等效的原则,提出了炮孔压力峰值的计算公式;Feldgun等[7]利用数值模拟方法研究了爆破荷载的变化过程,数值模拟结果与理论及试验结果非常一致。其他学者[8-10]也研究了炮孔的压力峰值问题,并考虑了轴向装药系数的影响,提出了各种不同形式的计算公式。总体上,由于爆破孔内炸药的爆轰过程、爆炸冲击波与炮孔壁相互作用的复杂性以及爆炸荷载测试技术的局限性,难以直接测量出孔壁压力峰值,而且计算值与实测值的差别也较大:炮孔孔壁压力峰值的问题一直吸引着众多学者的关注。
目前最常用的轮廓爆破孔壁压力峰值计算方法有2种。
第1种常用方法,基于爆生气体的膨胀过程计算。在工程误差允许范围内,将爆生气体的膨胀过程分为等熵膨胀和绝热膨胀2个阶段[11],且考虑爆生气体与炮孔壁的相互作用。爆炸压力大于炸药临界压力的阶段,按等熵膨胀分析;爆炸压力小于炸药临界压力的阶段,按绝热膨胀计算。
若装药不耦合系数较小,则爆生气体的膨胀只有等熵膨胀状态,孔壁的压力峰值:
pr=npw(dcdb)2k=nρeD22(k+1)(dcdb)2k (1) 若装药不耦合系数较大,爆生气体的膨胀需经历等熵膨胀和绝热膨胀2个阶段,孔壁的压力峰值:
pr=(pwpk)γknpk(dcdb)2γ (2) 式中:
pr 为孔壁压力峰值;pw 为平均爆轰压力,pw=(ρeD2)/[2(k+1)] ;dc为装药直径;db为炮孔直径;db/dc为装药不耦合系数;ρe为炸药密度;D为炸药爆速;k、γ为绝热指数,通常取k=3,γ=1.3;n为冲击波碰撞压力增大倍数,n=8~11,一般取n=8;pk 为炸药的临界压力,一般中等威力炸药取为200 MPa。第2种常用方法,基于临近炮孔壁的空气冲击波特性计算。其计算方法:
pr=2nˉk+1ρaD2a (3) 式中:
ρa 为空气密度,取1.25 kg/m3;Da 为孔壁某点空气冲击波的传播速度;¯k 为空气间隙内空气的平均绝热指数,范围为1.17~1.25;n的取值与式(2)中的不同,n=0~20,取决于入射波的压力,参照钮强[12]给出的对应曲线取值。这2种方法都包含冲击波碰撞压力增大倍数n,n取值的大小对孔壁压力峰值的影响最显著。方法1中n取值变化范围较小;方法2认为n随着入射压力的变化而变化。实际上,由于爆炸空气冲击波与炮孔壁相互作用的复杂性,比较合理地确定n值的大小仍有难度,导致轮廓爆破孔壁压力峰值难以准确确定。
综上所述,目前关于轮廓爆破孔壁压力峰值问题仍有待进一步研究,本文采用理论与数值模拟相结合的方法,研究了空气冲击波与爆破孔孔壁相互作用后,爆破孔壁上的压力与空气冲击波强度之间的关系,建议了一种新的轮廓爆破孔壁压力峰值计算方法。
1. 空气冲击波与孔壁的相互作用
由于炮孔为圆柱形,炸药爆炸时炮孔内的空气冲击波作用过程复杂,并存在透、反射的叠加效应。为便于研究,简化爆破孔壁与空气冲击波的作用界面为一平面,并假定爆破孔壁处于弹性状态,此时将炮孔壁简化为图1所示的弹性界面F-F,设入射波I从介质Ⅰ传播到另一种声阻抗不同的介质Ⅱ中,传播方向垂直于界面,即讨论正入射的情况。当入射波扰动到达界面时,不论对于第一种介质而言,还是对于第2种介质而言,都引起了一个扰动,即分别向2种介质中传播反射波扰动R和透射波扰动T[13]。
下面从理论上推求分析空气冲击波与弹性壁的相互作用。
冲击波自左向右传播如图1所示,设
p0 、ρ0 、v0 为入射介质初始压力、密度和质点速度;p1 、ρ1 、v1 为冲击波波后参数,c1 为入射冲击波波速度;p2 、ρ2 、v2 、c2 为反射波波后参数;p3 、ρ3 、v3 、c3 为透射波波后参数;p30 、ρ30 、v30 为透射初始参数。间断面上的冲击波质量、动量、能量守恒关系式就是冲击波的基本关系式[14]:
ρ(c−v)=ρ0(c−v0) (4) ρ(c−v)2+p=ρ0(c−v0)2+p0 (5) e+pρ+12(c−v)2=e0+p0ρ0+12(c−v0)2 (6) 式中:c为冲击波速度;p、ρ、v和e分别为介质的压力、密度、速度和比内能,带下标“0”的量表示波前参数。
多方气体状态方程为[14]:
e=pτγ−1 (7) 式中:τ为比容,γ为绝热指数。
按照动量守恒定律,冲击波传播过程中,单位时间内作用于介质的冲量等于其动量的变化,对透射波则有[14]:
p3−p30=ρ30(c3−v30)(v3−v30) (8) 由式(8)中
p30≈0 ,v30≈0 可得:ρ30c3v3=p3−p30 (9) 假定两介质界面始终保持接触,于是根据牛顿第三定律,界面两侧质点根据应力连续条件得到[15]:
p1+p2=p3 (10) 冲击波在岩石中迅速衰减为弹性应力波,并以恒速传播,对于大多数岩石来说,冲击波作用范围很小,可忽略不计,
c3 可近似为岩石纵波波速c30 。由式(9)可推得:v3=p3ρ30c30 (11) 联立式(4)~(7)、(10)~11)求解可得:
(p3p1)3−I1(p3p1)2+I2(p3p1)−I3=0 (12) 为求解透射波的压力与入射波的压力比值关系,即
p3/p1 ,基于冲击波质量、动量、能量守恒关系,可建立:s3−J1s2−J2s−J3=0 (13) 其中:
J1=0,J2=−I2+13I21,J3=I3−13I1I2+227I31,I1=QP,I2=NP,I3=TP,Q=2B(γ+1)M+2M+A,N=(γ+1)B2+4BM+4A,T=2B2+4A,P=γ+1M2,A=(γ+1γ−1+p1p0)p1p01+(γ+1γ−1)p1p0(2γ),B=√2γ(p1p0−1)2(γ+1)p1p0+(γ−1),M=ρ30c30c0p1,c0=√p0γρ0,s=2√J2√3sinθσ,θσ=13sin−1(−√27J32(J2)3╱2) (14) 式中:
c0 为入射波波前气体声速。利用式(12)中
p3/p1 与式(13)中s的关系得:p3p1=s+13I1 (15) 由式(15)可知:在给定入射压力
p1 和透射介质波阻抗后,便可求解p3/p1 。为探寻压力增大倍数n的影响因素,图2给出了不同绝热指数条件下,绝热指数γ分别取为1.2和1.3;不同入射压力时
p3/p1 与透射介质波阻抗的关系,可以很明显的看出冲击波碰撞炮孔壁后的压力增大倍数n先会随着孔壁材料波阻抗的增大而稍微增大,但是变化幅度较小,尤其是当透射介质波阻抗到一定程度时,n就趋于稳定。入射压力p1 、绝热指数γ对冲击波碰撞压力增大倍数n影响明显,尤其是绝热指数γ,当γ=1.2时,n最大值接近14,远大于8,这是因为高温、高压条件下空气分子会发生离解和电离,不同不耦合系数时爆生气体成分不同,膨胀所经历的绝热指数分布在1.2~3.0之间,并且不同不耦合系数时,炮孔的透、反射的叠加效应也不相同,相应的压力增大倍数有很大变化,说明压力增大倍数n与炮孔介质条件、不耦合系数、炸药性能有关。上述研究中,将炮孔壁简化为平面状态,忽略了反射冲击波因炮孔壁实际上的弧面特征可引起的二次冲击波斜碰撞进一步增大炮孔壁近区的压力,可能导致理论推导的n值较实际的增大倍数偏小。下面采用流固耦合数值分析方法,模拟分析常用的传统轮廓爆破参数条件下的炮孔壁压力峰值。
2. 轮廓爆破孔壁压力峰值的数值模拟
本数值计算中采用LS-DYNA的流-固耦合任意拉格朗日-欧拉(arbitrary Lagrangian-Eulerian,ALE)算法模拟炸药的冲击作用,其中炸药、空气采用Eulerian算法,岩石、堵塞采用Lagrange算法,其原理是实现材料在网格中流动,可有效计算爆炸冲击过程中的大变形问题。
2.1 计算工况
为进一步研究轮廓爆破孔壁压力峰值与周围介质条件、不耦合系数、炸药性能的关系,基于实际工程中常见的工程岩体特性、爆破参数及炸药类型,选择粉砂岩、石灰岩、花岗岩3种岩体分别代表软岩、硬岩及坚硬岩类炮孔介质,选择装药直径与炮孔直径比分别为25/42、25/50、32/76、32/90、32/110的5种常用轮廓爆破不耦合装药结构,选用乳化炸药、多孔粒状铵油炸药2种炸药展开研究。为研究轴向不耦合装药结构时炮孔壁的压力峰值状况,继续建立了轴向装药系数分别为0.6和0.3的对比数值模型,轴向装药系数为装药长度与炮孔药室长度的比值。
2.2 计算模型
因模型具有对称性,采取1/4模型,如图3所示,尺寸为1.5 m×1.5 m×3.0 m,其中堵塞长度为0.5 m,装药长度为1.5 m,孔底岩石长度为1.0 m,为能模拟出炸药的真实爆炸效果以及确保不同工况结果之间具有可比性,模型炸药和空气单元的尺寸控制在2 mm以内,岩石单元尺寸也和炸药、空气单元尺寸相近,不同装药条件下控制模型单元尺寸相同,不同介质条件,不同炸药种类作用时保证模型网格一致,所建立的统一模型的单元数量约为38万,节点数量约为40万。同时计算的时间步也要和模型最小单元尺寸相匹配,以便观察到冲击波与孔壁的透反射效果。为控制模型边界的影响,计算模型中的人工截断边界分别设置为无反射边界和对称边界,如图3所示。
2.3 计算参数
粉砂岩、石灰岩、花岗岩3种岩石材料选用常用的双线性随动硬化塑性模型,并根据Cowper-Symonds计算式考虑应变率对岩石动态强度的影响[16],参照《岩石力学参数手册》[17],各种岩石的参数取值见表1。
表 1 岩石物理力学参数Table 1. Physical and mechanical parameters of rock岩石种类 密度/(kg·m−3) 泊松比 弹性模量/GPa 屈服应力/MPa 切线模量/GPa 粉砂岩 2 170 0.25 6.70 39.20 0.6 石灰岩 2 600 0.25 32.5 72.9 3.0 花岗岩 2 700 0.24 68.00 150.00 7.0 注:Cowper-Symonds 参数 C 取 2.5 s−1,Cowper-Symonds 参数 P 取 4.0。 炸药选用MAT_HIGH_EXPLOSIVE_BURN材料模型,并结合JWL状态方程模拟炸药爆炸过程中压力与体积的关系:
p=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωE0V(16) 式中:p为JWL状态方程决定的压力,V为相对体积,E0为初始比内能,A、B、R1、R2和
ω 为描述JWL方程的独立常数。以上参数的取值方法均参考LS-DYNA用户手册[16]。乳化炸药、多孔粒状铵油炸药的相关参数取值详见表2。空气材料模型采用线性多项式状态方程,空气压力计算公式:
p=C0+C1μ+C2μ2+C3μ3+(C4+C5μ+C6μ2)e (17) 式中:C0=C1=C2=C3=C6=0,C4=C5=0.4,μ=ρ/ρ0,ρ、ρ0分别为初始材料密度和当前材料密度,e为比内能。
2.4 模拟结果分析
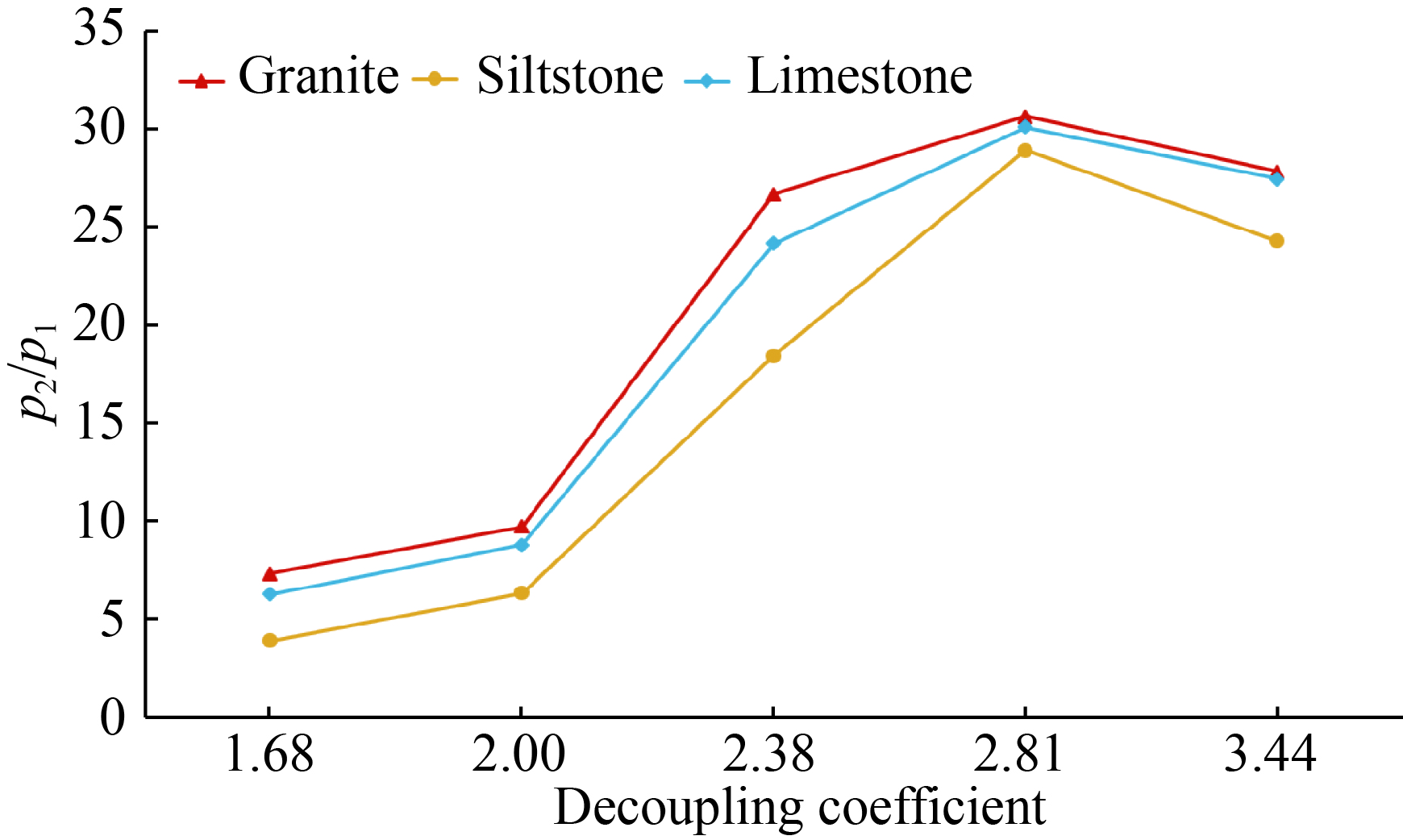
图4给出了部分典型工况下空气冲击波与孔壁作用的压力时程曲线。从图4可以看出压力时程曲线有一个明显的拐点,空气冲击波与孔壁作用后压力有一个显著的提高,拐点前最大压力为入射压力p1,拐点后最大压力为反射压力p2,孔壁处径向应力为透射压力p3即孔壁压力峰值。表3、表4分别给出模拟反射压力p2、透射压力p3与入射压力p1的比值,图5、图6分别给出了相对应的曲线。表5给出了径向不耦合装药时数值模拟与常用方法1、2理论计算得出的孔壁压力峰值;表6给出了径向与轴向均不耦合装药时,装药系数分别为0.6和0.3的炮孔壁单元的最大径向应力的平均值,以及装药系数为1.0时孔壁压力峰值,分析径向和轴向不耦合装药系数对孔壁压力峰值的影响。
表 3 乳化炸药作用下空气冲击波反射压力与入射压力比值Table 3. Ratio between reflected pressure and incident pressure of air blast wave induced by emulsion explosive装药条件 不耦合系数 p1/MPa p2/MPa p2/p1 粉砂岩 石灰岩 花岗岩 粉砂岩 石灰岩 花岗岩 25/42 1.68 96.9 313 518 610 3.2 5.3 6.3 25/50 2.00 52.3 290 398 437 5.5 7.6 8.4 32/76 2.38 21.3 334 444 488 15.7 20.8 22.9 32/90 2.81 13.5 330 354 355 24.4 26.2 26.3 32/110 3.44 12.9 269 304 308 20.9 23.6 23.9 表 4 乳化炸药作用下空气冲击波透射压力与入射压力比值Table 4. Ratio between transmission pressure and incident pressure of air blast wave induced by emulsion explosive装药条件 不耦合系数 p1/MPa P3/MPa p3/p1 粉砂岩 石灰岩 花岗岩 粉砂岩 石灰岩 花岗岩 25/42 1.68 96.9 619 761 858 6.4 7.9 8.9 25/50 2.00 52.3 534 677 695 10.2 12.9 13.3 32/76 2.38 21.3 465 528 502 21.8 24.8 23.6 32/90 2.81 13.5 400 428 402 29.6 31.7 29.8 32/110 3.44 12.9 324 304 310 25.1 23.6 24.0 表 5 模拟与理论计算孔壁压力峰值对比Table 5. Comparison of borehole peak pressure between simulation and theoretical calculation炸药种类 装药条件 孔壁压力峰值/MPa 数值模拟 理论计算 粉砂岩 石灰岩 花岗岩 平均值 方法1(n=8) 方法2 乳化炸药 25/42 619 761 858 746 925 792 25/50 534 677 695 635 802 602 32/76 465 528 502 498 513 439 32/90 400 428 402 410 331 319 32/110 324 304 310 313 196 232 多孔粒状铵油炸药 25/42 521 625 671 606 835 649 25/50 454 542 551 516 531 493 32/76 379 413 389 394 339 358 32/90 322 329 303 318 219 258 32/110 248 229 233 237 130 187 表 6 不同装药条件下的孔壁压力峰值Table 6. Borehole peak pressure under different charge conditions炸药种类 装药
条件孔壁峰值压力的
0.6 倍/MPa装药系数 0.6 时的
平均压力/MPa孔壁峰值压力的
0.3 倍/MPa装药系数 0.3 时的
平均压力/MPa花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 乳化炸药 25/42 515 457 371 527 472 386 257 228 186 286 263 225 25/50 417 406 320 410 411 334 208 203 160 222 223 219 32/76 301 317 279 287 300 277 151 158 140 171 178 165 32/90 241 257 240 234 244 232 121 128 120 136 142 136 32/110 186 182 194 175 178 180 93 91 97 100 102 104 多孔粒状
铵油炸药25/42 403 375 313 388 382 318 201 188 156 214 211 180 25/50 331 325 272 316 315 274 165 163 136 170 172 157 32/76 233 248 227 216 231 218 117 124 114 126 133 127 32/90 182 197 193 177 183 178 91 99 97 102 104 103 32/110 140 137 149 130 131 135 70 69 75 75 75 76 由表3~5、图5~6可知冲击波的反射、透射压力增大倍数均与不耦合系数、透射介质波阻抗有关,而不是一个固定不变的值,不耦合系数影响尤其明显,当不耦合系数到2.81以后,冲击波反射、透射压力增大倍数都会有一个下降的过程,这是因为当考虑炮孔壁的曲面特征及爆轰波的柱面特征时,不耦合系数大到一定程度后,冲击波在炮孔的透、反射的叠加效果开始减小。虽然不同透射介质空气冲击波透射压力与入射压力的比值不同,但是可以发现粉砂岩、石灰岩、花岗岩3种介质条件的透射压力与入射压力比值差别并不大,这与图2给出的结论一致。表6中给出的不同装药条件下的孔壁压力结果表明,轴向装药系数分别为0.6和0.3时的平均压力值与轴向耦合装药时压力峰值的0.6和0.3倍大致相等,说明轴向不耦合装药的孔壁压力平均值与轴向装药系数密切相关,近似成正比关系。
3. 轮廓爆破孔壁压力峰值的确定方法
由上述分析可知,不耦合系数、被爆介质波阻抗、炸药性能等因素是影响轮廓爆破孔壁压力峰值的关键参数,从表5中不同方法计算得到的结果对比也可看出,不同方法得到的结果差别较大;不耦合系数较大时,常用计算方法1和方法2的结果均明显偏小,而且已有主要方法也较少考虑轴向装药系数的影响。因此有必要寻找一种较准确又较简单方便的爆破孔壁峰值压力计算方法。本文中根据理论分析及数值模拟结果,基于3种数值模拟中爆破介质条件孔壁压力峰值的平均值,研究轮廓爆破孔壁压力峰值计算方法。
传统的轮廓爆破,径向不耦合系数一般都大于1.5,爆生气体的膨胀过程有等熵膨胀和绝热膨胀2个阶段,因此以爆生气体在炮孔中完成等熵和绝热膨胀时的压力
pb=(pw/pk)γ/kpk(dc/db)2γ 为入射压力,数值模拟的透射压力为孔壁压力峰值pr ,定义压力增大倍数n′=pr/pb ,得到不同不耦合系数时的压力增大倍数见表7,将表7的数值按炸药种类分类绘成压力增大倍数n´的曲线见图7。表 7 不同条件下的压力增大倍数Table 7. Pressure increase multiples under different conditions装药条件 不耦合系数 n' 乳化炸药 多孔粒状铵油炸药 25/42 1.68 4.7 5.8 25/50 2.00 6.3 7.8 32/76 2.38 7.8 9.3 32/90 2.81 9.9 11.6 32/110 3.44 13.2 14.6 同时考虑炮孔径向不耦合系数、轴向装药系数、炸药性能以及空气冲击波与炮孔壁作用后的压力增大系数等影响因素,基于pb拟合炮孔壁的爆炸压力峰值,提出采用式(18)计算轮廓爆破孔的孔壁压力峰值:
pr=(pw/pk)γ/kpk(dc/db)2γn′ls=pbn′ls (18) 式中:ls为轴向装药系数;其余参数含义见前述方法1。
4. 结 论
(1)轮廓爆破时,爆炸冲击波作用于炮孔壁后,炮孔壁处的压力会显著增大,压力增大倍数主要与炸药特性、不耦合系数、岩体介质相关,并不是一个常值。轴向不耦合装药的孔壁压力峰值与轴向装药系数近似成正比关系。
(2)
pr=(pw/pk)γ/kpk(dc/db)2γn′ls 形式的计算模型可较好地确定轮廓爆破孔壁压力峰值,压力增大倍数n′ 可根据炸药类别、不耦合装药系数取值。需要说明的是,本文分析过程中,理论模型有一定的简化,轮廓爆破孔壁压力峰值计算模型是基于理论推导和数值仿真结果,尚缺少试验成果验证,这方面的内容有待进一步研究。
-
表 1 岩石物理力学参数
Table 1. Physical and mechanical parameters of rock
岩石种类 密度/(kg·m−3) 泊松比 弹性模量/GPa 屈服应力/MPa 切线模量/GPa 粉砂岩 2 170 0.25 6.70 39.20 0.6 石灰岩 2 600 0.25 32.5 72.9 3.0 花岗岩 2 700 0.24 68.00 150.00 7.0 注:Cowper-Symonds 参数 C 取 2.5 s−1,Cowper-Symonds 参数 P 取 4.0。 表 2 炸药相关参数
Table 2. Parameters of explosive
表 3 乳化炸药作用下空气冲击波反射压力与入射压力比值
Table 3. Ratio between reflected pressure and incident pressure of air blast wave induced by emulsion explosive
装药条件 不耦合系数 p1/MPa p2/MPa p2/p1 粉砂岩 石灰岩 花岗岩 粉砂岩 石灰岩 花岗岩 25/42 1.68 96.9 313 518 610 3.2 5.3 6.3 25/50 2.00 52.3 290 398 437 5.5 7.6 8.4 32/76 2.38 21.3 334 444 488 15.7 20.8 22.9 32/90 2.81 13.5 330 354 355 24.4 26.2 26.3 32/110 3.44 12.9 269 304 308 20.9 23.6 23.9 表 4 乳化炸药作用下空气冲击波透射压力与入射压力比值
Table 4. Ratio between transmission pressure and incident pressure of air blast wave induced by emulsion explosive
装药条件 不耦合系数 p1/MPa P3/MPa p3/p1 粉砂岩 石灰岩 花岗岩 粉砂岩 石灰岩 花岗岩 25/42 1.68 96.9 619 761 858 6.4 7.9 8.9 25/50 2.00 52.3 534 677 695 10.2 12.9 13.3 32/76 2.38 21.3 465 528 502 21.8 24.8 23.6 32/90 2.81 13.5 400 428 402 29.6 31.7 29.8 32/110 3.44 12.9 324 304 310 25.1 23.6 24.0 表 5 模拟与理论计算孔壁压力峰值对比
Table 5. Comparison of borehole peak pressure between simulation and theoretical calculation
炸药种类 装药条件 孔壁压力峰值/MPa 数值模拟 理论计算 粉砂岩 石灰岩 花岗岩 平均值 方法1(n=8) 方法2 乳化炸药 25/42 619 761 858 746 925 792 25/50 534 677 695 635 802 602 32/76 465 528 502 498 513 439 32/90 400 428 402 410 331 319 32/110 324 304 310 313 196 232 多孔粒状铵油炸药 25/42 521 625 671 606 835 649 25/50 454 542 551 516 531 493 32/76 379 413 389 394 339 358 32/90 322 329 303 318 219 258 32/110 248 229 233 237 130 187 表 6 不同装药条件下的孔壁压力峰值
Table 6. Borehole peak pressure under different charge conditions
炸药种类 装药
条件孔壁峰值压力的
0.6 倍/MPa装药系数 0.6 时的
平均压力/MPa孔壁峰值压力的
0.3 倍/MPa装药系数 0.3 时的
平均压力/MPa花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 花岗岩 石灰岩 粉砂岩 乳化炸药 25/42 515 457 371 527 472 386 257 228 186 286 263 225 25/50 417 406 320 410 411 334 208 203 160 222 223 219 32/76 301 317 279 287 300 277 151 158 140 171 178 165 32/90 241 257 240 234 244 232 121 128 120 136 142 136 32/110 186 182 194 175 178 180 93 91 97 100 102 104 多孔粒状
铵油炸药25/42 403 375 313 388 382 318 201 188 156 214 211 180 25/50 331 325 272 316 315 274 165 163 136 170 172 157 32/76 233 248 227 216 231 218 117 124 114 126 133 127 32/90 182 197 193 177 183 178 91 99 97 102 104 103 32/110 140 137 149 130 131 135 70 69 75 75 75 76 表 7 不同条件下的压力增大倍数
Table 7. Pressure increase multiples under different conditions
装药条件 不耦合系数 n' 乳化炸药 多孔粒状铵油炸药 25/42 1.68 4.7 5.8 25/50 2.00 6.3 7.8 32/76 2.38 7.8 9.3 32/90 2.81 9.9 11.6 32/110 3.44 13.2 14.6 -
[1] 朱瑞赓, 王雪峰. 不耦合装药爆破孔壁压力计算: 一 [J]. 爆破, 1990, 7(3): 1–4. [2] 费鸿禄, 李守巨, 何庆志. 光面爆破装药不偶合系数的计算 [J]. 爆炸与冲击, 1992, 12(3): 270–274. DOI: 10.11883/1001-1455(1992)03-0270-05.FEI Honglu, LI Shouju, HE Qingzhi. Calculation of de-coupling coefficient of smooth blasting charge [J]. Explosion and Shock Waves, 1992, 12(3): 270–274. DOI: 10.11883/1001-1455(1992)03-0270-05. [3] 王志亮, 李永池. 工程爆破中径向水不耦合系数效应数值仿真 [J]. 岩土力学, 2005, 26(12): 1926–1930. DOI: 10.3969/j.issn.1000-7598.2005.12.012.WANG Zhiliang, LI Yongchi. Numerical simulation on effects of radial water-decopling coefficient in engineering blast [J]. Rock and Soil Mechanics, 2005, 26(12): 1926–1930. DOI: 10.3969/j.issn.1000-7598.2005.12.012. [4] 凌伟明. 岩石爆破炮孔孔壁压力的试验研究 [J]. 矿冶, 2004, 13(4): 13–16. DOI: 10.3969/j.issn.1005-7854.2004.04.004.LING Weiming. Experimental research on explosion pressure on the wall of a borehole in rock [J]. Mining and Metallurgy, 2004, 13(4): 13–16. DOI: 10.3969/j.issn.1005-7854.2004.04.004. [5] 王伟, 李小春, 石露, 等. 深层岩体松动爆破中不耦合装药效应的探讨 [J]. 岩土力学, 2008, 29(10): 2837–2842. DOI: 10.3969/j.issn.1000-7598.2008.10.046.WANG Wei, LI Xiaochun, SHI Lu, et al. Discussion on decoupled charge loosening blasting in deep rock mass [J]. Rock and Soil Mechanics, 2008, 29(10): 2837–2842. DOI: 10.3969/j.issn.1000-7598.2008.10.046. [6] 刘云川, 汪旭光, 刘连生, 等. 不耦合装药条件下炮孔初始压力计算的能量方法 [J]. 中国矿业, 2009, 18(6): 104–107. DOI: 10.3969/j.issn.1004-4051.2009.06.031.LIU Yunchuan, WANG Xuguang, LIU Liansheng, et al. An energy method for calculate borehole pressure under decoupled charging [J]. China Mining Magazine, 2009, 18(6): 104–107. DOI: 10.3969/j.issn.1004-4051.2009.06.031. [7] FELDGUN V R, KARINSKI Y S, YANKELEVSKY D Z. Experimental simulation of blast loading on structural elements using rarefaction waves-theoretical analysis [J]. International Journal of Impact Engineering, 2017, 102: 86–101. DOI: 10.1016/j.ijimpeng.2016.12.010. [8] SAHARAN M R, MITRI H S. Numerical procedure for dynamic simulation of discrete fractures due to blasting [J]. Rock Mechanics and Rock Engineering, 2008, 41(5): 641–670. DOI: 10.1007/s00603-007-0136-9. [9] OZGUR Yilmaz, TUGRUL Unlu. Three dimensional numerical rock damage analysis under blasting load [J]. Tunnelling and Underground Space Technology, 2013, 38: 266–278. DOI: 10.1016/j.tust.2013.07.007. [10] YI C, JOHANSSON D, GREBER J. Effects of in-situ stresses on the fracturing of rock by blasting [J]. Computers and Geotechnics, 2018, 104: 321–330. DOI: 10.1016/j.compgeo.2017.12.004. [11] HENRYCH J. The dynamics of explosion and its use [M]. New York: Elsevier Scientific Publishing Company, 1979. [12] 钮强. 岩石爆破机理 [M]. 沈阳: 东北工学院出版社, 1990: 18−19. [13] 王礼立. 应力波基础 [M]. 北京: 国防工业出版社, 2005: 45−47. [14] 李维新. 一维不定常流与冲击波 [M]. 北京: 国防工业出版社, 2003: 204−210. [15] 戴俊. 岩石动力学特性与爆破理论 [M]. 北京: 冶金工业出版社, 2013: 88−91. [16] Livermore Software Technology Corporation. LS-DYNA theoretical manual [M]. California: Livermore Software Technology Corporation, 2003: 1012−1013. [17] 水利水电科学研究院. 岩石力学参数手册 [M]. 北京: 水利电力出版社, 1991: 429−434. [18] 夏祥, 李海波, 李俊如, 等. 岭澳核电站二期工程基岩爆破安全阈值分析 [J]. 岩土力学, 2008, 29(11): 2945–2951. DOI: 10.3969/j.issn.1000-7598.2008.11.010.XIA Xiang, LI Haibo, LI Junru, et al. Research on vibration safety threshold for rock under blasting excavation [J]. Rock and Soil Mechanics, 2008, 29(11): 2945–2951. DOI: 10.3969/j.issn.1000-7598.2008.11.010. [19] 刘军. 岩体在冲击载荷作用下的各向异性损伤模型及其应用 [J]. 岩石力学与工程学报, 2004, 23(4): 635–640. DOI: 10.3321/j.issn:1000-6915.2004.04.020.LIU Jun. Anisotropic damage model and its application to rock materials under impact load [J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23(4): 635–640. DOI: 10.3321/j.issn:1000-6915.2004.04.020. 期刊类型引用(23)
1. 董源, 胡英国, 刘美山, 李庚泉, 马晨阳. 均质岩石高边坡开挖爆破累积损伤的演化机制研究. 岩土力学. 2025(09)  百度学术
百度学术2. 曾华恒, 解叶龙, 胡艳, 陈必港, 魏勇, 霍晓锋. 不耦合液氧膨胀破岩法孔壁压力计算方法研究. 爆破. 2025(02)  百度学术
百度学术3. 高瑞,赵鸿杰,徐冰琪,于斌,姜泽. “射流+爆轰”微差复合爆破定向造缝机制及其全过程研究. 岩石力学与工程学报. 2025(01): 81-98 .  百度学术
百度学术4. 楼晓明,林杰,王新龙,刘怀宇,王广. 球面爆轰波下不同孔径耦合装药孔壁压力沿炮孔轴向分布特征. 哈尔滨工业大学学报. 2025(03): 148-159 .  百度学术
百度学术5. 楼晓明,唐志恒,牛明远,林日宗,曾令峰. 深孔轴向多段间隔装药孔壁冲击压力分布. 矿冶工程. 2024(01): 6-11 .  百度学术
百度学术6. 李启月,魏快快,马晶晶,田军,魏新傲,徐恒阳,肖宇航. 炮孔壁初始冲击压力直接测量试验研究. 工程爆破. 2024(03): 1-7+19 .  百度学术
百度学术7. 张贺,何志伟,郭子如,谢守冬,李萍丰,胡前浩,刘伟,尹涛,汪泉,苏洪. 3种现场混装炸药耦合装药炮孔壁峰值压力计算. 工程爆破. 2024(04): 26-33 .  百度学术
百度学术8. 陈建华,郭文康,卜禄坤,林日宗,牛明远. 巷道光面爆破周边孔间距确定. 福建交通科技. 2023(04): 28-36 .  百度学术
百度学术9. 张想,李启月,曾海登,赵明生. 偏/同心不耦合非装药段孔壁压力分布规律研究. 工程爆破. 2023(04): 18-23+34 .  百度学术
百度学术10. 魏海霞,祝杰,杨小林,褚怀保. 高压气体爆破作用下层状岩体的地表振动效应预测方法. 振动与冲击. 2023(20): 1-11 .  百度学术
百度学术11. 江堃,邓守春,李海波. 甲烷燃爆压裂技术的实验研究. 煤炭学报. 2023(12): 4297-4307 .  百度学术
百度学术12. 李卓,雷兴海,李恒,张智宇,徐斌. 岩石爆破损伤中水不耦合系数变化效应研究. 有色金属(矿山部分). 2022(01): 15-21 .  百度学术
百度学术13. 李康贵,夏鹏,米元桃,陈明,李桐. 水耦合强抛掷台阶爆破技术研究. 爆破. 2022(02): 23-29 .  百度学术
百度学术14. 李桐,陈明,叶志伟,卢文波,魏东,郑祥. 混凝土含水裂隙中爆炸压力传播的模型试验研究. 岩土力学. 2022(S2): 205-213 .  百度学术
百度学术15. 刘玉丰,方芳,李海谦,李启月. 不耦合装药结构爆炸孔壁压力分布特性的数值模拟. 矿冶工程. 2022(05): 30-33 .  百度学术
百度学术16. 杨赛群,李洪伟,吴立辉,刘伟. 影响光面爆破效果和空孔处能量分布的试验研究. 工程爆破. 2022(06): 58-65 .  百度学术
百度学术17. 翟小鹏,杨军,陈占扬,汤宇,陈忠辉. 基于应变率变化的爆破块度预测模型及应用. 工程爆破. 2022(06): 73-79+118 .  百度学术
百度学术18. 魏东,陈明,叶志伟,卢文波,李桐. 基于应变率相关动力特性的岩体爆破破坏区范围研究. 工程科学与技术. 2021(01): 67-74 .  百度学术
百度学术19. 孙颖,李桐,陈明,叶志伟. 水介质耦合爆破中爆炸能量的传输特性研究. 水电与新能源. 2021(02): 35-40 .  百度学术
百度学术20. 叶志伟,陈明,魏东,卢文波,刘涛,吴亮. 不耦合装药爆破孔壁压力峰值的实验研究. 爆炸与冲击. 2021(05): 115-124 .  本站查看
本站查看21. 叶志伟,陈明,李桐,卢文波,严鹏. 小不耦合系数装药爆破孔壁压力峰值计算方法. 爆炸与冲击. 2021(06): 119-129 .  本站查看
本站查看22. 叶志伟,陈明,李桐,卢文波,严鹏. 一种水耦合轮廓爆破孔壁压力峰值的简化计算方法. 岩土力学. 2021(10): 2808-2818 .  百度学术
百度学术23. 张恒根,王卫华,王永强. 空气不耦合装药孔壁初始冲击压力的计算. 工程爆破. 2020(03): 8-15+22 .  百度学术
百度学术其他类型引用(18)
-


















 下载:
下载:




























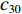



























 下载:
下载:






 百度学术
百度学术