Connecting modes of two cracks under impact loads
-
摘要: 脆性材料内部含有大量裂纹,当某一裂纹扩展时,其他裂纹会对扩展裂纹产生影响。为了研究冲击载荷下,脆性材料内两裂纹的相互影响、连通规律及裂纹尖端应力强度因子的变化规律,利用有机玻璃板制作了含非平行双裂纹的实验试件,利用落板冲击设备进行了中低速冲击实验,结合有限元分析软件ABAQUS计算出裂纹尖端应力强度因子,利用有限差分软件AUTODYN进行了动态数值模拟研究,并将其模拟结果与实验结果进行对比分析。实验及模拟结果表明:裂纹破坏形态与AUTODYN数值模拟破坏形态基本一致;试件的断裂形态随着两裂纹间距不同而不同;裂纹间的相互影响程度随着裂纹间间距增大而减小;裂纹尖端应力强度因子KI随着裂纹间距的增大而减小,而KII随着裂纹间距增大而增大。Abstract: A multitude of flaws always exists in brittle material and the influence will be exerted on by other flaws when one flaw is propagating. To investigate the propagation, coalition behavior and stress intensity factors of two cracks in brittle materials under impact loading, Polymethyl methacrylate (PMMA) was selected to manufacture the double cracked specimens. By using the medium-low speed impact system, impact experiments were conducted, and the crack tip SIFs were calculated by using finite element code ABAQUS. The finite difference code AUTODYN was used in the simulation crack propagation behavior, and the simulation results were compared with the test results. The results of experiment and simulation show that the simulation results generally agree with the experimental results in crack propagation paths; the crack propagation behavior varies with the change of the distance between the two cracks; the effect between the two cracks decreases with the increase of the distance between the two cracks; the stress intensity factors KI decrease with the increase of the distance between the two cracks while KII is the opposite.
-
脆性材料往往存在裂纹等缺陷,对于裂纹的起裂、扩展方向及多裂纹的搭接形态等一直是学者研究的重点。起裂韧度、裂纹扩展中极限应力强度因子、多裂纹间搭接形态等作为表征裂纹起裂扩展重要参数,对其进行深入研究是当前重要课题。对于该课题,无论是在理论,数值模拟或是实验方面,均有众多学者对其进行了大量的研究。
在理论上,20世纪初,Inglis[1]发现裂纹尖端应力集中效应,随后Irwin[2]提出应力强度因子K是表征裂纹尖端应力场唯一需要确定的参量。基于此,大量学者利用复变函数、椭圆积分等数学工具推导了大量规则排列的裂纹的应力强度因子。范天佑[3]采用复势法、Westergaard方法、叠加法、Green函数法等推导了无限大板含中心裂纹的应力强度因子,给后来学者研究裂纹尖端应力强度因子提供了可靠理论基础。朱哲明等[4]、Zhu等[5-8]基于复变函数理论推导了部分特定排列情况下裂尖应力强度因子的解析表达式。Itou[9]利用拉普拉斯变换得出冲击载荷下平形双裂纹的动态应力强度因子与裂纹间距和几何形态有关。但这些理论推导都只得出了规则排列裂纹尖端的应力强度因子,然而自然界裂纹分布往往具有随机性,这就对研究更多随机分布裂纹的破坏形态及其应力场提出了新的要求。并且理论推导主要研究静压作用下的应力强度因子,但是脆性材料在动载作用下的断裂特性研究甚少。
为了解决含复杂裂纹试件的加工困难问题及动载加载不易等问题,一些学者开始使用各种数值模拟软件进行含裂纹试件分析。钟波波等[10]和Louis等[11]分别利用RFPA-dynamic和AOTODYN软件研究了裂纹倾角、岩桥倾角在双向拉载荷下的裂纹扩展方式,认为裂纹的几何分布形式对裂纹的贯通有显著影响。采用软件进行数值模拟可以预先得到目前还没有办法实现实验的裂纹的排列形态及作用荷载形式,同时可以作为以后实验结果对照的一个依据。但是模拟通常比较理想化,与实际情况往往存在差异,因此,实验研究十分必要。在静载实验方面,对平形裂纹[12-14]、变角度非平形裂纹[15]、三裂纹[16]问题,裂纹间联通规律都已经研究较为成熟。而冲击动载实验方面,大多研究都基于含单裂纹的三点弯曲梁[17]或单裂纹[18-20, 24, 26-27]研究。对于非平形双裂纹研究,数据尚不够充足。但自然界中脆性材料原生裂纹存在方式并非仅限于共线、平形排列方式。而且,脆性材料也常在动载作用下工作,并且材料对动载与静载响应差异很大。所以,对于含多裂纹脆性材料在动载作用下的断裂形态,力学特性等的研究是亟待解决的问题。
基于上述原因,本文中将采用落板冲击实验装置,研究非平行双裂纹在冲击载荷下裂纹间搭接方式、裂纹起裂韧度及扩展过程。并采用实验-数值-解析法[19]计算裂纹尖端起裂韧度。落板冲击设备[20-21]工作原理类同霍普金森压杆设备类似,但是能够应用于大型试件的实验研究。实验前期和后期分别结合有限元分析软件AUTODYN、ABAQUS进行模拟,将结果与实验结果对照,确定出非平行双裂纹缺陷试件断裂形态及裂纹起裂韧度、裂纹扩展中极限强度因子,并分析裂纹断裂成因。
1. 实验研究
1.1 试件模型及准备
本次实验采用脆性材料有机玻璃板(polymethyl methacrylate, PMMA)进行实验,采用应变片和裂纹扩展计(crack propagate gauge, CPG)进行数据采集。
1.1.1 试件几何尺寸及材质介绍
因PMMA具有透光性好、均质等特性,所以研究脆性材料裂纹扩展形态具有较大优越性。实验中的有机玻璃采用工厂定制,预制裂纹均采用激光切割,以避免机械切割裂纹过宽对实验结果产生影响。有机玻璃的密度为1 180 kg/m3,弹性模量为6.1 GPa,体积模量为5.35 GPa,剪切模量为2.33 GPa,泊松比为0.31。本文中采用的实验构型如图1所示,本次试件的竖直裂纹和倾斜裂纹均为25 mm,D表示竖直裂纹尖端到倾斜裂纹中心的距离,本次实验共设7组不同D值实验详见表1(试件编号含义解释:如D100-A45表示裂纹间距为100 mm,倾斜裂纹倾角为45°),其他与此同);
θ 表示倾斜裂纹与水平轴的夹角,本文中为45°。本文采用数值模拟和落板冲击设备研究当θ 为45°,随着D值变化时双裂纹的扩展过程、连通规律及竖直裂纹、倾斜裂纹相互影响作用下两条裂纹尖端应力强度因子的变化规律。表 1 试件样本和实验时落板高度及冲击速度Table 1. Height of drop weight plate and impact velocity for each specimen试件编号 D/mm H/mm v/(m·s−1) D100−A45 100 1.840 6.005 D75−A45 75 1.865 6.046 D50−A45 50 1.827 5.984 D25−A45 25 1.834 6.000 D20−A45 20 1.854 6.028 D15−A45 15 1.847 6.017 D10−A45 10 1.828 5.986 1.1.2 CPG的使用及介绍
采用贴型号为BKX3.5-10CY的裂纹扩展计(CPG)测试裂纹扩展速度及裂纹扩展到某处的断裂时刻。CPG示意图如图2,由玻璃丝布基底和敏感栅丝两部分组成,栅丝为宽度不同(电阻不同)的卡玛铜薄片组成。一片CPG中共含有21根栅丝,宽度b为10 mm,栅丝间间距l0为2.2 mm,裂纹扩展计的有效总长度L0为44 mm,初始电阻为3.5 Ω。实验前将CPG第一根栅丝与竖直裂纹尖端垂直贴合。实验中,将CPG与50 Ω电阻并联,再于另一个50 Ω电阻串联,电路中由16 V稳压源提供电压,这种链接方式既可以保证电压为恒压,也可以保证CPG两端电压变化太大导致测试不准。
1.2 落板冲击实验设备
本次实验采用了落板冲击实验设备,实验设备见图3。实验设备主要部件有落板、入射板、透射板、阻尼器。材料参数如表2所示。实验时,在距离入射板底端500、750和1 000 mm处分别竖向粘贴应变片,在距离透射板上端500 mm距离粘贴应变片,以便测量入射波、反射波和透射波。由于落板冲击较大入射杆接受的冲击波容易产生弥散效应和高频振荡,因此在入射杆上端放置黄铜整形器,以延长入射波的上升沿。因试件上下端和实验设备部件均非光滑的,因此在加载过程中,试件上下断面容易与入射杆底部,透射杆顶部接触处产生环箍效应,因此实验前在试件上下端面涂抹黄油做润滑。透射杆底端放置阻尼器消能。
表 2 落板冲击设备部件参数Table 2. Parameters of impacting test system部件名称 高度/mm 宽度/mm 厚度/mm 弹性模量/GPa 泊松比 落板 150 480 30 − − 入射板 3 000 300 30 72 0.33 透射板 2 000 300 30 72 0.33 实验中主要测试仪器为示波器(型号:DS1004Z),超动应变仪信号(型号:CS-1D)。应变片(SG,实验中采用的型号有BX120-5AA和BX120-3AA两种)主要用于测得竖直裂纹起裂时刻,实验前将应变片上的栅丝与竖直裂纹尖端垂直贴合,如图3所示。当竖直裂纹起裂时,应变片同时受拉断裂,电压信号发生突变,该时刻即为裂纹起裂时刻,该起裂时刻将用于第4节计算应力强度因子后确定竖直裂纹的起裂韧度。
实验测量电路为桥式电路,本文采用单臂测量,即电桥中只有一个桥臂参与机械变形。电桥的输出电压和应变满足:
ΔU=nEKSε4 (1) 式中:
ΔU 为电桥输出电压,即应变片测的电压信号;n为超动态应变仪的增益,实验时设定为1 000;E为供桥电压,值为2 V;KS为电阻灵敏度系数,为2.1;ε 为输入应变量10−6。实验中测得电压信号后需通过式(1)转换成应变ε 。1.3 实验结果及处理
将试件放入实验设备后进行实验设备调试,并用红外测距仪测试落板底面距离入射板顶面高度H,并做记录。利用动能定理即可计算得落板冲击速度v,本次实验各对照组落板高度H及速度v已统计于表1。实验中各组冲击速度均约为6 m/s,该值也是数值模拟AUTODYN中的加载条件。
1.3.1 加载曲线及其处理
每组实验完成后,入射板及透射板上的应变片即可测得冲击作用下入射波、反射波及透射波电压信号。由于实验室不可避免的存在噪音等影响,在取得波形之后先利用Origin软件进行降噪、飘零处理及寻找波头[22]。处理后的入射波和透射波电压信号见图4。实曲线为入射板上测的入射波和反射波信号,虚线为透射板上测的透射波信号。通过式(1)即可将图4电压信号波形转化为入射板、透射板应变信号波形。根据一维弹性应力波假设[23],入射板对试件的载荷因为入射波和反射波叠加,透射板对试件载荷可直接由透射波得到。计算式子如下:
pi=EiA(εi+εr)pt=EtAεt (2) 式中:pi为入射板对试件载荷;pt为透射板对试件载荷;Ei为入射板弹性模量;Et为入射板弹性模量;A为入射板和透射板横截面积。叠加后的载荷曲线如图5所示(受压为正),该曲线将用于第4节ABAQUS模拟计算应力强度因子荷载条件。
1.3.2 预制竖直裂纹起裂时刻确定方法
实验中,试件由于冲击波作用下,产生垂直于裂纹面的拉应力,当该拉应力大于材料的抗拉强度时,裂纹开始起裂。粘贴于竖直裂纹尖端应变片也同时被拉断,测试电路由于应变片断裂,产生电路断路,电压信号产生突变,如图6实线所示。为获得精确的起裂时刻,对该曲线求导,即图6虚线,导数峰值所对应的时间tf即为竖直裂纹起裂时刻。图中为试件D15-A45的起裂时刻,其他试件的起裂时刻确定方式与此相同,已和计算的起裂韧度一同列于表3中。
表 3 各对照组起裂时刻及起裂韧度Table 3. Initiation time and initiation toughness for each specimen试件编号 起裂时刻tf /μs 起裂韧度 D10−A45 272 4.99 D15−A45 267 4.85 D20−A45 275 4.53 D25−A45 259 4.36 D20−A45 264 4.24 D15−A45 260 4.19 D10−A45 263 4.22 2. 数值模拟
2.1 数值模拟软件简介及关键参数设定
本文采用数值模拟软件AUTODYN进行试件在冲击作用下裂纹破坏形态的模拟。AUTODYN是一款基于有限差分法进行数值模拟的软件,该软件致力于用集成的方法解决结构和流体的非线性性。本文裂纹扩展规律的研究中,试件破坏属于塑性变形,也为非线性问题,因此采用AUTODYN进行数值拟具有合理性。该软件的模拟结果的稳定性也被国内外众多学者验证,如Zhu等[24-25]、朱哲明等[26]利用AUTODYN软件模拟脆性材料动态荷载作用下裂纹的断裂行为,对后来研究爆炸作用提供了参考;周磊等[27]利用AUTODYN进行数值模拟研究了巷道围岩裂纹在冲击荷载下的起裂、扩展及止裂力学行为;实验结果证明AUTODYN可以稳定的模拟材料在动载荷作用下的响应。
本文中,试件在CAD中按照试件实际尺寸建立三维模型,再导入ICEM中进行网格划分,网格类型采用四面体网格(Tetra/Mixed)类中的Tetrahedron。导出所画的体网格成K文件。在AUTODYN中按照1∶1的比例建立设备部件,即落板、入射板、透射板、阻尼器的计算模型,将从ICEM中导出的试件K文件导入AUTODYN中进行计算。材料均按照实际实验中的材料参数定义。初始条件为y方向速度,模拟时设为6 m/s,与实验室落板冲速度(见表1)近似。求解器采用Lagrange算法,为避免网格大变形扭曲采用侵蚀模型。失效准则采用修正后的最大主应力准则。
对于状态方程,可表示为如下形式:
p=F(ρ,T) (3) 式中:p为静水压力,F为状态方程相关的函数,其变量可以是密度、温度等物理量。本文中,由于没有产生大变形,所以采用线性状态方程即可获得较好的结果
p=K(ρρ0−1) (4) 式中:K为体积模量,
ρ 为当前材料密度,ρ0 为参考密度。2.2 模拟结果与实验对比
图7为试件在冲击荷载下裂纹的断裂形态及模拟断裂形态(紫红色为裂纹扩展路径,绿色为试件模型)。由于实验中,不可避免地存在误差,并且数值模拟并不能完全模拟实验中的真实情况,所以实验结果与模拟存在细微差异。但从图7可知,实验与模拟结果大致保持相同。不同 D值时,竖直裂纹尖端扩展到倾斜裂纹搭接形态大致分为3种:(1)D值为10~25 mm时,竖直裂纹直接扩展到倾斜裂纹中部但在靠近倾斜裂纹时,扩展路径微向倾斜裂纹偏转;(2)D为50 mm时,竖直裂纹到倾斜裂纹搭接呈不稳定型,部分直接从竖直裂纹扩展至倾斜裂纹中部,部分直接从竖直裂纹扩展至倾斜裂纹上尖端,模拟时裂纹产生分叉;(3)D值为75~100 mm时,扩展路径从竖直裂纹到倾斜裂纹上尖端。当D值在10、15、20和25 mm时,竖直裂纹起裂后将稳定的延伸至倾斜裂纹中部,然后再从倾斜裂纹下尖端继续扩展试件下端面(限于篇幅,图7中仅取D值为10和25 mm展示)。说明此时竖直裂纹到倾斜裂纹的扩展过程中裂纹始终为纯I型断裂。此断裂形态与刘凯等[20, 28]研究的I型裂纹断裂形态相一致。从图8的裂纹扩展与应力云图叠加图可知,竖直裂纹扩展到倾斜裂纹过程中,竖直裂纹尖端一直存在应力集中区域。倾斜裂纹上尖端虽有应力集中但并未引起倾斜裂纹上尖端开裂,说明在裂纹扩展过程中,竖直裂纹到倾斜裂纹中部这一路径所需断裂能量小于竖直裂纹到倾斜裂纹上尖端这一路径。随后倾斜裂纹下尖端由于应力集中而起裂,其形态表现为受压状态I/II复合型裂纹断裂形态[29]。
当D = 50 mm时,裂纹断裂形态处于不稳定情况,从图7及图8的模拟中可以看到,竖直裂纹与倾斜裂纹的中间部位,扩展裂纹产生了分叉,其中一条有向倾斜裂纹中部扩展趋势,另一条扩展向倾斜裂纹上尖端。从图8的应力云图亦可看出,竖直裂纹尖端在扩展过程中出现了应力集中区分叉现象,其中右边的应力集中区与倾斜裂纹上尖端应力集中区汇合,左边应力集中区一直延伸至倾斜裂纹中部。同时,实验中也出现了断裂形态不稳定,为排除实验时与实验目的不相关因素影响,故对该对照组增加了试件样本,经过多次重复实验发现,裂纹扩展路径表现为,一部分直接从竖直裂纹尖端扩展至倾斜裂纹中部,另一部分直接从竖直裂纹尖端扩展至倾斜裂纹上尖端。为了清楚对照,图8中D = 50 mm组试件展示了两种不同搭接形态实验结果(即图8(e)、(g))。可以认为D = 50 mm是裂纹搭接形态发生变化的一个转折点。
随着D值继续增加,当D值为75 mm和100 mm时,裂纹扩展又趋于稳定,断裂形态为从竖直裂纹扩展至倾斜裂纹上尖端,再从倾斜裂纹下尖端扩展至试件下端面中部。图8的应力云图也反映了竖直裂纹扩展路径尖端的应力集中区与倾斜裂纹尖端的应力集中区汇合,裂纹的扩展路径也偏向倾斜裂纹尖端。说明此时倾斜裂纹开始表现为受压状态下I/II复合型断裂形态。
根据实验与模拟结果,我们猜测,材料中存在裂纹的地方,通常比不存在裂纹的地方更薄弱,当竖直裂纹与倾斜裂纹相距较近时,裂纹间区域由于两裂纹同时存在,故该区域均比其他部位更薄弱,故在冲击作用下,竖直裂纹直接扩展至倾斜裂纹中部。随着D值增大,两裂纹间的相互作用减弱,从实验和模拟可知,当两裂纹相距50 mm时,裂纹断裂形态出现不稳定,说明此时两者的相互影响已经很弱。当D为75、100 mm时,两裂纹间已无相互影响,可分别视为单裂纹处理。
2.3 模拟及实验结果分析讨论
脆性断裂第一强度理论认为,材料破坏是因为最大拉应力达到临界值。实验及模拟结果显示,裂纹扩展形态主要为I型裂纹,因此是有机玻璃承受的拉应力达到临界值导致裂纹扩展。为了更加清楚解释裂纹分叉、拐弯,分别取D = 25 mm扩展到靠近倾斜裂纹处、D = 50 mm扩展路径的分叉点处、D = 75 mm裂纹拐弯点处为研究对象,计算距离裂纹尖端r = 2 mm处半圆弧上周向拉应力,如图9所示。
从图9中可以看出,当D = 25,75 mm时,其周向拉应力最大值仅在一处位置存在。D = 25 mm时最大值出现在与y轴夹角为8°~9°范围内,即图9(a)中A点处。从图7~图8的模拟和实验结果也可以看到,竖直裂纹扩展到靠近倾斜裂纹时略微偏向倾斜裂纹。D = 75 mm时最大值出现在与y轴夹角为25°~26°范围内,即图9(c)中D点处。但当D = 50 mm时,从图8(c)中可以看到,半圆弧上有两处出现最大拉应力值,即B、C两点处。B点在y轴上,也即裂纹径向方向上,C点在与y轴呈约27°方向上,该角度与D = 75 mm时最大周向拉应力出现的位置十分贴近。并且,结合实验及模拟结果可知,裂纹出现最大周向拉应力的地方正是裂纹断裂的方向。这说明竖直裂纹扩展到倾斜裂纹过程为受拉断裂,并且裂纹沿着周向拉应力最大处扩展,其断裂机理与脆性断裂强度理论吻合。
3. 动态应力强度因子计算
本文中应力强度因子采用有限元分析软件ABAQUS进行计算。该软件已经被多位学者用于岩土工程进行数值-实验模拟。周磊等[30]研究巷道裂纹动态扩展规律时,验证该软件在模拟动载作用下裂纹应力强度因子时程曲线的有效性,并与经典的Chen[31]问题进行了结果比对,验证得两者的结果十分接近,以及ABAQUS在处理复杂固体力学的非线性问题有着独到的优越性。
3.1 ABAQUS建模及参数设定
在ABAQUS中按照试件的设计尺寸建立模型,特性参数采用PMMA板参数。由于冲击加载的加载率较高,因此分析步采用动力隐式(dynamic implicit),该分析步能够较好地分析试件在冲击荷载下的瞬时力学特性。将实验中测的入射波、透射波,即图5中的应力时程曲线,分别导入到试件模型的上下端。根据岩石断裂力学[2]知,应力在裂纹尖端具有奇异性,为提高计算的精度,在划分网格时对裂纹尖端应力集中区域进行细化,其网格采用6节点三角形网格(CPS6),其余部位采用8节点四边形网格(CPS8),如图10所示。
3.2 计算结果
如图11所示,绘出了不同D值(即竖直裂纹尖端至倾斜裂纹中心间距)竖直裂纹尖端应力强度因子时程曲线。试样D15-A45的竖直裂纹尖端应力强度因子时程曲线及起裂韧度标识于图中,由2.3节所确定的起裂时刻tf可知,试件D15-A45起裂韧度
KCI = 4.85 MPa·m1/2。其余组试件起裂韧度列于表3。从表3可知,倾斜裂纹对于竖直裂纹尖端起裂时刻影响不大,但是起裂韧度KCI 整体呈渐降的趋势。成因分析,由3.2节可知,两裂纹间距较小时,两裂纹间相互影响较大,故竖直裂纹更容易扩展,所以其竖直裂纹尖端应力强度因子时程曲线值更大。随着裂纹间距增大,裂纹间相互影响逐渐减小,并且影响程度也逐渐减小。从图11也可以看出,随着D值增大,应力强度因子时程曲线呈下降趋势,并且这种下降趋势逐渐减弱,当D为50、75和100 mm时,裂纹的应力强度因子时程曲线重叠在一起,说明此时竖直裂纹、倾斜裂纹间已经近乎无相互影响。该现象与陈景杰等[32]对平形双裂纹的研究结论有共通之处,陈景杰等认为裂纹间距大于小裂纹一倍长度时,两裂纹间的影响可忽略不计,可视为单裂纹处理。但在本文的裂纹分布形态下,当D值(即竖直裂纹尖端到倾斜裂纹中心距离)为两倍裂纹长度值时,才是裂纹扩展搭接形态发生变化的阈值。
图12为倾斜裂纹上下尖端的KI、KII。倾斜裂纹的上下尖端KI均随着D值(即竖直裂纹尖端至倾斜裂纹中心间距)的增大而减小,KII均随D值增到而增大,并且减小(增大)比率逐渐降低,该现象也说明随着D值增大,竖直裂纹和倾斜裂纹间相互影响逐渐减弱。当D为50、75、100 mm时,此3组应力强度因子随时间变化曲线总是近乎重合,这也再次验证当D大于50 mm后,竖直裂纹和倾斜裂纹可视为单裂纹处理。
当D值较小,如10、15 mm时,竖直裂纹、倾斜裂纹间相互影响明显,并且倾斜裂纹上尖端受竖直裂纹影响程度大于倾斜裂纹下尖端,所以倾斜裂纹的上尖端的KI值明显大于倾斜裂纹下尖端KI值。并且这种影响比倾斜裂纹下尖端更持久,所以上尖端KI值在D为10和15 mm时几乎叠合,而下尖端的KI下降率却很大。对于倾斜裂纹上下尖端的KII,其上尖端KII上升的比率明显大于下尖端的KII上升率。上尖端KII在D值较小时,几乎为零,该现象与倾斜裂纹上尖端在D值较小时未发生断裂的情况相符;而下尖端KII峰值明显大于上尖端KII峰值,这表明倾斜裂纹上下尖端断裂情况不同,应力强度因子值也不同,这也应证了应力强度因子是反应裂纹尖端应力场性质的唯一参量。
随着D值增大,结合图12(a)、(b)或(c)、(d)可以看出倾斜裂纹同一尖端的KI和KII值越来越接近。表明随着D值的增大,倾斜裂纹越来越表现为I/II复合型断裂形态,这也与图7中表现出的断裂形态相吻合。
4. 裂纹扩展过程中的应力强度因子变化规律
由第3节结论可知,当D = 50~100 mm时,裂纹间相互影响已经不明显,研究裂纹扩展意义不大。而裂纹扩展计测试需要裂纹可以扩展一定距离才能采集到足够多的数据,因此我们选取D = 25 mm试件作为研究对象,研究竖直裂纹扩展到倾斜裂纹的裂纹扩展速度及极限应力轻度因子。1.1.2节我们知道CPG由21根栅丝并联组成,当裂纹扩展时,栅丝随材料的破坏而被逐根拉断,测试电路因电阻发生变化导致采集到的电压信号产生台阶型变化,如图13所示。为精准获得各栅丝断裂处时刻,我们对测得电压信号求导,导数极值处即为断裂时刻,根据栅丝断裂时刻,并结合ABAQUS计算的应力强度因子即可求得裂纹在扩展过程中的极限应力强度因子。
4.1 动态应力强度因子修正
第3节中采用ABAQUS计算裂纹尖端的应力强度因子,直接根据应变片断裂时刻确定竖直裂纹的断裂韧度。但当裂纹以一定速度扩展过程中,Freund[33]认为其应力强度因子应等于适用于该处静止裂纹应力强度因子乘以瞬时裂纹速度的普实函数k(v),即:
KdI(t)=KdI[t,a(t),v(t)]=k(v)K(0)I(t,a) (5) 式中:
KdI (t)为裂纹以速度v(t)扩展、时刻t对应的动态应力强度因子;a(t)为t时刻对应的裂纹长度;v(t)为t时刻裂纹的扩展速度,从上往下数CPG第i根栅丝计算公式为vi=2l0/(ti+1+ti−1) ;k(v)为与裂纹扩展速度v有关的普实函数;K(0)I (t, a)为时刻t裂纹具有相同尺寸a(t)的不扩展裂纹,在同样的荷载情况下,其只与裂纹长度、试件构型有关。对于普实函数k(v)其近似计算公式为:k(v)≈1−v/cR√1−v/cd (6) 式中:cR为材料Rayleigh波速,本文中有机玻璃cR=1 260 m/s;cd为纵波波速,本文中有机玻璃
cd=2320m/s 。当裂纹扩展速度为0时,k(v)为1,即不需要修正。本文中对于竖直裂纹起裂时刻(CPG第一根栅丝断裂时刻)的应力强度因子时程曲线即不用修正。要计算其余每根栅丝处动态应力强度因子时程曲线,需先计算该处静态裂纹的动态应力强度因子。如图14所示,以第4根栅丝为例,此时裂纹长度a(t) = 31.6 mm。根据图13确定t = 314 μs,对应修正后的应力强度因子时程曲线上的极限应力强度因子为KdI =3.44 MP·m1/2。4.2 计算结果及分析
图15为竖直裂纹到倾斜裂纹之间断裂韧度和裂纹扩展速度。从图15中不难看出,裂纹扩展过程中极限应力强度因子逐渐减小且均小于起裂韧度,说明需要裂纹起裂,其所需要的应力水平大于裂纹扩展过程中所需要的应力水平。裂纹扩展速度不是一个常数。因为竖直裂纹的扩展主要为I型断裂,但实验和模拟中均显示裂纹路径有弯曲,这说明裂纹在扩展过程中一直寻找耗能少及该扩展裂纹尖端周向拉应力最大的路径,所以其扩展速度随着断裂位置不同,裂纹速度也不相同。
竖直裂纹扩展到离倾斜裂纹比较近处,扩展速度减慢,在竖直裂纹扩展到临近倾斜裂纹上表面时,扩展速度减慢甚至几乎停滞,扩展极限应力强度因子增大。当a(t) = 47.0 mm时,v = 13 m/s,其值为裂纹平均扩展速度155 m/s的1/10不到。根据汪小梦等[21]关于V型边界侧开单裂纹半孔板(V-shape boundary single cleavage semi-circle specimen, VB-SCSC)岩石试样的实验研究,冲击波触及倾斜自由面时,原本压缩的荷载反射后会形成拉伸荷载,当该拉伸波在裂纹尖端与原本的压缩荷载叠加时造成集中应力减小,从而使裂纹扩展后期速度急剧减小,如图14所示。并且,在图7~8的实验及模拟结果中也可以看到,竖直裂纹扩展到临近倾斜裂纹时,裂纹扩展路径发生转向,在发生转向时也会对竖直裂纹扩展速度造成一定影响。但尚不清楚倾斜裂纹的存在是否会使竖直裂纹的扩展形成止裂,如果是止裂,倾斜裂纹的长度,倾角对竖直裂纹的扩展如何影响,这些将是下一步工作的研究重点。
5. 结 论
本文中采用落板冲击实验设备,研究了含双裂纹试样在动态荷载下的裂纹扩展、连通规律及裂纹尖端应力强度因子的变化规律,并将实验结果和模拟结果进行对照,将实验所得的数据代入ABAQUS模拟软件进行数值分析,得到试样竖直裂纹尖端的应力强度因子时程曲线。通过分析裂纹形态和模拟结果,得出了以下结论:
(1)在冲击载荷下,实验裂纹破坏形态与AUTODYN数值模拟破坏形态一致。当D (即竖直裂纹尖端到倾斜裂纹中心的距离)值较小时,裂纹沿原竖直裂纹方向扩展至倾斜裂纹中部,再由倾斜裂纹下尖端继续扩展至试件下端面中部。当D值约为50 mm时,裂纹扩展形态出现不稳定。部分裂纹断裂形态为从竖直裂纹尖端断裂向倾斜裂纹尖端,部分从竖直裂纹尖端断裂向倾斜裂纹中部。当D值较大时,在本文中为75和100 mm时,裂纹沿原竖直裂纹方向扩展至距离倾斜裂纹一定距离时,裂纹路径发生转向,转至倾斜裂纹上尖端,然后再从倾斜裂纹下尖端起裂至试样下端面中部。
(2)两裂纹间的相互影响随着裂纹间距增大而减小,当裂纹间距D值大于50 mm,两裂纹间的影响可忽略不计,即这两条裂纹可视为单裂纹分析。
(3)竖直裂纹尖端的应力强度因子时程曲线随着D值增大逐渐下降,下降率随着裂纹间距的增大而减小。倾斜裂纹尖端KI随D值增大而减小,下降率随着裂纹间距的增大而减小,KII随D值增大而增大,增大率随着裂纹间距的增大而减小。
(4)倾斜裂纹的存在会影响竖直裂纹的扩展速度及扩展中裂纹极限应力强度因子值。
-
表 1 试件样本和实验时落板高度及冲击速度
Table 1. Height of drop weight plate and impact velocity for each specimen
试件编号 D/mm H/mm v/(m·s−1) D100−A45 100 1.840 6.005 D75−A45 75 1.865 6.046 D50−A45 50 1.827 5.984 D25−A45 25 1.834 6.000 D20−A45 20 1.854 6.028 D15−A45 15 1.847 6.017 D10−A45 10 1.828 5.986 表 2 落板冲击设备部件参数
Table 2. Parameters of impacting test system
部件名称 高度/mm 宽度/mm 厚度/mm 弹性模量/GPa 泊松比 落板 150 480 30 − − 入射板 3 000 300 30 72 0.33 透射板 2 000 300 30 72 0.33 表 3 各对照组起裂时刻及起裂韧度
Table 3. Initiation time and initiation toughness for each specimen
试件编号 起裂时刻tf /μs 起裂韧度 D10−A45 272 4.99 D15−A45 267 4.85 D20−A45 275 4.53 D25−A45 259 4.36 D20−A45 264 4.24 D15−A45 260 4.19 D10−A45 263 4.22 -
[1] 劳恩. 脆性固体断裂力学 [M].2版.龚江洪, 译. 北京: 高等教育出版社; 2010: 2−5. [2] 李世愚, 和泰名, 尹祥础. 岩石断裂力学[M]. 北京: 科学出版社, 2015. 42−48. [3] 范天佑. 断裂理论基础[M]. 北京: 科学出版社; 2003. [4] 朱哲明, 汪元, 周章涛. 脆性材料在压缩载荷作用下的断裂破坏准则 [J]. 四川大学学报(工程科学版), 2008, 40(5): 13–21. DOI: 10.15961/j.jsuese.2008.05.003.ZHU Zheming, WANG Yuan, ZHOU Zhangtao, et al. A new fracture criterion for brittle materials under compression [J]. Journal of Sichuan University (Engineering Science Edition), 2008, 40(5): 13–21. DOI: 10.15961/j.jsuese.2008.05.003. [5] ZHU Zheming. An alternative form of propagation criterion for two collinear cracks under compression [J]. Mathematics and Mechanics of Solids, 2009, 14(8): 727–746. DOI: 10.1177/1081286508090043. [6] ZHU Zheming, XIE Heping, JI Shaocheng. The mixed boundary problems for a mixed mode crack in a finite plate [J]. Engineering Fracture Mechanics, 1997, 56(5): 647–655. DOI: 10.1016/S0013-7944(96)00123-3. [7] ZHU Zheming, WANG Laigui, MOHANTY B, et al. Stress intensity factor for a cracked specimen under compression [J]. Engineering Fracture Mechanics, 2006, 73(4): 482–489. DOI: 10.1016/j.engfracmech.2005.08.008. [8] ZHENG Tao, ZHU Zheming, WANG Bo, et al. Stress intensity factor for an infinite plane containing three collinear cracks under compression [J]. Journal of Applied Mathematics and Mechanics, 2015, 94(10): 853–861. DOI: 10.1002/zamm.201300001. [9] ITOU S. 3D dynamic stress intensity factors at three rectangular cracks in an infinite elastic medium subjected to a time-harmonic stress wave [J]. Archive of Applied Mechanics, 1999, 69(4): 286–298. DOI: 10.1007/s004190050220. [10] 钟波波, 张永彬, 李宏. 基于RFPA2D的岩石裂纹扩展模式的研究 [J]. 武汉理工大学学报, 2014, 36(2): 82–88. DOI: 10.3963/j.issn.1671-4431.2014.02.016.ZHONG Bobo, ZHANG Yongbin, LI Hong. Study of mechanisms of crack propagation of rock based on RFPA [J]. Journal of Wuhan University of Technology, 2014, 36(2): 82–88. DOI: 10.3963/j.issn.1671-4431.2014.02.016. [11] LOUIS N, LI Huaqiang. Numerical study on coalescence of two pre-existing coplanar flaws in rock [J]. International Journal of Solids and Structures, 2013, 50(22/23): 3685–3706. DOI: 10.1016/j.ijsolstr.2013.07.010. [12] YANG Shengqi, HUANG Yanhua. Erratum to: An experimental investigation on strength, deformation and crack evolution behavior of sandstone containing two oval flaws under uniaxial compression [J]. Engineering Geology, 2017, 217. DOI: 10.1016/j.enggeo.2016.12.004. [13] NASRI K, ABBADI M, ZENASNI M, et al. Double crack growth analysis in the presence of a bi-material interface using XFEM and FEM modelling [J]. Engineering Fracture Mechanics, 2014, 132: 189–199. DOI: 10.1016/j.engfracmech.2014.07.016. [14] HAERI H, SARFARAZI V, MARJI M F, et al. Experimental and numerical study of shear fracture in brittle materials with interference of initial double cracks [J]. Acta Mechanica Solida Sinica, 2016, 29(5): 555–566. DOI: 10.1016/S0894-9166(16)30273-7. [15] ZHANG Xiaoping, LIU Quansheng, WU Shunchuan, et al. Crack coalescence between two non-parallel flaws in rock-like material under uniaxial compression [J]. Engineering Geology, 2015, 199: 74–90. DOI: 10.1016/j.enggeo.2015.10.007. [16] CAO Ping, LIU Taoying, PU Chengzhi, et al. Crack propagation and coalescence of brittle rock-like specimens with pre-existing cracks in compression [J]. Engineering Geology, 2015, 187: 113–121. DOI: 10.1016/j.enggeo.2014.12.010. [17] 岳中文, 宋耀, 邱鹏, 等. 冲击载荷下双预置裂纹三点弯曲梁动态断裂实验 [J]. 振动与冲击, 2017, 36(4): 151–156. DOI: 10.13465/j.cnki.jvs.2017.04.024.YUE Zhongwen, SONG Yao, QIU Peng, et al. A dynamic fracture experiment of a three-point-bend beam containing double pre-existing cracks under impact load [J]. Journal of Vibration and Shock, 2017, 36(4): 151–156. DOI: 10.13465/j.cnki.jvs.2017.04.024. [18] 张华, 郭继鑫, 傅玉珍, 等. 冲击作用下混凝土裂纹扩展试验研究及数值模拟 [J]. 振动与冲击, 2016, 35(17): 107–112. DOI: 10.13465/j.cnki.jvs.2016.17.018.ZHANG Hua, GUO Jixin, FU Yuzhen, et al. Test and simulation for concrete crack propagation under impact load [J]. Journal of Vibration and Shock, 2016, 35(17): 107–112. DOI: 10.13465/j.cnki.jvs.2016.17.018. [19] 杨井瑞, 张财贵, 周妍, 等. 用SCDC试样测试岩石动态断裂韧度的新方法 [J]. 岩石力学与工程学报, 2015, 34(2): 279–292. DOI: 10.13722/j.cnki.jrme.2015.02.007.YANG Jingrui, ZHANG Caigui, ZHOU Yan, et al. A new method for determining dynamic fracture toughness of rock using SCDC specimens [J]. Chinese Journal of Rock Mechanics and Engineering, 2015, 34(2): 279–292. DOI: 10.13722/j.cnki.jrme.2015.02.007. [20] 刘凯, 朱哲明, 何廉. 脆性材料裂纹动态扩展规律研究 [J]. 四川大学学报(工程科学版), 2015, 47(S2): 81–88. DOI: 10.15961/j.jsuese.2015.s2.013.LIU Kai, ZHU Zheming, HE Lian. The study of crack dynamic propagation law of brittle materials [J]. Journal of Sichuan University (Engineering Science Edition), 2015, 47(S2): 81–88. DOI: 10.15961/j.jsuese.2015.s2.013. [21] 汪小梦, 朱哲明, 施泽彬, 等. 基于VB-SCSC岩石试样的动态断裂韧度测试方法研究 [J]. 岩石力学与工程学报, 2018, 37(2): 302–311. DOI: 10.13722/j.cnki.jrme.2017.0351.WANG Xiaomeng, ZHU Zheming, SHI Zebin, et al. A method measuring dynamic fracture toughness of rock using VB-SCSC specimens [J]. Chinese Journal of Rock Mechanics and Engineering, 2018, 37(2): 302–311. DOI: 10.13722/j.cnki.jrme.2017.0351. [22] 刘德顺, 李夕兵. 冲击机械系统动力学 [M]. 北京: 科学出版社, 1999. [23] 郭伟国, 李玉龙, 索涛编. 应力波基础简明教程 [M]:西安: 西北工业大学出版社; 2007: 44−52. [24] ZHE Zheming, XIE Heping, MOHANTY B. Numerical investigation of blasting-induced damage in cylindrical rocks [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(2): 111–121. DOI: 10.1016/j.ijrmms.2007.04.012. [25] ZHU Zheming, MOHANTY B, XIE Heping. Numerical investigation of blasting-induced crack initiation and propagation in rocks [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(3): 412–424. DOI: 10.1016/j.ijrmms.2006.09.002. [26] 朱哲明, 刘凯, 康骥鸣, 等. 缓慢卸载P波诱发岩体不连续断裂破坏的可能性研究 [J]. 岩石力学与工程学报, 2014, 33(2): 3948–3955. DOI: 10.13722/j.cnki.jrme.2014.s2.070.ZHU Zheming, LIU Kai, KANG Jiming, et al. Study of slowly unloading P-waves causing discrete fracture mechanism [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(2): 3948–3955. DOI: 10.13722/j.cnki.jrme.2014.s2.070. [27] 周磊, 朱哲明, 董玉清, 等. 中低速冲击载荷下巷道内裂纹的动态响应 [J]. 岩石力学与工程学报, 2017, 36(6): 1363–1372. DOI: 10.13722/j.cnki.jrme.2016.1403.ZHOU Lei, ZHU Zheming, DONG Yuqing, et al. Dynamic response of cracks in tunnels under impact loading of medium-low speed [J]. Chinese Journal of Rock Mechanics and Engineering, 2017, 36(6): 1363–1372. DOI: 10.13722/j.cnki.jrme.2016.1403. [28] 刘瑞峰, 朱哲明, 李盟, 等. 爆炸载荷下I型裂纹的起裂及扩展规律研究 [J]. 岩石力学与工程学报, 2018, 37(2): 392–402. DOI: 10.13722/j.cnki.jrme.2017.1126.LIU Ruifeng, ZHU Zheming, LI Meng, et al. Initiation and propagation of mode I crack under blasting [J]. Chinese Journal of Rock Mechanics and Engineering, 2018, 37(2): 392–402. DOI: 10.13722/j.cnki.jrme.2017.1126. [29] 王蒙, 朱哲明, 王雄. 冲击荷载作用下的Ⅰ/Ⅱ复合型裂纹扩展规律研究 [J]. 岩石力学与工程学报, 2016, 35(7): 1323–1332. DOI: 10.13722/j.cnki.jrme.2015.1260.WANG Meng, ZHU Zheming, WANG Xiong. The growth of mixed-mode Ⅰ/Ⅱ crack under impacting loads [J]. Chinese Journal of Rock Mechanics and Engineering, 2016, 35(7): 1323–1332. DOI: 10.13722/j.cnki.jrme.2015.1260. [30] 周磊, 朱哲明, 应鹏,等. 巷道内I型及I/II复合型裂纹在冲击载荷作用下的断裂行为分析 [J]. 振动与冲击, 2018, 37(13): 33. DOI: 10.13465/j.cnki.jvs.2018.13.033.ZHOU Lei, ZHU Zhe-ming, YING Peng, et al. Analysis of fracture behavior of mode I and mixed mode I/II cracks in tunnel under impact loads [J]. Journal of Vibration and Shock, 2018, 37(13): 33. DOI: 10.13465/j.cnki.jvs.2018.13.033. [31] CHEN Y M. Numerical computation of dynamic stress intensity factors by a Lagrangian finite-difference method (the HEMP code) [J]. Engineering Fracture Mechanics, 1975, 7(4): 653–660. DOI: 10.1016/0013-7944(75)90021-1. [32] 陈景杰, 黄一, 吴智敏. 平行双裂纹应力强度因子的计算方法 [J]. 上海交通大学学报, 2012, 46(8): 1263–1268. DOI: 10.16183/j.cnki.jsjtu.2012.08.015.CHEN Jinjie, HUNAG Yi, WU Zhimin. A new method to calculate stress intensity factor for parallel double cracks [J]. Journal of Shanghai Jiao Tong University, 2012, 46(8): 1263–1268. DOI: 10.16183/j.cnki.jsjtu.2012.08.015. [33] FREUND L B. Dynamic fracture mechanics [J]. Cambridge Monographs on Mechanics and Applied Mathematics, 1992, 72(8): 383–384. DOI: 10.1002/zamm.19920720818. 期刊类型引用(3)
1. 蔡安江,梅浩鹏,于海滨. 成组立模成型机模板结构优化设计研究. 机械设计与制造. 2024(04): 379-383 .  百度学术
百度学术2. 王春彭,高汝鑫,廉艳平,成湛山,李明健. 面向抗冲击结构尺寸优化的自训练分类判断优化设计方法. 力学学报. 2024(07): 1861-1875 .  百度学术
百度学术3. 乔赫廷,高群,熊展. 基于偶应力理论的双向进化结构优化法. 机电工程技术. 2022(07): 10-14+44 .  百度学术
百度学术其他类型引用(3)
-










 下载:
下载:







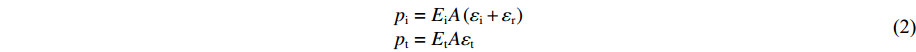
























 下载:
下载:













 百度学术
百度学术












