Similarity law of cylindrical ammunition explosions in air
-
摘要: 针对战场损伤试验中弹药费用高、使用风险系数大的问题,采用量纲分析的方法对圆柱形弹药相似性模型的建立方法进行分析,研究原型同相似性模型之间冲击波峰值压力的关系,利用有限元仿真软件AUTODYN对冲击波传播过程进行数值仿真,并结合实弹试验数据对相似模型的有效性进行验证。研究结果表明,AUTODYN仿真结果同量纲分析结果一致,同时与实弹试验结果也吻合较好,说明在实际试验中可以使用缩比模型替代原型进行试验。该研究为弹药缩比模型在战场损伤试验中的使用提供了理论基础,具有一定的工程价值。Abstract: In order to solve the issues of high cost and large risk factor of ammunition explosion in the battlefield damage test, the method of dimensional analysis is used to analyze the establishment method of cylindrical ammunition similar models. The explosive power field relationship between different similar models and an original model is studied. The AUTODYN finite element simulation software is used to simulate the explosive power field, and the effectiveness of the similar models is verified by real-experiment data. The results show that the simulated results are consistent with the results of both the dimensional analysis and the actual tests. Therefore, in the actual test, the similar model can be used instead of the original model. This study provides a theoretical basis for the use of the ammunition similar model in battlefield damage test and has certain engineering value.
-
Key words:
- battlefield damage test /
- similarity theory /
- dimensional analysis /
- shock wave
-
钻地弹终点毁伤效应研究一直是武器研发和工程防护领域共同关注的热点问题,其中动能弹体打击混凝土靶体的侵彻深度是主要研究对象之一,是表征靶体毁伤破坏程度最重要的参数。近几十年来,学者们围绕弹体侵彻开展了大量的研究工作,取得了一系列理论、数值及实验成果[1-11]。然而上述成果大多基于缩比侵彻实验展开,能否直接推广至真实场景尚待商榷。其中的关键问题在于侵彻相似律是否成立,如果成立,则侵彻深度不存在尺寸效应,上述成果才可直接推广至原型。
已有关于弹体侵彻混凝土靶体相似律的研究中,多数持相似律成立的观点。徐建波[12]针对不含粗骨料的砂浆靶体(单轴抗压强度fc=16.5 MPa),采用比例系数为2的几何相似尖卵形弹体(长径比为10,弹径d=10,20 mm、弹体质量M=58/460 g)对侵彻相似律是否成立进行了实验探讨,结果显示两组实验的无量纲侵彻深度(侵深除以弹径)基本重合,证明该弹径范围内弹体侵彻砂浆靶体的相似律是成立的。而对于含粗骨料的混凝土靶体,Frew等[8]进行了类似的实验研究,其中几何相似尖卵形弹体的比例系数为1.5,靶体抗压强度fc=58.4 MPa,两组侵彻实验的无量纲侵彻深度数据大致重合(d=30.5 mm弹的数据稍高)。许三罗等[13]基于量纲分析再结合徐建波[12]实验认为侵彻满足相似律。卢江仁等[14]基于相似分析和数值模拟认为弹体侵彻混凝土的深度满足相似律。高光发等[15]通过数值模拟探讨了应变率对侵彻相似律的影响,结果表明尺寸为1∶9的两组相似模型实验的无量纲侵彻深度相差只有2.9%,仍可认为满足相似律。
然而,上述研究结论缺乏缩比幅度更大的实验数据支撑,讨论范围窄。Forrestal等[16]在采用相对较大直径弹体(d=76.2 mm)开展的侵彻实验中发现侵彻似乎存在尺寸效应,因为该团队前期基于弹径12.9 mm≤d≤30.5 mm侵彻实验提出的侵深半理论公式[7]并不适用于这些大弹实验,公式预测值明显低于实验值。同时,半理论公式[7]也不适用于Gomez等[17]开展的小直径(d=6.35 mm)弹体侵彻混凝土实验,计算结果明显高于实验值(详见第2节)。由此可见,弹体侵彻混凝土存在尺寸效应,这意味着大多数侵彻研究成果并不能直接应用于实际工程。
本文中从相似模型、缩比侵彻实验数据出发,分析了侵彻相似律成立的条件,探讨了引起弹体侵彻混凝土靶中尺寸效应的原因。为研究侵彻尺寸效应,开发了粗骨料形状、位置随机的混凝土二维细观有限元建模程序,并开展了不同尺寸弹体侵彻非均质混凝土材料的数值实验。
1. 弹体侵彻混凝土靶体的相似模型
弹体垂直侵彻混凝土靶体的侵彻深度P可表述为弹、靶材料性质以及几何参数的函数:
P=f(M,d,L,ψ,ρp,σp,Ep,νp,V0,ρt,σt,Et,νt,H,W) (1) 式中:M、d、L以及ψ分别为弹体质量、直径、长度以及头部曲率半径与弹径之比(CRH);ρp、σp、Ep、vp以及V0分别为弹体的密度、强度、弹性模量、泊松比以及侵彻初速度;ρt、σt、Et、vt分别为混凝土靶体的密度、强度、弹性模量、泊松比;H、W分别为混凝土靶体的厚度、边长(方形靶面)或直径(圆形靶面)。
以ρp、d、σt作为独立量纲基本量,对式(1)无量纲化可得
Pd=f1(Mρpd3,Ld,σpσt,Epσt,V0(σt/ρp)0.5,ρtρp,Etσt,νt,νp,ψ,Hd,Wd) (2) 根据Π定律,对于两组侵彻实验,如果式(2)中所有无量纲自变量保持一致,则侵彻实验结果一致。对于原型及缩比侵彻实验,弹、靶材料不变,弹体外形完全相似,式(2)可简化为
Pd=f1(V0(σt/ρp)0.5,Hd,Wd) (3) 当模型与原型实验中弹体侵彻初速度V0保持不变,靶体尺寸与弹径变化成比例,则原型实验的无量纲侵彻深度与模型实验的无量纲侵彻深度相等,即
(Pd)prototype=(Pd)model (4) 式中:下标prototype、model分别代表原型(或大尺寸实验)和模型(或小尺寸实验)。表1列出了侵彻原型与缩比模型几何比例为λ时,原型与模型实验中各物理量的比值。
表 1 相似模型中原型相对于模型的各物理量Table 1. Parameters in the prototype model relative to the reduced model变量 比例 变量 比例 弹径 λ 侵彻深度 λ 弹长 λ 加速度 λ−1 弹体密度 1 侵彻持续时间 λ 弹体质量 λ3 弹体轴向阻力 λ2 侵彻初速度 1 阻应力 1 近几十年来提出的理论公式、半理论半经验公式几乎都暗含侵彻深度满足相似律的假定,即几何形状相似但尺寸不同的弹体其无量纲侵彻深度相同,如图1所示。图1(a)给出了几何相似的四种不同尺寸弹体的示意图,其中左侧最小弹体与Hanchak等[18]所用弹体完全相同,该弹质量M=0.5 kg、直径d=25.4 mm、头部曲率半径与弹径之比为3。其余弹体与左侧弹体外形完全相似,放大比例分别为5∶1、10∶1及15∶1。图1(b)给出了采用Forrestal等[7]、Frew等[8]半理论公式计算出的几何相似弹体侵彻单轴抗压强度fc=35 MPa半无限厚混凝土靶体的无量纲侵彻深度,从图中可以看出几何尺寸比为15∶1的两种弹体的无量纲侵彻深度完全重合。
上述相似理论是用缩比侵彻实验来预测原型侵彻实验的理论基础。但如果上述分析或者实际实验中漏掉了可影响结果的重要参数,则侵彻相似律可能并不成立,从而出现侵彻尺寸效应。
2. 侵彻实验中的尺寸效应
20世纪末,美国桑迪亚实验室Forrestal等[7]、Frew等[8]、Forrestal等[19]和先后开展了一系列侵彻缩比实验,并基于空腔膨胀模型给出的阻力函数形式建立了半理论-半经验的侵彻深度计算模型:
Pd=2Mπd3ρN2ln(1+N2ρV12Sfc)+2 (5a) 式中:N2为弹体头型系数[7],fc为混凝土单轴抗压强度,V1为弹体在开坑区-隧道区界面处的瞬时速度[7];无量纲阻应力系数S由侵彻实验数据反推得到,即通过反解式(5)得到S表达式,再将侵彻实验数据代入表达式得到不同强度混凝土的S值,最后拟合S与混凝土单轴抗压强度fc关系[8]得到:
S=82.6(fc/106)−0.544 (5b) 表2给出了拟合式(6)所用到的全部侵彻实验相关信息。
fc/MPa 13.5 21.6 36.2 51 58.4 62.8 96.7 a1)/mm 4.8 4.8 9.52) 9.5 9.5 9.5 9.52) d/mm 12.9 12.9 26.9 30.5 20.3/30.5 20.3 26.9 d/a 2.69 2.69 2.83 3.21 2.14/3.21 2.14 2.83 M/kg 0.064 0.064 0.906 1.6 0.478/1.62 0.478 0.904 注:1) a 为混凝土靶体中粗骨料的最大粒径,2) 表示粒径没有明确给出。 如果相似律满足,由表1可知S值与弹体尺寸无关。对此,Frew等[8]进行了相关实验探讨,并认为侵彻相似律是成立的。实验中两组弹体几何形状完全相似,d=30.5,20.3 mm,fc=58.4 MPa。两组实验中P/d基本重合,如图2所示。由两组侵彻实验数据反推的针对fc=58.4 MPa混凝土的S值分别为8.7(小弹)和7.9(大弹),文献[8]中认为两者差别不大,拟合式(6)时取其平均值8.3。基于这一系列研究,Forrestal等[7]、Frew等[8]提出的相似律总是成立的侵彻式(5)得到了广泛认可和引用。
但到2003年,Forrestal等[16]为研究弹体侵彻减速度,需将加速度测试仪载入弹体内部,因而该弹直径(d=76.2 mm)比表2中最大弹径还大一倍以上。实验中,混凝土抗压强度分别为23、39 MPa,粗骨料最大粒径都为9.5 mm。实验结果表明,侵彻深度有很明显的尺寸效应,该团队侵彻深度式(5)计算的侵彻深度明显低于实验数据,如图3(a)所示;相应的由d=76.2 mm弹体侵彻实验反推的S值要明显低于式(6)计算值。另一组实验也表明弹体阻应力似乎和弹径有关:文献[17]中报道了直径d=6.35 mm弹体侵彻fc=38.15 MPa混凝土靶体时(粗骨料为最大粒径9.5 mm的石灰岩),由式(6)计算的S值为11.39,而由实验数据反推的S值为15.47,相差35.78%;相应的由式(5)计算的侵彻深度明显高于实验值,如图3(b)所示。现在反过来看Frew等[8]进行的侵彻实验,从图2中可以看出实际上大弹(d=30.5 mm)侵彻深度确实要比小弹(d=20.3 mm)的实验值高,只是因为两几何相似弹体的尺寸差别太小,导致作者忽略了实验结果的差别,得出了不合适的结论。
此外,早期的混凝土侵彻经验公式也表明侵彻深度存在尺寸效应,这些公式基本都由不同尺寸侵彻实验数据拟合而来,代表着早期的实验数据。比如ACE公式[6, 20]中实验弹径范围为37~155 mm,而修正的NDRC公式[6, 21]中包含d=12.7~155 mm范围实验数据[22]。图4给出了采用ACE公式以及修正的NDRC公式计算的4组几何相似弹体(见图1)侵彻fc=35 MPa混凝土靶的无量纲侵彻深度。从图中可以明显看出,随着弹体几何尺寸的变大,弹体侵彻能力有大幅提升(计算曲线并没有和图1(b)中一样重合),例如在图4(a)中,在侵彻初速度200~1 000 m/s范围内,15倍尺寸弹体(d=381 mm)的侵彻深度比通过1倍模型弹体侵彻深度按相似律换算的值要高64.98%~77.51%。
从上述实验及经验公式看,侵彻相似律似乎并不成立,其原因可能在于相似模型以及实验中忽略掉了某一重要因素的影响,也有可能侵彻混凝土尺寸效应的原因就如混凝土单轴压缩实验强度随尺寸增大而变小一样。笔者更倾向于前一种可能,因为上述实验中有一个重要细节需要特别注意,即图2~3混凝土靶中粗骨料的粒径及力学特性在抵抗大、小弹体侵彻时没有变化,图4经验公式拟合所用实验中的靶体也很有可能具有相同的粗骨料。如果粗骨料对侵彻深度有影响,则不变的骨料特征会让相似模型出现紊乱,而已往的分析模型基本都忽略了粗骨料的影响。
3. 侵彻深度相似律成立的条件
实际上,实验研究[23, 24]表明相同速度弹体侵彻混凝土靶体的侵彻阻力确实与粗骨料的材性、尺寸、体积率相关。所以在推导侵彻相似模型时,必须考虑粗骨料的影响,于是相似模型式(3)将变为
Pd=f2(V0(σt/ρp)0.5,Hd,Wd,ad,Va,fc,aσt) (6) 式中:Va为骨料体积率,fc,a为骨料强度,a表面上是粗骨料的最大粒径,实际上表征的是某特定级配粗骨料的最大粒径,脱离级配单独提粗骨料最大尺寸是不合适的。
当模型与原型实验中弹体侵彻初速度V0保持不变,靶体尺寸与弹径变化成比例,但骨料粒径不变时,原型实验的无量纲侵彻深度与模型实验的无量纲侵彻深度并不相等,这就可以解释上节几个实验中侵彻相似律不成立的原因。如果混凝土靶体中的粗骨料材料、体积率不变,尺寸随着弹体进行同样的放大或缩小,即d/a保持不变,那么侵彻深度应该满足相似律。幸运的是正好有一组由Canfield等[25]报道的实验可以验证这一观点:作者进行了两组弹、靶完全成比例的侵彻实验,实验中原型弹体直径d=76.2 mm,质量M=5.9 kg,而1/10缩比弹体d=7.62 mm,M=0.005 9 kg,两组弹体的长径比相同且CRH都等于1.5。同时,作者将靶中钢筋以及粗骨料的尺寸也按10∶1进行了等比例缩放,以确保靶体细节也严格相似(两组实验中混凝土靶的强度相差0.5 MPa,应可忽略不计)。图5给出了文献[25]中两组实验的无量纲侵彻深度与侵彻初速度的关系,从图中可以看出两组实验数据基本重合,可认为满足相似律。从这一重要的实验可知:只要模型以及原型实验中的弹体与混凝土靶(包括粗骨料)严格按照相似律进行等比例设计,侵彻深度相似律是成立的。此外,结合式(7)、图5以及第2节分析,可以认为不变的骨料特征(粗骨料尺寸未随着弹体的尺寸进行同样的放大或缩小)是引起不同尺寸弹体侵彻实验以及经验公式中侵彻深度不满足相似律(出现尺寸效应)的原因。这也说明侵彻尺寸效应与混凝土强度实验中的尺寸效应并不是同一回事。当然,需要指出的是,需要更多等比例侵彻实验来验证侵彻相似律是否对更大尺寸弹体以及不同强度混凝土靶都成立。
4. 基于细观有限元模型的数值模拟
虽然当模型、原型实验中的弹靶严格相似时,侵彻相似律成立,但是确定粗骨料未同步缩放时侵彻深度的尺寸效应幅值仍然非常重要。因为,在实际防护结构中,混凝土靶的骨料特征是固定不变的,不可能随来袭武器尺寸的变化而变化。从这一角度看,近30年来发展的一系列理论侵彻公式、半理论侵彻公式,例如式(5),由于其总是满足侵彻相似律而又只得到了小尺寸缩比弹体侵彻实验数据的验证,所以直接采用这些公式来进行混凝土防护结构抗原型钻地弹打击的设计和建设是偏于危险的。
本节拟采用细观数值模拟的方法来探讨由骨料引起的侵彻尺寸效应。混凝土细观有限元模型是指将混凝土组分包括砂浆、骨料等分别建模的模型。为提高效率,本节将基于二维有限元模型展开讨论。
4.1 混凝土二维细观有限元模型
本节混凝土二维细观模型的建立基于将代表粗骨料的几何模型在代表砂浆的规则背景有限元网格上进行投影从而确定骨料网格分布的思想。具体建模流程可简化为以下5步:(1)采用正方形网格对混凝土靶体进行网格划分并将网格属性定义为砂浆,在保证可计算前提下尽量将网格画细;(2)生成一个具有随机尺寸、随机形状的骨料二维几何模型;(3)将骨料几何模型随机投放在混凝土试件覆盖的范围内,并保证新投放骨料不与已投放骨料有重叠或接触;(4)将落入步骤3中投放的骨料几何范围内的网格属性由砂浆变为粗骨料;(5)重复步骤(2)~(4),直到骨料体积率满足要求。表征粗骨料几何形状的随机多边形生成步骤如图6所示,最后生成的有限元模型如图7所示,可以看出本文建立的混凝土二维有限元模型在骨料随机分布、随机形状以及高体积率方面都有不错的效果。
4.2 弹、靶的材料模型及参数
弹体采用*MAT_RIGID材料模型[26],密度为8 020 kg/m3;砂浆和粗骨料的力学行为则用*MAT_ JOHNSON_HOLMQUIST_CONCRETE(HJC)材料模型[27]来描述。表3列出了砂浆以及粗骨料的HJC模型参数,参数的选取方法详见文献[28],未列参数沿用文献[27]中取值。为避免单元过度畸变,引入*MAT ADD EROSION来判断材料失效,并将砂浆及粗骨料单元的侵蚀准则都定义为最大主应变0.42。
表 3 砂浆及粗骨料的HJC材料模型参数Table 3. Parameters of the HJC material model for cement and aggregate参数 砂浆 骨料 参数 砂浆 骨料 单轴抗压强度 fc/MPa 12 120 弹性极限静水压力Pcrush/MPa 4.0 40 密度 ρ /(kg·m−3) 2 000 2 660 弹性极限体积应变Ucrush 5.4×10−4 1.1×10−3 剪切模量 G/GPa 5.55 26.93 转折静水压力Plock/GPa 1 1 极限面参数A 0.79 0.79 压实体积应变Ulock 0.1 0.1 极限面参数B 1.6 1.6 压力系数K1/GPa 17 17 压力硬化系数 N 0.61 0.61 压力系数K2/GPa 38 38 抗拉强度 T/MPa 1.1 12 压力系数K3/GPa 29.8 29.8 4.3 数值模拟实验及分析
基于有限元程序LS_DYNA开展弹体侵彻混凝土靶的数值模拟研究。混凝土细观数值模型由4.1节中开发的程序生成,弹体建模及网格划分在ANSYS中完成,所建二维轴对称模型如图9所示。
为探讨侵彻尺寸效应,开展了3种几何相似尖卵形弹体侵彻相同材料靶体的数值实验。以文献[18]中弹体为参考弹体,3种弹体的尖卵形头部CRH都为3,长径比都为5.66,直径分别为12.7、25.4(参考弹体)、50.8 mm,侵彻初速度都为400 m/s;混凝土模型的网格单元为0.5 mm的正方形,骨料体积率为42%,骨料粒径范围为5~10 mm,靶体尺寸根据弹体尺寸进行等比例缩放,建模时先建出最大尺寸(300 mm×1 000 mm2)靶体,其余尺寸的靶体模型在最大尺寸模型上以相同弹着点为基准按比例切割。
图8给出了参考弹体侵彻过程中,细观混凝土有限元模型(150 mm×500 mm2)中的von Mises应力分布,可以看出靶内的应力分布不均匀,弹体附近的粗骨料上明显存在应力集中。图9给出了相同弹体侵彻均质混凝土靶体时的von Mises应力分布,其中均质混凝土的强度为48 MPa,相关材料参数选取参见文献[28]。与图8中非均质模型相比,均质靶体中的应力分布非常规则,这也是后文中为什么均质模型不能模拟出尺寸效应而细观模型可以的原因。
3种几何相似弹体侵彻均质混凝土靶体(fc=48 MPa)的侵彻深度分别为0.100 1、0.198 0、0.393 1 m。以参考弹体的无量纲侵彻深度为基准,则三者的无量纲侵彻深度之比为1.011∶1.0∶0.995,可以认为基本重合。严格来说,均质模型模拟的大弹侵彻能力反而比小弹要稍微弱一点,这与第2节中的侵彻尺寸效应是相反的。
3种弹体侵彻非均质混凝土靶体的侵彻深度为别为0.134 8、0.283 9、0.598 8 m。图10给出了相应模拟结果,图中纵坐标为相对无量纲侵彻深度P/(λPref)=[P/(λdref)]/(Pref/dref),dref=25.4 mm为参考弹径,Pref为该弹的侵彻深度,λ为与参考弹体(dref=25.4 mm)相比的弹体相似缩放系数。如果侵彻相似律成立,则图中P/(λPref)始终等于1。从图中可以看出基于混凝土细观模型的数值模拟结果成功地反映出了无量纲侵彻深度随弹体尺寸变大而增大的尺寸效应,这也进一步验证了第3节中是不变的骨料特征(粗骨料尺寸未随着弹体的尺寸进行同样的放大或缩小)引起侵彻尺寸效应这一观点。
在半理论半经验侵彻公式,即式(5)计算结果基础上,引入图10中数值模拟给出的侵彻尺寸效应,得到图11中与实验结果的对比。其中尺寸效应的引入即在式(5)基础上乘以(d/2.54a)0.09,其中2.54为数值模拟中参考弹体直径25.4 mm与粗骨料最大粒径10 mm的比值,当然这其中还暗含式(6)中S所依据的侵彻实验中d/a约等于2.54这一条件。从图11可以看出,考虑侵彻尺寸效应后的公式预测值与实验数据基本吻合,但仍然有一定差距,即在预测小尺寸(相对于25.4 mm)弹体时稍高而在预测大尺寸侵彻时稍低。这说明数值计算所得的侵彻尺寸效应幅值小于真实的尺寸效应幅值,这有可能是因为二维对称模型中粗骨料实际表征的是环形圈,而非真实粗骨料形状所致。另外,需要指出的是,根据式(7)侵彻尺寸效应的幅值很有可能还与砂浆强度、粗骨料强度以及体积率相关,这还需要进行更多的深入研究,尤其是实验研究。
5. 结 论
为探讨刚性弹体侵彻混凝土靶体中的尺寸效应,基于相似模型、实验数据、经验公式以及细观数值模拟技术,开展了一系列的分析讨论,主要工作及结论如下:
(1)通常情况下,弹体打击混凝土靶体的侵彻深度存在尺寸效应,且无量纲侵彻深度随弹体尺寸变大而增大。但如果模型以及原型实验中的弹体与混凝土靶体(包括粗骨料)严格按照相似律进行等比例设计,刚性弹侵彻深度相似律是成立的。不变的骨料特征是引起侵彻实验及经验公式中尺寸效应的主要原因。
(2)为研究由不变的混凝土粗骨料所引起的侵彻尺寸效应,开发了混凝土二维细观有限元建模程序,开展的数值实验成功地反映出了无量纲侵彻深度随弹体尺寸变大而增大的尺寸效应。
-
表 1 弹药空气中爆炸相关参数及其量纲
Table 1. Explosion-related parameters and their dimensions
物理量 符号 量纲 物理量 符号 量纲 峰值压力 pm ML−1T−2 空气初始状态压力 p0 ML−1T−2 装药质量 Q M 空气密度 ρa ML−3 装药密度 ρe ML−3 空气绝热指数 γa 1 质量化学能 e L2T−2 弹壳密度 ρs ML−3 膨胀指数 γe 1 弹壳弹性模量 Es ML−1T−2 长径比 k 1 弹壳泊松比 μs 1 爆距 R L 弹壳屈服极限 Ys ML−1T−2 赤道面夹角 α 1 弹壳厚度 hs L 轴向夹角 φ 1 表 2 弹药原型及缩比模型结构参数值
Table 2. Ammunition prototype and scale model structural parameter values
弹药模型 TNT质量/kg 长度/mm 半径/mm 外壳厚度/mm 原型 64.000 480 160 6.00 1/2缩比模型 8.000 240 80 3.00 1/3缩比模型 2.370 162 54 2.00 1/4缩比模型 1.000 120 40 1.25 1/5缩比模型 0.512 96 32 1.20 表 3 缩比模型中同原型具有相同冲击波峰值压力的爆距
Table 3. Detonation distance with same peak pressure of original model in the similar model
弹药类型 爆距/m 原型 1.000 2.000 3.000 4.000 5.000 6.000 7.000 1/2缩比模型 0.500 1.000 1.500 2.000 2.500 3.000 3.500 1/3缩比模型 0.333 0.667 1.000 1.333 1.667 2.000 2.333 1/4缩比模型 0.250 0.500 0.750 1.000 1.250 1.500 1.750 1/5缩比模型 0.200 0.400 0.600 0.800 1.000 1.200 1.400 表 4 轴向方向上冲击波峰值压力
Table 4. Peak pressure of shock wave in the axial direction
弹药类型 峰值压力/kPa 原型 12 744.1 4 763.6 1 894.9 911.9 552.1 376.7 277.7 1/2缩比模型 12 719.5 4 761.2 1 892.5 907.7 540.0 366.3 273.6 1/3缩比模型 12 764.2 4 819.8 1 922.4 929.8 560.6 382.6 280.3 1/4缩比模型 12 739.8 4 752.3 1 897.0 910.3 551.0 374.6 275.7 1/5缩比模型 12 709.5 4 714.0 1 891.2 910.6 543.1 380.5 279.4 表 5 径向方向上冲击波峰值压力
Table 5. Peak pressure of shock wave in the radial direction
弹药类型 峰值压力/kPa 原型 9 927.2 4 357.5 2 160.9 1 145.5 692.0 466.4 349.6 1/2缩比模型 9 925.5 4 354.0 2 161.2 1 144.3 691.9 464.4 345.4 1/3缩比模型 10 099.0 4 465.6 2 198.9 1 171.7 701.6 474.8 353.6 1/4缩比模型 9 921.2 4 356.2 2 158.8 1 138.9 690.0 463.3 347.3 1/5缩比模型 9 916.9 4 355.2 2 157.2 1 134.9 685.9 465.3 343.5 表 6 缩比模型轴向方向峰值压力绝对值偏差
Table 6. Absolute deviation of peak pressure of scale model in the axial direction
弹药类型 缩比模型轴向峰值压力同原型偏差/% 1/2缩比模型 0.20 0.05 0.13 0.46 2.19 2.76 1.46 1/3缩比模型 0.16 1.18 1.45 1.96 1.55 1.58 2.77 1/4缩比模型 0.04 0.24 0.11 0.18 0.19 0.55 0.72 1/5缩比模型 0.27 1.04 0.21 0.14 1.63 1.02 0.63 表 7 缩比模型径向方向峰值压力绝对值偏差
Table 7. Absolute deviation of peak pressure of scale model in the radial direction
弹药类型 缩比模型径向峰值压力偏差/% 1/2缩比模型 0.02 0.08 0.01 0.10 0.02 0.43 1.19 1/3缩比模型 1.73 2.48 1.76 2.29 1.39 1.80 1.16 1/4缩比模型 0.06 0.03 0.10 0.58 0.29 0.67 0.66 1/5缩比模型 0.10 0.05 0.17 0.93 0.89 0.24 1.73 表 8 冲击波峰值压力测量距离
Table 8. Measure distance of peak pressure
弹药类型 测量距离/m 原型 14.00 19.00 24.00 缩比模型A1 11.61 15.75 19.90 缩比模型A2 7.31 9.92 12.53 缩比模型A3 4.61 6.25 7.90 表 9 现场试验与仿真试验冲击波峰值压力
Table 9. Peak pressure in field and simulation tests
试验类型 冲击波峰值压力/kPa 原型实际试验 257.3 185.5 145.3 缩比模型A1仿真试验 248.2 176.5 137.2 缩比模型A2仿真试验 246.0 176.0 137.2 缩比模型A3仿真试验 247.5 177.0 136.7 表 10 冲击波峰值压力误差
Table 10. Error of peak pressure
试验
类型误差/% 缩比模型A1仿真试验 3.5 4.9 5.6 缩比模型A2仿真试验 4.4 5.1 5.6 缩比模型A3仿真试验 3.8 4.6 5.9 -
[1] 吴晓颖, 李帆, 张万君, 等. 装备毁伤模拟试验方案的设计与优化 [J]. 兵器装备工程学报, 2014, 35(10): 5–7. DOI: 10.11809/scbgxb2014.10.002.WU Xiaoying, LI Fan, ZHANG Wanjun, et al. Design and optimization of equipment damage simulation test [J]. Journal of Ordnance Equipment Engineering, 2014, 35(10): 5–7. DOI: 10.11809/scbgxb2014.10.002. [2] 石全. 装备战伤理论与技术 [M]. 北京: 国防工业出版社, 2007: 243−244. [3] 杨亚东, 李向东, 王晓鸣, 等. 密闭空间内爆炸缩比相似模型研究 [J]. 振动与冲击, 2014, 33(2): 128–133. DOI: 10.3969/j.issn.1000-3835.2014.02.025.YANG Yadong, LI Xiangdong, WANG Xiaoming, et al. Scale similarity model of internal explosion in closed field [J]. Journal of Vibration and Shock, 2014, 33(2): 128–133. DOI: 10.3969/j.issn.1000-3835.2014.02.025. [4] 杨亚东, 李向东, 王晓鸣, 等. 钢筋混凝土结构内爆炸相似模型试验研究 [J]. 南京理工大学学报, 2016, 40(2): 135–141.YANG Yadong, LI Xiangdong, WANG Xiaoming, et al. Experimental study on similarity model of reinforce concrete structure under internal explosion [J]. Journal of Nanjing University of Science and Technology, 2016, 40(2): 135–141. [5] 吕祥锋, 潘一山, 李忠华, 等. 冲击波作用下巷道破坏规律相似模拟研究 [J]. 振动与冲击, 2011, 30(10): 212–215. DOI: 10.3969/j.issn.1000-3835.2011.10.041.LV Xiangfeng, PAN Yishan, LI Zhonghua, et al. Similarity simulation tests for failure law of a rock burst roadway under shock wave [J]. Journal of Vibration and Shock, 2011, 30(10): 212–215. DOI: 10.3969/j.issn.1000-3835.2011.10.041. [6] 张玉磊, 王胜强, 袁建飞, 等. 不同量级TNT爆炸冲击波参数相似律实验研究 [J]. 弹箭与制导学报, 2016, 36(6): 53–56.ZHANG Yulei, WANG Shengqiang, YUAN Jianfei, et al. Experimental research on similarity law of explosive shock wave parameters with different orders of magnitude TNT [J]. Journal of Projectiles, Rocks, Missiles and Guidance, 2016, 36(6): 53–56. [7] 荣吉利, 何轩, 项大林, 等. 定向战斗部水下爆炸相似性研究 [J]. 兵工学报, 2014, 35(9): 1329–1334. DOI: 10.3969/j.issn.1000-1093.2014.09.001.RONG Jili, HE Xuan, XIANG Dalin, et al. The similarity research on underwater explosion of directional warhead [J]. Acta Armamentarii, 2014, 35(9): 1329–1334. DOI: 10.3969/j.issn.1000-1093.2014.09.001. [8] 刘文韬, 姚熊亮, 李帅, 等. 离心机水下爆炸缩比实验原理及数值研究 [J]. 爆炸与冲击, 2016, 36(6): 789–796. DOI: 10.11883/1001-1455(2016)06-0789-08.LIU Wentao, YAO Xiongliang, LI Shuai, et al. Experimental principle and numerical study of scaled-down underwater explosion model on a centrifuge apparatus [J]. Explosion and Shock Waves, 2016, 36(6): 789–796. DOI: 10.11883/1001-1455(2016)06-0789-08. [9] LI Jian, RONG Jili. Experimental and numerical investigation of the dynamic response of structures subjected to underwater explosion [J]. European Journal of Mechanics, 2012, 32(1): 59–69. DOI: 10.1016/j.euromechflu.2011.09.009. [10] 朱如意. 杀爆榴弹动静态爆炸仿真及毁伤威力差异性分析[D]. 石家庄: 军械工程学院, 2016: 31−34. 期刊类型引用(14)
1. 徐天涵,谢方,何勇. 刚性弹侵彻缩比实验尺寸效应分析. 南京理工大学学报. 2024(02): 141-147 .  百度学术
百度学术2. 余庆波,钟世威,葛超. 破片侵彻混凝土毁伤效应研究. 北京理工大学学报. 2023(03): 259-266 .  百度学术
百度学术3. 徐立志,韩志远,周峰,胖世铭,杜忠华,高光发. PELE侵彻金属靶破碎效应的相似分析. 高压物理学报. 2023(01): 141-150 .  百度学术
百度学术4. 程月华,周飞,吴昊. 抗战斗部侵彻爆炸作用的混凝土遮弹层设计. 爆炸与冲击. 2023(04): 113-130 .  本站查看
本站查看5. 洪智捷,杨耀宗,孔祥振,方秦. 刚性弹侵彻/贯穿混凝土靶体的工程实用化计算模型. 爆炸与冲击. 2023(08): 67-80 .  本站查看
本站查看6. 李旭,刘彦,闫俊伯,时振清,王虹富,许迎亮,黄风雷. 基于区块划分并行填充的混凝土细观建模方法及其在弹体超高速侵彻中应用. 兵工学报. 2023(12): 3543-3561 .  百度学术
百度学术7. 高飞,邓树新,张国凯,纪玉国,刘晨康,王明洋. 缩比模型弹侵彻岩石靶尺寸效应试验研究与理论分析. 兵工学报. 2023(12): 3601-3612 .  百度学术
百度学术8. 程月华,姜鹏飞,吴昊,谭可可,方秦. 考虑尺寸效应的典型钻地弹侵彻混凝土深度分析. 爆炸与冲击. 2022(06): 85-99 .  本站查看
本站查看9. 张见升,孙浩,李超,李波. 典型破片破坏混凝土靶毁伤试验研究. 兵器装备工程学报. 2022(09): 309-314 .  百度学术
百度学术10. 高伟亮,孙桂娟,杨建超,金栋梁,吴飚,邓国强. 国外钻地武器侵彻试验用弹等效模拟技术研究. 防护工程. 2022(05): 21-25 .  百度学术
百度学术11. 徐柳云,张元迪. 爆炸载荷作用下混凝土靶板动态响应的细观模拟. 爆炸与冲击. 2022(12): 37-51 .  本站查看
本站查看12. 米振国,石云波,张婕,滑志成,都捷豪. 侵彻效应混凝土靶HJC本构模型研究. 中国测试. 2021(07): 31-35 .  百度学术
百度学术13. 王安宝,邓国强,杨秀敏,任王军,周布奎,张磊,张想柏. 一个新的通用型侵彻深度计算公式. 土木工程学报. 2021(10): 36-46 .  百度学术
百度学术14. 吴飚,任辉启,陈力,杨建超,黄家蓉,高伟亮,金栋梁. 弹体侵彻混凝土尺度效应试验研究与理论分析. 防护工程. 2020(02): 1-10 .  百度学术
百度学术其他类型引用(2)
-











 下载:
下载:

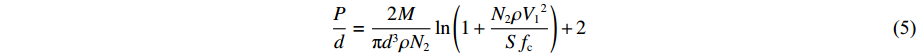













 下载:
下载:





 百度学术
百度学术









