A high-strain-rate shear testing method based on the DIHPB technique
-
摘要: 在测试材料动态力学性能时,直接撞击式霍布金森压杆(direct impact Hopkinson pressure bar,DIHPB)实验系统相对于分离式霍布金森压杆(split Hopkinson pressure bar,SHPB),往往能获得更高的应变率。本文中采用一种新型双剪切试样,在DIHPB系统下对603钢进行了动态剪切测试。获得了603钢在应变率1 500~33 000 s−1的剪应力-剪应变曲线,并与SHPB系统下的测试结果进行了对比。结果表明,由两种测试方法获得的流动应力具有较好的一致性,但曲线的上升沿存在明显区别。采用数值模拟对DIHPB方法的准确性进行了验证,并对该实验方法的适用条件进行了分析。采用DIHPB方法,可以观察到603钢的流动应力存在明显的应变率效应,但在较高的加载速度下材料的失效应力随着加载速度的增加而呈降低趋势。
-
关键词:
- 直接撞击式霍布金森压杆(DIHPB) /
- 分离式霍布金森压杆(SHPB) /
- 高应变率 /
- 动态剪切
Abstract: Compared to the split Hopkinson pressure bar (SHPB) technique, the direct impact Hopkinson pressure bar (DIHPB) method can usually obtain a higher strain rate in dynamic tests of material properties. Based on the DIHPB system, a new double shear specimen was used to measure the shear stress-shear strain curves of 603 steel at strain rates ranging from 1 500 s−1 to 33 000 s−1. Through comparison with the testing results achieved in a SHPB system, it is found that the flow stresses determined by the two methods have a good consistency, but a difference exists in the rising parts of the flow stress curves. Numerical simulation was carried out to validate the DIHPB method, and the proper testing condition of this method was analyzed. With this method, it was observed that the flow stress of 603 steel showed an obvious strain rate effect. At higher loading speeds, however, the failure stress of the material presented a decreasing tendency with the increase of the loading speed. -
金属材料在受到爆炸、冲击、侵彻及高速切削等高应变率载荷时,往往处于剪切主导的应力状态,并进而引发失效。在上述过程中,材料的剪应变率有时可以达到105 s−1。因此,对金属材料在高应变率下剪切力学性能及失效机理的研究,具有重要的科学意义及工程价值[1-3]。
为研究材料的动态剪切性能,Baker等[4]首次提出了Hopkinson扭杆装置。采用该方法,可以获得材料在动态加载下的剪应力-剪应变曲线,剪应变率一般在103 s−1量级[5-7]。此外,还可以采用分离式霍布金森压杆(SHPB)技术对不同形状的试样进行加载,使其局部发生剪切变形,从而获得材料的动态剪切性能。例如,Hartmann等[8]提出的帽型试样被广泛用于材料绝热剪切带的测试研究[9-11]。但是,采用该试样很难获得均匀变形场和纯剪切的应力状态,因此难以准确测得材料的剪应力-剪应变曲线[12]。Rusinek等[13]利用改进的SHPB实验装置对双剪切试样进行了动态剪切测试,但是需要通过数值模拟对所测的参数进行修正。Rittle等[14]利用预制刻槽的圆柱试样,对材料在压缩-剪切状态下的动态性能进行测试。Guo等[15]采用改进的微型压杆装置,对双剪切试样进行加载,获得材料的剪切性能。近年,许泽建等[16-17]提出了一种新型双剪切试样,可以在传统SHPB装置下获得104 s−1以上的剪应变率,对材料在剪切载荷下的塑性流动及失效行为[18-19]进行研究。
直撞式霍布金森压杆(DIHPB)技术在传统的SHPB中,去掉入射杆而使子弹直接撞击试样,从而避免入射杆弹性对子弹速度的限制以获得更高的应变率。例如:Gorham[20]利用DIHPB系统,测量了铜在105 s−1应变率下的压缩应力-应变曲线;Dharan等[21]和Zhao[22]分别采用该方法对铝和混凝土类材料的压缩特性进行了研究;陶俊林等[23-24]对DIHPB方法进行了数值模拟及理论分析。本文中,基于近期提出的新型双剪切试样,采用DIHPB技术对603钢进行高应变率下的动态剪切测试,并与SHPB实验结果进行对比。采用数值模拟验证该方法的准确性,并对该方法的适用条件进行分析。
1. 实验方法
实验材料为603装甲钢,所采用的双剪切试样及配套夹具见图1。双剪切试样具有一个加载端和两个支撑端,他们之间是两个对称的剪切区,本文中使用的剪切区宽度为0.5 mm。
实验所用DIHPB系统包括子弹、透射杆和能量吸收装置。子弹长度为50和20 mm,透射杆长度为500 mm,子弹和透射杆直径均为19 mm;子弹材料为18Ni钢,透射杆材料为7075铝合金,透射杆上的应变信号由应变片测得。实验中,采用激光测速装置对子弹初速度进行测量。先通过标尺对电压和位移的对应关系进行标定,再根据子弹经过时的电压曲线计算子弹的初速度。由测试结果可知,子弹在接触试样前后的速度变化较小,因此可近似认为子弹在撞击过程的速度为恒定值。该方法的实验原理如图2所示。
对于DIHPB系统,要求在加载过程中子弹和透射杆均只发生弹性变形。本文中,假设子弹速度恒定且试样两端载荷相等。若子弹入射速度为v0,透射杆与试样接触面的弹性位移为u2,取v0方向为正,则试样的剪应变
γs(t) 、剪应力τs(t) 及剪应变率˙γs(t) 分别为:γs(t)=(v0t−u2)/l0 (1) ˙γs(t)=(v0−˙u2)/l0 (2) τs(t)=σT(t)AT/2As (3) σT(t)=ETεT(t) (4) 式中:
l0 为试样剪切区宽度,˙u2 为透射杆与试样接触面的速度,ET 为透射杆弹性模量,σT 和εT 分别为透射杆上测得的应力和应变,AT 为透射杆截面积,As 为试样剪切区截面积。根据一维应力波理论,有:
u2(t)=∫t0˙u2dδ=1ρTcT∫t0σT(δ)dδ=ETρTcT∫t0εT(δ)dδ=cT∫t0εT(δ)dδ (5) 式中:
ρT 、cT 分别为透射杆的密度和弹性波速。由式(1)~(5)可知,试样的剪应变率、剪应变和剪应力分别为:
˙γs(t)=[v0−cTεT(t)]/l0 (6) γs(t)=[v0t−cT∫t0εT(δ)dδ]/l0 (7) τs(t)=σTAT/(2As)=ETεTAT/(2As) (8) 因此,根据式(6)~(8),可由实验测得的透射应变信号和子弹初速度,得到试样的剪应力-剪应变曲线。
由于603钢屈服应力约为800 MPa,密度ρs为7.8 g/cm3,弹性波速cs为5 189 m/s。根据假设,由
σ=ρscsvc ,可知603钢试样加载端发生屈服的临界子弹速度vc约为19.77 m/s。当撞击速度超过该值时,试样的加载端会发生塑性变形,此时由式(7)计算得到的剪应变会存在一定误差。因此,采用上述方法测试材料的剪应力-剪应变曲线时,子弹速度不应高于临界速度vc。2. 实验结果
分别在不同子弹速度下进行了冲击剪切测试,实验前后的典型试样形貌见图3。图中对比了未加载及在9.52和72.79 m/s速度下加载后的试样。在两种加载速度下试样剪切区均发生断裂;在9.52 m/s加载速度下,试样加载端未发生塑性变形,但在72.79 m/s速度下试样的加载端发生了明显的塑性变形。图4为在4种加载速度下由透射杆上的应变片测得的应变曲线。只有1.96 m/s速度下试样未发断裂,因此该速度下得到的透射应变脉宽明显大于其他曲线。此外,随着子弹速度的增加透射应变的脉宽逐渐减少,这说明随着加载速度的增大,试样发生断裂的时间逐渐缩短。在以上速度下获得的603钢剪应力-剪应变曲线见图5。
由图5可知,603钢的流动应力存在明显的应变率效应。随着应变率的增加,实验曲线幅值逐渐升高。当应变率为1 500 s−1时,剪应力-剪应变曲线的流动应力段较短,稳定段幅值约为642 MPa。当应变率为16 000 s−1时,曲线初始段出现明显的尖峰,但此后的流动段较稳定,幅值约为730 MPa。曲线初始段的峰值可能是由压杆端部的横向惯性效应而引起的波形弥散,该现象随着应变率的增加而趋于明显。当应变率超过28 000 s−1时,曲线没有明显的流动段而只有两个峰值,且第2个峰值明显较低。结合实验观测可知,第2个波峰由试样的失效造成,即材料在该处发生剪切破坏,因此剪应力此后呈现迅速下降趋势。此外,随着加载速度的提高,曲线上升沿的斜率逐渐减小。这是由于,在较高的加载速度下材料在初始阶段的变形速度也相应较高,因此材料的剪应力在到达第1个峰值时的应变值也相对较大。
为了验证DIHPB系统的准确性,使用相同的双剪切试样在SHPB系统下测得近似应变率下的剪应力-剪应变曲线,并与DIHPB结果进行对比,如图6所示。在应变率接近16 000 s−1时,由SHPB和DIHPB系统测得的剪应力-剪应变曲线在流动段吻合较好,但SHPB曲线的上升沿斜率大于DIHPB曲线。在剪应变率接近30 000 s−1时,两条曲线均存在两个波峰,而且峰值较接近,但DIHPB曲线的峰值明显偏后于SHPB曲线。为分析该现象,将不同测试系统下获得的应变率曲线进行对比(见图7)。可以看出,DIHPB方法在加载过程中剪应变率先由大变小并趋于稳定,而SHPB方法中剪应变率由零上升至峰值并趋于稳定,即初始阶段DIHPB方法获得的剪应变较大,这就导致DIHPB曲线上升沿斜率明显小于SHPB曲线。但是由图7可知,由SHPB和DIHPB方法获得的应变率曲线均能达到稳定阶段,说明两种方法下均能获得较恒定的剪应变率。另外,当只考虑材料的塑性流动阶段时,两种方法所测得的结果具有较好的一致性。
在更高的加载速度下获得的剪应力曲线与17.80 m/s下的曲线对比,如图8所示。在17.80 m/s加载速度下,剪应力曲线仍然存在两个峰值;当加载速度分别提高到52.37和72.79 m/s时,试样加载端已出现塑性变形,可观察到两条曲线均只有1个峰值。这是由于,在较高的撞击速度下,材料在加载波的上升沿即发生失效。而且由于,随着应变率的增加材料内的绝热温升现象更加明显,导致剪应力峰值随着加载速度的增加而逐渐减小。同时可知,在较高的加载速度下,由于试样迅速失效(<20 μs),因此加载过程并未达到恒定的应变率;另外,此时试样内部的应力分布尚未均匀,试样两端难以达到受力平衡,因此由式(8)得到的剪应力也存在误差。由以上分析可知,在采用DIHPB方法对该双剪切试样进行加载时,当加载速度超过临界速度以后,很难获得准确的材料参数,必须借助数值模拟对受力情况进行具体分析。
3. 数值模拟
为进一步验证实验结果的准确性,采用有限元软件ABAQUS/Explicit对DIHPB实验过程进行了数值模拟。模拟采用三维实体模型,包括子弹、试样、夹具及透射杆,模型的几何尺寸、位置关系及接触情况与实际情况一致。通过对子弹施加实测的预定义速度对试样进行加载,各接触面的接触关系均为“硬”接触。子弹、夹具和透射杆均采用C3DR8六面体缩减积分单元;考虑到试样发生剪切变形时存在明显的局部温升,因此试样采用C3D10MT四面体位移温度耦合单元,同时对试样剪切区的网格进行加密处理,见图9。试样的预定义温度场为298 K。
由于撞击杆不发生塑性变形,因此定义为弹性材料;试样和透射杆则采用Johnson-Cook热黏塑性本构模型,本构关系如下:
σ=(A+Bεn)(1+Cln˙ε∗)(1−T∗m) (9) T∗=(T−Tr)(Tm−Tr) (10) 式中:A、B、C、n和m为材料常数,
˙ε∗=˙ε/˙ε0 无量纲塑性应变率,ε为塑性应变,Tm为材料熔化温度,Tr为参考温度。模拟所用到的材料本构参数见表1,各材料物理参数见表2。表 1 模拟中的材料本构Table 1. Material constants for Johnson-Cook model材料 A/MPa B/MPa C n m ˙ε0/s−1 Tm/K Tr/K 603 钢 1 276.1 262.7 0.009 43 0.061 6 0.584 69 1 1 723 288 7075 铝合金 503 303.58 0.97 0.39 0.77 1 600 298 表 2 模拟中的材料物理参数Table 2. Material parameters used in finite element simulation部位 材料 ρ/(g⋅cm−3) E/GPa ν λ/(W·m−1·K−1) c/(J·kg−1·K−1) 入射杆 18 镍钢 8.0 190 0.3 试样 603 钢 7.8 210 0.3 45 480 夹具 高强钢 7.8 210 0.3 透射杆 7075 铝合金 2.7 70 0.3 为了验证在实验过程中试样两端面的受力平衡,在模拟结果中输出撞击杆与透射杆端面的受力曲线,如图10所示。可以看出,在不同加载速度条件下,试样两端受力过程接近平衡。在17.80 m/s时,加载的初始阶段由于试样中的应力波尚未达到均匀传播,因此导致两端的受力存在一定差别,随着应力波多次反射试样两端的受力逐渐趋于平衡。
透射杆中应变信号的模拟与实验结果对比见图11,模拟与实验曲线的幅值吻合较好。两条曲线都具有两个明显的峰值,实验曲线在第2个峰值以后迅速降为零,这是由于试样在实验过程发生断裂而导致的;由于在模拟中并未考虑材料的失效,因此模拟曲线未出现迅速下降。将实验测得的剪应力、剪应变转化为等效应力和等效应变:
σeq=√3τ (11) εeq=ln√1+γ+γ2/2 (12) 并比较实验和模拟得到的剪应力-剪应变曲线和等效应力-等效应变曲线,如图12所示。由于实验结果是由透射杆上测得的应变信号得到的,因此实验曲线存在由应力波的传播而引发的波动;而模拟曲线是由试样剪切区所有单元计算结果的平均值获得的,因而表现出较平滑的特征。但是,由模拟得到的剪应力-剪应变曲线和等效应力-等效应变曲线的幅值均与实验结果吻合较好,说明DIHPB系统测得的动态剪应力-剪应变曲线具有较好的准确性。
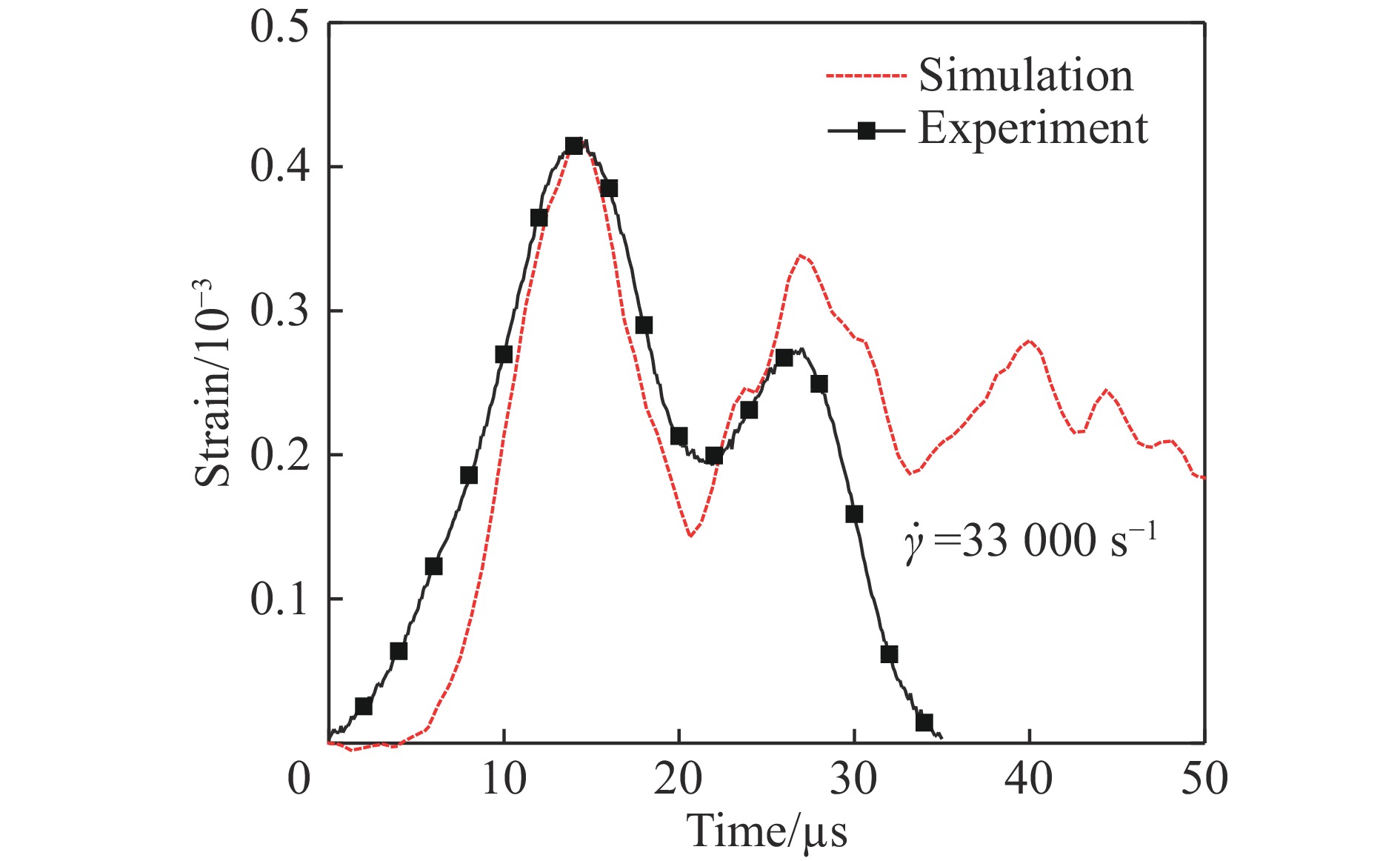
当子弹速度超过临界加载速度时,由于试样加载端将发生塑性变形,因此试样的实际响应必须通过数值模拟获得。例如,当子弹速度为72 m/s时,对实测结果和模拟得到的透射杆应变信号的第1个波峰进行对比,如图13所示。由图13可知:两曲线在前期吻合较好,且出现峰值的时刻较一致;但在约11 μs时两曲线发生分离,且模拟结果大于实测结果。这可能是由于,在实际加载下材料在高速剪切过程中产生了绝热剪切带或微裂纹等形式的损伤,但模拟中并未考虑材料的损伤及失效,从而引起实验曲线低于模拟结果。
4. 结 论
采用DIHPB加载技术对新型双剪切试样进行了冲击加载,获得了603钢在高应变率下的动态剪切特性。通过与SHPB测试结果及有限元模拟结果的对比分析,验证了该方法的有效性。主要结论如下。
(1)基于DIHPB系统测试方法,使用近年来提出的新型双剪切试样得到了603钢在剪应变率1 500~33 000 s−1之间的剪应力-剪应变曲线。通过SHPB对比试验,发现两种方法下均能获得较恒定的剪应变率,但流动应力曲线的上升沿存在差别。当只考虑材料的塑性流动阶段时,两种方法所测得的结果具有较好的一致性。
(2)采用ABAQUS/Explicit对DIHPB方法的加载过程进行了模拟。通过与实验结果进行对比,发现两者的透射应变信号及应力-应变曲线均吻合较好,且试样两端满足载荷平衡条件,进一步验证了DIHPB加载方法的可靠性。
(3)采用DIHPB方法可以观察到:603钢的流动应力存在明显的应变率强化效应,即随着应变率的增加而逐渐增加;但在较高的加载速度下,材料的失效应力随着加载速度的增加而出现降低趋势。
(4)使用DIHPB系统对新型双剪切试样进行加载时应注意:子弹速度应低于临界速度,否则由式(7)得到的剪应变会存在一定误差;当子弹速度超过临界速度,试样两端的受力情况也趋于复杂,可能引起试样的受力平衡条件不再满足,因此由式(8)得到的剪应力也存在误差。
-
表 1 模拟中的材料本构
Table 1. Material constants for Johnson-Cook model
材料 A/MPa B/MPa C n m ˙ε0/s−1 Tm/K Tr/K 603 钢 1 276.1 262.7 0.009 43 0.061 6 0.584 69 1 1 723 288 7075 铝合金 503 303.58 0.97 0.39 0.77 1 600 298 表 2 模拟中的材料物理参数
Table 2. Material parameters used in finite element simulation
部位 材料 ρ/(g⋅cm−3) E/GPa ν λ/(W·m−1·K−1) c/(J·kg−1·K−1) 入射杆 18 镍钢 8.0 190 0.3 试样 603 钢 7.8 210 0.3 45 480 夹具 高强钢 7.8 210 0.3 透射杆 7075 铝合金 2.7 70 0.3 -
[1] LIAO S C, DUFFY J. Adiabatic shear bands in a Ti-6Al-4V titanium alloy [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(11): 2201–2231. DOI: 10.1016/S0022-5096(98)00044-1. [2] RITTEL D, WANG Z G. Thermo-mechanical aspects of adiabatic shear failure of AM50 and Ti-6Al-4V alloys [J]. Mechanics of Materials, 2008, 40(8): 629–635. DOI: 10.1016/j.mechmat.2008.03.002. [3] PEIRS J, VERLEYSEN P, DEGRIECK J, et al. The use of hat-shaped specimens to study the high strain rate shear behaviour of Ti-6Al-4V [J]. International Journal of Impact Engineering, 2010, 37(6): 703–714. DOI: 10.1016/j.ijimpeng.2009.08.002. [4] BAKER W E, YEW C H. Strain-rate effects in the propagation of torsional plastic waves [J]. Journal of Applied Mechanics, 1966, 33(4): 917–923. DOI: 10.1115/1.3625202. [5] BAI Y L, XUE Q, Xu Y, SHEN L. Characteristics and microstructure in the evolution of shear localization in Ti-6Al-4V [J]. Mechanics of Materials, 1994, 17(2/3): 155–64. DOI: 10.1016/0167-6636(94)90056-6. [6] DUFFY J, CAMPBELL J D, HAWLEY R H. On the use of a torsional split Hopkinson bar to study rate effects in 1100-0 aluminum [J]. Journal of Applied Mechanics, 1971, 38(1): 83–91. DOI: 10.1115/1.3408771. [7] CAMPBELL J D, ELEICHE A M, TSAO M C C. Strength of metals and alloys at high strains and strain rates [C] // JAFFEE R I, WILCOX B A. Fundamental Aspects of Structural Alloy Design. Boston, MA: Springer, 1977: 545−563. DOI: 110.1007/978-1-4684-2421-8_19. [8] HARTMANN K H, KUNZE H D, MEYER L W. Metallurgical effects on impact loaded materials [C] // MEYERS M A, MURR L E. Shock Waves and High-Strain-Rate Phenomena in Metals. Boston, MA: Springer, 1981: 325−337. DOI: 10.1007/978-1-4613-3219-0_21. [9] MINNAAR K, ZHOU M. An analysis of the dynamic shear failure resistance of structural metals [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(10): 2155–2170. DOI: 10.1016/S0022-5096(98)00020-9. [10] PURSCHE F, MEYER L W. Correlation between dynamic material behavior and adiabatic shear phenomenon for quenched and tempered steels [J]. Engineering Transactions, 2011, 59(2): 67–84. [11] MURR L E, STAUDHAMMER K P, MEYERS M A. Metallurgical applications of shock-wave and high-strain-rate phenomena[M]. New York: Marcel Dekker, 1986. [12] MEYER L W, PURSCHE F. Experimental methods [C] // DODD B, BAI Y. Adiabatic Shear Localization: Frontiers and Advances. London: Elsevier, 2012. [13] RUSINEK A, KLEPACZKO J R. Shear testing of a sheet steel at wide range of strain rates and a constitutive relation with strain-rate and temperature dependence of the flow stress [J]. International Journal of Plasticity, 2001, 17(1): 87–115. DOI: 10.1016/S0749-6419(00)00020-6. [14] RITTEL D, LEE S, RAVICHANDRAN G. A shear-compression specimen for large strain testing [J]. Experiment Mechanics, 2002, 42(1): 58–64. DOI: 10.1007/BF02411052. [15] GUO Y, LI Y. A novel approach to testing the dynamic shear response of Ti-6Al-4V [J]. Acta Mechanica Solida Sinica, 2012, 25(3): 299–311. DOI: 10.1016/S0894-9166(12)60027-5. [16] 许泽建, 丁晓燕, 张炜琪, 等. 一种用于材料高应变率剪切性能测试的新型加载技术 [J]. 力学学报, 2016, 48(3): 654–659. DOI: 10.6052/0459-1879-15-445.XU Zejian, DING Xiaoyan, ZHANG Weiqi, et al. A new loading technique for measuring shearing properties of materials under high strain rates [J]. Chinese Journal of Theoretical and Applied Mechanics, 2016, 48(3): 654–659. DOI: 10.6052/0459-1879-15-445. [17] XU Zejian. A novel method in dynamic shear testing of bulk materials using the traditional SHPB technique [J]. International Journal of Impact Engineering, 2017, 101: 90–104. DOI: 10.1016/j.ijimpeng.2016.11.012. [18] XU Zejian. On shear failure behaviors of an armor steel over a large range of strain rates [J]. International Journal of Impact Engineering, 2018, 118: 24–28. DOI: 10.1016/j.ijimpeng.2018.04.003. [19] 张炜琪, 许泽建, 孙中岳, 等. Ti-6Al-4V在高应变率下的动态剪切特性及失效机理 [J]. 爆炸与冲击, 2018, 38(5): 1137–1144. DOI: 10.11883/bzycj-2017-0107.ZHANG Weiqi, XU Zejian, SUN Zhongyue, et al. Dynamic shear behavior and failure mechanism of Ti-6Al-4V at high strain rates [J]. Explosion and Shock Waves, 2018, 38(5): 1137–1144. DOI: 10.11883/bzycj-2017-0107. [20] GORHAM D A. Measurement of stress-strain properties of strong metals at very high strainrates [C] // HARDING J. Mechanical properites at high rates of strain.1979: 16−24. [21] DHARAN C K H, HAUSER F E. Determination of stress-strain characteristics at very high strain rates [J]. Experimental Mechanics, 1970, 10(9): 370–376. DOI: 10.1007/BF02320419. [22] ZHAO Han. A study on testing techniques for concrete-like materials under compressive impact loading [J]. Cement and Concrete Composites, 1998, 20(4): 293–299. DOI: 10.1016/S0958-9465(98)00008-0. [23] 陶俊林, 陈裕泽, 陈刚, 等.直接撞击Hopkinson压杆系统数值模拟[J].固体力学学报, 2003, 24(S): 198−203.TAO Junlin, CHEN Yuze, CHEN Gan, et al. Numerical simulation of direct impact Hopkinson pressure bar system[J]. Acta Mechanica Solida Sinica, 2003, 24(S): 198−203. [24] 陶俊林. 直接撞击Hopkinson实验技术讨论 [C] // 中国科学技术大学冲击动力学实验室.第三届全国爆炸力学实验技术交流会论文集. 2004: 11−23. 期刊类型引用(2)
1. 杜雨田,许泽建,虎宏智,黄风雷. 金属材料动态剪切测试方法. 兵工学报. 2023(05): 1502-1512 .  百度学术
百度学术2. 秦彩芳,许泽建,窦旺,杜雨田,黄风雷. 金属材料在复杂应力状态下的塑性流动特性及本构模型. 爆炸与冲击. 2022(09): 79-89 .  本站查看
本站查看其他类型引用(3)
-








 下载:
下载:








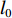


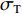



























 下载:
下载:











 百度学术
百度学术











