Intelligent collaborative optimization of structural parameters for hook-sheet specimens used in split Hopkinson tensile bar
-
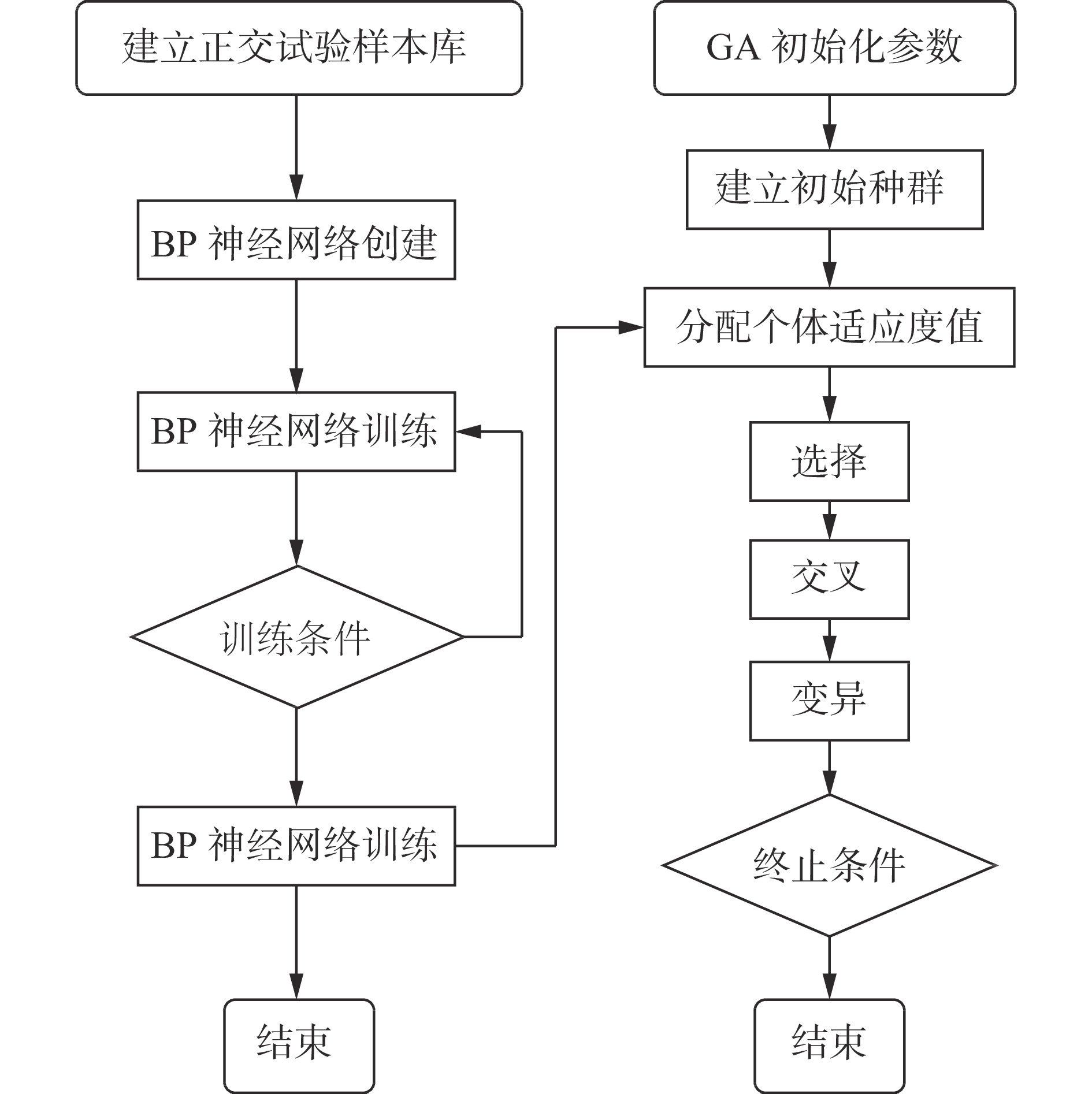
摘要: 与霍普金森拉杆装置中常用的螺纹、胶粘等固定连接方式相比,平板挂钩试件具有连接形式简单、可实现快速组装等优势。针对平板挂钩试件在拉伸过程中因结构几何效应引起的数据测量误差问题,基于影响拉伸试件测量精度的指标:应力平衡达到时间、变形均匀程度、过渡段相对变形以及非轴向力水平,采用正交试验设计、反向传播(back propagation,BP)神经网络与遗传算法相结合的多目标智能协同优化算法对平板挂钩试件的结构参数进行优化,得到了平板挂钩试件最优的结构参数组合,有限元模拟和实验验证了最优结构参数的有效性。该研究结果可为基于平板挂钩试件的霍普金森拉伸实验的数据可靠性分析提供参考。Abstract: Compared with the fixed connection methods such as thread and adhesive commonly used in the split Hopkinson tensile bar experiments, the hook-sheet specimen has the advantages of simple connection form and quick assembly process. Aiming at measurement uncertainty caused by structural geometric effect of the hook-sheet specimen during the stretching process, based on the indicators for measurement accuracy of hook-sheet specimen, such as response of stress equilibrium, deformation uniformity, relative deformation of the transition zones and non-axial stress level, this paper adopted the multi-objective intelligent collaborative optimization algorithm which comprises orthogonal experimental design, back propagation (BP) neural network and genetic algorithm to optimize the structural parameters of hook-sheet specimen. The optimal structural parameters for hook-sheet specimen is thus obtained and the validity of the optimal structural parameters is verified by finite element simulations and experiments. The results provide a reference for data reliability analysis of split Hopkinson tensile bar experiments based on hook-joint sheet specimen.
-
表 1 试件各段变形量及过渡段相对变形
Table 1. The deformation of each section of the specimen and the relative deformation of the transition zone
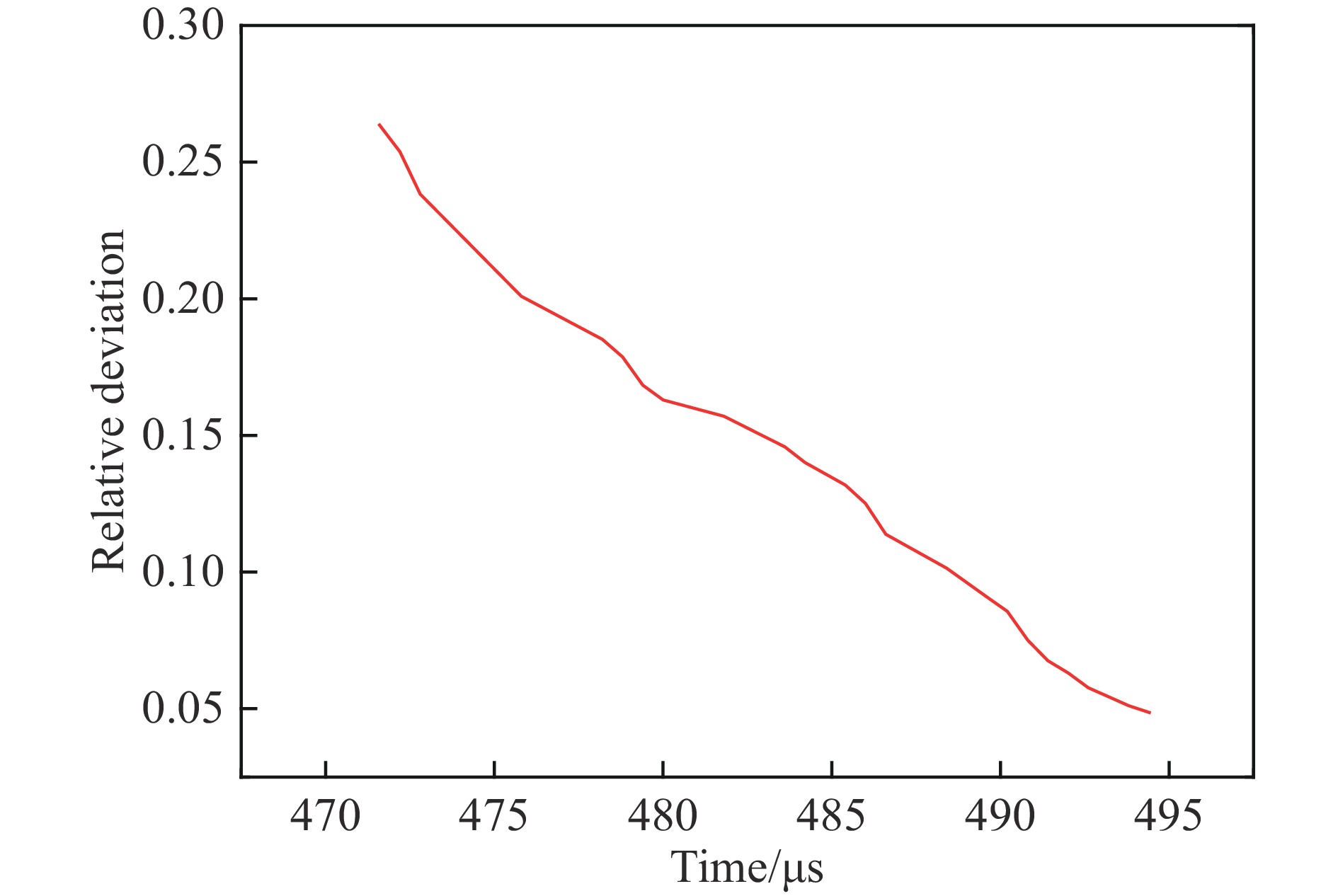
前过渡段变形量 标距段变形量 后过渡段变形量 过渡段相对变形 0.20 mm 1.62 mm 0.21 mm 20.20% 表 2 结构参数正交试验设计表
Table 2. Orthogonal test table of structural parameters
试验编号 结构参数(单位:mm) 应力平衡达到时间E/μs 应变方差V/10−3 过渡段相对
变形D非轴向应力
水平NL1 W1 L2 W2 R T 01 6 2 12 3 0.5 0.6 18.00 0.470 5 0.051 7 0.038 1 02 6 3 13 4 1 0.9 18.00 0.442 9 0.136 0 0.042 1 03 6 4 14 5 2 1.2 18.61 0.566 7 0.249 9 0.049 7 04 6 6 15 6 2.5 1.5 18.85 0.571 3 0.309 1 0.056 6 05 6 8 16 7 3 1.8 20.80 0.525 6 0.351 4 0.081 5 06 7 2 13 5 2.5 1.8 21.45 0.139 6 0.221 9 0.021 4 07 7 3 14 6 3 0.6 21.00 0.195 4 0.283 1 0.023 8 08 7 4 15 7 0.5 0.9 21.00 0.879 6 0.014 9 0.075 0 09 7 6 16 3 1 1.2 21.50 0.950 6 0.114 0 0.082 9 10 7 8 12 4 2 1.5 22.00 0.805 5 0.230 2 0.081 0 11 8 2 14 7 1 1.5 22.50 0.228 9 0.074 0 0.032 9 12 8 3 15 3 2 1.8 23.00 0.235 8 0.196 7 0.030 0 13 8 4 16 4 2.5 0.6 22.79 0.281 7 0.244 5 0.032 9 14 8 6 12 5 3 0.9 22.81 0.371 7 0.287 4 0.046 2 15 8 8 13 6 0.5 1.2 22.81 1.593 2 0.074 6 0.125 3 16 9 2 15 4 3 1.2 24.00 0.085 9 0.196 9 0.011 0 17 9 3 16 5 0.5 1.5 23.50 0.774 2 0.034 6 0.048 7 18 9 4 12 6 1 1.8 24.00 0.317 8 0.061 0 0.044 9 19 9 6 13 7 2 0.6 23.41 0.472 1 0.194 6 0.050 4 20 9 8 14 3 2.5 0.9 24.00 0.607 0 0.230 4 0.060 7 21 10 2 16 6 2 0.9 25.20 0.154 7 0.138 7 0.013 5 22 10 3 12 7 2.5 1.2 25.20 0.192 6 0.191 7 0.019 4 23 10 4 13 3 3 1.5 25.00 0.211 6 0.224 4 0.022 7 24 10 6 14 4 0.5 1.8 25.01 1.442 4 0.027 0 0.086 6 25 10 8 15 5 1 0.6 24.00 1.117 4 0.096 9 0.088 8 表 3 测试样本数据
Table 3. The data of test samples
测试试验 结构参数(单位:mm) 应力平衡达到时间E/μs 应变方差V/10−3 过渡段相对
变形D非轴向应力
水平NL1 W1 L2 W2 R T 01 6 3 14 6 1 1.5 18.77 0.285 4 0.119 2 0.047 7 02 8 2 12 5 2 1.2 22.68 0.203 5 0.162 4 0.018 7 03 10 4 16 7 2.5 0.9 25.12 0.276 9 0.205 3 0.029 0 表 4 各项指标及目标值网络预测与实际情况的比较
Table 4. The comparison of predicted and actual values of indicators and objective function
指标/目标值 1 2 3 实际值 预测值 误差/% 实际值 预测值 误差/% 实际值 预测值 误差/% 应力平达到衡时间 E/μs 18.77 17.89 4.7 22.68 20.15 11.2 25.12 27.45 9.2 应变方差 V/10−3 0.285 4 0.263 3 7.7 0.203 5 0.185 7 8.7 0.276 9 0.299 4 8.1 过渡段相对变形 D 0.119 2 0.104 0 12.8 0.162 4 0.163 8 0.9 0.205 3 0.210 7 2.6 非轴向应力水平 N 0.047 7 0.050 1 5.0 0.018 7 0.020 8 11.2 0.029 0 0.029 5 1.7 目标函数值 Obj 3.009 9 2.887 7 4.1 2.716 7 2.620 6 3.5 3.417 3 3.604 6 5.5 表 5 优化试件各段变形量及过渡段相对变形
Table 5. The deformation of each section of the optimized specimen and the relative deformation of the transition zone
前过渡段变形量 标距段变形量 后过渡段变形量 过渡段相对变形 0.16 mm 2.03 mm 0.16 mm 13.62% 表 6 优化前后各项指标的比较
Table 6. The comparison of various indicators before and after the optimization
指标 优化前 优化后 增大(↑)或降低(↓) 应力平衡达到时间E/μs 22.81 24.75 8.51%↑ 应变方差V/10−3 0.598 8 0.135 1 77.44%↓ 过渡段相对变形 D 0.202 0 0.136 2 32.57%↓ 非轴向应力水平 N 0.056 3 0.018 8 66.61%↓ 表 7 各段变形值实验与计算的比较
Table 7. Comparison of deformation of transition zones between simulations and experiments
变形段 计算值 实验值 相对偏差 前过渡段 0.16 mm 0.13 mm 18.75% 后过渡段 0.16 mm 0.15 mm 6.25% 标距段 2.03 mm 2.12 mm 4.43% 相对变形 13.62% 11.67% 14.32% 表 8 参考试件与优化试件数值模拟和实验结果的对比
Table 8. The comparison of simulated and experimental results between reference specimen and optimized specimen
指标 参考试件 优化试件 计算 实验 相对偏差 计算 实验 相对偏差 过渡段相对变形/% 20.20 22.31 10.45% 13.62 11.67 14.32% 标距段变形均匀度/10−3 0.598 8 0.544 6 9.05% 0.135 1 0.145 5 7.70% -
[1] KOLSKY H. An investigation of the mechanical properties of material at very high rates of loading [J]. Proceedings of the Physical Society: Section B, 1949, 62(1): 676–700. [2] HARDING J, WOOD E O, CAMPBELL J D. Tensile testing of materials at impact rates of strain [J]. Journal of Mechanical Engineering Science, 1960, 2(2): 88–96. DOI: 10.1243/JMES_JOUR_1960_002_016_02. [3] OGAWA K. Impact-tension compression test by using a split Hopkinson bar [J]. Experimental Mechanics, 1984, 24(2): 81–86. DOI: 10.1007/BF02324987. [4] 申海艇, 蒋招绣, 王贝壳, 等. 基于超高速相机的数字图像相关性全场应变分析在SHTB实验中的应用 [J]. 爆炸与冲击, 2017, 37(1): 15–20. DOI: 10.11883/1001-1455(2017)01-0015-06.SHEN Haiting, JIANG Zhaoxiu, WANG Beike, et al. Full field strain measurement in split Hopkinson tension bar experiments by using ultra-high-speed camera with digital image correlation [J]. Explosion and Shock Waves, 2017, 37(1): 15–20. DOI: 10.11883/1001-1455(2017)01-0015-06. [5] GILAT A, GOLDBERG R K, ROBERTS G D. Experimental study of strain-rate-dependent behavior of carbon/epoxy composite [J]. Composites Science and Technology, 2002, 62(10/11): 1469–1479. DOI: 10.1016/S0266-3538(02)00100-8. [6] CHEN X, LI Y, ZHI Z, et al. The compressive and tensile behavior of a 0/90 C fiber woven composite at high strain rates [J]. Carbon, 2013, 61: 97–104. DOI: 10.1016/j.carbon.2013.04.073. [7] OWENS A T, TIPPUR H V. A tensile split Hopkinson bar for testing particulate polymer composites under elevated rates of loading [J]. Experimental Mechanics, 2008, 49(6): 799–811. DOI: 10.1007/s11340-008-9192-7. [8] 朱耀, 庞宝君, 盖秉政. 一种用于动态拉伸试验装置的新型试件装卡方式 [J]. 实验力学, 2009, 24(5): 433–438. DOI: 1001-4888(2009)05-0433-06.ZHU Yao, PANG Baojun, GAI Bingzheng. A new specimen fastener for dynamic tensile testing apparatus [J]. Journal of Experimental Mechanics, 2009, 24(5): 433–438. DOI: 1001-4888(2009)05-0433-06. [9] TAN X, GUO W, GAO X, et al. A new technique for conducting split Hopkinson tensile bar test at elevated temperatures [J]. Experimental Techniques, 2017, 41(2): 191–201. DOI: 10.1007/s40799-017-0167-4. [10] VERLEYSEN P, DEGRIECK J. Non-homogeneous and multi-axial stress distribution in concrete specimens during split Hopkinson tensile tests [J]. Computers and Structures, 2000, 77(6): 669–676. DOI: 10.1016/S0045-7949(00)00022-5. [11] CHEN W, LU F, CHENG M. Tension and compression tests of two polymers under quasi-static and dynamic loading [J]. Polymer Testing, 2002, 21(2): 113–121. DOI: 10.1016/S0142-9418(01)00055-1. [12] PRABOWO Q A, KARIEM M A, GUNAWAN L. The effect of specimen dimension on the results of the split Hopkinson tension bar testing [J]. Procedia Engineering, 2017, 173: 608–614. DOI: 10.1016/j.proeng.2016.12.114. [13] VERLEYSEN P, DEGRIECK J, VERSTRAETE T, et al. Influence of specimen geometry on split Hopkinson tensile bar test on sheet materials [J]. Experimental Mechanics, 2008, 48(5): 587–598. DOI: 10.1007/s11340-008-9149-x. [14] NGUYEN K H, KIM H C, SHIN H, et al. Numerical investigation into the stress wave transmitting characteristics of threads in the split Hopkinson tensile bar test [J]. International Journal of Impact Engineering, 2017, 109: 253–263. DOI: 10.1016/j.ijimpeng.2017.07.004. [15] 王礼立. 应力波基础[M]. 第2版. 北京: 国防工业出版社, 2005: 52−60. [16] SMERD R, WINKLER S, SALISBURY C, et al. High strain rate tensile testing of automotive aluminum alloy sheet [J]. International Journal of Impact Engineering, 2005, 32(1): 541–560. DOI: 10.1016/j.ijimpeng.2005.04.013. [17] SONG B, CHEN W. Dynamic stress equilibrium in split Hopkinson pressure bar tests on soft materials [J]. Experimental Mechanics, 2004, 44: 300–312. DOI: 10.1007/BF02427897. [18] 施建俊, 李庆亚, 张琪, 等. 基于Matlab和BP神经网络的爆破振动预测系统 [J]. 爆炸与冲击, 2017, 37(6): 1087–1092. DOI: 10.11883/1001-1455(2017)06-1087-06.SHI Jianjun, LI Qingya, ZHANG Qi. Forecast system for blasting vibration velocity peak based on Matlab and BP neural network [J]. Explosion and Shock Waves, 2017, 37(6): 1087–1092. DOI: 10.11883/1001-1455(2017)06-1087-06. [19] WEN J, LIU C H, YAO H, et al. A nonlinear dynamic model and parameters identification method for predicting the shock pulse of rubber waveform generator [J]. International Journal of Impact Engineering, 2018, 120: 1–15. DOI: 10.1016/j.ijimpeng.2018.05.009. [20] 李守巨, 刘迎曦, 刘玉静, 等. 基于遗传算法的爆炸冲击荷载参数识别方法 [J]. 爆炸与冲击, 2002, 22(4): 295–300.LI Shouju, LIU Yingxi, LIU Yujing, et al. Parameter identification procedures of explosion shock loading based on genetic algorithm [J]. Explosion and Shock Waves, 2002, 22(4): 295–300. -








 下载:
下载: