Dynamic response of mesoscopic plain/reinforced concrete slabs under blast loading
-
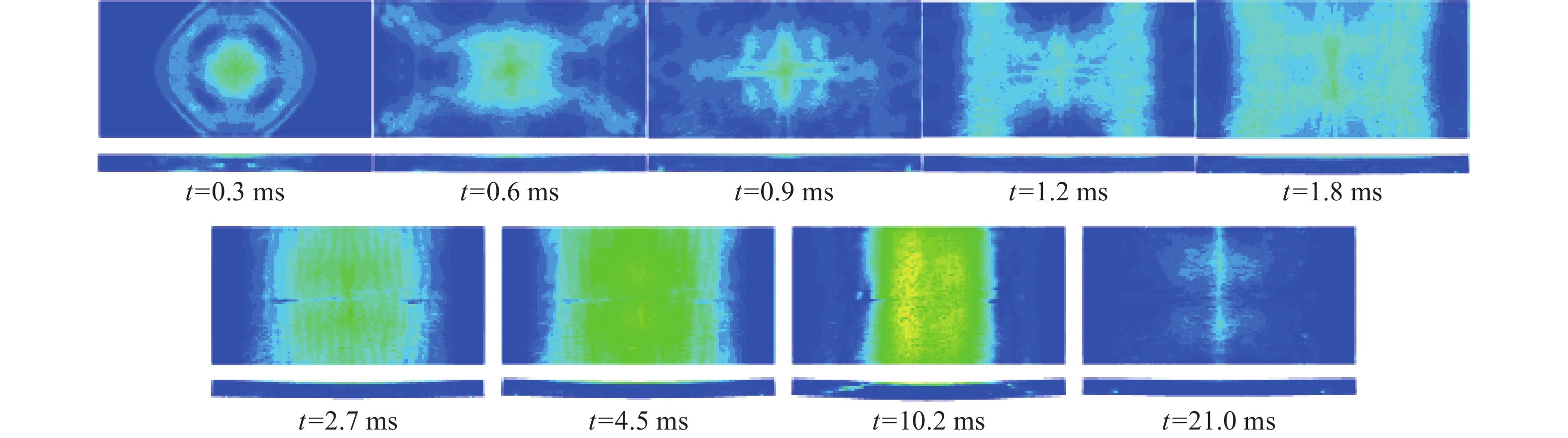
摘要: 为了获得爆炸荷载下细观结构对素/钢筋混凝土板的影响，采用随机骨料投放建立了素/钢筋混凝土板细观模型。利用LS-DYNA对基于细观建模的钢筋混凝土板进行爆炸荷载作用下的数值模拟，通过与实验以及均质建模方法进行比较，验证了细观建模方法的准确性。进而研究了基于细观建模的素/钢筋混凝土板在不同爆炸荷载下的结构响应，获得了素/钢筋混凝土板的响应过程和破坏模式。结果表明：在低药量（1、2 kg）爆炸荷载下，细观结构对素/钢筋混凝土板的影响较小，其破坏模式以纵横塑性铰线破坏为主，药量越大，铰线越多；在高药量（5、10和15 kg）爆炸荷载下，细观结构对素/钢筋混凝土板的影响较大，与均质模型相比存在较大差异，细观素/钢筋混凝土板以爆坑为中心，产生环向与径向裂纹，药量越大，圆坑越大，裂纹越多，板局部破坏越严重。Abstract: In order to obtain the effect of meso-structure on plain/reinforced concrete slabs under explosive loading, a meso-structure model of plain/reinforced concrete slabs with stochastic aggregate method was adopted. LS-DYNA was used for numerical simulation of reinforced concrete slabs based on meso-modeling under explosive loading. The accuracy of the meso-modeling method was verified by comparing with the experimental and homogeneous modeling methods. Furthermore, the structural dynamic response of plain/reinforced concrete slabs based on meso-modeling under different explosive loads was studied, and the response process and failure mode of plain/reinforced concrete slabs were obtained. The results show that the meso-structure has little effect on the plain/reinforced concrete slab under low explosive loading (1 kg and 2 kg). The failure mode is mainly based on the vertical and horizontal plastic hinge damage. The larger the dose, the more the hinge line. Comparatively, the meso-structure has a great influence on the plain/reinforced concrete slab under the high explosive load (5 kg, 10 kg and 15 kg), and there is a big difference compared with the homogeneous model. The plain/reinforced concrete slab is centered on the blasting pit and produces circumferential and radial cracks under high explosive loading (5 kg, 10 kg and 15 kg). The larger the dose, the larger the round pit, the more cracks, the more serious the local damage.
-
Key words:
- blast load /
- mesoscopic model /
- concrete slab /
- structural dynamic response
-
图 6 钢筋混凝土板迎爆面与长边中心剖面等效应力图
Figure 6. Equivalent stress diagrams of front and long side central section of reinforced concrete slab
图 8 2 kg TNT素凝土板迎爆面与长边中心剖面有效应力图
Figure 8. Equivalent stress diagrams of front and long side center section of homogeneous plain concrete slab under 2 kg TNT
图 9 2 kg TNT素凝土板迎爆面与长边方向中心剖面塑性应变图
Figure 9. Plastic strain diagrams of front and long side center section of homogeneous plain concrete slab under 2 kg TNT
图 10 2 kg TNT钢筋混凝土板迎爆面与长边方向中心剖面有效应力图
Figure 10. Equivalent stress diagrams of front and long side center section of mesoscopic reinforced concrete slab under 2 kg TNT
图 12 较大应力区边界距离时程曲线
Figure 12. Time history curve of large stress zone boundary distance
图 14 2 kg TNT钢筋凝土板迎爆面与长边方向中心剖面塑性应变图
Figure 14. Plastic strain diagrams of front and long side center section of mesoscopic reinforced concrete slab under 2 kg TNT
图 15 爆炸荷载下素混凝土板塑性应变图
Figure 15. Plastic strain diagrams of plain concrete slab under explosive loading
图 16 爆炸荷载下钢筋混凝土板塑性应变图
Figure 16. Plastic strain diagrams of reinforced concrete slab under explosive loading
表 1 工况表
Table 1. Working condition details
模型 TNT 药量 W/kg 爆距 R/m 比例距离 Z/（m·kg−1/3） 素/钢筋混凝土 1 0.5 0.500 2 0.5 0.397 5 0.5 0.292 10 0.5 0.232 15 0.5 0.203 
下载: 导出CSV
表 2 混凝土及其细观组分的材料参数与失效判据
Table 2. Material parameters and failure criteria of concrete and its meso-components
模型 材料 ρi/（kg·m−3） νi fti/MPa fci/MPa εimax εimin Rs Uc 均质 混凝土 2 440 0.20 4.8 48 0.008 −0.023 3.94×102 145 细观 砂浆 2 280 0.22 5.7 40 0.011 −0.040 骨料 2 660 0.16 16.0 160 0.010 −0.020 ITZ1 2 000 0.16 3.0 30 0.005 −0.015 ITZ2 2 000 0.16 2.5 25 0.006 −0.018 注：ρi为密度，νi为泊松比，fti为抗拉强度，fci为抗压强度，εimax为最大主应变，εimin为最小主应变，Rs为长度单位转换因子，Uc为应力单位换算系数。
下载: 导出CSV
表 3 钢筋材料参数
Table 3. Steel bar parameters
参数 ρs/(kg·m−3) E/GPa νs σy/MPa Et/GPa C/s−1 Ps Fs 钢筋 7 850 210 0.28 440 4.7 45 5 0.12 注：ρs为密度，E为弹性模量，νs为泊松比，σy为屈服强度，Et为剪切模量，C为应变率参数，Ps为应变率参数，Fs为失效应变。
下载: 导出CSV
-
[1] 张晓伟, 汪庆桃, 张庆明, 等. 爆炸冲击波作用下混凝土板的载荷等效方法 [J]. 兵工学报, 2013, 34(3): 263–268.ZHANG Xiaowei, WANG Qingtao, ZHANG Qingming, et al. Equivalence method for the dynamic loading of concrete slab subjected to explosion [J]. Acta Armamentarii, 2013, 34(3): 263–268. [2] 汪维, 张舵, 卢芳云, 等. 钢筋混凝土楼板在爆炸荷载作用下破坏模式和抗爆性能分析 [J]. 兵工学报, 2010(S1): 102–106.WANG Wei, ZHANG Duo, LU Fangyun, et al. Analysis for blast resistance and damage mode of reinforced concrete slab subjected to explosive load [J]. Acta Armamentarii, 2010(S1): 102–106. [3] WANG W, ZHANG D, LU F, et al. Experimental study on scaling the explosion resistance of a one-way square reinforced concrete slab under a close-in blast loading [J]. International Journal of Impact Engineering, 2012, 49(2): 158–164. [4] STOLZA A, FISCHERA K, ROLLER C. Dynamic bearing capacity of ductile concrete plates under blast loading [J]. International Journal of Impact Engineering, 2014, 69(7): 25–38. [5] 陈万祥, 卢红标, 候小伟, 等. 高强钢筋加强混凝土板抗爆性能试验研究 [J]. 振动与冲击, 2015, 34(10): 135–141.CHEN Wanxiang, LU Hongbiao, HOU Xiaowei, et al. Tests for anti-blast performance of concrete slabs with high-strength reinforcements under blast loading [J]. Journal of Vibration and Shock, 2015, 34(10): 135–141. [6] ALENGARAM U J, MOHOTTIGE N H W, WU C, et al. Response of oil palm shell concrete slabs subjected to quasi-static and blast loads [J]. Construction and Building Materials, 2016, 116: 391–402. [7] LV T H, CHEN X W, CHEN G. The 3D meso-scale model and numerical tests of split Hopkinson pressure bar of concrete specimen [J]. Construction and Building Materials, 2018, 160: 744–764. [8] SCHWER L E, MALVAR L J. Simplified concrete modeling with *MAT_CONCRETE_DAMAGE_REL3 [C] // JRI LS-Dyna User Week, Nagoya, Japan, 2005: 49-60. [9] GOBLE C F, COHEN M D. Influence of aggregate surface area on mechanical properties of mortar [J]. ACI Materials Journal, 1999, 96(6): 657–662. [10] GOPALARATNAM. Softening response of plain concrete in direct tension [J]. ACI Materials Journal, 1985, 82(3): 310–323. [11] 唐春安, 朱万成. 混凝土损伤与断裂[M].北京: 科学出版社, 2003.TANG Chunan, ZHU Wancheng. Concrete damage and fracture [M]. Beijing: Science Press, 2003. [12] CLARK L A. CEB-FIP Model Code 1990 [J]. Programs Usenix Unix Supplementary Documents, 2008, 40(95): 233–235. [13] 龚顺风, 金伟良, 何勇. 内部爆炸荷载作用下钢筋混凝土板的动力响应研究 [J]. 振动工程学报, 2008, 21(5): 516–520. DOI: 10.3969/j.issn.1004-4523.2008.05.016.GONG Shunfeng, JIN Weiliang, HE Yong. Dynamic response of reinforced concrete slab subjected to internal blast loading [J]. Journal of Vibration Engineering, 2008, 21(5): 516–520. DOI: 10.3969/j.issn.1004-4523.2008.05.016. [14] 龚顺风, 金伟良. 内部爆炸荷载作用下钢筋混凝土板碎片抛射速度的预测 [J]. 工程力学, 2009, 26(9): 225–230.GONG Shunfeng, JIN Weiliang. Prediction of debris launch velocity of reinforced concrete slab subjected to internal blast loading [J]. Engineering Mechanics, 2009, 26(9): 225–230. 期刊类型引用(7)
1. 王毅. 爆炸荷载作用下钢筋混凝土板性能分析. 砖瓦. 2024(03): 56-59 . 
百度学术2. Hao-nan Zhao，Hong-yuan Fang，Xiao-hua Zhao. The dynamic response and damage models of rebar reinforced polymer slabs subjected to contact and near-field explosions. Defence Technology. 2023(10): 330-342 . 必应学术3. 张正义，郑宇，肖海英，张笑瀛，彭航，陈俊翰. 骨料粒径对爆炸加载下混凝土破碎性的影响研究. 兵器装备工程学报. 2023(11): 84-89 . 百度学术4. 亓晓鹏，张杰，赵婷婷，王志勇，王志华. 考虑骨料级配的混凝土靶板接触爆炸破坏模式. 兵工学报. 2023(12): 3641-3653 . 百度学术5. 杜永峰，靳振飞. 灌浆套筒连接装配式框架-剪力墙爆炸响应与破坏. 灾害学. 2021(01): 106-111+133 . 百度学术6. 荣誉，任会兰，许香照. 弹体高速冲击载荷下钢筋混凝土的破坏行为实验与细观数值模拟. 中国科学:技术科学. 2021(03): 259-271 . 百度学术7. 杜永峰，靳振飞. 灌浆套筒连接装配式剪力墙爆炸响应及参数分析. 世界地震工程. 2020(04): 62-70 . 百度学术其他类型引用(9)
-
百度学术
计量
- 文章访问数: 6559
- HTML全文浏览量: 1694
- PDF下载量: 146
- 被引次数: 16