Dynamic compressive response of metal orthogonal corrugated sandwich structure
-
摘要: 通过理论和数值方法,对冲击载荷下金属正交波纹夹芯结构的动态压缩响应进行了研究。考虑材料应变率影响,建立了金属正交波纹夹芯结构动态响应的理论模型,同时对它的动态压缩响应进行了有限元模拟。结果表明,考虑材料应变率影响的理论模型的预测结果与有限元模拟结果吻合较好。进一步对多层正交波纹夹芯结构的动态压缩响应进行了数值模拟,获得了不同速度冲击下的变形模式,分析了层数对其动态响应的影响。研究发现,通过增加层数能够有效地增强结构的缓冲吸能能力,但层数超过4层以后增强效果不明显。Abstract: In this paper, dynamic compression response of metal orthogonal corrugated sandwich structures under impact loading is investigated theoretically and numerically. Considering the effect of strain rate of material, analytical models of dynamic response of metal orthogonal corrugated sandwich structure is developed. Finite element simulation of its dynamic compressive response is carried out. It is shown that there is a good agreement between the results based on the analytical model and finite element simulation. Furthermore, the dynamic compressive response of multi-layer orthogonal corrugated sandwich structure is studied using finite element method. Deformation modes under different impact velocities are obtained and the influence of the number of layers on the dynamic response is analyzed. It is found that the mitigation and energy absorption capacity of the sandwich structures can be effectively enhanced by increasing the number of layers while the number of layers have mild influence after exceeding four.
-
随着工业社会的发展,高性能的工程材料需要满足质量轻、强度高、多功能应用等要求,而多孔材料及结构在能够有效地减轻结构质量的同时实现其他功能。因此,多孔材料及其结构在民用和国防等领域有着广泛的应用前景。人们已经成功制造了无序泡沫材料[1-2]、有序点阵/格栅结构[3-6]等。根据微结构形式的不同,可将点阵材料分为二维和三维点阵材料,二维点阵材料主要由二维排列的多边形组成,在第3个方向拉伸成棱柱[3],它也被称为格栅材料。三维点阵材料是由具有一定规则的杆、板组成的空间桁架结构[4-6]。研究表明,泡沫的比强度和比刚度略小于格栅材料[3, 5, 7]。近年来,对二维点阵材料给予了更多的关注[3, 8-12],且已应用于一些航空航天结构。三维点阵结构由于其较大的比强度和比刚度,内部的开放空间易于实现多功能特性[13],也作为一种新型的轻质多孔材料被提出[4-6]。
Kooistra等[10]从理论上给出了波纹夹芯板的失效机理图,并且得到了一、二阶波纹夹芯板的压缩刚度和强度、剪切刚度和强度,并通过实验对理论结果进行了验证。Foo等[14]研究了蜂窝夹芯板在低速冲击下的能量吸收和失效。Zhang等[15-16]分别通过理论和模拟的方法,研究了爆炸载荷下梯形波纹夹芯板的动态响应以及冲击载荷下正弦形波纹夹芯板的动态响应。Mcshane等[17]还研究了波纹夹芯结构的动态屈曲行为,得到了前、后面板反力的变化,给出了结构的变形机制图。Deshpande等[5]通过理论和实验,研究了四面体点阵夹芯梁的三点弯曲问题。Xue等[18]从理论上研究了爆炸载荷作用下四面体点阵夹芯板的动态响应,并且用有限元验证了其理论。由于多层结构具有较好的缓冲能力和能量吸收能力,Hou等[19]提出了3种不同的多层波纹夹芯结构布置形式,并研究了他们在准静态压缩下的力学性能,分别是常规布置、交错布置以及0°/90°正交布置,发现正交布置性能最好、交错布置性能次之、常规布置性能最差。Wadley等[20]通过研究多层金字塔点阵的准静态力学行为,发现具有层间板的多层金字塔点阵有较高的平面内强度,而对多层金字塔点阵受水下爆炸时的动态响应研究发现,多层金字塔点阵的多层结构导致的芯材软化,使其背面板的传递压力低于实体板,起到了缓冲的效果。Dharmasena等[21]发现,多层波纹夹芯结构的芯体强度比金字塔结构芯体强度更低、具有更好的缓冲能力,并且层间板的质量是不容忽视的。
在二维点阵/格栅材料中,波纹夹芯结构以其质量轻、强度高、容易成型、制造成本低等优点,被广泛应用于航空航天、船舶、高速列车等运输工具中[10-12]。但波纹夹芯结构具有明显的各向异性,为了克服这些局限性,学者们采用编织的方法[22]结合传统波纹夹芯结构,提出了一种新型轻质正交波纹夹芯结构[23-24]。该结构在其平面内两个方向的抗剪和抗弯性能基本相同,具有横观正交各向同性的特点。同时,由于芯体采用了正交波纹设计,使结构抗局部屈曲的能力大大提高。芯层正交设计形成的缝隙为结构的多功能设计提供了足够的空间,功能材料可根据工程应用的需要进行配置实现多功能化设计。在强动载荷作用下,芯材可以变形吸收外部冲击能量,从而有效地保护放置在正交波纹夹芯结构背面的目标。Hu等[25]、Li等[26]分别研究了由复合材料组成的该结构的板和圆柱壳的性能。同时,根据多层结构的优点,继而基于单层正交波纹夹芯结构,我们提出了一种多层正交波纹夹芯结构。
本文中,拟通过理论和模拟研究冲击载荷下金属正交波纹结构的动态压缩响应,建立其动态响应的理论分析模型,分析材料应变率、结构层数、冲击速度等因素对其载荷缓冲和吸能能力的影响,揭示金属正交波纹夹芯结构抗冲击缓冲和吸能机理,研究结果将为该结构的工程应用提供重要技术支持。
1. 理论建模
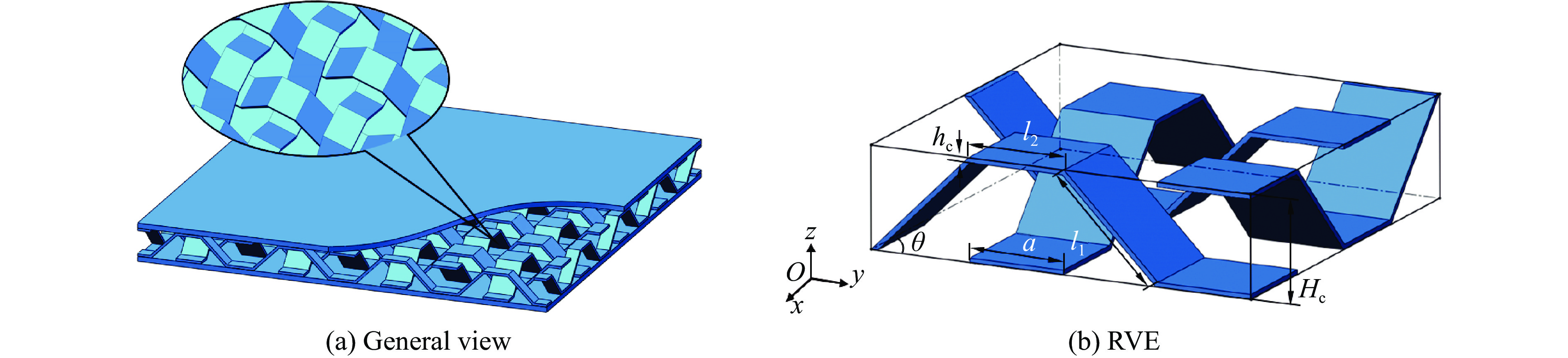
单层正交波纹夹芯结构如图1(a)所示,该夹芯结构是由上下面板和芯体组成3层夹芯结构,芯体由多个梯形波纹条状结构正交铺设而成,芯体和面板之间的连接可以通过激光焊接或强力胶粘接实现。代表体积单元(representative volume element,RVE)是一个能够在空间沿着一定方向排列、从而形成整个点阵夹芯结构的单元。单层正交波纹结构芯体的代表体积单元如图1(b)所示,根据代表体积单元,单层正交波纹夹芯结构的相对密度
ˉρs 为:ˉρs=ρsρ0=2ahc(l1+l2)(l1cosθ+l2)2(l1sinθ+hc) (1) 式中:
hc 为芯材厚度,l1 为梯形波纹斜边长度,l2 为梯形波纹底边长度,a 为波纹宽度,θ 为波纹倾斜角,ρs 为单层正交波纹结构的密度,ρ0 为构成单层正交波纹芯体的母材密度。基于单层正交波纹夹芯结构,提出了一种多层正交波纹夹芯结构,如图2(a)所示。由于正交波纹夹芯结构在面内已经是正交布置,所以在多层布置方式上采用交错布置,最大限度的提升多层结构的性能。与单层正交波纹夹芯结构类似,多层夹芯结构的代表体积单元如图2(b)所示,则多层正交波纹夹芯结构的相对密度
ˉρm 为:ˉρm=ρmρ0=ˉρc+ˉρi=2a(l1+l2)N∑n=1hcn(l1cosθ+l2)2Hc+N−1∑n=1hinHc (2) 式中:
hcn 为第n层的芯材厚度,hin 为第n层层间板的厚度,N 为层数,Hc=Nl1sinθ+N−1∑n=1hin+N∑n=1hcn 为芯材的总高度;ρm 为多层正交波纹夹芯结构的密度,ˉρc 为正交波纹芯体对整体结构相对密度的贡献,ˉρi 为层间板对整体结构相对密度的贡献。当N=1 时,式(2)也可以退化为单层结构的相对密度,即式(1)。Mcshane等[17]建立了冲击载荷下传统波纹夹芯结构在“打桩”变形模式下的理论分析模型,但没有考虑材料的应变率敏感性。在此理论分析模型的基础上,提出了一个考虑应变率敏感性适用于单层结构的理论模型。在该模型中,考虑材料的应变率敏感性,并且采用Cowper-Symonds方程描述材料的应变率敏感性,即:
σd0σy=1+(˙εD)1/q (3) 式中:
σd0 为材料的动态流动应力,σy 为材料的静态屈服应力,˙ε 为材料的应变率;D、q为材料的常数。假设构成母材的动态屈服应力
σdy 可以由动态流动应力σd0 代替,即:σdy=σd0=σy[1+(˙εD)1/q] (4) 同样,假设材料应变率用结构的等效应变率
˙εeq 近似,即:˙ε=˙εeq=d(vtHc)dt=vHc=vl1sinθ+hc (5) 式中:
v 为结构受冲击的速度,t 为时间。将式(5)代入式(4),可得材料在动态下的屈服应力
σdy 为:σdy=σy[1+(vHcD)1/q] (6) 定义夹芯结构前、后面板等效应力
σf、σb 分别为:σf=FfA=Ff4(l1cosθ+l2)2 (7) σb=FbA=Fb4(l1cosθ+l2)2 (8) 式中:
Ff、Fb 分别为前、后面板受到的纵向反力,A=4(l1cosθ+l2)2 为面板的面积。在“打桩”模式下,假设整个正交波纹芯体都处于屈服状态,所以由平衡方程以及对正交波纹芯体的代表体积单元的等效化处理,可得后面板受到的反力
Fb 为:Fb=8σdyahcsinθ (9) 因此,由式(6)、(8)~(9),可得夹芯结构后面板的等效应力为:
σbσy=2ahc[1+(vDHc)1/q]sinθ(l1cosθ+l2)2 (10) 对结构采用动量定理,可得:
Ff=Fb+d(mv)dt (11) 式中:
m=8ρ0vahctsinθ 为被压缩部分的正交波纹芯体的总质量。所以,由式(7)、(9)和(11),可得夹芯结构前面板的等效应力为:
σf=σb+2ρ0v2ahcsinθ(l1cosθ+l2)2 (12) 2. 有限元模型
利用有限元软件ABAQUS/Explicit分析冲击载荷下正交波纹夹芯结构的动态响应。由于正交波纹夹芯结构是由代表体积单元在空间按照一定方向周期排列而成,结构中一般包含很多个代表体积单元,所以边界条件对结构受冲击下的动态压缩响应的影响较小,因此可以采用具有周期边界条件的代表体积单元模拟夹芯结构的动态响应。有限元模拟中所采用的几何参数见表1,一般情况下层间板厚度都比芯材厚度大[20-21],因为更厚的层间板厚度可以减小芯材和层间板之间的相互作用。对于单层结构而言,层间板的厚度
hi=0 。为了方便计算,多层结构采用的层间板厚度是芯材厚度的2倍。表 1 正交波纹夹芯结构的几何尺寸Table 1. Geometric dimensions of orthogonal corrugated sandwich structurel1/cm l2/cm θ/(°) a/cm hc/cm hi/cm √2 1 45 1 0.05 0.1 单层结构的模型由面板和芯材组成,认为其面板强度足够大,可以假设为解析刚体。芯材采用八节点缩减积分实体单元建模(C3D8R),且假设芯材和面板之间是理想黏接不会产生滑动,因此采用绑定约束。多层结构与单层结构类似,不同层数的结构相对密度可以由式(2)得出,见表2。由表2可以看出,在不同层数情况下,芯材和层间板对于整体结构相对密度的贡献不同,但层间板对于多层结构的动态响应不能忽略,所以层间板建模也采用八节点缩减积分实体单元而不是解析刚体。通过网格敏感性分析得出,结构网格最小尺寸为0.062 5 mm时,既能保持良好的精度,又能节约计算时间。
表 2 不同层数的正交波纹夹芯结构的相对密度Table 2. Relative density of orthogonal corrugated sandwich structure with different layersN ˉρc/% ˉρi/% ˉρm/% 1 5.75 0 5.75 2 5.49 4.55 10.04 3 5.41 5.97 11.38 4 5.37 6.67 12.04 5 5.34 7.07 12.41 选用钢作为模拟的基体材料,假定它为理想弹塑性,密度
ρ0=8000kg/m3 、屈服强度σy=200MPa 、弹性模量E0=200GPa 、泊松比μ=0.3 ;与应变率有关的材料常数D=39000,q=5 [27]。在有限元模型中,采用了通用的自接触,假定所有表面之间的接触为法向硬接触,并将动摩擦因数设为0.2[28]。采用背板完全固定,前面板以恒定速度v=20~300 m/s压缩芯材。为了方便描述结构的动态响应,定义芯材压缩应变为:
εc=δHc (13) 式中:
δ 为前面板的位移。同样,定义前、后面板的无量纲化平均等效应力分别为:
ˉσf=σavgfσy (14) ˉσb=σavgbσy (15) 式中:
σavgf、σavgb 为前、后面板的等效应力在εc =0~0.7的平均值。定义夹芯结构的比吸能为:
χ=UpM (16) 式中:
Up 为εc=0.7 时结构的塑性耗散能,M为结构芯体的总质量。3. 结果与讨论
3.1 单层正交波纹夹芯结构的动态响应
图3对比了不同速度冲击下单层正交波纹夹芯结构前、后面板平均等效应力的理论预测值和有限元模拟结果。从图3可以清晰地看出,考虑材料应变率效应的理论模型的预测值与有限元结果吻合得较好,不考虑芯材应变率效应的理论模型低估了平均等效应力。
有限元计算给出了不同冲击速度下单层正交波纹夹芯结构的动态响应,如图4~6所示。冲击速度为20 m/s时(见图4),夹芯结构的变形模式为近似整体屈曲的模式,这里定义为B模式,进一步分析可以看出,芯体结构的不断屈曲导致前、后面板的等效应力的不停震荡;屈曲的时候应力减小,之后随着它和面板接触应力又增大。冲击速度为120 m/s时(见图5),夹芯结构的变形模式为屈曲波的模式,这里定义为W模式,该模式由屈曲模式和“打桩”模式交替出现而组成;当芯体结构发生屈曲模式时应力震荡,而发生“打桩”模式时应力几乎保持不变。冲击速度为300 m/s时(见图6),夹芯结构的变形模式为“打桩”模式,这里定义为S模式;当芯体结构发生“打桩”的时候,应力几乎是不变的。这3种典型的变形模式在传统波纹夹芯结构中已有类似的变形模式发生[17],但是在正交波纹夹芯结构中考虑了材料的应变率效应,并且正交波纹夹芯结构不同于传统的波纹夹芯结构。因此,具体的变形模式以及响应也有所不同,同时芯体的正交设计导致结构芯材在变形的过程中有更多接触发生。
3.2 多层正交波纹夹芯结构的动态响应
冲击载荷作用下多层正交波纹夹芯结构每层的变形模式和响应与单层正交波纹夹芯结构类似,两层正交波纹夹芯结构的动态响应如图7~10所示。
冲击速度为20 m/s(见图7)时,正交波纹结构芯材的变形模式为整体近似屈曲的模式,同时层间板几乎没有变形,这里采用每层正交波纹芯体的变形模式来定义整个结构的变形模式,称为B-B模式。冲击速度为60 m/s(见图8)时,上层夹芯结构的变形模式为W模式,下层夹芯结构的变形模式为B模式,定义这种混合变形模式为W-B模式;特别地,在
εc=0.46 时,前面板等效应力变得很大,这是因为刚性的前面板直接接触到了层间板,而前、后面板的应力在εc =0.1~0.2几乎保持不变,这是因为芯材发生了W模式变形。冲击速度为120 m/s(见图9)时,上下芯材的变形模式均呈W模式,定义夹芯结构的变形模式为W-W模式,前面板等效应力的突然增大同样是由于刚性的前面板直接接触到了层间板而导致的。冲击速度为300 m/s(见图10)时,上下芯材的变形模式均呈S模式,这时定义夹芯结构的变形模式为S-S模式;需要注意的是,由于前面板的冲击速度太大,导致层间板有较大变形,除了层间面板接触导致前面板的等效应力突然变化,其他变形时刻前后面板的等效应力几乎不变,这是芯材发生“打桩”模式导致的。3层正交波纹夹芯结构的动态响应与两层夹芯结构类似。然而当冲击速度为260 m/s时,3层夹芯结构的变形模式和两层夹芯结构不同,如图11所示。从上到下,第1夹芯层和第2夹芯层的变形模式为S模式,第3夹芯层的变形模式为W模式,定义夹芯结构的这种混合变形模式为S-S-W模式,这是在两层正交波纹夹芯结构中没有发现的模式。这可能是因为,第2夹芯层的层间板缓冲了上面结构的冲击,在第3夹芯层出现了W模式。4、5层正交波纹夹芯结构的动态响应和变形模式与3层结构类似。表3为不同冲击载荷下不同层数正交波纹夹芯结构的变形模式。
表 3 正交波纹夹芯结构的变形模式Table 3. Deformation modes of orthogonal corrugated sandwich structureN 变形模式 v=20 m/s v=60 m/s v=160 m/s v=260 m/s v=300 m/s 1 B W W S S 2 B-B W-B W-W S-S S-S 3 B-B-B W-B-B W-W-W S-S-W S-S-S 4 B-B-B-B W-B-B-B W-W-W-W S-S-W-W S-S-S-S 5 B-B-B-B-B W-B-B-B-B W-W-W-W-W S-S-W-W-W S-S-S-S-S 3.3 层数对正交波纹夹芯结构动态响应的影响
在结构防护设计中,缓冲吸能性能是一个很重要的衡量指标。结构的缓冲能力可以通过前、后面板的应力来表征。在动态压缩响应过程中,作用在面板的应力越小,结构的缓冲能力越好。结构的吸能能力可以通过比吸能来表征,结构的比吸能越大,结构的吸能能力越好。
不同冲击速度下正交波纹夹芯结构的后面板平均等效应力如图12所示。由图12可以看出,随着冲击速度从20 m/s增加到300 m/s,单层夹芯结构的平均应力先增大后减小,之后又增大最后减小。当冲击速度为80 m/s时有一个极小值,这可能是因为结构变形模式由B模式变为W模式导致;当冲击速度超过180 m/s以后,平均应力减小,这可能是因为结构的变形模式发生变化已变为S模式,由于开始时冲击速度过大,应力波没有传播到后面板,导致变形初始后面板的应力为零,并且随着冲击速度的增加整体加载时间会变短,这个应力为零的时间相对加载时间就显得就越长,整个的平均应力也就越小。对于两层夹芯结构,结构的后面板平均应力先减小后增大最后又略有减小。当冲击速度为40 m/s时有一个极小值,这可能是因为结构变形模式由B-B模式变为W-B模式导致,这点与单层结构相类似;当速度超过240 m/s后,平均应力减小,这个现象的出现与单层结构最后的应力变小的原因类似。对于3~5层结构,后面板平均应力变化均与1~2层结构类似。从图12中还可以看出,随着层数的增加,正交波纹夹芯结构的后面板平均等效应力减小,这说明随着正交波纹夹芯结构的层数增加会增强结构的缓冲能力,但随着层数的增加,增强效果会慢慢地减弱。
不同冲击速度下正交波纹夹芯结构的比吸能,如图13所示。可以看出,在较低的速度(v=20,60 m/s)下,增加层数会使结构的比吸能降低,这是因为层间板在较低的速度冲击下几乎没有变形,大部分没有参与吸能。然而在高速冲击(v=260,300 m/s)下,随着夹芯结构的层数增加,夹芯结构的比吸能在增加,这是因为此时层间板发生较大变形吸收了部分冲击能量,但是当层数超过4层以后比吸能增加不太明显。
4. 结 论
基于单层正交波纹夹芯结构,提出了一种多层正交波纹夹芯结构,并且考虑材料应变率效应,建立了金属正交波纹夹芯结构动态压缩过程中结构响应的理论模型,理论预测值与有限元模拟结果吻合较好;进一步分析发现,夹芯结构的变形模式和速度以及层数有关,多层结构的变形模式由单层结构的变形模式组成,多层结构每层的变形模式沿着冲击方向由单层结构的高速模式向低速模式变化;对比冲击载荷下不同层数正交波纹夹芯结构的动态压缩响应发现,多层设计能够有效地增强结构的缓冲吸能能力,但层数超过4层以后增强效果不明显。
-
表 1 正交波纹夹芯结构的几何尺寸
Table 1. Geometric dimensions of orthogonal corrugated sandwich structure
l1/cm l2/cm θ/(°) a/cm hc/cm hi/cm √2 1 45 1 0.05 0.1 表 2 不同层数的正交波纹夹芯结构的相对密度
Table 2. Relative density of orthogonal corrugated sandwich structure with different layers
N ˉρc/% ˉρi/% ˉρm/% 1 5.75 0 5.75 2 5.49 4.55 10.04 3 5.41 5.97 11.38 4 5.37 6.67 12.04 5 5.34 7.07 12.41 表 3 正交波纹夹芯结构的变形模式
Table 3. Deformation modes of orthogonal corrugated sandwich structure
N 变形模式 v=20 m/s v=60 m/s v=160 m/s v=260 m/s v=300 m/s 1 B W W S S 2 B-B W-B W-W S-S S-S 3 B-B-B W-B-B W-W-W S-S-W S-S-S 4 B-B-B-B W-B-B-B W-W-W-W S-S-W-W S-S-S-S 5 B-B-B-B-B W-B-B-B-B W-W-W-W-W S-S-W-W-W S-S-S-S-S -
[1] ASHBY M F, EVANS A, FLECK N A, et al. Metal foams: a design guide [M]. Oxford, UK: Butterworth-Heinemann, 2000. DOI: 10.1016/S0261-3069(01)00049-8. [2] GIBSON L J A A, ASHBY M F. Cellular solids: structure and properties [M]. Cambridge: Cambridge University Press, 1997. DOI: 10.1016/0921-5093(90)90295-E. [3] WANG A J, MCDOWELL D L. In-plane stiffness and yield strength of periodic metal honeycombs [J]. Journal of Engineering Materials & Technology, 2004, 126(2): 137–156. DOI: 10.1115/1.1646165. [4] DESHPANDE V S, FLECK N A, ASHBY M F. Effective properties of the octet-truss lattice material [J]. Journal of the Mechanics & Physics of Solids, 2001, 49(8): 1747–1769. DOI: 10.1016/s0022-5096(01)00010-2. [5] DESHPANDE V S, FLECK N A. Collapse of truss core sandwich beams in 3-point bending [J]. International Journal of Solids & Structures, 2001, 38(36): 6275–6305. DOI: 10.1016/S0020-7683(01)00103-2. [6] FAN H L, FANG D N, JING F N. Yield surfaces and micro-failure mechanism of block lattice truss materials [J]. Materials & Design, 2008, 29(10): 2038–2042. DOI: 10.1016/j.matdes.2008.04.013. [7] ASHBY M F, BRÉCHET Y J M. Designing hybrid materials [J]. Acta Materialia, 2003, 51(19): 5801–5821. DOI: 10.1016/S1359-6454(03)00441-5. [8] EBRAHIMI H, GHOSH R, MAHDI E, et al. Honeycomb sandwich panels subjected to combined shock and projectile impact [J]. International Journal of Impact Engineering, 2016, 95: 1–11. DOI: 10.1016/j.ijimpeng.2016.04.009. [9] RUBINO V, DESHPANDE V S, FLECK N A. The dynamic response of end-clamped sandwich beams with a Y-frame or corrugated core [J]. International Journal of Impact Engineering, 2008, 35(8): 829–844. DOI: 10.1016/j.ijimpeng.2007.10.006. [10] KOOISTRA G, DESHPANDE V, WADLEY H. Hierarchical corrugated core sandwich panel concepts [J]. Journal of Applied Mechanics, 2007, 74(2): 259–268. DOI: 10.1115/1.2198243. [11] KAZEMAHVAZI S, ZENKERT D. Corrugated all-composite sandwich structures. Part 1: Modeling [J]. Composites Science and Technology, 2009, 69(7/8): 913–919. DOI: 10.1016/j.compscitech.2008.11.030. [12] KAZEMAHVAZI S, TANNER D, DAN Z. Corrugated all-composite sandwich structures. Part 2: Failure mechanisms and experimental programme [J]. Composites Science and Technology, 2010, 69(7): 920–925. DOI: 10.1016/j.compscitech.2008.11.035. [13] CHEN M, PEI Y, FANG D. Computational method for radar absorbing composite lattice grids [J]. Computational Materials Science, 2009, 46(3): 591–594. DOI: 10.1016/j.commatsci.2008.12.011. [14] FOO C C, SEAH L K, CHAI G B. Low-velocity impact failure of aluminium honeycomb sandwich panels [J]. Composite Structures, 2008, 85(1): 20–28. DOI: 10.1016/j.compstruct.2007.10.016. [15] ZHANG J X, QIN Q H, WANG T J. The resistance of metallic sandwich plates to blast loading [J]. Key Engineering Materials, 2011, 462-463: 349–354. DOI: 10.4028/www.scientific.net/KEM.462-463.349. [16] ZHANG J, QIN Q, WANG T J. Compressive strengths and dynamic response of corrugated metal sandwich plates with unfilled and foam-filled sinusoidal plate cores [J]. Acta Mechanica, 2013, 224(4): 759–775. DOI: 10.1007/s00707-012-0770-5. [17] MCSHANE G J, PINGLE S M, DESHPANDE V S, et al. Dynamic buckling of an inclined strut [J]. International Journal of Solids and Structures, 2012, 49(19/20): 2830–2838. DOI: 10.1016/j.ijsolstr.2012.03.045. [18] XUE Z, HUTCHINSON J W. A comparative study of impulse-resistant metal sandwich plates [J]. International Journal of Impact Engineering, 2004, 30(10): 1283–1305. DOI: 10.1016/j.ijimpeng.2003.08.007. [19] HOU S, SHU C, ZHAO S, et al. Experimental and numerical studies on multi-layered corrugated sandwich panels under crushing loading [J]. Composite Structures, 2015, 126: 371–385. DOI: 10.1016/j.compstruct.2015.02.039. [20] WADLEY H, DHARMASENA K, CHEN Y, et al. Compressive response of multilayered pyramidal lattices during underwater shock loading [J]. International Journal of Impact Engineering, 2008, 35(9): 1102–1114. DOI: 10.1016/j.ijimpeng.2007.06.009. [21] DHARMASENA K, QUEHEILLALT D, WADLEY H, et al. Dynamic response of a multilayer prismatic structure to impulsive loads incident from water [J]. International Journal of Impact Engineering, 2009, 36(4): 632–643. DOI: 10.1016/j.ijimpeng.2008.06.002. [22] FAN H L, MENG F H, YANG W. Mechanical behaviors and bending effects of carbon fiber reinforced lattice materials [J]. Archive of Applied Mechanics, 2006, 75(10/11/12): 635–647. DOI: 10.1007/s00419-006-0032-x. [23] 秦庆华, 郝文乾, 郭奕蓉, 等. 一种轻质正交波纹夹芯复合结构及其制备方法: 中国, ZL201510117807.8 [P]. 2015-03-17. [24] LEEKITWATTANA M, BOYD S W, SHENOI R A. Evaluation of the transverse shear stiffness of a steel bi-directional corrugated-strip-core sandwich beam [J]. Journal of Constructional Steel Research, 2011, 67(2): 248–254. DOI: 10.1016/j.jcsr.2010.07.010. [25] HU Y, LI W, AN X, et al. Fabrication and mechanical behaviors of corrugated lattice truss composite sandwich panels [J]. Composites Science and Technology, 2016, 125: 114–122. DOI: 10.1016/j.compscitech.2016.02.003. [26] LI W, SUN F, WANG P, et al. A novel carbon fiber reinforced lattice truss sandwich cylinder: fabrication and experiments [J]. Composites Part A: Applied Science and Manufacturing, 2016, 81: 313–322. DOI: 10.1016/j.compositesa.2015.11.034. [27] HSU S S, JONES N. Quasi-static and dynamic axial crushing of thin-walled circular stainless steel, mild steel and aluminium alloy tubes [J]. International Journal of Crashworthiness, 2004, 9(2): 195–217. DOI: 10.1533/ijcr.2004.0282. [28] KILIÇASLAN C, GÜDEN M, ODACI İ K, et al. The impact responses and the finite element modeling of layered trapezoidal corrugated aluminum core and aluminum sheet interlayer sandwich structures [J]. Materials & Design, 2013, 46: 121–133. 期刊类型引用(5)
1. 胡旭初,付涛. 低速冲击下波纹夹芯板的动态响应分析. 哈尔滨工程大学学报. 2025(04): 797-805 .  百度学术
百度学术2. 崔天宁,秦庆华. 轻质多孔夹芯结构的弹道侵彻行为研究进展. 力学进展. 2023(02): 395-432 .  百度学术
百度学术3. 程曦,刘志芳,李世强. 面外压缩载荷下多层夹芯板的理论分析与数值研究. 应用力学学报. 2023(05): 1164-1170 .  百度学术
百度学术4. 胡朝磊,孙海亮,王志鹏,包兆鹏,崔天宁,秦庆华. 高速颗粒流冲击下负泊松比力学超材料夹芯梁的动态响应及缓冲吸能机理. 爆炸与冲击. 2022(12): 22-36 .  本站查看
本站查看5. 任会兰,申海艇,栗建桥. 单轴压缩下Ti-6Al-4V空心点阵结构的力学行为. 中国科学:技术科学. 2021(03): 293-304 .  百度学术
百度学术其他类型引用(3)
-









 下载:
下载:







































































 下载:
下载:












 百度学术
百度学术