Attenuation of blast wave in a large-section tunnel
-
摘要: 隧道开挖爆破产生的空气冲击波的破坏效应，将会对人员、机具设备与周围环境造成危害。隧道钻孔爆破冲击波的影响因素比裸露药包爆炸更多、更复杂，研究其衰减规律对采取合适的防护措施意义重大。本文中开展了时速350 km双线铁路大断面隧道钻孔爆破空气冲击波的现场测试，分析了不同工况下冲击波传播规律及影响因素。结果表明：钻爆冲击波超压时程曲线存在多个不同幅值的超压波峰，波峰之间具有明显微差延时的短间隔性，传播至远场未形成稳定的单一平面波，与单一药包爆炸冲击波的传播规律存在差异；钻爆冲击波超压信号由多段与微差延时相对应的子信号叠加而成，子信号数量与毫秒延期雷管段数相同，呈现出典型的时域特征；相同爆破条件下，大断面隧道钻爆时的乳化炸药冲击波转化因数小于小断面巷道工况下的；相较于总药量及最大段药量，按掏槽药量计算的超压峰值与实测超压峰值之间的相关性最强，钻爆冲击波最大超压峰值宜按掏槽段炸药TNT当量确定；隧道内大型机械设备等障碍物改变了钻爆冲击波流场的传播规律，呈现较明显的叠加放大效应。Abstract: The blasting air shock wave produced by tunnel excavation results in considerable casualties and damage to equipments and environments. Compared with those of the explosion of bare charges, the influencing factors of the blast wave induced by tunnel drilling are more complicated, so it is of considerable significance to study its attenuation law for taking appropriate protective measures. In this paper, a field test of blasting shock wave was carried out during the drilling and blasting of a large cross-section tunnel with a speed of 350 km/h, and the propagation law and influence factors of blasting shock wave under different conditions were analyzed. The results display that there are multiple overpressure peaks with different amplitudes in the shock wave overpressure-time curve, showing the short time intervals with significant millisecond delay characteristics between wave peaks. When the shock wave propagates to the far field, it does not form a stable plane wave, and it is different from the propagation law of shock wave of the single charge explosion. The shock wave overpressure signal is superimposed by multiple sub-signals, showing typical time domain properties, and the number of sub-signals is the same as that of the millisecond delay detonator segments. Under the same blasting conditions, the conversion factor of emulsion explosive energy into shock wave in a large-section tunnel is smaller than that in a small-section tunnel. Compared with the total charge and the maximum charge, the linear correlation between the peak values of shock wave overpressure calculated by the cut-hole charge and the measured peak values is the strongest. Then the maximum peak value of the blasting shock wave overpressure should be determined according to TNT equivalent of the cut-hole charge. Obstacles such as the large equipment in the tunnel will change the propagation law of the shock wave, showing a significant superimposed amplification effect.
-
隧道钻孔爆破（以下简称钻爆）法是当今隧道建设中不可或缺的挖掘方式之一，在较长时间内仍会发挥至关重要的作用。钻爆中炸药爆炸反应释放的能量并非完全用于破岩，部分能量分别转化为围岩中的振动波和空气中的冲击波，少部分被爆破飞石消耗。若对隧道钻爆冲击波控制不当，则其会对施工人员、机具设备和周围环境产生不利影响。
区别于地面自由场，空气冲击波在隧道等受限空间中受壁面约束使其衰减更慢，影响距离更远。长期以来，许多学者对独头或双向开口巷道内裸露药包爆炸引起的冲击波传播规律进行了大量研究。Benselama等[1]发现，冲击波在隧道内传播的纵向空间分布可划分为两个区域：在靠近爆源的近爆区呈现空气自由场超压衰减模式，而在远离爆源的远场可视为一维超压衰减模式。杨科之等[2]通过数值模拟和模型试验，得到了双向开口长坑道中心离地1.5 m的炸药爆炸冲击波传播规律，并通过量纲分析拟合了冲击波超压计算公式。李秀地等[3]通过9次模型试验，分析了药包堵口爆炸情况下等截面直坑道内冲击波的传播规律。刘晶波等[4]通过数值模拟，分析了双向开口等截面直坑道内药包爆炸冲击波的传播规律，并拟合了冲击波超压峰值计算公式。田志敏等[5]通过数值模拟，获得了直径为3.5 m的带封闭端墙的独头和双向开口隧道爆炸冲击波流场及作用于隧道衬砌表面冲击波荷载峰值分布规律。Yan等[6]数值模拟了双向开口地铁隧道中心药包爆炸冲击波的传播，通过量纲分析拟合了冲击波超压计算公式。李玉民等[7]通过量纲分析导出了井巷内冲击波传播规律的基本关系式，基于实测数据拟合了巷道中心爆炸冲击波超压经验式。庞伟宾等[8-9]进行了直坑道和T型坑道坑口内、外爆炸模型试验，分别建立了预测高能炸药爆炸冲击波到达时间公式。以上文献引用的现场数据均源自于小断面巷道中裸露药包离地爆炸。在隧道独头掘进钻爆冲击波传播规律方面，赵晓磊[10]现场测试并探讨了小断面浅埋地铁隧道钻爆冲击波的衰减规律及不同段药量的影响，得到了不同掏槽形式和孔深条件下冲击波能量转化因数。陈明等[11]基于现场爆破试验理论分析和数值模拟，研究了隧洞开挖爆破空气冲击波超压诱发围岩振动的机理及特性。
综上所述，已有研究重点在独头或双向开口巷道内裸露药包离地爆炸冲击波的衰减规律，对大断面交通隧道施工钻爆冲击波传播规律研究甚少，且以往针对冲击波超压的计算参数研究多局限于矿井巷道等小断面隧道。高速铁路隧道因断面面积大、炸药用药总量大、独头开挖距离长、洞内大型机械设备多等特点，使冲击波传播规律更复杂。本文中，依托设计时速350 km的郑万铁路双线大断面隧道工程，采用现场实测和理论分析的方法，探讨大断面隧道钻爆冲击波的传播和衰减规律，以期研究结果可为钻爆冲击波的安全评价和防护提供参考。
1. 现场试验
1.1 工程概况
钻爆冲击波试验工点为设计时速350 km的郑万铁路七峰山隧道：全长5 152 m；断面高约12.5 m，宽约14.8 m，面积约110 m2；系大断面隧道。试验段为较完整的弱风化花岗岩，Ⅲ级围岩，台阶法施工，上台阶开挖高度为8.0m，接近全断面开挖。爆破采用浅孔分段毫秒延期爆破，选用2号岩石乳化炸药，炮孔直径为42 mm，楔形掏槽，内掏槽孔深度为3.0 m，外掏槽孔深度为5.5 m，周边光面爆破，孔深为4.2 m，导爆管起爆网格，隧道炮孔布置及段别布置见图1。典型的上台阶钻爆设计参数见表1。
表 1 上台阶钻爆设计参数Table 1. Design parameters of borehole blasting for the upper bench炮孔分类 段别 孔数 孔深/m 单孔药卷数 单孔用药量/kg 延期时间/ms 总药量/kg 内掏槽 1 4 3.0 5 1.0 0 4.0 外掏槽 1 16 5.5 12/15 2.4/3.0 0 44.4 辅助孔 3 16 4.8 13 2.6 50 41.6 辅助孔 5 10 4.8 12 2.4 110 24.0 辅助孔 7 12 4.5 11 2.2 200 26.4 辅助孔 9 18 4.5 11 2.2 310 39.6 辅助孔 11 6 4.3 11 2.2 460 13.2 辅助孔 13 12 4.2 8/9 1.6/1.8 650 20.4 上周边孔 15 47 4.2 4 0.8 880 37.6 下周边孔 15 8 4.2 8/9/10/11 1.6/1.8/2.0/2.2 880 15.2 压孔 7 6 4.5 8 1.6 200 9.6 压孔辅助 9 8 4.5 8 1.6 310 12.8 二圈孔 13 10 4.2 6 1.2 650 12.0 抬孔 3 2 4.3 3 0.6 50 1.2 抬孔 5 6 4.2 9 1.8 110 10.8 抬孔 7 5 4.2 10 2.0 200 10.0 底板孔 11 16 4.5 8/11/12 1.6/2.2/2.4 460 39.6 角孔 15 2 4.5 12 2.4 880 4.8 合计 367.2 1.2 测试方案
用TC-4850爆破测试仪和CYG1401型冲击波压力传感器采集数据，其量程为0～250 kPa，灵敏度为20 V/MPa，测试触发压力为0.5 kPa，预保留触发前0.1 s数据，记录总时长2.0 s。测试时每台仪器接入2路冲击波测试通道，当位于前方的通道(测点1)触发时，仪器同时记录2通道数据。测试仪器和现场仪器布置分别如图2、3所示。
按图4所示方案，沿隧道轴线方向距掌子面不同距离布置冲击波超压测点，距底板高度为1.2 m。据田志敏等[5]的研究成果，在爆心距R大于5倍洞径D的爆源远场，冲击波在隧道内将以平面波的形式传播，同一横截面上的超压峰值基本相同。本试验的测点爆心距R均大于5倍洞径59.15 m，因此测试中忽略测点与爆源高差和夹角的影响。
图 4 隧道洞内冲击波超压测点布置Figure 4. Measuring points of blasting shock wave overpressure in the tunnel1.3 测试结果
现场记录了6组共20个测点的有效试验数据，测试结果见表2。需说明的是，隧道中衬砌台车和砼罐车等障碍物会对冲击波流场传播规律造成影响，表中带“*”超压峰值为衬砌台车或砼罐车后方隧道中线处实测数据。
表 2 隧道上台阶钻爆冲击波测试结果Table 2. Records of shock wave test in upper bench cutting试验工况 总药量/kg 掏槽药量/kg 最大段药量(段别)/kg 测点 爆心距/m 超压峰值/kPa A1 367.2 48.4 57.6 (MS15) 1 74.5 4.259 3 136.0 2.818 4 166.0 1.894 A2 359.5 49.6 90.4 (MS09) 1 80.0 3.549 2 110.0 2.408 3 140.0 2.802* 4 170.0 2.645* 5 210.0 2.269* A3 359.5 49.6 90.4 (MS09) 1 64.0 4.608 2 99.0 3.019 3 144.0 2.567 4 184.0 1.863 A4 343.7 47.2 62.4 (MS11) 1 165.0 3.213 3 255.0 2.292* 4 300.0 2.764* A5 343.7 47.2 62.4 (MS11) 1 147.0 3.380 2 173.0 2.959 3 279.0 3.089* A6 351.7 52.0 63.2 (MS11) 1 146.0 3.870 2 174.0 2.590 2. 实测数据分析
2.1 冲击波超压时程曲线衰减特征
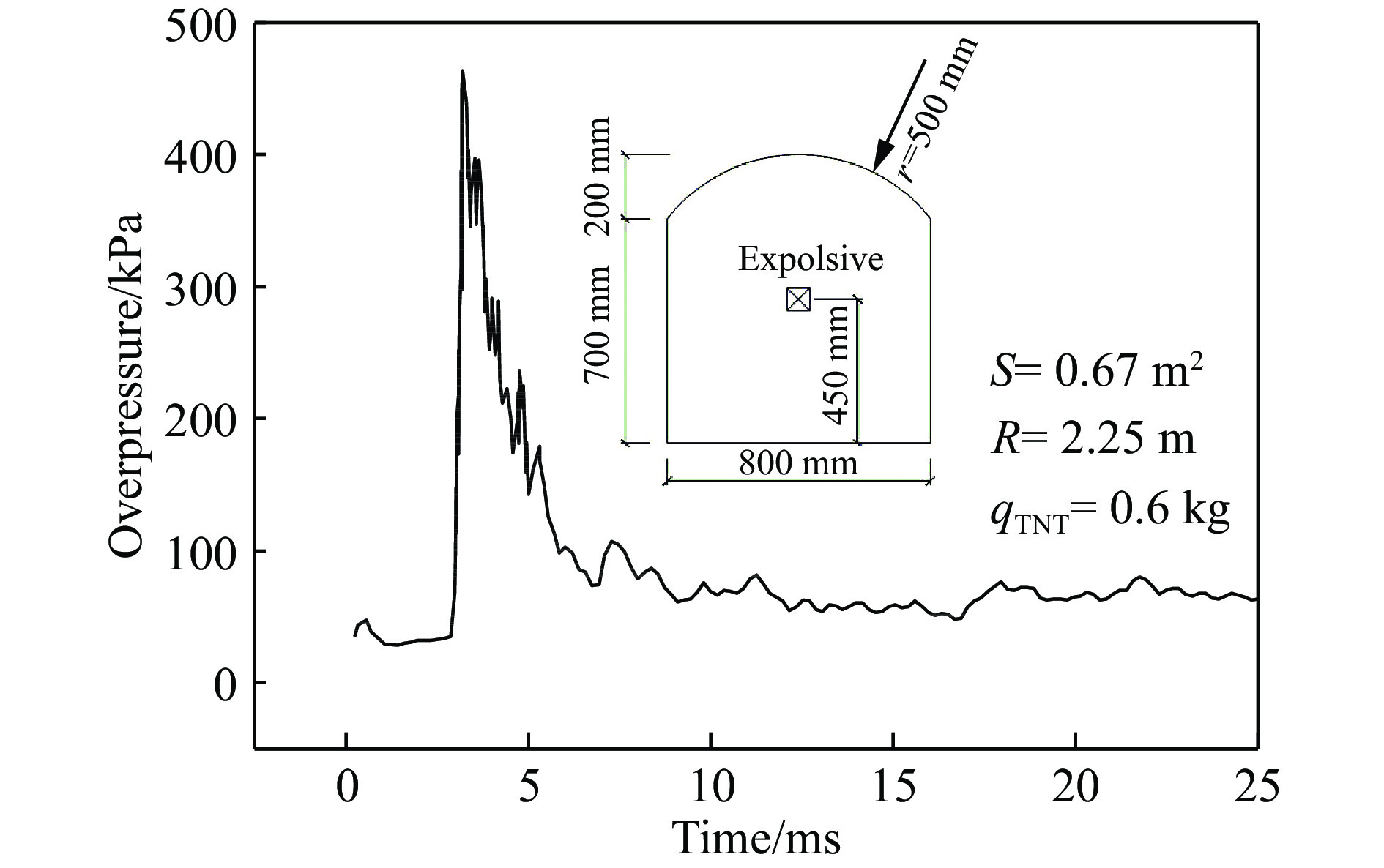
《爆破安全规程实施手册》[12]规定，在平坦地形条件下计算爆破冲击波超压，秒延期爆破选取最大一段药量计算，毫秒延期爆破按总药量计算。对于巷道爆破的超压计算未见明确的药量规定，如何合理选取钻爆冲击波超压的计算药量有待于进一步探讨。为此，首先选取图5(a)所示的测点A1-1处的钻爆冲击波超压时程曲线，提取时程曲线起波阶段波形，如图5(b)所示，与坑道内裸露药包离地爆炸试验[4]得到的空气冲击波超压时程曲线(见图6)进行对比分析。
图 5 隧道钻爆冲击波超压时程曲线Figure 5. Shock wave overpressure-time curves in railway tunnel borehole blasting
观察图5(b)和图6可以发现，隧道钻爆冲击波起波阶段波形与裸露药包爆炸冲击波波形具有以下相同点：起波初期，冲击波波阵面到达测点，冲击波超压急剧上升，形成超压峰值。在超压达到最大峰值后，受隧道壁面多次反射作用及叠加影响，波形呈明显的锯齿状衰减[3]。比较图5(a)和图6可知，两者的差异在于裸露药包爆炸冲击波为单次超压峰值，而独头隧道钻爆冲击波波形曲线毛刺多，呈多次超压峰值。初步分析认为，隧道钻爆冲击波波形多次超压峰值的出现由分段毫秒微差爆破引起。
为进一步探讨隧道微差爆破对冲击波超压波形及衰减特征的影响机制，在测点A1-3处同时测试了冲击波超压和隧道边墙处(距拱脚高度1.2 m)爆破振速，如图7所示。
图 7 隧道钻爆振速和冲击波超压时程曲线Figure 7. Vibration velocity-time curve and shock wave overpressure-time curve in tunnel borehole blasting由图7可知，相同爆心距下，经围岩传播的振动衰减很快，总历时约1.0 s，而钻爆冲击波具有持续时间长、衰减较慢的特点。爆破振速和冲击波超压的最大峰值均出现在起波阶段，对应的雷管段别为掏槽段(MS1)，而非最大药量段(MS15)。同时可见，在测点A1-3处0.86 s时刻冲击波出现了明显的超压波峰。分析微差延期时序发现，该时刻超压峰值由周边孔最大药量段(MS15)爆破引起的。此外，结合表1中各段别药量统计，因MS3、MS5、MS7、MS9、MS11、MS13段的装药量相对较小，加之波形的部分叠加，图7(b)中不同段别雷管延期爆破作用下的冲击波信号无法直接区分，时域局部化特性并不显著。但可以说明，钻爆冲击波在隧道内传播至远场处并未形成稳定的单一平面波，受多段微差爆破作用而出现了多个不同幅值的超压波峰。
为精确识别微差爆破引起的振动和冲击波信号的时域特征，采用模式自适应小波时能密度法[13]分别对测点A1-3处的振动和冲击波信号进行时能密度分析，以确定实测微差爆破振动和冲击波信号的实际延期时间。图8为采用模式自适应小波作为基函数，尺度下限为0.1、上限为10和间隔为0.1的时能密度曲线，图中纵坐标|Wf(a,b)|2表示小波系数的模的平方。图8中爆破振动和冲击波信号的时能密度曲线中均出现了8个奇异点，表明实测的微差爆破振动和冲击波信号是由8段爆破子信号叠加而成，分别出现于段间微差延期时间相对应的8次峰值。
图 8 微差爆破振动与冲击波信号的模式自适应小波时能密度曲线Figure 8. Pattern adapted wavelet time-energy density curves of millisecond blast vibration and shock wave signal根据隧道掘进微差爆破理论，因隧道掌子面仅有一个临空面，四周岩石夹制力大，掏槽爆破成为隧道掘进爆破技术的关键，且通常情况下最大振速也由掏槽段药量决定。为降低隧道爆破振动，每段之间延期时差应避免爆破地震波主振相的相互叠加，让炸药能量在临空面方向快速释放，更多的能量用于岩体破碎和抛掷。工程中一般按跳段使用毫秒延期雷管，各段雷管起爆时间间隔50～200 ms，从而使得爆破振动信号具有显著的时域特征。据图7(b)和图8(b)可知，分段微差爆破冲击波超压信号也具有明显的时域特征，超压峰值的出现具有短间隔性，与微差延期时间间隔存在较好的对应关系。
由上述分析可知，隧道钻爆冲击波超压最大峰值主要取决于掏槽药量的大小，估算钻爆冲击波超压峰值时，计算药量宜按掏槽药量取值，以体现微差爆破冲击波信号的时域特性。这与现行《爆破安全规程》[14]中，计算分段微差爆破振动安全允许距离时炸药量应取最大单段药量的原理相同。
2.2 冲击波超压峰值计算参数
隧道掘进爆破中炸药能量转化为冲击波初始能量的影响因素多，《爆破安全规程实施手册》[12]列出了炮孔爆破中影响炸药能量转化为冲击波初始能量的因素包括炸药类型、岩石物理力学性质、装药密度、起爆药包位置和起爆方式等。手册[12]中同时给出了巷道爆破空气冲击波超压计算公式：
Δp=(3270qmyR∑S+780√qmyR∑S)e−βRdn (1) 式中：Δp为冲击波超压值，kPa；R为爆心距，m；q为炸药质量，kg；my为炸药转化为冲击波的转化因数(以下简称炸药冲击波转化因数)；ΣS为与药包毗邻的巷道总面积，m2；β为巷道壁面粗糙性因数；dn为巷道等效水力学直径，m。
按式(1)估算钻爆冲击波超压，需要重点确定影响冲击波传播衰减规律的巷道壁面粗糙性因数β和炸药冲击波转化因数my。同时，应注意式(1)是针对爆热为4 200 kJ/kg的炸药推导出来的[15]，对于其他类型炸药，炸药质量应乘以当量因数，转化为爆热为4 200 kJ/kg的TNT炸药质量。
冲击波波阵面上的压力衰减强度取决于巷道断面大小和其壁面粗糙性，当冲击波沿着一定断面的巷道传播时，可按式(2)计算冲击波的衰减[15]。已知测点爆心距R和超压峰值Δp，即可反算砼壁面粗糙性因数β。
Δp+=Δp0⋅R0R0+ΔRe−βRdn (2) 式中：Δp+为与选定点距离为ΔR处的冲击波超压，kPa；Δp0为某选定点处的冲击波超压，kPa；R0为某选定点的爆心距，m；ΔR为两个选定点爆心距之间的距离，m。
根据现场测试结果，选择表2测试工况A3(无障碍物)中测点1和测点4的爆心距R和超压峰值Δp，代入式(2)可得隧道支护砼壁面粗糙性因数β=0.014。
另，式(1)中隧道钻爆炸药冲击波转化因数my，可通过下式计算确定[15]：
my=EpEq=EpqcutQv (3) 式中：Ep为钻爆冲击波的初始能量，kJ；Eq为炸药爆炸能量，kJ；qcut为微差爆破掏槽段TNT炸药当量，kg；Qv为TNT炸药爆热，4 200 kJ/kg；
将式(3)代入式(1)，整理并简化后可得关于钻爆冲击波初始能量Ep的一元二次方程：
7.8Ep10RS+38√Ep10RS−ΔpeβRdn=0 (4) 求解式(4)可得：
Ep=10RS(√361+7.8ΔpeβRdn−197.8)2 (5) 根据隧道内无障碍物工况下的现场测试结果，由式(5)、式(3)可确定炸药冲击波转化因数my，其中试验用2号岩石乳化炸药的爆热为3 200 kJ/kg。计算结果表明，反向起爆炸药冲击波转化因数my在0.004～0.012之间，平均值为0.007。计算值明显小于文献[15]中推荐的浅孔反向不堵塞炮孔爆破条件下的转化因数0.07～0.10。分析认为，在隧道围岩条件、炸药类型、装药结构、起爆方法等爆破条件基本相同的情况下，一方面是2号岩石乳化炸药与Ⅲ级硬质围岩的匹配性较好，炸药能量利用率高，另一方面主要是因交通隧道断面大，相对小断面巷道而言冲击波初始能量降低。
2.3 冲击波超压峰值与段别药量对应关系
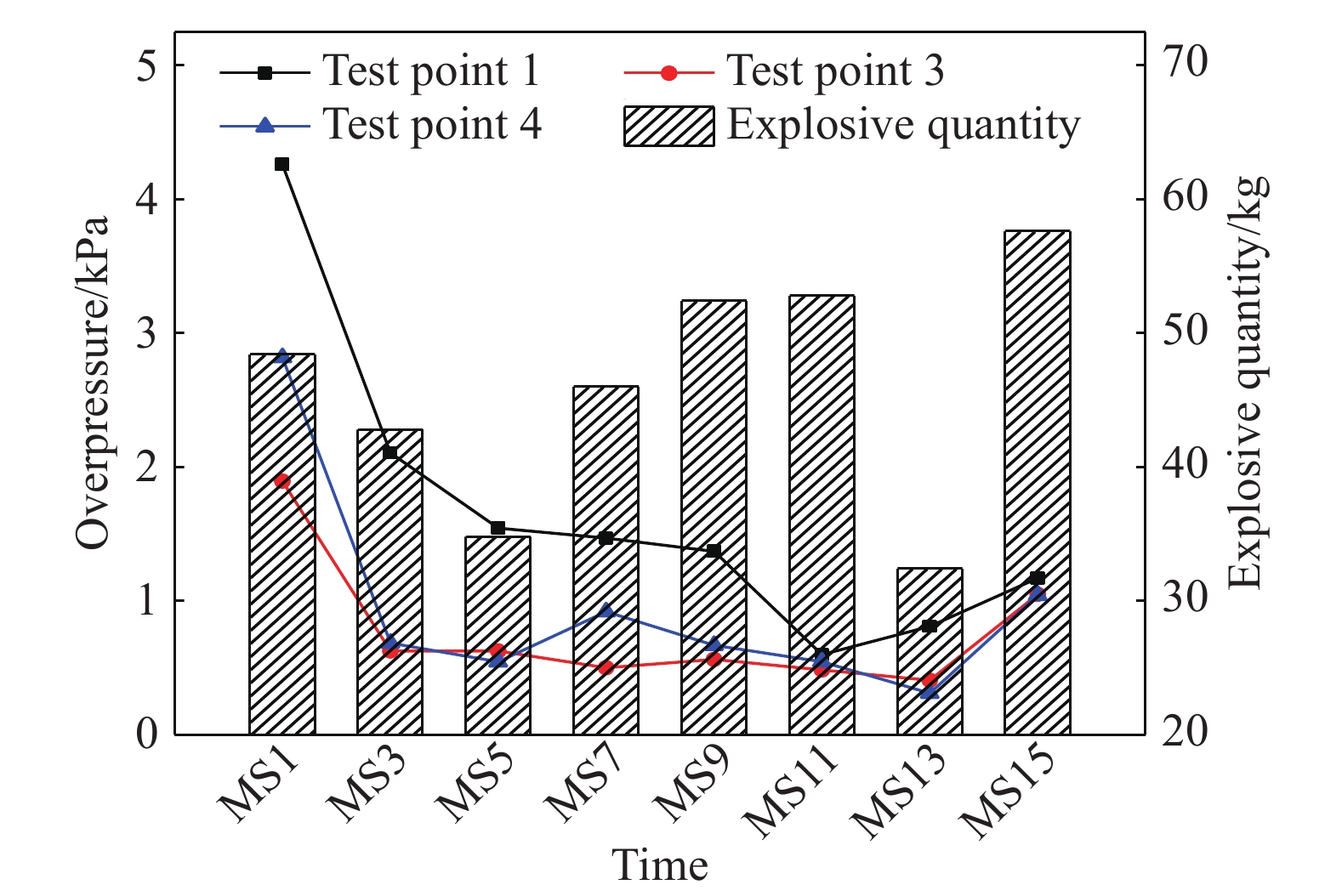
为进一步分析钻爆冲击波超压峰值与装药量之间的对应关系，选择试验工况A1中3个实测超压数据，提取MS1～MS15对应的超压峰值，结果如图9所示。由图9可知，各段别时刻内的超压峰值与各段别药量之间无直接的相关性，尽管最大药量段为周边孔MS15段，且MS9、MS11段药量也明显大于掏槽段，但超压峰值依然出现在掏槽MS1段起爆时刻。这进一步说明，隧道掘进掏槽孔、崩落孔及周边孔在临空面条件和破岩作用等方面的差异，导致掏槽孔MS1段起爆诱发的冲击波超压值最大。
图 9 各段别时刻对应的超压峰值与药量关系Figure 9. Relations between peak overpressure and explosive quantity at different segments将2.2节壁面粗糙性因数β和炸药冲击波转化因数my代入式(1)，分别获得掏槽药量qcut、总药量及分段最大药量情况下的计算超压峰值，与实测最大超压峰值同时绘制在图10中。由图10可知，按掏槽药量qcut计算的超压峰值与实测超压峰值均匀分散在45°线两侧，两者之间具有相对较好的线性相关性(r2=0.61)。因此，对于隧道分段毫秒延期爆破(微差延期间隔大于50 ms)，采用掏槽药量qcut计算超压峰值，可更好地表征超压峰值与分段微差延期爆破时刻的对应关系。
图 10 计算超压和实测超压之间的相关性Figure 10. Correlations between calculated and measured overpressures2.4 遇障碍物冲击波超压放大效应
前文据冲击波遇障碍物之前的超压数据确定了冲击波的计算参数。事实上，隧道内冲击波的传播过程十分复杂。隧道施工期内衬砌台车、砼罐车等障碍物(见图11)也会对冲击波传播流场产生干扰。
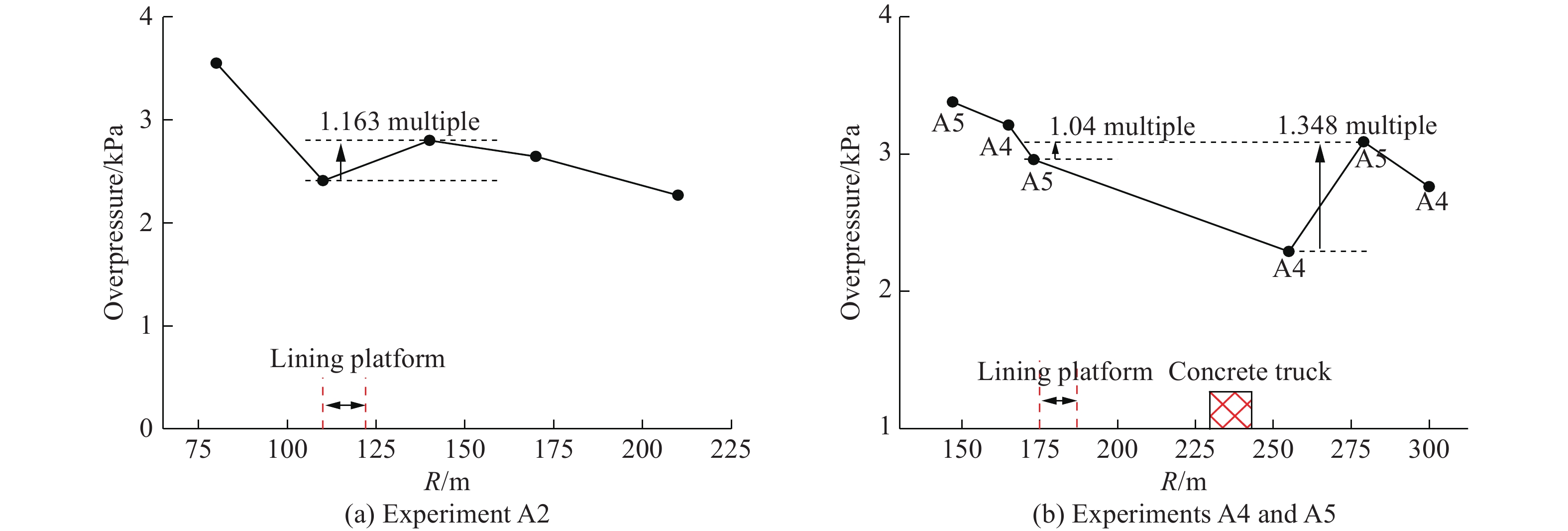
测试工况A2、A4和A5记录了冲击波穿越障碍物前后的冲击波超压变化过程，各测点超压峰值随爆心距变化如图12所示。其中测试A4和A5为连续测试，整理在同一图中。由图12可知，冲击波在障碍物前方持续衰减，在遇障碍物前30 m范围内，工况A2中冲击波超压由3.549 kPa衰减为2.408 kPa，而工况A5中冲击波超压由3.38 kPa衰减到2.959 kPa，衰减率分别为32%和12%。当冲击波穿越障碍物后一定范围内冲击波超压出现增大，尔后又再度减小，其中工况A2中超压值由2.408 kPa增大至2.802 kPa，而工况A4、A5中超压值由2.292 kPa增大至3.089 kPa，放大因数分别为1.163和1.348。由上述分析可知，隧道内衬砌台车与砼罐车等障碍物的存在相当于隧道断面由大变小，冲击波发生新的压缩、反射和绕流，改变了冲击波流场的传播特征，从而使得冲击波超压呈现增大趋势。
图 12 钻爆冲击波遇障碍物前后超压峰值变化曲线Figure 12. Overpressure peak changes of shock waves induced by drilling and blasting operation in the explosive fields with obstacles由上述分析可知，隧道内大型障碍物(如衬砌台车)对冲击波的影响是显著的。在无障碍物试验工况A1、A3和A6中，隧道内远场冲击波较好地符合非线性衰减规律，而对于工况A2、A4和A5，冲击波在穿越障碍物前后，冲击波出现增强，流场传播规律变得比较复杂，是一个值得继续探讨的现象。
3. 钻爆冲击波超压峰值探讨
当今业界多以爆破振速单一指标评价隧道爆破的破坏效应，忽视了冲击波的毁伤效应。《爆破安全规程》[14]在考虑爆破冲击波安全允许距离时，仅给出了平坦地形条件下爆破时空气冲击波超压计算公式，缺少隧道钻爆冲击波超压估算公式。本文中，通过现场实测，分析了超压峰值与计算药量的关系，初步确定了大断面隧道钻爆冲击波计算参数，即隧道支护砼壁面粗糙性因数β和不堵炮泥浅孔分段延期反向起爆时2号岩石乳化炸药的冲击波转化因数my，相应的钻爆冲击波超压峰值可按下式估算：
Δp=(3270qcutmyRS+780√qcutmyRS)eβRdn (6) 式中：qcut为分段毫秒延期爆破掏槽段药的TNT当量，kg；my为炸药冲击波转化因数，my=0.007；β为隧道壁面粗糙性因数，β=0.014；其他参数同式(1)。
图13是按式(1)计算的超压峰值和实测数据分别与比例距离的关系曲线。由图13可知，对于试验工况A2、A4、A5，受障碍物的影响使得计算超压值与实测值存在较大偏离，而未受障碍物影响的超压数据(工况A1、A3、A6)与理论预测值吻合度较好(相关系数r为0.74)，可为大断面隧道钻爆冲击波安全评价和防护提供参考。
图 13 钻爆冲击波超压峰值与比例距离的关系Figure 13. Relation of blasting air shock wave overpressure peak values versus scaled distance4. 结 论
在高速铁路双线大断面隧道钻爆冲击波现场测试的基础上，分析了钻爆冲击波的传播规律。研究结果表明：
（1）隧道钻爆冲击波时程曲线毛刺多，波形呈锯齿状，出现多个不同幅值的超压波峰，远场处未形成稳定的单一平面波，明显区别于隧道内裸露药包爆炸冲击波的衰减特征。钻爆冲击波信号的模式自适应小波时能密度分析结果表明，冲击波超压信号由多段与微差延期时间相对应的子信号叠加而成，具有显著的微差延期时域特征。
（2）隧道分段毫秒延期钻爆冲击波超压峰值与段别药量有着紧密联系，超压峰值按掏槽药量计算时与实测超压值的相关性最强。估算冲击波超压峰值时，宜按掏槽段炸药的TNT当量确定计算药量。
（3）基于现场试验数据，初步获得了适用于估算大断面隧道钻爆冲击波超压的计算参数：高速铁路大断面隧道支护砼壁面粗糙性因数β=0.014；不堵炮泥浅孔微差延期反向起爆时2号岩石乳化炸药的冲击波转化因数my=0.007。
（4）隧道内障碍物对冲击波传播规律具有显著影响，穿越障碍物过程中冲击波发生新的压缩、反射、绕流和叠加，冲击波呈现衰减-增强-再衰减的传播特性。
（5）结合现场实测数据对比分析了隧道钻爆冲击波的计算超压值与实测超压值，验证了冲击波超压预测公式的可行性，为大断面隧道钻爆冲击波安全评价和防护提供参考。
需要说明的是，大(特大)断面隧道钻爆施工条件复杂，钻爆冲击波衰减规律受多种因素的影响，有关钻爆冲击波信号的时频特性、合理的超压估算公式及计算参数取值问题，尚有待进一步研究。
-
图 4 隧道洞内冲击波超压测点布置
Figure 4. Measuring points of blasting shock wave overpressure in the tunnel
图 5 隧道钻爆冲击波超压时程曲线
Figure 5. Shock wave overpressure-time curves in railway tunnel borehole blasting
图 7 隧道钻爆振速和冲击波超压时程曲线
Figure 7. Vibration velocity-time curve and shock wave overpressure-time curve in tunnel borehole blasting
图 8 微差爆破振动与冲击波信号的模式自适应小波时能密度曲线
Figure 8. Pattern adapted wavelet time-energy density curves of millisecond blast vibration and shock wave signal
图 9 各段别时刻对应的超压峰值与药量关系
Figure 9. Relations between peak overpressure and explosive quantity at different segments
图 10 计算超压和实测超压之间的相关性
Figure 10. Correlations between calculated and measured overpressures
图 12 钻爆冲击波遇障碍物前后超压峰值变化曲线
Figure 12. Overpressure peak changes of shock waves induced by drilling and blasting operation in the explosive fields with obstacles
图 13 钻爆冲击波超压峰值与比例距离的关系
Figure 13. Relation of blasting air shock wave overpressure peak values versus scaled distance
表 1 上台阶钻爆设计参数
Table 1. Design parameters of borehole blasting for the upper bench
炮孔分类 段别 孔数 孔深/m 单孔药卷数 单孔用药量/kg 延期时间/ms 总药量/kg 内掏槽 1 4 3.0 5 1.0 0 4.0 外掏槽 1 16 5.5 12/15 2.4/3.0 0 44.4 辅助孔 3 16 4.8 13 2.6 50 41.6 辅助孔 5 10 4.8 12 2.4 110 24.0 辅助孔 7 12 4.5 11 2.2 200 26.4 辅助孔 9 18 4.5 11 2.2 310 39.6 辅助孔 11 6 4.3 11 2.2 460 13.2 辅助孔 13 12 4.2 8/9 1.6/1.8 650 20.4 上周边孔 15 47 4.2 4 0.8 880 37.6 下周边孔 15 8 4.2 8/9/10/11 1.6/1.8/2.0/2.2 880 15.2 压孔 7 6 4.5 8 1.6 200 9.6 压孔辅助 9 8 4.5 8 1.6 310 12.8 二圈孔 13 10 4.2 6 1.2 650 12.0 抬孔 3 2 4.3 3 0.6 50 1.2 抬孔 5 6 4.2 9 1.8 110 10.8 抬孔 7 5 4.2 10 2.0 200 10.0 底板孔 11 16 4.5 8/11/12 1.6/2.2/2.4 460 39.6 角孔 15 2 4.5 12 2.4 880 4.8 合计 367.2 
下载: 导出CSV
表 2 隧道上台阶钻爆冲击波测试结果
Table 2. Records of shock wave test in upper bench cutting
试验工况 总药量/kg 掏槽药量/kg 最大段药量(段别)/kg 测点 爆心距/m 超压峰值/kPa A1 367.2 48.4 57.6 (MS15) 1 74.5 4.259 3 136.0 2.818 4 166.0 1.894 A2 359.5 49.6 90.4 (MS09) 1 80.0 3.549 2 110.0 2.408 3 140.0 2.802* 4 170.0 2.645* 5 210.0 2.269* A3 359.5 49.6 90.4 (MS09) 1 64.0 4.608 2 99.0 3.019 3 144.0 2.567 4 184.0 1.863 A4 343.7 47.2 62.4 (MS11) 1 165.0 3.213 3 255.0 2.292* 4 300.0 2.764* A5 343.7 47.2 62.4 (MS11) 1 147.0 3.380 2 173.0 2.959 3 279.0 3.089* A6 351.7 52.0 63.2 (MS11) 1 146.0 3.870 2 174.0 2.590
下载: 导出CSV
-
[1] BENSELAMA A M, WILLIAM-LOUIS MAMEJ P, MONNOYER F, et al. A numerical study of the evolution of the blast wave shape in tunnels [J]. Journal of Hazardous Materials, 2010, 181(1-3): 609–616. DOI: 10.1016/j.jhazmat.2010.05.056. [2] 杨科之, 杨秀敏. 坑道内化爆冲击波的传播规律 [J]. 爆炸与冲击, 2003, 23(1): 37–40.YANG K Z, YANG X M. Shock waves propagation inside tunnels [J]. Explosion and Shock Waves, 2003, 23(1): 37–40. [3] 李秀地, 郑颖人. 坑道中冲击波冲量传播模型的试验 [J]. 解放军理工大学学报(自然科学版), 2007, 8(5): 425–428. DOI: 10.7666/j.issn.1009-3443.20070504.LI X D, ZHENG Y R. Scale model tests to determine in tunnel blast impulse from HE charges at tunnel entrance [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2007, 8(5): 425–428. DOI: 10.7666/j.issn.1009-3443.20070504. [4] 刘晶波, 闫秋实, 伍俊. 坑道内爆炸冲击波传播规律的研究 [J]. 振动与冲击, 2009, 28(6): 8–11. DOI: 10.3969/j.issn.1000-3835.2009.06.003.LIU J B, YAN Q S, WU J. Analysis of blast wave propagation inside tunnels [J]. Journal of Vibration and Shock, 2009, 28(6): 8–11. DOI: 10.3969/j.issn.1000-3835.2009.06.003. [5] 田志敏, 邬玉斌, 罗奇峰. 隧道内爆炸冲击波传播特性及爆炸荷载分布规律研究 [J]. 振动与冲击, 2011, 30(1): 21–26. DOI: 10.3969/j.issn.1000-3835.2011.01.005.TIAN Z M, WU Y B, LUO Q F. Characteristics of in-tunnel explosion-induced air shock wave and distribution law of reflected shock wave load [J]. Journal of Vibration and Shock, 2011, 30(1): 21–26. DOI: 10.3969/j.issn.1000-3835.2011.01.005. [6] YAN Q S, DU X L. Forecasting research of overpressure of explosive blast in subway tunnels [J]. Journal of Vibroengineering, 2015, 17(6): 33810–3391. [7] 李玉民, 胡峰. 井下爆破空气冲击波传播规律的量纲分析与拟合 [J]. 矿冶工程, 1993, 13(2): 13–17.LI Y M, HU F. The dimensional and regression analyses on the propagation regulation of air blast waves in mines [J]. Mining and Metallurgical Engineering, 1993, 13(2): 13–17. [8] 庞伟宾, 何翔, 李茂生, 等. 空气冲击波在坑道内走时规律的实验研究 [J]. 爆炸与冲击, 2003, 23(6): 573–576.PANG W B, HE X, LI M S, et al. The formula for air blast time of arrival in tunnel [J]. Explosion and Shock Waves, 2003, 23(6): 573–576. [9] 庞伟宾, 李永池, 何翔. 化爆冲击波在T型通道内到时规律的实验研究 [J]. 爆炸与冲击, 2007, 27(1): 63–67. DOI: 10.11883/1001-1455(2007)01-0063-05.PANG W B, LI Y C, HE X. The regularity of arrival time in T-shaped tunnel for shock wave due to explosions from high explosive charges [J]. Explosion and Shock Waves, 2007, 27(1): 63–67. DOI: 10.11883/1001-1455(2007)01-0063-05. [10] 赵晓磊. 城市地铁浅埋隧道爆破振动及空气冲击波传播规律探索[D]. 大连: 大连理工大学, 2015.ZHAO X L. Exploration of laws of blasting vibration and air blast propagation for urban shallow tunnels [D]. Dalian, China: Dalian University of Technology, 2015. [11] 陈明, 何文学, 卢文波, 等. 隧洞开挖爆破空气超压诱发围岩振动机理 [J]. 振动与冲击, 2017, 36(12): 12–17. DOI: 10.13465/j.cnki.jvs.2017.12.003.CHEN M, HE W X, LU W B, et al. Studies on the vibration of tunnel surrounding rock induced by air overpressure [J]. Journal of Vibration and Shock, 2017, 36(12): 12–17. DOI: 10.13465/j.cnki.jvs.2017.12.003. [12] 汪旭光, 于亚伦, 刘殿中. 爆破安全规程实施手册[M]. 北京: 人民交通出版社, 2004: 157−158. [13] 张胜, 凌同华, 刘浩然, 等. 模式自适应小波时能密度法及其在微差爆破振动信号分析中的应用 [J]. 煤炭学报, 2014, 39(10): 2007–2013. DOI: 10.13225/j.cnki.jccs.2014.0325.ZHANG S, LING T H, LIU H R, et al. Pattern adapted wavelet time-energy density method and its application in millisecond blast vibration signal analysis [J]. Journal of China Coal Society, 2014, 39(10): 2007–2013. DOI: 10.13225/j.cnki.jccs.2014.0325. [14] 汪旭光, 郑炳旭, 张正忠, 等. 爆破安全规程GB6722-2014 [S]. 北京: 中国标准出版社, 2015: 42−46. [15] 萨文科, 古林, 马雷. 井下空气冲击波[M]. 龙维祺, 丁亚伦, 译. 北京: 冶金工业出版社, 1979: 98−100. -
下载:
计量
- 文章访问数: 5594
- HTML全文浏览量: 2018
- PDF下载量: 141
- 被引次数: 0