Frequency and duration dependence analysis of structural blasting vibration response
-
摘要: 明确结构爆破振动响应对频率与持续时间的依赖性有助于进行爆破参数设计和爆破振动安全评价。本文从频域上推导单段爆破振动、多段爆破振动、单自由度系统振动响应三者之间的关系,以延迟时间和爆破段数作为纽带分析爆破振动频率和持续时间对结构爆破振动响应的影响,最后以一组实测试验数据进行验证。结果表明,在延迟时间∆τ下,多段爆破振动出现间隔1/∆τ的频带现象,频率成分向优势频率fi=n/∆τ集中(n
∈ Z +),且随段数增加,优势频率幅值增大。爆破振动中接近结构自振频率fn的优势频率成分使结构产生较大振动响应,为此延迟时间的选择应保证在n/∆τ优势频率处不会引起结构的共振。多段爆破振动在其多个优势频率n/∆τ附近的结构振动响应放大系数均比单段大,其余处与单段相差不大,特别的,当优势频率fi、单段爆破振动主频fm和结构物自振频率fn三者相近时,结构可能产生最大的响应。爆破段数的增加,使爆破振动持续时间增加,但仅在一定范围内使结构爆破振动响应增加,增加到一定值后,结构响应与持续时间关系不大。Abstract: It is helpful to blasting parameter design and blasting vibration safety evaluation to make clear the dependence of blasting vibration response on frequency and duration. The relationship between single-delay blasting vibration, multi-delay blasting vibration and single-degree-of-freedom system response is derived from the frequency domain. The influence of blasting vibration frequency and duration on the vibration response of structure is analyzed by taking the delay time and the number of blasting stages as the link. Finally, a set of measured test data is used to verify. The results show that under the delay time ∆τ, the multi-delay blasting vibration has a frequency band phenomenon of 1/∆τ. The frequency component is concentrated to the dominant frequencyfi=n/Δτ(n∈Z+) , and as the number of segments increases, the dominant frequency amplitude increases. The structure produces a large vibration response when the dominant frequency component in blasting vibration is close to the natural frequency fn of the structure. Therefore, the delay time should be selected to ensure that the resonance of the structure is not caused at the dominant frequency of n/∆τ. In the response spectrum, the structural amplification factor of multi-delay blasting vibration is larger than that of a single-delay when frequency near its multiple dominant frequencies fi, which is basically consistent with the single-delay at the rest. In particular, when the dominant frequency fi, the single delay blasting vibration frequency fm, and the structure natural vibration frequency fn are similar, the structure may produce the maximum response. The increase of the number of blasting sections increases the duration of blasting vibration when the sections within a certain range. After increasing to a certain value, the structural response has little relationship with the duration.-
Key words:
- multi-delay /
- single-degree-of freedom systems /
- delay time /
- response spectrum
-
爆破技术广泛应用在水利、矿山、交通和城建等工程中,但爆破过程中诱发的爆破振动不可避免地将会对附近建(构)筑物或周边居民与民用设施造成负面影响[1-2],爆破振动的安全控制越来越受到重视。
爆破振动峰值粒子速度、频率和持续时间三参数在爆破振动与结构安全之间的关系中起着重要作用,目前国内外爆破振动安全规范将爆破振动峰值和主频作为两个重要参照特征值[1,3-6],其中我国《爆破安全规程》(GB6722-2014)更是明确指出将视主频作为爆破振动主频的特征值[7]。这对于多段爆破而言,进行爆破振动安全评价时仅考虑峰值所在的单段爆破振动PPV和视主频,而实际上多段相比于单段爆破振动持续时间增加,爆破振动频率也发生改变,结构可能产生不同的振动响应。Yang等[8]认为在采用毫秒延迟爆破时,整个振动的全局频率值不能真实反映振动频率特性。那么这种情况下分析结构爆破振动响应的频率和持续时间的依赖性是十分有必要的。
首先需要对影响单段和多段爆破振动的频率特性和持续时间的因素进行分析,Blair等[9]从频域角度出发,得出在延迟时间
Δτ 下多段爆破振动将出现间隔1/Δτ 的频带现象,但Blair等[10]又指出可利用短延迟时间使爆破能量向高频集中,但仅在一定距离范围内有效;Richards等[11]通过实测数据发现在微差爆破中,随着距离的增加,主频将随着延迟频率n/Δτ 及其半倍递减,并非连续变化;Qiu等[12]通过不同延迟时间爆破实验,认为短延迟时间可降低爆破振动中低频能量;凌同华[13]通过小波包分析实测多段微差爆破信号的能量分布,发现随雷管段数的增加,能量最大峰值对应的频率向中高频发展;赵明生等[14]通过叠加单段发现多段爆破振动主频呈现一定阶跃性。持续时间显然跟段数成正相关,段数越多,持续时间越长。其次是结构在爆破振动下的响应规律分析,凌同华等[15]分析单自由度系统在单段爆破振动下结构响应,发现单段爆破振动反应谱结构简单;陈士海等[16]、魏海霞[17]分析多自由度体系在实测单段爆破振动下结构响应,认为结构第一自振频率在分析响应幅值中起主导地位;李夕兵等[18, 19]对矿山实测单段和多段爆破振动进行反应谱分析,发现多段爆破振动反应谱更加复杂。
综上,前人的研究主要针对单段和多段爆破振动的频谱特性对比,或是单段爆破振动下结构振动响应,而对于单段爆破振动、多段爆破振动和结构振动响应三者之间的关系研究并未涉及。本文从多段和单段爆破振动频域联系出发,分析结构在其激励下的不同响应规律。
1. 单自由度系统的振动响应
多段比单段爆破振动频谱复杂,为明确频谱特性的异同给结构振动带来的影响,以单自由度系统进行分析是方便且有效的[20]。
如图1所示,单自由度系统在地面运动激励下的振动响应,其动力方程为[20-21]:
m¨x+c˙x+kx=−m¨xg(1) 式中:m为系统质量;c为系统阻尼;k为系统刚度;x为系统相对于地面初始位置的位移;xg为地面质点位置。
式(1)两边同时除以m得到振动响应方程[21]:
¨x+4πεfn˙x+4π2f2nx=−¨xg (2) 式中:
ε 为阻尼比,ε=c2√km ;fn为系统自振频率,fn=√k/m2π 。对方程(2)中各项进行傅里叶变换有[22]:
(i2πf)2ˆx(f)+i8π2εfnˆx(f)f+4π2f2nˆx(f)=−i2πˆ˙xg(f)f (3) 可得到振动速度响应的频域解为[22]:
ˆ˙x(f)=i2πˆx(f)f=ˆ˙xg(f)f2f2n−f2+2εffni=ˆ˙xg(f)γ21−γ2+2εγi (4) 式中:
γ=ffn 为频率比。振动速度响应时域解可由频域解进行傅里叶逆变换得到
˙x(t)=∫ˆ˙x(f)ei2πtfdf=∫ˆ˙xg(f)γ21−γ2+2εγiei2πtfdf (5) 根据式(5)可以得到振动速度响应峰值,将其与地面振动峰值相比即可得到放大系数。
为明确地面振动与结构响应频域之间的关联,这里进一步考虑振动速度响应的功率谱
Px(f) 。一般在荷载作用结束后,结构作自由衰减振动,也即结构在强迫振动阶段内有最大的速度响应,那么以强迫振动阶段进行分析:Px(f)=|ˆ˙x(f)|2d (6) 式中:d为结构强迫振动持时,也即地面爆破振动持续时间。
将式(4)代入式(6)得:
Px(f)=|ˆ˙xg(f)|2d|γ21−γ2+2εγi|2=Pg(f)R(γ) (7) 式中:令
Pg(f)=|ˆ˙xg(f)|2d ,为地面质点振动的功率谱;R(γ)=|γ21−γ2+2εγi|2=1[1γ2−(1−2ε2)]2+4ε2(1−ε2) ,为复频反应因子[21],工程中阻尼比ε 取0.01~0.1,函数在频率比γ=1附近时有极大值14ε2(1−ε2) 。2. 爆破振动响应影响因素分析与比较
2.1 毫秒延迟爆破振动的频域表达
爆破振动频率成分丰富,尤其是多段爆破频域特性更加复杂,而结构对不同频率成分将会有不同的响应[23, 24]。为此有必要从频域角度去分析爆破振动作用下结构的振动响应,这里首先给出单段和多段爆破振动频域表达之间的关系。
设有一单段爆破振动
˙xgs(t) ,持续时间为ts,其频域表达为ˆ˙xgs(f) ,那么功率谱密度为Pgs(f)=|ˆ˙xgs(f)|2ts 。假定各段爆破振动相同,延迟时间Δτ 相同,那么多段爆破振动的时域表达˙xgm(t) 、频域表达ˆ˙xgm(f) 和功率谱密度Pgm(f) 分别为[9]:˙xgm(t)=N∑i=1˙xgs(t−(i−1)Δτ) (8) ˆ˙xgm(f)=ˆ˙xgs(f)1−e−i2πfNΔτ1−e−i2πfΔτ (9) Pgm(f)=Pgs(f)sin2(πfNΔτ)sin2(πfΔτ)tstm (10) 式中:N为爆破段数;tm为多段爆破振动持续时间,
tm=ts+(N−1)Δτ 。令
M(f)=sin2(πfNΔτ)sin2(πfΔτ) ,称M为爆破振动功率谱因子,是关于f的周期函数,周期为1Δτ ,且有:M(f)=sin2(πfNΔτ)sin2(πfΔτ)={N2f=n/Δτn∈Z+0 (11) 将式(9)代入到式(5)得到多段爆破振动作用下的结构速度响应的时域表达
˙xm(t) :˙xm(t)=∫ˆ˙xgs(f)1−e−i2πfNΔτ1−e−i2πfΔτγ21−γ2+2ελiei2πftdf (12) 将式(10)代入到式(7)得到多段爆破振动作用下的结构速度响应功率谱
Pxm(f) :Pxm(f)=Pgm(f)R(γ)=Pgs(f)M(f)R(γ)tstm (13) 特别地,当式(12)~(13)中N=1,可得到单段爆破振动作用下的结构速度响应的时域表达
˙xs(t) 和功率谱密度Pxs(f) :˙xs(t)=∫ˆ˙xgs(f)γ21−γ2+2ελiei2πftdf (14) Pxs(f)=Pgs(f)R(γ) (15) 通过式(12)和(13)可以将单段爆破振动、多段爆破振动和结构振动响应三者联系起来。功率谱密度可以从频域的角度表示结构响应特性,式(13)表明结构响应特性受结构自身和爆破振动特性的影响,结构自身特性主要包括自振频率和阻尼比,其影响表示为
R(γ) ;而对于爆破振动特性,多段可由单段表示,当单段为Pgs(f) 时,多段为Pgs(f)M(f)ts/tm 。那么由此可见结构多段和单段爆破振动响应的差异则主要由爆破振动功率谱因子M(f)和爆破振动持续时间比值ts/tm 确定,其中M(f)由段数和延迟时间决定,ts/tm 的任意值均可通过一定的段数和延迟时间取得。为突出影响因素,且在实际爆破中段数和延迟时间相比于单段持续时间的应用更为常见,为此本文采用爆破参数段数和延迟时间作为纽带去分析比较单段和多段爆破振动频率特性和持续时间的差异性对结构响应的影响。下以一算例进一步描述该过程。图2所示为一典型多段爆破振动速度时程曲线,各段之间相互独立,爆破段数为8段、延迟时间大约为120 ms。振动峰值粒子速度位于第二段,为2.21 cm/s。单段爆破振动持续时间大约为60 ms,整个多段爆破振动持续时间大约为900 ms。取第二段和整个多段爆破振动进行对比,首先分析频谱特性,本文关于振动信号的频谱特性分析均采用功率谱密度ρe,见图3,单段爆破振动频谱结构简单,主频为31.0 Hz;多段爆破振动含多个优势频率,分别为16.6、24.9、33.0、40.8 Hz等,大约为8.3的倍数,且24.9 Hz和33.0 Hz频率成分占比最高。
再考虑单自由度系统爆破振动响应,设有两个自振频率分别为15.0和25.0 Hz的单自由度系统,阻尼比均为0.05。求出它们分别在单段和多段爆破振动下的速度响应功率谱,见图4。单段爆破振动的速度响应谱结构简单,频谱曲线单峰光滑,优势频率与单段爆破振动主频相近。多段爆破振动速度响应谱出现多个峰值,与多段爆破振动频谱类似,优势主频为16.6、24.9和33.0 Hz。特别地,当自振频率为15.0 Hz,响应谱主频为16.6 Hz;当自振频率为25.0 Hz时,响应谱主频为24.9 Hz。可见由于结构对不同频率成分的爆破振动选择放大作用,与结构自振频率相近的爆破振动频率成分将会使结构产生较大的响应。如表1所示,振动响应峰值上,当自振频率为15.0 Hz时,单段和多段的振动响应峰值分别为2.72和2.94 cm/s,多段要比单段大8%;当自振频率为25.0 Hz时,分别为4.11和5.72 cm/s,多段要比单段大39%。可以看到,当爆破振动优势频率接近自振频率时,多段爆破的振动响应更大,且接近自振频率的优势频率越高时,多段爆破振动响应越大。
表 1 单自由度系统的爆破振动速度响应特征值Table 1. Characteristic values of blasting vibration velocity response of single degree of freedom system自振频率/Hz 振动响应优势频率/Hz 振动响应峰值/(cm∙s−1) 单段 多段 单段 多段 15.0 30.0 16.6/24.9/33.0 2.72 2.94 25.0 28.8 24.9/33.0 4.11 5.72 2.2 振动响应影响因素分析与比较
单段和多段爆破振动在频率和持续时间上存在显著差异性,这种差异性使结构产生不同的振动响应,通过上节的分析,延迟时间和段数是影响爆破振动频率和持续时间的重要因素,也是毫秒延迟微差爆破设计中的两个重要参数,故以延迟时间和段数为纽带分析结构振动响应对爆破振动频率和持续时间的依赖性。
取图2中第二个单段爆破振动为子波,进行不同段数、不同延迟时间的线性叠加,得到段数分别为2、5和8,延迟时间分别为25和110 ms的多段爆破振动。
首先进行频谱分析,见图5。单段爆破振动频谱结构简单,主频为31.0 Hz。多段爆破振动频谱出现多个峰值,当延迟时间为25 ms时,见图5(a),多段爆破优势频率为40.0、80.0 Hz,其中主频为40.0 Hz,且随段数增加,优势频率处幅值越大;当延迟时间为110 ms时,见图5(b),多段爆破振动优势频率为18.2、27.3、36.4、45.5 Hz等,其中主频为27.3 Hz,同样,随段数增加,优势主频处幅值越大。那么,由此可见,无论高低延迟时间,多段爆破振动均将产生
fi=n/Δτ 的优势频率,且随段数增加,优势频率幅值越大,最接近单段爆破振动主频fm的优势频率成为主频。为进一步比较结构在单段和多段爆破振动作用下的响应峰值大小,可根据结构振动速度放大系数给出反应谱曲线,见图6。单段爆破振动反应谱结构简单,在37.0 Hz处有最大速度放大系数。多段爆破振动反应谱则在单段的基础上,在爆破振动优势频率附近存在多个峰值,其中当延迟时间为25 ms时,在40.0 Hz和80.0 Hz处有峰值;当延迟时间为110 ms时,在27.3、36.4 Hz等处有峰值。另外可看到,段数对峰值大小的影响,单段小于2段小于5、8段,5段和8段相差不大。
可以看到当多段爆破振动的优势频率接近结构自振频率时,将会产生比单段更大的结构响应;此外段数对上述影响仅在一定段数范围内有增强作用。
多段爆破振动优势频率受延迟时间控制,为明确结构振动响应对爆破振动频率的依赖性,这里同样采用上述子波信号进行不同延迟时间的线性叠加,模拟段数为10、延迟时间在10~100 ms内的不同多段爆破振动。为衡量多段相对于单段爆破振动对结构响应的增强作用,这里给出多段与单段爆破振动反应谱的比值和延迟时间的关系,见图7。
可以看到,当延迟时间倒数的整数倍(也即优势频率
fi=n/Δτ ,n∈Z+ )接近自振频率fn时,多段爆破振动会使结构振动响应显著增大。特别地,多段爆破的优势频率fi越接近自振频率fn、多段爆破的优势频率fi越接近单段主频fm,也即当fi→fn ,fi→fm 时,结构在多段爆破振动作用下将产生更大的结构振动响应。对于图7中所示例子,多段比单段的放大系数最大要高4倍以上。为进一步探讨爆破振动持续时间对结构振动响应的影响,这里给出多段与单段反应谱峰值的比值随爆破段数变化规律,见图8。可以看到,无论延迟时间多少,仅在段数较小时,段数增加使反应谱峰值比值增加,当段数增加到一定值时,段数的影响不大。对于此处的多段爆破振动,可认为当段数大于10段后,段数(也即爆破振动持续时间)的增加对结构动力响应的影响不大,但若考虑累计损伤和疲劳荷载的效应,爆破持续时间也不应设置过长。
为此在进行多段爆破振动安全评价时,除关注单段爆破振动主频fm外,多段的优势频率fi也不容忽视;由于优势频率fi跟延迟时间密切相关,所以在进行多段爆破参数设计时,延迟时间的设置应保证使优势频率fi远离保护对象自振频率。段数仅在一定范围内,随段数增加,结构振动响应有可能更大;但段数增加到一定值后,对结构振动响应的影响不大。
3. 实测地表爆破振动分析
3.1 试验概况
试验场地位于白鹤滩坝基保护层顶面EL.555.0 m~550.0 m梯段,光面爆破参数见表2。光爆孔多4孔一响,段间采用MS5接力,在爆区侧向布置地表测点,地表测点的传感器为三向传感器,爆破设计及测点布置示意见图9。
表 2 光面爆破参数Table 2. Parameters of smooth blasting钻孔类型 孔径/mm 孔深/mm 药卷直径/mm 孔距/m 单孔药量/kg 最大单响药量/kg 堵塞长度/m 光爆孔 76 10 32/70 0.6 2.2 13.2 1.0 3.2 结果分析
图10为实测爆破振动时程曲线,雷管延迟时间有误差,从实测波形图可知各段延迟时间大约为120 ms,8个单段相互独立,没有相互叠加。考虑到各段孔数不同,单响药量不同,各段峰值不同,为消除这种干扰,对各单段进行归一化处理,见图11。
为分析实测爆破振动频率对结构爆破振动响应的影响,这里分别取首段为单段、前4段和前8段(共8段)进行频谱和反应谱分析,见图12。首先分析单段与多段爆破振动的频谱特性,三个方向上多段爆破振动的功率谱均呈现出谱带现象,出现多个间隔为8.3 Hz的优势频率,8.3 Hz为实际延迟时间120 ms的倒数,且8段比4段谱带现象更为显著,在优势频率处峰值更大。其次在反应谱上,多段爆破振动的反应谱曲线在单段反应谱曲线上波动,在其优势频率
fi=n/Δτ 附近均有峰值,最大比单段要大1.6倍。为分析实测爆破振动持续时间对结构爆破振动响应的影响,这里给出多段反应谱峰值与单段的比值随段数变化关系,见图13,这里多段爆破振动分别取前2、3、···8段。可以看到,在z、y、z方向上,段数较小时,随段数增加,爆破振动持续时间增加,多段反应谱峰值增加,而分别当段数增加到4、5、3段后,多段反应谱峰值趋于稳定。
可以看到,尽管在这里单段和多段爆破振动峰值粒子速度相同,即便主频相近,结构响应也可能不同。显然若按照现有规范仅考虑峰值和所在单段视主频进行爆破振动评价,是不安全的。
由此可见,实测爆破振动结果很好地验证了理论推导,多段爆破振动频谱出现间隔8.3 Hz的谱带现象,8.3 Hz为实际延迟时间120 ms的倒数;且爆破段数越多,频带越显著。结构爆破振动响应主要受爆破振动频率的影响,在各优势频率
n/Δτ 处,结构速度响应放大系数均大于单段爆破振动;爆破段数仅在较小值时对结构响应有影响,当段数增加到一定值后,结构多段爆破振动响应与段数的关系不大。那么,爆破段数和延迟时间的设置,使爆破振动能量向n/Δτ 频率处集中,应避免使此频率接近结构的自振频率。4. 结 论
为明确结构爆破振动响应对频率和持续时间的依赖性,本文从频域角度给出单段爆破振动频谱、多段爆破振动频谱及结构物响应频谱的相互之间关系,以延迟时间和爆破段数作为纽带分析爆破振动频率和持续时间对结构爆破振动响应的影响。
(1)单段与多段爆破振动频率和持续时间的差异性,是使结构产生不同响应规律的重要原因,而延迟时间
Δτ 和段数是影响爆破振动频率和持续时间的重要因素。无论高延迟还是低延迟,均使多段爆破振动频谱产生间隔1/Δτ 的频带现象,出现优势频率fi=n/Δτ 。且随段数增加,频带现象更为显著,优势频率幅值增加。持续时间显然与延迟时间和段数均成正相关关系。(2)结构对于爆破振动频率的依赖性方面,由于结构对于不同频率成分的选择放大作用,爆破振动中接近自振频率的优势频率成分将使结构产生较大的响应。在反应谱上,多段爆破振动在其多个优势频率
n/Δτ 附近的结构放大系数均比单段大,其余处与单段相差不大。需要特别注意是,当优势频率fi=n/Δτ 、单段爆破振动主频fm和结构物自振频率fn三者相近时,即fi→fn ,fi→fm ,将可能产生最大的结构振动响应。(3)结构对于爆破振动持续时间的依赖性方面,当在段数较小时,段数增加使反应谱峰值增加,但当段数增加到一定值后时,反应谱峰值达到稳定值。随延迟时间的增加,达到稳定值所需的段数减小。对于本文的多段爆破振动可认为当段数大于10段后,段数(也即爆破振动持续时间)的增加对结构动力响应的影响不大,但若考虑累计损伤和疲劳荷载的效应,爆破持续时间也不应设置过长。
(4)通过对实测多段爆破振动进行频谱和反应谱分析,具有很明显的间隔
1/Δτ 频带现象,段数较小时,反应谱峰值随段数增加而增加。实测爆破振动基本吻合验证理论推导。文中关于多段爆破振动频谱和反应谱的讨论还有待于更多的现场实验验证,此外,还需针对各段不同、延迟时间不同工况下的情况分析。但研究的结论基本解释了结构爆破振动响应对爆破振动频率和持续时间的依赖性。 -
表 1 单自由度系统的爆破振动速度响应特征值
Table 1. Characteristic values of blasting vibration velocity response of single degree of freedom system
自振频率/Hz 振动响应优势频率/Hz 振动响应峰值/(cm∙s−1) 单段 多段 单段 多段 15.0 30.0 16.6/24.9/33.0 2.72 2.94 25.0 28.8 24.9/33.0 4.11 5.72 表 2 光面爆破参数
Table 2. Parameters of smooth blasting
钻孔类型 孔径/mm 孔深/mm 药卷直径/mm 孔距/m 单孔药量/kg 最大单响药量/kg 堵塞长度/m 光爆孔 76 10 32/70 0.6 2.2 13.2 1.0 -
[1] LU W B, LUO Y, CHEN M, et al. An introduction to Chinese safety regulations for blasting vibration [J]. Environmental Earth Sciences, 2012, 67(7): 1951–1959. DOI: 10.1007/s12665-012-1636-9. [2] SINGH P K, ROY M P. Damage to surface structures due to blast vibration [J]. International Journal of Rock Mechanics and Mining Sciences, 2010, 47(6): 949–961. DOI: 10.1016/j.ijrmms.2010.06.010. [3] 罗忆, 卢文波, 陈明, 等. 爆破振动安全判据研究综述 [J]. 爆破, 2010, 27(1): 14–22. DOI: 10.3963/j.issn.1001-487X.2010.01.004.LUO Yi, LU Wenbo, CHEN Ming, et al. View of research on safety criterion of blasting vibration [J]. Blasting, 2010, 27(1): 14–22. DOI: 10.3963/j.issn.1001-487X.2010.01.004. [4] KARADOGAN A, KAHRIMAN A, OZER U. A new damage criteria norm for blast-induced ground vibrations in Turkey [J]. Arabian Journal of Geosciences, 2014, 7(4): 1617–1626. DOI: 10.1007/s12517-013-0830-8. [5] ALDAS G G U. Explosive charge mass and peak particle velocity (PPV)-frequency relation in mining blast [J]. Journal of Geophysics & Engineering, 2010, 7(3): 223. DOI: 10.1088/1742-2132/7/3/001. [6] RAI R, SHRIVASTVA B K, SINGH T N. Prediction of maximum safe charge per delay in surface mining [J]. Mining Technology, 2005, 114(4): 227–231. DOI: 10.1179/037178405x84832. [7] 爆破安全规程: GB6722-2014 [S]. 北京: 中国标准出版社, 2014. [8] YANG J H, LU W B, JIANG Q H, et al. Frequency comparison of blast-induced vibration per delay for the full-face millisecond delay blasting in underground opening excavation [J]. Tunnelling & Underground Space Technology Incorporating Trenchless Technology Research, 2016, 51: 189–201. DOI: 10.1016/j.tust.2015.10.036. [9] BLAIR D P. The measurement, modelling and control of ground vibrations due to blasting [C] // Proceedings of the 2nd International Symposium on Rock Fragmentation by Blasting. Keystonse. Colorado, USA, 1987: 88−101. [10] BLAIR D P. Limitations of electronic delays for the control of blast vibration and fragmentation [C] // Proceedings of the 9th International Symposium on Rock Fragmentation by Blasting. Granada, Spain, 2009: 171−184. [11] RICHARDS A B, MOORE A J. Factors affecting frequency control of blast vibration [C] // WANG X. New Development on Engineering Blasting. Beijing, China: Metallurgical Industry Press, 2017: 305−310. [12] QIU X, SHI X, GOU Y, et al. Short-delay blasting with single free surface: results of experimental tests [J]. Tunnelling & Underground Space Technology, 2018, 74: 119–130. DOI: 10.1016/j.tust.2018.01.014. [13] 凌同华, 李夕兵. 多段微差爆破振动信号频带能量分布特征的小波包分析 [J]. 岩石力学与工程学报, 2005, 24(7): 1117–1122. DOI: 10.3321/j.issn:1000-6915.2005.07.005.LING Tonghua, LI Xibing. Analysis of energy distributions of millisecond blast vibration signals using the wavelet packet method [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(7): 1117–1122. DOI: 10.3321/j.issn:1000-6915.2005.07.005. [14] 赵明生, 张建华, 易长平. 基于单段波形叠加的爆破振动信号时频分析 [J]. 煤炭学报, 2010, 35(8): 1279–1282.ZHAO Mingsheng, ZHANG Jianhua, YI Changping. Time-frequency analysis based on single-stage addition of waveforms of blasting vibration signals [J]. Journal of China Coal Science, 2010, 35(8): 1279–1282. [15] 凌同华, 李夕兵, 王桂尧. 单段爆破震动的动态响应分析 [J]. 中南大学学报(自然科学版), 2007, 38(3): 551–554. DOI: 10.3969/j.issn.1672-7207.2007.03.032.LING Tonghua, LI Xibing, WANG Guiyao. Dynamic response analysis of single-interval-time in millisecond blast vibration [J]. Journal of Central South University (Science and Technology), 2007, 38(3): 551–554. DOI: 10.3969/j.issn.1672-7207.2007.03.032. [16] 陈士海, 魏海霞. 爆破地震波频率对多自由度弹性体系动力响应的影响分析 [J]. 振动与冲击, 2009, 28(11): 150–154.CHEN Shihai, WEI Haixia. Effect analysis of blasting seismic wave frequency on dynamic response of a multi-degrees of freedom elastic system [J]. Journal of Vibration and Shock, 2009, 28(11): 150–154. [17] 魏海霞. 爆破地震波作用下建筑结构的动力响应及安全判据研究 [D]. 济南: 山东科技大学, 2010: 56−78. [18] 李夕兵, 凌同华. 单段与多段微差爆破地震的反应谱特征分析 [J]. 岩石力学与工程学报, 2005, 24(14): 2409–2413. DOI: 10.3321/j.issn:1000-6915.2005.14.002.LI Xibing, LING Tonghua. Response spectrum analysis of ground vibration induced by single deck and multi-deck blasting [J]. Journal of Rock Mechanics and Engineering, 2005, 24(14): 2409–2413. DOI: 10.3321/j.issn:1000-6915.2005.14.002. [19] 李夕兵, 凌同华. 爆炸参量对爆破地震反应谱的影响 [J]. 爆炸与冲击, 2004, 24(5): 443–447. doi: 10.3321/j.issn:1001-1455.2004.05.011LI Xibing, LING Tonghua. Influence of explosion parameters on response spectrum for blast ground vibration [J]. Explosion and Shock Waves, 2004, 24(5): 443–447. doi: 10.3321/j.issn:1001-1455.2004.05.011 [20] DOWDING C H. Construction vibrations [M]. 2 ed. New Jersey: Prentice Hall Inc, 2000: 64−65. [21] ANIL K C. 结构动力学: 理论及其在地震工程中的应用 [M]. 4版. 谢礼立, 吕大刚, 译. 北京: 高等教育出版社, 2016: 36−37. [22] 姚端正, 梁家宝. 数学物理方法 [M]. 3版. 北京: 科学出版社, 2010: 168−169. [23] SISKIND D E, STAGG M S, KOPP J W, et al. Structure response and damage produced by ground vibration from surface mine blasting: report of investigation RI850-7 [R]. USA: Bureau of Mines, 1980. [24] 李洪涛, 舒大强, 卢文波, 等. 建筑物对爆破振动中不同频率能量成分的响应特征 [J]. 振动与冲击, 2010, 29(2): 154–158. DOI: 10.3969/j.issn.1000-3835.2010.02.035.LI Hongtao, SHU Daqiang, LU Wenbo, et al. Response characteristics of a structure to different frequency components in blasting vibration energy [J]. Journal of Vibration and Shock, 2010, 29(2): 154–158. DOI: 10.3969/j.issn.1000-3835.2010.02.035. 期刊类型引用(6)
1. 何理,殷琳,钟冬望,张鑫玥,赵永明,熊海涛,陈莎莎,NJAMBA Bruno. 爆破振动强度、波形与频谱研究综述:预测及主动控制. 爆破. 2024(03): 189-204+262 .  百度学术
百度学术2. 张文涛,汪海波,高朋飞,王梦想,吕闹,杨帆,程兵. 基于PCA-WOA-XGBoost的露天矿山爆破振动峰值振速预测. 工程爆破. 2024(06): 155-167+177 .  百度学术
百度学术3. 林之岳,黎俊华,王道林. 基于爆破振速演变规律的采场安全分析. 黄金. 2023(03): 5-11 .  百度学术
百度学术4. 王春华,陈洪凯. 危岩集合体激振特性试验研究. 华东交通大学学报. 2022(01): 12-18 .  百度学术
百度学术5. 吕江,赵晖,姚康,史吏. 隧洞爆破引起路堑高边坡振动的1/3倍频程实测分析. 浙江工业大学学报. 2021(03): 282-289 .  百度学术
百度学术6. 张彪. 孤石深孔爆破对临近竖井的振动影响研究. 爆破. 2021(02): 95-99 .  百度学术
百度学术其他类型引用(9)
-













 下载:
下载:

































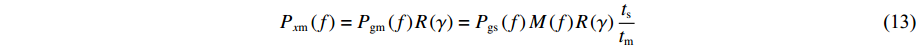






































 下载:
下载:












 百度学术
百度学术











