基于实测爆破振动计算岩体介质P波品质因子

杨招伟; 卢文波; 陈明; 严鹏; 胡英国; 刘美山; 吴新霞; 冷振东

doi:10.11883/bzycj-2019-0333

基于实测爆破振动计算岩体介质P波品质因子

doi: 10.11883/bzycj-2019-0333

1.
长江科学院水利部岩土力学与工程重点实验室，湖北武汉 430010
2.
武汉大学水资源与水电工程科学国家重点实验室，湖北武汉 430072
3.
中国葛洲坝集团易普力股份有限公司，重庆 401121

基金项目: 国家自然科学基金（51779190，51809016）

详细信息

作者简介:
杨招伟（1992－　），男，博士，yangzw@whu.edu.cn

通讯作者:
卢文波（1968－　），男，博士，教授，博士生导师，wblu@whu.edu.cn

中图分类号: O382
计量
- 文章访问数: 5074
- HTML全文浏览量: 1835
- PDF下载量: 70
- 被引次数: 11
出版历程
- 收稿日期: 2019-08-27
- 修回日期: 2019-11-17
- 刊出日期: 2020-06-01

Calculation of P wave quality factor of rock mass based on measured blasting vibrations

1.
Key Laboratory of Geotechnical Mechanics and Engineering of Ministry of Water Resources, Changjiang River Scientific Research Institute, Wuhan 430010, Hubei, China
2.
State Key Laboratory of Water Resources and Hydropower Engineering Science, Wuhan University, Wuhan 430072, Hubei, China
3.
China Gezhouba Grope Explosive Co. Ltd., Chongqing 401121, China

摘要

摘要: 爆破地震波传播衰减研究对爆破振动预测及安全控制有着重要的指导意义。针对爆破振动实测信号，结合P波、S波的初至识别结果，计算P波、S波在岩体中的传播速度及P波的上升时间，进一步得出岩体介质P波品质因子。结合现场实测振动数据，计算丰宁抽水蓄能电站及舟山绿色石化基地试验区域内岩体介质P波品质因子，计算结果分别为19.02和14.07。结果表明，通过实测地表爆破振动计算得到的P波品质因子远小于经验公式的计算值及一般原岩的品质因子，说明地表疏松层对爆破地震波的传播衰减有较大影响。
- 爆破试验 /
- 爆破振动 /
- 波速 /
- 上升时间 /
- 品质因子
Abstract: Studies on the attenuating characteristic of blasting seismic waves in propagating process are important in prediction and control of blasting vibration effects by engineering blasting. Field blasting tests of single-hole were conducted to study the quality factor of rock mass. The wave propagation velocities and the pulse rise times can be obtained by using the first arrival of P wave and S wave. Finally, based on the rise time method, the P wave quality factor of rock mass can be calculated. By analyzing the measured blasting vibration signals in Fengning hydropower station and Zhoushan large petrochemical industry, the average P wave quality factors in the above two regions are found to be 19.02 and 14.07, respectively. The experimental results show that the values derived by measuring blasting vibrations are far less than the values predicted by the empirical formulas and measured by the original rock mass. This results indicate that the soft covering layer has great influence on the seismic waves propagation induced by blasting.
- blast field tests /
- blasting vibration /
- propagation velocity /
- pulse rise time /
- quality factor

HTML全文

爆破开挖是人类工程生产尤其是岩土工程开挖过程中不可替代的重要手段，已广泛应用于水利工程、城市铁路、地下隧道工程等众多重大工程建设领域。随着爆破作业环境及岩石条件的复杂化，爆破振动造成的边坡和民房破坏屡有发生、损失惨重，使得爆破过程中的振动安全控制成为突出问题^[1-3]。因此，爆破地震波的传播衰减研究对指导爆破施工设计、爆破振动预测与控制都有重要意义。

多年来，爆破振动传播衰减的研究工作备受关注。李彰明等^[4]结合生产爆破振动信号及现场原位测试数据，通过回归分析给出了边坡应力波的衰减规律，并进一步探讨了相关的应用问题；李洪涛等^[5]基于地震学相关理论，对爆破地震波能量进行了分析，得出爆破地震波能量随距离的衰减系数为振动衰减系数的约2倍，并通过相关工程实际应用验证了结论的合理性；范勇等^[6]、周俊汝等^[7]、毕卫国等^[8]、叶根喜等^[9]也分别通过现场试验对爆破地震波在岩体中的传播特性及衰减特征进行了研究，为爆破地震波的衰减研究提供了理论参考。

在地震学领域，地震波的衰减常用品质因子描述。当前，品质因子的研究成果较为丰富：Frankel等^[10]通过尾波归一化方法，对纽约、南非等地区壳构造高频剪切波的衰减开展了大量的研究，并给出了对应区域内品质因子的参考值；Chung等^[11]通过扩展尾波归一化方法，利用实测地的震动数据对Yangsan断裂带品质因子进行了系统研究，并给出了不同频带品质因子的计算方法；Yoshimoto等^[12]、Jongmans等^[13]、Wang等^[14]也针对地震波的衰减作了大量工作，不过上述关于品质因子与地震波衰减的研究大多归于地震领域几百千米大尺度概念上的分析。爆破地震波不同于天然地震，因其震源堆在地表浅层，能量衰减较快。目前，在工程爆破方面：刘学伟等^[15]在充分考虑噪声干扰因素的基础上，提出了一种基于实测振动信号的地表风化层品质因子的反演计算方法，取得了较好的计算结果；卢文波等^[16]通过对典型单孔爆破试验实测振动信号的具体分析，提出了一种确定地震波中P波、S波与R波在岩体介质中传播衰减参数的方法；彭府华等^[17]通过现场爆破试验，主要针对中尺度特复杂岩体介质中应力波传播速度模型、应力波衰减特性与岩体品质因子等问题进行研究，为微震监测的评价研究工作提供了理论基础。上述研究工作只是将振动波形当作单一类型波进行分析，由于地震波中体波（P波、S波）及面波（R波）为不同类型波，它们在传播过程中的分量衰减特性相差往往较大，迄今，针对爆破地震波中不同波的衰减特性的研究较少。因此，迫切需要开展爆破地震波中不同波的衰减规律研究。

本文中以丰宁抽水蓄能电站及舟山绿色石化基地现场爆破试验为例，对爆破地震波在岩体介质传播过程中P波的品质进行分析研究，为爆破振动的准确预测及安全控制提供理论依据。

1. 计算理论

由于爆破开挖区有水工建筑物、水利设施和民居等，爆破施工作业环境复杂，在采用监测仪器对爆破地震波导致的地面振动进行监测的同时，必须掌握爆破地震波在不同岩体条件下的一些基本特性，以便达到指导爆破施工设计及爆破振动预测与控制的目的。本文中就不同岩体介质下地震波的传播特性，如传播波速和品质因子等开展现场试验及相关研究工作。

1.1 爆破地震波波速

爆破振动监测点布置方案如图1所示，各振动传感器的爆心距分别为R₁、R₂···R_n，根据杨招伟等^[18]的研究，识别出监测点振动信号的P波、S波的初至时间分别为t_1P、t_1S，t_2P、t_2S，···，t_n_P、t_n_S。其中，t_n_P为监测点n的P波初至时间，t_n_S为监测点n的S波初至时间。

图 1 爆破振动监测点布置

Figure 1. Arrangement of monitoring points of blast vibration

下载: 全尺寸图片幻灯片

由图1可知，定义 $R$ 为爆破地震波传播的任意距离，在这一传播距离内，P波、S波初至时间间隔为 $\Delta {t_{\rm{PS}}}$ ，则P波、S波的传播波速计算表达式为：

$\Delta {t_{\rm{PS}}} = R\left(\frac{1}{{{v_{\rm{S}}}}} - \frac{1}{{{v_{\rm{P}}}}}\right)$

(1)

式中： $\Delta {t_{\rm{PS}}} = {t_{\rm{S}}} - {t_{\rm{P}}}$ ，v_P、v_S分别为岩体介质中P波、S波的传播波速。

1.2 岩石品质因子

由于传播介质的非均质和非完全弹性的性质，爆破地震波在传播过程中能量被介质吸收，导致地震波的振幅随传播距离的增大而衰减。为了定量描述地震波传播过程中介质的吸收能力，引入了一个无量纲的因子Q，称为介质的品质因子。

通常将Q定义为一个振动周期内振动所损耗的能量与总振动能量的比值的倒数^[19-20]，表示为：

$\frac{1}{Q} = \frac{1}{{2{\text{π}} }}\frac{{\Delta E}}{E}$

(2)

式中： $\Delta E$ 、 $E$ 分别为振动过程中一个周期所损耗的能量与该周期内能量的最大值。

品质因子的测定方法可以分为室内试验及野外测定两大类，其中室内试验方法主要有驻波振动法、行波法及缓慢的应力周期法。在岩样取样过程中原始环境的改变及岩体本身的尺寸效应，测试结果不能很好地表示现场岩体状况，而现场爆破振动信号可间接反映岩体的物理力学特性及其结构特征，并且爆破振动监测点的布置范围较广。因此，可利用爆破实测振动信号测定岩体的品质因子。

本文中提出一种根据实测振动数据计算岩体P波品质因子的改进方法，该方法在上升时间法的基础上，结合P波、S波初至时间的判定结果，通过识别出的P波波形来计算P波上升时间及波速，最终求得P波品质因子。

上升时间法由Gladwin等^[21]提出，他们利用该方法研究了岩体介质的衰减特性。在此基础上，Kjartansson^[22]在恒定品质因子的前提下证明了该方法的有效性，进一步从理论上论证了利用上升时间法与地震波传播时间测定品质因子Q的可行性。

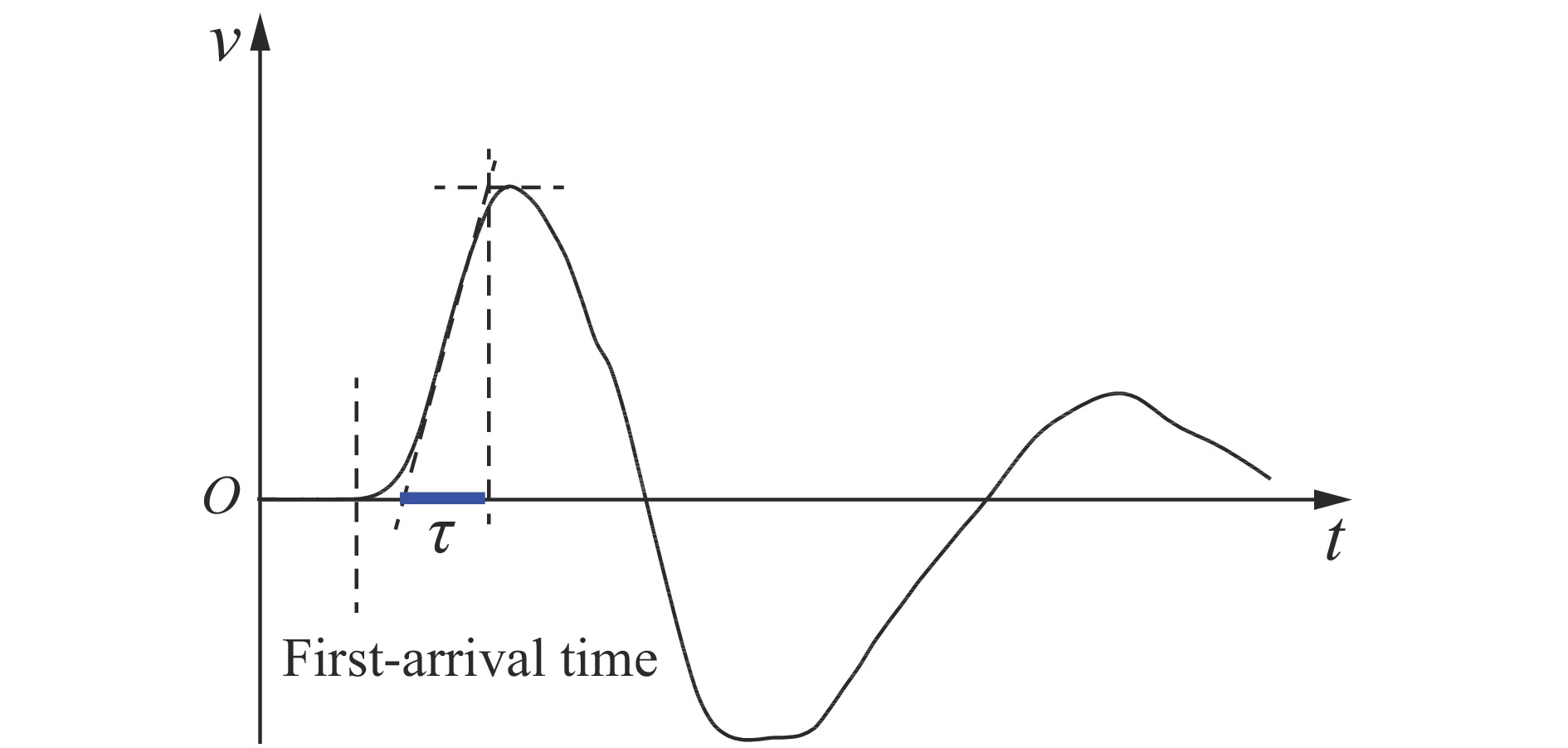
上升时间法建立于地震波在岩体介质传播过程中发生频散的基础上，主要通过上升时间和旅行时间来定义与品质因子Q的关系。根据Gladwin等^[21]的研究，上升时间被定义为实测爆破振动波形第一周期中最大峰值与最大斜率的比值，如图2所示。上升时间、地震波的旅行时间与品质因子三者之间的关系可表示为：

图 2 上升时间计算

Figure 2. Calculation of the rise time

下载: 全尺寸图片幻灯片

$\tau = {\tau _0} + \frac{{at}}{Q}$

(3)

式中： $\tau$ 为监测点监测信号上升时间； ${\tau _0}$ 为震源信号上升时间；a为常数，a ≈ 0.53±0.04； $t$ 为爆破地震波旅行时间，t = x/v，其中x为爆心距，v为爆破地震波波速。因此，式（3）还可表示为：

$\tau = {\tau _0} + \frac{{ax}}{{Qv}}$

(4)

由文献[23]，式（3）中a值往往与震源参数密切相关，当传播介质相同时，不同类型震源使用同样的 $a$ 值往往会给Q的计算带来较大差异。为了解决这个问题，Kavetsky等^[23]引入了脉冲有效波长的概念：

$\lambda = kv\tau$

(5)

式中：λ为有效波长；v为地震波波速；由于 $\tau \approx \dfrac {1} {4} T$ ，其中T为振动周期，因此k ≈ 4。

联合式（2）～（5），可得传播过程中振幅为：

$A(x) = {A_0}{\left(1 + \frac{{ax}}{{Qv{\tau _0}}}\right)^{ - {\text{π}} /(ka)}}$

(6)

地震波在传播过程中，除了考虑因介质材料的不完全弹性导致的衰减以外，还应考虑在传播过程中随着传播距离的增大而使波阵面增大导致的几何衰减，因此，在式（6）的基础上，引入几何阻尼 ${x^{ - n}}$ （其中 $n = 0,\;0.5,\;1$ ，分别代表平面波、柱面波和球面波），则式（6）可表示为：

$A(x) = {A_0} {x^{- n}} {\left(1 + \frac{{ax}}{{Qv{\tau _0}}}\right)^{ - {\text{π}} /(ka)}}$

(7)

对式（7）两边取对数可得：

$\ln ({x^n}A(x)) = \ln {A_0} + \frac{{\text{π}} }{{ka}}\ln {\tau _0} - \frac{{\text{π}} }{{ka}}\ln \tau$

(8)

由式（8）可知， $\ln ({x^{ - n}}A(x))$ 与 $\ln \tau$ 线性相关，根据不同爆心距测点处的 $A(x)$ 、 $\tau (x)$ 可拟合得出与品质因子无关的斜率 $- {{\text{π}} }/{{(ka)}}$ ，进一步可求得常数a，进而根据式（4）可求得岩体介质品质因子Q。

通过分析监测点振动传感器获取的地面爆破振动波形，可以很快推知地震波在岩体介质内的平均传播波速，并且能对该区域内岩体介质的品质因子大小作出预报。

2. 实测分析

为了验证上述方法的可行性，同时也为了对爆破地震波的衰减有更加直观和深入的认识，选取两次现场爆破试验的实测振动信号进行分析。

2.1 丰宁抽水蓄能电站垂直孔爆破试验

2.1.1 工程概况

丰宁抽水蓄能电站位于河北省丰宁满族自治县境内，工程分两期开挖建设。二期工程爆破施工时，爆破诱发的振动不可避免会对一期工程建（构）筑物产生一定的负面影响。为了更好地控制爆破振动，了解爆破地震波的传播衰减特性，利用二期地下厂房探洞开挖的时机和条件，在探洞内部合适区域开展了竖直孔爆破试验，爆破试验现场及仪器布置如图3所示。本次爆破试验共布置有6个竖直炮孔，孔径均为76 mm，各孔间用半秒雷管相连，逐孔起爆，详细孔网参数如表1所示。试验过程中采用TC-4850爆破振动智能监测仪（见图3）对爆破振动进行监测，沿测线在爆心距20 ～150 m处共布有6个振动监测点，如图4所示。振动波形明显分为6段，分别为6个炮孔爆破产生。

表 1 竖直钻孔爆破实验参数表

Table 1. Parameters of blasting design of the field experiment

炮孔	起爆位置	孔径/mm	孔深/cm	药卷直径/mm	装药长度/cm	堵塞长度/cm	单响药量/kg
1	上、底部	76	800	50	600	200	12.0
2	底部	76	800	50	600	200	12.0
3	中部	76	600	50	420	180	8.4
4	底部	76	600	50	420	180	8.4
5	中部	76	450	50	270	180	5.4
6	底部	76	450	50	270	180	5.4

下载: 导出CSV

| 显示表格

图 3 爆破试验现场及仪器布置

Figure 3. Filed tests and arrangement of monitoring points

下载: 全尺寸图片幻灯片

图 4 炮孔及振动测点布置（单位：m）

Figure 4. Arrangement of testing points and blasting holes (Unit: m)

下载: 全尺寸图片幻灯片

2.1.2 地震波传播波速

地震波在岩体中传播的波速往往不是一个定值，而常常与岩体介质本身、岩体裂隙和所包含的节理面等因素有关。经地质勘探可知，该试验区域岩体主要以花岗岩为主，总体质量较好，因此在求取波速过程中，将该区域内岩体假设为一均质岩体。由1.1节可知，计算波速前，应首先完成对P波、S波的识别工作，杨招伟等^[18]对P波、S波进行了识别并给出了实测振动波形中S波的识别结果，识别效果如图5所示，P波、S波的识别结果如表2～3 所示。

表 2 实测振动波形P波初至识别结果

Table 2. P wave first arrivals identification results

炮孔	P波初至震相到时/ms
炮孔	1#	2#	3#	4#	5#	6#
1	429.25	−0.38	−0.63	−0.63	−1.25	−8.25
2	897.13	467.50	467.00	467.13	466.50	459.13
3	1 531.50	1 101.88	1 101.13	1 101.13	1 100.25	1 093.25
4	1 628.75	1 199.13	1 198.38	1 198.50	1 197.00	1 190.63
5	2 583.13	2 153.50	2 153.00	2 153.13	2 153.13	2 145.00
6	3 053.00	2 623.25	2 622.63	2 622.75	2 621.13	2 614.75

下载: 导出CSV

| 显示表格

表 3 实测振动波形S波初至识别结果

Table 3. S wave first arrivals identification results

炮孔	S波初至震相到时/ms
炮孔	1#	2#	3#	4#	5#	6#
1	431.63	3.25	5.50	8.75	12.13	11.25
2	899.50	471.13	473.13	476.50	479.88	478.63
3	1 533.63	1 105.13	1 107.00	1 110.25	1 113.38	1 112.50
4	1 630.88	1 202.50	1 204.25	1 207.50	1 210.13	1 209.88
5	2 585.00	2 156.50	2 158.50	2 161.88	2 164.00	2 164.13
6	3 054.88	2 626.38	2 628.25	2 631.63	2 634.00	2 633.75

下载: 导出CSV

| 显示表格

图 5 实测典型爆破振动时程曲线

Figure 5. Measured blasting vibration velocity curves

下载: 全尺寸图片幻灯片

将表2～3中P波、S波初至时间数据代入式（1）中，可求得该试验区域内P波、S波的平均传播波速为v_P=5 170.2 m/s，v_S=3 072.3 m/s。中国水电顾问集团北京勘测设计研究院工程地质报告所推荐的纵波波速范围为4 640 ～5 650 m/s，对比可知，由实测爆破振动波形反演计算所得P波、S波的传播波速介于设计院推荐波速，可认为该方法计算得到的传播波速值是合理的。

2.1.3 品质因子计算

众所周知，地震波在岩体介质传播过程中分为体波（P波、S波）和面波（R波），且相对于S波和R波，P波的传播速度更快。因此，在传感器监测到的振动波形中，一般是P波最先到达，其后才出现S波、R波等各种续至波形。由此可知，如果在振动波形第一周期内最大峰值出现前，这些续至波没有到达，则上升时间仅反映P波携带的信息。基于此，可对P波品质因子进行相关研究。结合表3中S波初至识别结果，选取符合上述要求的测点用于本研究。由于受篇幅所限，本文选取各传感器监测到起爆方式相同（底部起爆）的2、4、6号炮孔爆破所产生的水平径向振动波形进行整理分析。

根据图2给出的上升时间定义对所监测的振动波形计算上升时间，各振动监测波形的上升时间计算结果如表4所示。其中，v_max为实测振动波形第一周期中的最大峰值。

表 4 各测点上升时间计算结果

Table 4. Calculating results of rise time for various monitoring points

炮孔	3#测点		4#测点		5#测点		6#测点
炮孔	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)
2	0.55	2.51	0.59	1.30	0.83	0.75	1.18	0.23
4	0.53	2.20	0.64	1.05	0.75	0.60	1.08	0.16
6	0.80	1.24	0.64	0.58	0.79	0.34	1.09	0.09

下载: 导出CSV

| 显示表格

主要对P波品质因子进行分析，式（8）可改写为：

$\ln (xA(x)) = \ln {A_0} + \frac{{\text{π}} }{{ka}}\ln {\tau _0} - \frac{{\text{π}} }{{ka}}\ln \tau$

(9)

表4中的上升时间与各测点第一周期峰值及对应爆心距（见图4）按照式（9）进行拟合，拟合结果如图6所示。由式（9）可知， $\ln (xA(x))$ 与 $\ln \tau$ 线性相关，且斜率为 $- {{\text{π}}}/{{(ka)}}$ ，由图6可知：

图 6

$\ln (xA(x))$ 与

$\ln \tau$ 线性拟合结果

Figure 6. Results of linear regression between

$\ln (xA(x))$ and

$\ln \tau$

下载: 全尺寸图片幻灯片

$- \frac{{\text{π}} }{{ka}} = - 1.32$

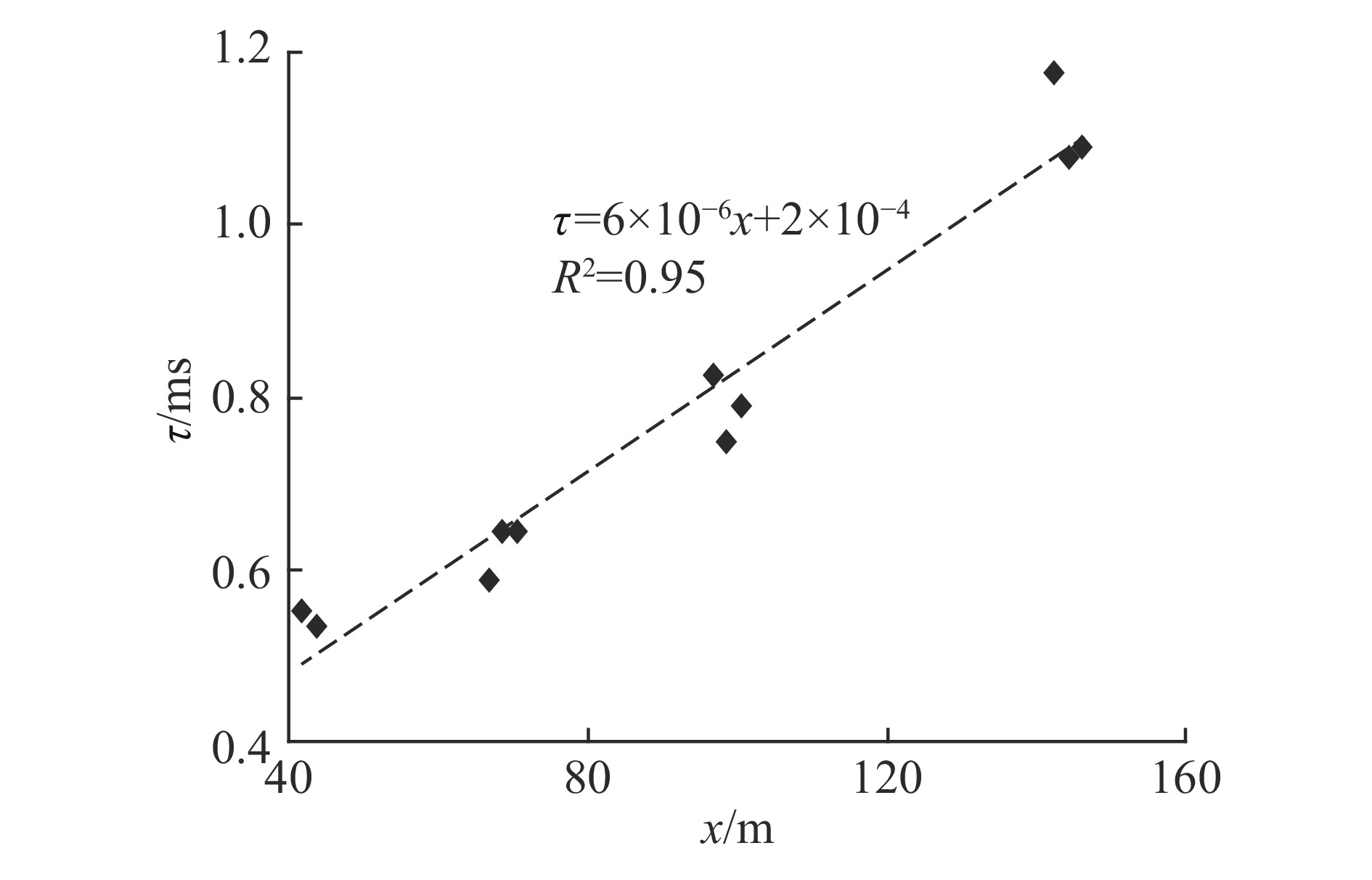
所以对该试验P波爆源，有 $a = 0.59$ 。

将表4中的上升时间与爆心距数据按照式（4）进行线性拟合，拟合结果如图7所示。由图7可知， $\tau (x)$ 与爆心距 $x$ 之间的斜率为 $\dfrac{a}{{{Q_{\rm{P}}}{v_{\rm{P}}}}} = 6 \times {10^{ - 6}}$ ，可求得该区域的P波的岩体品质因子 ${Q_{\rm{P}}} = 19.02$ 。

图 7 上升时间

$\tau$ 与爆心距

$x$ 线性拟合

Figure 7. Results of linear regression between

$\tau$ and

$x$

下载: 全尺寸图片幻灯片

2.2 舟山绿色石化基地单孔试验

2.2.1 工程概况

舟山绿色石化基地为“十三五”期间我国重点打造的七大世界级石化基地之一。基地分两期开挖建设，一期工程已经开始部分土建及基地配套设施设备的安装工作。因此二期土石方爆破开采时的爆破振动不可避免会对一期工程在建建筑物、设施设备及施工人员产生一定的负面影响。为了解该项目爆破振动衰减特性，准确预测爆破振动，在二期施工区域42 m平台进行了一次单孔爆破试验。该试验区域内岩体以流纹质含角砾玻屑熔结凝灰岩为主，区域内构造简单，纵横向变化小。

试验区布置1个炮孔，孔深10 m，孔径115 mm，药卷直径90 mm，堵塞段长度5 m，孔内采用双发雷管起爆，现场钻孔、装药及测点布置如图8所示，炮孔装药结构如图8（d）所示。为了监测爆破振动信号，该次爆破振动测试共布置5台TC-4850（1#～5#测点）及8台Blast-UM（6#～13#测点）型爆破测振仪（图8（b）），为了避免不同测振仪器对振动监测的影响，本文只分析由Blast-UM型爆破监测仪测得的振动信号。其中6#、8#测点典型振动时程曲线如图9所示。

图 8 单孔爆破试验现场

Figure 8. Field photographs of blasting tests

下载: 全尺寸图片幻灯片

图 9 典型测点振动曲线

Figure 9. Typical measured curves of blasting vibration velocity versus time

下载: 全尺寸图片幻灯片

2.2.2 地震波传播波速

10#、11#测点没有获得有效的爆破振动数据，7#测点由于现场施工条件影响，布置位置偏离测线，因此对其余5个Blast-UM型测振仪监测点进行P波、S波初至识别分析，识别结果如表5所示。将表5中P波、S波初至时间的数据代入式（1）中，可求得该试验区域内P波和S波的平均传播波速为v_P=5 442.0 m/s、v_S=2 908.9 m/s。

表 5 实测振动波形P波、S波初至识别结果

Table 5. Identification results of P and S wave first arrivals

测点	爆心距/m	初至时间/ms
测点	爆心距/m	P波	S波
6#	23.6	18.90	22.67
8#	40.0	18.70	25.11
9#	54.6	18.70	27.44
12#	160.9	18.90	44.65
13#	225.6	18.60	54.71

下载: 导出CSV

| 显示表格

2.2.3 品质因子计算

对该次单孔爆破试验所监测的振动波形计算上升时间，各振动监测波形的上升时间计算结果如表6所示。将表6中各测点的上升时间与第一周期峰值结合表5中对应爆心距按式（9）进行拟合，拟合结果如图10所示。

表 6 各测点上升时间计算结果

Table 6. Calculating results of rise times for various monitoring points

测点	$\tau$ /ms	v_max/(cm·s⁻¹)
6#	1.54	7.18
8#	1.65	2.63
9#	1.70	1.32
12#	2.61	0.30
13#	2.50	0.19

下载: 导出CSV

| 显示表格

图 10

$\ln (xA(x))$ 与

$\ln \tau$ 线性拟合结果

Figure 10. Results of linear regression between

$\ln (xA(x))$ and

$\ln \tau$

下载: 全尺寸图片幻灯片

由式（9）可知， $\ln (xA(x))$ 与 $\ln \tau$ 线性相关，且斜率为 $- {{\text{π}}}/{{(ka)}}$ ，由图10可知：

$- \frac{{\text{π}} }{{ka}} = - 2.05$

所以对该单孔试验P波爆源，有 $a = 0.38$ 。

将表6中的上升时间与爆心距数据按式（4）进行线性拟合，拟合结果如图11所示。由图11可知， $\tau (x)$ 与爆心距 $x$ 之间的斜率为 $\dfrac{a}{{{Q_{\rm{P}}}{v_{\rm{P}}}}} =$ 5×10⁻⁶，可求得该区域内P波的岩体品质因子为 ${Q_{\rm{P}}} = 14.07$ 。

图 11 上升时间

$\tau$ 与爆心距

$x$ 线性拟合

Figure 11. Results of linear regression between

$\tau$ and

$x$

下载: 全尺寸图片幻灯片

通过对以上两个工程的现场实测振动数据分析计算可知：丰宁抽水蓄能电站试验区域内岩体的纵波平均传播波速为5 170.2 m/s，横波平均传播波速为3 072.3 m/s；舟山绿色石化基地试验区域内岩体的纵波平均传播波速为5 442.0 m/s，横波平均传播波速为2 908.9 m/s；根据本文方法计算得到两地的P波岩体品质因子分别为19.02与14.07。李庆忠^[24]通过对各种岩性岩体实测数据给出品质因子与纵波波速之间的经验公式为 $Q = 14 v_{\rm{P}}^{2.2}$ ，根据该经验求得两工程岩体品质因子约500，远大于根据实测振动信号反演得到的P波品质因子。这种差异主要归因于上述经验公式主要是从深层固结状态好的岩体中得到的结论，而在工程爆破领域，爆源和爆破振动监测点主要分布于地表，浅层地表疏松层对爆破地震波的传播影响比深埋岩体大得多，地震波的吸收衰减明显增大。因此，根据实测爆破振动信号反演品质因子值明显小于计算的经验值。

3. 结　论

结合丰宁抽水蓄能电站和舟山绿色石化基地现场爆破试验，对爆破地震波传播过程中P波的岩体品质因子进行分析和计算，得出以下主要结论。

（1）根据地表实测爆破振动波形能够准确地反演地震波在岩体中的平均传播波速，以上两工程的纵波平均传播波速分别为5 170.2 m/s和5 442.0 m/s，横波平均传播波速分别为3 072.3 m/s和2 908.9 m/s；该方法监测范围广，为爆破振动衰减规律的研究提供了重要的参数。

（2）通过以上两工程的现场爆破试验实测振动数据反演得到P波岩体品质因子分别为19.02与14.07，远小于对以上两种岩性品质因子的常规认识，说明地表疏松层对地震波的传播有着极大的影响，地震波的衰减明显增大。需要说明的是，本文中对P波岩体品质因子的研究仅仅是爆破振动衰减研究课题的第一步，为了更深入地研究和解释爆破地震波在岩体介质中的传播规律，更好地预测和控制爆破振动，需进一步开展R波及S波的岩体品质因子的相关研究。

图 1 爆破振动监测点布置

Figure 1. Arrangement of monitoring points of blast vibration

下载: 全尺寸图片幻灯片

图 2 上升时间计算

Figure 2. Calculation of the rise time

下载: 全尺寸图片幻灯片

图 3 爆破试验现场及仪器布置

Figure 3. Filed tests and arrangement of monitoring points

下载: 全尺寸图片幻灯片

图 4 炮孔及振动测点布置（单位：m）

Figure 4. Arrangement of testing points and blasting holes (Unit: m)

下载: 全尺寸图片幻灯片

图 5 实测典型爆破振动时程曲线

Figure 5. Measured blasting vibration velocity curves

下载: 全尺寸图片幻灯片

图 6 $\ln (xA(x))$ 与 $\ln \tau$ 线性拟合结果

Figure 6. Results of linear regression between $\ln (xA(x))$ and $\ln \tau$

下载: 全尺寸图片幻灯片

图 7 上升时间 $\tau$ 与爆心距 $x$ 线性拟合

Figure 7. Results of linear regression between $\tau$ and $x$

下载: 全尺寸图片幻灯片

图 8 单孔爆破试验现场

Figure 8. Field photographs of blasting tests

下载: 全尺寸图片幻灯片

图 9 典型测点振动曲线

Figure 9. Typical measured curves of blasting vibration velocity versus time

下载: 全尺寸图片幻灯片

图 10 $\ln (xA(x))$ 与 $\ln \tau$ 线性拟合结果

Figure 10. Results of linear regression between $\ln (xA(x))$ and $\ln \tau$

下载: 全尺寸图片幻灯片

图 11 上升时间 $\tau$ 与爆心距 $x$ 线性拟合

Figure 11. Results of linear regression between $\tau$ and $x$

下载: 全尺寸图片幻灯片

表 1 竖直钻孔爆破实验参数表

Table 1. Parameters of blasting design of the field experiment

炮孔	起爆位置	孔径/mm	孔深/cm	药卷直径/mm	装药长度/cm	堵塞长度/cm	单响药量/kg
1	上、底部	76	800	50	600	200	12.0
2	底部	76	800	50	600	200	12.0
3	中部	76	600	50	420	180	8.4
4	底部	76	600	50	420	180	8.4
5	中部	76	450	50	270	180	5.4
6	底部	76	450	50	270	180	5.4

下载: 导出CSV

表 2 实测振动波形P波初至识别结果

Table 2. P wave first arrivals identification results

炮孔	P波初至震相到时/ms
炮孔	1#	2#	3#	4#	5#	6#
1	429.25	−0.38	−0.63	−0.63	−1.25	−8.25
2	897.13	467.50	467.00	467.13	466.50	459.13
3	1 531.50	1 101.88	1 101.13	1 101.13	1 100.25	1 093.25
4	1 628.75	1 199.13	1 198.38	1 198.50	1 197.00	1 190.63
5	2 583.13	2 153.50	2 153.00	2 153.13	2 153.13	2 145.00
6	3 053.00	2 623.25	2 622.63	2 622.75	2 621.13	2 614.75

下载: 导出CSV

表 3 实测振动波形S波初至识别结果

Table 3. S wave first arrivals identification results

炮孔	S波初至震相到时/ms
炮孔	1#	2#	3#	4#	5#	6#
1	431.63	3.25	5.50	8.75	12.13	11.25
2	899.50	471.13	473.13	476.50	479.88	478.63
3	1 533.63	1 105.13	1 107.00	1 110.25	1 113.38	1 112.50
4	1 630.88	1 202.50	1 204.25	1 207.50	1 210.13	1 209.88
5	2 585.00	2 156.50	2 158.50	2 161.88	2 164.00	2 164.13
6	3 054.88	2 626.38	2 628.25	2 631.63	2 634.00	2 633.75

下载: 导出CSV

表 4 各测点上升时间计算结果

Table 4. Calculating results of rise time for various monitoring points

炮孔	3#测点		4#测点		5#测点		6#测点
炮孔	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)	$\tau$ /ms	v_max/(cm·s⁻¹)
2	0.55	2.51	0.59	1.30	0.83	0.75	1.18	0.23
4	0.53	2.20	0.64	1.05	0.75	0.60	1.08	0.16
6	0.80	1.24	0.64	0.58	0.79	0.34	1.09	0.09

下载: 导出CSV

表 5 实测振动波形P波、S波初至识别结果

Table 5. Identification results of P and S wave first arrivals

测点	爆心距/m	初至时间/ms
测点	爆心距/m	P波	S波
6#	23.6	18.90	22.67
8#	40.0	18.70	25.11
9#	54.6	18.70	27.44
12#	160.9	18.90	44.65
13#	225.6	18.60	54.71

下载: 导出CSV

表 6 各测点上升时间计算结果

Table 6. Calculating results of rise times for various monitoring points

测点	$\tau$ /ms	v_max/(cm·s⁻¹)
6#	1.54	7.18
8#	1.65	2.63
9#	1.70	1.32
12#	2.61	0.30
13#	2.50	0.19

下载: 导出CSV

参考文献(24)

[1]	卢文波, 赖世骧, 朱传云, 等. 三峡工程岩石基础开挖爆破震动控制安全标准 [J]. 爆炸与冲击, 2001, 21(1): 67–71. LU W B, LAI S X, ZHU C Y, et al. Safety standards of blast vibrations adopted in rock base excavation of the three gorge project [J]. Explosion and Shock Waves, 2001, 21(1): 67–71.
[2]	YAN P, ZOU Y, ZHOU J, et al. Assessment of seismic impact on residences during blasting excavation of a large-scale rock slope in China [J]. Environmental Earth Sciences, 2016, 75(11): 949. DOI: 10.1007/s12665-016-5747-6.
[3]	王振宇, 梁旭, 陈银鲁, 等. 基于输入能量的爆破震动安全评价方法研究 [J]. 岩石力学与工程学报, 2010, 29(12): 2492–2499. WANG Z Y, LIANG X, CHEN Y L, et al. Study of safety evaluation method of blasting vibration based on input energy [J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(12): 2492–2499.
[4]	李彰明, 冯强. 岩质边坡中应力波衰减规律探讨 [J]. 岩石力学与工程学报, 1996, 15(S1): 460–463. LI Z M, FENG Q. Study on attenuation law of stress wave for rock slopes [J]. Chinese Journal of Rock Mechanics and Engineering, 1996, 15(S1): 460–463.
[5]	李洪涛, 卢文波, 舒大强, 等. 爆破地震波的能量衰减规律研究 [J]. 岩石力学与工程学报, 2010, 29(S1): 3364–3369. LI H T, LU W B, SHU D Q, et al. Study of energy attenuation law of blast-induced seismic wave [J]. Chinese Journal of Rock Mechanics and Engineering, 2010, 29(S1): 3364–3369.
[6]	范勇, 卢文波, 杨建华, 等. 深埋洞室开挖瞬态卸荷诱发振动的衰减规律 [J]. 岩土力学, 2015, 36(2): 541–549. DOI: 10.16285/j.rsm.2015.02.033. FAN Y, LU W B, YANG J H, et al. Attenuation law of vibration induced by transient unloading during excavation of deep caverns [J]. Rock and Soil Mechanics, 2015, 36(2): 541–549. DOI: 10.16285/j.rsm.2015.02.033.
[7]	周俊汝, 卢文波, 张乐, 等. 爆破地震波传播过程的振动频率衰减规律研究 [J]. 岩石力学与工程学报, 2014, 33(11): 2171–2178. DOI: 10.13722/j.cnki.jrme.2014.11.002. ZHOU J R, LU W B, ZHANG L, et al. Attenuation of vibration frequency during propagation of blasting seismic wave [J]. Chinese Journal of Rock Mechanics and Engineering, 2014, 33(11): 2171–2178. DOI: 10.13722/j.cnki.jrme.2014.11.002.
[8]	毕卫国, 石崇. 爆破振动速度衰减公式的优化选择 [J]. 岩土力学, 2004, 25(S1): 99–102. BI W G, SHI C. Optimization selection of blasting vibration velocity attenuation formulas [J]. Rock and Soil Mechanics, 2004, 25(S1): 99–102.
[9]	叶根喜, 姜福兴, 郭延华, 等. 煤矿深部采场爆破地震波传播规律的微震原位试验研究 [J]. 岩石力学与工程学报, 2008, 27(5): 1053–1058. DOI: 10.3321/j.issn:1000-6915.2008.05.022. YE G X, JIANG F X, GUO Y H, et al. Experiment research on seismic wave attenuation by field microseismic monitoring in deep coal mine [J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27(5): 1053–1058. DOI: 10.3321/j.issn:1000-6915.2008.05.022.
[10]	FRANKEL A, MCGARR A, BICKNELL J, et al. Attenuation of high-frequency shear waves in the crust: measurements from New York state, south Africa, and southern California [J]. Journal of Geophysical Research Solid Earth, 1990, 95(B11): 17441–17457. DOI: 10.1029/JB095iB11p17441.
[11]	CHUNG T W, SATO H. Attenuation of high-frequency P and S waves in the crust of southeastern south Korea [J]. Bulletin of the Seismological Society of America, 2001, 91(6): 1867–1874. DOI: 10.1785/0120000268.
[12]	YOSHIMOTO K, SATO H, OHTAKE M. Frequency-dependent attenuation of P and S waves in the Kanto area, Japan, based on the coda-normalization method [J]. Geophysical Journal International, 1993, 114(1): 165–174. DOI: 10.1111/j.1365-246X.1993.tb01476.x.
[13]	JONGMANS D, CAMPILLO M. The determination of soil attenuation by geophysical prospecting and the validity of measured Q, values for numerical simulations [J]. Soil Dynamics and Earthquake Engineering, 1993, 12(3): 149–157. DOI: 10.1016/0267-7261(93)90042-p.
[14]	WANG Q C, GAO J C, ZHENG S H, et al. Attenuation of ground motion in central and north area of north China [J]. Journal of Disaster Pnevention and Mitigation Engineering, 2004, 24(3): 313–319.
[15]	刘学伟, 邰圣宏, 何樵登. 一种考虑噪声干扰的地表风化层Q值反演方法 [J]. 石油地球物理勘探, 1996, 31(3): 367–373. LIU X W, TAI S H, HE J D. Weathered-layer Q-value inversion method with consideration of noise [J]. Oil Geophysical Prospecting, 1996, 31(3): 367–373.
[16]	卢文波, 董振华, 朱传云. 爆破地震波传播过程中衰减参数的确定 [J]. 工程爆破, 1997, 3(4): 12–16. LU W B, DONG Z H, ZHU C Y. Calculating of attenuation parameters of rock mass during the propagation of blasting seismic wave [J]. Engineering Blasting, 1997, 3(4): 12–16.
[17]	彭府华, 李庶林, 程建勇, 等. 中尺度复杂岩体应力波传播特性的微震试验研究 [J]. 岩土工程学报, 2014, 36(2): 312–319. DOI: 10.11779/CJGE201402007. PENG F H, LI S L, CHENG J Y, et al. Experimental study on characteristics of stress wave propagation in mesoscale and complex rock mass by microseismic monitoring [J]. Chinese Journal of Geotechnical Engineering, 2014, 36(2): 312–319. DOI: 10.11779/CJGE201402007.
[18]	杨招伟, 卢文波, 高启栋, 等. 爆破地震波中S波识别方法及其应用 [J]. 爆炸与冲击, 2018, 38(1): 28–36. DOI: 10.11883/bzycj-2017-0178. YANG Z W, LU W B, GAO Q D, et al. A S-wave phase picking method for blasting seismic waves and its application in engineering [J]. Explosion and Shock Waves, 2018, 38(1): 28–36. DOI: 10.11883/bzycj-2017-0178.
[19]	FUTTERMAN W I. Dispersive body waves [J]. Journal of Geophysical Research, 1962, 67(13): 5279–5291. DOI: 10.1029/JZ067i013p05279.
[20]	陈顒, 黄庭芳, 刘恩儒. 岩石物理学[M]. 合肥: 中国科技大学出版社, 2009: 64–68.
[21]	GLADWIN M T, STACEY F D. Anelastic degradation of acoustic pulses in rock [J]. Physics of the Earth and Planetary Interiors, 1974, 8(4): 332–336. DOI: 10.1016/0031-9201(74)90041-7.
[22]	KJARTANSSON E. Constant Q-wave propagation and attenuation [J]. Journal of Geophysical Research: Solid Earth, 1979, 84(B9): 4737–4748. DOI: 10.1029/JB084iB09p04737.
[23]	KAVETSKY A, CHITOMBO G P F, MCKENZIE C K, et al. A model of acoustic pulse propagation and its application to determine Q, for a rock mass [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1990, 27(1): 33–41. DOI: 10.1016/0148-9062(90)92891-H.
[24]	李庆忠. 走向精确勘探的道路[M]. 北京: 石油工业出版社, 1994: 31–39.

施引文献

期刊类型引用(5)

1.	孔凌浩，严鹏，刘晓，周朝，张翔宇，卢文波. 爆破地震波中P波及S波初至自动识别方法. 振动与冲击. 2025(05): 253-262 . 百度学术
2.	马明星，李剑，臧丹枫，曾援，郭陈莉. 基于衰减层析的地下浅层震动能量场重建方法. 电子测量技术. 2024(03): 42-47 . 百度学术
3.	苏银银，韩体飞，刘广鹏，刘赛，姜鑫. 减震沟爆破降振机理的试验研究. 现代矿业. 2024(03): 71-75 . 百度学术
4.	孙鹏昌. 城区单薄山体岩石高边坡爆破振动高程效应研究. 工程爆破. 2024(03): 152-159 . 百度学术
5.	魏海霞，祝杰，杨小林，褚怀保. 高压气体爆破作用下层状岩体的地表振动效应预测方法. 振动与冲击. 2023(20): 1-11 . 百度学术

其他类型引用(6)

资源附件(0)

访问统计

图(11) / 表(6)

计量

文章访问数: 5074
HTML全文浏览量: 1835
PDF下载量: 70
被引次数: 11

1. 计算理论
1.1 爆破地震波波速
1.2 岩石品质因子
2. 实测分析
2.1 丰宁抽水蓄能电站垂直孔爆破试验
2.2 舟山绿色石化基地单孔试验
3. 结　论

基于实测爆破振动计算岩体介质P波品质因子

doi: 10.11883/bzycj-2019-0333

作者简介: 杨招伟（1992－ ），男，博士，yangzw@whu.edu.cn

通讯作者: 卢文波（1968－ ），男，博士，教授，博士生导师，wblu@whu.edu.cn

计量

出版历程

Calculation of P wave quality factor of rock mass based on measured blasting vibrations

1. 计算理论

1.1 爆破地震波波速

1.2 岩石品质因子

2. 实测分析

2.1 丰宁抽水蓄能电站垂直孔爆破试验

2.1.1 工程概况

2.1.2 地震波传播波速

2.1.3 品质因子计算

2.2 舟山绿色石化基地单孔试验

2.2.1 工程概况

2.2.2 地震波传播波速

2.2.3 品质因子计算

3. 结 论

期刊类型引用(5)

其他类型引用(6)

计量

出版历程

目录

1. 计算理论

1.1 爆破地震波波速

1.2 岩石品质因子

2. 实测分析

2.1 丰宁抽水蓄能电站垂直孔爆破试验

2.2 舟山绿色石化基地单孔试验

3. 结 论

作者简介:
杨招伟（1992－　），男，博士，yangzw@whu.edu.cn

通讯作者:
卢文波（1968－　），男，博士，教授，博士生导师，wblu@whu.edu.cn

3. 结　论

3. 结　论