Discussion on essences of static resistance of two types of material under penetration
-
摘要: 以空腔膨胀理论为主要理论工具，通过比较侵彻近区塑性材料和脆性材料动力学行为的差异，对两类不同材料静阻力（Rt）的本质进行探讨，并对脆性材料侵彻的若干应用问题提出建议。研究表明：（1）Rt是靶体介质以固体特性抵抗局部扩孔、具有时间平均特性的弹体横截面平均应力，其具体取值随着材料的物理力学特性、侵彻模型、撞击速度等因素而变化，因此不是材料的固有特性。（2）对于塑性靶体的非变形侵彻问题，静态空腔膨胀理论的结果能够对Rt作出比较合理的预测；对于拟流体侵彻问题，一般需要对静态空腔膨胀理论的结果加以修正。（3）脆性材料的Rt主要取决于破碎后介质的力学特性而与完整材料的力学特性关系不大，且与单轴抗压强度之间不满足纯粹的单调关系；当侵彻速度较低时，应考虑侵彻速度对侵彻阻力的强化作用，这种强化作用的本质是内摩擦；当侵彻速度足够高时，脆性材料体现出恒定不变的“动力硬度”，其反映了材料的本征阻力特性。（4）提高脆性材料的侵彻阻力的关键在于减小应力波峰值后环向拉应力的幅值、抑制材料的破碎速度和程度，具体措施包括主动或被动地增加外围压、对基质中添加增韧增强纤维等；为了实现对脆性材料侵彻问题更高精度的数值模拟，建议更加重视对破碎介质动力学特性的研究。Abstract: Based on cavity expansion theories, the very essences of static target resistance, i.e. Rt of plastic and brittle materials are discussed by comparing the difference of dynamic behaviors in near region of penetration, and some suggestions about applications of brittle materials for penetration are given. The summary of discussion is shown as follows. Firstly, Rt is the mean and time-averaged stress on the cross section of the projectile, which is the resistance of target materials in solid state against local cavity expanding. The specific value of Rt varies withphysical and mechanical properties of materials, penetration models, impact velocity and other factors, and thus is not an intrinsic property of material. Secondly, for the non-deformable projectile penetrating plastic materials, static cavity expansion theory is proper to predict Rt. For semi-hydrodynamic penetration cases, the results of static cavity expansion theory should be modified. Thirdly, Rt of brittle materials mainly depends on fractured materials, while it is weakly related to intact materials and not completely positively related to uniaxial compressive strength of intact materials. If penetration velocity is relatively low, the strengthening effect of Rt of brittle materials by penetration velocity increasing should be considered in terms of internal-friction. If penetration velocity is high enough, the intrinsic and constant resistance of brittle materials is realized, which is named as dynamic hardness. Fourthly, the key measures to increase Rt of brittle materials are to reduce the amplitude of hoop tensile stress following the peak compressive stress, to lower the crack velocity of materials and to restrain the fragmentation degree of materials. These can be solved by increasing external confining pressure and intensifying the tensile strength and fracture toughness of materials. Furthermore, it is suggested that the dynamic properties of fractured materials should be emphasized to increase the precision of numerical calculations of brittle materials under penetration.
-
混凝土类材料已被广泛应用于民用建筑及防御工事[1-3]，它在爆炸冲击载荷作用下的动态力学性能和破坏机理，对于民用建筑及防御工事的设计和防护具有重要指导意义[4-6]，目前一般采用SHPB技术来研究不同应变率下混凝土类材料的动态力学性能[7-9]。混凝土作为一种脆性材料，其破坏应变非常小，通常只有千分之几，而动态力学性能实验的加载时间又非常短，产生的高应变率往往会引起试件的结构破坏而非材料破坏，从而对SHPB技术在混凝土材料中的应用提出了挑战[10-12]。Frew等[13]针对波形整形技术，在脆性材料SHPB实验中的应用展开研究, 认为应力均匀性问题相对比较容易解决, 同时指出恒应变率加载却很难实现。卢玉斌等[14]对于脆性材料SHPB实验中实现近似恒应变率加载的必要性进行了讨论，认为恒应变率加载实现难度较大。陶俊林等[15]提出了两种SHPB方法来实现对金属材料的恒应变率加载，但对于混凝土等脆性材料仍很难实现恒应变率加载。周子龙等 [16]认为加载应力和试样应力历程具有相同的变化规律时，试样变形将处于恒应变率状态，但是对于如何实现恒应变率加载并没有给出明确的方法。由于在SHPB实验中很难实现混凝土试件的恒应变率加载[17-19]，部分学者通过采用变截面杆[20]、波形整形技术[21-22]以及异形冲头法[23]等对输入波形进行了优化，从而实现了中低应变率下的恒应变率加载，但是目前已有的方法仍很难实现混凝土试件在较高应变率下的恒应变率加载。因此，如何处理非恒应变率加载下的实验数据，并确定其对应的应变率，就成为了一个迫切需要解决的问题。
为了确定混凝土类材料在SHPB实验中所对应的应变率，本文中对3种不同强度和4种不同钢纤维含量的混凝土试件进行SHPB实验，并针对实验得到的30组恒应变率加载下的实验数据进行归纳总结，探索一种混凝土类材料SHPB实验中确定应变率的方法，拟为处理非恒应变率加载下的实验数据并确定其对应的应变率提供合理的解决方法。
1. 试 件
试件为3种不同强度和4种不同钢纤维含量的混凝土。3种不同强度混凝土材料配比见表1，利用设计模具浇铸，尺寸为
∅ 70 mm×35 mm。试件养护过程符合工程要求，并利用磨床对两端面进行研磨，其不平行度在0.02 mm以内，试件最终长径误差在±0.02 mm。4种不同钢纤维含量混凝土试件的制作采用二次合成法工艺，基体强度为C40，坍落度为160～200 mm。采用∅ 70 mm×35 mm的圆柱钢模浇筑并振动成型，拆模后，室温条件标准养护28 d，实验时混凝土龄期为80 d以上。钢纤维采用0213型微钢纤，纤维直径d=0.2 mm、长度l=13 mm（特征比l/d=65，外形平直光洁）。钢纤维混凝土的实际配合比见表2。表 1 不同强度混凝土试件配合比Table 1. Mixture ratio of concrete specimen with different strengths混凝土 石子/(kg·m−3) 砂/(kg·m−3) 水/(kg·m−3) 水泥/(kg·m−3) 水灰比 外加剂/(kg·m−3) 28 d 抗压强度/MPa C20 1 100 725 205 350 0.586 7.5 23.05 C45 950 900 173 500 0.346 12.5 46.20 C70 1 150 600 150 600 0.250 16.0 70.06 表 2 不同钢纤维含量混凝土试件配合比Table 2. Mixture ratio of steel fiber reinforced concrete specimen混凝土 钢纤维体积含量/% 钢纤维/(kg·m−3) 石子/(kg·m−3) 砂/(kg·m−3) 水/(kg·m−3) 水泥/(kg·m−3) 水胶比 外加剂/(kg·m−3) C40 0 0 1 090 698 158 391 0.38 8.1 0.75 58.5 1 056 676 182 450 0.38 8.1 1.50 117.0 1 004 645 204 505 0.38 8.1 4.50 351.0 726 470 280 693 0.38 8.1 2. 实 验
实验在
∅ 74 mm的SHPB实验装置上完成，实验装置如图1所示。通过贴在入射杆和投射杆中部的应变片采集入射波εi、反射波εr和透射波εt，计算试样的动态平均应力σ、应变ε和应变率˙ε ：σ=EAAsεt (1) ε=−2c0L∫t0εrdt (2) ˙ε=−2c0Lεr (3) 式中：c0为压杆的弹性波速，L为试件的长度，A为压杆的横截面积，As为试件的横截面积，E为压杆的弹性模量。
由式（3）可知，试样变形的应变率由反射应力波确定，即应变率和反射波波形呈线性关系，因此在SHPB实验中实现恒应变率加载可以由在有效加载时间内在反射应力波上得到的一个近似恒定平台表征[24]。
3. 恒应变率加载
选取直径20 mm、厚度1.0～2.5 mm的黄铜材料作为波形整形器。为了得到恒应变率加载的实验数据，通过采用不同几何尺寸的黄铜波形整形器对不同强度（C20，C45，C70）和不同钢纤维含量（0%，0.75%，1.50%，4.50%）的混凝土进行了大量SHPB实验，获得的典型波形如图2所示。
图 2 不同强度和不同钢纤维含量的混凝土材料的典型波形Figure 2. Typical waveforms for concrete-like materials with different strengths and different fiber contentsSHPB实验主要基于两个基本假定：一维假定和均匀性假定。Ravichandran等[25]认为应力波在试件中传播3个来回，试件可近似达到应力均匀状态。3个来回的时间为Δt=6L/c，波速
c=√E/ρ ，ρ为试件的密度。以图2（b）中C45混凝土的典型波形为例，对应力与应变率曲线进行分析，其中L=35 mm、E=33.5 GPa、ρ=2 530 kg/m3，经计算波速c=3 700 m/s，Δt=57 μs，即试件在57 μs的时候已经达到应力均匀状态, 而此时对应的应力还不到峰值应力的15%。由图3可以看出，反射波脉冲上升沿作用时间为92 μs，即试件达到恒应变率加载段需要的时间为92 μs，该时刻对应的应力为峰值应力的50%，说明通过黄铜整形器得到的实验数据实现了混凝土材料的恒应变率加载并满足加载过程中的应力均匀性假定。图4分别给出了30组恒应变率实验数据中C20、C70和C40 (0.75%)混凝土试件在相近恒应变率加载下的应力应变曲线对比，从图中可以看出，实验数据重复性较好。
图 4 相近恒应变率加载下的应力应变曲线对比Figure 4. Comparison of stress-strain curves under similar constant strain rate loading4. 非恒应变率加载下实验数据修正系数的提出
由于SHPB实验中很难实现混凝土类材料的恒应变率加载，非恒应变率加载下的实验数据居多。为了处理非恒应变率加载下的实验数据，陶俊林等[26]采用应变率时程曲线的算数平均值来表征恒应变率；李为民等[27]采用应变率时程曲线的波头至应力峰值之间的算数平均值来表征恒应变率；Wang等[28]认为，应变率时程曲线可以取80%至峰值应变率之间为恒应变率加载段。确定应变率方法的不同，将导致同一组实验数据所对应的应变率的确定具有较强的主观性，从而为研究混凝土类材料的应变率效应带来误差，因此如何合理地确定混凝土类材料SHPB实验中的应变率尤为重要。
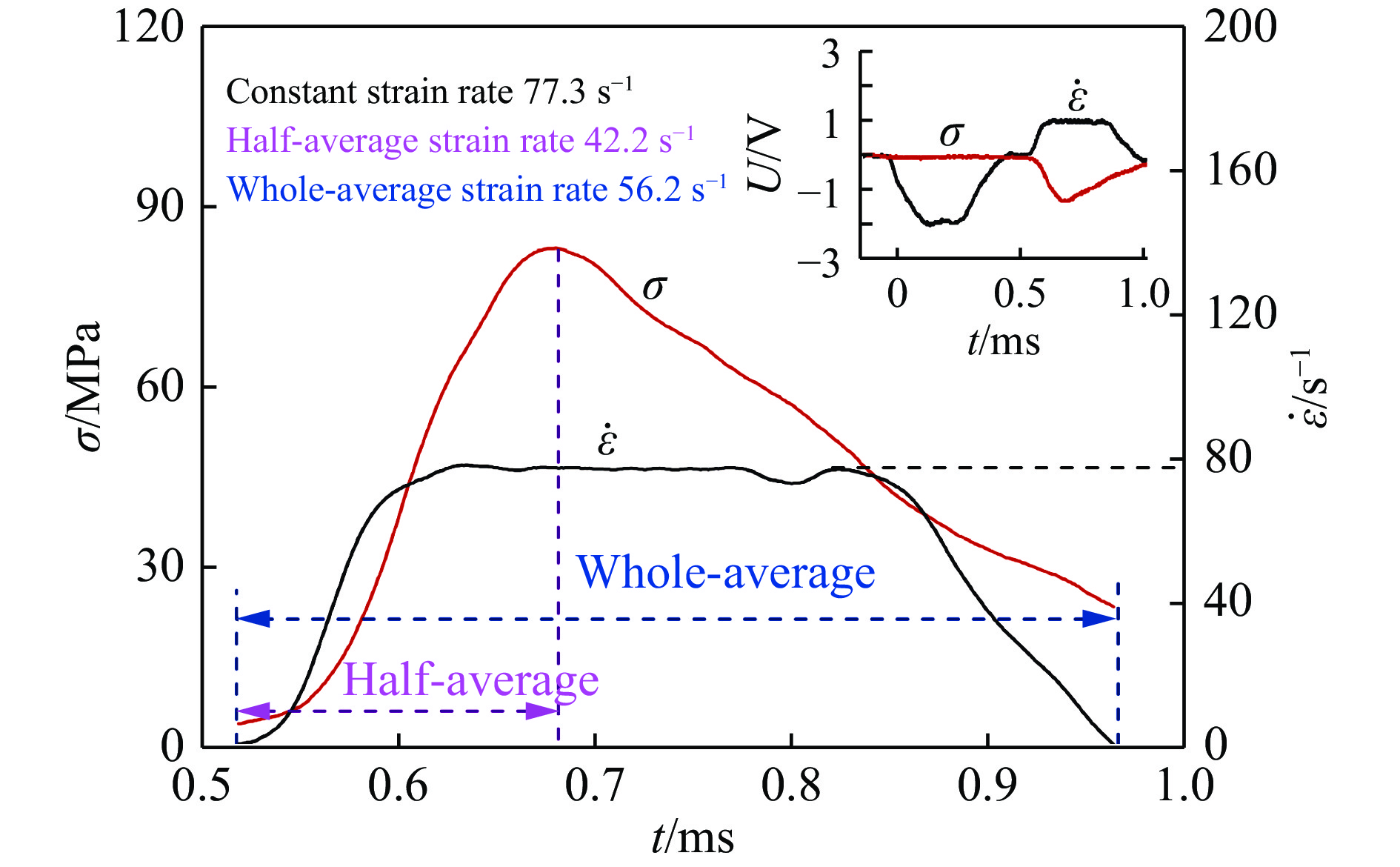
下面，将通过使用不同应变率选取方法来处理恒应变率加载下的实验数据，通过对比不同选取方法得到的应变率数值，探寻不同确定应变率方法之间是否存在一定的规律。图5为其中一组恒应变率加载下的实验数据，其对应的原始波形为图2(b)。如图5中所示，其实验数据对应的平台段恒应变率为77.3 s−1；半段平均应变率选取的是波头至应力峰值这个时间段内应变率算数平均值，通过计算得到的半段平均应变率为42.2 s−1，整段应变率平均值选取的是曲线波头至波尾这个段曲线内算数平均值，通过计算得到全段平均应变率为56.2 s−1。因此，可得恒应变率/半段平均应变率η1=77.3/42.2=1.832，而恒应变率/全段平均应变率η2=77.3/56.2=1.375。
对30组恒应变率加载下的应变率曲线进行同样的处理，得到这些曲线对应的恒应变率、半段平均应变率、全段平均应变率，并求得恒应变率/半段平均应变率以及恒应变率/全段平均应变率，见表3。图6对恒应变率/半段平均应变率和恒应变率/全段平均应变率进行了汇总，从图中可以发现，恒应变率/全段平均应变率基本保持在约1.38，而恒应变率/半段平均应变率浮动比较大，没有一定的规律。
表 3 恒应变率加载下的实验数据Table 3. Experimental data at constant strain rate loading实验 试件 恒应变率/s−1 半段平均应变率/s−1 全段平均应变率/s−1 η1 η2 1 C20 32.6 24.6 24.5 1.325 1.331 2 C20 44.2 34.4 31.9 1.285 1.386 3 C20 50.4 31.0 37.9 1.613 1.319 4 C20 51.2 28.6 45.1 2.182 1.384 5 C20 65.3 41.0 46.8 1.593 1.395 6 C20 77.2 55.1 54.9 1.401 1.406 7 C20 81.2 57.1 57.0 1.422 1.424 8 C45 37.3 23.9 25.8 1.561 1.446 9 C45 62.5 39.5 45.2 1.582 1.383 10 C45 70.3 51.4 51.5 1.368 1.365 11 C45 77.3 42.2 56.2 1.832 1.375 12 C45 80.7 60.1 62.5 1.343 1.291 13 C70 45.4 32.8 33.3 1.384 1.363 14 C70 53.6 38.7 41.8 1.385 1.282 15 C70 57.7 42.7 42.4 1.328 1.337 16 C70 69.4 56.1 50.2 1.237 1.382 17 C70 77.4 55.4 51.7 1.397 1.503 18 C70 87.1 60.1 65.6 1.449 1.328 19 C40 59.2 43.0 40.8 1.377 1.451 20 C40 66.8 33.0 49.5 2.024 1.349 21 C40 82.1 54.3 55.6 1.512 1.477 22 C40 (0.75%) 62.0 31.3 45.0 1.981 1.378 23 C40 (0.75%) 80.7 64.8 59.2 1.245 1.363 24 C40 (0.75%) 81.2 51.3 59.4 1.583 1.367 25 C40 (1.50%) 59.1 43.4 44.6 1.362 1.325 26 C40 (1.50%) 65.4 51.8 48.2 1.262 1.357 27 C40 (1.50%) 81.2 46.0 58.6 1.765 1.386 28 C40 (4.50%) 34.5 26.2 24.6 1.317 1.402 29 C40 (4.50%) 82.3 62.1 60.1 1.325 1.369 30 C40 (4.50%) 105.3 62.6 78.9 1.682 1.334 
图 6 恒应变率与半段（全段）平均应变率之比Figure 6. Constant strain rate versus average strain rate of half and whole-stage由此可以得到结论：对于恒应变率加载下的实验数据，其对应的应变率可以采用反射波平台段数值直接得到，也能够采用全段平均应变率乘以系数1.38来确定，并把全段平均应变率乘以系数1.38定义为等效应变率。由于把等效应变率确定为实验曲线所对应的应变率的方法是由恒应变率加载下的实验数据得到的，它对于非恒应变率加载下的实验数据是否适用还未进行有效验证。下面对非恒应变率加载下的实验数据采用该确定应变率的方法进行处理，得到该实验数据对应的等效应变率，并与相近数值恒应变率加载下的实验数据进行对比。图7为恒应变率和非恒应变率加载下的应力应变曲线的对比图，图中右上角为实验数据分别对应的应变率曲线的加载情况。
图 7 非恒应变率加载与相近恒应变率加载下的应力应变曲线对比Figure 7. Comparison of stress-strain curves under non-constant strain rate loading (NCSRL) and similar constant strain rate loading (CSRL)由图7可以看出，如果非恒应变率加载下的等效应变率和恒应变率加载下的恒应变率差相近，则两者对应的应力应变曲线重合度比较高。因此，采用全段平均应变率乘以系数1.38来表示实验数据所对应的等效应变率是完全可行的，并且该方法对于处理不同强度和不同钢纤维含量的混凝土材料在SHPB实验中的数据都适用。
5. 反射波时程曲线平台段较短的实验数据对应应变率的确定
目前，一般认为实验数据的反射波时程曲线存在平台段，即可认为是恒应变率加载，因此Wang等[28]把反射波时程曲线只有较短平台段的实验数据认为是恒应变率加载的。由于没有对平台段长度到底多长才算恒应变率的相关报道，因此把反射波时程曲线只存在较短平台段的实验数据认为是恒应变率加载的合理性还有待进步一考究。本文中结合前文所得到的方法，对反射波时程曲线只有较短平台段类型的数据进行处理，图8为C45混凝土某个反射波时程曲线只有较短平台段类型的一组实验数据的原始波形，其反射波时程曲线的平台段对应的应变率为72.6 s−1，而采用全段平均应变率乘以系数1.38得到的等效应变率为63.4 s−1。图9给出了C45混凝土5组恒应变率加载下的实验数据，与其进行对比。从图9可以看出，等效应变率为63.4 s−1的较短平台段类的应力应变曲线与恒应变率为62.5 s−1的实验数据重合度较好，与恒应变率为70.3 s−1的实验数据差异较大。因此，反射波时程曲线平台段较短的实验数据对应应变率不能直接采用平台段对应的应变率来表征，而应该采用本文中提出的全段平均应变率乘以系数1.38的等效应变率来表征。假如实验数据得到的反射波时程曲线平台段的长度不是太长，那么采用本文中所提的确定应变率的方法会更合理些。
图 9 C45混凝土在不同加载情况下的应力应变曲线对比Figure 9. Comparison of stress-strain curves of C45 concrete under different loading conditions6. 结 论
采用直径20 mm、厚度1.0～2.5 mm的黄铜材料作为波形整形器，针对不同强度（C20，C45，C70）和不同钢纤维含量（0%，0.75%，1.50%，4.50%）的
∅ 70 mm×35 mm混凝土材料进行了SHPB实验，得到以下结论。(1) 通过采用不同尺寸的黄铜材料作为波形整形器，能够得到低应变率下的恒应变率加载实验数据，但是很难得到高应变率下的恒应变率加载实验数据。
(2) 通过对实验得到的30组恒应变率加载下数据进行归纳总结，提出了混凝土类材料SHPB实验数据所对应的应变率可以采用全段平均应变率的1.38倍来表征的确定应变率的方法。
(3) 对比非恒应变率加载下的等效恒应变率和恒应变率加载下的恒应变率的数值相近的实验数据，由于其对应的应力应变曲线重合度非常高，从而表明采用本文中确定应变率的方法对于非恒应变率加载的实验数据是适用的。
(4) 反射波时程曲线平台段较短的实验数据不能视为恒应变率加载，其对应的应变率可以采用本文中确定应变率的方法来确定。
-
图 1 塑性材料中球形空腔膨胀的响应区域
Figure 1. Response regions of spherical cavity expansion in plastic materials
图 2 脆性材料中球形空腔膨胀的响应区域
Figure 2. Response regions of spherical cavity expansion in brittle materials
图 8 花岗岩和混凝土靶体Rt随撞击速度的变化规律
Figure 8. Rt of granite and concrete varying with impact velocity
-
[1] ANDERSON C E. Analytical models for penetration mechanics: a review [J]. International Journal of Impact Engineering, 2017, 108: 3–26. DOI: 10.1016/j.ijimpeng.2017.03.018. [2] FORRESTAL M J, LUK V K. Dynamic spherical cavity-expansion in a compressible elastic-plastic solid [J]. Journal of Applied Mechanics, 1988, 55(2): 275–279. DOI: 10.1115/1.3173672. [3] HILL R. The mathematical theory of plasticity [M]. Oxford: Oxford University Press, 1998. [4] FORRESTAL M J, TZOU D Y. A spherical cavity-expansion penetration model for concrete targets [J]. International Journal of Solids and Structures, 1997, 34(31/32): 4127–4146. [5] FORRESTAL M J, ALTMAN B S, CARGILE J D, et al. An empirical equation for penetration depth of ogive-nose projectiles into concrete targets [J]. International Journal of Impact Engineering, 1994, 15(4): 395–405. DOI: 10.1016/0734-743X(94)80024-4. [6] HE T, WEN H M, GUO X J. A spherical cavity expansion model for penetration of ogival-nosed projectiles into concrete targets with shear-dilatancy [J]. ActaMechanicaSinica, 2011, 27(6): 1001–1012. [7] 彭永, 方秦, 吴昊, 等. 对弹体侵彻混凝土靶体阻力函数计算公式的探讨 [J]. 工程力学, 2015, 32(4): 112–119.PENG Y, FANG Q, WU H, et al. Discussion on the resistance forcing function of projectiles penetrating into concrete targets [J]. Engineering Mechanics, 2015, 32(4): 112–119. [8] KONG X Z, WU H, FANG Q. Rigid and eroding projectile penetration into concrete targets based on am extended dynamic cavity expansion model [J]. International Journal of Impact Engineering, 2017, 100: 13–22. DOI: 10.1016/j.ijimpeng.2016.10.005. [9] 卢正操, 张元迪, 文鹤鸣, 等. 长杆弹侵彻半无限混凝土靶的理论研究 [J]. 现代应用物理, 2018, 9(4): 040102.LU Z C, ZHANG Y D, WEN H M, et al. Theoretical study on the penetration of long rods into semi-infinite concrete target [J]. Modern Applied Physics, 2018, 9(4): 040102. [10] FORRESTAL M J. Penetration into dry porous rock [J]. International Journal of Solids and Structures, 1986, 22(12): 1485–1986. [11] FREW D J, FORRESTAL M J, HANCHAK S J. Penetration experiments withlimestone targets and ogive-nose steel projectiles [J]. Journal of Applied Mechanics, 2000, 67(4): 841–845. DOI: 10.1115/1.1331283. [12] 张德志, 张向荣, 林俊德, 等. 高强钢弹对花岗岩正侵彻的实验研究 [J]. 岩石力学与工程学报, 2005, 24(9): 1612–1618. DOI: 10.3321/j.issn:1000-6915.2005.09.024.ZHANG D Z, ZHANG X R, LIN J D, et al. Penetration experiments for normal impact into granite targets with high-strength steel projectile [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(9): 1612–1618. DOI: 10.3321/j.issn:1000-6915.2005.09.024. [13] LI J C, MA G W, YU M H. Penetration analysis for geo-material based on unified strength criterion [J]. International Journal of Impact Engineering, 2008, 35: 1154–1163. DOI: 10.1016/j.ijimpeng.2008.01.003. [14] SATAPATHY S, BLESS S. Calculation of penetration resistance of brittle materials using spherical cavity expansion analysis [J]. Mechanics of Materials, 1996, 23: 323–330. DOI: 10.1016/0167-6636(96)00022-1. [15] GALANOV B A, KARTUZOV V V, IVANOV S M. New analytical model of expansion of spherical cavity in brittle material basedon the concepts of mechanics of compressible porous and powder materials [J]. International Journal of Impact Engineering, 2008, 35: 1522–1528. DOI: 10.1016/j.ijimpeng.2008.07.016. [16] ROSENBERG Z, DEKEL E. A numerical study of the cavity expansion process and its application to long-rod penetration mechanics [J]. International Journal of Impact Engineering, 2008, 35: 147–154. DOI: 10.1016/j.ijimpeng.2007.01.005. [17] TATE A. A theory for the deceleration of long rods after impact [J]. Journal of the Mechanics and Physics of Solids, 1967, 15(6): 387–399. DOI: 10.1016/0022-5096(67)90010-5. [18] RIEDELW, WICKLEIN M, THOMA K. Shock properties of conventional and high strength concrete: experimental and mesomechanical analysis [J]. International Journal of Impact Engineering, 2008, 35: 155–171. DOI: 10.1016/j.ijimpeng.2007.02.001. [19] 李干, 王明洋, 宋春明, 等. 超高速飞片撞击花岗岩实验及其动态力学性能研究 [C] // 第六届全国工程安全与防护学术会议. 湘潭: 中国岩石力学与工程学会工程安全与防护分会, 2018. [20] PETERSEN CF. Shock wave studies of selected rocks [D]. California: Stanford University, 1969. [21] 王明洋, 李杰, 李海波, 等. 岩石的动态压缩行为与超高速动能弹毁伤效应计算 [J]. 爆炸与冲击, 2018, 38(6): 1200–1217. DOI: 10.11883/bzycj-2018-0173.WANG M Y, LI J, LI H B, et al. Dynamic compression behavior of rock and simulation of damage effects of hypervelocity kinetic energy bomb [J]. Explosion and Shock Waves, 2018, 38(6): 1200–1217. DOI: 10.11883/bzycj-2018-0173. [22] ROSENBERG Z, TSALIAH J. Applying Tate’s model for the interaction of long rod projectiles with ceramic targets [J]. International Journal of Impact Engineering, 1990, 9(2): 247–251. DOI: 10.1016/0734-743X(90)90016-O. [23] FORRESTAL M J, TZOU D Y, ASKARI E, et al. Penetration into ductile metal targets with rigid spherical-nose rods [J]. International Journal of Impact Engineering, 1995, 16(5/6): 699–710. [24] ROSENBERG Z, DEKEL E. On the deep penetration of deforming long rods [J]. International Journal of Solids and Structures, 2010, 47: 238–250. DOI: 10.1016/j.ijsolstr.2009.09.030. [25] TATE A. Long rod penetration models: Part Ⅱ: extensions to the hydrodynamic theory of penetration [J]. International Journal of Mechanical Sciences, 1986, 28(9): 599–612. DOI: 10.1016/0020-7403(86)90075-5. [26] WALKER J D, ANDERSON C E. A time-dependent model for long-rod penetration [J]. International Journal of ImpactEngineering, 1995, 16(1): 19–48. [27] ROSENBERG Z, DEKEL E. The deep penetration of concrete targets by rigid rods: revisited [J]. International Journal of Protective Structure, 2010, 1: 125–144. DOI: 10.1260/2041-4196.1.1.125. [28] ZHANG M H, SHIM V P, LU G, et al. Resistance of high-strength concrete to projectile impact [J]. International Journal of ImpactEngineering, 2005, 31: 825–841. DOI: 10.1016/j.ijimpeng.2004.04.009. [29] GRADY D E. Shock-wave compression of brittle solids [J]. Mechanics of Materials, 1998, 29: 181–203. [30] LUNDBORG N. Strength of rock-like materials [J]. International Journal of Rock Mechanics and Mining Sciences, 1968, 5: 427–454. DOI: 10.1016/0148-9062(68)90046-6. [31] ROSENBERG Z. On the relation between the Hugoniot elastic limit and the yield strength of brittle materials [J]. Journal of Applied Physics, 1993, 74(1): 752–753. DOI: 10.1063/1.355247. [32] 王礼立. 应力波基础[M]. 北京: 国防工业出版社, 2005. [33] 胡进军, 谢礼立. 地震超剪切破裂研究现状 [J]. 地球科学进展, 2011, 26(1): 39–47.HU J J, XIE L L. Review of the state-of-art researches on earthquake super-shear rupture [J]. Advance in Earth Science, 2011, 26(1): 39–47. [34] SATAPATHY S, BLESS S. Cavity expansion resistance of brittle materials obeying a two-curve pressure-shear behavior [J]. Journal of Applied Physics, 2000, 88(7): 4004–4012. DOI: 10.1063/1.1288007. [35] BAVDEKAR S, PARSARD G, SUBHASH G, et al. Animproved dynamic expanding cavity model for high-pressureand high-strain rate responseofceramics [J]. International Journal of Solids and Structures, 2017, 26: 39–47. [36] STERNBERG J. Material properties determining the resistance of ceramics to high velocity penetration [J]. Journal of Applied Physics, 1989, 65(9): 3417–3424. DOI: 10.1063/1.342659. [37] KOZHUSHKO A A, RYKOVA I I, SINANI A B. Resistance of ceramics to penetration at impact velocities above 5 km/s [J]. Journal de Physique IV, 1991, 1(C3): C3–117. 1. [38] ISBELL W M, ANDERSON C E, ASAY J R, et al. Penetration mechanics research in the former Soviet Union [R]. San Diego, California: Science Applications International Corp, 1992. [39] VLASOVA M V, KAKAZEI N G, KOVTUN V I. Failure of self-bonded silicon carbide under dynamic pressure [J]. Powder Metallurgy and Metal Ceramics, 1988, 27(4): 325–329. [40] 徐建波. 长杆弹对混凝土的侵彻特性研究[D]. 长沙: 国防科学技术大学, 2001. [41] 刘桂武, 倪长也, 金峰, 等. 陶瓷/金属复合装甲抗弹约束效应述评 [J]. 西安交通大学学报, 2011, 45(3): 7–15.LIU G W, NI C Y, JIN F, et al. Review of anti-ballistic confinement effects of ceramic-metal composite armor [J]. Journal of Xi’an Jiaotong University, 2011, 45(3): 7–15. [42] 徐松林, 单俊芳, 王鹏飞, 等. 三轴应力状态下混凝土的侵彻性能研究 [J]. 爆炸与冲击, 2019, 39(7): 071101. DOI: 10.11883/bzycj-2019-0034.XU S L, SHAN J F, WANG P F, et al. Penetration performance of concrete under triaxial stress [J]. Explosion and Shock Waves, 2019, 39(7): 071101. DOI: 10.11883/bzycj-2019-0034. [43] 蒙朝美, 宋殿义, 蒋志刚, 等. 多边形钢管约束混凝土靶抗侵彻性能试验研究 [J]. 振动与冲击, 2018, 37(13): 14–19.MENG C M, SONG D Y, JIANG Z G, et al. Tests for anti-penetration performance of polygonal steel tube-confined concrete targets [J]. Journal of Vibration and Shock, 2018, 37(13): 14–19. [44] 任劼, 党发宁, 马宗源, 等. 复杂地应力条件下聚能射流装药侵彻深部砂岩穿透深度研究 [J]. 岩石力学与工程学报, 2018, 37(3): 679–688.REN J, DANG F N, MA Z Y, et al. Penetration depth of shaped charge into deep sandstone under complex geostress [J]. Chinese Journal of Rock Mechanics and Engineering, 2018, 37(3): 679–688. [45] FAN P X, WANG M Y, SONG C M. Anti-strike capability of steel-fiber reactive powder concrete [J]. Defence Science Journal, 2013, 63(4): 363–368. DOI: 10.14429/dsj.63.2407. 期刊类型引用(8)
1. 魏俊，廖华林，梁红军，李宁，高富成，张端瑞，陈龙，冯惠. 三向应力下砾岩的动力学特性及能耗分析. 中国石油大学学报(自然科学版). 2023(04): 102-110 . 
百度学术2. 张仲昊，汪维，张国凯，王振，吴汩. 不同高温作用后混凝土劣化损伤性能. 兵工学报. 2023(S1): 152-159 . 百度学术3. 赵行之，宗琦，吕闹，汪海波. 冲击荷载作用下橡胶混凝土动态力学性能研究. 河南科技. 2022(13): 73-79 . 百度学术4. 文彦博，黄瑞源，李平，马剑，肖凯涛. 高温高应变率下混凝土材料的损伤演化方程. 高压物理学报. 2021(02): 54-63 . 百度学术5. 陈振富，叶涛，陶秋旺，吴文涛，倪康，唐寿康. 掺铅锌尾矿砂混凝土动态劈拉性能试验研究. 混凝土与水泥制品. 2021(09): 86-90 . 百度学术6. 谢立栋，东兆星，姜慧，朱炯，齐燕军. 早龄期混凝土动强度应变率系数的统计方法. 兵工学报. 2021(S1): 159-166 . 百度学术7. 龙旭，毛明晖，卢昶衡，苏天雄，贾冯睿. 基于人工神经网络的混凝土类材料SHPB动态压缩性能预测. 南京航空航天大学学报. 2021(05): 789-800 . 百度学术8. 王明俊，梁永威，杨飞，冯万辉. 某隧道地基岩体的应变率效应研究. 路基工程. 2021(05): 148-152 . 百度学术其他类型引用(11)
-
下载:
下载:
百度学术
计量
- 文章访问数: 4468
- HTML全文浏览量: 2124
- PDF下载量: 118
- 被引次数: 19