Damage grades of reinforced concrete bent structures against blast
-
摘要: 为了研究钢筋混凝土排架结构在大当量爆炸冲击波下的破坏规律,依据最大TNT当量为3 t的爆炸试验,对排架主体结构的抗爆破坏等级进行数值模拟研究。通过量纲分析得到1/2缩比模型的荷载参数和结构尺寸。基于Abaqus有限元软件,利用CONWEP方法实现爆炸加载,分别计算装药0.5 t爆距33 m和装药3 t爆距33 m两种工况下排架结构的破坏形态,并与试验结果进行对比。进一步通过控制药量和距离,计算不同超压和冲量下缩比模型的破坏形态。研究结果表明,排架的关键破坏特征为中间承重柱的倾覆转动;数值计算与试验破坏形态吻合较好,特征位移和特征转角的最大相对误差分别为5.6%和4.6%。以承重柱的倾覆角作为划分依据,将计算结果分为3种破坏等级,拟合得到的超压-冲量曲线和药量-距离曲线可用于厂房安全距离和仓库容量设计以及意外爆炸下的破坏程度预估。Abstract: To study the failure law of reinforced concrete bent structures under large equivalent explosions, the damage grades of the bent structures against blast were numerically calculated based on the explosion test with the maximum equivalent of 3 t TNT. The load parameters and structural dimensions of the 1/2 scaled model were obtained through dimensional analysis. Based on the Abaqus finite element software, the CONWEP method was used to achieve the blast loading. The failure modes of the structures, under the explosion loads with TNT equivalent 0.5 t and blast distance 33 m as well as TNT equivalent 3 t and blast distance 33 m, were calculated, respectively, and compared with the test results. Further, the failure patterns of the scale model under different overpressures and impulses were calculated by controlling the TNT equivalent and blast distance. The research results show that the middle column of the bent structure is prone to damage in the form of overall overturning under a lateral blast load; the calculated failure morphologies are in good agreement with the experimental ones, and the maximum relative errors of the characteristic displacements and characteristic corners are 5.6% and 4.6%, respectively. The overturning angle of the load-bearing column was used as the basis for the damage-grade division, and the calculated results were divided into three damage levels. The fitted overpressure-impulse and equivalent-distance curves can be used in the design of safety distance and warehouse capacity and the estimation of the damage degree of accidental explosion.
-
Key words:
- reinforced concrete bent structure /
- blast /
- damage grade /
- overpressure-impulse curve /
- scaled model /
- CONWEP
-
美国自9·11事件后,加强了对建筑结构抗爆的研究,根据爆炸冲击荷载、结构材料及尺寸、结构功能,制定了《结构抗偶然性爆炸设计手册TM5-1300》、《工程防恐设计暂行规程DOD2001》、《结构抗连续倒塌设计规程DOD2005》[1]等一系列抗爆设计标准。继美国之后,加拿大于2012年颁布了《抗爆设计规范CSA/S850-12》。在我国针对现有建筑结构的抗爆设计标准还不完善,相关研究较少。钢筋混凝土排架结构作为典型的大跨度空间建筑结构,被广泛应用于军用和民用工业中,在大型生产中往往成组出现。一旦发生生产意外事故、恐怖袭击,甚至武器打击,结构将遭受大当量爆炸冲击威胁。为了研究钢筋混凝土排架抗爆破坏等级,为军用和民用工业具有整体爆炸危险厂房的选址和设计提供依据,研究大当量爆炸下钢筋混凝土排架结构的破坏规律具有重要的意义。然而大当量爆炸试验的成本极高,无法大规模的开展,因此本文通过数值模拟方法在缩比试验的基础上进行参数校核,并基于此开展抗爆破坏等级研究。
由于原尺寸试验的难度较大,国内外开展了大量的缩比试验设计研究。Wang等[2]研究不同比例距离下3种不同缩比尺寸模型与原尺寸钢筋混凝土模型的损伤情况,结果表明缩比模型与原型的损伤和断裂基本一致。Yao等[3]根据相似律设计了3种缩比尺寸的箱体钢结构模型进行抗爆研究,计算结果与试验结果均表明相似系数越大两者吻合度越高。武海军等[4]对钢筋混凝土的侵彻和贯穿行为进行量纲分析,并进行了1∶1和1∶2.5缩比试验及1∶2.5和1∶4的缩比数值模拟计算,二者结果均与缩比相似律吻合较好。杨亚东等[5]为了研究冲击波特征参量和结构毁伤的相似规律,建立了钢筋混凝土结构内爆炸相似理论模型,结果发现不同缩比模型可以得到相同的破坏程度和相似的破坏形态。
钢筋混凝土结构是目前应用最为广泛的结构,国内外有不少关于钢筋混凝土结构抗爆破坏等级的研究。Jayasooriya 等[6]分别采用 SAP2000 和 LS-DYNA 软件进行模拟研究钢筋混凝土框架结构和关键构件在近场爆炸荷载作用下的响应以及残余变形能力。Carta等[7]提出了一种用于预测钢筋混凝土梁抗爆破坏程度的理论模型,并将该连续模型与等效单自由度方法进行对比,在该理论模型中考虑了材料应变率效应并能给出最大位移时程曲线。Shi等[8]、师燕超等[9]利用数值模拟方法开展了钢筋混凝土柱在爆炸荷载下的破坏等级研究,所建立的预测公式相比于单自由度分析方法能够更准确地预测钢筋混凝土柱的p-I曲线。汪维等[10]、Wang等[11]利用相互耦合等效单自由度系统对爆炸载荷作用下钢筋混凝土构件损伤程度进行了超压-冲量(p-I)曲线评估,提出了通过等效单自由度方法确定钢筋混凝土构件 p-I 曲线的简化方法。倪晋峰[12]基于CONWEP方法分析了K8型单层球面网壳结构在外爆荷载作用下的动力响应,研究炸药参数和材料参数对结构响应的影响。
从上面的讨论中发现,对于钢筋混凝土结构的抗爆数值模拟研究主要集中在单一构件(梁、柱、板)在小药量近场爆炸下的破坏,破坏等级p-I曲线的获得方法主要以理论研究为主,对于钢筋混凝土大型结构在大当量爆炸下的数值模拟研究较少。本文中围绕最大TNT当量为3 t的爆炸试验,利用数值模拟方法对钢筋混凝土排架主体结构的抗爆破坏等级进行研究。借助量纲分析方法得到1/2缩比模型的荷载参数和结构尺寸,根据破坏结果和试验数据校正数值模型,对不同超压-冲量荷载下的结构响应进行计算,拟合得到评估钢筋混凝土排架结构抗爆破坏等级的超压-冲量(p-I)曲线和当量-距离(Q-R)曲线。
1. 缩比模型设计
1.1 荷载与响应的量纲分析
在爆炸作用的中远距离处可以忽略爆轰产物直接作用,只考虑爆炸空气冲击波对于结构的作用。排架结构的特征响应主要有挠度y和转角θ,影响这2个特征参量的因素主要如下:
(1)炸药参数:炸药质量Q,炸药密度
ρe ,单位质量炸药释放的化学能Ee ,爆炸产物的膨胀指数γe 。(2)空气参数:初始状态的压力
pa ,空气密度ρa ,空气绝热指数γa 。(3)作用点与爆心的距离:R。
(4)结构尺寸参数:整体结构特征尺寸l,钢筋混凝土内部骨料和钢筋特征尺寸d(比如骨料大小、钢筋分布间距和直径)。
(5)结构材料参数:钢的材料密度
ρs ,材料弹性常数Es 和νs ,塑性阶段和破坏特征应力参数σs 和特征应变参数εs ;混凝土的材料参数ρc ,Ec ,νc ,σc ,εc 。于是挠度y和转角θ应当是上述控制参数的函数,即有
{y=f(Q,ρe,Ee,γe;pa,ρa,γa;R;l,d;ρs,Es,νs,σs,εs;ρc,Ec,νc,σc,εc)θ=g(Q,ρe,Ee,γe;pa,ρa,γa;R;l,d;ρs,Es,νs,σs,εs;ρc,Ec,νc,σc,εc) (1) 可取
R 、ρe 、Ee 作为基本量,上式可化为下面的无量纲关系:{y/R=f((Q/ρe)1/3/R,γe;pa/(ρeEe),ρa/ρe,γa;l/R,d/R;ρs/ρe,Es/(ρeEe),νs,σs/(ρeEe),εs;ρc/ρe,Ec/(ρeEe),νc,σc/(ρeEe),εc)θ=g((Q/ρe)1/3/R,γe;pa/(ρeEe),ρa/ρe,γa;l/R,d/R;ρs/ρe,Es/(ρeEe),νs,σs/(ρeEe),εs;ρc/ρe,Ec/(ρeEe),νc,σc/(ρeEe),εc) (2) 当采用相同种类的炸药和相同的结构材料在空气中做缩比模型试验,则有16个有关的控制参数与原型保持一致,即
(ρe,Ee,γe;pa,ρa,γa;ρs,Es,νs,σs,εs;ρc,Ec,νc,σc,εc)=const 则无量纲式(2)可以简化为
{y/R=f((Q/ρe)3/1/R,l/R,d/R)θ=g((Q/ρe)3/1/R,l/R,d/R) (3) 类似的,可以得到超压和冲量与炸药参数、空气参数、爆心距之间的关系:
{Δp/(ρeEe)=f((Q/ρe)1/3/R)i/[(Q/ρe)1/3ρeEe1/2]=g((Q/ρe)1/3/R) (4) 此时如果能保证试验的几何相似条件:
Q1/3mQ1/3p=RmRp=lmlp=dmdp=λ (5) 式中:下标p表示原型,下标m表示模型。
将式(5)代入式(3),炸药参数
ρe 不变,可以得到ymRm=ypRp ,θm=θp ,则缩比距离处的结构变形挠度ym=λyp ,转角θm=θp 。将式(5)代入式(4),炸药参数
ρe ,Ee 不变,可以得到Δpm=Δpp ,imQ1/3m=ipQ1/3p ,则缩比距离处的超压Δpm=Δpp ,冲量im=λip 。如果炸药参数
ρe 不变,式(4)中的参数(Q/ρe)1/3/R 正是用于计算超压的经验公式中的比例距离R/Q1/3 的变形公式。其实目前的超压经验公式正是通过前期量纲分析加后期试验确定参数的方法得到的。本文中关于荷载(当量、爆距)与钢筋混凝土和钢结构响应(位移、转角)之间的量纲分析方法也同样在很多研究[2-5]中被使用,其合理性已得到证明。
1.2 型钢与配筋限制
由于选材和施工条件的限制,很多参数需要适当调整。在尽量保证几何相似的基础上,保证结构的强度不变。例如屋架所用的L型钢和H型钢,可选型号有限,无法满足完全几何相似。为了保证与原型同样的变形程度(同样的转角和挠度比),就必须保证模型截面的极惯性矩和惯性矩的缩比[3]:
I′m=Im=λ4Ip (6) 式中:
Im 为理论缩比后的极惯性矩或惯性矩,I′m 为实际缩比的极惯性矩或惯性矩,λ 为缩比因子(λ<1 ),Ip 为原型的极惯性矩或惯性矩。另外,钢筋混凝土配筋的直径选择有限,无法做到严格的几何缩比,为了保证钢筋混凝土截面强度不变,需要适当调整钢筋间距和钢筋直径,以保证模型配筋率与原型相同。
为了尽量减小尺寸效应等因素造成的误差,考虑到选材(HN250辅助梁两个方向的惯性矩为4080 cm4和294 cm4,惯性矩最小的H型钢HN100两个方向的惯性矩为192 cm4和14.9 cm4,限定的缩比大于0.47)和施工等限制条件,最终选择1∶2缩比方案。
缩比试验中的当量为原型的1/8,爆距为原型的1/2,结构特征尺寸为原型的1/2,型钢惯性矩近似为原型的1/16,钢筋混凝土配筋率近似与原型相同,使用相同的炸药和相同的钢筋和混凝土材料,在相同的空气环境下进行试验。按照量纲分析结果,缩比模型所受到的冲击波超压与原型相同,冲量为原型的1/2,结构响应的特征挠度为原型的1/2,转角与原型相同。
2. 有限元计算模型
排架结构是由2排多个立柱和屋架组成的狭长结构,本文选取钢筋混凝土排架结构的一个单元进行分析,该单元主要由2排共6个钢筋混凝土立柱和将它们连接在一起的屋架组成。具体设计参照《混凝土结构设计规范》(GB50010—2017)[13]和《钢结构设计规范》(GB50017—2017)[14]。
2.1 数值建模
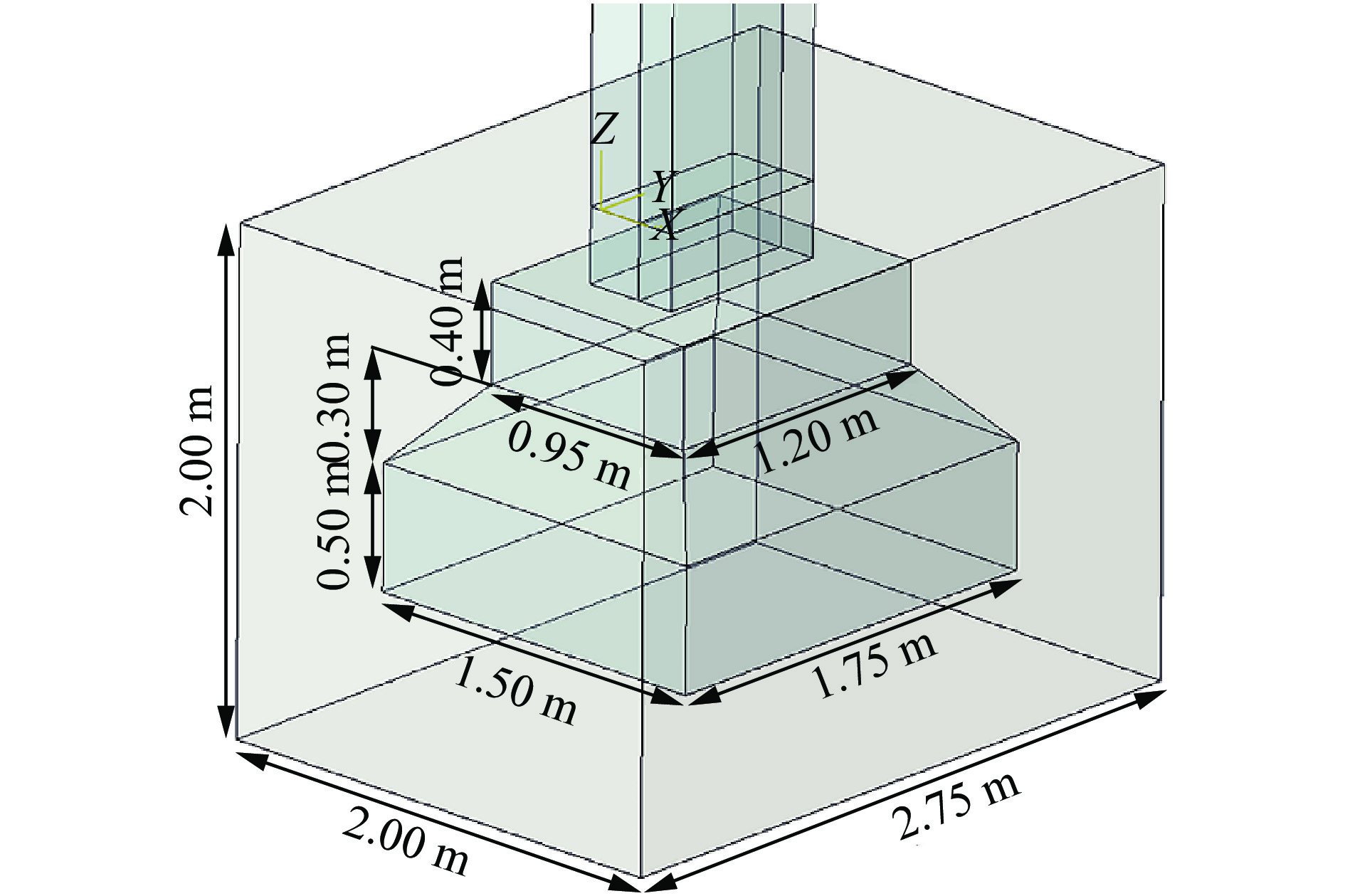
利用Abaqus有限元软件对缩比模型进行建模,整体尺寸如图1所示,排架跨度12 m,柱高10.5 m,图中X方向为排架延伸方法,柱间距4.5 m。屋架与立柱之间采用铰接约束,钢筋与混凝土使用分离式模型分别建模,两者使用嵌入式接触。土体的六个面中,除上表面外,均设置为固定约束。
屋架按照截面属性差异显示的三维效果如图2所示,图中每一种颜色代表一种截面,包括不同类型的L型钢和H型钢。屋架为beam梁单元,单元类型为B31。梁柱结合处的剖视细节如图3所示,主筋和箍筋完全按照试验设计建模。混凝土为solid六面体单元,单元类型为C3D8R;钢筋为truss单元,单元类型为T3D2。立柱基础及周围土的几何模型参数如图4所示,单元为solid六面体单元,单元类型为C3D8R。
单元大小是在网格敏感性分析的基础上综合考虑计算精度和计算效率选取的。混凝土和土等实体单元的单元小于6 cm时对结构变形的影响较小,考虑到计算效率,单元大小设置为6 cm;屋架和钢筋等单元的网格敏感性较低,单元大小设置为20 cm(小于结构整体尺寸两个数量级)。
2.2 材料参数
钢结构屋架使用Q235B碳素结构钢,对试验样本进行应变率范围为0.001~2 747 s−1的材料试验。使用Johnson-Cook本构模型,拟合所得材料弹塑性参数如表1所示。
表 1 Q235B钢的弹塑性参数Table 1. Elastoplastic parameters of steel Q235Bρ/(kg·m−3) E/GPa μ A/MPa B/MPa n m C ˙ε0/s−1 7835.5 200.6 0.259 314 527.5 0.436 0.757 0.02 0.001 钢筋为HRB400型材料,对试验样本进行应变率范围为0.001 s−1~2 162 s−1,同样使用Johnson-Cook本构模型,拟合所得材料弹塑性参数如表2所示。
表 2 HRB400钢的弹塑性参数Table 2. Elastoplastic parameters of steel HRB400ρ/(kg·m−3) E/GPa μ A/MPa B/MPa n m C ˙ε0/s−1 7766 200.5 0.241 476.2 719.8 0.44 0.757 0.014 0.001 混凝土考虑拉压异性,使用塑性损伤模型(concrete damaged plasticity,CDP),并考虑了应变率效应,采用的CDP基本参数可参考文献[15]。
试验场地土质为粉质黏土,考虑土体硬化阶段,采用Drucker-Prager模型描述土体本构。材料参数可参考文献[16-17]。
3. CONWEP模拟爆炸加载
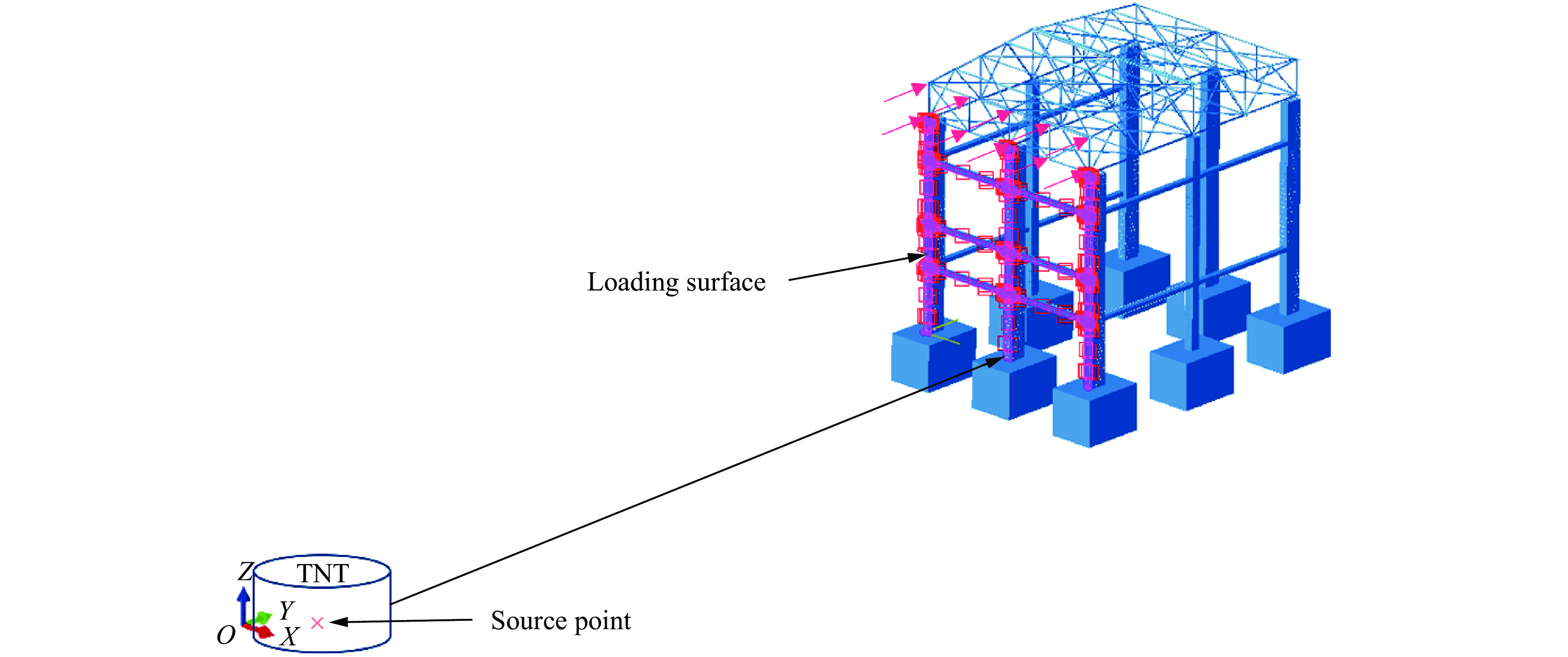
CONWEP是来源于美国军方试验数据的爆炸荷载计算程序,可准确高效地计算结构受到的外爆冲击波荷载[18-19]。对于给定的爆炸源点、加载面、爆炸类型和TNT当量,CONWEP方法可根据加载方向和距离,分别计算加载面上不同点的荷载时程曲线并进行加载。图5所示为CONWEP爆炸加载示意图,图中指定了爆炸源点和爆炸加载面(右侧红色区域),并设置了TNT当量和爆炸类型。CONWEP可根据TNT当量和相对距离分别计算出加载面上不同点的压力曲线并加载。相比简化的压力时程曲线加载方法,CONWEP考虑了加载点的差异性和加载曲线的精确度。相比于建立空气和炸药单元的流固耦合方法,CONWEP计算效率较高[20-22]。综合考虑计算效率和精度,选用CONWEP方法模拟爆炸加载。
本文主要研究钢筋混凝土排架主体结构抗爆破坏等级,由于填充墙和屋面板等围护结构在侧向荷载下易被破坏且其与主体结构连接较弱,极易分离,所以本研究忽略了维护结构的建模。维护结构的破坏相对冲击波传播较慢,考虑到维护结构的阻挡作用,这里只选择前排迎爆面进行加载。由于排架结构为外凸半封闭式结构,基本可以忽略空气冲击波在结构上反射的作用。试验中也并未发现空气冲击波反射和绕射造成的明显破坏。所以本文忽略了空气冲击波反射和绕射对结构的作用。
另外,迎爆面的屋面板与荷载方向近似垂直,在冲击波荷载下的压力荷载可直接传递给屋架。由于CONWEP荷载无法直接作用在梁单元上,此处的荷载可利用CONWEP方法计算压力时程曲线后,根据加载面积转换为力时程曲线施加在屋架节点上,如图5中红色箭头所示。
试验中炸药放在地上且正对着结构,所以这里的爆炸类型为表面爆炸。爆距为爆炸源点到结构前排中间柱的距离。CONWEP计算3 t当量33 m爆距(简称3 t-33 m)和24 t当量66 m爆距(简称24 t-66 m)下的超压曲线和冲量曲线分别如图6和图7所示。3 t-33 m的超压峰值与24 t-66 m相同,加载时间和冲量是24 t-66 m的1/2,这与1.1节中关于爆炸荷载的量纲分析结论相同。
4. 计算结果对比与分析
本研究设计了0.5 t-33 m(0.5 t TNT,33 m爆距)和3 t-33 m(3 t TNT,33 m爆距)这2个工况下的爆炸试验。试验系统布置如图8所示,在大当量试验中利用网桥传输拉开子站与基站的距离,提高数据采集成功率,但受维护结构破坏作用影响,可用动态数据有限。
缩比试验宏观破坏形态航拍图如图9所示。图中可以发现,0.5 t爆后,主体结构并未发生明显变形,部分屋面板脱落,这也说明了屋面板与主体结构连接强度较弱。3 t爆后,主体结构发生明显变形,迎爆面立柱倾斜,屋架部分破坏,屋面板飞落至背爆面。
试验测得0.5 t工况下,迎爆面中间立柱内侧距地1 m高处Y方向的位移时程曲线,与模拟计算结果对比见图10。模拟结果与试验吻合较好,峰值位移、残余位移和振动周期基本一致,相对误差分别为2.1%、5.6%和2.1%。
3 t工况下的模拟结果与试验结果的宏观破坏特征相似,对比如图11所示。可以发现中间立柱保持笔直,向后方(Y方向)倾斜,并未发现明显X方向的位移以及立柱自身的扭转变形。屋架围绕中间立柱柱顶的部分发生失效破坏,圈梁在梁柱结合处被拉断后散落在中间立柱的柱脚附近。清除中间立柱柱脚附近的砖和土之后可以发现,立柱连带基础在爆炸冲击下发生了倾覆转动,而立柱和基础本身没有发生明显变形。如图12所示,模型计算结果成功再现了试验关于基础和圈梁的局部破坏特征。试验测得3 t工况下,迎爆面中间立柱的转角为7.66°,侧面中间立柱的转角为1.53°,如图13所示。模拟结果分别为7.73°和1.60°,与试验基本一致,相对误差分别为0.91%和4.58%。
从破坏过程的分析可以发现,排架首尾两端的立柱由于具有侧面圈梁的支撑,较为稳固;排架中间柱在侧向爆炸荷载下极易发生破坏。如图14所示,在冲击波作用下,与中间柱连接的屋架首先发生破坏,然后中间柱与圈梁的连接发生破坏,完全失去上部约束的中间立柱脱离整个排架系统,随后在冲击波作用下发生进一步的倾覆转动。这为排架结构的防爆设计提供了一定参考,例如地基和侧向支撑的加强。
综上所述,0.5 t和3 t工况下的模拟结果在整体和局部破坏形态上与试验结果相似,位移和转角等动态响应与试验吻合较好。排架的关键破坏特征为中间立柱的倾覆转动,破坏过程的分析可为排架结构的抗爆设计提供参考。
5. 抗爆破坏等级计算
根据试验结果,参考美国UFC 3-340-02 《结构抗偶然爆炸手册》第四章“钢筋混凝土结构设计”中的规定[23],以排架立柱的最大转角作为钢筋混凝土排架主体结构破坏等级划分的关键参数。排架立柱2°和6°转角作为轻度破坏、中度破坏和严重破坏的分界线,如表3所示。通过调节比例距离控制超压峰值,通过调节爆炸距离和当量控制冲量。利用验证后的数值模型计算得到缩比模型的p-I数据,根据相似关系拟合得到钢筋混凝土排架原型主体结构的破坏等级划分p-I曲线,拟合结果如图15所示,拟合公式如下:
lni={0.1487ln2p−1.7035lnp+12.4547θ=2∘0.1168ln2p−1.4048lnp+12.2016θ=6∘ (7) 相对于具有超压渐近线和冲量渐近线的双渐近线形式的p-I曲线,该曲线在高超压低冲量的脉冲荷载区有右倾趋势。此时荷载具有作用时间短峰值高的特点,这种情况下结构局部可能发生破坏,局部破坏和整体破坏综合作用可能是造成上述右倾趋势的原因,相关文献[24-25]中同样出现了特征。但具体的作用机理和影响规律需要进一步做针对性研究。
图15中钢筋混凝土排架主体结构的破坏等级划分p-I曲线可直接用于24 m跨钢筋混凝土排架,该p-I曲线可以用于评估2 000 t TNT当量以内的工况产生的冲击波对结构造成的最大破坏程度。为了方便安全距离设计,可画出破环等级划分当量-距离(Q-R)曲线,如图16所示,拟合公式下:
lgQ={0.8774+1.7965logRθ=2∘1.2397+1.7485logRθ=6∘ (8) 该Q-R曲线可为国防工业中具有爆炸危险的厂房的安全距离和仓库容量设计提供具体的参考依据。
6. 结 论
本文围绕最大当量为3 t TNT的爆炸试验,开展钢筋混凝土排架主体结构在大当量外部爆炸下的破坏等级划分数值模拟研究。研究主要得到以下结论:
模拟计算结果与试验吻合较好,0.5 t工况下特征点的峰值位移、残余位移和振动周期与试验相对误差分别为2.1%、5.6%和2.1%。3 t工况下的整体破坏形态和有关圈梁和基础的局部破坏形态,计算结果与试验结果相似,立柱转角计算结果与试验最大相对误差为4.6%。
排架首尾两端的立柱由于具有侧面圈梁的支撑,较为稳固;排架中间柱在侧向爆炸荷载下易发生整体的倾覆转动破坏。地基和侧向支撑的加强可作为排架结构的防爆设计重点。
以承重柱的倾覆角作为划分依据,将计算结果分为3种破坏等级,拟合得到的破坏等级划分P-I曲线可用于评估2 000 t TNT当量以内24 m跨钢筋混凝土排架结构的最大破坏程度。Q-R曲线可用于厂房安全距离和仓库容量设计。
-
表 1 Q235B钢的弹塑性参数
Table 1. Elastoplastic parameters of steel Q235B
ρ/(kg·m−3) E/GPa μ A/MPa B/MPa n m C ˙ε0/s−1 7835.5 200.6 0.259 314 527.5 0.436 0.757 0.02 0.001 表 2 HRB400钢的弹塑性参数
Table 2. Elastoplastic parameters of steel HRB400
ρ/(kg·m−3) E/GPa μ A/MPa B/MPa n m C ˙ε0/s−1 7766 200.5 0.241 476.2 719.8 0.44 0.757 0.014 0.001 -
[1] Department of Defense. Design of buildings to resist progressive collapse: UFC4-023-03 [S]. Washington: U S Department of Defense, 2005: 1−232. [2] WANG W, ZHANG D, LU F Y, et al. Experimental study on scaling the explosion resistance of a one-way square reinforced concrete slab under a close-in blast loading [J]. International Journal of Impact Engineering, 2012, 49: 158–164. DOI: 10.1016/j.ijimpeng.2012.03.010. [3] YAO S J, ZHANG D, LU F Y, et al. A combined experimental and numerical investigation on the scaling laws for steel box structures subjected to internal blast loading [J]. International Journal of Impact Engineering, 2017, 102: 36–46. DOI: 10.1016/j.ijimpeng.2016.12.003. [4] 武海军, 黄风雷, 陈利, 等. 动能弹侵彻钢筋混凝土相似性分析 [J]. 兵工学报, 2007, 28(3): 276–280. DOI: 10.3321/j.issn: 1000-1093.2007.03.005.WU H J, HUANG F L, CHEN L, et al. Similarity law analyses of penetration behavior in reinforced concrete [J]. Acta Armamentarii, 2007, 28(3): 276–280. DOI: 10.3321/j.issn: 1000-1093.2007.03.005. [5] 杨亚东, 李向东, 王晓鸣, 等. 钢筋混凝土结构内爆炸相似模型试验研究 [J]. 南京理工大学学报, 2016, 40(2): 135–141. DOI: 10.14177/j.cnki.32-1397n.2016.40.02.002.YANG Y D, LI X D, WANG X M, et al. Experimental study on similarity model of reinforced concrete structure under internal explosion [J]. Journal of Nanjing University of Science and Technology, 2016, 40(2): 135–141. DOI: 10.14177/j.cnki.32-1397n.2016.40.02.002. [6] JAYASOORIYA R, THAMBIRATNAM D P, PERERA N J, et al. Blast and residual capacity analysis of reinforced concrete framed buildings [J]. Engineering Structures, 2011, 33(12): 3483–3495. DOI: 10.1016/j.engstruct.2011.07.011. [7] CARTA G, STOCHINO F. Theoretical models to predict the flexural failure of reinforced concrete beams under blast loads [J]. Engineering Structures, 2013, 49: 306–315. DOI: 10.1016/j.engstruct.2012.11.008. [8] SHI Y C, HAO H, Li Z X. Numerical derivation of pressure–impulse diagrams for prediction of RC column damage to blast loads [J]. International Journal of Impact Engineering, 2008, 35(11): 1213–1227. DOI: 10.1016/j.ijimpeng.2007.09.001. [9] 师燕超. 爆炸荷载作用下钢筋混凝土结构的动态响应行为与损伤破坏机理[D]. 天津: 天津大学, 2009: 1−8. [10] 汪维. 钢筋混凝土构件在爆炸载荷作用下的毁伤效应及评估方法研究[D]. 长沙: 国防科学技术大学, 2012: 1−7. [11] WANG W, ZHANG D, LU F Y, et al. The influence of load pulse shape on pressure-impulse diagrams of one-way RC slabs [J]. Structural Engineering and Mechanics, 2012, 42(3): 363–381. DOI: 10.12989/sem.2012.42.3.363. [12] 倪晋峰. 单层球面网壳基于CONWEP外爆响应分析及试验设计[D]. 哈尔滨: 哈尔滨工业大学, 2012: 13−19. [13] 中华人民共和国住房和城乡建设部. 混凝土结构设计规范: GB 50010—2010 [S]. 北京: 中国建筑工业出版社, 2017: 1−284. [14] 中华人民共和国住房和城乡建设部. 钢结构设计标准规范:GB 50017—2017 [S]. 北京: 中国建筑工业出版社, 2018: 1−226. [15] 任光. 爆炸载荷下建构筑物连续倒塌数值模拟[D]. 北京: 北京理工大学, 2016: 1−6. [16] 郭春, 彭振斌. 建筑抗震中单桩摩阻力动力效应分析 [J]. 湖南大学学报(自然科学版), 2015, 42(3): 57–62. DOI: 10.16339/j.cnki.hdxbzkb.2015.03.009.GUO C, PENG Z B. Analysis for the dynamic effect of friction forces of single pile [J]. Journal of Hunan University (Natural Sciences), 2015, 42(3): 57–62. DOI: 10.16339/j.cnki.hdxbzkb.2015.03.009. [17] 李世平, 张珂, 程龙. 基于ABAQUS软件研究载体桩在焦作某工程应用 [J]. 水利与建筑工程学报, 2018, 16(1): 28–35. DOI: 10.3969/j.issn.1672-1144.2018.01.006.LI S P, ZHANG K, CHENG L. Research on application of ram-compacted piles with bearing base in Jiaozuo based on ABAQUS software [J]. Journal of Water Resources and Architectural Engineering, 2018, 16(1): 28–35. DOI: 10.3969/j.issn.1672-1144.2018.01.006. [18] IMBALZANO G, LINFORTH S, NGO T D, et al. Blast resistance of auxetic and honeycomb sandwich panels: comparisons and parametric designs [J]. Composite Structures, 2018, 183: 242–261. DOI: 10.1016/j.compstruct.2017.03.018. [19] CASTEDO R, SEGARRA P, ALAÑON A, et al. Air blast resistance of full-scale slabs with different compositions: numerical modeling and field validation [J]. International Journal of Impact Engineering, 2015, 86: 145–156. DOI: 10.1016/j.ijimpeng.2015.08.004. [20] SOUTIS C, MOHAMED G, HODZIC A. Modelling the structural response of GLARE panels to blast load [J]. Composite Structures, 2011, 94(1): 267–276. DOI: 10.1016/j.compstruct.2011.06.014. [21] DU H, LI Z X. Numerical analysis of dynamic behavior of RC slabs under blast loading [J]. Transactions of Tianjin University, 2009, 15(1): 61–64. DOI: 10.1007/s12209-009-0012-7. [22] 杨军, 张帝, 任光. 基于CONWEP动态加载的建筑物爆破拆除数值模拟 [J]. 工程爆破, 2016, 22(5): 1–6, 91. DOI: 10.3969/j.issn.1006-7051.2016.05.001.YANG J, ZHANG D, REN G. Numerical simulation of blasting demolition of buildings and structures based on CONWEP dynamic loading [J]. Engineering Blasting, 2016, 22(5): 1–6, 91. DOI: 10.3969/j.issn.1006-7051.2016.05.001. [23] Department of Defense. Structures to resist the effects of accidental explosions: UFC3-340-02[S]. Washington: U S Department of Defense, 2008: 1042−1280. [24] 李天华. 爆炸荷载下钢筋混凝土板的动态响应及损伤评估[D]. 西安: 长安大学, 2012: 100−104. [25] WEI X Y, HUANG T, LI N. Numerical derivation of pressure-impulse diagrams for unreinforced brick masonry walls [J]. Advanced Materials Research, 2011, 368-373: 1435–1439. DOI: 10.4028/www.scientific.net/AMR.368-373.1435. 期刊类型引用(8)
1. 陈泰年,陶西贵,李红卫,曾丹,张珊珊,尹帅,张雨帅. 泄压坑道式工房内爆效应工程计算方法研究. 北京理工大学学报. 2024(06): 588-596 .  百度学术
百度学术2. 郭玉旭,席丰,谭英华,胡亚超,刘锋. 超高车辆与箱梁立交桥碰撞简化计算模型评估分析. 爆炸与冲击. 2023(03): 102-116 .  本站查看
本站查看3. 杨允锴,武双章,高振儒,毛益明,杨陈山. 装药参数影响钢筋混凝土柱毁伤效应的试验与数值仿真研究. 爆破. 2023(03): 143-150 .  百度学术
百度学术4. 杨允锴,武双章,高振儒,毛益明. 近爆作用下钢筋混凝土柱损伤判据的试验研究. 工程爆破. 2023(05): 47-56 .  百度学术
百度学术5. 王成,杨靖宇,迟力源,王万里,陈泰年. 钢筋混凝土端面重墙结构的抗爆性能规律. 兵工学报. 2022(01): 131-139 .  百度学术
百度学术6. 王晓磊,孙炳鑫,刘历波,史红伟. 香蕉纤维加固钢筋混凝土板爆炸作用下动力响应数值模拟研究. 混凝土. 2022(09): 44-48+55 .  百度学术
百度学术7. 杨成林,徐迎,洪建,孔新立. 强动载作用下海水海砂混凝土应用研究进展. 硅酸盐通报. 2021(02): 415-422 .  百度学术
百度学术8. 张文岚,杜礼明,章芝霖,李梓豪. 爆炸荷载作用下高速列车车窗玻璃的动态响应. 大连交通大学学报. 2021(05): 7-12 .  百度学术
百度学术其他类型引用(4)
-


























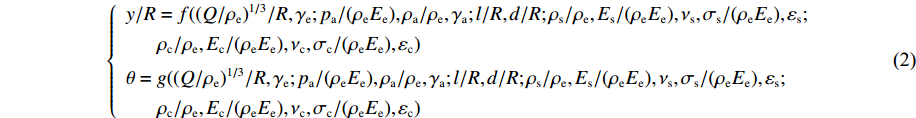

























 下载:
下载:


















 下载:
下载:















 百度学术
百度学术