A novel technique for determining the dynamic Bauschinger effect by electromagnetic Hopkinson bar
-
摘要: 金属材料在复杂载荷条件下的动态力学行为研究一直备受关注,但受限于实验设备,金属材料的动态包辛格效应响应一直都难以获得。为了探究金属材料的包辛格效应与应变率效应之间的关系,本文中提出一种基于电磁霍普金森杆(electromagnetic split Hopkinson bar,ESHB) 的非同步加载实验技术,为测试金属材料在高应变率加载下的包辛格效应提供了一种有效的实验方法。本文中,首先介绍了非同步加载装置的主要特点,即可以用两列由脉冲发生器产生的应力波对受载试样进行连续的一次动态拉-压循环加载,且加载过程保证了应力波的一致性。分析了应力波对试样加载过程中的波传播历程,确保了加载过程的连续性。随后介绍了动态加载过程,数据处理方法和波形分离手段,并对动态加载过程进行应力平衡性分析,论证了实验装置的可靠性。最后采用该方法测试了5%预应变下6061铝合金动态压缩-动态拉伸的包辛格效应,并与准静态下的实验结果进行对比。实验结果表明,该材料单轴压缩没有明显的应变率效应,但其包辛格效应具有应变率依赖性,高应变率下材料的包辛格应力影响因子由0.07增大至0.17,具有显著的提升,这对传统意义上铝合金材料应变率不敏感的结论提出了挑战。Abstract: Dynamic mechanical behavior of metallic materials under complicated loading conditions has attracted much attention. However, it is hard to obtain the dynamic Bauschinger effect of metallic materials due to the limitation of loading equipment. In order to investigate the relationship between the Bauschinger effect and strain rate effect of metallic materials, this paper proposes an asynchronous loading technique based on electromagnetic split Hopkinson bar system, which could provide an effective way to study the Bauschinger effect of metallic materials under high strain rate loading. We first introduce the main characteristics of the asynchronous loading device, that is, the specimen can be loaded by one cycle of continuous dynamic tension-compression loading pulse in which the two separate stress waves are created by electromagnetic pulse generators and prove to maintain their consistency. The propagation of stress waves was analyzed to ensure the continuity of the loading process. Then the dynamic loading process and the methods of data processing and stress wave separation are presented. Stress equilibrium was also analyzed in order to demonstrate the reliability of the equipment. Finally, the Bauschinger effect of 6061 aluminum alloy at 5% pre-strain during the process of dynamic compression to dynamic tension loading was studied using this method, and the corresponding quasi-static tests were also conducted for comparison. It was found that the material shows less strain-rate sensitivity under axial compression loading, while its Bauschinger stress parameter increases from 0.07 in quasi-static loading to 0.17 in dynamic loading. The results indicate that the Bauschinger effect of 6061 aluminum alloys depends on the strain rate and can be significantly enhanced under dynamic loading. This conclusion presents a challenge to the traditional conception that aluminum alloys are insensitive to strain rate.
-
金属材料在复杂应力环境下的动态响应一直是学者们关注的问题,特别是随着我国航空航天和国防事业的发展,越来越多的工作环境要求金属材料在高应变率受载的同时还可能受到多次、循环加载,例如装甲受连续冲击导致的冲击疲劳问题等。因此,研究复杂载荷条件下金属材料的动态力学行为具有重要意义。实际上,即使在遭受单一冲击源加载时,结构中的材料也往往不是仅受到单一脉冲的作用,该冲击源在边界或界面处的反射有可能会对材料进行二次甚至多次加载。例如:板材在受到面外冲击时材料首先被压缩,而压缩脉冲在自由面反射后转化成拉伸脉冲,随后该拉伸脉冲再次对材料进行加载。当载荷强度超过材料的屈服强度时,材料在多次冲击加载下的变形行为不能用简单的线性叠加来计算,其与材料本身的塑性流动行为密切相关,必须要通过实验才能准确获得。
包辛格效应是指金属材料在加工过程中预先加载产生一定的塑性变形,随后反向加载屈服应力下降的效应[1]。低应变率下金属的材料包辛格效应目前已经有较为完备的实验技术和诸多文献报道[2-6],但高应变率加载下材料的包辛格效应却鲜见报道。包辛格效应被定义为反向屈服应力受正向变形影响的程度,如图1所示,
σ0 为正向屈服应力,σf 为正向最大流动应力,σr 为反向屈服应力。为了量化这种影响,本文中采用包辛格应力参数 (Bauschinger stress parameter,BSP)用于描述包辛格效应[7]:βσ=(|σf|−|σr|)/2|σf| (1) βσ 越大,则说明材料的包辛格效应越明显。研究表明[7-8],材料的包辛格效应源自变形过程中材料位错密度变化产生的短程内应力和母相与硬质相不相容导致的长程内应力,它反映了材料在不同预应变下反向屈服应力的变化程度,可以用于衡量材料在预变形过程中内应力对于后继屈服应力的影响,因此材料的包辛格效应与变形历史有关。金属材料在高应变率加载下会产生屈服应力和流动应力提高的现象,即应变率效应[9],其产生的原因被认为与材料高速变形过程中的能量势垒提高有关,由于应变速率影响了位错和滑移的发展,使得微观层面材料的变形历史可能与准静态不同,从而导致应变率效应存在着与包辛格效应产生耦合的可能性,因此有必要对动态包辛格效应进行研究,以期观察应变率对材料包辛格效应的影响。
金属材料的包辛格效应可能会因应力循环实验中应变率的变化的影响而产生与预设情况不符的结果,准静态包辛格效应实验可采用标准化装置进行来规避该问题,而动态包辛格效应实验则缺乏相应的设备。霍普金森杆实验技术最早于1914年被提出[10],目前已经成为用于材料动态力学性能测试的主要手段之一[11-16]。然而由于实验技术条件的限制,常规霍普金森杆无法实现材料的动态加载-卸载-再加载。为了解决这一问题,Thakur[17]等采用单次加载的霍普金森杆实验技术,先对材料进行单次拉伸加载至一定塑性,再截取试样的部分标距段进行单次的压缩加载,该方法可以获得材料的动态包辛格效应响应,但是缺点在于实验过程复杂且不连续,无法计及连续冲击过程中的热的影响。Nie等[18]利用电磁能量装置转换技术,开发了电磁霍普金森杆实验装置。该装置不仅可以较好地实现常规霍普金森杆设备的功能,并且在同步性、可控性等方面也有很大的提升,能够有效地产生重复性很好的应力波,且能够对试样进行同步双向加载。
基于电磁霍普金森杆实验技术,本文中提出一种单轴双向非同步加载的霍普金森杆(asynchronous-loading split Hopkinson bar)实验技术,使得试样两端的波导杆分别向试样施加压缩波和拉伸波,并在时间上非同步加载,使试样能够先后受到压缩波和拉伸波的加载,从而实现对材料动态包辛格效应的测试工作。
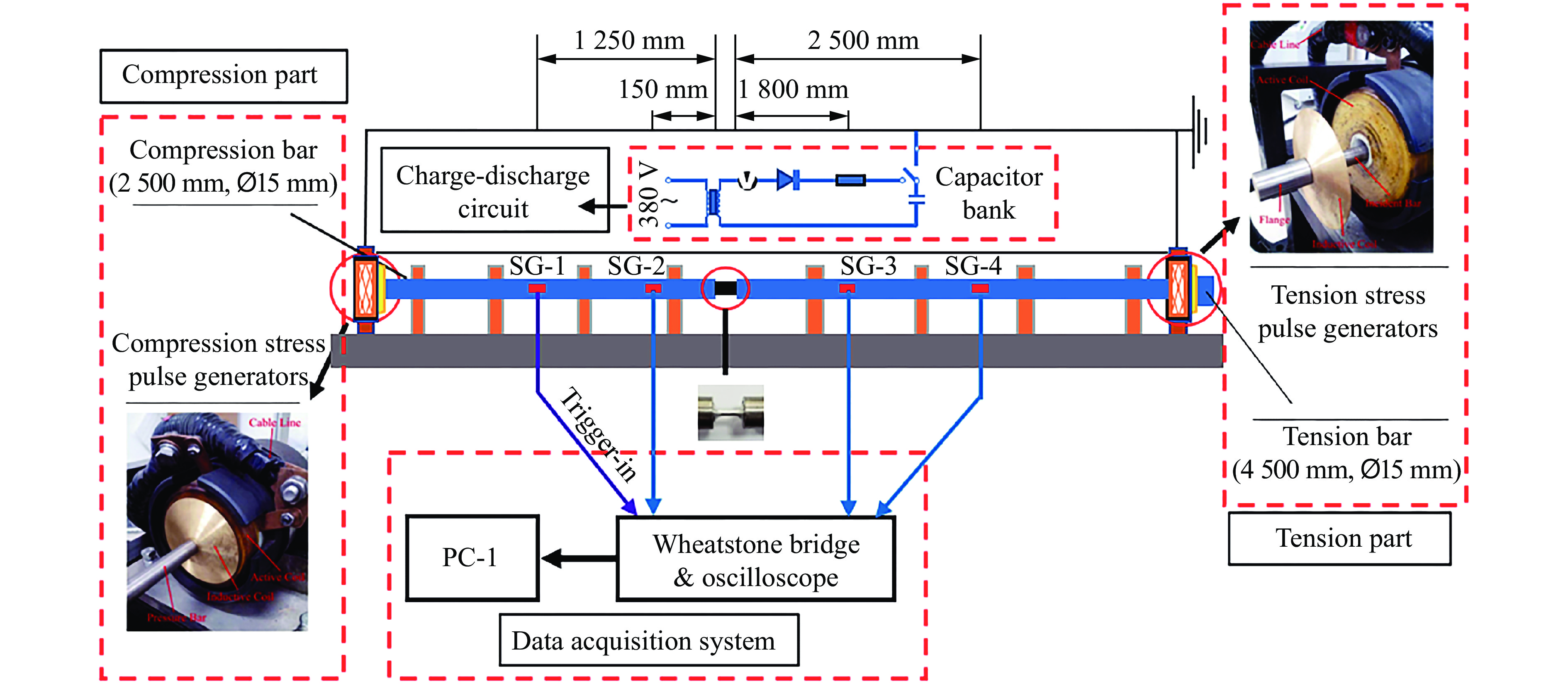
1. 动态单轴双向非同步加载装置
本文中基于电磁霍普金森杆提出了一种动态单轴双向非同步加载实验装置,如图2所示,组成部分包括了电路的充放电部分,应力波发生器电路、波导杆、数据采集系统等。关于电磁霍普金森杆的详细信息可以参考文献[18]。装置的主要特点在于:两个应力波发生装置分别产生压缩波与拉伸波,且能保持波形的一致性,由于采用并联电路进行电磁驱动,因此应力波的同步性可以控制在2 μs内。利用一维应力波理论,计算并设计两根波导杆的长度,从而使两列加载波无叠加地连续作用于试样。其加载过程为连续的动态压缩-卸载-再动态拉伸加载的过程,即为动态压缩-动态拉伸。
在波传播的过程中,需要注意波形的分离与采集问题,如图2所示,在−1 250 、−150、1 800和2 500 mm处分别设置采集点SG-1、SG-2、SG-3及SG-4,可以采集到较为理想的完整波形,其特点在于各个波形之间的分离,且保证了两列波的加载之间保留了100 μs的间隔,具体的内容可见表1。
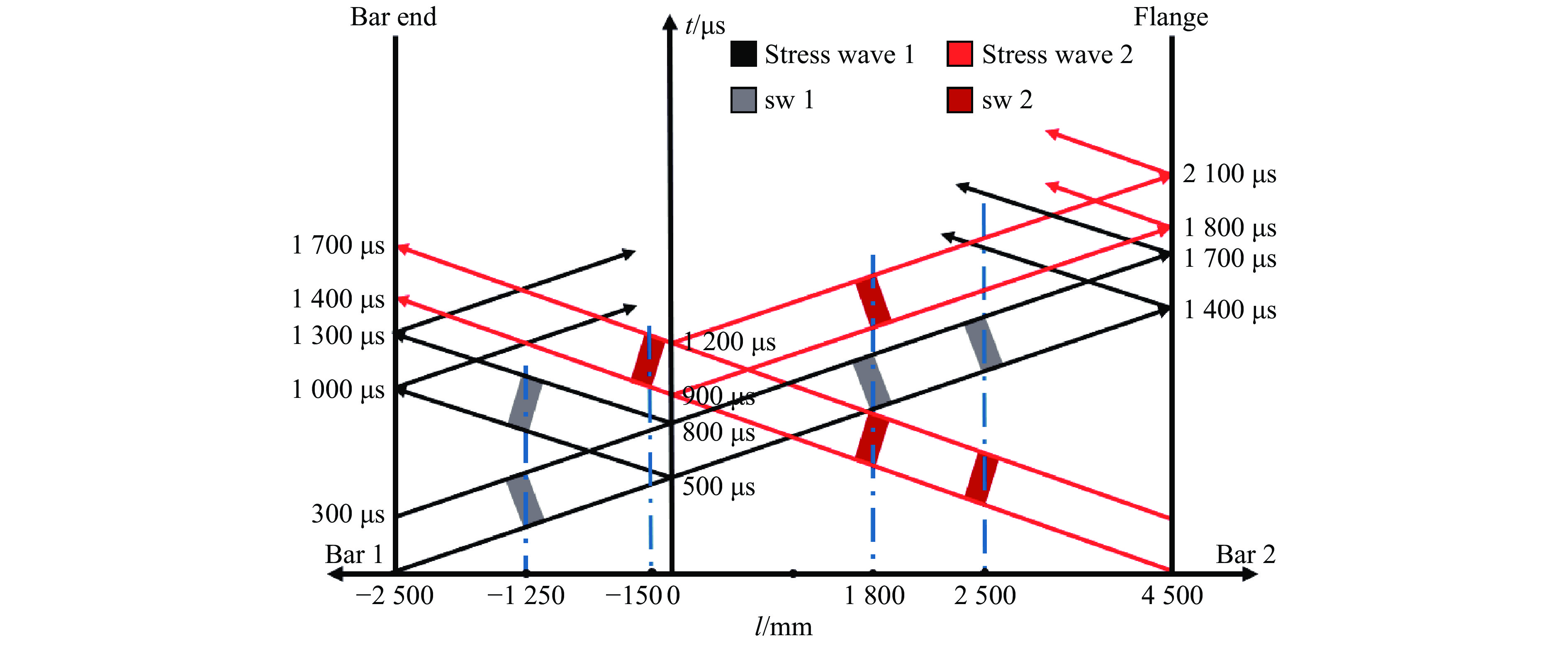
表 1 采集点可采集到的应力波Table 1. Stress waves collected at acquisition points采集点 −1 250 mm −150 mm 1 800 mm 2 500 mm εI1 − εI2 εI2 采集波形 εR1 εT2. εT1 εT1 − − εR2 − 注:(1) εI1为第1列波的入射波;(2) εR1为第1列波的反射波;(3) εT1为第1列波的透射波;(4) εI2为第2列波的入射波;(5) εR2为第2列波的反射波;(6) εT2为第2列波的透射波。 电磁霍普金森杆可以通过调节电容器电容值来控制应力波脉宽[18],以300 μs脉宽的两列先压缩再拉伸的加载波为例,通过对加载波的传播过程分析,加载可以分为以下几个过程,如图3所示,其中Stress wave 1指第1列应力波,Stress wave 2指第2列应力波,sw1和sw2为指定位置应变片采集到的两列应力波信号。
0~500 μs过程:两列应力波
εI1 和εI2 同时从应力波发生器产生并沿着波导杆传播,其中一列压缩波,另一列拉伸波;500~800 μs过程:短杆上的应力波对试样进行加载,其加载原理与传统霍普金森杆一致,即入射波
εI1 在端面一分为二,一部分反射波εR1 被远端的应变片采集,另一部分透射波εT1 穿过试样由长杆上的远端应变片采集,此时另一列入射波εI2 仍在沿着波导杆传播。在这一过程中第1列波的透射波εT1 与第2列波的入射波εI2 会产生部分叠加,但随着波的传播,两者会分离,不影响采集过程;800~1 200 μs过程:此时第1列波对试样的加载过程结束,且中间经历100 μs的间隔,此后另一列波对试样进行加载,其过程与第1列波类似,即入射波
εI2 在加载界面分离为反射波εR2 和透射波εT2 ,信号分别被相应的应变片采集,加载完成。根据前文的分析,由于两次加载实际是分开的,因此其数据处理的方式同传统Hopkinson杆的数据处理方式相同,对于本装置而言,两根波导杆实际可以先后分别作为入射杆与透射杆。如图4所示,粘贴在波导杆上的应变片可以分别测得入射波
εI1,2 、反射波εR1,2 和透射波εT1,2 。根据一维应力波理论[19],两次加载中试样的应力、应变和应变率曲线均可由下式计算得到:
˙ε(t)=2c0LεR(t) (2) εs(t)=2c0L∫t0εR(t)dt (3) σs(t)=E(AAs)εT(t) (4) 式中:
A 为杆的截面积,E 和c0 分别为杆的弹性模量和弹性波波速,As 和L 分别为试样标距段的截面积和长度。2. 6061铝合金的动态包辛格效应
2.1 实验方案
实验选用材料为6061铝合金,其基本材料参数如表2所示,实验设定为测试一次压-拉循环下的包辛格效应响应。
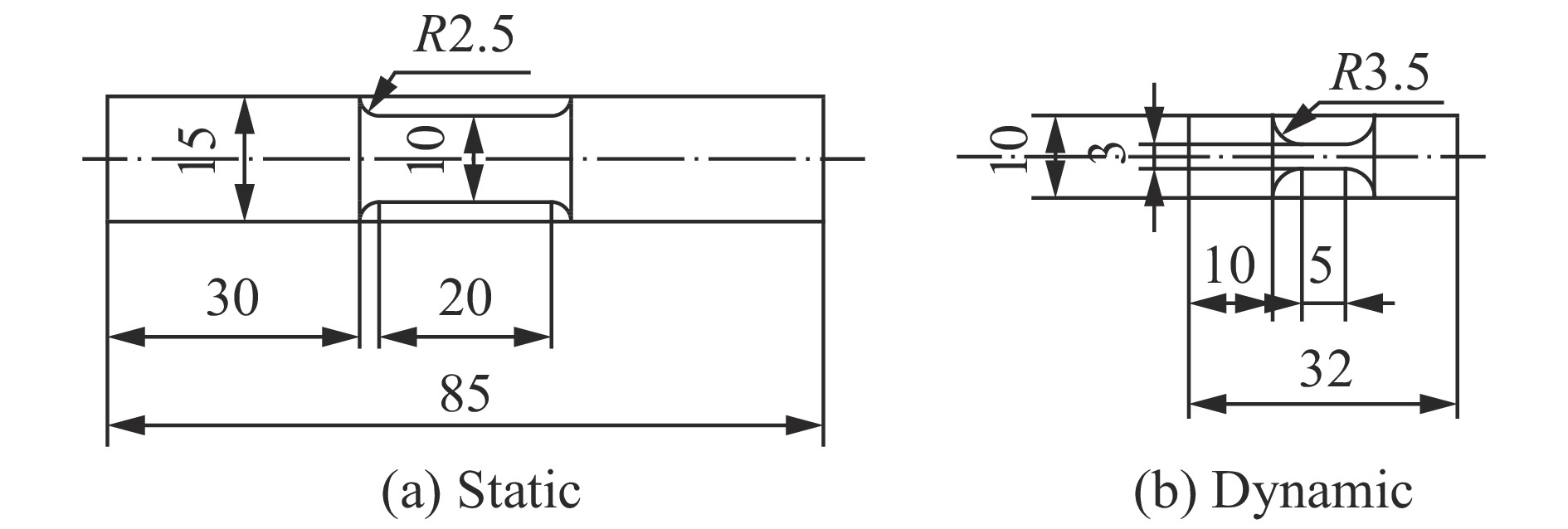
表 2 材料参数Table 2. Material Parameters密度/(kg·m−3) 弹性模量/GPa 屈服强度/MPa 泊松比 2.7×103 70 360 0.33 准静态实验采用万能试验机(LVF 100-T1000 HH)对受试样品进行连续的预压缩-卸载-拉伸实验,使用了数字图像相关(digital image correlation, DIC)技术修正了试样的标距段应变。试样形状尺寸如图5(a)所示,实验中使用液压卡盘垂直夹持试件,使其承受轴向拉伸载荷,预压缩过程中的最大应变为5%,全局应变率为10 −3 s−1,所测应力由机器中的力传感器测量。在实验过程中,将试样先压缩至预先指定的塑性应变,然后在相同条件下,对这些预变形试样进行连续拉伸实验。
动态实验使用直径15 mm的TC4钛合金杆作为波导杆,两杆长度分别为4500和2500 mm。波导杆的弹性模量为110.78 GPa,波速为4961 m/s。试样形状尺寸如图5(b)所示,采用螺接的方式与波导杆连接。通过粘贴在波导杆上的应变片测定实验所需的应变信号。为了获得与准静态实验一致的预应变,通过控制入射波幅值的方式使动态压缩过程的最大应变为5%,波导杆上加载的应力峰值为40 MPa。为了保证实验结果的可靠性,每组实验均保证重复2~3次。
2.2 实验波形分析
动态连续非同步加载实验的原始波形如图6所示,即为5%预应变下的波形图,与理论分析一致,从原始波形中可以直接得到压缩与拉伸的入射波、反射波和透射波,波形的变化历程也反映了加载历程,表明了加载过程中应力波不叠加。
如图7所示,两列入射波均呈近似正弦波形状,其原因在于加载过程中放电电流为正弦波电流,经过电磁感应后产生的应力波也为正弦波形,两列波的脉宽均为260 µs,峰值应力约为40 MPa,两列波实际在试样上加载时间间隔为130 µs。由于卸载过程速度更慢,如图8所示,本实验中透射波脉宽约为380 µs,则实际间隔小于10 µs,可以认为加载是连续的。在Nie等[20]的研究中,该波形对于常规金属材料的加载是适用的。
动态加载中应当保证加载过程的应力平衡[21],实现波形的分离后可以分别得到拉伸和压缩过程中的3个波形,根据应力平衡的原则,当
εI1,2=εR1,2+εT1,2 时,说明加载过程达到了应力平衡,如图8所示,可以证明实验过程应力平衡,设备可以用于测试材料的动态包辛格效应。2.3 实验结果与讨论
图9为0.001 s−1应变率下材料预压缩-拉伸加载的应力应变曲线,实验设定其预压缩加载至5%时卸载并反向加载,实际最大正向应变为5.2%,压缩时有明显的应变硬化,最大流动应力为(387±5) MPa,随后卸载并反向加载,对于没有明显屈服点的应力应变曲线,采用0.2%应变时的弹性模量与曲线交点来确定屈服应力[7],拉伸屈服应力为(330±5) MPa。实验结果表明6061铝合金在预压缩-拉伸条件下表现出包辛格效应,且屈服后出现了应变硬化,反向加载的流动应力超过正向加载。根据式(1)计算可得准静态下6061铝合金的
βσ 值为0.07。根据2.2节中分离后的波形,可以通过计算分别得到其应力应变、应变率曲线,如图10所示。由材料的应力-应变/应变率曲线可知,材料的应变率是呈正弦波变化的,这意味在现有的实验条件下无法实现常规的恒应变率加载,但Nie等[20]的实验证明,对于诸如铝合金等率不敏感材料,虽然应变率未能实现恒定,但通过与恒应变率实验结果的对比表明,两者没有明显的差异,因此应变率呈正弦波状是可以接受的,本文中取应变率的平均值。
图10所示的实验的平均应变率为350 s−1,最大正向应变为5.5%,由于加载初期应力不平衡的问题[11]可能导致应变不准,但随着变形增加其应变逐渐趋于准确。动态压缩最大流动应力为(390±2) MPa,反向加载时动态拉伸的应力应变曲线存在圆化和软化现象,采用0.2%应变结合弹性段的变化来确定其屈服点时,存在2%的误差,属于合理范围,动态拉伸时的屈服应力为(253±3) MPa。实验结果表明,与多数其它铝合金类似[22],动态压缩时6061铝合金未表现出明显的应变率效应,但应变硬化现象降低甚至消失。该材料同样会表现出包辛格效应,但屈服应力下降更明显,且屈服后流动应力未能超过正向加载。根据式(1)计算可得动态下6061铝合金的
βσ 值为0.17。准静态与动态实验结果表明,6061铝合金在动态压缩-动态拉伸条件下的包辛格效应受应变率的影响,从准静态到动态,材料的
βσ 值从0.07增大至0.17,这说明在5%动态压缩-动态拉伸的加载条件下应变率对于6061铝合金的包辛格效应有增强作用。此外,通过对比反向加载时准静态和动态加载的应力应变曲线,可以发现动态加载会导致材料的流动应力出现明显软化,其原因有待进一步分析。一般认为,常用铝合金是率不敏感材料,即其屈服和流动应力都具有较低的应变率依赖性,因此在很多工程应用中都不考虑铝合金的应变率效应,而是都以准静态数据来代替动态数据。实验结果表明,虽然6061铝合金的初始屈服和流动应力基本不受应变率的影响,但是其后续动态响应(包辛格效应)明显依赖于应变率的大小。从这个意义上说,此种材料的力学性能具有很强的应变率依赖性,如果完全采用准静态数据来描述其动态力学行为必然会导致较大误差。3. 结 论
本文中提出了一种基于电磁霍普金森杆平台的非同步加载实验装置,该装置可以用于材料动态包辛格效应的测试工作,其原理是基于电磁作用产生的两列应力波对试样进行连续拉伸-压缩(或者压缩-拉伸)加载,该方法的优势在于动态连续加载,且可以保证拉伸与压缩过程的一致性。该装置可以保证某些高应变率下温升较高的材料在连续变形时的软化效应不被忽略。采用该实验装置测试了6061铝合金的动态包辛格效应,并与同等条件下的准静态实验结果进行对比,实验结果表明,5%预应变的压缩-拉伸条件下应变率对材料的包辛格效应有强化作用,且动态加载过程中反向流动应力有明显的下降。6061铝合金的首次加载屈服、流动应力基本不受应变率影响,而其在动态下的包辛格应力参数与准静态相比却提升了143%,这说明6061铝合金的包辛格效应具有强烈的应变率依赖性。从该角度上说,一般认为的铝合金力学性能应变率敏感性低的观点并不严谨和全面,而以静态数据代替动态数据的做法可能会带来很大误差。
参加本文实验工作的还有硕士研究生赵先航、江斌、李建,博士研究生侯乃丹为本文提供了修改建议,在此表示谢意。
-
表 1 采集点可采集到的应力波
Table 1. Stress waves collected at acquisition points
采集点 −1 250 mm −150 mm 1 800 mm 2 500 mm εI1 − εI2 εI2 采集波形 εR1 εT2. εT1 εT1 − − εR2 − 注:(1) εI1为第1列波的入射波;(2) εR1为第1列波的反射波;(3) εT1为第1列波的透射波;(4) εI2为第2列波的入射波;(5) εR2为第2列波的反射波;(6) εT2为第2列波的透射波。 表 2 材料参数
Table 2. Material Parameters
密度/(kg·m−3) 弹性模量/GPa 屈服强度/MPa 泊松比 2.7×103 70 360 0.33 -
[1] BAUSCHINGER J. Changes of the elastic limit and the modulus of elasticity on various metals [J]. Zivilingenieur, 1881, 27: 289–348. [2] STOLTZ R E, PELLOUX R M. The Bauschinger effect in precipitation strengthened aluminum alloys [J]. Metallurgical Transactions A, 1976, 7(8): 1295–1306. DOI: 10.1007/BF02658814. [3] STOUT M G, ROLLETT A D. Large-strain Bauschinger effects in fcc metals and alloys [J]. Metallurgical Transactions A, 1990, 21(12): 3201. DOI: 10.1007/BF02647315. [4] FREDERICK C O, ARMSTRONG P J. A mathematical representation of the multiaxial Bauschinger effect [J]. Materials at High Temperatures, 2007, 24(1): 1–26. DOI: 10.3184/096034007X207589. [5] BUCKLEY S N, ENTWISTLE K M. The Bauschinger effect in super-pure aluminum single crystals and polycrystals [J]. Acta Metallurgica, 1956, 4(4): 352–361. DOI: 10.1016/0001-6160(56)90023-2. [6] ATKINSON J D, BROWN L M, STOBBS W M. The work-hardening of copper-silica: IV: the Bauschinger effect and plastic relaxation [J]. Philosophical Magazine, 1974, 30(6): 1247–1280. DOI: 10.1080/14786437408207280. [7] MOAN G D, EMBURY J D. Study of the Bauschinger effect in Al-Cu alloys [J]. Acta Metallurgica, 1979, 27(5): 903–914. DOI: 10.1016/0001-6160(79)90125-1. [8] HIDAYETOGLU T K, PICA P N, HAWORTH W L. Aging dependence of the Bauschinger effect in aluminum alloy 2024 [J]. Materials Science and Engineering, 1985, 73: 65–76. DOI: 10.1016/0025-5416(85)90296-4. [9] 唐长国, 朱金华, 周惠久. 金属材料屈服强度的应变率效应和热激活理论 [J]. 金属学报, 1995, 31(6): 248–253. DOI: 10.1007/BF02943514.TANG C G, ZHU J H, ZHOU H J. Correlation between yield stress and strain rate for metallic materials and thermal activation approach [J]. Acta Metallrugica Sinica, 1995, 31(6): 248–253. DOI: 10.1007/BF02943514. [10] HOPKINSON B. X A method of measuring the pressure produced in the detonation of high, explosives or by the impact of bullets [J]. Philosophical Transactions of the Royal Society of London: Series A: containing Papers of a Mathematical or Physical Character, 1914, 213(497-508): 437–456. DOI: 10.1098/rsta.1914.0010. [11] MIAO Y, DU B, SHEIKH M Z. On measuring the dynamic elastic modulus for metallic materials using stress wave loading techniques [J]. Archive of Applied Mechanics, 2018, 88(11): 1953–1964. DOI: 10.1007/s00419-018-1422-6. [12] MIAO Y, DU B, MA C, et al. Some fundamental problems concerning the measurement accuracy of the Hopkinson tension bar technique [J]. Measurement Science and Technology, 2019, 30(5): 055009. DOI: 10.1088/1361-6501/ab01b5. [13] 胡时胜, 王礼立, 宋力, 等. Hopkinson压杆技术在中国的发展回顾 [J]. 爆炸与冲击, 2014, 34(6): 4–20. DOI: 10.11883/1001-1455(2014)06-0641-17.HU S S, WANG L L, SONG L, et al. Review of the development of Hopkinson pressure bar technique in China [J]. Explosion and Shock Wave, 2014, 34(6): 4–20. DOI: 10.11883/1001-1455(2014)06-0641-17. [14] 李玉龙, 索涛, 郭伟国, 等. 确定材料在高温高应变率下动态性能的Hopkinson杆系统 [J]. 爆炸与冲击, 2005, 25(6): 487–492. DOI: 10.11883/1001-1455(2005)06-0487-06.LI Y L, SUO T, GUO W G, et al. Determination of dynamic behavior of materials at elevated temperatures and high strain rates using Hopkinson bar [J]. Explosion and Shock Waves, 2005, 25(6): 487–492. DOI: 10.11883/1001-1455(2005)06-0487-06. [15] 李玉龙, 郭伟国. 微型 Hopkinson 杆技术 [J]. 爆炸与冲击, 2006, 26(4): 303–308. DOI: 10.11883/1001-1455(2006)04-0303-06.LI Y L, GUO W G. Miniature-Hopkinson bar technique [J]. Explosion and Shock Waves, 2006, 26(4): 303–308. DOI: 10.11883/1001-1455(2006)04-0303-06. [16] 果春焕, 周培俊, 陆子川, 等. 波形整形技术在Hopkinson杆实验中的应用 [J]. 爆炸与冲击, 2015, 35(6): 881–887. DOI: 10.11883/1001-1455(2015)06-0881-07.GUO C H, ZHOU P J, LU Z C, et al. Application of pulse shaping technique in Hopkinson bar experiments [J]. Explosion and Shock Waves, 2015, 35(6): 881–887. DOI: 10.11883/1001-1455(2015)06-0881-07. [17] THAKUR A, NEMAT-NASSER S, VECCHIO K S. Dynamic Bauschinger effect [J]. Acta materialia, 1996, 44(7): 2797–2807. DOI: 10.1016/1359-6454(95)00385-1. [18] NIE H, SUO T, WU B, et al. A versatile split Hopkinson pressure bar using electromagnetic loading [J]. International Journal of Impact Engineering, 2018, 116: 94–104. DOI: 10.1016/j.ijimpeng.2018.02.002. [19] 苗应刚, 李玉龙, 邓琼, 等. Investigation on experimental method of low-impedance materials using modified Hopkinson pressure bar [J]. Journal of Beijing Institute of Technology, 2015, 24(2): 269–276. DOI: 10.15918/j.jbit1004-0579.201524.0220.MIAO Y G, LI Y L, DENG Q, et al. Investigation on experimental method of low-impedance materials using modified Hopkinson pressure bar [J]. Journal of Beijing Institute of Technology, 2015, 24(2): 269–276. DOI: 10.15918/j.jbit1004-0579.201524.0220. [20] NIE H, SUO T, SHI X, et al. Symmetric split Hopkinson compression and tension tests using synchronized electromagnetic stress pulse generators [J]. International Journal of Impact Engineering, 2018, 122: 73–82. DOI: 10.1016/j.ijimpeng.2018.08.004. [21] RAVICHANDRAN G, SUBHASH G. Critical appraisal of limiting strain rates for compression testing of ceramics in a split Hopkinson pressure bar [J]. Journal of the American Ceramic Society, 1994, 77(1): 263–267. DOI: 10.1111/j.1151-2916.1994.tb06987.x. [22] FAN X, SUO T, SUN Q, et al. Dynamic mechanical behavior of 6061 Al alloy at elevated temperatures and different strain rates [J]. Acta Mechanica Solida Sinica, 2013, 26(2): 111–120. DOI: 10.1016/S0894-9166(13)60011-7. -











 下载:
下载:




































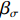

 下载:
下载: