Energy absorption of new thin-walled, multi-cellular, tubular structures with Sierpinski hierarchical characteristics under axial impact
-
摘要:
为提高薄壁结构的吸能能力,基于Sierpinski分形结构提出了一种具有层级特性的新型薄壁管,即Sierpinski层级管(Sierpinski hierarchical tube, SHT)。采用非线性有限元法对SHTs在轴向冲击载荷作用下的变形模式和能量吸收特性进行了数值分析,并与普通三角形薄壁管在轴向冲击载荷作用下的变形模式和能量吸收特性进行了对比。结果表明:SHTs的变形模式为轴对称渐进屈曲模式,在薄壁管中引入Sierpinski层级特性后,胞壁弯曲过程的半折叠波长减小,促使压缩过程中形成更多的塑性折叠单元,有利于提高薄壁结构能量吸收能力。进一步基于能量守恒理论和塑性铰理论对SHTs的轴向压缩应力进行理论求解,并通过有限元数值模拟验证其准确性。在相同的相对密度下,一阶、二阶及三阶SHTs的动态压缩应力较普通三角形薄壁管的动态压缩应力提高了85.8%、138.2%和183.8%。将Sierpinski层级特性引入薄壁管的设计中,能够有效提高薄壁管的耐撞性能。
-
关键词:
- 薄壁结构 /
- Sierpinski分形 /
- 轴向压缩 /
- 变形模式 /
- 耐撞性
Abstract:In order to improve the energy absorption capacity of thin-walled structures, a new type of thin-walled tube (SHT) with hierarchical characteristic was proposed based on the Sierpinski fractal structure. The deformation mode and energy absorption characteristics of SHTs under axial impact load were simulated using the nonlinear finite element method, and compared with those of ordinary triangular thin-walled tubes. The results show that the deformation mode of the new SHT is an axisymmetric progressive buckling mode. With the introduction of the Sierpinski hierarchical characteristics, the half-folded wavelength of the cell wall bending process is reduced, hence more plastic folding elements are formed and more energy is absorbed. Furthermore, theoretical expressions of the axial compression stress were obtained based on the energy conservation theory and plastic hinge theory. The correctness of the theoretical formula was verified by comparing with the finite element simulation. The results display that under the same relative density, the dynamic compressive stresses of the first-, second- and third-order SHTs are 85.8%, 138.2% and 183.8%, respectively, higher than that of the ordinary triangular thin-walled tubes. The introduction of the Sierpinski hierarchical characteristics into the design of the thin-walled tubes can effectively improve the crashworthiness of the thin-walled tubes, and it can provide a reference for the research and design of new energy absorbers.
-
Key words:
- thin-walled structure /
- Sierpinski fractal /
- axial impact /
- deformation mode /
- crashworthiness
-
混凝土类材料(混凝土、砂浆、岩石等压缩强度对静水压力敏感材料的统称)被广泛应用于高层建筑、洞库、水坝、安全壳等各类国防和民用结构工程中,其力学性能的研究始终是工程界关注的热点。爆炸、冲击等强动载荷作用下,混凝土类材料的动态行为与其在准静态加载下所表现出的力学行为有显著区别,主要体现为应变率对材料本构关系及强度的影响,即应变率效应。自Abrams[1]发现混凝土类材料的动态压缩强度随应变率提高而增加的现象以来,基于落锤、霍普金森杆、液压伺服器、爆炸装置等动态加载方式的实验研究已被广泛开展。其中,分离式霍普金森压杆(split Hopkinson pressure bar,SHPB)技术是该类材料在中高应变率(102~104 s−1)下动态压缩力学性能测试的主要手段[2-4]。
由实验测得的结果被用来发展混凝土类材料的动态本构关系并确定其中的参数, 进而在强动载荷作用下的结构分析与数值模拟中被广泛应用[5-6]。这些实验结果的合理性和正确认知将直接影响结构抗冲击能力的预测、设计和评估的可靠性。尽管已有的实验结果均证实混凝土类材料的动态压缩强度遵守随应变率增大而提高的趋势,但其数值却存在相当大的离散性[2, 4],并由此导致了不同的溯源分析和应变率效应产生机制的解释。例如,有些学者认为实验观测所得应变率效应是该类材料的固有特性,结果的离散性应归因于材料离散性、测试技术差异、尺寸效应、边界条件等因素[7-8];另外一些学者则认为该类材料在高应变率下所表现出的特性可能代表试件中应力状态的改变,实验所得的表观应变率效应并不能代表真实的材料性能,观测到的应变率效应部分归结于上述因素,但其主要原因为材料特性(如密度、泊松比、摩擦角、膨胀角等)、试件尺寸、端面摩擦等所导致的不同程度的径向约束效应。后一观点最早来自于Green等[9]对岩石动态压缩强度测量结果的分析,他们基于典型岩石材料在SHPB实验中所测得的结果,指出试件中应力状态的改变导致了动态压缩强度随应变率的快速提高。Brace等[10]针对3种岩石材料进行了一维应变加载路径下的实验研究,并将其动态和静态压缩强度进行了比较,在动态强度提高应归因于材料固有特性还是应力状态改变的问题上,他们倾向于支持Green等[9]的观点。Jonach[11]针对两类岩石进行了系统的实验测试和理论分析,提出快速加载产生的径向惯性效应引发了围压,进而改变了试件的应力状态,从而导致静、动态加载体现不同的强度和迥异的破坏形式。Bishoff等[2, 12]针对混凝土材料开展了类似的研究,也提出了同样的定性观点。此后,Donze等[13]采用离散元方法对简化的混凝土SHPB实验模型进行了模拟,进一步证实横向惯性产生的围压导致了该类材料动态压缩强度的应变率相关性。由于种种原因,这些观点与研究在当时并没有被从事该类材料动态强度应变率效应的研究与应用群体普遍接受,从而导致大量直接基于实验数据的应变率效应公式[7-8, 14](一些代表性公式见图1)在较长一段时间内被错误应用。
Li 等[15]采用数值SHPB方法对不同应变率下试件中的应力状态进行了研究,发现在应变率较低(即低于转换应变率)时,试件可保持一维应力状态;当应变率高于转换应变率时,试件不再保持一维应力状态,基于此,明确提出转换应变率并不代表从应变率非敏感区到应变率敏感区的转换,而是代表SHPB试件中应力状态的转变。与此同时,明确指出基于实验的动态强度提高系数在计算与分析中被大量错误使用,使得混凝土类材料的动态压缩强度在转换应变率之上被人为的大大提高,从而导致错误的理论模型和危险的结构设计。这一工作在近二十年中从定性、定量分析和机理方面得到了较为系统的研究和发展(Li等[16], Zhang等[17-18],Lu等[19-21],Flores-Johnson等[22],Liu等[23]),为脆性材料SHPB实验技术的完善提供了坚实的理论支持。这些研究工作一方面带动了学术界对该问题的重视[24-34],另一方面引起了应用领域的改变,比如,LS-DYNA计算程序自971版本[5]起对K-C材料模型(Mat-072R3)使用的动态强度提高系数进行了最大阈值截断,以减小惯性围压引起的应变率效应影响。
需要特别指出的是,脆性材料动态强度提高系数被错误使用的问题在部分霍普金森杆实验者和测试结果的应用者中仍未被引起足够重视,并存在误解[35-39]。例如,Field等[4]尽管在其有关材料动态性能测试的综述文章中提到SHPB试件中应力状态的转变(即Brace等[10]的观点),但却将横向惯性对附加应力的影响与横向惯性对压缩强度的影响混为一谈。Field所在的剑桥大学卡文迪许实验室是霍普金森杆技术的发源地, 在材料动态性能测试方面作了大量世界领先的工作。这一事实表明, 当时的国际主流学术界尚未对混凝土类材料的转换应变率以及在此之上的应变率效应正确认知。
合理考虑应变率效应是建立混凝土类材料宏观动态强度模型的关键。目前,在这一研究工作方面,该类材料的宏观动态强度模型均采用一维应力加载路径下的动态压缩强度实验结果成比例放大宏观准静态强度模型的方法来实现,并将一维应力加载路径下的实验结果外推到一般应力加载路径的情况,如LS-DYNA中的K-C 模型[5],AUTODYN 中的R-H-T 模型[6]。然而,这一外推方法的合法性并没有得到充分验证,尤其是在一维应力路径下测得的表观应变率效应在转换应变率之上无法代表材料的真实应变率效应的背景下,此动态模型的建立方法就更缺乏存在的基础。准确的强度模型应充分体现材料的真实应变率效应以及该效应的应力状态依赖性。然而,相关研究工作尚未见报道。
基于当前的研究现状,本文将概述混凝土类材料动态压缩应变率效应研究的发展及问题,揭示混凝土类材料在不同应力状态下应变率效应的表现特征并分析其适用条件,最终在动态强度模型的建立中提出多维应力状态下应变率效应考虑方法。
1. 动态抗压强度提高系数
基于一维应力和一维应变路径的实验研究是冲击动力学领域研究材料性能的两类基本实验。两者所对应的应力分量(
σ11、σ22、σ33 )或应变分量(ε11、ε22、ε33 )分别具有如下特征:σ11≠0,σ22=σ33=0 ;ε11≠0,ε22=ε33=0 。在实验过程中,根据应力分量的特点,试件的应力状态可通过应力三轴系数η 表征:η=√2(σ1+σ2+σ3)3√(σ1−σ2)2+(σ1−σ3)2+(σ2−σ3)2 (1) 基于广义胡克定律,对应一维应力和一维应变的压缩状态,
η 的数值分别为[23]:η1D-stress=−13, η1D-strain=−1+ν3(1−2ν) (2) 式中:
ν 为材料泊松比。为了方便讨论,采用γ 表征该类材料的应变率效应[15],其定义为:γ=σy,dσy,s (3) 式中:
σy,d 、σy,s 分别为被测材料的动态和准静态压缩强度。下面将首先基于以上两类基本实验,简要概括混凝土类材料动态压缩强度提高系数的基本特征,进而扩展至复杂应力状态,探究该系数对应力状态的依赖性。
1.1 基于一维应力路径的霍普金森杆实验
绝大多数针对混凝土类材料动态压缩强度应变率效应的实验是基于一维应力状态假设开展的。图1中给出了该类实验的典型结果和基于实验数据所发展的有代表意义的理论模型。如图所示,在该应力状态下,动态强度提高系数随应变率的增加而增大,当应变率超越某一特定应变率(即转换应变率
˙εtr ,对混凝土类材料,˙εtr ≈101~102 s−1)后进入快速增加阶段。在应用领域,转换应变率标志着材料强度对应变率敏感的开始,其后续应变率所对应的提高系数应在强度模型中给予明确考虑。近年来,基于类似图1所示的实验数据,研究人员提出了大量动态强度提高系数模型,并被广泛应用于防护结构的响应预测和抗冲击设计中。其中,最具代表性的是欧洲混凝土协会[7]推荐的模型(CEB模型),该模型取30 s−1作为转换应变率,采用两段函数方式表达:
γ(˙ε)=(˙ε˙εs)1.026αs˙ε≤30s−1 γ(˙ε)=ςs(˙ε˙εs)1/3˙ε>30s−1 式中:
αs=1/(5+9fc/fco) ,ςs=106.156αs−2.0 ,˙εs=3.0×10−5s−1 ,fco=10MPa ,fc 为材料准静态压缩强度。为了在材料动态强度模型中考虑应变率效应,当前普遍采用按照一维应力路径测得的强度提高系数比例放大准静态强度的方式(如文献[5-6]):
σd(ε,˙ε)=σs(ε)γ1D-stress(˙ε) (5) 式中:
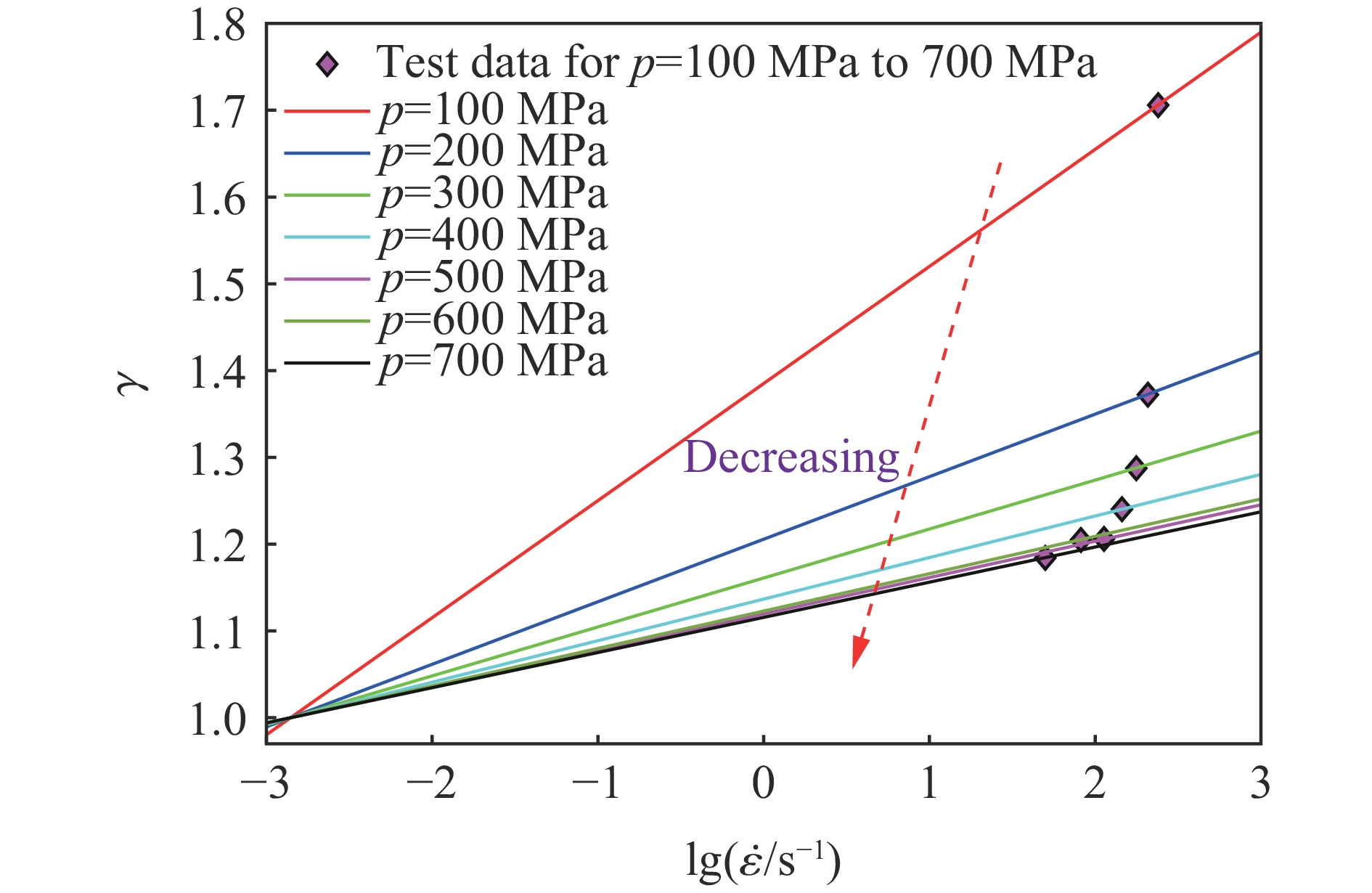
σd(ε,˙ε) 为动态强度,σs(ε) 为准静态强度,γ1D-stress(˙ε) 为基于一维应力路径的强度提高系数。这一处理方法在未被严格验证的情况下按照几何相似比例外推到一般加载路径,并在工程实际中被广泛应用。然而,近年来,针对图1所示实验数据产生机制的理解促使研究人员对动态强度提高系数的取值和宏观动态强度获得的合理性进行了严格审视和重新思考。如图2所示的系列研究表明[15,23],在SHPB实验中超越转换应变率后,试件动态强度的提高总是伴随试件应力状态自一维应力经多维应力到一维应变状态的演化。对于压缩强度为静水压力敏感性的混凝土类材料来讲,快速加载导致的横向惯性效应约束了试件的侧向变形,进而产生了可观的围压,从而导致了轴向强度的大幅提高。试件中围压数值的大小受外部条件(如端面摩擦)和内部条件(如试件尺寸、材料密度、泊松比、摩擦角、膨胀角等)的共同影响[22]。在该实验下实测的表观应变率效应包含大量的伪应变率效应(也称结构效应),不能代表该类材料的真实应变率效应,因此在使用前必须对其进行严格的修正[15,21,41]。学者们曾尝试采用空心试件[17]、波形整形[19]等技术手段克服这一缺陷,但均被证明只能缓解、不能完全消除横向惯性导致围压的影响,因此无法彻底排除伪应变率效应的存在。
基于以上分析,以往大量开展的所谓一维应力状态下的动态压缩实验其实是动态单轴压缩实验,在转换应变率之上无法在试件中实现真实的一维应力状态,因此也无法获取真实一维应力状态下材料的动态强度提高系数。将一维应力路径下的测试结果直接用于获取一维应力压缩强度随应变率的变化,将过高估计这类材料的动态压缩强度,必然导致错误的理论模型。
1.2 基于一维应变路径的实验研究
相比于一维应力路径下的实验,基于一维应变路径的研究要少得多。图3所示为花岗岩试件在一维应变实验条件下的准静态和动态应力、应变数据,准静态应变率为10−5 s−1,动态应变率范围为105~106 s−1。与一维应力路径下的实验显著不同,在3%的轴向应变(等同体积应变)下,径向应力始终处于相对较高的水平,轴向的动态与静态压缩强度相比无显著提高。
因该路径下实测的应力-应变关系没有明显的屈服点,Brace等[10,42]采用轴向应变为0.7%的静、动态强度计算其动态强度提高系数。采用相同的处理手段,花岗岩、石灰石和透水石3种材料的动态强度提高系数随应变率提高的变化关系可以统一表示为(见图4):
γ(˙ε)=1.0+k(lg˙ε˙ε0 + 4)−4<lg˙ε˙ε0<6 (6) 式中:
˙ε0 =1 s−1;k为拟合参数,对于花岗岩、石灰石和透水石分别取值为0.005、0.014及0.024。分析图4中数据的演化特点,可得如下两点启示:(1)对比图1所展示的数值,一维应变路径下测得的动态强度提高系数近似线性演化,缺失转换应变率,并且在高应变率下其数值大大减小;(2)尽管明确的应变率强化机理尚未被完全清楚地阐述,且不同材料可能具有不同的应变率敏感性,但混凝土类材料的应变率强化效应真实存在,其与应力状态密切相关,这种依赖性需要在强度方程中给予考虑。
鉴于爆炸、冲击等强动载荷作用下,材料可能更接近处于一维应变状态,Liu 等 [23]提出在进行材料动态强度计算时,采用基于一维应变路径下测量的强度提高系数,即:
σd(ε,˙ε)=σ1D-strain,s(ε)γ1D-strain(˙ε) (7) 式中:
σ1D-strain,s(ε) 、γ1D-strain(˙ε) 分别为一维应变路径下的准静态强度和动态强度提高系数。基于此动态强度模型开展的数值模拟及对比分析表明其可以获得较好的预测结果。1.3 复杂应力状态下的实验研究
意识到混凝土类材料的应变率效应可能是加载路径和应力状态相关的,学者们尝试应用围压设备配合霍普金森杆[43-44]或多组霍普金森杆联动[45-46]的方式开展研究。Johnson等[47]根据Piotrowska等[44]基于约束混凝土试件开展的霍普金森杆实验结果进行了分析,根据实验过程中实测的围压数值,总结了动态强度提高系数随应变率和围压数值的演化关系(见图5)。图中数据分布没有反映转换应变率的存在,表现出动态强度提高系数增长率随围压数值增大而逐渐降低的趋势,其演化规律更接近于一维应变路径下实验结果所体现的特点。然而,鉴于该类材料压缩强度的静水压力敏感性特征,当前该类技术的最大障碍在于在实验过程中无法保持恒定的应力状态,从而无法获得特定应力状态下的动态强度提高系数。
在动态强度模型的建立方面,目前尚无考虑应变率效应对应力状态依赖性的理论与方法,这正是本文中研究的主要内容。
2. 多维应力状态下应变率效应模型和实现方法
2.1 动态强度提高系数
以上分析和比较表明,针对混凝土类材料,两类基本实验所测得的动态强度提高系数变化趋势显著不同,即应变率效应与加载路径(即应力状态路径)密切相关。因此,合理的动态强度预测需要准确考虑加载路径和应力状态的影响,应采用如下更为合理的形式:
σd(ε,˙ε,η)=σs(ε,η)γ(˙ε,η) (8) 式中:
σs(ε,η) 、γ(˙ε,η) 分别为相同加载路径和应力状态下的准静态强度和动态强度提高系数。图6为基于不同加载路径所测的动态强度提高系数表现特征。在动态强度模型中对其合理考虑尚存在两个难点:(1)如1.3节分析,到目前为止,尚无法获得任意应力状态下该类材料的动态强度提高系数;(2)如1.1节所述,高应变率状态下(大于转换应变率),当前实验手段无法获得真实一维应力状态下的动态强度提高系数。
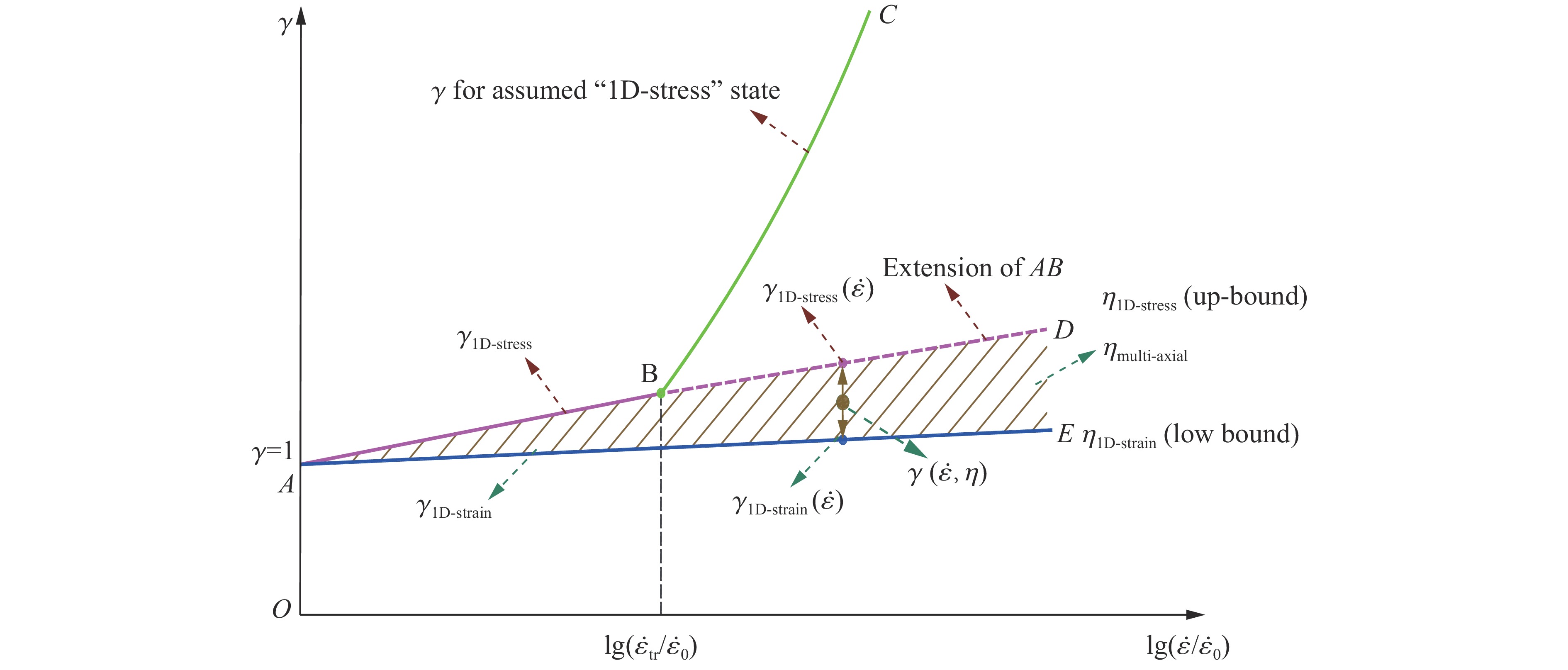
基于以上第一个难点,且考虑到一维应力和一维应变路径对应该类实验的两个极端工况,它们分别对应的动态强度提高系数应为其数值的包络(即上界与下界)。因此,建议采用线性插值的方式得到计及应力状态影响的动态强度提高系数,即:
γ(˙ε,η)=γ1D-strain(˙ε)+η−η1D-strainΔηΔγ(˙ε) (9) 式中:
Δγ(˙ε)=γ1D-stress(˙ε)−γ1D-strain(˙ε) Δη=η1D-stress−η1D-strain 其中:
η1D-stress 、η1D-strain 由式(2)确定,若η<η1D-strain ,取η=η1D-strain ;γ1D-strain(˙ε) 可由实验确定;γ1D-stress(˙ε) 的确定方法在下文讨论。由于实验手段无法得到
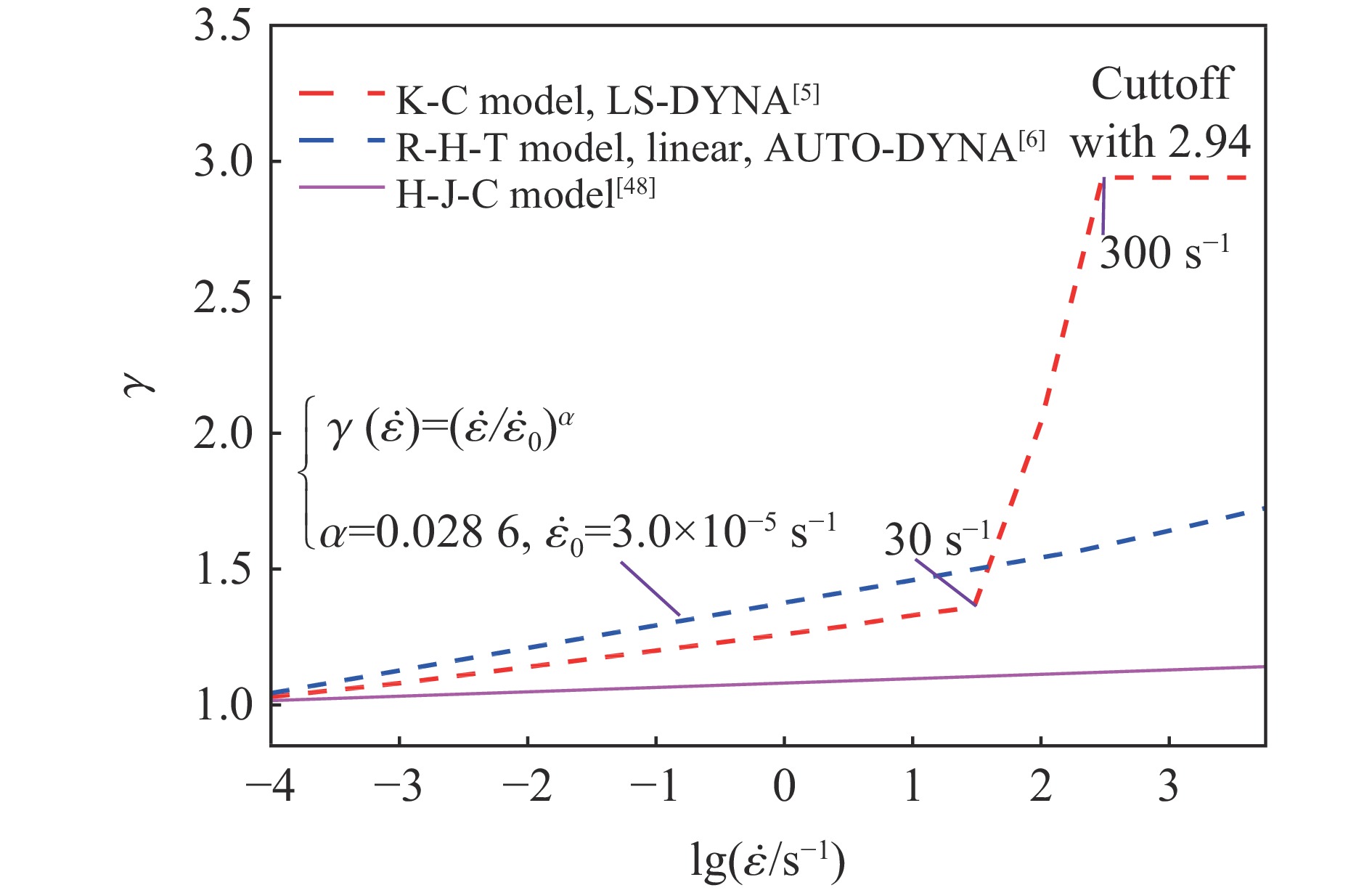
γ1D-stress(˙ε) ,因此需要给出一个合理可行的确定方法。研究人员就如何处理图6中BC段所包含的伪应变率效应进行了诸多尝试,处理方法大致分为2种:(1)基于实验数据处理或数值模拟的数据修正;(2)对实验数据采用线性延伸或阈值截断。第1种处理方法的典型代表是H-J-C模型[48]的建立。Holmquist等[48]意识到围压对混凝土类材料动态压缩强度的显著影响,因此,针对传统的一维应力路径下的实验数据,没有直接由测量得到的动、静态强度获得动态强度提高系数,而是在动态强度中去除围压影响后再计算该数值 [23]。其建议模型为(表现为不存在转换应变率的线性演化形式(见图7),与图6中AD段类似):
γ(˙ε)=1+Clg(˙ε/˙ε0) (11) 式中:
C=0.007 ,˙ε0 =1 s−1。第1种处理方法的另一代表性做法是基于数值模拟和迭代方法,通过反复对比模拟结果和实验数据对动态强度提高系数进行多次修正。Lu 等[21]和Liu等[41]应用此方法,针对岩石和聚合物的真实应变率效应进行了识别,发现高应变率下材料的真实动态强度提高系数显著低于实测数据,其差异程度与试件尺寸、材料特性相关,但采用图6中AD段的形式将获得该系数相对保守的估计。
第2种处理方法的典型代表是AUTO-DYNA计算程序中R-H-T材料模型[6]对应变率效应的考虑。该模型完全忽略转换应变率后的实验数据,直接基于转换应变率前的数据开展回归分析,建立类似式(4(a))的表达式(见图7)。此处理手段等同于将转换应变率前的实验数据线性拟合后再线性外延。
第2种处理方法的另一代表性做法是K-C模型[5]对动态强度提高系数阈值的设定。该模型的开发者们意识到CEB模型在高应变率下对应动态强度提高系数过大将导致错误的预测结果,因此对其适用范围进行了严格限制。具体做法是当压缩应变率大于300 s−1时不再按照式(4(b))进行该系数的预测,而是直接应用常数2.94作为阈值给予截断(见图7)。
第1种及第2种方法中的线性延伸法都可用来间接得到一维应力状态下材料的动态强度提高系数。根据图7,上述方法均体现了图6所示的通过线性延伸AB至AD得到一维应力状态下高应变率区域的动态强度提高系数的理念。第2种方法比第1种方法更简单直接,更易于被实验操作者和应用者接受,同时它们之间的差别也不是很大。鉴于此,在不失一般性的前提下,本文中采用线性延伸的方法考虑转换应变率后的动态强度提高系数。
需要特别指出的是,仍有大量的研究人员没有认识到如图6中BC段所示的动态强度提高系数存在大量伪应变率效应的事实,从而直接采用实测数据(或其回归公式)作为输入值进行模拟工作,这将导致错误的预测并可能引发灾难性的后果。Liu等[23]对比了不同计算模型在转换应变率后的动态强度提高系数,它具有如下排列关系:
γK-C\&γR-H-T-bilinear>>γR-H-T-linear>γH-J-C (12) 因此,建议在缺乏其他路径下动态强度提高系数的情况下,优先选择H-J-C模型和线性R-H-T模型进行数值模拟,以获得相对正确或偏于保守的响应预测。
2.2 多维应力状态下动态强度提高系数模型在强度方程中的实现
选择简单有效的Druker-Prager准则[49]配合上述应变率效应模型预测该类材料的动力行为,其表达式为:
Δσ=ptanβ+d (13) 式中:
Δσ=√3J2 ,p=−13(σ1+σ2+σ3) ,d=(1−13tanβ)fc ,β 为摩擦角,fc 为静态抗压强度,J2 为应力偏量第二不变量。在材料动态强度确定时,首先根据静态屈服面
Δσ(p) 计算Δσ(p/γ) ,然后通过动态放大系数γ 得到动态提高后的屈服面Δσe(p) [23, 50]:Δσe(p)=γd+ptanβ (14) 此外,应用p-α方程[51]考虑压力与体积的关系,其与强度(式(14))和应变率效应模型(式(9))通过Fortran语言编译为用户子程序后嵌入ABAQUS[51]计算平台。本文中仅选取Drucker-Prager准则和p-α方程作为示例阐明多维应力状态下应变率效应模型的有效性,其他可以准确体现静水压力影响的混凝土类材料模型均可以应用相同处理手段应用此模型。
3. 数值计算结果和讨论
3.1 数值霍普金森杆实验和计算参数
基于上述理论开展典型霍普金森杆实验的数值模拟(也称数值霍普金森杆实验,numerical split Hopkinson pressure bar,NSHPB),其装置如图8(a)所示。通过对比在无侧向约束、刚性侧向约束和非刚性侧向约束(分别对应图8(c)~(e))下数值预测结果与实验数据,展示不同应变率模型的特点并验证本文建议模型的有效性。
因部分文献未给出实验加载的细节(如撞击杆的长度和质量、入射速度和波型整形方式等),本文数值实验采用在入射杆端施加脉冲波的方式实现动态加载,并通过调整加载、持续时长和应力幅值实现各级应变率(如图8(b))。基于数值计算结果,应用下式进行轴向应力、应变和应变率的计算:
σaxial(t)=p1(t)+p2(t)2A0,εaxial(t)=∫t0˙εaxial(t)dt,˙εaxial(t)=v1(t) - v2(t)L0 (15) 式中:
v1(t) 、v2(t) 分别为入射与透射杆端的速度,p1(t) 、p2(t) 为相应杆端的接触力,L0 、A0 为试件原始厚度和截面面积。为保证结果准确,对数值模型进行了严格的网格敏感性分析,按照收敛性原则确定了网格尺寸。此外,通过下式对实验过程中是否达到动力平衡进行了检验(取R(t)≤5 %为评判阈值):R(t)=2|p1(t)−p2(t)p1(t)+p2(t)| (16) 为了方便与实验数据进行对比,在表1中列出了典型实验所涉及的材料、设备等参数,并将其作为基本输入被应用于本文的数值霍普金森杆实验中。对于在参考文献中缺失的部分必需参数,本文中采用类似实验对应的参数(如摩擦角和膨胀角等)给予了合理替代。尽管端面摩擦效应对实验数据的影响很大[15, 52],但考虑到实验操作者总是通过涂抹各类润滑剂等措施减小摩擦因数,同时文献中也没有明确端面摩擦参数的数值,因此本文中分析未考虑此效应的影响。一维应变和非刚性侧向约束的对比实验均给出了压力与体积应变的关系曲线,它们被直接用于数值模拟;此类数据在无侧向约束工况下普遍缺失,其状态方程按文献[23]中给出的建议考虑。
表 1 数值霍普金森杆实验中装置、试件的尺寸和材料参数Table 1. The dimension and material parameters in NSHPB test材料参数 一维应力 一维应变 多维应力 Zhang [17] Al-Salloum[53] Brace [10] Piotrawska [44] 试件直径/mm 37 73 18.5 40 试件密度/(kg∙m−3) 2116 2000 2650 2278 厚径比 0.5 0.5 2 1.25 准静态强度/MPa 51.0 63 — — 弹性模量/GPa 17.2 20 — — 泊松比ν 0.19 0.2 0.2 0.2 摩擦角/(°) 50[15] 50[15] 50[15] 50[15] 膨胀角/(°) 50[15] 50[15] 50[15] 50[15] 霍普金森杆直径/mm 37 75 37 80 霍普金森杆材料密度/(kg∙m−3) 7850 7850 7850 7850 霍普金森杆弹性模量/GPa 200 200 200 200 数值实验可获得实际测试中无法获得的除轴向以外的应力、应变分量以及应力状态等参数,从而为深入理解试件响应机理提供可靠依据。本文中基于如下方式表征以上变量 [23]:
ˉσk=1m×nm∑i=1n∑j=1σk(i,j)k = 1,2,3 ˉεk=1m×nm∑i=1n∑j=1εk(i,j)k = 1,2,3 ˉη=1m×nm∑i=1n∑j=1√2[σ1(i,j)+σ2(i,j)+σ3(i,j)]3√[σ1(i,j)−σ2(i,j)]2+[σ2(i,j)−σ3(i,j)]2+[σ3(i,j)−σ1(i,j)]2 式中:
σk(i,j) ,εk(i,j) 分别为(i,j) 位置处的k分量应力和应变,数字 1、2、3代表轴向、径向和环向分量。因为缺乏系统的实验数据,且鉴于CEB模型[7]被广泛使用,因此在本部分计算中选取该模型的首段表达式(式4(a))及其高应变率区的延伸线确定
γ1D-stress(˙ε) 。与此对应,按照式(6)确定γ1D-strain(˙ε) 。以上数据在相应实验完善后可以进行补充和修订。3.2 无侧向约束下的数值霍普金森杆实验
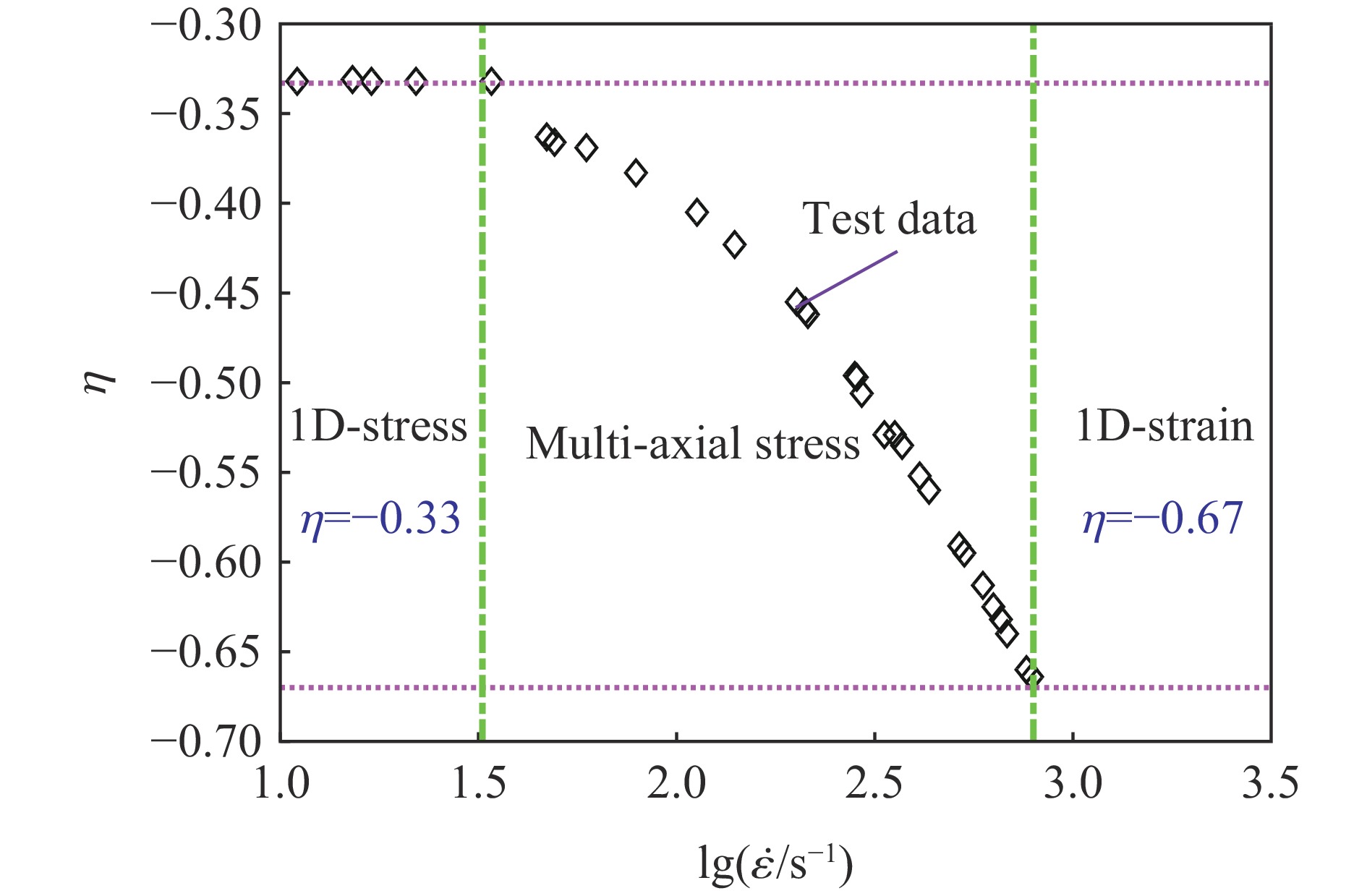
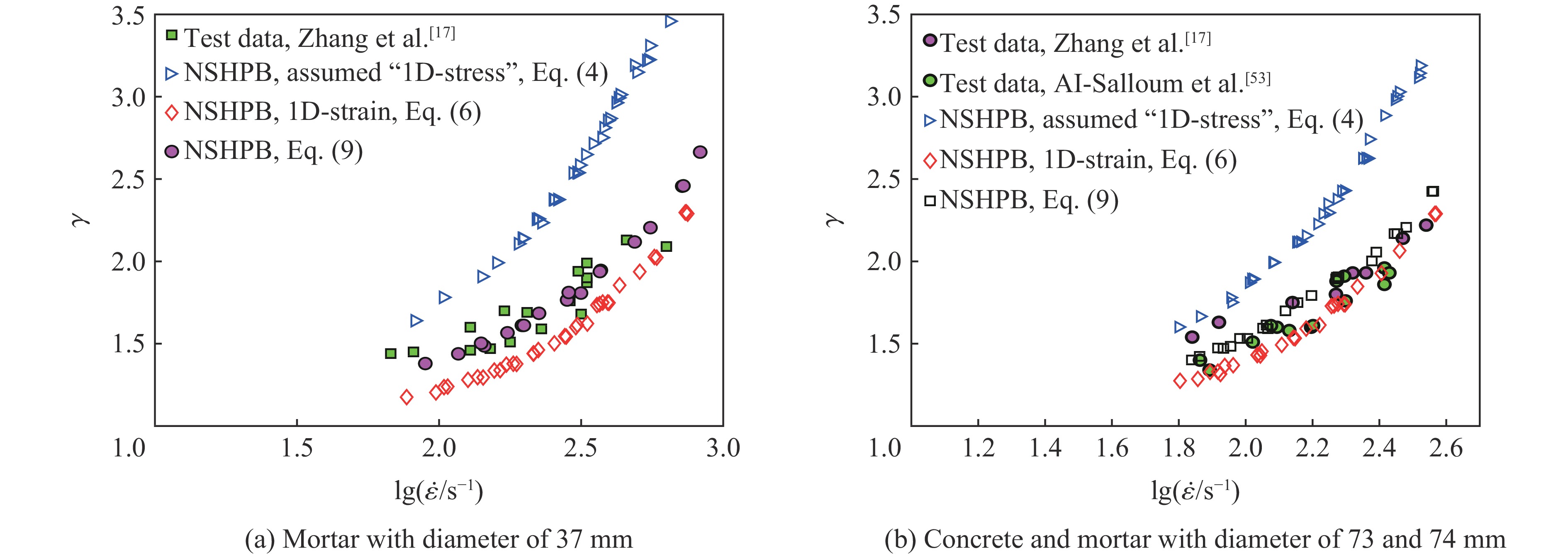
图8(c)中自由边界是该类实验的基本边界形式,通常用来测量试件的单轴动态抗压性能,在实际实验中应用最广。基于式(9)的多维应力状态应变率效应模型开展针对砂浆试件[17](直径37 mm)的数值实验,图9中给出了应变率282 s−1的加载下各应力分量的演化过程,其强度提高系数为1.76(静力单轴强度为51 MPa),后续的对比分析显示该数值与实验结果一致性较好。径向和环向应力的演化特征将响应过程分为特征明显的两个阶段:在轴向应变达到0.21%之前,径向和环向应力接近零,静水压力与轴向应力维持约1∶3的关系,说明此阶段试件处于严格的一维应力状态(对应应力三轴系数为−0.33);轴向应变大于0.21%后,径向和环向应力骤增,并在轴向应力达到峰值(90.01 MPa)时分别为19.19 和11.55 MPa,使试件应力三轴系数降至−0.54,表明试件已脱离初期的一维应力状态进入多维应力状态。
对应图9,图10中给出了试件在峰值应力时刻的应力三轴系数分布云图(据式(1))。图10表明,沿试件厚度方向,应力三轴系数分布具有较好的均匀性,处于动力平衡状态;在径向上此均匀性被完全打破:自试件外缘到内核,应力三轴系数逐渐降低,自一维应力状态(
η=−0.33 )到多维应力再到一维应变状态(η=−0.64 ,由泊松比为0.19,根据式(2)计算)顺次演化。以上分布特征准确反映了动力加载过程中横向惯性效应所产生的侧向围压以及由此导致的应力分布不均匀,此机制提高了该类静水压力敏感性材料的抗压强度,这与文献[15, 23]中的分析一致。为了清楚地考察压缩强度提高、应力状态改变与应变率的关系,图11总结了动态强度提高系数、应力三轴系数随应变率提高的演化过程。数据表明,阈值应变率89.5和554.3 s−1将试件应力状态分隔为一维应力、多维应力和一维应变三个应力状态。该类材料抗压强度的提高伴随应力状态由一维应力状态经多维应力到一维应变状态的演化,试件在达到峰值应力时刻其大部分材料可能处于多维应力状态(应变率89.5~554.3 s−1)或一维应变状态(应变率大于554.3 s−1)。复杂应力状态的出现显然违背了霍普金森杆实验关于试件应处于一维应力状态的基本假定,由此测量所得的试件表观强度并不能完全体现材料在一维应力状态下的真实应变率效应。
为了展现2.1节所述不同应变率模型的特点并验证本文提出模型的有效性,以下分别基于CEB模型(式(4))、一维应变路径应变率模型(式(6),k取为3个数值的中间值0.014)和本文所提多维应力状态模型(式(9))进行数值实验,并与实验结果进行对比。考虑到该加载路径下应变率效应与材料参数和试件几何尺寸具有强关联性[22-23],模拟分别针对直径37、73 mm的砂浆和混凝土试件开展。数值预测结果及相应的实验数据同时绘于图12中(为了增加实验数据点,直径74 mm的砂浆实验结果也在图12(b)中示出)。两者对比体现如下显著特点:(1)绝大多数实验数据处于基于CEB模型和一维应变路径数据模型的预测结果之间,可以认为,以上两个模型可作为动态强度提高系数的上限和下限;(2)基于CEB模型的预测数据明显高于实验数据,并且两者的差异随应变率的提高而显著增大,如图12(a),应变率分别对应81.21和457.09 s−1,动态强度提高系数的预测误差由0.19增至0.87,分别达到实测数值的42.2%和141.3%,表明CEB模型会导致动态强度的过高估计;(3)实验数据在转换应变率之上的较低应变率时更接近于本文建议模型(式(9))的预测数据,在较高应变率工况下,其数值更接近于一维应变路径应变率模型的预测数据,这与针对试件应力状态的分析结论相一致,同时也验证了选取CEB模型首段表达式(式(4a))的延伸线作为高应变率情况下动态强度提高系数的有效性。总的来讲,后两个应变率模型得到的结果相差不是很大。
3.3 一维应变路径下的数值霍普金森杆实验
选取文献[10, 42]中的花岗岩为研究对象,采用图8(d)所示的约束方式开展数值霍普金森杆实验,动态加载过程中试件的径向和环向位移被严格约束,符合一维应变加载路径要求。试件的静态加载下的状态方程和强度如图13所示,数值实验中应变率效应模型采用式(6)形式,k取为0.005。
图14中给出了典型加载下(应变率为515 s−1)的数值霍普金森杆预测数据,其与实验结果具有较好的一致性。在此加载路径下,应变率效应模型式(9)等同于式(6),因此两种模型的预测结果完全相同。以上对比分析表明,本文建议的多维应力状态动态强度提高系数模型在针对一维应变加载路径下也具有良好的适用性。
3.4 非刚性侧向约束下的数值霍普金森杆实验
本节针对图8(e)所示的具有非刚性侧向约束的试件[44]开展数值霍普金森杆实验,其约束方式如图15所示:直径40 mm、厚50 mm的试件被内径40.2 mm、外径80 mm、长50 mm的高强钢质圆环包裹,左右分别借助直径40 mm、高30 mm的高强钢质圆柱体垫块传递动力载荷。实际实验中,试件与约束环之间具有0.2 mm的缝隙,用以通过涂抹润滑剂减小试件与约束环内壁的摩擦。因无法准确把握此润滑层的力学性能,数值模拟未对此细节给予考虑,而是在模型建立时将约束环内径设为与试件直径相同并指定两者之间光滑接触(摩擦因数为零)。
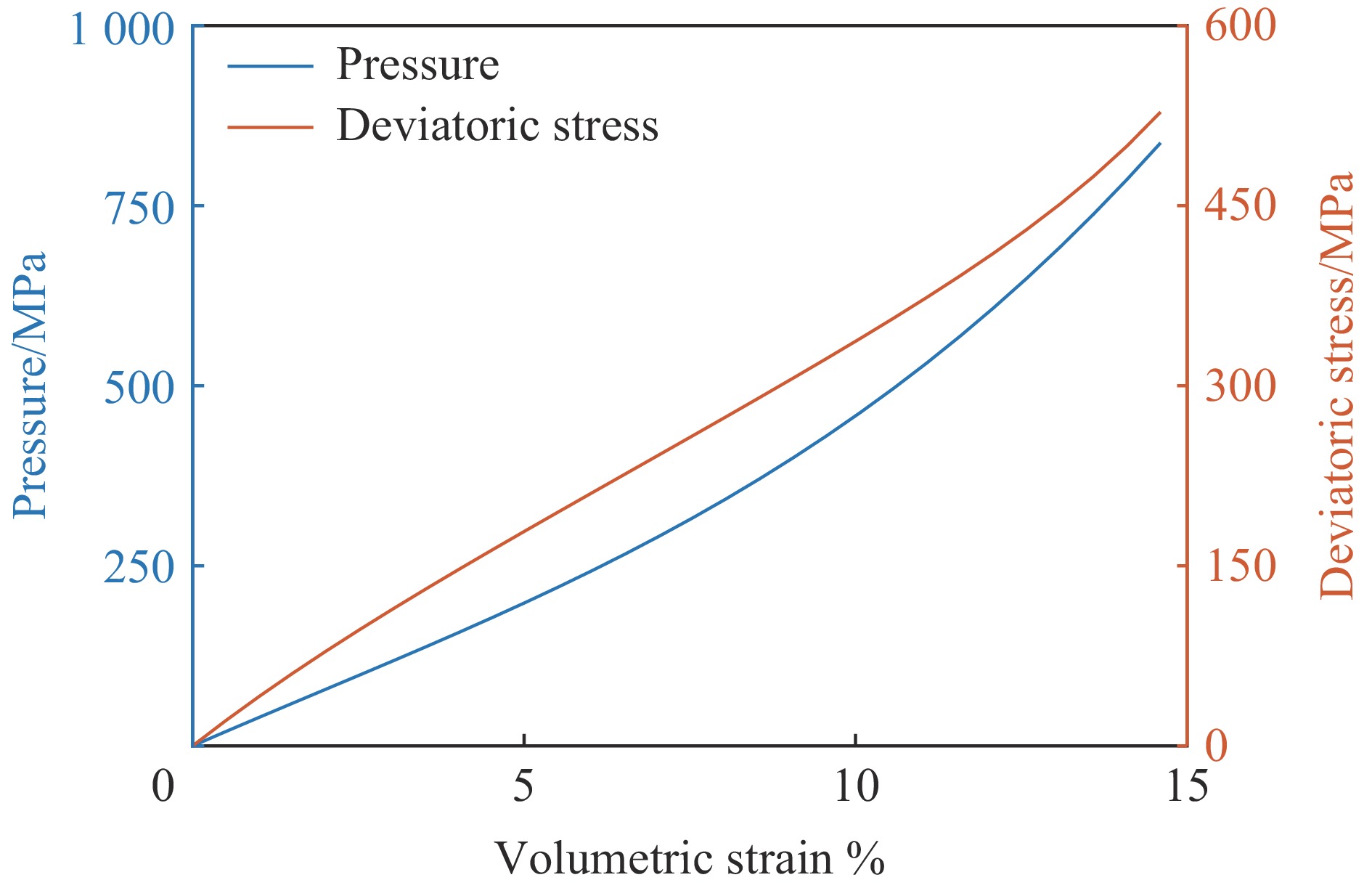
经静态围压实验测定的材料状态方程和偏应力强度如图16所示,将其分别作为基本输入数据用于后续的动力计算。约束圆环和垫块的弹性模量约为(
198±7 ) GPa[44](本文中计算取200 GPa),泊松比0.29,屈服强度1.8 GPa。实际实验中,试件的应力、应变分量和应力状态基于环向应变与内压的函数关系(针对约束装置)计算确定,在数值实验中,以上结果仍直接通过式(17)的处理方式获得。为了考察应变率对偏应力和状态方程的影响,分别通过下式计算试件偏应力、静水压力和体积应变[44]:
σdeviatoric=σaxial−σradial,p=σaxial+σradial+σhoop3,εvolumic=1−(1+εaxial)(1+εradial)2 (18) 应用式(9)对应的应变率效应模型,一维应力状态下动态强度提高系数取为CEB模型的首段延伸线,一维应变状态下动态强度提高系数取k值为0.014(式(6),材料为石灰石)对应的数据进行数值实验,图17中给出了试件应变、应力分量和应变率随加载时间(
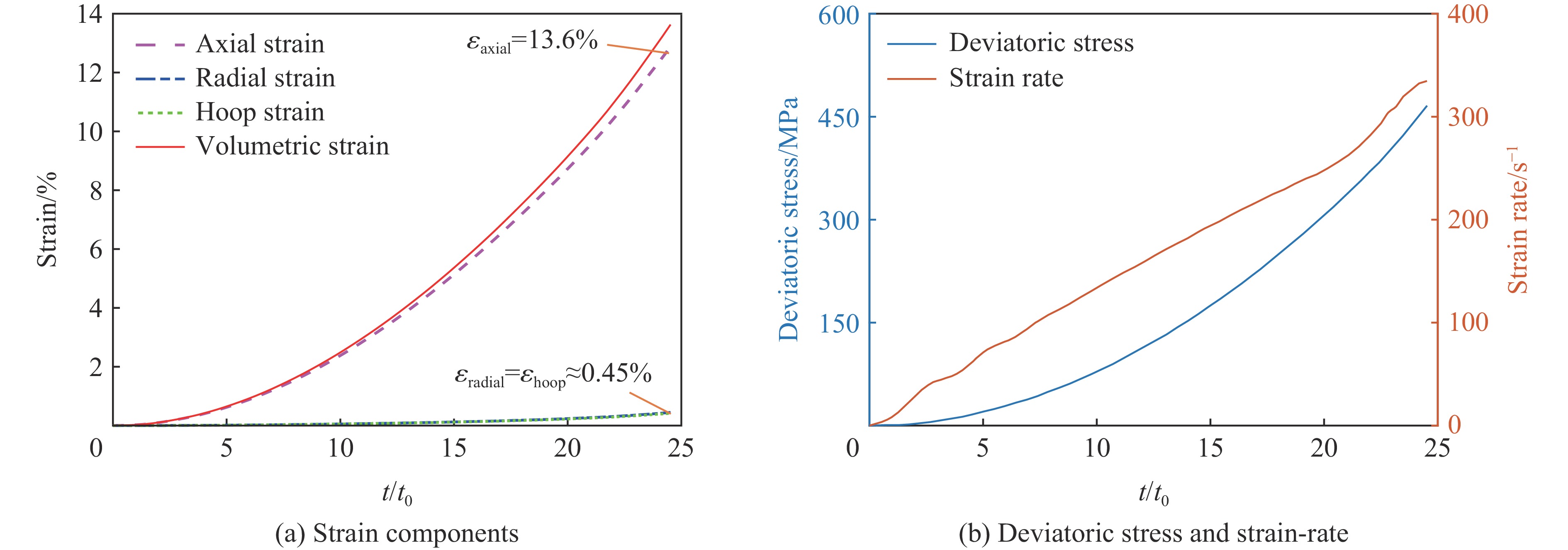
t0 为入射波在试件中单次传播经历的时间)的演化过程。加载过程中钢质垫块和约束圆环最大Mises应力分别为961.8和1081.4 MPa,小于材料弹性极限(1800 MPa),表明约束装置仍处于弹性状态,这与实验测量一致。从图17(a)中可以看到,随着试件轴向应变随加载时间的快速增加,径向和环向应变维持在较低的水平(轴向应变达到最大值13.6%时,径向和环向应变最大值约为0.45%),应力三轴系数接近−0.67(由泊松比为0.2,根据式(2)计算),表明试件在整个加载过程中处于准一维应变状态。该类实验无法获得恒定应变率下的材料动态强度,参考文献 [43-44]的处理方法,取加载过程的平均应变率(约151 s−1)作为此加载工况的应变率数值,这与实验数值(146 s−1)基本一致。图18中给出了数值实验得到的偏应力和状态方程预测数值以及与实验结果[44]的对比。在偏应力方面,依次采用分别对应花岗岩、石灰石和透水石的k值(式(6))将得到逐渐趋向实验值的结果,但在围压50~300 MPa范围内仍有较大差别,其原因主要来自以下3个实验中的不确定性。(1) 式(6)的数值来自3种不同类型的岩石,而文献[44]涉及的实验材料为添加硅质骨料的混凝土,这类骨料具有更高的压缩强度(330 MPa)和更小的孔隙率(低于2%)。因此,虽同属混凝土类材料,但其组分、微观结构与岩石及普通混凝土存在显著差异,进而导致其动态强度较高一些。(2) 实际实验对于该混凝土仅给出了2个实验工况,它们在具有几乎相同应变率(143、146 s−1)下,其实验数据在50~300 MPa围压范围内具有较大差别(见文献[44]中的图14),另一工况的实验结果(对应应变率143 s−1)与图18(a)所示的数据(对应应变率146 s−1)相比,数值较小,但因测试手段原因其数据振荡过大,不便使用。因此,图18(a)中的实验数据可能正确体现了材料动态性能演化的趋势,但在定量上有一定偏差。(3) 如前文所述,试件动态偏应力数值基于实测约束环的应变推算得到,该应变与环壁内压(对应试件的径向应力)作用面积、位置和量值密切相关,在实验前由静态加载下的数值模拟确定。实际实验中,动态加载导致的惯性效应和波传播效应使得响应行为较静态下复杂,而本模拟未考虑的试件与约束环间的缝隙也可能会放大此效应。此外,大的轴向压缩变形将导致试件与约束环内壁的接触面积减小并偏离初始位置(因试件与约束环近乎无摩擦)。在此情形下,采用初始按理想化情况确定的静态推演关系进行各应力分量等数值的计算也可能会引入误差,进而导致本文预测结果失去较为准确的评判标准。
在状态方程的对比方面,数值实验预测(采用3组k值)结果与输入曲线(静态实验结果)一致,但明显低于动态实验所测得的结果。此现象可能由2个因素造成,即:(1) 前述实验测量中的不确定性;(2) 与抗压强度类似,该类材料的状态方程可能也具有应变率敏感性,这在Cui等[54]研究者的工作中已经开始给予关注。因缺乏明确、足够的数据支持,本文模型对此未予考虑,可在未来相应研究工作完善后再补充。
4. 结 论
研究了不同应力状态下混凝土类材料动态压缩应变率效应的表现特征,将仅包含应变率的动态强度提高系数模型扩展至同时计及应变率和应力状态的多维应力状态形式。结合Drucker-Prager强度准则和p-α状态方程,该模型被成功应用于霍普金森杆实验的数值模拟。针对自由边界、刚性约束和非刚性约束SHPB试件的模拟及与实验数据的对比分析表明,发展多维应力状态下的应变率效应模型是必要的,它可以体现应变率效应随应力状态改变的特点,从而更准确地预测该类材料的动态压缩强度。研究结果有助于正确认识混凝土类材料的动态压缩应变率效应,并在分析与数值模拟中正确使用该类材料的动态压缩强度模型。
-
表 1 薄壁方形管动态平均压缩力模拟结果与实验数据[14]的比较
Table 1. Comparison between simulated and experimental mean dynamic compressive forces[14] for thin-walled square tubes
编号 Pdm/kN 误差/% 实验[14] 模拟 S2 10.26 10.86 5.8 S3 15.71 16.70 6.3 S4 18.83 19.47 3.4 S5 29.83 31.92 7.0 表 2 各采样点有限元结果与理论预测值对比
Table 2. Comparison of finite element results and theoretical predictions for all the sampling points
层级级数 ˉρ ti/mm li/mm σdm/MPa 相对误差/% 模拟 理论 0th 0.077 1.00 90 1.925 1.968 −2.18 0.100 1.30 2.840 2.917 −2.64 0.123 1.60 4.118 3.982 3.42 1st 0.077 0.67 45 3.577 3.523 1.54 0.100 0.87 4.948 5.221 −5.23 0.123 1.07 7.046 7.129 −1.17 2nd 0.077 0.44 22.5 4.586 4.446 3.14 0.100 0.58 6.407 6.590 −2.77 0.123 0.71 9.186 8.998 2.09 3rd 0.077 0.30 11.25 5.464 5.271 3.67 0.100 0.39 7.720 7.813 −1.19 0.123 0.47 10.972 10.669 2.84 -
[1] MCFARLAND R K. Hexagonal cell structures under post-buckling axial load [J]. AIAA Journal, 1963, 1(6): 1380–1385. DOI: 10.2514/3.1798. [2] WIERZBICKI T. Crushing analysis of metal honeycombs [J]. International Journal of Impact Engineering, 1983, 1(2): 157–174. DOI: 10.1016/0734-743X(83)90004-0. [3] WIERZBICKI T, ABRAMOWICZ W. On the crushing mechanics of thin-walled structures [J]. Journal of Applied Mechanics, 1983, 50(4a): 727–734. DOI: 10.1115/1.3167137. [4] TRAN T N, HOU S J, HAN X, et al. Theoretical prediction and crashworthiness optimization of multi-cell triangular tubes [J]. Thin-Walled Structures, 2014, 82: 183–195. DOI: 10.1016/j.tws.2014.03.019. [5] 尹汉锋, 文桂林. 基于简化基本折叠单元法的蜂窝耐撞性优化设计 [J]. 机械工程学报, 2011, 47(16): 93–100. DOI: 10.3901/JME.2011.16.093.YIN H F, WEN G L. Crashworthiness optimization design of honeycombs based on the simplified basic folding element method [J]. Journal of Mechanical Engineering, 2011, 47(16): 93–100. DOI: 10.3901/JME.2011.16.093. [6] SUN G Y, JIANG H, FANG J G, et al. Crashworthiness of vertex based hierarchical honeycombs in out-of-plane impact [J]. Materials and Design, 2016, 110: 705–719. DOI: 10.1016/j.matdes.2016.08.032. [7] MOUSANEZHAD D, EBRAHIMI H, HAGHPANAH B, et al. Spiderweb honeycombs [J]. International Journal of Solids and Structures, 2015, 66: 218–227. DOI: 10.1016/j.ijsolstr.2015.03.036. [8] SUN Y T, WANG B, PUGNO N, et al. In-plane stiffness of the anisotropic multifunctional hierarchical honeycombs [J]. Composite Structures, 2015, 131: 616–624. DOI: 10.1016/j.compstruct.2015.06.020. [9] 张越, 李世强, 王志华. 二阶层级自相似四边形蜂窝动力压缩行为数值模拟 [J]. 高压物理学报, 2017, 31(4): 358–363. DOI: 10.11858/gywlxb.2017.04.002.ZHNAG Y, LI S Q, WANG Z H. Dynamic crushing response of self-similar second order hierarchical square honeycombs [J]. Chinese Journal of High Pressure Physics, 2017, 31(4): 358–363. DOI: 10.11858/gywlxb.2017.04.002. [10] 于国际, 李飞鹏, 李世强, 等. 二阶层级自相似六边形蜂窝的面内压缩行为 [J]. 太原理工大学学报, 2018, 49(3): 494–500. DOI: 10.16355/j.cnki.issn1007-9432tyut.2018.03.021.YU G J, LI F P, LI S Q, et al. In-plane compressive behavior of a self-similar second order hierarchical hexagonal honeycomb [J]. Journal of Taiyuan University of Technology, 2018, 49(3): 494–500. DOI: 10.16355/j.cnki.issn1007-9432tyut.2018.03.021. [11] 赖燕辉, 江五贵, 吴瑶. 自相似多级纳米蜂窝铝结构力学性能的分子动力学模拟 [J]. 复合材料学报, 2019, 36(4): 946–953. DOI: 10.13801/j.cnki.fhclxb.20180517.001.LAI Y H, JIANG W G, WU Y. Molecular dynamics simulation on mechanical properties of nano self-similar hierarchical honeycomb Al [J]. Acta Materiae Compositae Sinica, 2019, 36(4): 946–953. DOI: 10.13801/j.cnki.fhclxb.20180517.001. [12] SIERPINSKI W. Sur une série potentielle qui, étant convergente en tout point de son cercle de convergence, représente sur ce cercle une fonction discontinue [J]. Rendiconti del Circolo Matematico di Palermo, 1916, 41(1): 187–190. DOI: 10.1007/BF03018294. [13] SANTOSA S P, WIERZBICKI T, HANSSEN A G, et al. Experimental and numerical studies of foam-filled sections [J]. International Journal of Impact Engineering, 2000, 24(5): 509–534. DOI: 10.1016/S0734-743X(99)00036-6. [14] ZHANG X, ZHANG H. Energy absorption of multi-cell stub columns under axial compression [J]. Thin-Walled Structures, 2013, 68: 156–163. DOI: 10.1016/j.tws.2013.03.014. [15] ABRAMOWICZ W, JONES N. Dynamic axial crushing of square tubes [J]. International Journal of Impact Engineering, 1984, 2(2): 179–208. DOI: 10.1016/0734-743X(84)90005-8. [16] CHEN W G, WIERZBICKI T. Relative merits of single-cell, multi-cell and foam-filled thin-walled structures in energy absorption [J]. Thin-Walled Structures, 2001, 39(4): 287–306. DOI: 10.1016/S0263-8231(01)00006-4. [17] HANSSEN A G, LANGSETH M, HOPPERSTAD O S. Static and dynamic crushing of circular aluminum extrusions with aluminum foam filler [J]. International Journal of Impact Engineering, 2000, 24(5): 475-507. DOI: 10.1016/S0734-743X(99)00170-0. 期刊类型引用(4)
1. 马俊,谢全民,万鹏,孙热,章逸锋,彭磊. 早龄期喷射混凝土冲击动力学响应及损伤演化试验研究. 振动与冲击. 2025(04): 176-183+206 .  百度学术
百度学术2. 宁建国,李玉辉,杨帅,许香照. 冲击载荷作用下含损伤的低温混凝土本构模型研究. 兵工学报. 2024(12): 4339-4349 .  百度学术
百度学术3. 韩家翀,吕林梅,胡利,高政,王润林,陈叶青. 钢纤维超高性能混凝土动态破坏机制及力学性能增强效应. 防护工程. 2024(06): 9-17 .  百度学术
百度学术4. 王海宁,徐为,张英姿,徐佳翔. 受酸雨腐蚀混凝土不同应变率作用下抗压性能试验. 沈阳大学学报(自然科学版). 2023(04): 348-355 .  百度学术
百度学术其他类型引用(5)
-












 下载:
下载:

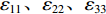






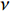

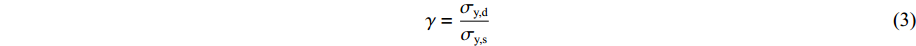





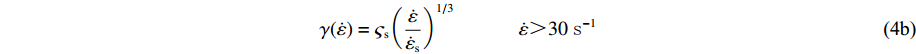






















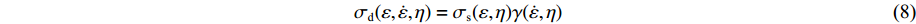








































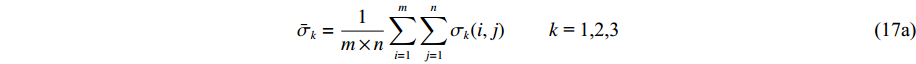




























 下载:
下载:









 百度学术
百度学术