Energy absorption of new thin-walled, multi-cellular, tubular structures with Sierpinski hierarchical characteristics under axial impact
-
摘要:
为提高薄壁结构的吸能能力,基于Sierpinski分形结构提出了一种具有层级特性的新型薄壁管,即Sierpinski层级管(Sierpinski hierarchical tube, SHT)。采用非线性有限元法对SHTs在轴向冲击载荷作用下的变形模式和能量吸收特性进行了数值分析,并与普通三角形薄壁管在轴向冲击载荷作用下的变形模式和能量吸收特性进行了对比。结果表明:SHTs的变形模式为轴对称渐进屈曲模式,在薄壁管中引入Sierpinski层级特性后,胞壁弯曲过程的半折叠波长减小,促使压缩过程中形成更多的塑性折叠单元,有利于提高薄壁结构能量吸收能力。进一步基于能量守恒理论和塑性铰理论对SHTs的轴向压缩应力进行理论求解,并通过有限元数值模拟验证其准确性。在相同的相对密度下,一阶、二阶及三阶SHTs的动态压缩应力较普通三角形薄壁管的动态压缩应力提高了85.8%、138.2%和183.8%。将Sierpinski层级特性引入薄壁管的设计中,能够有效提高薄壁管的耐撞性能。
-
关键词:
- 薄壁结构 /
- Sierpinski分形 /
- 轴向压缩 /
- 变形模式 /
- 耐撞性
Abstract:In order to improve the energy absorption capacity of thin-walled structures, a new type of thin-walled tube (SHT) with hierarchical characteristic was proposed based on the Sierpinski fractal structure. The deformation mode and energy absorption characteristics of SHTs under axial impact load were simulated using the nonlinear finite element method, and compared with those of ordinary triangular thin-walled tubes. The results show that the deformation mode of the new SHT is an axisymmetric progressive buckling mode. With the introduction of the Sierpinski hierarchical characteristics, the half-folded wavelength of the cell wall bending process is reduced, hence more plastic folding elements are formed and more energy is absorbed. Furthermore, theoretical expressions of the axial compression stress were obtained based on the energy conservation theory and plastic hinge theory. The correctness of the theoretical formula was verified by comparing with the finite element simulation. The results display that under the same relative density, the dynamic compressive stresses of the first-, second- and third-order SHTs are 85.8%, 138.2% and 183.8%, respectively, higher than that of the ordinary triangular thin-walled tubes. The introduction of the Sierpinski hierarchical characteristics into the design of the thin-walled tubes can effectively improve the crashworthiness of the thin-walled tubes, and it can provide a reference for the research and design of new energy absorbers.
-
Key words:
- thin-walled structure /
- Sierpinski fractal /
- axial impact /
- deformation mode /
- crashworthiness
-
薄壁结构质量轻并且具有较好的能量吸收特性,常被用来生产各种能量吸收或缓冲装置,已被广泛应用于航天航空、车辆工程及国防装备等领域。薄壁结构在轴向冲击载荷作用下通过胞壁的塑性变形吸收大量的冲击能量,所以研究薄壁结构的轴向压缩特性具有重要的工程意义。
McFarland[1]最先提出正六边形多胞结构轴向准静态平均压缩应力的计算方法,Wierzbicki[2]修正了McFarland提出的基本折叠模式,并根据超折叠单元理论[3]对正六边形多胞结构的轴向平均压缩应力和折叠波长进行了理论求解;Tran等[4]通过理论和数值手段获得了多种薄壁管在冲击载荷作用下的轴向压缩应力理论模型;尹汉锋等[5]基于超折叠单元理论对几种胞元构型蜂窝的平均压缩应力进行求解,并开展耐撞性优化设计。
薄壁结构的几何构型对其轴向冲击载荷作用下的吸能特性有着较大的影响。为提高普通构型(三角形、正六边形、圆形、正方形等)薄壁管的吸能能力,许多学者对一些新型层级薄壁结构产生了兴趣。Sun等[6]将规则六边形多胞结构的每个顶点替换为一个较小的六边形结构,并分析了其轴向冲击载荷作用下的耐撞性能。Mousanezhad等[7]提出了具有高刚度和高韧性的蜘蛛网层级多胞结构,并研究了结构参数对其面内及面外力学性能的影响。Sun等[8]用同性子结构替换了规则六边形多胞结构的实心胞壁,研究发现该层级特性的引入能大幅度提高其面内刚度。张越等[9]运用数值模拟方法研究了二阶自相似四边形蜂窝结构参数对其面外动态压缩性能的影响。于国际等[10]研究了二阶层级六边形蜂窝的面内动态压缩性能。赖燕辉等[11]基于多级蜂窝构型法分析了层级蜂窝的弹性模量等力学参数。
受Sierpinski三角形的启发,本文中将Sierpinski分形特性引入薄壁吸能管的层级设计,从而提出一种具有Sierpinski层级特性的新型薄壁多胞管(Sierpinski hierarchical tube, SHT),并对SHTs在轴向冲击作用下的变形模式和吸能特性进行数值模拟分析。进一步基于能量守恒原理对SHTs在轴向压缩下的平均压缩应力理论模型进行求解,以期为新型薄壁构型轴向缓冲吸能装置的设计提供指导。
1. SHTs的层级结构设计
分形结构可以通过多种方法产生,Sierpinski[12]于1916年提出了一个典型的自相似集,并命名为Sierpinski三角形。如图1所示,Sierpinski垫是一个由若干个三角形构成的自相似几何结构。
基于Sierpinski[12]的研究工作,本文研究的具有Sierpinski层级特性的多胞管是通过胞壁连接规则三角形薄壁管胞壁中点形成的。在每个SHT中有3i个边长为
li 的单位三角形,通过重复这一迭代过程能够得到更高阶的SHTs,i表示层级数,同时普通三角形薄壁管可以定义为零阶SHTs。第零~三阶SHTs的结构示意图如图1所示。由图1中(i−1)阶SHTs发展为i阶SHTs的典型过程可知,层次结构参数可以定义为:
γ=lili−1 (1) 式中:
li 和li−1 表示每个单位三角形的胞元壁长。值得注意的是,SHTs的结构参数γ将保持在0.5不变。l0 为普通三角形薄壁管的胞壁长。因此,i阶SHTs的所有胞壁长度之和为:Li=3i+12il0 (2) 本文中假设这些结构的胞壁厚度是均匀的。作为薄壁结构的重要结构参数,根据面积比理论,i阶SHTs的表观密度可以表示为:
ρi=4√3⋅(32)itil0ρs (3) 式中:
ti 为i阶SHTs中单位三角形的胞壁厚度,ρs 为基体材料密度。因此,SHTs的相对密度由下式给出:
¯ρi=ρiρs=4√3⋅(32)itil0 (4) 构成SHTs的单位三角形薄壁长度随着层级参数i的增大而变短,这就会给数值模拟带来很大的麻烦,因此本文中只考虑到三阶SHTs。本文中
l0 为90 mm,多胞管轴向长度h为250 mm。考虑3组壁厚,即t0 =1.0,1.3,1.6 mm,薄壁管的相对密度分别为0.077、0.100和0.123。由于Sierpinski层级结构特征的引入,本文中所研究的SHTs结构变得更复杂。然而,SHT作为一种典型的薄壁结构,其仍可以被划分为若干个典型单元。如图2所示,所有的SHTs均可看作由2种基本单元组成,即V形角单元和K形角单元。
2. SHTs的轴向压缩模拟分析
2.1 SHTs轴向压缩有限元模型
利用通用显式非线性有限元分析软件LS-DYNA模拟轴向冲击载荷作用下SHTs的动态压缩特性,计算模型如图3所示。构建上下端面刚性板,当顶端刚性板沿轴向以10 m/s的恒定速度冲击薄壁试件时,底端刚性板固定。为了准确地模拟薄壁管的大变形,胞壁采用Beltschko-Tsay四边形壳单元,单元厚度方向采用五点积分,面内采用单点积分。基体材料为铝合金AA6060T4,材料的杨氏模量
EY=68.2GPa ,屈服应力σy=80MPa ,极限应力σu=173MPa ,密度ρs=2700kg/m3 ,泊松比μ=0.3 ,幂指强化因数n=0.23 [13]。由于铝合金对应变率不敏感,本文中未考虑应变率的影响。薄壁管胞壁采用自动单面接触算法来考虑自身变形产生的接触;薄壁管与刚性板之间采用自动点-面接触算法。数值模型中的静摩擦因数及动摩擦因数均取0.2。2.2 有限元模型验证
为验证该模型的可靠性,首先对薄壁方形管[14]的压缩行为进行模拟。通过有限元计算后处理可以提取冲击端应力应变曲线,名义应力
σ 可表示为:σ=F(d)/S (5) 式中:
F(d) 为冲击端压力,d为冲击端压缩距离,S为薄壁结构所占面积。应变
ε 可表示为:ε=d/h (6) 动态平均压缩应力
σdm 则表示为:σdm=1εd∫εd0σdε (7) 式中:
εd 为密实化应变。计算出应力σdm 后,动态平均压缩力则可表示为:Pdm=σdm⋅S (8) 模拟结果与实验数据[14]的比较见表1,误差均小于10%,表明模拟结果与实验结果吻合较好。
2.3 变形模式和塑性机理
图4给出了
ˉρ=0.077 的不同SHTs结构在轴向冲击载荷作用下不同时刻的典型变形图。由于多胞管的底部被固定,胞壁均从冲击端开始发生塑性变形,随着冲击端的不断下压,胞元逐层发生折叠变形。研究结果还表明,具有Sierpinski层级特性的多胞管在轴向压缩过程中均呈现出轴对称渐进屈曲模式。轴对称渐进屈曲是理想的屈曲模式,已有研究表明薄壁结构在受到轴向冲击载荷作用时也多发生轴对称的向外屈曲变形[2-5],并且塑性变形主要集中在管壁的连接处。与此同时,Sierpinski层级特征的引入导致胞元节点段数的增加。为保持结构的相对密度不变,胞壁厚度会变小,从而在某种程度上削弱了薄壁结构的弯曲变形能。与规则三角形管相比,引入Sierpinski分级特性的多胞管变形吸能最显著的区别就是折叠单元的数量。SHTs完全折叠单元的数量会随着层级数的增加而增加。Sierpinski分级特性的引入大大缩短了胞壁变形的半折叠波长,这也就意味着在压缩过程中更多的塑性变形能被耗散,增强了薄壁结构的抗压缩能力。
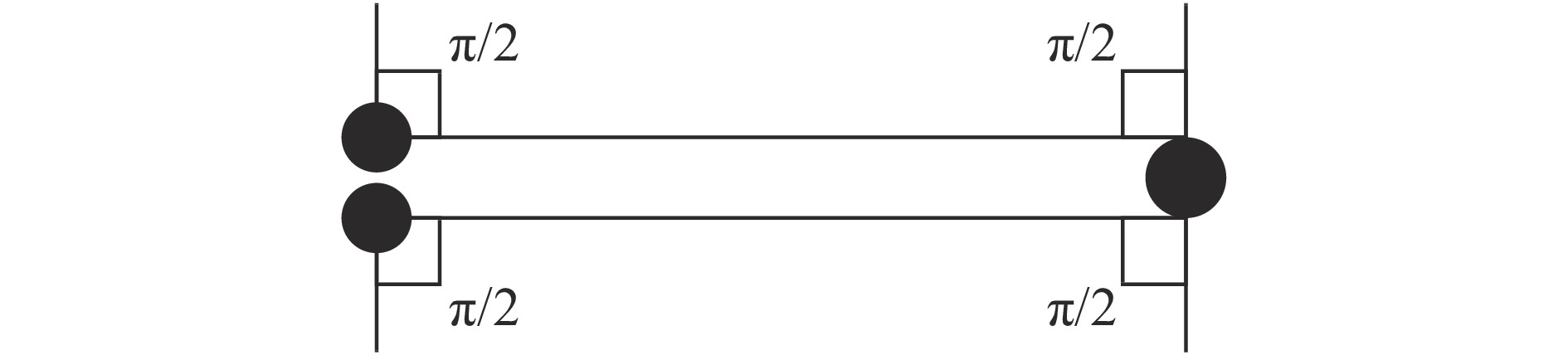
Abramowicz等[15]指出,薄壁管在塑性变形过程中有2类基本的变形单元,即非延展性基本单元和延展性基本单元,如图5所示。图5中2H为折叠单元高度,图5(a)中延展吸能区域阴影面积为S*,图5(b)中延展吸能区域阴影面积为S#。
前文介绍SHTs结构时提及到作为一种典型的薄壁结构,其仍可以被划分为若干个典型单元,即如图2所示,所有的SHTs均可看作由2种基本单元组成,即V形角单元和K形角单元。
图6给出了2种角单元的典型变形轮廓图,通过对变形模式的仔细观察能得到SHT结构在整个变形过程中的2种典型变形模式。这些变形模式主要取决于相邻胞壁的相对运动。对于V形角单元,在每一个折叠单元变形过程中,2个胞壁都向同一方向移动,其变形轮廓如图6(a)所示,这属于传统的非延展性变形模式(Ⅰ型)。
对于K形单元,4个胞壁在折叠变形过程中均向外移动,如图6(b)所示,此时相邻胞壁间的变形机理属于延展性变形模式(Ⅱ型)。
3. SHTs的轴向压缩应力计算
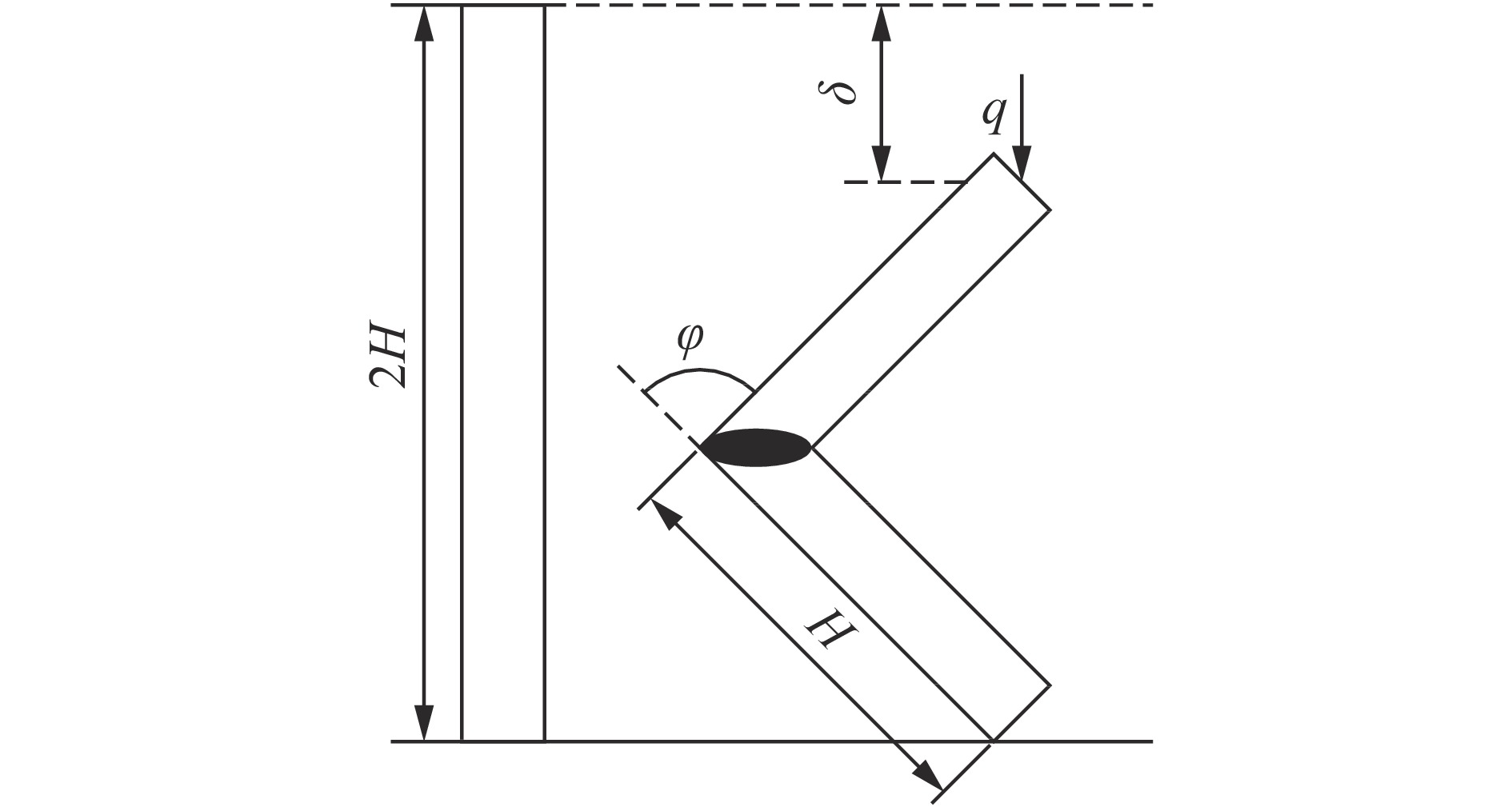
图7为薄壁结构的胞壁受轴向冲击载荷作用时的变形示意图,其中H被称为胞元折叠单元半波长塑性铰长度,
δ 为轴向压缩长度,q 为冲击载荷。轴对称渐进屈曲是理想的屈曲模式,已有研究[2-5]表明薄壁结构在受到轴向冲击载荷作用时也多发生轴对称渐进屈曲变形。学者们基于简化超折叠单元(simplified super folded element, SSFE)理论求解了大量该变形模式下薄壁管的塑性坍塌问题[3-5]。
SHTs是一种典型的薄壁结构,通过数值模拟和分析可知其在压缩载荷作用下发生轴向渐进屈曲模式变形,这就启发我们运用SSFE理论来推导SHTs的轴向压缩力。为确定SHTs的轴向压缩应力,还需作如下假设:
(1)薄壁管材料塑性好,可视为理想刚塑性材料;
(2)如图7所示,薄壁管在轴向压缩载荷作用下发生向外的轴对称渐进屈曲,变形过程中各个折叠的塑性铰长度相等,为2H;
(3)在变形过程中,同一胞元上下表面保持平行,即胞元的各边变形量相同;
(4)压缩过程中胞壁间粘接强度足够大,不发生破裂,可忽略粘接对薄壁结构力学性能的影响。
考虑到冲击能量主要由薄壁结构胞壁的塑性变形耗散,根据能量守恒原理,外力做功等于薄壁结构塑性变形所耗散的能量,即弯曲耗能Ebending和拉伸耗散能
Emembrane 。相应的能量平衡方程可表示为:Pmη(2H)=Ebending+Emembrane (9) 式中:
Pm 为准静态平均压缩力。事实上,折叠单元在塑性变形过程中无法完全被压实。因此,这里给出了考虑有效压缩的修正因数。η 为有效压缩距离δe 与半折叠波长H 的比值。Wierzbicki等[15]提出η 可能在0.70~0.75之间变化。为简单起见,本文中采用η=0.70 。3.1 SHTs的弯曲耗能计算
Chen等[16]指出通过将折叠单元的水平铰线的弯曲耗散能量相加,可以估算出压缩过程中的全部弯曲耗能,即:
Ebending=j∑i=1M0φiLi (10) 式中:
M0 为胞元单位长度的塑性极限弯矩,M0=σ0t2i/4 ;φi 表示第i 条铰线的旋转角度,j 表示铰线的数量;Li 是所有胞壁的总长度;σ0 为蜂窝基体材料的流动应力[13]。σ0 的表达式为:σ0=√σyσu/(1+n) (11) 式中:
σy 和σu 分别为SHTs基体材料的屈服应力和极限应力,n 为硬化指数。如图8所示,假设薄壁管胞壁被完全压实,水平铰线的弯曲角度即为
π/2 、π 和π/2 。因此,弯曲耗散能可表示为:Ebending=2πM0Li (12) 3.2 角单元的拉伸耗散能
3.2.1 V形角单元
图6给出了简化超折叠单元理论中的基本折叠单元延展吸能示意图,本文中考虑了SSFE理论中的2种拉伸变形模式。图5(a)所示为变形模式,延展吸能区由3个三角形阴影部分组成,通过对阴影面积积分可求得该变形模式下单个胞壁的拉伸耗散能:
Easymmembrane_f=∫S∗σ0tidS∗=12σ0tiH2=2M0H2ti (13) Chen等[16]指出2个互相连接的胞壁对角单元的拉伸形变能具有相似的贡献。因此,在非延展变形的情况下,直角单元的拉伸耗散吸能可表示为单个角折叠单元拉伸耗散吸能的2倍:
Easymmembrane_r=2Easymmembrane_f=4M0H2ti (14) Tran等[4]在角单元的拉伸耗散吸能方面做了大量的研究,指出与直角单元相比,V形角单元的拉伸耗散吸能较小。如图9所示,V形角单元在完全塑性坍塌过程中的拉伸耗散吸能可表示为:
EV-shapedmembrane=Easymmembrane_rcosα=4M0H2ticosα (15) 式中:
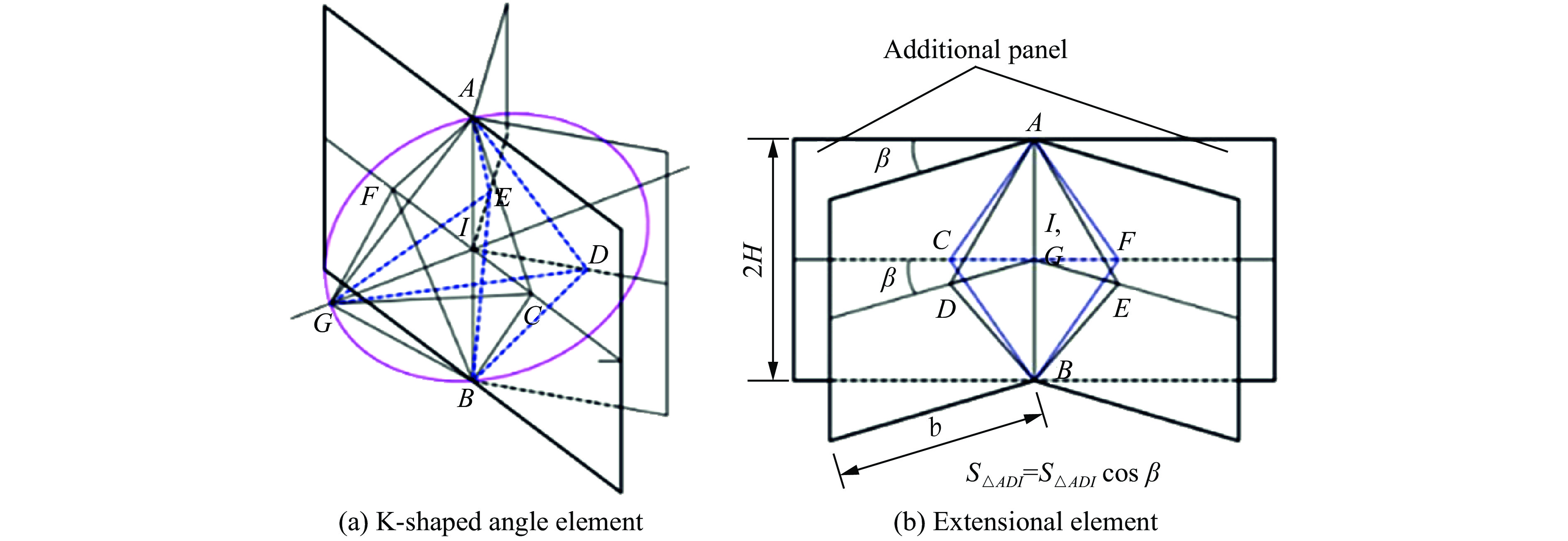
α 为V形角单元两胞壁的夹角。图9中β 为V形角单元胞壁与直角单元胞壁之间的夹角。3.2.2 K形角单元
通过对变形轮廓图的分析,不难发现K形角单元的变形机理比直角角单元的变形机理复杂。将K形角单元的薄膜耗散吸能简化为由一个V形角单元和两个附加平面的薄膜耗散吸能组成。K形角单元中的每个胞壁都具有相似的变形模式。K形角单元中包含的V形角单元与单个V形角单元具有相同的抗压强度,需注意的是根据仿真结果(图7)可知此时的V形角单元处于延展性变形模式,如图6(b)所示。阴影部分为延展吸能区,通过积分阴影区域来评估完全塌陷期间每个胞壁的薄膜耗散吸能:
Esymmembrane_f=∫S#σ0tidS#=σ0tiH2=4M0H2ti (16) 图10给出了在K形角单元的拐角处形成的延展吸能三角形单元。K形角单元中包含的V形角单元的薄膜耗散能可表示为:
EV-shaped′membrane=2Esymmembrane_fcosβ=8M0H2ticosβ (17) 另外两个附加胞壁的薄膜耗散吸能为:
E2-additionalmembrane=2Esymmembrane_f=8M0H2ti (18) 因此,在一个折叠波长压缩期间,K形角单元的薄膜耗散吸能由一个V形角单元和两个附加胞壁的薄膜耗散吸能组成:
EK-shapedmembrane=EV-shaped′membrane+E2-additionalmembrane=8M0H2ti(1+1cosβ) (19) 3.3 SHTs的动态平均压缩应力计算
普通三角形薄壁管,即零阶SHTs是由3个V形角单元组合而成。将式(12)和(15)代入式(9),平均压缩力
Pm,0th 的理论计算方程可写为:Pm,0th(2H)η=Ebending+3EV-shapedmembrane=2πM0L0+2M0H2t0(6cosα) (20) 对式(20)进行转换可得:
Pm,0thηM0=πL0H+Ht0(6cosα)=πL0H+Ht0F(α) (21) 根据准静态压缩过程中的能量最低原理可得
∂Pm,0th/∂H=0 ,对式(21)求导得:0=−πL0H2+F(α)t0⇒H=√πL0t0F(α)=√0.518πl0t0 (22) 将式(22)代入式(20),可求得普通三角形薄壁管的准静态平均压缩载荷为:
Pm,0th=πM0L0ηH+M0Hηt0F(α)=π0.5σ0t1.50L0.50√F(α)2η=√17.387π2ησ0t1.50l0.50 (23) 式中:
F(α)=6cosα 。而薄壁管所占面积为:
S=√3l20/4 (24) 所以,普通三角形薄壁管的轴向准静态平均压缩应力为:
σm,0th=Pm,0th/S=2√5.796πησ0(t0l0)3/2 (25) 同理,一阶SHTs可看作由3个V形角单元和3个K形角单元组合而成;二阶SHTs可看作由3个V形角单元和12个K形角单元组合而成;三阶SHTs可看作由3个V形角单元和39个K形角单元组合而成。对3种SHTs的轴向准静态平均压缩应力进行求解,可得轴向准静态平均压缩应力分别为:
σm,1st=2√62.693πησ0(t1l0)3/2 (26) σm,2nd=2√337.04πησ0(t2l0)3/2 (27) σm,3rd=2√1599.06πησ0(t3l0)3/2 (28) 研究表明动态压缩应力一般比相应的准静态压缩应力高[5],而上文的理论推导过程中忽略了动态压缩(包括惯性和应变率)的影响。考虑到铝的应变速率效应可以忽略不计,采用增强因子
λ 来考虑惯性效应。Hanssen等[17]指出λ可在1.3~1.6范围内变化。为简化计算,取10 m/s冲击速度下的增强因子为1.3。因此,可以得到零~三阶SHTs的动态压缩应力理论计算值分别为:σdm,0th=2λ√5.796πησ0(t0l0)3/2 (29) σdm,1st=2λ√62.693πησ0(t1l0)3/2 (30) σdm,2nd=2λ√337.04πησ0(t2l0)3/2 (31) σdm,3rd=2λ√1599.06πησ0(t3l0)3/2 (32) 3.4 理论模型模拟验证
本文中
t0 分别取1.0、1.3和1.6 mm,胞壁长l0 取90 mm,分别对这些新型SHT多胞结构进行轴向压缩的数值模拟。用式(29)~(32)计算出动态压缩应力的理论值,并将其与模拟结果进行比较,如表2所示,当
t0=1.0 mm(ˉρ=0.077 )时,一阶、二阶及三阶SHTs的动态压缩应力较普通三角形薄壁管分别升高了85.8%、138.2%和183.8%。这表明:将Sierpinski层级特性应用到薄壁管的设计中,可以有效提高薄壁管的吸能特性。4种薄壁管的动态压缩应力模拟值和理论值的差异分别为−2.64%~3.42%、−5.23%~1.54%、−2.77%~3.14%和−1.19%~3.67%,结果基本吻合,进一步验证了本文动态压缩应力理论推导方法的可行性,说明理论计算结果具有工程应用价值,可用于指导新型薄壁结构轴向缓冲吸能装置的设计。表 2 各采样点有限元结果与理论预测值对比Table 2. Comparison of finite element results and theoretical predictions for all the sampling points层级级数 ˉρ ti/mm li/mm σdm/MPa 相对误差/% 模拟 理论 0th 0.077 1.00 90 1.925 1.968 −2.18 0.100 1.30 2.840 2.917 −2.64 0.123 1.60 4.118 3.982 3.42 1st 0.077 0.67 45 3.577 3.523 1.54 0.100 0.87 4.948 5.221 −5.23 0.123 1.07 7.046 7.129 −1.17 2nd 0.077 0.44 22.5 4.586 4.446 3.14 0.100 0.58 6.407 6.590 −2.77 0.123 0.71 9.186 8.998 2.09 3rd 0.077 0.30 11.25 5.464 5.271 3.67 0.100 0.39 7.720 7.813 −1.19 0.123 0.47 10.972 10.669 2.84 4. 结 论
将Sierpinski分形结构引入到薄壁吸能管的层级设计中,提出了一种具有Sierpinski层级特性的新型薄壁多胞管(SHT),并通过数值模拟和理论方法研究其受轴向冲击载荷作用下的变形模式和能量吸收特性,研究结果表明:
(1)具有Sierpinski层级特性的SHTs在轴向压缩过程中均呈现出理想的轴对称渐进屈曲模式。SHTs完全折叠单元的数量会随着层级数的增加而增加。Sierpinski分级特性的引入大大缩短了胞壁变形的半折叠波长,压缩过程中更多的塑性变形能量被耗散,增强了薄壁结构的抗压缩能力。
(2)SHTs均可看作由两种基本单元组成,即V形角单元和K形角单元。V形角单元在每一个折叠单元变形过程中,两个胞壁都向同一方向移动,属于传统的非延展性变形模式(Ⅰ型)。K形角单元4个胞壁在折叠变形过程中均向外移动,相邻胞壁间的变形机理属于延展性变形模式(Ⅱ型)。
(3)基于能量守恒理论和塑性铰理论建立了SHTs的塑性屈曲理论模型,获得了压溃过程中的塑性弯曲耗散能、薄膜耗散能、轴向压缩应力的理论表达式,模拟结果与理论计算结果基本吻合,验证了本文中动态压缩应力理论推导方法是可行的,理论计算结果具有工程应用价值。
(4)在相同的相对密度下,一阶、二阶及三阶SHTs的动态压缩应力较普通三角形薄壁管分别增高了85.8%、138.2%和183.8%。将Sierpinski层级特性引入到薄壁管的设计中,能够有效提高薄壁管的耐撞性能,这可为新型吸能元件的研究和设计提供参考。
-
表 1 薄壁方形管动态平均压缩力模拟结果与实验数据[14]的比较
Table 1. Comparison between simulated and experimental mean dynamic compressive forces[14] for thin-walled square tubes
编号 Pdm/kN 误差/% 实验[14] 模拟 S2 10.26 10.86 5.8 S3 15.71 16.70 6.3 S4 18.83 19.47 3.4 S5 29.83 31.92 7.0 表 2 各采样点有限元结果与理论预测值对比
Table 2. Comparison of finite element results and theoretical predictions for all the sampling points
层级级数 ˉρ ti/mm li/mm σdm/MPa 相对误差/% 模拟 理论 0th 0.077 1.00 90 1.925 1.968 −2.18 0.100 1.30 2.840 2.917 −2.64 0.123 1.60 4.118 3.982 3.42 1st 0.077 0.67 45 3.577 3.523 1.54 0.100 0.87 4.948 5.221 −5.23 0.123 1.07 7.046 7.129 −1.17 2nd 0.077 0.44 22.5 4.586 4.446 3.14 0.100 0.58 6.407 6.590 −2.77 0.123 0.71 9.186 8.998 2.09 3rd 0.077 0.30 11.25 5.464 5.271 3.67 0.100 0.39 7.720 7.813 −1.19 0.123 0.47 10.972 10.669 2.84 -
[1] MCFARLAND R K. Hexagonal cell structures under post-buckling axial load [J]. AIAA Journal, 1963, 1(6): 1380–1385. DOI: 10.2514/3.1798. [2] WIERZBICKI T. Crushing analysis of metal honeycombs [J]. International Journal of Impact Engineering, 1983, 1(2): 157–174. DOI: 10.1016/0734-743X(83)90004-0. [3] WIERZBICKI T, ABRAMOWICZ W. On the crushing mechanics of thin-walled structures [J]. Journal of Applied Mechanics, 1983, 50(4a): 727–734. DOI: 10.1115/1.3167137. [4] TRAN T N, HOU S J, HAN X, et al. Theoretical prediction and crashworthiness optimization of multi-cell triangular tubes [J]. Thin-Walled Structures, 2014, 82: 183–195. DOI: 10.1016/j.tws.2014.03.019. [5] 尹汉锋, 文桂林. 基于简化基本折叠单元法的蜂窝耐撞性优化设计 [J]. 机械工程学报, 2011, 47(16): 93–100. DOI: 10.3901/JME.2011.16.093.YIN H F, WEN G L. Crashworthiness optimization design of honeycombs based on the simplified basic folding element method [J]. Journal of Mechanical Engineering, 2011, 47(16): 93–100. DOI: 10.3901/JME.2011.16.093. [6] SUN G Y, JIANG H, FANG J G, et al. Crashworthiness of vertex based hierarchical honeycombs in out-of-plane impact [J]. Materials and Design, 2016, 110: 705–719. DOI: 10.1016/j.matdes.2016.08.032. [7] MOUSANEZHAD D, EBRAHIMI H, HAGHPANAH B, et al. Spiderweb honeycombs [J]. International Journal of Solids and Structures, 2015, 66: 218–227. DOI: 10.1016/j.ijsolstr.2015.03.036. [8] SUN Y T, WANG B, PUGNO N, et al. In-plane stiffness of the anisotropic multifunctional hierarchical honeycombs [J]. Composite Structures, 2015, 131: 616–624. DOI: 10.1016/j.compstruct.2015.06.020. [9] 张越, 李世强, 王志华. 二阶层级自相似四边形蜂窝动力压缩行为数值模拟 [J]. 高压物理学报, 2017, 31(4): 358–363. DOI: 10.11858/gywlxb.2017.04.002.ZHNAG Y, LI S Q, WANG Z H. Dynamic crushing response of self-similar second order hierarchical square honeycombs [J]. Chinese Journal of High Pressure Physics, 2017, 31(4): 358–363. DOI: 10.11858/gywlxb.2017.04.002. [10] 于国际, 李飞鹏, 李世强, 等. 二阶层级自相似六边形蜂窝的面内压缩行为 [J]. 太原理工大学学报, 2018, 49(3): 494–500. DOI: 10.16355/j.cnki.issn1007-9432tyut.2018.03.021.YU G J, LI F P, LI S Q, et al. In-plane compressive behavior of a self-similar second order hierarchical hexagonal honeycomb [J]. Journal of Taiyuan University of Technology, 2018, 49(3): 494–500. DOI: 10.16355/j.cnki.issn1007-9432tyut.2018.03.021. [11] 赖燕辉, 江五贵, 吴瑶. 自相似多级纳米蜂窝铝结构力学性能的分子动力学模拟 [J]. 复合材料学报, 2019, 36(4): 946–953. DOI: 10.13801/j.cnki.fhclxb.20180517.001.LAI Y H, JIANG W G, WU Y. Molecular dynamics simulation on mechanical properties of nano self-similar hierarchical honeycomb Al [J]. Acta Materiae Compositae Sinica, 2019, 36(4): 946–953. DOI: 10.13801/j.cnki.fhclxb.20180517.001. [12] SIERPINSKI W. Sur une série potentielle qui, étant convergente en tout point de son cercle de convergence, représente sur ce cercle une fonction discontinue [J]. Rendiconti del Circolo Matematico di Palermo, 1916, 41(1): 187–190. DOI: 10.1007/BF03018294. [13] SANTOSA S P, WIERZBICKI T, HANSSEN A G, et al. Experimental and numerical studies of foam-filled sections [J]. International Journal of Impact Engineering, 2000, 24(5): 509–534. DOI: 10.1016/S0734-743X(99)00036-6. [14] ZHANG X, ZHANG H. Energy absorption of multi-cell stub columns under axial compression [J]. Thin-Walled Structures, 2013, 68: 156–163. DOI: 10.1016/j.tws.2013.03.014. [15] ABRAMOWICZ W, JONES N. Dynamic axial crushing of square tubes [J]. International Journal of Impact Engineering, 1984, 2(2): 179–208. DOI: 10.1016/0734-743X(84)90005-8. [16] CHEN W G, WIERZBICKI T. Relative merits of single-cell, multi-cell and foam-filled thin-walled structures in energy absorption [J]. Thin-Walled Structures, 2001, 39(4): 287–306. DOI: 10.1016/S0263-8231(01)00006-4. [17] HANSSEN A G, LANGSETH M, HOPPERSTAD O S. Static and dynamic crushing of circular aluminum extrusions with aluminum foam filler [J]. International Journal of Impact Engineering, 2000, 24(5): 475-507. DOI: 10.1016/S0734-743X(99)00170-0. 期刊类型引用(7)
1. 黄翠萍,邓小林,蔡珍珍. 自相似嵌套金属多胞管耐撞性研究. 机械强度. 2024(03): 568-578 .  百度学术
百度学术2. 杨永宝,奚杰,李志超. 阶梯多胞方管横向弯曲响应特性研究. 农业装备与车辆工程. 2024(12): 98-105 .  百度学术
百度学术3. 蔡玮雯,马其华,甘学辉. 低速冲击载荷下CFRP-Al多胞薄壁管的耐撞性. 塑性工程学报. 2023(04): 187-196 .  百度学术
百度学术4. 覃善甘,邓小林,刘鑫炎,刘夫云. 新型层级梯度六边形管的耐撞性多目标优化. 塑性工程学报. 2023(07): 194-204 .  百度学术
百度学术5. 孔志成,胡俊,郭智平. 星形混合多胞管的耐撞性数值与理论研究. 爆炸与冲击. 2023(08): 43-55 .  本站查看
本站查看6. 邓敏杰,刘志芳. 仿马尾草薄壁结构的设计与耐撞性研究. 高压物理学报. 2022(03): 111-120 .  百度学术
百度学术7. 贺璞,邓庆田,李新波. 层合多孔圆柱壳的轴向冲击吸能特性. 高压物理学报. 2022(04): 145-154 .  百度学术
百度学术其他类型引用(12)
-








 下载:
下载:


























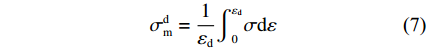





























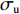




































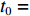


 下载:
下载:









 百度学术
百度学术