Numerical simulation of pre-shock desensitization in TATB-based heterogeneous explosive
-
摘要: 为了对一种TATB基非均质炸药的预冲击起爆现象展开数值模拟研究,将基于冲击温度及压力的AWSD反应率模型耦合进二维结构网格拉氏弹塑性流体力学程序。利用炸药及其产物的冲击雨贡纽实验数据校验了未反应炸药及产物的状态方程参数,通过一维冲击起爆的模拟,标定了反应速率模型参数。模拟了炸药在弱冲击0.45 μs后跟随的强冲击波的二次冲击实验,结果表明,受预压缩区域的炸药反应变慢,到爆轰距离增长了约1 mm,与该炸药二次冲击实验减敏现象相符。模拟拐角效应时,爆轰波经过拐角后,在拐角附近形成稳定的不起爆区域,与主要成分相同的LX-17炸药的拐角效应实验的死区特征相符。数值模拟结果表明,基于冲击温度及压力的AWSD反应率模型可以较好地模拟非均质炸药预冲击减敏问题。Abstract: To study and simulate the pre-shock desensitization in the TATB-based heterogeneous explosive, the impact temperature and pressure based AWSD reaction flow model was implemented in a 2D structured mesh Lagrangian elastoplastic hydrodynamics program. The reactant and product EOS parameters were calibrated against the Hugoniot experimental data. To calibrate the parameters of the reaction flow model, one-dimensional numerical simulations of the shock initiation experiments were carried out. We simulated the double-shock experiments in which a first weak shock was followed by a second strong shock with a time interval of 0.45 μs. The results indicate the reaction becomes slower in the precompressed region and the run-to-detonation distance is about 1 mm longer than that in the uncompressed region, which is consistent with the desensitization in double-shock experiments. When simulating the corner-turning, the detonation wave passes through the corner and forms a stable non-initiation region near the corner, which is consistent with the dead zone characteristics of the corner-turning experiment of the LX-17 explosive with the same main composition. The numerical simulation results show that the AWSD reaction rate model based on the impact temperature and pressure can well simulate the pre-shock desensitization of heterogeneous explosives.
-
Key words:
- TATB-based /
- heterogeneous explosive /
- pre-shock /
- desensitization /
- AWSD model
-
高能炸药安全性在民用和军事领域受到广泛关注,其中多次冲击转爆轰行为是炸药安全性研究的重要方向之一。在一些非理想起爆条件下,炸药可能会发生预冲击压缩行为,如隔爆、绕爆或炸药外部包裹高声速材料的复杂装置等。在弱冲击的预压缩条件下,炸药感度会下降,后续的强冲击压缩时,炸药的爆轰距离变长或无法起爆形成爆轰。研究预冲击减敏问题,对掌握多次冲击条件下装置起爆、爆轰过程或爆轰驱动的可靠性,具有重要应用价值和意义。Campbell等[1]通过聚苯乙烯和铜组合层反射波产生二次冲击的楔形实验,研究了HMX基黏塑性炸药在3.9 GPa预冲击下后续10 GPa的二次冲击无法实现炸药点火的现象,Campbell等[2]还研究了PBX9404炸药预冲击压力与需要的减敏时间的关系为p2.2τ=1 150(p的单位为100 MPa,τ的单位为μs)。对HMX基、RDX基、TNT基和TATB基等PBX炸药的减敏现象,已开展了许多实验研究[3-6]。通过实验及理论分析[7-9],认为弱冲击压缩清除了潜在热点是非均质炸药预冲击减敏的主要物理机制。因此,非均质炸药的预冲击压缩减敏过程的合理描述,可能需要考虑孔隙率、空穴塌陷、热点等物理机制的细观反应速率模型。但细观反应速率模型计算量大、模型中的物理参数较难确定,难推广到工程的实际应用。在唯象反应速率模型上,开展非均质炸药的预冲击压缩减敏研究,仍是主要手段之一。
需要指出,目前被广泛使用的大部分唯象反应速率模型(如Lee-Tarver点火增长模型[10]、JTF模型[11]和WSD模型[12]等),尚不足描述非均质炸药一次冲击与多次冲击(到相同压力)时炸药状态的差异,无法应用于减敏模拟。因此,DeOliveira等[13]在Lee-Tarver点火增长模型中引入减敏模块,减敏进程作用于反应率模型中的点火项和增长项,较好地模拟了LX-17炸药的拐角效应中的死区现象。Hussain等[14]、郝鹏程等[15]也做过类似的研究,在减敏模块方法中加入了一些经验参数来模拟炸药的减敏现象,不改变点火增长反应率形式及参数。但是,对于较弱冲击条件下,点火项和增长项都被抑制,爆轰不能正常进行。Starkenberg[16]则在HVRB模型[17]中扩展了依赖冲击压力的热点密度项和依赖压力的衰退率项,并引入表征冲击波产生的伪熵,进一步将热点密度项修改为伪熵函数即XHVRB模型,伪熵函数的引入使该模型具备模拟炸药多次冲击起爆的能力。近来逐渐发展了依赖熵或温度的唯象反应速率模型,如CREST模型[18]、WSD(T)模型[19]及AWSD模型[20]等。这类模型无需增加减敏模块,用熵或温度表征一次冲击与多次冲击时炸药状态的差异,从而实现减敏的预测。
本文中,将AWSD模型耦合进二维结构网格拉氏弹塑性流体力学程序,对主要成分为TATB的一种TATB基非均质炸药的预冲击起爆现象开展数值模拟研究。利用炸药及其产物的冲击雨贡纽实验数据,校验未反应炸药及产物的状态方程参数,通过一维冲击起爆的模拟,标定反应速率模型参数。然后,模拟该炸药的二次冲击减敏问题及其他一些预冲击减敏问题,以期为非均质炸药预冲击减敏现象的数值模拟提供参考。
1. 计算方法
采用二维结构网格拉氏弹塑性流体力学程序,它具有空间一阶离散精度和时间二阶离散精度,动量方程和大多数状态方程与能量方程的联立求解采用显示格式,具有较高的计算效率。以下重点介绍耦合的反应速率模型方程、未反应炸药和产物状态方程及混合法则。
1.1 反应速率模型方程
在WSD模型基础上,AWSD模型中引入冲击波阵面后未反应炸药的温度
TSH ,使模型同时基于冲击压力和温度,适合模拟不同初始温度条件下的炸药冲击到爆轰及直径效应实验[20]。总反应速率方程为:dλdt=Fp(F1+F2)Fλ (1) 式中:Fp为压力p的函数,表征当地压力对反应速率的影响;F1和F2为冲击温度TSH的函数,分别控制冲击起爆过程反应率以及爆轰阶段反应率;Fλ为控制反应快结束时的慢反应项。分别为:
Fp={exp[−(psp)np]p>pζ0p≤pζ (2) F1=k1exp(−T1TSH)(λ+a1Fp)(1−λ)b1 (3) F2=k2exp(−T2TSH)(1−λ)b2 (4) Fλ=fs+0.5(1−fs)[1−tanh(λ−λcδλ)] (5) 式中:np、ps、k1、T1、a1、b1、k2、T2、fs、λc和
δλ 为反应率参数。1.2 状态方程及参数
1.2.1 未反应物状态方程及参数
炸药未反应物采用Mie-Grüneisen形式的Davis状态方程[20],以等熵线为参考线,未反应炸药状态方程为:
ps=pi(vs)+Γs(vs)vs(Es−Ei(vs)) (6) 式中: ps、vs和Es为未反应炸药的压力、比体积和比内能,pi和Ei为等熵线上的压力和比内能,Γs为Grüneisen系数(当vs≤v0时,Γs=Γ0s+Zy,当vs>v0时,Γs=Γ0s)。Z为用于描述Grüneisen系数随密度变化的经验系数,Γ0s为初始Grüneisen系数,Γ0s=β
c20 /cps(β为膨胀系数,c0为体积声速,cps为炸药常压比热),y=1−vs/v0,v0为初始比体积。依据冲击绝热线与其任意点开始的等熵膨胀线近似的镜像对称,可得到[12]:pi(vs)={A24v0B[exp(4By)−1]vs>v0A24v0B[3∑j=1(4By)jj!+C(4By)44!+y2(1−y)4]vs≤v0 (7) Ei(vs)=v0∫y0pi(ˉy)dˉy+E0 (8) 式中:E0为初始比内能,A、B、C为根据未反应炸药冲击Hugoniot数据标定的常数。
未反应炸药的温度为:
Ts(vs,Es)=Ti(vs){1+αstcVsTi(vs)[Es−Ei(vs)]+1}1/(1+αst) (9) 式中:
cVs 为定容比热,cVs=cps(1+βΓ0sT)−1,Ti(vs)为沿等熵线上的温度。有:Ti(vs)=T0sexp(−Zy)(vsv0)−(Γ0s+Z) (10) 本文的炸药冲击雨贡纽实验数据来自文献[21-23],实验样品中炸药初始密度范围为1.883~1.898 g/cm3,炸药的D-u关系为D=2.417 km/s+2.141 u。由文献[24],按实验样品炸药初始密度中间值ρ0=1.890 g/cm3标定参数,标定的未反应炸药状态方程参数见表1。
表 1 未反应TATB基炸药的Davis状态方程参数Table 1. Davis EOS parameters of unreacted TATB-based explosiveA/(mm·μs−1) B C Z Γ0s E0/(kJ·g−1) αst cVs/(kJ·g−1·K−1) 1.93 4.26 0.30 0 0.56 3.80 0.757 0 0.000 967 1.2.2 爆轰产物状态方程及参数
爆轰产物也采用Mie-Grüneisen形式状态方程[20],以等熵线为参考线,爆轰产物状态方程为:
pg=pi(vg)+Γg(vg)vg[Eg−Ei(vg)] (11) 式中:pg、vg和Eg分别为产物的压力、比体积和比内能,pi和Ei为等熵线上的压力和比内能,Grüneisen系数Γg(vg)=k−1+(1−b)F(vg)(F(vg)=2
av2nc /(v2ng +v2nc ),a、k、vc、n和b为待定参数)。考虑低压下和高压下绝热指数的变化,由多方气体状态方程得到过CJ点的等熵线为:
pi=pc[0.5(vg/vc)n+0.5(vg/vc)−n]a/n(vg/vc)k+ak−1+F(vg)k−1+a (12) Ei=Ec[0.5(vg/vc)n+0.5(vg/vc)−n]a/n(vg/vc)k−1+a (13) 式中:Ec=pcvc/(k−1+a)。产物温度为:
Tg(vg,Eg)=Ti(vg)+Eg−Ei(vg)cVg (14) 等熵线上的温度Ti(vg)为:
Ti(vg)=2−ab/nk−1+apcvccVg[0.5(vg/vc)−n+0.5(vg/vc)n](a/n)(1−b)(vg/vc)k−1+a(1−b) (15) 参数a、k、vc、n和b通过拟合爆轰产物冲击雨贡纽实验数据来标定。标定的原则为:(1)等熵线必须过CJ点;(2)等熵线在CJ点处必须与Rayleigh线相切;(3)截断体积需简单地用Gurney 能量矫正;(4)气体产物完全膨胀时对外做的功等于化学能;(5)在vg较大时,等效绝热指数等于理想气体的;(6)遵循前5个原则确定了a、k、vc、pc和n后,通过调整参数b使超压爆轰条件下产物冲击雨贡纽曲线位于炸药冲击雨贡纽曲线上方。参考实验数据[25],标定得到炸药产物状态方程参数见表2。标定的未反应炸药及产物状态方程p-v雨贡纽曲线与实验结果的比较如图1所示,在高压段,未反应炸药p-v曲线低于爆轰产物p-v曲线。
表 2 TATB基炸药产物的Davis状态方程参数Table 2. Davis EOS parameters of reaction products of TATB-based explosiveA k vc/(cm3·g−1) pc/GPa n b cVg/(kJ·g−1·K−1) 0.835 603 1.30 0.926 85 1.485 1 4.242 66 0.85 0.001 072 1.2.3 混合法则
采用温度压力平衡的混合法则,假设固体未反应炸药与气体产物之间压力平衡且温度平衡。λ为反应度,有:
p=ps=pg,T=Ts=Tg,v=(1−λ)vs+λvg,E=(1−λ)Es+λEg,vs=Φv/[λ+(1−λ)Φ],vg=v/[λ+(1−λΦ)],Φ=vs/vg (16) 2. 一次冲击起爆数值模拟及模型参数标定
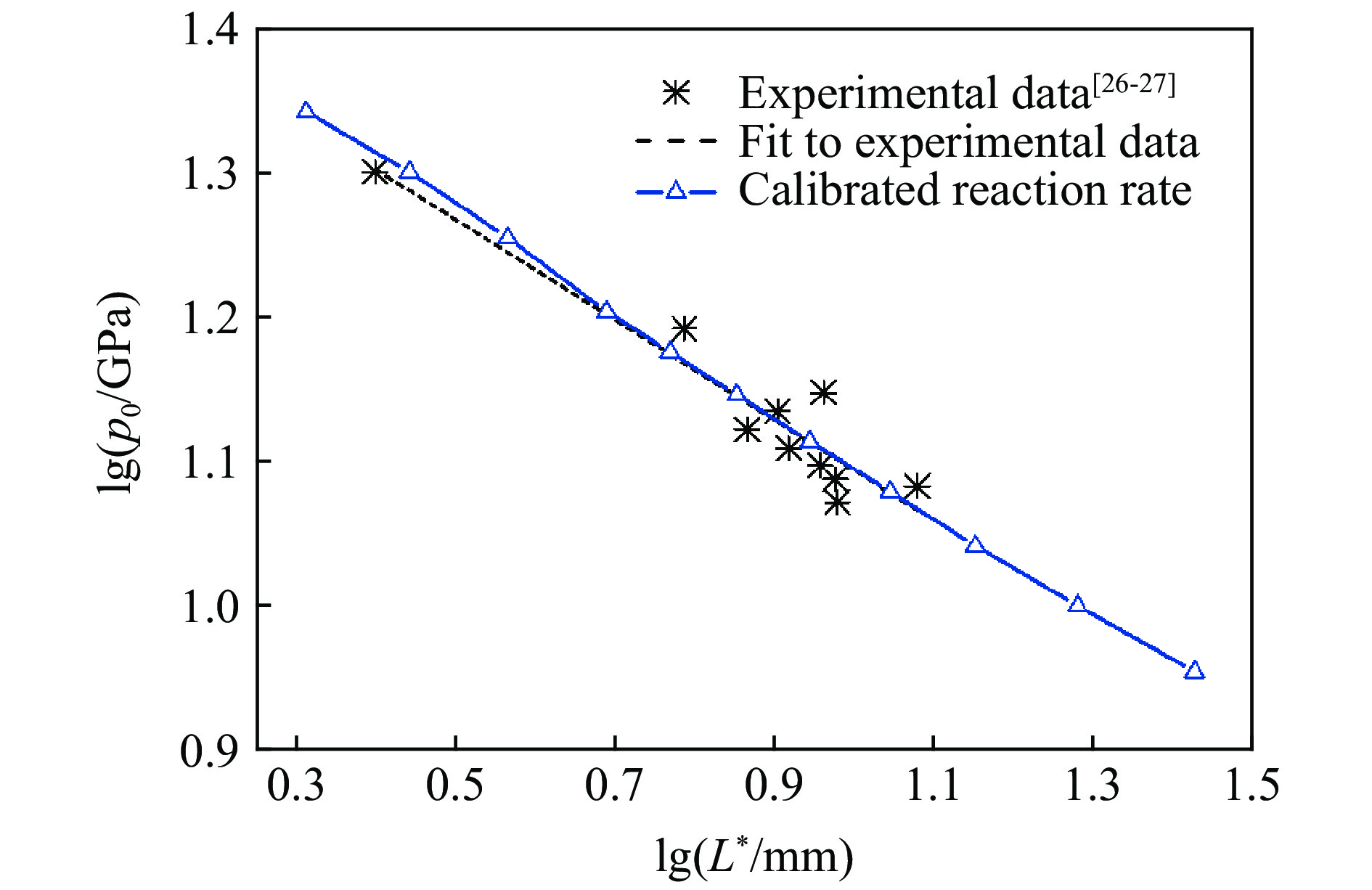
参考AWSD模型模拟PBX9502炸药的模型参数[10],根据本文的TATB基炸药冲击起爆实验数据的POP图[28-29],对个别参数进行微调。模型参数见表3,计算模型为长2 cm铝飞片以不同初始速度撞击长4 cm炸药,炸药初始密度为1.890 g/cm,一维冲击起爆实验及模拟的POP图见图2。拟合得到实验的冲击压力与到爆轰距离的关系为lg(p0/GPa)=(1.44±0.04)−(0.34±0.04)lg(L*/mm),数值模拟的冲击压力与到爆轰距离的关系为lg(p0/GPa)=(1.45±0.004)−(0.35±0.004)lg(L*/mm),图3为入射压力p0=12.514 GPa条件下,不同位置炸药粒子速度历程曲线的模拟结果与实验结果的对比。数值模拟的爆轰增长行为与实验结果符合较好,但是波速比实验结果偏高,分析认为与未反应炸药冲击雨贡纽关系有关。张琪敏等[28]统计了锰铜法、反向撞击法、电磁法及PDV方法测D-u关系数据,认为不同方法测量的实验结果存在一定差异,采用不同类型实验数据来标定未反应炸药状态方程对模拟冲击波在炸药中的传播波速会存在偏差。
表 3 TATB基炸药AWSD模型参数Table 3. Parameters of the AWSD model for the TATB-based explosivenp ps/GPa k1/s−1 T1/K a1 b1 b2 k2/s−1 T2/K fs λc δλ 0.651 5 27.60 336 1 724 0.060 81 2.122 0.9 10 200 6 278 0.035 87 0.876 4 0.021 68 3. 预冲击减敏现象的数值模拟
3.1 TATB基炸药二次冲击起爆实验的数值模拟
二次压缩实验在口径57 mm火炮上实施,其原理与另一种炸药的二次压缩实验[30]相同。飞片由蓝宝石和约1 mm的Kel-F粘合而成,由于蓝宝石波阻抗远高于Kel-F,撞击炸药后向Kel-F传入的冲击波在蓝宝石界面形成高压力反射波,反射波再入射炸药形成二次冲击压缩。典型的二次冲击压缩炸药粒子速度曲线如图4所示。由图4(a)可见:前驱波为不会形成爆轰的弱波,波后压力为6.136 GPa;前驱波入射后约0.45 μs,第二次入射波进入炸药,其波后压力为12.650 GPa。第二次压缩波未追赶上前驱波前,炸药无明显反应增长行为。第二次压缩波在距离炸药端面约4 mm位置,追上第一次入射波。从第二次压缩波进入炸药开始,到爆轰距离为9.85 mm,到爆轰时间为1.72 μs。图3为入射压力12.514 GPa的一次冲击起爆的粒子速度曲线,入射压力比二次加载的主波略小,一次冲击起爆到爆轰距离为8.54 mm,到爆轰时间为1.60 μs,可以判断预冲击压缩对炸药的减敏作用。
图5为二次冲击起爆数值模拟结果,当主波进入炸药后且未追上前驱波前,粒子速度无增长行为,而追上前驱波后,反应才明显发生并发展为爆轰。表4为二次冲击起爆实验和数值模拟的到爆轰距离L*和到爆轰时间T*,L*和T*均以主波进入炸药开始计。由表4可见,数值模拟结果与实验结果较好符合。图6为一次冲击起爆、二次冲击起爆不同位置计算单元化学反应份额的变化曲线,二次冲击时:距离炸药端面2 mm处,受前驱波的作用发生减敏,反应份额的增长为一条缓慢曲线,可以理解为受压力和温度影响的缓慢燃烧过程;距离炸药端面5 mm处,单元不受前驱波影响,反应份额迅速增长,理解为受冲击作用的剧烈燃烧过程。一次冲击时,距离炸药端面2、5 mm的计算单元反应份额增长与二次冲击距离端面5 mm的单元类似,都为迅速增长。这说明,AWSD模型在不影响弱冲击起爆时又可以较好模拟预冲击减敏过程。图7为不同位置的温度变化,多次冲击时,炸药单元(2 mm位置处)温升比只经过一次冲击(5 mm位置)的温升低,因此,依赖于温度的AWSD模型可以区分多次冲击炸药状态的差异。
表 4 二次冲击起爆实验的到爆轰距离和到爆轰时间的实验和数值模拟结果Table 4. Experimental and simulated results of distance and time of run to detonation by double-shock initiation实验 方法 ρ0/(g·cm−3) v0/(km·s−1) p0/GPa pm/GPa L*/mm T*/μs 3 实验 1.881 1.669 6.135 6 12.649 9 9.845 1.716 数值模拟 10.141 1.679 2 实验 1.881 1.732 6.470 6 12.706 4 9.145 1.356 数值模拟 9.240 1.505 3.2 TATB基炸药拐角效应的数值模拟
钝感炸药爆轰波经过拐角后,由于侧向稀疏作用,爆轰波被稀疏为5~9 GPa范围的无法起爆炸药的弱冲击波,使拐角附近炸药经历一次预冲击压缩;随着后续爆轰波的发展,也逐渐扩展到拐角区域炸药,但受到弱波预冲击区域的炸药始终无法形成爆轰,即为死区现象[31]。本文中采用文献[13, 15]中的模拟LX-17炸药爆轰波过拐角的凸井实验[32]的相似模型。图8为实验图像[32],由于炸药比LX-17略微钝感,且采用拉氏方法模拟,完全按照凸井实验建模会出现大变形问题,所以将模拟模型作了适当调整,但是不改变爆轰波过拐角的爆轰过程。图9为计算模型,起爆器区域给一个高压区并采用比体积起爆模拟雷管组件引爆起爆器,其他区域炸药分别采用AWSD模型和WSD模型[12]模拟,炸药起爆后爆轰波沿着金属钨滑移传播,到达金属钨右上角后发生绕爆。网格尺度为0.1 mm,可以保证一个反应区内有15个网格。
图10为AWSD模型模拟结果,到4 μs时爆轰波到达拐角,由于左边侧向稀疏作用,爆轰波被稀疏使拐角附近炸药经历一次预冲击压缩,随着向上的爆轰波的发展,也逐渐扩展到左边区域炸药,但是受到弱波预冲击区域的炸药始终无法形成爆轰,形成了稳定的死区。死区特征与文献[13, 31-33]的实验和数值模拟结果相似。
图11为WSD模型模拟结果,爆轰波过拐角后,拐角附近炸药也经历一次预冲击压缩,但上方的爆轰波迅速向预压缩区域发展,约2 μs再次形成稳定爆轰,预压缩区域的炸药也逐渐反应完成,无法形成死区。对比图10~11可见,依赖温度的AWSD模型可以较好模拟拐角效应的死区的形成过程,而依赖压力的WSD模型无法模拟死区现象。
4. 结 论
在二维结构网格拉氏弹塑性流体力学程序中,引入基于温度和压力的AWSD反应速率模型及未反应炸药、产物的Davis状态方程。通过冲击雨贡纽实验、一维冲击起爆实验标定了状态方程参数及反应率模型参数后,针对一种TATB基钝感炸药的二次冲击起爆及拐角效应等预冲击减敏问题开展数值模拟研究,得到以下结论:
(1)二次冲击的模拟,受预压缩区域的炸药反应变慢,到爆轰距离增长约为1 mm,与该炸药二次冲击实验减敏现象相符;
(2)模拟拐角效应时,爆轰波经过拐角后,在拐角附近形成稳定的不起爆区域,与炸药主要成分相同的LX-17炸药的拐角效应实验的死区特征相符;
(3)无需引入冲击减敏模块,依赖温度的AWSD模型适用于模拟预冲击减敏现象,可以为工程中炸药预冲击减敏问题的模拟提供参考,由于模型包含冲击温度,后续还可以开展不同初始温度条件下的预冲击减敏的模拟研究。
-
表 1 未反应TATB基炸药的Davis状态方程参数
Table 1. Davis EOS parameters of unreacted TATB-based explosive
A/(mm·μs−1) B C Z Γ0s E0/(kJ·g−1) αst cVs/(kJ·g−1·K−1) 1.93 4.26 0.30 0 0.56 3.80 0.757 0 0.000 967 表 2 TATB基炸药产物的Davis状态方程参数
Table 2. Davis EOS parameters of reaction products of TATB-based explosive
A k vc/(cm3·g−1) pc/GPa n b cVg/(kJ·g−1·K−1) 0.835 603 1.30 0.926 85 1.485 1 4.242 66 0.85 0.001 072 表 3 TATB基炸药AWSD模型参数
Table 3. Parameters of the AWSD model for the TATB-based explosive
np ps/GPa k1/s−1 T1/K a1 b1 b2 k2/s−1 T2/K fs λc δλ 0.651 5 27.60 336 1 724 0.060 81 2.122 0.9 10 200 6 278 0.035 87 0.876 4 0.021 68 表 4 二次冲击起爆实验的到爆轰距离和到爆轰时间的实验和数值模拟结果
Table 4. Experimental and simulated results of distance and time of run to detonation by double-shock initiation
实验 方法 ρ0/(g·cm−3) v0/(km·s−1) p0/GPa pm/GPa L*/mm T*/μs 3 实验 1.881 1.669 6.135 6 12.649 9 9.845 1.716 数值模拟 10.141 1.679 2 实验 1.881 1.732 6.470 6 12.706 4 9.145 1.356 数值模拟 9.240 1.505 -
[1] CAMPBELL A W, DAVIS W C, RAMSAY J B, et al. Shock initiation of solid explosives [J]. Physics of Fluids, 1961, 4(4): 511. DOI: 10.1063/1.1706354. [2] CAMPBELL A W, TRAVIS J R. The shock desensitization of PBX-9404 and composition B-3 [C] // Proceedings of the 8th International Detonation Symposium. Albuquerque: Naval Surface Weapons Center, 1985: 1057−1068. [3] MULFORD R N, SHEFFIELD S A, ALCON R R. Preshock desensitization of PBX explosives [J]. AIP Conference Proceedings, 1994, 309(1): 1405–1408. DOI: 10.1063/1.46243. [4] TARVER C M, COOK T M, URTIEW P A, et al. Multiple shock initiation of LX-17 [C] // Proceedings of the 10th International Detonation Symposium. Boston: Office of Naval Research, 1993: 676−703. [5] BAT’KOV Y V, GLUSHAK B L, NOVIKOV S A. Desensitization of pressed explosive compositions based on TNT, RDX, and HMX under double shock-wave loading [J]. Combustion, Explosion and Shock Waves, 1995, 31(4): 482–485. DOI: 10.1007/BF00789372. [6] GUSTAVSEN R L, SHEFFIELD S A, ALCON R R, et al. Double shock initiation of the HMX based explosive EDC-37 [J]. AIP Conference Proceedings, 2002, 620(1): 999–1002. DOI: 10.1063/1.1483706. [7] MADER C L. Numerical modeling of explosives and propellants [M]. 3rd ed. Boca Raton: CRC Press, 2008. [8] HUSSAIN T, LIU Y, HUANG F L, et al. Modeling and simulation of preshock desensitization in heterogeneous explosives using a mesoscopic reaction rate model [J]. Simulation, 2015, 91(11): 980–988. DOI: 10.1177/0037549715608962. [9] BORDZILOVSKII S A, KARAKHANOV S M. Desensitization of pressed RDX/paraffin and HMX/paraffin compounds by multiple shock waves [J]. Combustion, Explosion and Shock Waves, 1995, 31(2): 227–235. DOI: 10.1007/BF00755754. [10] LEE E L, TARVER C M. Phenomenological model of shock initiation in heterogeneous explosives [J]. The Physics of Fluids, 1980, 23(12): 2362–2372. DOI: 10.1063/1.862940. [11] JOHNSON J N, TANG P K, FOREST C A. Shock-wave initiation of heterogeneous reactive solids [J]. Journal of Applied Physics, 1985, 57(9): 4323–4334. DOI: 10.1063/1.334591. [12] WESCOTT B L, STEWART D S, DAVIS W C. Equation of state and reaction rate for condensed-phase explosives [J]. Journal of Applied Physics, 2005, 98(5): 053514. DOI: 10.1063/1.2035310. [13] DEOLIVEIRA G, KAPILA A K, SCHWENDEMAN D W, et al. Detonation diffraction, dead zones and the ignition and growth model [C] // Proceedings of the 13th International Detonation Symposium. Norfolk: Office of Naval Research, 2006. [14] HUSSAIN T, LIU Y, HUANG F L, et al. Desensitization by pre-shocking in heterogeneous explosives and its numerical modelling [J]. Central European Journal of Energetic Materials, 2016, 13(2): 357–379. DOI: 10.22211/cejem/64990. [15] 郝鹏程, 冯其京, 洪滔, 等. 钝感炸药点火增长模型的欧拉数值模拟 [J]. 爆炸与冲击, 2012, 32(3): 243–250. DOI: 10.11883/1001-1455(2012)03-0243-08.HAO P C, FENG Q J, HONG T, et al. Eulerian simulation on insensitive explosives with the ignition-growth reactive model [J]. Explosion and Shock Waves, 2012, 32(3): 243–250. DOI: 10.11883/1001-1455(2012)03-0243-08. [16] STARKENBERG J. Shock-pressure and pseudo-entropic approaches to explosive initiation modeling [C] // Proceedings of the 15th International Detonation Symposium. San Francisco, 2014: 908−916. [17] STARKENBERG J. Modeling detonation propagation and failure using explosive initiation models in a conventional hydrocode [C] // Proceedings of the 12th International Detonation Symposium. Annapolis: Office of Naval Research, 2002. [18] HANDELEY C A. CREST reactive flow model [C] // Proceedings of the 13th International Detonation Symposium. Norfolk, 2006. [19] DESBIENS N, MATIGNON C, SORIN R. Temperature-based model for condensed-phase explosive detonation [J]. Journal of Physics: Conference Series, 2014, 500(15): 152004. DOI: 10.1088/1742-6596/500/15/152004. [20] ASLAM T D. Shock temperature dependent rate law for plastic bonded explosives [J]. Journal of Applied Physics, 2018, 123(14): 145901. DOI: 10.1063/1.5020172. [21] 裴红波, 刘俊明, 张旭, 等. 基于反向撞击法的JB-9014炸药Hugoniot关系测量 [J]. 爆炸与冲击, 2019, 39(5): 052301. DOI: 10.11883/bzycj-2017-0395.PEI H B, LIU J M, ZHANG X, et al. Measurement of Hugoniot relation for unreacted JB-9014 explosive with reverse-impact method [J]. Explosion and Shock Waves, 2019, 39(5): 052301. DOI: 10.11883/bzycj-2017-0395. [22] 刘俊明, 张旭, 裴红波, 等. JB-9014钝感炸药冲击Hugoniot关系测量 [J]. 高压物理学报, 2018, 32(3): 033202. DOI: 10.11858/gywlxb.20170669.LIU J M, ZHANG X, PEI H B, et al. Measurement of Hugoniot relation for JB-9014 insensitive explosive [J]. Chinese Journal of High Pressure Physics, 2018, 32(3): 033202. DOI: 10.11858/gywlxb.20170669. [23] 张旭, 池家春, 冯民贤. JB9014钝感炸药冲击绝热线测量 [J]. 高压物理学报, 2001, 15(4): 304–308. DOI: 10.11858/gywlxb.2001.04.011.ZHANG X, CHI J C, FENG M X. Hugoniot relation of JB9014 insensitive high explosive [J]. Chinese Journal of High Pressure Physics, 2001, 15(4): 304–308. DOI: 10.11858/gywlxb.2001.04.011. [24] 刘俊明. JB-9014炸药未反应状态方程研究[D]. 四川绵阳: 中国工程物理研究院, 2018. [25] 徐辉, 孙占峰. 钝感高能炸药JB-9014做功能力的实验研究 [J]. 高压物理学报, 2013, 27(4): 582–586. DOI: 10.11858/gywlxb.2013.04.018.XU H, SUN Z F. An experimental study on the capacity for work of insensitive high explosive [J]. Chinese Journal of High Pressure Physics, 2013, 27(4): 582–586. DOI: 10.11858/gywlxb.2013.04.018. [26] 曾代朋, 陈军, 谭多望. 超压爆轰产物冲击绝热线的实验研究 [J]. 高压物理学报, 2010, 24(1): 76–80. DOI: 10.11858/gywlxb.2010.01.014.ZENG D P, CHEN J, TAN D W. Experiment research on the Hugoniot of JB-9014 explosive over-driven detonation product [J]. Chinese Journal of High Pressure Physics, 2010, 24(1): 76–80. DOI: 10.11858/gywlxb.2010.01.014. [27] TANG P K, ANDERSON W W, FRITZ J N, et al. A study of the overdriven behaviors of PBX 9501 and PBX 9502 [C] // Proceedings of the 11th International Detonation Symposium. Snowmass, CO, 1998. [28] 张琪敏, 张旭, 赵康, 等. TATB基钝感炸药JB-9014的冲击起爆反应增长规律 [J]. 爆炸与冲击, 2019, 39(4): 041405. DOI: 10.11883/bzycj-2018-0050.ZHANG Q M, ZHANG X, ZHAO K, et al. Law of reaction growth of shock initiation on the TATB based insensitive explosive JB-9014 [J]. Explosion and Shock Waves, 2019, 39(4): 041405. DOI: 10.11883/bzycj-2018-0050. [29] 黄文斌, 文尚刚, 谭多望, 等. 在球面散心冲击波作用下JB-9014炸药冲击引爆过程实验研究 [J]. 爆炸与冲击, 2006, 26(4): 373–376. DOI: 10.11883/1001-1455(2006)04-0373-04.HUANG W B, WEN S G, TAN D W, et al. Experimental study on shock initiation process of JB-9014 under loading conditions of divergent spherical shock wave [J]. Explosion and Shock Waves, 2006, 26(4): 373–376. DOI: 10.11883/1001-1455(2006)04-0373-04. [30] 訾攀登, 陈军, 张蓉, 等. 二次压缩条件下JOB-9003炸药特性研究 [J]. 高压物理学报, 2017, 31(2): 155–161. DOI: 10.11858/gywlxb.2017.02.007.ZI P D, CHEN J, ZHANG R, et al. Characteristics of JOB-9003 in double shocks experiments [J]. Chinese Journal of High Pressure Physics, 2017, 31(2): 155–161. DOI: 10.11858/gywlxb.2017.02.007. [31] FERM E N, MORRIS C L, QUINTANA J P, et al. Proton radiography examination of unburned regions in PBX 9502 corner turning experiments [J]. AIP Conference Proceedings, 2002, 620(1): 966–969. DOI: 10.1063/1.1483699. [32] SOUERS P C, ANDRESKI H G, COOK Ⅲ C F, et al. LX-17 corner-turning [J]. Propellants, Explosives, Pyrotechnics, 2004, 29(6): 359–367. DOI: 10.1002/prep.200400067. [33] WHITWORTH N J. CREST modelling of PBX 9502 corner turning experiments at different initial temperatures [J]. Journal of Physics: Conference Series, 2014, 500(5): 052050. DOI: 10.1088/1742-6596/500/5/052050. 期刊类型引用(3)
1. 韩甲旭,王健,刘通有. 装药密度和约束效应对冲击起爆特性规律研究. 兵器装备工程学报. 2024(03): 76-85 .  百度学术
百度学术2. 樊辉,刘坤,谷岩,孙占峰. 爆轰加载下TATB基钝感炸药的冲击-卸载-再冲击实验装置设计与模拟. 高压物理学报. 2024(04): 81-91 .  百度学术
百度学术3. 周蕊,李理,田保林. 爆轰驱动多介质问题的Lagrange多分区自适应数值模拟研究. 力学学报. 2023(11): 2675-2692 .  百度学术
百度学术其他类型引用(0)
-









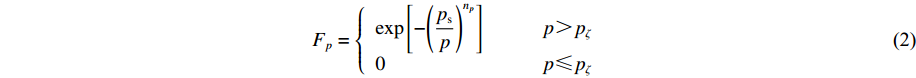


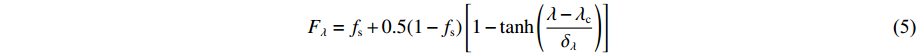
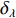







 下载:
下载:
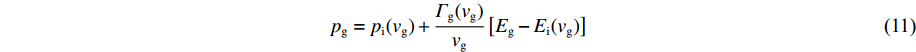





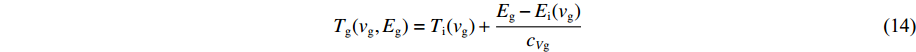














 下载:
下载:










 百度学术
百度学术