Propagation of combined wave with phase transformation in pseudo-elastic TiNi alloy thin-walled tubes
-
摘要: 相变可以改变材料的性质,从而严重影响波在介质中传播的结构。采用考虑静水压力和偏应力联合作用的增量型相变本构模型,研究了在拉(压)-扭联合作用下半无限长TiNi合金薄壁管内相变复合波的传播规律。基于广义特征理论分析了相变复合波的特征波速及简单波解的基本性质。利用数值方法研究了两种典型情况下管内相变耦合波传播的规律,管内传播的应力路径和波的结构与初始状态及加载幅值有关,展现出和普通弹塑性材料截然不同的性质。Abstract: Phase transformation can seriously modify the properties of the materials and therefore impact the stress wave propagation features inside the materials. A simplified incremental phase transformation constitutive model, considering both the hydrostatic pressure and the deviatoric stress, is used to study the propagation of phase transformation coupled waves in a semi-infinite thin-walled tubes under the combined tension (compression) and torsion impact loading. The generalized characteristic theory is used to analyze the basic properties of the characteristic wave velocity and simple wave solution. Two kinds of typical solutions are studied by using numerical method. The stress paths and wave structure are related to the initial state and the loading amplitude, exhibiting the different properties from conventional elastoplastic materials.
-
Key words:
- phase transformation /
- combined stress wave /
- pseudo-elastic /
- TiNi alloy /
- thin-walled tubes
-
相变是一种典型的自然现象,固体材料在受到爆炸、高速撞击等强冲击载荷作用时,可能会发生屈服甚至相变。冲击下材料的相变特性对于材料和结构的动态响应有着重大影响,因为相变后的材料实质上已经成为一种新材料[1]。TiNi合金是一种典型的相变材料,其响应受热弹性马氏体相变支配,具有独特的伪弹性效应和形状记忆效应。由于其独特的热力学性质,在航空航天、交通运输、生物医学等高技术领域得到大量的应用[2]。
相变引起的材料非线性会强烈改变冲击波波形,造成冲击相变特有的三波结构和卸载冲击波,这些奇特的现象引起越来越多的关注。基于一维的应力-应变关系,以半无限长杆为研究对象,Chen等[3]和Bekker等[4]研究了阶跃载荷作用下相变波的传播问题,研究发现冲击载荷高于某一幅值时,相变区的应力和温度不连续,但对于卸载问题,并没有涉及。王文强等[5]利用简化的相变本构模型来研究宏观相边界的传播规律,在缓慢卸载的应力边界条件下,处于混合相的试件会成为变密度梯度材料。基于相同的理论模型,Bouvet等[6]提出了一种冲击载荷作用下制备功能梯度材料的新方法。和常规弹塑性材料不同的是,相变材料在卸载时会产生卸载冲击波。在矩形脉冲载荷作用下,由于应力波和宏观相边界的相互作用,Dai等[7]发现了一些奇异的现象,如不可逆相变可能会出现成分连续变化的混合相区,可逆相变的卸载相变界会出现分叉等。此外,采用不同的本构模型以及考虑边界条件的影响,进行了大量有关相变波和相边界传播规律方面的研究[8-11]。
以上的这些研究都是基于一维应力状态条件下进行的。大量的研究结果表明,复杂应力状态下的相变现象和单轴加载会有所不同。Sittner等[12]在研究多晶Cu-Al-Zn-Mn形状记忆合金时发现马氏体相变过程和加载路径有关。Sun等[13]对形状记忆合金薄壁管进行了一系列的实验方面的研究,揭示了外部应力状态及内部微观结构对材料宏观行为的影响。为了确定相变材料在复杂应力状态下的力学行为,大量实验和理论方面的工作就此展开[14-20]。这些研究主要是在准静态复合加载条件下进行,目的是为了得到描述材料相变行为的本构模型。而在复杂应力状态下,有关冲击相变方面的研究并不多。
近年来,复合应力状态下相变波的研究已有零星的文献发表。Song等[21]采用广义特征理论,研究了TiNi合金薄壁圆管在复合加载下相变波的传播规律。在薄壁圆管端部施加阶跃纯扭转载荷至混合相,首先会出现弹性压缩波,而在传统弹塑性材料中不会出现轴向应力的变化。在强冲击载荷作用下,材料具有二相硬化效应,奥氏体会直接转化为马氏体。Wang等[22]考虑了二相硬化效应的影响,采用数值方法,研究了拉-扭联合作用下弹性波追赶相变耦合波过程中波系结构的变化。然而,相变材料通常具有明显的拉压不对称性[1],相变面在σ-τ平面上沿着σ轴移动,与拉-扭联合加载相比,压−扭联合加载下薄壁圆管内会出现一些新的现象。本文在以上工作的基础上,以半无限长处于伪弹性状态的TiNi合金薄壁圆管为研究对象,综合考虑材料的二相硬化效应和拉压不对称性,得到TiNi合金薄壁管内相变耦合波传播的特征波速和简单波解,并分析两类典型初始条件下相变波传播的应力路径。
1. 动力学方程和相变本构模型
1.1 动力学基本方程
考虑半无限长TiNi合金薄壁圆管,壁厚为δ,内外圆截面的平均半径为R,且δ
≪ R。以其端部截面的中心为圆点O,以轴线为X轴建立如图1所示的坐标系。假设薄壁管除端部受有拉(压)扭载荷作用外,不受其他外载。由于管壁较薄,忽略惯性效应的影响,各物理量在厚度方向是均匀的,从而可以把管内波的传播简化为一维复合应力波沿着X轴的传播。薄壁圆管的运动方程为:
ρ∂u∂t−∂σ∂x=0 (1) ρ∂v∂t−∂τ∂x=0 (2) 式中:ρ为密度,σ和τ分别为轴向应力和环向切应力,u和v分别为质点的轴向速度和环向速度。根据柱坐标中应变和质点速度的定义,可以得到系统的连续性方程为:
∂ε∂t−∂u∂x=0 (3) ∂γ∂t−∂v∂x=0 (4) 式中:ε为轴向应变,γ为环向切应变。
1.2 相变准则
对TiNi合金而言,由应力诱发的相变在宏观上有一定的体积改变,主要体现为剪切变形。由于相变过程中体积发生变化,其相变行为同时受到静水压力及偏应力的影响,具有明显的拉压不对称性。郭扬波等[23]研究了TiNi合金的相变行为时提出了同时考虑偏应力和静水压力的相变临界准则:
pεv+σeγe=Φ(ξ,T) (5) 式中:
p=σkk/3 为静水压力,εv 为相变过程中的体积应变,σe=√3SijSij/2 为Mises等效应力,Sij 为偏应力张量分量,γe 为相变过程中的等效偏应变。本文中主要考虑相变应力波在薄壁管内的传播规律,忽略冲击过程中温度的变化。TiNi合金薄壁圆管在拉(压)-扭联合作用下,其静水压力p = σ/3,Mises等效应力
σe=√σ2+3τ2 。引入拉压不对称系数α=εv/(3γe) 和相变功正相关的参数k=Φ/γe√3(1−α2), ,从而式(5)可简化为:f(σ,τ)=(σ+βkθ)2+τ2−k2=0 (6) 式中:
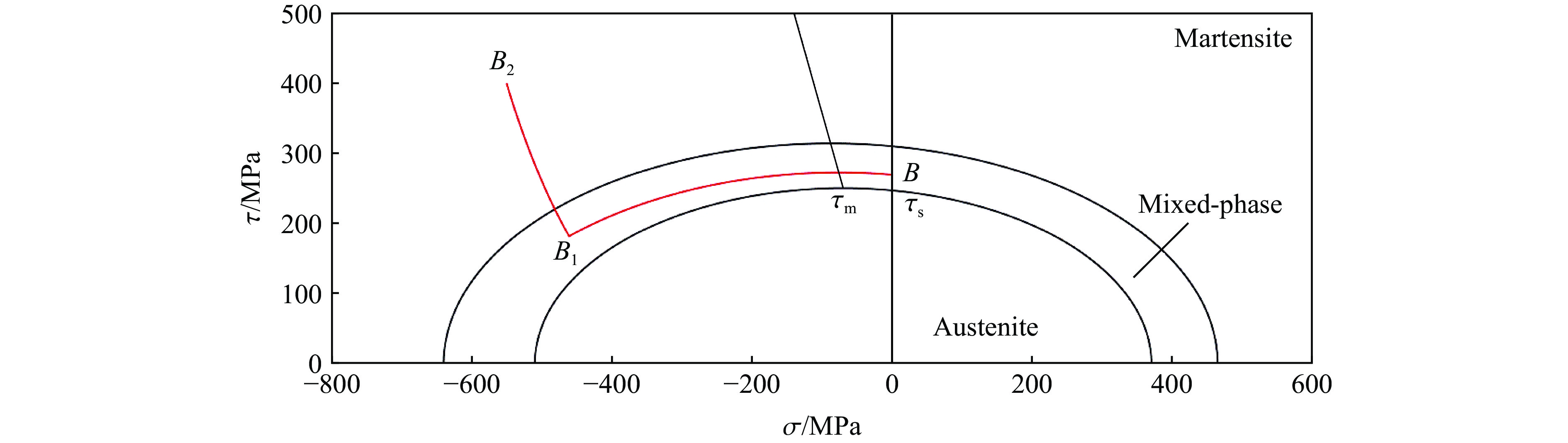
θ=√3/(1−α2) ,β=α√3/(1−α2) ,相变参数α和k可以通过一维拉伸实验确定。根据式(6),在σ-τ平面上的相变临界面是中心偏离原点的椭圆,偏移的距离为βk,如图2所示。对于管内某点处的应力状态(σ, τ),通过式(6)都可以唯一确定相应的正数k,假设
k1 和k2 分别为相变起始和相变结束时对应的相变参数。若0<k<k1 ,材料处于奥氏体弹性状态;当k1<k<k2 时,材料进入混合相;而当k≥k2 时,材料完全转化为马氏体。1.3 增量型的本构关系
对处于伪弹性状态的TiNi合金而言,其一维应力条件下准静态拉伸的应力-应变曲线如图3所示。图中A和B分别为马氏体相变的起始点和完成点,D和F为逆相变的起始点和完成点,AB和DF之间的区域为混合相区。
材料的相变演化行为,不同文献中有很多不同的描述形式。这里采用一种相对简单的增量型本构关系,用以描述相变材料的力学行为。材料发生相变时其应变率分为如下两部分:
˙ε=˙εe+˙εp (7) ˙γ=˙γe+˙γp (8) 式中:下标“e”和“p”分别代表弹性应变和相变应变部分。对于各向同性的TiNi合金而言,应变率的弹性部分和相变部分可分别表示为:
˙εe=σtE,˙εp=˙λ∂f∂σ (9) ˙γe=τtG,˙γp=˙λ∂f∂τ (10) 式中:
˙λ 为表征相变程度的相变因子,其值恒为正。采用各向同性硬化的加载准则,相变功率w可以表示为:w=σ˙εp+τ˙γp (11) 联立式(9)~(11),可得到
˙λ 的表达式为:˙λ=dwdk˙k2(σ(σ+βk)θ2+τ2) (12) 假设TiNi合金在简单拉伸条件下的一维应力−应变的函数关系为
σ=F(ε) (13) 则有:
dσ=F′[ε(σ)](dεe+dεp)=Em(dσE+dwσ) (14) 式中:
Em=F′(ε) 为应力-应变曲线的斜率。当材料加载至混合相时,Em 为混合相的模量;而当冲击载荷σ的幅值超过相变完成应力σB时,材料由奥氏体直接转变为马氏体,Em 为虚线AQ的斜率,如图3所示。定义和材料相关的参数θv ,其表达式为:θv={−(β−θ)σ≥0−(β+θ)σ<0 (15) 联立式(11)、(12)、(14)和式(15),可以得到相变因子的表达式为
˙λ=θ2θ2v(1Em−1E)k˙k2(k2θ2−σvβk) (16) 式中:
σv=σ+βk 。根据式(6)可得:˙k2=2k˙k=2(σv˙σθ2+τ˙τ) (17) 将式(15)、(16)和(17)代入式(7)和(8),可得到描述材料相变行为的本构关系:
˙ε=(1E+Sσ2vθ2)˙σ+Sσvτ˙τ (18) ˙γ=Sσvτ˙σ+(1G+Sτ2θ2)˙τ (19) 式中:
S=θ2θ2v(1Em−1E)k2θ2−σvβk 。将本构关系式(18)~(19)代入式(3)~(4),并联立式(1)~(2),可以得到应力波在TiNi合金薄壁管内传播的控制方程,写成矩阵形式为:
A⋅˜Wt+B⋅˜Wx=0 (20) 式中:
˜W=(u,v,σ,τ)T ,上标“T”表示转置。A=(ρIOOH) ,B=(O−I−IO) ,I=(1001) ,O=(0000) ,柔度张量H=(1E+Sσ2vθ2SσvτSσvτ1G+Sτ2θ2) 。1.4 特征关系和简单波解
矩阵A和B及柔度张量H都是实对称矩阵。由广义特征理论可知,薄壁管中的特征波速C由矩阵B相对于矩阵A的特征值决定的,即
||B−CA||=0 (21) 采用分块矩阵的记法,可以得到:
B−CA=(−ρCI−I−ICH)=(−ρCIO−II−ρC2H)(II/(ρC)OI/(ρC)) (22) 利用式(22),特征方程(21)可以简化为:
||B−CA||=(ρC2)||I−ρC2H||(1ρC)2=0 (23) 即:
||H−φI||=0 (24) 式中:
φ=1/(ρC2) 。展开特征方程(24),可得
Φ(φ)=(1E−φ)(1G−φ)+S[(1E−φ)τ2θ2+(1G−φ)σ2vθ2]=0 (25) 显然有
Φ(+∞)>0 ,Φ(1G)≤0 ,Φ(1E)≥0 ,因此式(26)的两个根φs 和φf 必然满足如下的关系:1E≤φf≤1G≤φs<+∞ (26) 设C0和C2分别表示弹性纵波波速和弹性横波波速,即
E=ρC20 和G=ρC22 。式(27)可以改写为:0<Cs≤C2≤Cf≤C0 (27) 式(27)表明处于伪弹性状态的TiNi合金薄壁管内相变耦合慢波的波速
Cs 必然小于或等于弹性横波波速,相变耦合快波的波速Cf 介于弹性横波波速C2 和弹性纵波波速C0 之间。得到特征波速之后,可以得到特征向量R的表达式为:R=(H12ρC(H11−φ),−1ρC,−H12H11−φ,1)T (28) 因此,简单波解为:
duH12ρC(H11−φ)=dv−1ρC=dσ−H12H11−φ=dτ1=ηdζ (29) 式中:η为任意常数。根据式(29),决定TiNi合金薄壁管内相变耦合慢波和快波应力路径的微分方程为:
dσdτ=1G+Sτ2θ2−φ−Sσvτ (30) 进一步化简式(30),可得如下形式
dσdτ=1−(CC2)21−(CC0)2σvτθ2 (31) 以各种不同的应力状态
(σ0,τ0) 为初始条件对方程(30)或(31)进行积分,可以得到相应的应力路径σ=σ(τ,σ0,τ0) 。以上的分析并没有指明薄壁管是处于冲击拉-扭加载还是压-扭加载;在分析过程中也并没有指明加载到最终状态时的材料是处于混合相还是马氏体相,因此,本文中的分析对上述各种情况都是适用的。2. 数值计算及讨论
在薄壁管的拉-扭或压-扭动态试验中,Clifton等[24-26]基于霍普金森压杆,建立了一套薄壁圆管预扭冲击加载装置,对3003铝材和α-Ti进行了实验研究,观察到了复合应力波在薄壁管内的传播。TiNi合金压缩时的临界应力较高且材料“偏脆”,在进行预扭冲击压缩实验时,薄壁管容易发生屈曲。加载幅值在相变临界应力附近,在靠近薄壁管的端部出现了局部破裂。无法在实验中观察到相变耦合波的传播。考虑到TiNi合金的拉压不对称性,且拉伸比压缩更容易发生相变,王波等[27]对TiNi合金薄壁管进行了预扭冲击拉伸实验,观察到了拉-扭复合应力状态下相变波的传播。数值计算的结果和实验吻合的较好,可以较好地区分出相变耦合快波和慢波的波系结构。在以上工作的基础上,为避免实验上的不确定性,本文中采用数值方法来研究应力波在TiNi合金薄壁管中的传播规律。
2.1 差分格式
采用一阶Lax-Friedrichs格式对控制方程进行离散,联立相变材料的本构方程和初、边值条件,就可以得到拉(压)-扭载荷联合作用下相变复合波在薄壁管内的传播规律。控制方程的离散形式为:
{un+1j=12(unj−1+unj+1)+12ρΔtΔx(σnj+1−σnj−1)vn+1j=12(vnj−1+vnj+1)+12ρΔtΔx(τnj+1−τnj−1)εn+1j=12(εnj−1+εnj+1)+12ΔtΔx(unj+1−unj−1)γn+1j=12(γnj−1+γnj+1)+12ΔtΔx(vnj+1−vnj−1) (32) 式中:Δt和Δx为时间增量和空间步长。将本构方程(18)和(19)进行改写后的离散格式为
{σn+1j=σnj+(1G+Snjτnj2θ2)(εn+1j−εnj)−Snjσnvjτnj(γn+1j−γnj)(1E+Snjσnvjθ2)(1G+Snjτnj2θ2)−(Snjσnvjτnj)2τn+1j=τnj+(1E+Snjσnvjθ2)(γn+1j−γnj)−Snjσnvjτnj(εn+1j−εnj)(1E+Snjσnvjθ2)(1G+Snjτnj2θ2)−(Snjσnvjτnj)2 (33) 根据相应的初始条件和边界条件,知道了n时间步的应力、应变和粒子速度之后,通过控制方程和本构方程的离散形式(32)和(33),可以确定第n+1时间步的应力、应变和粒子速度,从而得到了相变波在管内的传播规律。计算中所用的材料参数如表1所示。
表 1 TiNi合金的材料参数Table 1. Material Parameters of TiNi Alloyρ/(kg∙m−3) E/GPa μ Em/GPa α k1/MPa k2/MPa 6450 63.7 0.3 5 0.159 250.8 314.8 需要说明的是,表1中的Em为材料进入混合相时的模量,材料由奥氏体直接转变为马氏体时的模量根据加载前后应力的幅值重新计算。
2.2 计算结果
如果管内材料始终处于奥氏体弹性状态,薄壁管内传播的是互不耦合的弹性纵波和弹性横波,我们更关心管内材料发生相变后波的传播规律。假设初始应力状态(σi, τi)时的相变参数为
ki ,最终应力状态(σf , τf)时的相变参数为kf ,以下的算例都基于ki<kf 且k1<kf 。需要说明的是,若k1<kf<k2 ,此时薄壁管内相变波的结构同常规弹塑性材料类似;若kf>k2 ,加载后材料由奥氏体直接转变为马氏体,管内形成相变耦合冲击波,在σ-τ空间的应力路径为从初始相变面上某点出发的广义Hugoniot线[28]。两者虽然有本质的不同,但在σ-τ应力空间内很容易区分,以下统一称为相变耦合波。根据不同的初始条件和边界条件,讨论以下两种典型情况。(1)假定TiNi合金薄壁管最初是静止的且不受任何作用力,在端部突然施加正应力σ =10 MPa和剪应力τ = 480 MPa。
根据前面的理论分析,在图4的σ-τ平面上的应力路径为:
OC0→A2(−8.01,0)C2→A3(−8.01,248.3)Cs→A1(10,480) (34) 式(34)中的单位为MPa。不同时刻管内的应力分布如图5所示。图4上τs为纯扭转时的相变起始应力,τm为相变起始面上剪应力最大值点。对比图4和图5可以发现,尽管突然施加在管端部的轴向载荷为拉应力,但在管内首先是以波速C0传播的弹性压缩波,将材料由零应力状态加载至A2(−8.01 MPa, 0),随后依次传播的是剪切波和相变耦合慢波。计算的结果表明,对于初始状态自然静止的半无限长TiNi合金薄壁管,杆端突然施加的恒值载荷位于应力空间中蓝色区域(包括区域的边界)时,管内都会出现类似的现象。这种现象在常规弹塑性材料的薄壁管中并不会出现。原因在于材料发生相变时引起体积变化,在σ-τ平面上相变起始面左移,相变耦合慢波路径的起点A3位于相变起始面上,其剪应力幅值处于τs和τm之间。在奥氏体弹性阶段,管内传播的是互不耦合的弹性纵波和弹性横波,在σ-τ平面上的应力路径只能是沿着OA2传播的弹性纵波及随后沿着A2A3传播的弹性横波。
(2) 薄壁管的端部预先施加扭转载荷τi = 270 MPa,在t = 0时刻突然施加恒值的压−扭载荷至最终应力状态B2(−550 MPa,400 MPa)。其应力路径如图6所示。
B(0,270)Cs→B1(−460.8,182.8)Cf→B2(−550,4000) (35) 材料由初始状态B经相变复合快波以波速Cf加载至B1时,管内正应力的幅值单调增大,而切应力的幅值则先增大后减小,如图7所示。这种现象在施加拉−扭阶跃载荷时并不会出现。根据式(28),相变耦合快波的速度Cf高于剪切波波速C2,在跨过相变椭圆的最高点的右侧,根据式(32), dσ/dτ<0,因此随着正应力幅值的增大,剪应力的幅值也会增大;而在相变椭圆最高点的左侧,根据式(32), dσ/dτ>0,因此随着正应力幅值的增大,剪应力的幅值会降低。从而在快波区剪应力的幅值会出现先增大后减小这种现象。
3. 结 论
相变过程伴随着体积和形状的改变,使得相变材料具有明显的拉压不对称性。本文采用考虑静水压力和偏应力联合作用的相变准则,基于增量型相变本构模型,得到相变耦合波在半无限长TiNi合金薄壁圆管内传播的控制方程。利用广义特征理论分析了TiNi合金薄壁管的特征波速和简单波解,确定了决定应力路径的微分方程。利用一阶Lax-Friedrichs有限差分格式对控制方程及相变本构关系进行离散。数值算例分析了两种典型初始条件,即初始状态静止以及预扭至混合相时,在阶跃联合载荷作用下薄壁管内相变耦合波传播的应力路径,以及各阶段应力随时间的变化,并对反常现象进行了解释。
-
表 1 TiNi合金的材料参数
Table 1. Material Parameters of TiNi Alloy
ρ/(kg∙m−3) E/GPa μ Em/GPa α k1/MPa k2/MPa 6450 63.7 0.3 5 0.159 250.8 314.8 -
[1] 唐志平. 冲击相变[M]. 北京: 科学出版社, 2008. [2] SELLITTO A, RICCIO A. Overview and future advanced engineering applications for morphing surfaces by shape memory alloy materials [J]. Materials, 2019, 12(5): 708. DOI: 10.3390/ma12050708. [3] CHEN Y, LAGOUDAS D C. Impact induced phase transformation in shape memory alloys [J]. Journal of the Mechanics and Physics of Solids, 2000, 48(2): 275–300. DOI: 10.1016/s0022-5096(99)00044-7. [4] BEKKER A, JIMENEZ-VICTORY J C, POPOV P, et al. Impact induced propagation of phase transformation in a shape memory alloy rod [J]. International Journal of Plasticity, 2002, 18(11): 1447–1479. DOI: 10.1016/s0749-6419(02)00025-6. [5] 王文强, 唐志平. 冲击下宏观相边界的传播 [J]. 爆炸与冲击, 2000, 20(1): 25–31.WANG W Q, TANG Z P. Propagation of macroscopic phase boundary under shock loading [J]. Explosion and Shock Waves, 2000, 20(1): 25–31. [6] BOUVET C, CALLOCH S, LEXCELLENT C. Experimental investigations under biaxial loadings on Cu-Al-Be shape memory alloy[M]. Amsterdam: Elsevier Science, 2000:793-798. [7] DAI X, TANG Z P, XU S L, et al. Propagation of macroscopic phase boundaries under impact loading [J]. International Journal of Impact Engineering, 2004, 30(4): 385–401. DOI: 10.1016/s0734-743x(03)00090-3. [8] 刘永贵, 沈玲燕. 固定温度界面对相变波传播规律的影响 [J]. 高压物理学报, 2018, 32(4): 33–39. DOI: 10.11858/gywlxb.20170559.LIU Y G, SHEN L Y. Effect of the fixed temperature interface on the propagation of the phase transition wave [J]. Chinese Journal of High Pressure Physics, 2018, 32(4): 33–39. DOI: 10.11858/gywlxb.20170559. [9] 徐薇薇, 唐志平, 张兴华. 有限杆中不可逆相边界的传播规律及其应用 [J]. 高压物理学报, 2006(4): 365–371. DOI: 10.3969/j.issn.1000-5773.2006.04.005.XU W W, TANG Z P, ZHANG X H. Propagation of irreversible macroscopic phase boundaries along a finite rod under dynamic loading [J]. Chinese Journal of High Pressure Physics, 2006(4): 365–371. DOI: 10.3969/j.issn.1000-5773.2006.04.005. [10] ZHU P P, DAI H H. Wave Propagation in a shape memory alloy bar under an impulsive loading [J]. Journal of Applied Mechanics, 2016, 83(10): 104502. DOI: 10.1115/1.4034115. [11] LIU Y G, SHAN L Y, SHAN J F, et al. Experimental study on temperature evolution and strain rate effect on phase transformation of TiNi shape memory alloy under shock loading [J]. International Journal of Mechanical Sciences, 2019, 156(6): 342–354. DOI: 10.1016/j.ijmecsci.2019.04.005. [12] SITTNER P, HARA Y, TOKUDA M. Experimental study on the thermoelastic martensitic transformation in shape memory alloy polycrystal induced by combined external forces [J]. Metallurgical and Materials Transactions A, 1995, 26(11): 2923–2935. DOI: 10.1007/bf02669649. [13] SUN Q P, LI Z Q. Phase transformation in superelastic NiTi polycrystalline micro-tubes under tension and torsion–from localization to homogeneous deformation [J]. International Journal of Solids and Structures, 2002, 39(13): 3797–3809. DOI: 10.1016/S0020-7683(02)00182-8. [14] FANG D N, LU W, YAN W Y, et al. Stress–strain relation of CuAlNi SMA single crystal under biaxial loading: constitutive model and experiments [J]. Acta Materialia, 1998, 47(1): 269–280. DOI: 10.1016/s1359-6454(98)00303-6. [15] GRABE C, BRUHNS O T. Path dependence and multiaxial behavior of a polycrystalline NiTi alloy within the pseudoelastic and pseudoplastic temperature regimes [J]. International Journal of Plasticity, 2009, 25(3): 513–545. DOI: 10.1016/j.ijplas.2008.03.002. [16] CISSE C, ZAKI W, ZINEB T B. A review of modeling techniques for advanced effects in shape memory alloy behavior [J]. Smart Materials and Structures, 2016, 25(10). DOI: 10.1088/0964-1726/25/10/103001. [17] CHATZIATHANASIOU D, CHEMISKY Y, HATZIGEORGIOU G, et al. Modeling of coupled phase transformation and reorientation in shape memory alloys under non-proportional thermomechanical loading [J]. International Journal of Plasticity, 2016, 82: 192–224. DOI: 10.1016/j.ijplas.2016.03.005. [18] MEHRABI R, ANDANI M T, KADKHODAEI M, et al. Experimental study of NiTi thin-walled tubes under uniaxial tension, torsion, proportional and non-proportional loadings [J]. Experimental Mechanics, 2015, 55(6): 1151–1164. DOI: 10.1007/s11340-015-0016-2. [19] WANG X M, ZHOU Q T, LIU H, et al. Experimental study of the biaxial cyclic behavior of thin-wall tubes of NiTi shape memory alloys [J]. Metallurgical and Materials Transactions A, 2012, 43(11): 4123–4128. DOI: 10.1007/s11661-012-1225-2. [20] FARAJPOUR M R, SHAHIDI A R, FARAJPOUR A. A nonlocal continuum model for the biaxial buckling analysis of composite nanoplates with shape memory alloy nanowires [J]. Materials Research Express, 2018, 5(3): 035026. DOI: 10.1088/2053-1591/aab3a9. [21] SONG Q Z, TANG Z. Combined stress waves with phase transition in thin-walled tubes [J]. Applied Mathematics and Mechanics, 2014, 35(3): 285–296. DOI: 10.1007/s10483-014-1791-7. [22] WANG B, TANG Z P. Study on the propagation of coupling shock waves with phase transition under combined tension-torsion impact loading [J]. Science China (Physics, Mechanics & Astronomy), 2014, 57(10): 1977–1986. DOI: 10.1007/s11433-014-5468-3. [23] 郭扬波, 唐志平, 徐松林. 一种考虑静水压力和偏应力共同作用的相变临界准则 [J]. 固体力学学报, 2004(4): 417–422. DOI: 10.3969/j.issn.0254-7805.2004.04.009.GUO Y B, TANG Z P, XU S L. A critical criterion for phase transformation considering both hydrostatic pressure and edviatoric stress effects [J]. Aata Mechanica Solida Sinica, 2004(4): 417–422. DOI: 10.3969/j.issn.0254-7805.2004.04.009. [24] LIPKIN J, CLIFTON R J. An experimental study of combined longitudinal and torsional plastic waves in a thin-walled tube [M]. Berlin Heidelberg: Springer, 1969. DOI: 10.1007/978-3-642-85640-2_22. [25] LIPKIN J, CLIFTON R J. Plastic waves of combined stresses due to longitudinal impact of a pretorqued tube: Part 2: comparison of theory with experiment [J]. Journal of Applied Mechanics, 1970, 37(4): 1113. DOI: 10.1115/1.3408667. [26] LIPKIN J, CLIFTON R J. Plastic waves of combined stresses due to longitudinal impact of a pretorqued tube: Part 1: experimental results [J]. Journal of Applied Mechanics, 1970, 37(4): 1113. DOI: 10.1115/1.3408666. [27] 王波, 张科, 唐志平. 薄壁管拉扭复合相变波的实验研究 [J]. 振动与冲击, 2017(22): 35–39. DOI: 10.13465/j.cnki.jvs.2017.22.005.WANG B, ZHANG K, TANG Z P. An experimental study on the stress waves with phase transition under combined tension-torsion loading [J]. Journal of Vibration and Shock, 2017(22): 35–39. DOI: 10.13465/j.cnki.jvs.2017.22.005. [28] TING T C, LI Y. Eulerian formulation of transport equations for three-dimensional shock waves in simple elastic solids [J]. Journal of Elasticity, 1983, 13(3): 295–310. DOI: 10.1007/bf00042998. -









 下载:
下载:

















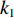












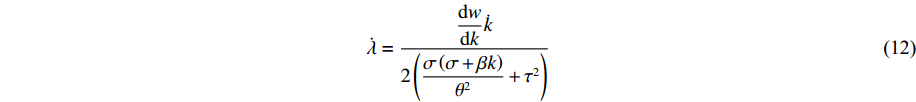









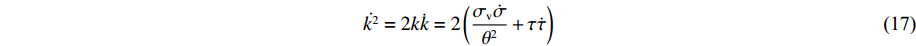


















































 下载:
下载: