De-noising method of tunnel blasting signal based on CEEMDAN decomposition-wavelet packet analysis
-
摘要: 针对隧道爆破施工中采集到的实测振动信号,引入一种基于总体平均经验模态分解方法(CEEMDAN分解)联合小波包分析的降噪方法。首先,通过CEEMDAN分解得到多个本征模态分量,利用相关系数筛选出包含噪声的模态分量,并通过模态分量的频谱图及方差贡献率进行校核。然后,利用小波包阈值降噪方法对含有噪声的模态分量进行处理。最后,将未经处理的模态分量与去噪完成的分量重构得到最终纯净的爆破振动信号。同时,通过小波包能量谱分析验证此降噪方法的可行性。本文引入的方法兼具CEEMDAN分解及小波包分析的优点,与现有方法相比,去噪效果较好,可以应用于类似隧道爆破信号的去噪处理中。Abstract: Aiming at the measured vibration signals collected during tunnel blasting construction, a noise reduction method based on the overall average empirical mode decomposition method (CEEMDAN decomposition) combined with wavelet packet analysis was in troduced. First, a series of multiple intrinsic modal components were obtained by CEEMDAN decomposition, and the modal components containing noise were selected using correlation coefficients, checked by the spectrogram and the variance contribution rate of the modal components. Then, the wavelet packet threshold noise reduction method was used to process the modal components containing noise. Finally, the unprocessed modal components and the de-noised components were reconstructed to obtain the final pure blasting vibration signal. At the same time, the feasibility of this noise reduction method has been verified by wavelet packet energy spectrum analysis. This method combines the advantages of CEEMDAN decomposition and wavelet packet analysis. Compared with existing methods, the de-noising effect is better, and it can be applied to the de-noising processing of similar tunnel blasting signals.
-
高性能脆性材料,无论从基础研究还是从应用研究的角度,一直被力学家、材料学家和工程师们所重点关注。以现代陶瓷材料为例,具有硬度高、强度高、密度低、模量大等诸多优点,以它为核心元件的复合防护结构在国防军事防护领域具有广泛的应用。深入了解陶瓷材料的动态破坏特性,对陶瓷材料及相关防护结构的设计与性能评估具有重要的工程实用价值。陶瓷材料在受到强冲击载荷时,材料内部的裂纹成核及扩展所需时间与加载时间及应力波传播时间相仿,因此材料的动态破坏常伴随多裂纹的生成。陶瓷材料的强度、碎片尺度及其统计特征均是多裂纹破坏机理的外在表现,而上述外在表象的实验研究对深入了解脆性破坏机理尤为重要。
目前,有关陶瓷材料的动态强度实验大都是针对压缩载荷[1-3],而由于碎裂现象大部分与材料局部的瞬时拉伸作用相关[4],故而强动载荷下的陶瓷材料拉伸强度实验尤其重要。脆性材料的冲击拉伸实验技术一直具有相当的挑战性。以Hopkinson拉杆为代表的传统拉伸试件的轴向对正非常困难,而三点或四点弯曲实验、巴西劈裂实验等试件内部的应力状态又过于复杂[5-6],并且上述加载方式均不可避免应力波在加载阶段对试件的作用,难以实现试件的拉伸碎裂。膨胀环实验是目前固体冲击拉伸碎裂的重要加载手段,膨胀圆环在均匀拉伸载荷作用下可发生多点碎裂。但目前国内外尚未有成熟的脆性膨胀环实验技术,最常用的爆炸膨胀环实验技术[7-8]和电磁膨胀环技术[9-10]均主要用于金属膨胀环的动态拉伸,并不适用于脆性膨胀环。为此,王永刚等[11]发明一种基于分离式Hopkinson压杆(SHPB)的氧化铝陶瓷圆环动态拉伸碎裂实验装置,该加载装置采用锥杆来挤压三瓣式刚性驱动环从而使其分离,通过驱动环给氧化铝陶瓷圆环施加径向载荷,一旦试件开始膨胀,试件圆环和驱动环曲率即不匹配,将导致部分陶瓷碎片由于弯曲载荷而断裂。郑宇轩等[12]和张佳等[13]提出了一种液压冲击膨胀环实验技术,实验采用水作为传压介质,利用其体积近似不可压特性,通过液压缸内截面积的大比例缩小,使得圆环试件获得较大的膨胀速度。该加载方法已经在韧性金属和粘弹性材料中得到较好应用[14-15],但是对陶瓷类高脆性材料,其加载和测试技术尚不成熟。
给定脆性材料的力学性能和外载条件,其碎片平均尺寸和特征分布一直是众多研究者们所重点关注的问题。Grady等[16]早期应用能量分析方法得到了脆性碎裂过程中的平均碎片长度,Glenn等[17]考虑了断裂时刻每单位体积储存的弹性应变能对脆性碎裂的影响,对Grady等[16]的预测公式进行了修正。能量平衡模型的物理图像清晰简单,预测公式曾一度被用于估计脆性碎片尺度。而Miller等[18]、Shenoy等[19]、Zhou等[20-22]和熊迅等[23]大量的理论与数值模拟结果表明:Grady等[16]提出的简单能量平衡模型可能极大的高估了脆性碎片尺寸。但是,对上述模型的分析均缺少脆性拉伸碎裂实验数据的支持。
本文拟建立一种液压冲击脆性膨胀环实验技术,对加载装置中的试件进行精准定位安装,对碎片进行有效回收,采用无基底半导体应变片直接测量获取膨胀环的拉伸应变信号;应用该技术对典型脆性材料SiC陶瓷在同一气压加载下进行动态拉伸碎裂实验,分析SiC在冲击载荷下的拉伸断裂强度、平均碎片尺寸及其特征分布。
1. 脆性膨胀环实验
1.1 实验原理及加载装置
图1(a)为液压冲击膨胀环实验装置的示意图,将实验装置作为结构试件,置于
∅ 74 mm的SHPB (split Hopkinson pressure bar)的入射杆与透射杆之间。利用撞击杆撞击入射杆从而冲击活塞,加载过程为力载荷加载,采用水作为传压液体,通过装置的活塞口和膨胀环内侧截面积的大比例缩小,将较低的轴向撞击速度转化为试件的高速径向膨胀,从而驱动膨胀环动态拉伸碎裂,文献[13]详细介绍实验装置的结构和部件。液压冲击脆性膨胀装置采用膨胀环与装置上下接触式固定试件,安装试件时,中空万向节旋转下压过程极易造成试件水平位置的微小移动。对于韧性金属,由于其拉伸断裂应变通常都是10−1量级,整个膨胀拉伸过程较长,略微的偏心膨胀对韧性碎裂来说可以忽略不计。而陶瓷材料的拉伸断裂应变只有10−4量级,偏心膨胀对脆性碎裂结果影响较大。
如图1(b)所示,设计了一个可自由升降的凸台。凸台外径配合一个可上下移动的尖齿环,采用螺纹啮合的方式旋转套在凸台外侧。尖齿环逆时针旋转会凸起4个尖角,尖角略高于台面(可调),尖角内侧直径略大于膨胀环外侧直径(约超出100 μm),用于固定膨胀环,防止其在安装过程中发生水平移动。当膨胀环安装完毕,即装置上下面均和试件接触,由于试件的外径和凸台的外径一致,故此时试件和出水口完全对心。由于整个加载装置内部为封闭环境,绑在尖齿环上的细铜线可通过预留的VISAR (velocity interferometer system for any reflector)测量孔而置于装置外部,通过细铜线拉动尖齿环顺时针旋转,即可使得尖齿顶点低于凸台台面,以保证实验过程中尖齿不会阻碍膨胀环的膨胀拉伸。
陶瓷材料在拉伸碎裂后仍具有较高的自由飞行速度,若直接撞击在实验装置的金属保护罩上极易造成二次破坏,从而导致其碎片尺寸和分布规律失真。通过碎片的双重软回收设计,即软回收装置内层为高黏性的不溶水油泥,能有效阻碍碎片飞行,外层为高弹性的硅胶,能避免碎片和装置的刚性碰撞,从而尽可能地保证膨胀环碎片不经受二次撞击。
1.2 实验测试技术
传统的膨胀环实验测试技术通常采用VISAR激光干涉仪来实时获得圆环的径向速度,进而间接获得材料的各种力学量,并通过高速摄影获得圆环试件的膨胀碎裂过程。但由于陶瓷材料的断裂应变极小,目前高速摄影很难捕捉清晰的脆性拉伸碎裂过程,并且陶瓷圆环在加载阶段即会发生破坏,碎裂产生的碎片仍将被水驱动而继续飞行,因此陶瓷圆环的断裂时刻将难以确定,各种力学特性也很难辨识。虽然对石英玻璃圆环的数值模拟显示[22],脆性膨胀环拉伸碎裂过程中,其外表面粒子径向速度会出现显著抖动,该时刻即为脆性试件裂纹产生时刻,但是在实验过程中,由于VISAR激光干涉仪的精度限制,此现象难以被观察到。
由于陶瓷极小的断裂应变,故此在陶瓷圆环试件上粘贴半导体应变片,用以测量陶瓷圆环拉伸碎裂过程中的应变信息。而常见的半导体应变片的基底尺寸相对圆环试件(圆环高度仅有1.5 mm)过大,无法有效地粘贴在试件外侧,因此需要去除基底,采用无基底的半导体应变片。粘贴应变片位置的圆环断裂将导致应变片不能测得有效的应变信号,在圆环试件多处粘贴半导体应变片,同时测量陶瓷圆环的断裂信息,能提高应变片获取圆环拉伸应变的成功率。
2. SiC陶瓷动态拉伸碎裂过程
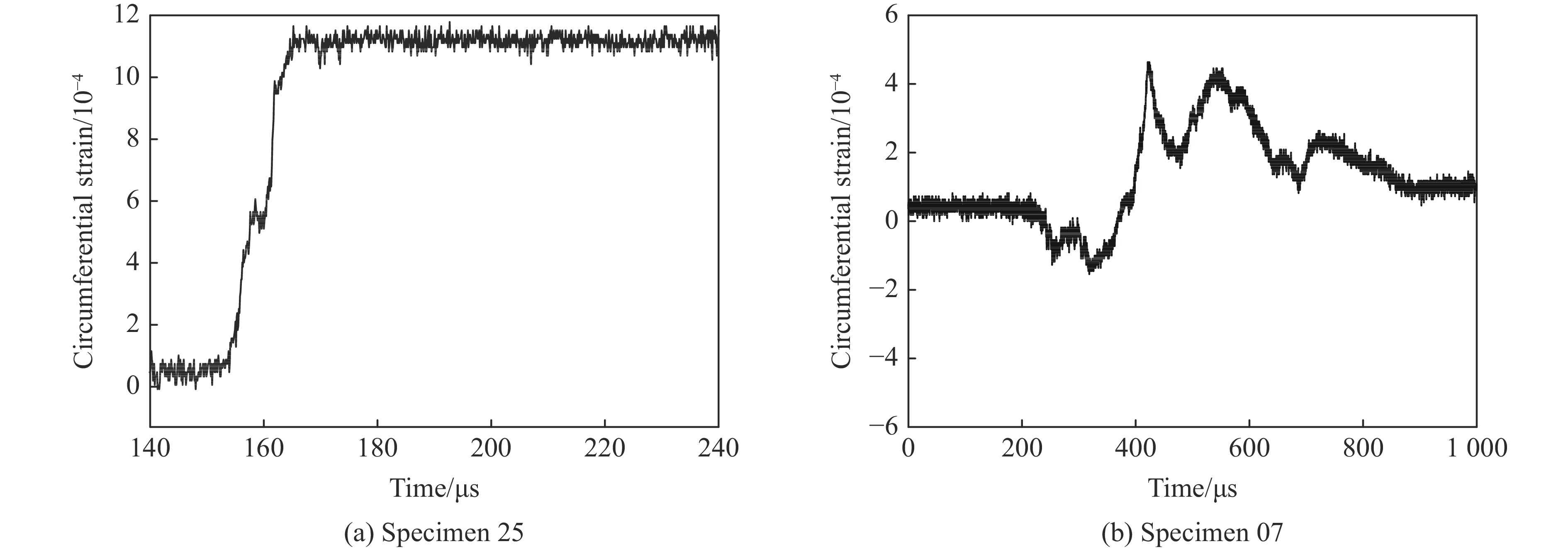
采用SiC陶瓷圆环作为典型的脆性膨胀环实验对象,SiC陶瓷圆环由纯度99.5%的碳化硅陶瓷粉末烧结的圆柱体切割而成。SiC陶瓷圆环设计几何尺寸为外径40 mm、内径37 mm、厚度1.5 mm,单个SiC陶瓷圆环平均质量约为0.8 g。SHPB撞击杆的发射气压为0.2 MPa,对同批次SiC陶瓷进行37次重复实验,但由于SiC陶瓷圆环碎裂严重,半导体应变片所在的陶瓷圆环的位置极易发生断裂,硅条的断裂导致大多数试件均无法采集到完整、有效的拉伸信号,如图2(a)所示;同时,由于液压加载的复杂性和脆性膨胀环断裂的随机性,半导体应变片偶尔会采集到无法合理阐述的应变信号,如图2(b)所示:半导体应变片首先测得压缩信号,同时采集到异常的低加载应变率信号。
类似图2中测得的周向应变,均认为是无效拉伸应变信号。剔除上述实验结果后,半导体应变片能采集到有效拉伸应变信号的概率极低(仅为7/37)。图3给出了SiC陶瓷圆环试件在冲击载荷作用下4组典型的周向应变时程曲线,在冲击拉伸过程前期,SiC陶瓷圆环周向拉伸应变单调增长,一旦圆环试件断裂,周向拉伸应变迅速下降,主要原因为断口处产生的卸载波迅速将周围的拉伸应力卸载。后期应力波在碎片中来回传播,并且还将和其他裂纹处产生的卸载波叠加,形成复杂的类周期性的弹性波振荡。将图3的应变信号的显著上升段(红色段)进行线性拟合,其斜率即为圆环拉伸断裂过程的平均应变率,分别为23.6、82.2、39.9、23.6 s−1。相应地,圆环发生断裂(碎裂)时刻的局部应变为3.8×10−4、3.8×10−4、5.6×10−4、3.7×10−4,曲线中蓝色圆圈处即为断裂应变。因为SiC陶瓷圆环中存在的固有初始缺陷,冲击拉伸过程中裂纹随机产生,半导体应变片采集的信号事实上由试件中的拉伸应力和裂纹卸载相互作用的共同结果。
虽然撞击杆的发射气压一致,但由于SiC陶瓷圆环性能分散性较大,并且驱动液体的压力、试件与装置的贴合度等均对实际加载应变率和材料断裂应变造成一定的影响,故而实验结果存在一定的离散性。整体而言,单个SiC陶瓷圆环的回收碎片数量分布在15~30个范围内,SiC陶瓷圆环的拉伸断裂应变分布在3.7×10−4~7.4×10−4范围内,平均拉伸断裂应力为206 MPa,SiC陶瓷圆环临断裂时刻的加载应变率分布在20~90 s−1范围内。
图4给出了试件11、22、28、33的SiC陶瓷圆环回收碎片复原图,碎片质量回收率为97%以上,对应的碎片分别为20、24、20、16块,其碎裂过程产生的周向应变时程曲线对应图3。结果表明,大部分试件碎片的长度都在3 mm以上,由于SiC陶瓷为高脆性材料,冲击拉伸碎裂过程中产生的碎片较多,存在个别圆环碎片的周向长度小于横向尺寸。并且在圆环断裂后高压液体会从裂纹处高速飞溅,相互作用下将剥落下圆环断口处些许微小碎片,碎片的边角由于复杂的应力波相互作用也可能进一步破坏形成了更小的碎片。剥落的微小碎片尺寸基本为1 mm以下,呈三角薄片状,只有少数尺寸较大的剥落碎片能在后期被回收,其他部分镶嵌在软回收装置中,由于尺寸过小无法有效回收。
3. SiC陶瓷圆环拉伸碎裂产生的碎片平均尺寸
将Grady能量平衡分析[16]用于一维脆性拉伸碎裂,碎片平均长度的表达式为:
SGrady=(24Gcρ˙ε2)1/3 (1) 式中:Gc为试件的断裂能,
ρ 为试件的密度,˙ε 为试件的临断裂时刻的加载应变率。Glenn等[17]考虑了脆性材料在断裂时刻单位体积储存的弹性应变能,计及这项能量,对式(1)进行修正,可推导出碎片的平均尺度的表达式:
SG&C=4√α3sinh(ϕ3)(ϕ=arsinh[β(3α)3/2],α=3σc2ρE˙ε2,β=32Gcρ˙ε2) (2) 式中:
σc 为试件的拉伸强度,E为试件的弹性模量。Zhou等[21]采用一维特征线方法对大量脆性材料进行了不同应变率下的数值实验,获得了各种材料的无量纲平均尺度
ˉS 与无量纲应变率ˉ˙ε 的数据点,并发现所有数据点汇于一条曲线,其表达式为:ˉS=4.5/(1+6.0ˉ˙ε1/3) (3) 其中碎片的无量纲尺寸
ˉS=S/S0 只依赖于无量纲应变率ˉ˙ε=˙ε/˙ε0 。而特征碎片尺度S0 和特征应变率˙ε0 完全由材料力学参数所确定,具体表达式为:S0=EGcσ2c,˙ε0=cσ3c/(E2Gc) (4) 式中:c为试件的弹性波速。
本文圆环使用的是文献[24]中的SiC陶瓷,其材料的力学参数为:弹性模量E=420 GPa,密度
ρ =3.15 kg/m3,弹性波速c=11 540 m/s,断裂能Gc=22.9 N/m,拉伸强度σc =Eεc ,εc 可根据实验结果获得。通过式(4)可得陶瓷材料的特征碎片长度S0= 0.34 mm,特征应变率˙ε0= 1.35×104 s−1。SiC陶瓷碎片平均尺寸Save=πd(1+εc)/N ,其中:d=38.5 mm为圆环试件中轴直径,εc 为圆环试件的断裂应变,N为碎片个数。将本文低压(0.2 MPa左右)加载下的SiC陶瓷圆环膨胀过程中产生的碎片平均尺寸与应变率无量纲化,并与现有各种碎裂模型和脆性碎裂模拟数据进行比较[15-19, 21, 23, 25-26],如图5所示。加载应变率越低,SiC陶瓷碎片的无量纲化平均尺寸越大。该平均碎片尺寸基本落于多种脆性碎裂预测模型的区间内,但是还是显著高于式(2)和(3)的结果。造成此偏差的主要原因为:(1) 式(3)得到的脆性碎片平均尺度公式是基于一维特征线理论求得,虽然膨胀环实验可以近似认为是准一维拉伸实验,但膨胀环断口处的断裂仍是三维应力状态,碎片内部的大量微裂纹无法实现圆环的断裂;(2) SiC陶瓷的高脆性导致碎片相对韧性材料呈量级式增加,低应变率下仍产生大量碎片,导致部分碎片的轴向尺寸甚至小于其横向尺寸,脆性碎裂偏离了膨胀环的准一维断裂;(3) 脆性碎裂实验无法像韧性碎裂实验一样将碎裂过程产生的碎片完全回收,而无法回收的碎片通常都是尺寸微小的碎片,3%~7%质量的碎片丢失率也会低估碎裂过程中产生的碎片数量,从而使得碎片平均尺寸偏大。
4. SiC陶瓷圆环碎片分布规律
4.1 碎片尺寸分布函数
近似认为37组实验给定的外部载荷是一致的,从而对碎裂过程中产生的碎片尺寸进行分布统计分析,且对碎片分布规律的讨论不依赖于半导体应变片测量的应变信息。由于部分实验组碎片回收率较低,故此选取碎片回收率在93%以上的28组实验产生的碎片进行统计分析,绘出相同加载条件下碎片尺寸分布图,如图6(a)所示。如果不考虑尺寸1 mm以下的剥落的微小碎片颗粒,对明显的拉伸碎片进行统计分析,可以得到相应的碎片尺寸分布,如图6(b)所示。
无论是否考虑剥落的微小碎片,碎片的尺寸分布均可以用一个三参数Weibull分布来描述。本文沿用描述脆性碎片分布的Weibull累积分布函数[24]:
N(S)=N0exp[−(S−SminS0)n](S>Smin) (5) 式中:N(S)为碎片尺寸大于S的个数,N0为碎片总数,Smin为最小碎片尺寸,S0和n为Weibull参数。Weibull分布的概率密度函数为:
n(S)=(2(S−Smin)S20)exp[−(S−SminS0)n](S>Smin) (6) 对碎片分布进行拟合发现,近似采用n=2时的Weibull概率密度函数,即Rayleigh概率密度函数即能较好地描述SiC陶瓷碎片的分布规律。
4.2 碎片尺寸归一化分布规律
采用28组实验产生的碎片平均尺寸Save将每个碎片尺寸归一化处理,如果考虑全部的碎片颗粒,可得
ˉSmin=Smin/Save=0.002,¯S0=S0/Save=1.126 ;如果不考虑1 mm以下的微小剥落碎片,可得ˉSmin=Smin/Save=0.135,¯S0=S0/Save=0.976 。图7给出了20~90 s−1应变率范围内的碎片的尺寸的归一化分布曲线N(S)/N0 与归一化尺寸S/Save 之间的关系,从图中可见Rayleigh分布能较好地描述陶瓷碎片尺寸的归一化数据,但是在细小尺寸和大尺寸两端的区域内,实验结果和Rayleigh分布还存在一定差异。在大尺寸碎片方面,由于陶瓷类脆性材料结构内部天然存在大量初始缺陷,较大的初始缺陷容易导致碎片尺寸过大,而偏离统计规律;在小尺寸碎裂方面,相对于韧性材料,脆性碎裂过程中产生的小碎片数量远大于韧性金属,一般而言韧性金属的无量纲化最小碎片尺寸在0.3~0.4之间[27],而脆性材料的无量纲化最小碎片尺寸远小于该数值,该统计还是在未完全回收碎片颗粒的情况下,如果计及3%~7%的未回收到的更细小碎片,该数值会进一步变小。由此可见,脆性碎裂过程和韧性碎裂过程在产生碎片的方式和数量上有本质的区别,还需进一步的深入研究。5. 结 论
(1)在液压膨胀环实验装置的基础上,进一步优化了加载装置和测试手段,设计了升降凸台定位膨胀环试件从而避免了圆环的偏心膨胀,较好地实现了脆性材料的冲击拉伸碎裂。利用无基底的半导体应变片有效测量了陶瓷类脆性材料的拉伸应变,从而获得试件的加载应变率和断裂应变。
(2)当临断裂时刻的加载应变率为20~90 s−1时,单个SiC陶瓷圆环的回收碎片数量分布在15~30个碎片范围内,SiC陶瓷圆环的拉伸断裂应变为3.7×10−4~7.4×10−4,平均拉伸断裂应力为206 MPa。无量纲化的SiC陶瓷平均碎片尺寸基本落于多种脆性碎裂预测模型的区间内,但是和目前的碎裂模型和一维脆性碎裂数值模拟结果还存在一定差异。碎裂产生的碎片尺寸分布基本符合Rayleigh分布,但是在细小尺寸上和大尺寸碎片分布上具有较大偏差,主要由于陶瓷材料的脆性碎裂模式和内在初始缺陷所决定的。
(3)陶瓷类脆性材料的膨胀环试件尺寸较小,断裂应变极低,实验加载和测试难度均很大。如何避免碎片断口处二次甚至多次产生细小碎片,如何有效得观测脆性碎裂过程,如何更高效地获得韧性圆环在膨胀过程中的粒子速度、应变信号等物理力学参量,是接下来脆性膨胀环实验需要进一步研究的。
-
图 5 测点布置[21]
Figure 5. Layout of measuring points
表 1 本征模态分量(IMF)的相关系数
Table 1. Correlation coefficients of modal components (IMF)
模态分量 C1 C2 C3 C4 C5 C6 C7 C8 C9 C10 C11 C12 C13 C14 ri 0.129 0.103 0.097 0.063 0.051 0.687 0.760 0.562 0.260 0.139 0.023 0.003 0.007 0.001 表 2 模态分量(IMF)的方差贡献率
Table 2. Variance contribution rate of modal component (IMF)
方差贡献率 C1 C2 C3 C4 C5 C6 C7 C8 C9 C10 C11 C12 C13 C14 e(j) 1.65 0.07 0.41 0.29 0.23 13.42 38.31 34.98 8.71 1.59 0.28 0.24 0.04 0.01 表 3 去噪效果对比
Table 3. Comparison of noise reduction effects
去噪方法 η σ 小波包阈值去噪 66.412 1.40×10−4 EMD-小波包联合去噪 84.9511 2.55×10−5 EEMD-小波包联合去噪 84.0313 2.43×10−5 新方法去噪 94.0802 2.40×10−5 -
[1] 张乐文, 王洪波, 邱道宏, 等. 小波降噪与粒子群优化综合回归爆破震动参数 [J]. 岩土力学, 2014, 35(S2): 338–341. DOI: 10.16285/j.rsm.2014.s2.037.ZHANG L W, WANG H B, QIU D H, et al. Blasting vibration parameters using comprehensive regression of wavelet denoising and particle swarm optimization algorithm [J]. Rock and Soil Mechanics, 2014, 35(S2): 338–341. DOI: 10.16285/j.rsm.2014.s2.037. [2] 王翔, 葛晓霞. 基于小波变换的汽轮机振动信号软阀值消噪技术研究 [J]. 汽轮机技术, 2009, 51(3): 204–206, 228. DOI: 10.3969/j.issn.1001-5884.2009.03.014.WANG X, GE X X. A study on signal thresholding de-noising technique based on the wavelet transform [J]. Turbine Technology, 2009, 51(3): 204–206, 228. DOI: 10.3969/j.issn.1001-5884.2009.03.014. [3] 杨孟, 王瑾, 周西峰, 等. 基于CEEMD和小波包的降噪方法研究 [J]. 南京邮电大学学报(自然科学版), 2018, 38(2): 41–47. DOI: 10.14132/j.cnki.1673-5439.2018.02.007.YANG M, WANG J, ZHOU X F, et al. De-noising method based on CEEMD and wavelet packet [J]. Journal of Nanjing University of Posts and Telecommunications (Natural Science Edition), 2018, 38(2): 41–47. DOI: 10.14132/j.cnki.1673-5439.2018.02.007. [4] CHEN G, LI Q Y, LI D Q, et al. Main frequency band of blast vibration signal based on wavelet packet transform [J]. Applied Mathematical Modelling, 2019, 74: 569–585. DOI: 10.1016/j.apm.2019.05.005. [5] YUAN H P, LIU X L, LIU Y, et al. Analysis of acoustic wave frequency spectrum characters of rock mass under blasting damage based on the HHT method [J]. Advances in Civil Engineering, 2018, 2018: 9207476. DOI: 10.1155/2018/9207476. [6] 邓青林, 赵国彦. 基于EEMD和小波的爆破振动信号去噪 [J]. 爆破, 2015, 32(4): 33–38. DOI: 10.3963/j.issn.1001-487X.2015.04.007.DENG Q L, ZHAO G Y. De-noising of blast vibration signal based on EEMD and wavelet [J]. Blasting, 2015, 32(4): 33–38. DOI: 10.3963/j.issn.1001-487X.2015.04.007. [7] 贾贝, 凌天龙, 侯仕军, 等. 变分模态分解在爆破信号趋势项去除中的应用 [J]. 爆炸与冲击, 2020, 40(4): 045201. DOI: 10.11883/bzycj-2019-0092.JIA B, LING T L, HOU S J, et al. Application of variable mode decomposition in the removal of blasting signal trend items [J]. Explosion and Shock Waves, 2020, 40(4): 045201. DOI: 10.11883/bzycj-2019-0092. [8] 费鸿禄, 刘梦, 曲广建, 等. 基于集合经验模态分解-小波阈值方法的爆破振动信号降噪方法 [J]. 爆炸与冲击, 2018, 38(1): 112–118. DOI: 10.11883/bzycj-2016-0148.FEI H L, LIU M, QU G J, et al. A method for blasting vibration signal denoising based on ensemble empirical mode decomposition-wavelet threshold [J]. Explosion and Shock Waves, 2018, 38(1): 112–118. DOI: 10.11883/bzycj-2016-0148. [9] 陈仁祥, 汤宝平, 马婧华. 基于EEMD的振动信号自适应降噪方法 [J]. 振动与冲击, 2012, 31(15): 82–86. DOI: 10.13465/j.cnki.jvs.2012.15.015.CHEN R X, TANG B P, MA J H. Adaptive de-noising method based on ensemble empirical mode decomposition for vibration signal [J]. Journal of Vibration and Shock, 2012, 31(15): 82–86. DOI: 10.13465/j.cnki.jvs.2012.15.015. [10] 单仁亮, 白瑶, 宋永威, 等. 冻结立井模型爆破振动信号的小波包分析 [J]. 煤炭学报, 2016, 41(8): 1923–1932. DOI: 10.13225/j.cnki.jccs.2015.1526.SHAN R L, BAI Y, SONG Y W, et al. Wavelet packet analysis of blast vibration signals of freezing shaft model [J]. Journal of China Coal Society, 2016, 41(8): 1923–1932. DOI: 10.13225/j.cnki.jccs.2015.1526. [11] 单仁亮, 宋永威, 白瑶, 等. 基于小波包变换的爆破信号能量衰减特征研究 [J]. 矿业科学学报, 2018, 3(2): 119–128. DOI: 10.19606/j.cnki.jmst.2018.02.003.SHAN R L, SONG Y W, BAI Y, et al. Research on the energy attenuation characteristics of blasting vibration signals based on wavelet packet Transformation [J]. Journal of Mining Science and Technology, 2018, 3(2): 119–128. DOI: 10.19606/j.cnki.jmst.2018.02.003. [12] 邹德臣, 王海亮, 王春慧, 等. 基于HHT分析的浅埋隧道爆破振动控制研究 [J]. 隧道建设, 2014, 34(8): 760–764. DOI: 10.3973/j.issn.1672-741X.2014.08.009.ZHOU D C, WANG H L, WANG C H, et al. Study on blasting vibration control of shallow tunnel based on HHT analysis [J]. Tunnel Construction, 2014, 34(8): 760–764. DOI: 10.3973/j.issn.1672-741X.2014.08.009. [13] 叶红宇, 卓越, 杨小林. 隧道爆破振动信号EEMD分解后小波包降噪法研究 [J]. 铁道建筑, 2018, 58(7): 83–86. DOI: 10.3969/j.issn.1003-1995.2018.07.21.YE H Y, ZHUO Y, YANG X L. Research on wavelet packet denoising of tunnel blasting vibration signals after EEMD decomposition [J]. Railway Engineering, 2018, 58(7): 83–86. DOI: 10.3969/j.issn.1003-1995.2018.07.21. [14] 刘霞, 宋启航. CEEMDAN自适应阈值去噪算法在地震方向的应用 [J]. 重庆大学学报, 2019, 42(7): 95–104. DOI: 10.11835/j.issn.1000-582X.2019.07.011.LIU X, SONG Q H. CEEMDAN adaptive threshold denoising algorithm in application to seismic direction [J]. Journal of Chongqing University, 2019, 42(7): 95–104. DOI: 10.11835/j.issn.1000-582X.2019.07.011. [15] HASSAN A R, SUBASI A, ZHANG Y C. Epilepsy seizure detection using complete ensemble empirical mode decomposition with adaptive noise [J]. Knowledge-Based Systems, 2020, 191: 105333. DOI: 10.1016/j.knosys.2019.105333. [16] HE C B, NIU P, YANG R, et al. Incipient rolling element bearing weak fault feature extraction based on adaptive second-order stochastic resonance incorporated by mode decomposition [J]. Measurement, 2019, 145: 687–701. DOI: 10.1016/j.measurement.2019.05.052. [17] LI L, WANG F, SHANG F, et al. Energy spectrum analysis of blast waves based on an improved Hilbert–Huang transform [J]. Shock Waves, 2017, 27(3): 487–494. DOI: 10.1007/s00193-016-0667-7. [18] 王明同. 基于小波包分析法的电压暂降检测研究与LabVIEW实现[D]. 广州: 华南理工大学, 2013. [19] 李洪涛. 基于能量原理的爆破地震效应研究[D]. 武汉: 武汉大学, 2007. [20] 王海龙, 赵岩, 王永佳, 等. 草帽山隧道爆破振动监测与分析 [J]. 铁道建筑, 2017, 57(12): 67–70. DOI: 10.3969/j.issn.1003-1995.2017.12.18.WANG H L, ZHAO Y, WANG Y J, et al. Blasting vibration monitoring and analysis of caomaoshan tunnel [J]. Railway Engineering, 2017, 57(12): 67–70. DOI: 10.3969/j.issn.1003-1995.2017.12.18. [21] 王海龙, 赵岩, 王永佳, 等. 新建京张高铁立体交叉隧道爆破振动控制研究 [J]. 铁道标准设计, 2018, 62(7): 130–134. DOI: 10.13238/j.issn.1004-2954.201710120003.WANG H L, ZHAO Y, WANG Y J, et al. Study on blasting vibration control of three-dimensional cross tunnel on Beijing to Zhangjiakou high-speed railway [J]. Railway Standard Design, 2018, 62(7): 130–134. DOI: 10.13238/j.issn.1004-2954.201710120003. [22] 黄金, 吴庆良, 陈钒. 基于CEEMDAN-WPT联合去噪的灾后求救信号能量分布特征研究 [J]. 南京理工大学学报, 2020, 44(2): 194–201. DOI: 10.14177/j.cnki.32-1397n.2020.44.02.010.HUANG J, WU Q L, CHEN F. Study on energy distribution character about post-disaster rescue signal based on CEEMDAN-WPT denoising [J]. Journal of Nanjing University of Science and Technology, 2020, 44(2): 194–201. DOI: 10.14177/j.cnki.32-1397n.2020.44.02.010. [23] 李火坤, 刘世立, 魏博文, 等. 基于方差贡献率的泄流结构多测点动态响应融合方法研究 [J]. 振动与冲击, 2015, 34(19): 181–191. DOI: 10.13465/j.cnki.Jvs.2015.19.029.LI H K, LIU S L, WEI B W, et al. Multi-point dynamic response data fusion method for a flood discharge structure based on variance dedication rate [J]. Journal of Vibration and Shock, 2015, 34(19): 181–191. DOI: 10.13465/j.cnki.Jvs.2015.19.029. [24] SIMAR L, ZELENYUK V. Improving finite sample approximation by central limit theorems for estimates from Data Envelopment Analysis [J]. European Journal of Operational Research, 2020, 284(3): 1002–1015. DOI: 10.1016/j.ejor.2020.01.036. [25] 谢德红, 李俊锋, 刘菂, 等. 基于改进Hodrick-Prescott分解模型的近红外自适应降噪方法 [J]. 光谱学与光谱分析, 2020, 40(5): 1650–1655. DOI: 10.3964/j.issn.1000-0593(2020)05-1650-06.XIE D H, LI J F, LIU D, et al. An Improved Hodrick-Prescott decomposition based near-infrared adaptive denoising method [J]. Spectroscopy and Spectral Analysis, 2020, 40(5): 1650–1655. DOI: 10.3964/j.issn.1000-0593(2020)05-1650-06. [26] 李宗春, 邓勇, 张冠宇, 等. 变形测量异常数据处理中小波变换最佳级数的确定 [J]. 武汉大学学报 (信息科学版), 2011, 36(3): 285–288. DOI: 10.13203/j.whugis2011.03.006.LI Z C, DEND Y, ZHANG G Y, et al. Determination of best grading of wavelet transform in deformation measurement data filtering [J]. Geomatics and Information Science of Wuhan University, 2011, 36(3): 285–288. DOI: 10.13203/j.whugis2011.03.006. [27] HUANG D, CUI S, LI X Q. Wavelet packet analysis of blasting vibration signal of mountain tunnel [J]. Soil Dynamics and Earthquake Engineering, 2019, 117: 72–80. DOI: 10.1016/j.soildyn.2018.11.025. 期刊类型引用(71)
1. 闫鹏,张云鹏,周倩倩,杨曦. 基于改进EMD的爆破振动信号降噪方法研究. 振动与冲击. 2025(01): 212-220 .  百度学术
百度学术2. 张险峰,高亚州,雒德宏,马璐,傅广泽. 海上风电机组塔架微弱振动信号自动化检测. 自动化与仪器仪表. 2025(01): 35-38 .  百度学术
百度学术3. 薛威,王永东,柏文军,胡志豪,任雨桐,史林猛. 基于VMD-ICA算法的隧道爆破振动信号去噪方法. 工程爆破. 2025(01): 156-166 .  百度学术
百度学术4. 苏有斌. 基于改进HHT算法的重载铁路信号自动化解调方法设计. 自动化与仪表. 2024(01): 125-129 .  百度学术
百度学术5. 张孟昕,陈波,刘伟琪,漆一宁,张明. SSA-XGBoost与时空特征选取的大坝变形预测模型. 水力发电学报. 2024(01): 84-98 .  百度学术
百度学术6. 童晓玲,曾斐宇,江轲,梁磊. 基于CEEMD-MPE-IMPROVED WTD的爆炸振动信号噪声抑制方法. 振动与冲击. 2024(01): 184-192 .  百度学术
百度学术7. 王子豪,王硕,关博凯,鲍晓华. CEEMDAN与改进形态差值滤波结合的滚动轴承故障诊断. 微特电机. 2024(01): 20-25+30 .  百度学术
百度学术8. 廖铖,蔡德所,李苗,林金城. 基于光纤陀螺的堆石坝内部沉降分布式监测技术研究. 土木工程学报. 2024(02): 77-86 .  百度学术
百度学术9. 上官小雨,汤雅莉. 用于视觉传达效果优化的退化图像复原仿真. 计算机仿真. 2024(01): 222-226 .  百度学术
百度学术10. 赵智强,帕孜来·马合木提,李高原. 逆变器的电容老化故障诊断方法研究. 现代电力. 2024(01): 182-190 .  百度学术
百度学术11. 夏焰坤,李欣洋,任俊杰,寇坚强. 基于CEEMDAN-HT的永磁同步电机匝间短路振动信号故障特征提取研究. 振动与冲击. 2024(05): 72-81 .  百度学术
百度学术12. 严志豪,高文学,汪艮忠,胡宇,张声辉,张小军. 连拱隧道中导洞不同起爆位置振动效应研究. 工程爆破. 2024(01): 141-148 .  百度学术
百度学术13. 钱晓仲. 基于小波包分析的低压配电系统接地故障诊断. 电工技术. 2024(03): 42-44+48 .  百度学术
百度学术14. 杨孟,李永福,梁云,陈艺征,顾冲时. 基于多层次滤波降噪的IGA-NARX混凝土坝变形预测模型. 水电能源科学. 2024(04): 142-146 .  百度学术
百度学术15. 李大柱,梁树林,池茂儒,许文天. 基于形态学滤波和CEEMDAN-WVD的车轮失圆诊断. 铁道机车车辆. 2024(02): 8-14 .  百度学术
百度学术16. 伍福寿,张学民,韩淼,陈进,胡涛,周贤舜,王树英,朱凯. 近接既有隧道爆破激发地震波成分构成及特性研究. 中南大学学报(自然科学版). 2024(04): 1406-1417 .  百度学术
百度学术17. 辛志军. 大断面通风斜井爆破施工对邻近隧道振动特性的影响. 施工技术(中英文). 2024(09): 47-52+83 .  百度学术
百度学术18. 曲春刚,朱胜翔,冯正兴. CEEMDAN-SE-WT降噪方法在航空发动机燃油流量信号中的应用. 科学技术与工程. 2024(15): 6525-6533 .  百度学术
百度学术19. 闫鹏,张云鹏,侯善营,张为为,杨曦. 基于改进EMD-小波包的爆破振动信号降噪方法研究. 振动与冲击. 2024(11): 264-271+287 .  百度学术
百度学术20. 田婕,张云鹏,闫鹏,孙文诚,杨曦. 基于CEEMD的露天深孔爆破振动信号降噪光滑模型. 爆破. 2024(02): 143-150+159 .  百度学术
百度学术21. 薛睿,高文学,梅敏,张小军,张军灵. 基于EEMD-HHT的边坡爆破振动信号分析. 市政技术. 2024(07): 101-108 .  百度学术
百度学术22. 唐斌,池茂儒,赵明花,李大柱,许文天. 基于ICEEMDAN和共振解调的轴承故障检测方法. 铁道机车车辆. 2024(04): 84-91 .  百度学术
百度学术23. 刘金山. 基于改进EMD的爆破振动信号降噪方法. 工程爆破. 2024(04): 156-164 .  百度学术
百度学术24. 石佳晨,岳春芳,朱明远,皮李浪. 基于CEEMDAN-改进小波阈值的大坝变形数据处理方法. 水利水电科技进展. 2024(05): 80-86+102 .  百度学术
百度学术25. 谷宇,苏怀智,张帅,姚可夫,刘明凯,漆一宁. 基于自适应时序分解筛选的大坝变形预测模型. 水利学报. 2024(09): 1045-1057+1070 .  百度学术
百度学术26. 李士林,朱旋,周冬冬. ICEEMDAN-小波阈值法在谐波信号中的应用. 河北电力技术. 2024(05): 20-26+33 .  百度学术
百度学术27. 望远福,李云赫,赵岩,范杰林. 隧道爆破振动中掏槽段的波形预测方法. 中国测试. 2024(10): 150-156 .  百度学术
百度学术28. 杨德志,赵岩,王海龙. 铁路隧道初期支护混凝土爆破累积损伤特征. 混凝土. 2024(10): 40-45 .  百度学术
百度学术29. 真嘉捷,赖丰文,黄明,李爽,许凯. 基于LightGBM-Informer的盾构隧道管片上浮长时间序列预测模型. 岩土力学. 2024(12): 3791-3801 .  百度学术
百度学术30. 尚雪义,陈勇,陈结,陈林林,蒲源源. 基于Adaboost_LSTM预测的矿山微震信号降噪方法及应用. 煤炭学报. 2024(11): 4406-4416 .  百度学术
百度学术31. 崔芷宁,于晓光,孙杰,于喜金,冉子晴,张小龙. 基于CEEMD航空液压管路故障诊断方法研究. 机床与液压. 2023(01): 189-195 .  百度学术
百度学术32. 杨智中,林军志,汪魁,程梓益,刘攀. 基于CEEMDAN-小波包自适应阈值混凝土声发射信号降噪研究. 振动与冲击. 2023(03): 139-149 .  百度学术
百度学术33. 李大柱,牛江,梁树林,池茂儒. 基于多尺度时频图与卷积神经网络的车轮故障智能诊断. 铁道科学与工程学报. 2023(03): 1032-1043 .  百度学术
百度学术34. 杨森,王恒迪,崔永存,李畅,唐元超. 基于改进AFSA的参数优化VMD和ELM的轴承故障诊断. 组合机床与自动化加工技术. 2023(04): 67-70 .  百度学术
百度学术35. 宋青波,柏皓博,王海龙,赵岩,张奇. 基于CEEMDAN-交叉小波变换的爆破延时识别. 工程爆破. 2023(02): 10-16+24 .  百度学术
百度学术36. 章芳情,袁方,贺玉,王成城,郭江. 基于NLM-CEEMDAN和样本熵的水电机组振动信号去噪. 中国农村水利水电. 2023(06): 286-294 .  百度学术
百度学术37. 陆俊杰,刘柱,丁雪兴,丁俊华,高德. 局部特征尺度与小波阈值协同下密封摩擦面AE信号降噪研究. 振动与冲击. 2023(12): 205-211+219 .  百度学术
百度学术38. 廖胜军,何国建,邢健文,魏呈霖. 高温高速VSP地震信号的面波压制处理. 声学与电子工程. 2023(02): 32-35 .  百度学术
百度学术39. 宿利平,严志豪,王辉,郭明洋,张小军,高文学. 基于EEMD-HHT的隧道爆破振动信号分析. 施工技术(中英文). 2023(12): 21-26 .  百度学术
百度学术40. 闫鹏,张云鹏,田婕,王晗. 基于CEEMDAN-K-means算法的爆破振动信号去噪研究. 爆破. 2023(03): 184-190 .  百度学术
百度学术41. 孙坤,尹晓红. 基于数据去噪和CNN-BiGRU的SO_2排放预测. 电子测量技术. 2023(13): 66-72 .  百度学术
百度学术42. 司小昆,郭明,赵岩. 下穿隧道爆破振动测试及能量分布研究. 中国测试. 2023(10): 57-63 .  百度学术
百度学术43. 杨志飞,崔春生,杜桂云,刘双峰,赵海霞. 融合CEEMDAN-GMM和小波阈值方法的爆炸冲击波降噪算法. 弹箭与制导学报. 2023(05): 80-86 .  百度学术
百度学术44. 杨春杰,李聪聪,刘满仓. 一种塔吊钢丝绳损伤检测信号降噪方法. 西安邮电大学学报. 2023(04): 102-110 .  百度学术
百度学术45. 高军伟,赵岩,王奔. 立体交叉铁路隧道爆破振动效应的研究. 爆破器材. 2023(06): 39-47 .  百度学术
百度学术46. 王婷婷,李方,霍雨佳,王振豪,赵万春. 基于AO优化VMD-小波包的岩石破裂声发射信号去噪算法. 采矿与岩层控制工程学报. 2023(06): 82-95 .  百度学术
百度学术47. 赵民强. 下穿山岭隧道爆破振动效应的数值模拟分析. 科技与创新. 2023(24): 95-97 .  百度学术
百度学术48. 项荣军,刘传鹏,李胜林,凌天龙. 隧道内部爆破振动传播规律与降振技术研究. 爆破. 2023(04): 82-88+200 .  百度学术
百度学术49. 赵建恩,赵岩,王海龙. 下穿既有重载铁路隧道支护措施数值模拟研究. 河北建筑工程学院学报. 2023(04): 9-14 .  百度学术
百度学术50. 刘中飞,王海龙,霍昊,吕良玉,信博研. 铁路隧道爆破对地表振动响应研究. 河北建筑工程学院学报. 2023(04): 15-25+48 .  百度学术
百度学术51. 刘连生,钟抒亮,易文华,刘伟,杨砚,柴耀光. 基于PEMD的露天边坡不同高程爆破振动信号频谱特征研究. 金属矿山. 2022(01): 148-153 .  百度学术
百度学术52. 付晓强,俞缙,刘纪峰,杨仁树,戴良玉. 隧道爆破振动信号畸变校正与混沌多重分形特征研究. 振动与冲击. 2022(06): 76-85 .  百度学术
百度学术53. 魏立恒,倪修能,郭洪雨,孙飞,葛艺超,夏兆平,王国波. 城市越岭长隧道爆破开挖振动影响研究. 爆破. 2022(01): 159-167 .  百度学术
百度学术54. 万书亭,贾东亮,孙瑞滨,王向东,刘杰,贾伯岩. 基于相关系数特征的输电铁塔螺栓连接完全松动检测方法研究. 中国工程机械学报. 2022(02): 173-177+183 .  百度学术
百度学术55. 梁娟. 基于粒子群算法的爆破振动速度智能预测方法. 工程爆破. 2022(03): 117-121+136 .  百度学术
百度学术56. 李伟光,余秋霖,骆春林,孙菁瑶,林守金,龚德明. Savitzky Golay-WPT在滚刀主轴振动信号降噪中的应用. 机床与液压. 2022(14): 159-165 .  百度学术
百度学术57. 单仁亮,赵岩,王海龙,董捷,仝潇,李兆龙,王东升. 下穿铁路隧道爆破振动衰减规律研究. 爆炸与冲击. 2022(08): 145-159 .  本站查看
本站查看58. 孙继平,余星辰. 基于CEEMD分量样本熵与SVM分类的煤矿瓦斯和煤尘爆炸声音识别方法. 采矿与安全工程学报. 2022(05): 1061-1070 .  百度学术
百度学术59. 赵岩,王小敬,王海龙,王东升. 交叉隧道爆破振速回归分析及对比研究. 工程爆破. 2022(05): 121-127 .  百度学术
百度学术60. 周春煦,赵岩. 下穿铁路隧道爆破振动分析及控制方法研究. 中国安全生产科学技术. 2022(11): 133-140 .  百度学术
百度学术61. 刘杰,王向东,贾伯岩,贾东亮,万书亭. 基于振动特性的铁塔螺栓完全松动检测方法. 科学技术与工程. 2022(32): 14213-14220 .  百度学术
百度学术62. 付茂盛,耿建,张仰飞. 基于CEEMDAN-LOF的企业能耗异常辨识研究. 机械与电子. 2022(12): 48-52+58 .  百度学术
百度学术63. 徐雨,郑威,程怡. 基于单通道脑电信号中眼电伪迹去除的方法. 生物医学工程研究. 2022(04): 369-375+416 .  百度学术
百度学术64. 蔡俊,张翔风. CEEMDAN-小波包联合算法在ECG中的降噪应用. 黑龙江工业学院学报(综合版). 2022(12): 41-49 .  百度学术
百度学术65. 任文斌,付晓强,俞缙. 小近距隧道台阶法开挖爆破振动信号特征分析. 工程爆破. 2022(06): 119-129 .  百度学术
百度学术66. 王海龙,柏皓博,赵岩,王彬,王海军. 基于傅里叶分解-小波包分析的爆破信号去噪方法. 爆破. 2021(02): 37-44 .  百度学术
百度学术67. 王海龙,李帅,赵岩,王晟华. CEEMDAN-小波包联合降噪的优化方法. 爆破器材. 2021(04): 48-53 .  百度学术
百度学术68. 王海龙,柏皓博,王晟华. 基于FDM的爆破振动信号时频分析. 爆破. 2021(03): 143-151 .  百度学术
百度学术69. 王海龙,李云赫,赵岩. k值优化VMD-小波包分析联合降噪方法在隧道爆破信号中的应用. 爆破器材. 2021(05): 50-57 .  百度学术
百度学术70. 张佳,赵岩. 基于CEEMDAN法的隧道爆破信号趋势项去除. 爆破器材. 2021(06): 58-64 .  百度学术
百度学术71. 宋青波,张奇,单洪涛,王海龙,赵岩. 交叉隧道施工安全监测及控制技术研究. 河北建筑工程学院学报. 2021(03): 52-59 .  百度学术
百度学术其他类型引用(53)
-









 下载:
下载:















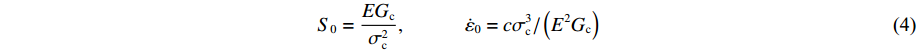






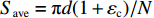











 下载:
下载:










 百度学术
百度学术