Optimization of vehicle protection components based on reliability
-
摘要: 在传统的优化流程中,不考虑设计变量的不确定性将引起设计目标的性能波动,甚至设计失败。为提高军用车辆底部防护能力,针对一种车辆防护组件进行了可靠性优化。在爆炸防护优化中引入多目标可靠性优化,通过实验设计和灵敏度分析筛选设计变量,再构建并选择准确度最高的代理模型,运用多目标遗传算法完成防护组件的可靠性优化。实验和数值模拟结果表明,优化后的防护组件满足防护性和轻量化的要求,且设计的可靠性得到了提升,可为后续防护组件设计生产提供参考。Abstract: In order to improve the bottom protection capability of military vehicles, a reliable optimization method was proposed for the design of vehicle protection components. In the traditional optimization process, if the uncertainty of design variables is not considered, the performance of design objectives will fluctuate or even fail. In this paper, multi-objective reliability optimization was introduced into the optimization of explosion protection. The surrogate model with the highest accuracy was constructed and chosen after the design variables were selected through experimental design and sensitivity analysis. The reliability optimization of the protection components was achieved by the multi-objective genetic algorithm. Eventually, through experiment and simulation verification, the optimized protective components met the requirements of protection and lightweight, and the reliability was improved, which can provide reference for the subsequent design and production of protective components.
-
在非对称局部战争的环境下,简易爆炸装置(improvised explosive device, IED)及地雷的威胁日益增大,军用车辆自身的防护及作战能力需得到更大的提升[1]。防地雷反伏击车主要通过底部防护组件来抵挡地雷和简易爆炸装置的威胁,防雷组件的厚度及质量的增加能在一定程度上提升车辆防护性能,但同时也会影响车辆的机动性。因此,在确保车辆防护能力的同时对底部防护组件进行轻量化设计是必要的。然而,在优化设计过程中,设计变量的不确定性以及爆炸工况的不确定性会影响目标性能,如防护组件的厚度变化、材料性能变化、爆炸环境下的土壤密度和炸药当量的变化等,这些设计变量的波动可能会导致最终设计失败,因此需在优化设计过程中引入可靠性优化。
对传统车辆碰撞安全性能的优化,已有了大量可靠性优化研究。吕晓江等[2]将多目标可靠性优化分析应用到一种车型正面40%重叠可变型壁障碰撞中,达到了车辆耐撞性和轻量化的要求,并提高了车身设计的可靠性。Pan等[3]通过补偿代理模型拟合误差的保守策略,在车辆正面碰撞的可靠性优化中实现了可行的最佳设计。陈崇等[4]提出一种综合量化多种不确定性的概率统计方法,并将它运用在车辆前纵梁的稳健性设计中,实现了轻量化。Gu等[5]在车辆前部结构耐撞性设计中,分别对比了确定性、可靠性和稳健性多目标优化,说明通过不确定性优化可以显著提高设计的可靠性和稳健性。在车辆爆炸防护领域,相关研究仅限于确定性优化。魏然等[6]对一种防护型车辆底部结构和乘员系统进行多参数优化,引入降维技术,不仅缩减了样本空间,而且提高了优化的精度。李明星等[7]通过径向基函数构建神经网络代理模型,并应用多目标遗传算法,对V型防护组件进行优化设计,通过实验验证了该手段的可行性。
在本文中,提出车辆底部防护结构的多目标可靠性优化流程,考虑结构尺寸的不确定性,同时引入多种材料的离散变量,再通过灵敏度分析完成设计变量的筛选,构造并选择合适的代理模型进行结构的可靠性优化,并与确定性优化结果进行对比,拟为车辆防护提供可靠的设计方案和建议。
1. 优化理论
1.1 确定性多目标优化
传统的多目标优化基本为确定性多目标优化,此类问题的数学模型通常为:
minf(x)=(f1(x),f2(x),⋯,fi(x))s.t.{gj(x)≤0xL≤x≤xHi=1,2,⋯,mj=1,2,⋯,n (1) 式中:fi(x)为设计目标,gj(x)为约束函数,m为目标的数量,n为约束的数量,xL和xH分别为设计变量x的下限和上限。
工程实践中已广泛运用这类优化方法,但该方法所得结果易将有些设计变量取为约束边界值,导致对设计参数过于敏感。设计参数的变化会导致设计目标的严重性能损失,使设计变得不可靠[8]。在实际工程问题中,常存在一些不确定因素,如材料尺寸、材料性能、载荷偏差等,而确定性优化在设计初期未考虑这些因素,因此得到的优化结果与实际应用中的效果可能相差较大,甚至无法满足设计要求[9]。
1.2 可靠性多目标优化
与确定性多目标优化不同,可靠性多目标优化在设计前期就考虑了多种不确定因素,数学模型为:
minf(x)=(f1(x),f2(x),⋯,fi(x))s.t.{P(gj(x))≥RjxL≤x≤xHi=1,2,⋯,mj=1,2,⋯,m (2) 式中:fi(x)和gj(x)分别为目标函数和约束函数,x为设计变量,Rj为满足约束gj(x)的期望可靠度(设计失败,gj(x)>0)。
在工程设计中,可靠性可以定义为满足约束的概率,图1为确定性设计和可靠性设计的函数分布。可以看出,确定性优化的目标函数曲线存在不满足约束的值,在实际应用中当设计变量取到正态分布边界值时,对应目标函数将无法满足约束,导致设计失败。而可靠性设计中,设计变量对应的目标函数偏离约束较远,设计具有较高的可靠性。在爆炸防护设计中,不可靠的设计可能会导致人员的严重损伤,因此需引入可靠性优化设计。
1.3 优化流程
针对多变量与强非线性的车辆防护组件防护性及轻量化设计,引入可靠性优化设计方法[10],主要流程如图2所示。
建立有限元模型和实验验证,并将两者结果进行对比,验证模型精确性后开展后续优化流程。先定义优化问题,根据优化问题确定设计变量和优化目标函数,并定义各变量的取值范围和概率分布。通过优化拉丁超立方实验设计生成第一轮样本点,计算后得到对应响应,结合方差灵敏度分析方法完成设计变量的筛选。筛选变量后进行三类代理模型的构建拟合,比较并选择精确度最高的代理模型进行多目标优化,再采用蒙特卡洛方法进行可靠性分析得到可靠性优化结果[11]。
2. 模型验证
2.1 底部防护组件台架实验
代表设计状态的车辆底部防护组件和代表车辆乘员舱底板状态的基板,参照北约AEP-55—2011 《Procedures for evaluating the protection level of armored vehicles: mine threat, Vol.2》中的2b实验,爆炸物(6 kg TNT当量)位于台架底部正中心位置,掩埋于硬质土壤表面下100 mm处。
在实际测量中,将基板表面网格化,以便精确测量实验后基板的变形,并且和模拟进行对比。组件实验装置如图3所示。
2.2 车辆底部防护组件模型
为了比较车辆底部爆炸防护性能,设计了三明治夹层结构防护组件,防护组件主要由三层结构组成:上层为单面板,中间夹层包括蜂窝铝和横纵工字梁,底部面板为单层平板。防护组件整体尺寸为1 860 mm×1 500 mm×118 mm,防护组件底面离地高度为337 mm,组件上方进行铁块配重,配重后整体质量为9 011 kg,初始模型面板材料均为6252型防弹钢,加强梁材料为BS700型钢,材料本构模型选用Johnson-Cook模型。
参照AEP-55—2011中的2b实验,设置模型炸药当量为6 kg,炸药掩埋深度为100 mm。防护组件台架模型如图4所示,模型主要由防护组件、配重、台架、空气域、土壤、炸药组成[12]。在有限元模拟中,一定程度上减小网格尺寸和增加网格数量将提升计算精度,但需要更大的计算成本。综合考虑计算效率和精度,网格单元大小主要分布在10~20 mm,其中组件内部蜂窝铝结构的网格单元大小为4 mm。空气和土壤由六面体网格划分,其余部件均由壳单元组成。在计算过程中,伪应变能占总内能比的2.3%,小于5%,满足模拟有效性要求。
2.3 模型有效性验证
为确保后续优化工作的有效性[13],需对初始有限元模型进行准确性验证,比较模拟结果与实验结果,模型准确度主要通过比对组件基板最大变形量和组件整体变形情况来衡量。
实验后的基板变形状态如图5(a)所示,可以看到,基板呈双峰状变形,整体为上凸变形,中心局部区域存在凹陷情况,表面存在较多不规则凹陷,整体未出现大面积破裂。模拟的基板变形情况如图5(b)所示,与实验基本相符。实验前在基板上标记变形测量点,实验后以基板安装点为基准,横向等距取20个塑性变形量最大的点进行测量,实验和模拟的测量点塑性变形量曲线如图5(c)所示。两条曲线基本吻合,实验的基板最大塑性变形为190 mm,模拟的为185.9 mm,误差小于5%,满足模拟精度要求。但防护组件整体防护性能仍未达到要求,基板最大塑性变形量达到了190 mm,远超出了100 m的设计要求,且组件整体质量过大,需进行优化设计。
3. 优化设计
3.1 设计变量及目标的定义
整体模型共包含23个部件、1 333 851个单元、1 087 626个节点,配重后质量为9 000 kg。由图6可见,爆炸冲击后,防护组件基板在约7 ms达到最大变形,爆炸模拟计算至50 ms即可得到最大塑性变形。使用i7 CPU、8 GB内存的电脑计算,时长约为10 h。
在整车中,防护组件与车体之间的作用关系可用基板变形来衡量,同时在设计中应考虑轻量化。当前仅为台架实验,没有整车实验中的假人各项损伤指标作为约束。针对要求,选取防护组件质量M和基板最大塑性变形量D作为设计目标,初始值和目标见表1,唯一约束为基板最大塑性变形量。
表 1 设计目标和初始值Table 1. Design goals and initial values项目 M D 设计目标 函数 fM(x) fD(x) 初始值 430 kg 185.9 mm 目标值 最小 最小 防护组件整体部件参数并不多,因此在初期代理模型构建时选取所有可更改参数的目标变量,共计11个设计变量,变量位置如图7所示。其中:5个为连续变量(面板厚度x1,背板厚度x2,横梁厚度x3,纵梁厚度x4,蜂窝胞元厚度x5);6个为离散变量(纵梁数x6,横梁数x7,横纵梁材料x8,背板材料x9,面板材料x10,蜂窝材料x11)。变量x1~ x5均为连续型数值变量;变量x6取为1、2,变量x7取为1、2、3,对应六类防护组件基础模型,有限元模型如图8所示;变量x8~x11均为离散型材料变量,待选材料20种。因加工工艺限制,横梁、纵梁材料可选变量数为12种,背板材料可选变量数为15种,面板材料可选变量数为11种,蜂窝材料可选变量数为15种,选则范围和分布系数根据实际工程应用情况决定,见表2。各材料具体参数见表3。
表 2 设计变量的初始值和概率分布Table 2. Initial values and probability distributions of design variables设计变量 参数定义 概率分布 初始值 相关系数σ/μ 离散取值 x1 面板厚度 正态分布 9.00 mm 0.1 x2 背板厚度 正态分布 8.00 mm 0.1 x3 横梁厚度 正态分布 8.00 mm 0.1 x4 纵梁厚度 正态分布 8.00 mm 0.1 x5 蜂窝胞元厚度 正态分布 0.35 mm 0.1 x6 纵梁数 离散分布 1 1,2 x7 横梁数 离散分布 2 1,2,3 x8 横纵梁材料 离散分布 6 4,5,6,7,8,9,10,11,16,17,18,19 x9 背板材料 离散分布 7 1,2,3,4,7,8,9,10,11,12,13,14,5,18,19 x10 面板材料 离散分布 7 1,2,3,4,7,10,11,12,13,14,15 x11 蜂窝材料 离散分布 8 1,2,3,4,5,6,7,8,9,10,11,17,18,19,20 表 3 材料参数Table 3. Material parameters序号 材料 密度/(kg·m−3) 弹性模量/MPa 泊松比 屈服强度/MPa 切线模量/MPa 断后延伸率/% 1 NP-500 7 800 210 000 0.29 1 382 3 306.3 12 2 NP-550 7 800 210 000 0.29 1 540 4 391.633 9.5 3 Q1100 7 800 210 000 0.29 1 145 1 951 13 4 Q890 7 800 210 000 0.29 955 223 17.5 5 Q690 7 800 210 000 0.29 718 294.778 19 6 700E 7 800 210 000 0.29 700 1 683 14 7 960E 7 800 210 000 0.29 960 1 991 10 8 7A52 2 600 68 000 0.34 345 1 001 7 9 7B52 2 600 68 000 0.34 470 1 111 7 10 Ti-6Al-4V 4 460 113 800 0.30 1 100 300 11 11 Tii-6Cr-5Mo-5V-4Al 4 430 113 800 0.30 1 250 2 564 5 12 685 1 446 333 0.038 8 0.328 0.13 1.29 13 FD53 1 350 203 0.070 1 0.282 0.11 19.94 14 6252 1 889 438.3 0.045 06 0.394 89 0.320 94 2.520 56 15 LH 1 100 322 0.101 3 0.3587 0.2 7.2 16 Q620 620 524 11.1 0.54 0.59 2.12 17 H14 188 426 0.015 0.34 0.13 0.13 18 752 440 410 0.016 6 0.493 0.076 0.10 19 762 575 226 0 0.362 0.046 0.87 20 Q235 7 800 210 000 0.29 235 580 0.24 根据所选取的设计变量和设计目标,可得数学模型:
minf(x)=(fD(x),fM(x))s.t.{fD(x)≤100xL≤xi≤xHxj={1,2,⋯}i=1,2,3,4,5j=6,7,8,9,10,11 (3) 3.2 设计变量的筛选
优化拉丁超立方采样允许更多的点和更多的组合,且实验点分布均匀,可以捕捉到更高阶的效果,兼具了均匀采样和拉丁超立方采样的优点。本文中,设计采样过程选择优化拉丁超立方实验设计方法,对11个设计变量进行空间均匀填充,第1次采样样本点数量为120个,部分样本点数据见表4。
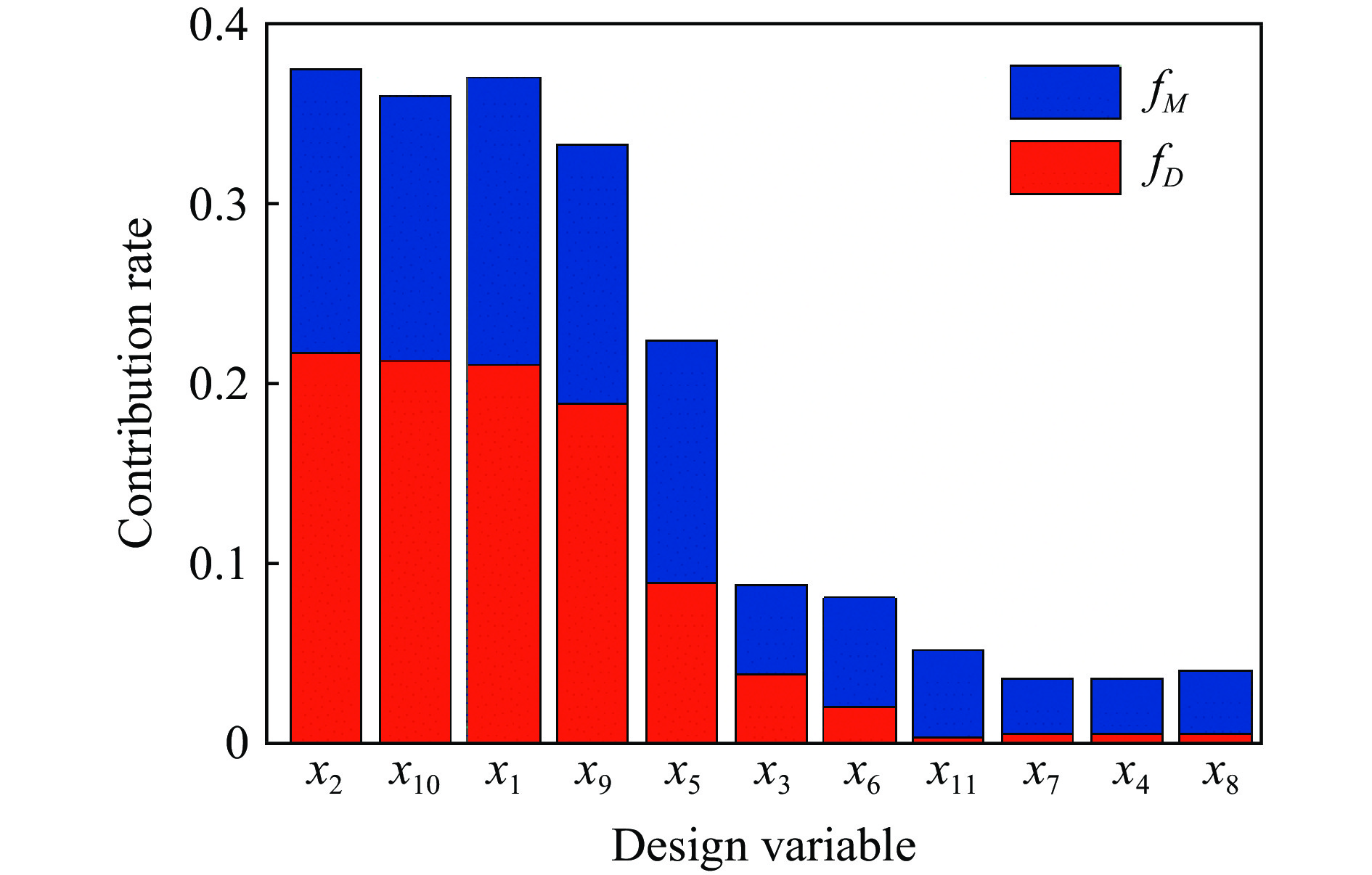
表 4 优化拉丁超立方采样样本Table 4. Optimization of Latin hypercube samples样本 x1/mm x2/mm x3/mm x4/mm x5/mm x6 x7 x8 x9 x10 x11 1 9.630 3 7.226 9 9.848 7 8.369 7 0.452 9 2 3 18 3 10 9 55 10.789 9 10.722 7 8.638 7 7.428 6 0.347 9 2 3 19 2 3 3 56 7.815 1 6.151 3 6.890 8 9.647 1 0.394 1 2 3 7 9 11 20 119 8.420 2 6.285 7 9.983 2 7.697 5 0.263 9 1 2 4 8 14 11 120 10.487 4 7.294 1 6.218 5 12.000 0 0.595 8 1 3 11 1 15 17 通过对上述120组样本点的计算,得到对应响应,运用方差分析(ANOVA)方法得到11个变量对2个设计目标的累积贡献度,如图9所示。在所有设计变量中,x3、x6、x11、x7、x4、x8对设计目标的累积贡献度较低,主要为防护结构中间夹层中的横纵梁数量和厚度及蜂窝材料。设计变量数量的增多易降低代理模型的精确度[14],因此在后续设计过程中将上述低贡献度变量设置为初始值。
3.3 代理模型拟合及选择
通常,单个有限元模型的计算量非常大,如直接基于数值模型进行结构优化设计,则计算资源负担巨大、效率极低,在工程上不可行。因此,需引入代理模型的概念,即根据输入(实验设计生成的设计变量集)与输出(对应的目标响应)之间的关系构建模型[15]。代理模型可以高效地预测在设计空间内其他点的响应,已被广泛运用于结构优化设计中[16]。但不同的代理模型构建方法所适用的对象不尽相同,本文中将应用三种不同的代理模型,并对比分析各代理模型的精度,选用最合适的模型。
响应面模型(response surface method, RSM)的构建较简单,它选取合适的基函数,建立设计变量与目标之间的近似关系,常用的近似方法为基于多项式基函数的多项式响应面法。该方法虽然较易获得显式的数学表达式,但对高度非线性的系统精度不高。
径向基函数模型(radial basis function, RBF)是一种单隐层的前馈神经网络,接收输入信号的单元层为输入层,输入信号通过传递函数映射到隐含层,将输入矢量直接映射到一个新的空间。RBF逼近具有训练速度快、网络结构紧凑等特点。该方法计算量相对较少,较适用多变量、非线性系统。
克里金模型(Kriging, KRG)是一种建立在变异函数理论基础上的半参数化近似模型,是一种空间局部插值法。通过已知的采样点数据,对估计样本点数据进行动态的选择和筛选,并对所选择的样本点进行拟合,具有全局和局部统计特性,对于非线性程度较高的系统有较好的拟合度。
代理模型精度评价由各模型拟合精度R2和相对均方根误差Erms组成,他们分别为:
R2=n∑k=1(ˆyk−¯yk)2n∑k=1(yk−¯yk)2 (4) Erms=√1NN∑k=1(yk−ˆyk)2 (5) 式中:k为样本数量,yk为评估点k函数的真实值;
ˆyk 为代理模型预测值;¯yk 为函数真实值的平均值;N为验证样本点的数目。R2越大,Erms越小,则代理模型越精确。通常情况下,R2>0.9且Erms<0.2,代理模型精度即可用。在完成120组基准样本点的计算及变量筛选后,通过优化超拉丁立方算法重新选取50个样本点进行计算并统计结果,分别构建RSM、RBF、KRG代理模型,并分析各代理模型精度。
由表5可见,三种类型的代理模型中,KRG模型的R2最接近1,Erms最接近0,整体拟合精度最高。因此,在优化流程中,选择KRG代理模型。
表 5 代理模型精度比较Table 5. Accuracy comparisons of different surrogate models代理模型 R2 Erms R2 Erms fD(x) fM(x) RSM 0.958 16 0.073 56 0.756 99 0.186 21 RBF 0.998 27 0.015 08 0.978 26 0.088 46 KRG 0.999 26 0.007 45 0.983 48 0.036 04 3.4 优化求解分析
根据筛选后的设计变量重新构建数学模型,多目标确定性优化和多目标可靠性优化分别为:
minf(x)=(fD(x),fM(x))s.t.{fD(x)≤1006≤x1≤124≤x2≤120.1≤x5≤0.6x9={1,2,3,4,7,8,9,10,11,12,13,14,15,18,19}x10={1,2,3,4,7,10,11,12,13,14,15}j=1,2,⋯,m (6) minf(x)=(fD(x),fM(x))s.t.{P(fD(x)≤100)≥Rj6≤x1≤124≤x2≤120.1≤x5≤0.6x9={1,2,3,4,7,8,9,10,11,12,13,14,15,18,19}x10={1,2,3,4,7,10,11,12,13,14,15}j=1,2,⋯,m (7) 基于KRG代理模型,运用NSGA-Ⅱ(非劣质分层遗传算法)求解帕累托(Pareto)前沿,设置种群大小为12、遗传代数为200、交叉概率为0.9,所得结果如图10所示。基板最大塑性变形量fD(x)和组件质量fM(x)之间成相互制约关系,即fD(x)随着fM(x)的增加而减少,反之亦然。对比两条散点曲线可见,可靠性优化的Pareto前沿比确定性优化更保守,在相同的基板最大塑性变形量下,可靠性优化设计的组件整体质量均略高于确定性优化的。
对设计目标的取值进行权衡后,在图10中得到最优解。对优化目标fD(x)进行可靠度分析,结果见表6。可见,通过可靠性优化[17]得到的基板最大塑性变形量远离约束边界,设计具有更高的可靠度,但防护组件整体质量略高。
表 6 模拟优化结果对比Table 6. Comparison of simulation optimization results优化目标 优化结果 可靠度/% 优化结果 可靠度/% 确定性优化 可靠性优化 fD(x) 99.26 mm 67.3 99.22 mm 99.7 fM(x) 290.8 kg − 315.4 kg − 3.5 实验验证对比
根据可靠性优化设计结果,将数据圆整处理后再次进行模拟计算和台架实验,最优设计参数见表7。实验工况与初次实验相同,实验后防护组件整体形变如图11所示。可见,优化后的防护组件在同等级爆炸冲击下未出现破裂,且基板最大塑性变形量明显降低,防护性能得到较大提升。
表 7 最优设计参数Table 7. Optimal design parameters部件 厚度/mm 材料 面板 7.1 960E 背板 4.2 Q890 横梁2根 8.0 700E 纵梁1根 8.0 700E 蜂窝胞元 0.6 7A52 将所有实验和模拟结果进行对比,具体结果见表8。通过模拟得到优化后的防护组件基板最大塑性变形量fD(x)为97.9 mm,代理模型为99.22 mm,相对误差为1.3%,代理模型准确度较高。实验后测得fD(x)为96.0 mm,与模拟结果相差1.9%,所得结果满足防护要求,组件整体质量fM(x)为320 kg,比初始值低了25.6%。在提高防护组件防护性能的同时,也实现了组件的轻量化,且结果具有更高的可靠度。
表 8 实验与模拟结果比较Table 8. Comparison of experimentand simulation results组件结构 结果 fD(x)/mm fM(x)/kg 初始结构 模拟 185.9 430 实验 190.0 430 优化结构 模拟 97.9 320 实验 96.0 320 4. 结 论
设计了一种车辆防护组件的多目标可靠性优化流程,通过数值模拟对比、灵敏度分析、代理模型构建、优化求解,分析确定性优化和可靠性优化,获得了最优的防护组件设计方案,并通过实验验证了优化方案的可行性,在提升防护组件轻量化和防护性能的同时提高了设计可靠性。主要结论如下。
(1)由防护组件所有设计变量对最大塑性变形量和质量响应的灵敏度分析,内部夹层结构中的梁结构对整体设计的影响较小,板件材料和厚度及蜂窝夹层厚度对防护性能影响较大。
(2)比较三种代理模型的拟合精度,发现KRG模型对本文中的多变量和强非线性工程问题具有更高的应用价值。
(3)可靠性优化相对于确定性优化,所得到的优化结果较保守,但可靠度更高,能为抗冲击防护设计带来更高的安全系数。
对于车辆底部爆炸防护,设计的可靠性要求高于稳健性要求,因此本文中仅做了防护组件的可靠性优化设计。在下一步工作中,如考虑组件的大批量生产应用,可再引入稳健性设计优化。
-
表 1 设计目标和初始值
Table 1. Design goals and initial values
项目 M D 设计目标 函数 fM(x) fD(x) 初始值 430 kg 185.9 mm 目标值 最小 最小 表 2 设计变量的初始值和概率分布
Table 2. Initial values and probability distributions of design variables
设计变量 参数定义 概率分布 初始值 相关系数σ/μ 离散取值 x1 面板厚度 正态分布 9.00 mm 0.1 x2 背板厚度 正态分布 8.00 mm 0.1 x3 横梁厚度 正态分布 8.00 mm 0.1 x4 纵梁厚度 正态分布 8.00 mm 0.1 x5 蜂窝胞元厚度 正态分布 0.35 mm 0.1 x6 纵梁数 离散分布 1 1,2 x7 横梁数 离散分布 2 1,2,3 x8 横纵梁材料 离散分布 6 4,5,6,7,8,9,10,11,16,17,18,19 x9 背板材料 离散分布 7 1,2,3,4,7,8,9,10,11,12,13,14,5,18,19 x10 面板材料 离散分布 7 1,2,3,4,7,10,11,12,13,14,15 x11 蜂窝材料 离散分布 8 1,2,3,4,5,6,7,8,9,10,11,17,18,19,20 表 3 材料参数
Table 3. Material parameters
序号 材料 密度/(kg·m−3) 弹性模量/MPa 泊松比 屈服强度/MPa 切线模量/MPa 断后延伸率/% 1 NP-500 7 800 210 000 0.29 1 382 3 306.3 12 2 NP-550 7 800 210 000 0.29 1 540 4 391.633 9.5 3 Q1100 7 800 210 000 0.29 1 145 1 951 13 4 Q890 7 800 210 000 0.29 955 223 17.5 5 Q690 7 800 210 000 0.29 718 294.778 19 6 700E 7 800 210 000 0.29 700 1 683 14 7 960E 7 800 210 000 0.29 960 1 991 10 8 7A52 2 600 68 000 0.34 345 1 001 7 9 7B52 2 600 68 000 0.34 470 1 111 7 10 Ti-6Al-4V 4 460 113 800 0.30 1 100 300 11 11 Tii-6Cr-5Mo-5V-4Al 4 430 113 800 0.30 1 250 2 564 5 12 685 1 446 333 0.038 8 0.328 0.13 1.29 13 FD53 1 350 203 0.070 1 0.282 0.11 19.94 14 6252 1 889 438.3 0.045 06 0.394 89 0.320 94 2.520 56 15 LH 1 100 322 0.101 3 0.3587 0.2 7.2 16 Q620 620 524 11.1 0.54 0.59 2.12 17 H14 188 426 0.015 0.34 0.13 0.13 18 752 440 410 0.016 6 0.493 0.076 0.10 19 762 575 226 0 0.362 0.046 0.87 20 Q235 7 800 210 000 0.29 235 580 0.24 表 4 优化拉丁超立方采样样本
Table 4. Optimization of Latin hypercube samples
样本 x1/mm x2/mm x3/mm x4/mm x5/mm x6 x7 x8 x9 x10 x11 1 9.630 3 7.226 9 9.848 7 8.369 7 0.452 9 2 3 18 3 10 9 55 10.789 9 10.722 7 8.638 7 7.428 6 0.347 9 2 3 19 2 3 3 56 7.815 1 6.151 3 6.890 8 9.647 1 0.394 1 2 3 7 9 11 20 119 8.420 2 6.285 7 9.983 2 7.697 5 0.263 9 1 2 4 8 14 11 120 10.487 4 7.294 1 6.218 5 12.000 0 0.595 8 1 3 11 1 15 17 表 5 代理模型精度比较
Table 5. Accuracy comparisons of different surrogate models
代理模型 R2 Erms R2 Erms fD(x) fM(x) RSM 0.958 16 0.073 56 0.756 99 0.186 21 RBF 0.998 27 0.015 08 0.978 26 0.088 46 KRG 0.999 26 0.007 45 0.983 48 0.036 04 表 6 模拟优化结果对比
Table 6. Comparison of simulation optimization results
优化目标 优化结果 可靠度/% 优化结果 可靠度/% 确定性优化 可靠性优化 fD(x) 99.26 mm 67.3 99.22 mm 99.7 fM(x) 290.8 kg − 315.4 kg − 表 7 最优设计参数
Table 7. Optimal design parameters
部件 厚度/mm 材料 面板 7.1 960E 背板 4.2 Q890 横梁2根 8.0 700E 纵梁1根 8.0 700E 蜂窝胞元 0.6 7A52 表 8 实验与模拟结果比较
Table 8. Comparison of experimentand simulation results
组件结构 结果 fD(x)/mm fM(x)/kg 初始结构 模拟 185.9 430 实验 190.0 430 优化结构 模拟 97.9 320 实验 96.0 320 -
[1] GRUJICIC M, BELL W C. A computational analysis of survivability of a pick-up truck subjected to mine detonation loads [J]. Multidiscipline Modeling in Materials and Structures, 2011, 7(4): 386–423. DOI: 10.1108/15736101111185289. [2] 吕晓江, 周大永, 孙光永, 等. 基于多目标可靠性优化的骡车车身耐撞性及轻量化设计 [J]. 汽车工程, 2018, 40(7): 790–794. DOI: 10.19562/j.chinasae.qcgc.2018.07.007.LYU X J, ZHOU D Y, SUN G Y, et al. Crashworthiness and lightweight design of mule-car body based on multi-objective reliability optimization [J]. Automotive Engineering, 2018, 40(7): 790–794. DOI: 10.19562/j.chinasae.qcgc.2018.07.007. [3] PAN F, ZHU P, CHEN W, et al. Application of conservative surrogate to reliability based vehicle design for crashworthiness [J]. Journal of Shanghai Jiaotong University (Science), 2013, 18(2): 159–165. DOI: 10.1007/s12204-012-1240-x. [4] 陈崇, 詹振飞, 李洁, 等. 基于材料参数不确定性量化的车身稳健性优化 [C] // 第十八届汽车安全技术学术会议. 江苏苏州, 2015. [5] GU X G, SUN G Y, LI G Y, et al. A Comparative study on multiobjective reliable and robust optimization for crashworthiness design of vehicle structure [J]. Structural and Multidisciplinary Optimization, 2013, 48(3): 669–684. DOI: 10.1007/s00158-013-0921-x. [6] 魏然, 王显会, 周云波, 等. 爆炸冲击下车辆底部结构与座椅系统多参数优化研究 [J]. 振动与冲击, 2016, 35(14): 90–95. DOI: 10.13465/j.cnki.jvs.2016.14.014.WEI R, WANG X H, ZHOU Y B, et al. Multi-parameter optimization of vehicle underbody configuration and occupant restraint system under explosion shock load [J]. Journal of Vibration and Shock, 2016, 35(14): 90–95. DOI: 10.13465/j.cnki.jvs.2016.14.014. [7] 李明星, 王显会, 周云波, 等. 基于神经网络的车辆抗冲击防护组件优化 [J]. 爆炸与冲击, 2020, 40(2): 024203. DOI: 10.11883/bzycj-2019-0055.LI M X, WANG X H, ZHOU Y B, et al. Research on optimization of vehicle anti-shock protection components based on neural network [J]. Explosion and Shock Waves, 2020, 40(2): 024203. DOI: 10.11883/bzycj-2019-0055. [8] GU X G, LU J W. Reliability-based robust assessment for multiobjective optimization design of improving occupant restraint system performance [J]. Computers in Industry, 2014, 65(8): 1169–1180. DOI: 10.1016/j.compind.2014.07.003. [9] YOUN B D, CHOI K K, YANG R J, et al. Reliability-based design optimization for crashworthiness of vehicle side impact [J]. Structural and Multidisciplinary Optimization, 2004, 26(3−4): 272–283. DOI: 10.1007/s00158-003-0345-0. [10] 张义民, 贺向东, 刘巧伶, 等. 非正态分布参数的车辆零件可靠性稳健设计 [J]. 机械工程学报, 2005, 41(11): 102–108. DOI: 10.3321/j.issn:0577-6686.2005.11.018.ZHANG Y M, HE X D, LIU Q L, et al. Reliability-based robust design of vehicle components with non-normal distribution parameters [J]. Chinese Journal of Mechanical Engineering, 2005, 41(11): 102–108. DOI: 10.3321/j.issn:0577-6686.2005.11.018. [11] 白阳阳. 某型车正碰乘员约束系统可靠性设计[D]. 长沙: 湖南大学, 2012: 38−48. [12] 李鹏, 周云波, 王显会, 等. 含蜂窝夹层的V型底部复合装甲仿真研究 [J]. 爆破, 2019, 36(1): 44–48, 69. DOI: 10.3963/j.issn.1001-487X.2019.01.007.LI P, ZHOU Y B, WANG X H, et al. Simulation on V-shaped bottom composite armor with honeycomb sandwich [J]. Blasting, 2019, 36(1): 44–48, 69. DOI: 10.3963/j.issn.1001-487X.2019.01.007. [13] 陈鑫, 王佳宁, 沈传亮, 等. 承载式车身结构局部改型的快速耦合参数化优化设计 [J]. 同济大学学报(自然科学版), 2019, 47(8): 1189–1194. DOI: 10.11908/j.issn.0253-374x.2019.08.016.CHEN X, WANG J N, SHEN C L, et al. Rapidly coupling parametric optimal design of partial structure modification for integral body [J]. Journal of Tongji University (Natural Science), 2019, 47(8): 1189–1194. DOI: 10.11908/j.issn.0253-374x.2019.08.016. [14] 刘德顺, 岳文辉, 杜小平. 不确定性分析与稳健设计的研究进展 [J]. 中国机械工程, 2006, 17(17): 1834–1841. DOI: 10.3321/j.issn: 1004-132X.2006.17.018.LIU D S, YUE W H, DU X P. Study on uncertainty analysis and robust design: a review [J]. China Mechanical Engineering, 2006, 17(17): 1834–1841. DOI: 10.3321/j.issn: 1004-132X.2006.17.018. [15] GAO F L, REN S, LIN C, et al. Metamodel-based Multi-objective reliable optimization for front structure of electric vehicle [J]. Automotive Innovation, 2018, 1(2): 131–139. DOI: 10.1007/s42154-018-0018-8. [16] 陈媛媛. 基于代理模型的汽车碰撞安全性多目标优化研究[D]. 重庆: 重庆大学, 2017: 6−8. [17] 王若冰, 赵志军, 肖和业, 等. 力学环境约束下剪切销可靠性分析及优化设计 [J]. 爆炸与冲击, 2019, 39(7): 075101. DOI: 10.11883/bzycj-2018-0146.WANG R B, ZHAO Z J, XIAO H Y, et al. Reliability analysis and design optimization of a shear pin constrained by mechanical boundaries [J]. Explosion and Shock Waves, 2019, 39(7): 075101. DOI: 10.11883/bzycj-2018-0146. 期刊类型引用(2)
1. 承姿辛,刘玉敏,邱光琦. 基于可靠性优化的空心半轴轻量化设计. 机械设计. 2023(01): 34-39 .  百度学术
百度学术2. 吉诚,周云波,张明,丛铭,付条奇. TNT对近距离接触爆炸下防爆钢结构响应的影响研究. 兵器装备工程学报. 2022(03): 47-53 .  百度学术
百度学术其他类型引用(4)
-








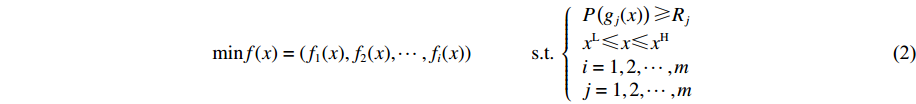

 下载:
下载:







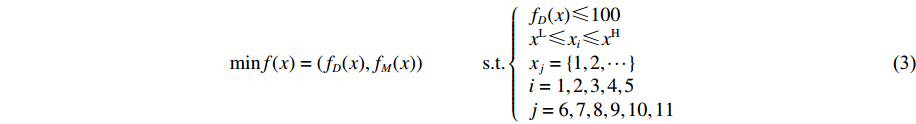

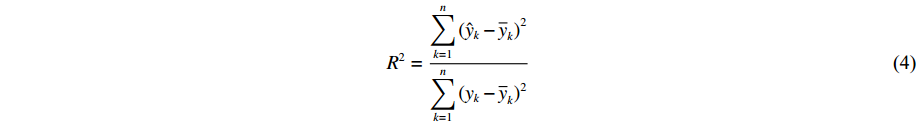
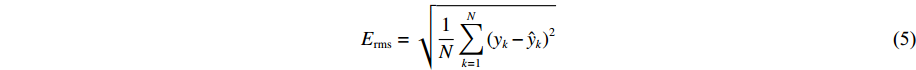







 下载:
下载:










 百度学术
百度学术