反舰导弹随着制导精度与战斗部药量的提升，已经成为海战的重要武器。半穿甲战斗部的反舰导弹对舰船的毁伤包含两个部分，首先战斗部侵彻舰船甲板或舷侧到达船体内部，然后在舱内爆炸造成毁伤。因此，战斗部侵彻能力对于反舰导弹具有重要意义，战斗部能穿透多少层甲板、侵彻弹道与姿态如何，这些都是侵彻过程所关注的重要问题。

战斗部侵彻过程中的受力与运动必然与船体结构有着密切联系。船体结构的特殊之处在于其由板架结构构成。板架结构由均质薄板和纵横分布的加强筋构成，其主要承力构件为纵横加强筋。试验表明，板架结构的加强筋可以显著影响战斗部弹道与姿态变化。

对于均质薄板和厚板的侵彻问题已有许多研究，而对于板架结构侵彻问题的研究工作较为稀少。在试验方面，张中国等^[1]采用模拟装药弹体对舰船单层和多层带筋结构靶进行了侵彻实验，根据实验结果建立了弹体侵彻结构靶板的剩余速度公式。段卓平^[2]对单层带加强筋的结构靶进行了斜侵彻实验，得到实验弹靶条件下加强筋结构靶的破坏模式。段卓平等^[3]开展了大型舰船缩比加筋靶垂直侵彻实验，得到了多种击靶条件下的终点弹道参数和靶板毁伤模式。姚熊亮等^[4]介绍了实尺度侵彻试验，提出了板架结构剩余速度一种改进的理论公式，并数值计算了弹体姿态偏转，理论结果和数值结果都和试验较为接近。

在理论研究方面，宋卫东等^[5]研究了半穿甲战斗部对多层加筋靶板的侵彻，将侵彻过程分为冲塞和花瓣型扩孔过程，提出侵彻的力学模型以及加筋靶板的等效方法。展婷变等^[6]采用动量守恒定理，分析了截卵形弹体正侵彻加强筋结构靶的过程，得出了弹体剩余速度与位移、侵彻位置等物理量之间的关系。巨圆圆等^[7]基于尖卵形弹丸侵彻十字形加筋薄靶的花瓣型破坏模式，利用能量守恒原理得到了弹丸穿甲后的剩余速度计算公式。陈长海等^[8]采用有限元计算程序MSC对弹体侵彻加筋板过程中的弹体剩余速度、靶板毁伤模式和吸能规律进行分析。张宁^[9]运用LS-DYNA对截卵形弹丸侵彻均质靶板和加筋靶板进行数值模拟研究，分析了靶板的破坏情况、 弹丸的剩余速度、弹丸的变形情况以及弹道的偏转。

目前对于板架结构侵彻问题的研究，主要借助了薄板侵彻理论。对于板架结构毁伤模式的认知不足，也只是停留于薄板侵彻毁伤模式。薄板侵彻毁伤模式包括冲塞与花瓣型，分别对应着剪切破坏与拉伸破坏。而加强筋的毁伤模式则更为复杂，包括拉伸、剪切、弯曲、扭转等破坏方式；加之与板耦合之后，板梁破坏模式则更为复杂。物质点方法（material point method，MPM）为粒子类方法，它将结构离散成物质粒子，采用拉格朗日方法对其进行描述。并通过欧拉方法建立网格，使其固定在空间中，作为背景结构。与有限元方法相比，其对裂纹的产生与扩展、冲塞和花瓣等复杂破坏模式有着更好的模拟。Ma等^[10]将物质点法与SPH法（smoothed particle hydrodynamics，SPH）这两种无网格法进行了对比研究，结果表明MPM法在模拟高速碰撞问题方面具有计算量小、不存在拉伸不稳定性、易施加边界条件等优势。

MPM 法发挥了拉格朗日方法和欧拉方法各自的优点，非常适合用于分析超高速碰撞、侵彻等问题。Lian等^[11]耦合了MPM方法与有限元方法，成功模拟了钨弹侵彻钢靶等问题。秦业志等^[12]采用MPM方法构建弹体侵彻舰船板壳结构的数值仿真模型，模拟弹体在侵彻过程中的破甲特性，结果表明MPM法的模拟结果与实验结果吻合较好。谢桂兰等^[13]采用MPM法建立了弹体侵彻靶板的数值模型，对平头弹、球形弹、卵形弹侵彻动靶过程进行了数值模拟，得到了弹体贯穿动靶后的剩余速度、偏转角、扭转角、靶板的毁伤效果。上述文献与本文中MPM的框架，质点离散和物质信息计算的方式是相同的。但本文采取了不同的失效判断方式，与之前文章中单纯的最大塑性应变失效相比，对失效的描述更为细致。并且研究了不同的问题，主要针对加筋板的失效模式问题进行研究探索。李依潇等^[14]采用MPM方法，并建立了一种可处理相变影响的新型物态方程，数值计算了超高速碰撞问题。Wang等^[15]采用MPM方法数值模拟了截顶弹体侵彻921A钢板问题，剩余速度和毁伤模式与实验结果吻合较好，之后又针对不同头部形状弹体比较了靶板毁伤模式的不同。

鉴于MPM方法在模拟高速侵彻问题复杂破坏行为的优势，本文中基于MPM方法研究船体板架结构高速侵彻毁伤模式。首先对MPM方法进行介绍，然后针对板架结构侵彻实验进行数值模拟，验证MPM法在模拟板架结构高速侵彻问题的有效性。在此基础上，针对半球头弹体正侵彻板架结构不同位置开展数值计算，分析板架结构毁伤模式及出现规律。

1.   物质点方法介绍

1.1   物质点法

在物质点方法中^[16]，物体都被离散为许多的质点，并置于背景网格中。其中质点通过拉格朗日方法描述，而网格则通过欧拉方法描述。这种建模方式使得物质信息可以很方便的映射到网格的节点上并进行相应的计算分析，因为网格的节点在空间上是始终固定的。每个时间步，都会将不同质点的质量和动量映射到节点上，如下式所示：

式中：下标$i$代表网格节点数，${n_{\text{p}}}$是质点的总个数，${m_{\text{p}}}$和${v_{\text{p}}}$是质点的质量和速度，${N_i}$是网格节点$i$的形函数。物质点法中，八节点的网格中${N_i}$由下式计算得到：

式中：${\xi _i}$ 、${\zeta _i}$ 、 ${\eta _i}$分别代表网格节点$i$的自然系坐标，数值可以取为$\pm 1$。

使用中心差分算法，可以通过以下公式更新网格节点的动量：

式中：上标$k$表示这个参数对应时间步是${t^k}$。节点内力$f_i^{k,{{\rm{int}}} }$和节点外力$f_i^{k,{\text{ext}}}$可以分别计算如下：

式中：$b_{\text{p}}^k = b_{}^k\left( {{x_{\text{p}}}} \right)$代表质点体力，$\sigma _{\text{p}}^k = \sigma _{}^k\left( {{x_{\text{p}}}} \right)$是质点的柯西力。随之，将节点上计算得到的物质信息重新映射回到质点中。由此，质点${\text{p}}$的空间坐标$x_{\text{p}}^{k + 1}$和速度$v_{\text{p}}^{k + {1 \mathord{\left/ {\vphantom {1 2}} \right. } 2}}$便可以通过下式获得：

此时，所有有用信息均以映射回到质点上，并且网格上的所有变量都将重置为零。随后在下一个时间步骤中，丢弃先前的网格并重建新的背景网格进行计算。

1.2   接触算法介绍

在物质点方法中，物体的运动是通过定义网格节点上的速度来实现的，因此物体之间不会相互作用、接触乃至穿透。接触算法的引入就显得十分必要，设置多重速度场，可以更加方便的计算不同物体各自的速度和动量，简化了物体间是否发生接触的判断。两个物体中的质点向相邻的节点映射物质信息，只有当它们同时对同一个节点$i$的动量产生贡献时，才表示两个物体在此节点处发生接触。

因此对接触问题，可以作假设处理，假设两物体始终没发生接触，将他们视作独立个体，分别计算。将两个物体离散成质点，通过网格节点分别计算他们的动量和速度，称其为假设动量和假设速度，如下式所示：

式中：物理量的上划线代表此参数为假设值，${f_i}$表示假设两个物体没有接触时，独立物体上计算得到的节点力。

此时，对得到的假设值进行分析，采取接触面法向量$n_i^k$计算方法，当他们满足下式条件时：

说明两个物体发生了接触，需要对假设值进行修正，得到接触后物体的速度及动量值。其中$\bar v_i^{{\text{cm}},k + 1}$是节点质心试探速度：

式中：$m_{1i}^k$和$m_{2i}^k$分别代表时间步是${t^k}$时两物体的质量， $\bar v_{1i}^{k + 1}$和$\bar v_{2i}^{k + 1}$分别表示两物体的质心速度。当明确已经发生接触时，在接触节点位置对黏着接触力$f_i^{{\text{ct}}}$进行计算分析，然后分别得到法向接触力$f_i^{{\text{nor}}}$和切向接触力$f_i^{\tan }$：

得到接触力后便可对之前假设的物质信息进行修正，得到满足接触条件的速度和动量：

2.   改变弹着点下的失效模式及其讨论

2.1   物质点方法验证

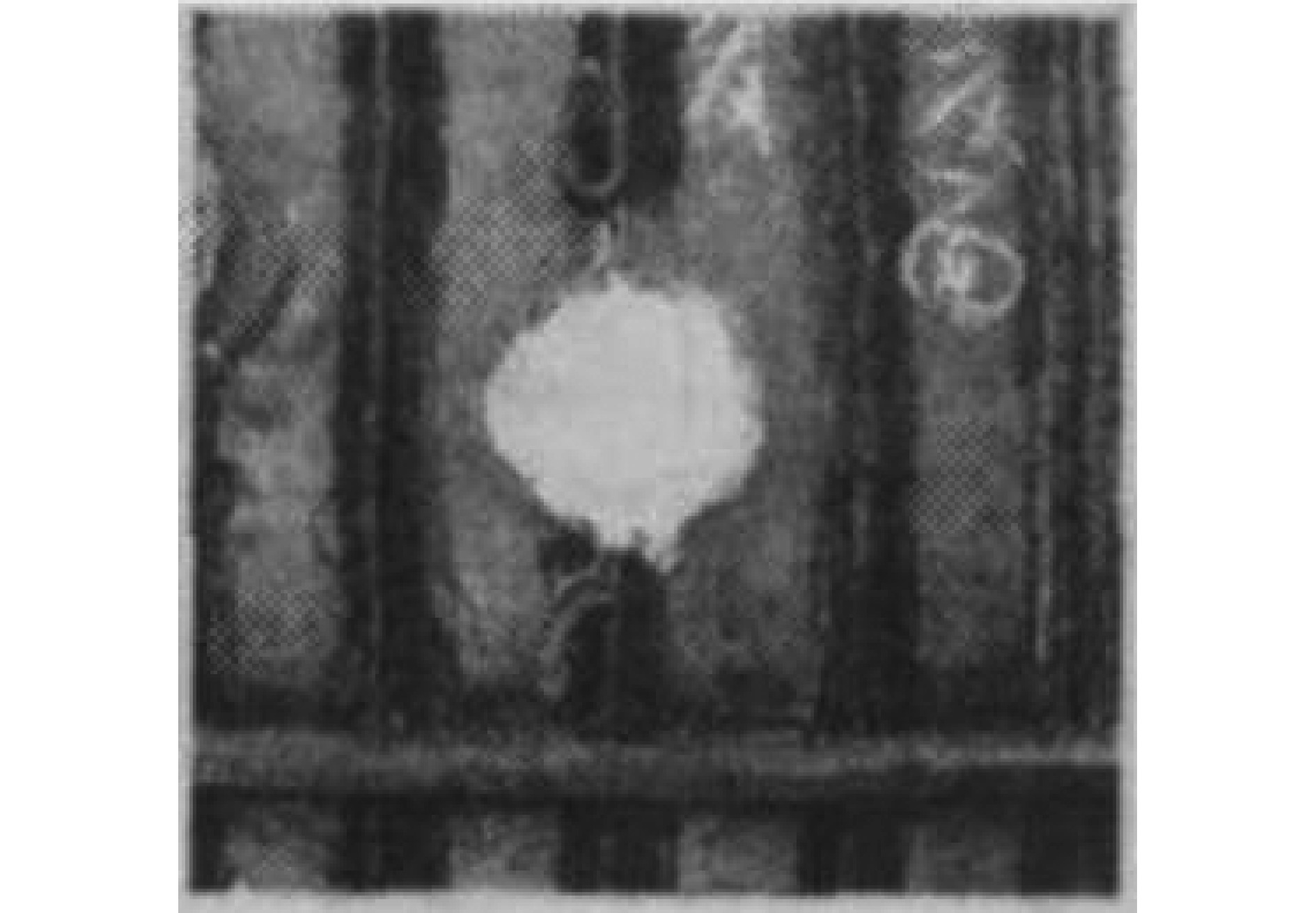
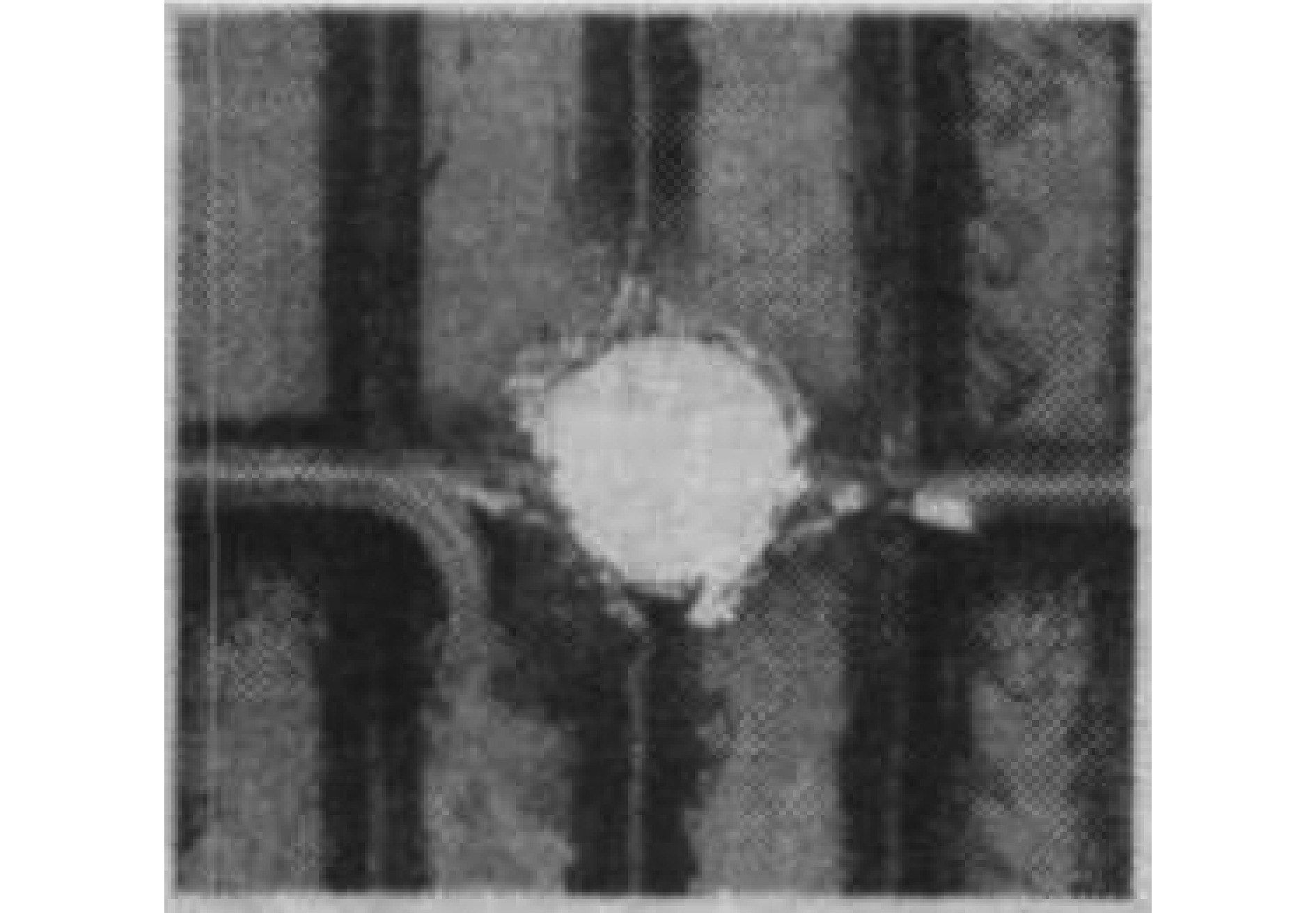
实验靶板由921A钢制成，它的材料属性与实船一致。带有纵横加筋的靶板长1400 mm，宽1000 mm，厚15.2 mm。大筋宽15.2 mm，高68 mm，间距为600 mm；小筋宽7 mm，高26 mm，间距为125 mm。弹体由30CrMnSiNi2A材料制成，质量为16.185 kg，长为370 mm，半径为52.5 mm。具体形状如图1～2所示^[3]。

图  1  靶板结构^[3]

Figure  1.  Target plate^[3]

下载: 全尺寸图片 幻灯片

图  2  弹体结构^[3]

Figure  2.  Projectile^[3]

下载: 全尺寸图片 幻灯片

弹体水平飞行，头部垂直打击到靶板上进行侵彻。在3种不同工况下，弹体的撞击速度分别为617.7、606.5和567.7 m/s。

为了更加良好地应用物质点方法解决侵彻问题，在建模时需要遵循几点规则。首先，根据实验结论提到的弹体在完成侵彻后几乎没有任何损伤，因此将弹体设为刚体。其次，由图1也可以看出将靶板四周边界条件设为刚性固定。最后，动响应及破坏并不是对称发生的，因此建模一定要完整。

靶板的动响应本构模型采取脱聚模型^[17-18]，屈服应力${\sigma _{{\text{eq}}}}$为：

式中：$A$、$B$、$n$、$C$和$m$是材料常数，${\dot \varepsilon ^*} = \dot \varepsilon /{\dot \varepsilon _0}$是无量纲塑性应变率，${T^*} = \left( {T - {T_{\text{r}}}} \right)/\left( {{T_{\text{m}}} - {T_{\text{r}}}} \right)$是无量纲温度，${T_{\text{r}}}$是室温，${T_{\text{m}}}$是材料的熔化温度。

失效方式由可承受的最大拉应力${s_{\max }}$和剪切应力${t_{\max }}$决定。失效模型单元的示意图如图3所示。其中，${\boldsymbol{n}}$代表脱聚面的单位法向，${\boldsymbol{t}}$代表脱聚面单位切向，${\boldsymbol{m}}$为脱聚演化的方向，虚线是脱聚失效发生后产生的自由表面。

图  3  失效模型示意图

Figure  3.  Sketch of the failure model

下载: 全尺寸图片 幻灯片

对每个物质点进行应力更新并对应力进行计算分析，得到质点上的主应力${s_1}$、${s_2}$和${s_3}$以及它们的单位法向${{\boldsymbol{n}}_{\bf 1}}$、${{\boldsymbol{n}}_{\bf{2}}}$和${{\boldsymbol{n}}_{\bf 3}}$。

（1）最大主应力为${s_1}$，当${s_1} \text{＞} {s_{\max }}$，${\tau _t} \text{＜} {t_{\max }}$时，发生第1种拉应力失效。失效的位置处于最大主应力作用面，方向即为主应力的单位方向矢量，即${\boldsymbol{n}} = {{\boldsymbol{n}}_{\bf 1}}$。

（2）剪切应力${\tau _t} = \dfrac{{{s_1} - {s_3}}}{2}$，当${\tau _t} \text{＞} {t_{\max }}$，${s_1} \text{＜} {s_{\max }}$时，发生第2种剪切应力失效。失效的位置为最大剪切应力作用面，${\boldsymbol{n}} = \dfrac{{{{\boldsymbol{n}}_{\bf 2}} \times \left( {{{\boldsymbol{n}}_{\bf 1}} + {{\boldsymbol{n}}_{\bf 3}}} \right)}}{{\left| {{{\boldsymbol{n}}_{\bf 2}} \times \left( {{{\boldsymbol{n}}_{\bf 1}} + {{\boldsymbol{n}}_{\bf 3}}} \right)} \right|}}$。

（3）当同时满足${s_1} \text{＞} {s_{\max }}$和${\tau _t} \text{＞} {t_{\max }}$时，发生混合失效。拉应力失效和剪应力失效同时发生，方向${\boldsymbol{n}}$取最大主应力作用面法向和最大剪切应力作用面法向的平均值。设置脱聚条件函数${f^{\text{d}}}$为：

式中：$\lambda$为流动参数，表征脱聚更新进度；${\tau ^{\text{e}}}$是等效力，${U_0}$是初始单位面积表面能，$q$为模型参数，调节界面拉力和脱聚量的关系。当${f^{\text{d}}}$=0时，脱聚发生。对脱聚条件函数，式（19）进行一阶Taylor展开：

式中：下标${\text{tr}}$代表当前处于弹性预测状态。由此便可以得到进入脱聚状态后，每个时间步的$\Delta \lambda$，即$\lambda$增量。随后，修正脱聚质点的物质信息状态。其中，应力状态$\sigma$为：

式中：${\boldsymbol{E}}$为弹性张量，${\sigma _{{\text{bef}}}}$是上一时间步的应力状态，脱聚应变增量$\Delta {\varepsilon ^{\text{d}}}$形式为：

式中：${L_{\text{e}}}$是单元的等效尺寸。脱聚应变${\varepsilon ^{\text{d}}}$则为上一步的总应变与当前时间步增量的加和结果。程序循环计算，直到某一时刻$\lambda = 1$时，脱聚面两边的物质点实现完全脱离，形成自由表面后，其上应力分量归为零。

具体的靶板材料参数数值在表1中展示^[2-3]。

表  1  靶板材料参数数值^[2-3]

Table  1.  Material parameters used for target^[2-3]

泊松比	A/MPa	B/MPa	n	C	m	$\lambda$	smax/MPa	tmax/MPa
0.35	685	760	0.587	0.015	1.027	1	685	342

下载: 导出CSV 

| 显示表格

表2展示出通过物质点方法计算得到的剩余速度与实验结果，图4则更为形象生动地展示了实验和数值模拟得到的剩余速度对比图。表3通过无量纲形式$\dfrac{{{v_{\rm{r}}}}}{{{v_{\rm{i}}}}}$（${v_{\rm{r}}}$为剩余速度，${v_{\rm{i}}}$为冲击速度）对速度损失程度进行表征，并计算数值与实验结果之间的误差，发现吻合良好。

表  2  剩余速度的数值和实验结果

Table  2.  Numerical values and experimental results of residual velocity

工况	冲击速度/ （m·s−1）	弹着点位置		实验剩余速度/ （m·s−1）	物质点剩余速度/ （m·s−1）
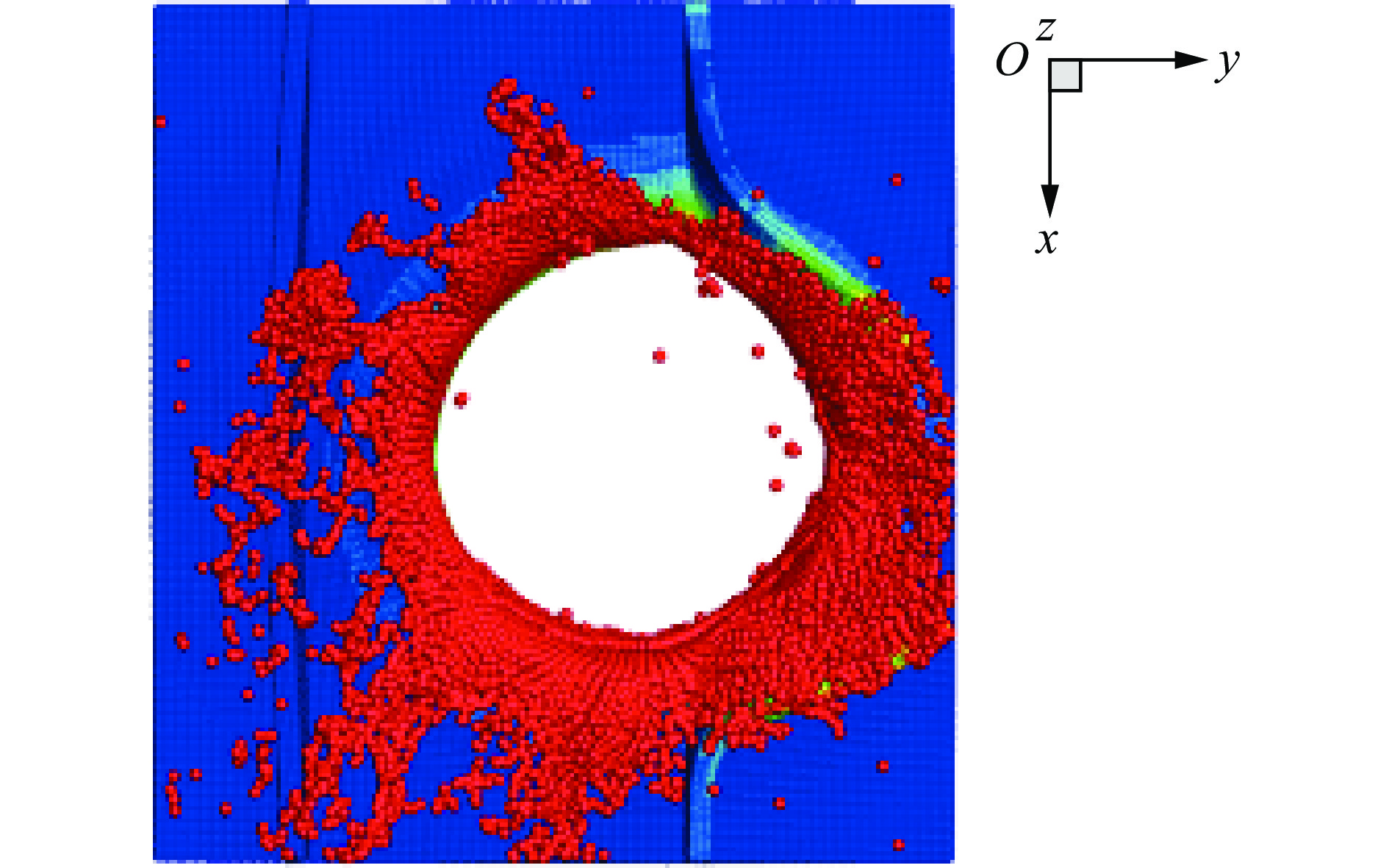
		距大筋/ mm	距小筋/ mm
1	617.7	0.0	31.0	574.9	571.7
2	606.5	−	−	583.8	560.1
3	567.7	55.0	52.0	526.8	515.0

下载: 导出CSV 

| 显示表格

图  4  剩余速度随3种不同冲击速度的变化情况

Figure  4.  Variation of residual velocity with three different impact velocities

下载: 全尺寸图片 幻灯片

表  3  数值和实验无量纲数结果对比

Table  3.  Comparison of numerical and experimental dimensionless number results

工况	无量纲数 $\dfrac{{{v_r}}}{{{v_i}}}$		误差/%
	实验	数值
1	0.931	0.926	0.5
2	0.963	0.923	4.2
3	0.928	0.908	2.2

下载: 导出CSV 

| 显示表格

通过观察表2～3可以发现，工况2中速度损失很小，工况1和3中速度损失很明显，而它们之间的主要区别是是否含有加强筋。因为加强筋会吸收很多能量并使得靶板的失效模式发生改变，因此弹着点位置发生改变会改变与加强筋的接触显得尤为重要。在实验中，靶板的失效模式如图5～6所示。通过物质点模拟的结果如图7～8所示。提取图7中显示的工况，对靶板应力状态进行分析判断，考察所对应的引入脱聚模型的物质点法得到的失效模式，如图9～10所示。随后，将实验结果与数值模拟结果进行对比，验证了物质点方法的适用性。

图  5  弹着点位于小筋^[3]

Figure  5.  Impact point on small stiffener^[3]

下载: 全尺寸图片 幻灯片

图  6  弹着点位于纵横加筋^[3]

Figure  6.  Impact point on cross-stiffener stiffener ^[3]

下载: 全尺寸图片 幻灯片

图  7  弹着点位于小筋

Figure  7.  Impact point on small stiffener

下载: 全尺寸图片 幻灯片

图  8  弹着点位于纵横加筋

Figure  8.  Impact point on cross-stiffener stiffener

下载: 全尺寸图片 幻灯片

图  9  主应力分布图

Figure  9.  Principal stress distribution diagram

下载: 全尺寸图片 幻灯片

图  10  剪切应力分布图

Figure  10.  Shear stress distribution diagram

下载: 全尺寸图片 幻灯片

从图9中可以看到在弹体刚刚接触到靶板时，接触部位及加强筋顶端的主应力超过容许应力。从图10中则可以发现，剪切应力基本呈现出中间最大向周围逐渐减小的趋势。对比两幅图，可以从中观察到靶板与弹体接触部位直接产生混合的失效模式，加强筋的顶部产生第1种拉应力失效毁伤模式。当弹体持续侵彻，破坏扩展的过程主要以第2种剪切应力失效模式为主，因为在接触部位周围的拉应力呈现小值（环状蓝色应力），而剪切应力超过容许剪切应力。

图7～8中毁伤云图包含失效和飞散粒子，为了更直观清晰地观察失效模式，将这些粒子隐藏则得到图11～12。

图  11  弹着点位于小筋

Figure  11.  Impact point on small stiffener

下载: 全尺寸图片 幻灯片

图  12  弹着点位于纵横加筋

Figure  12.  Impact point on cross-stiffener stiffener

下载: 全尺寸图片 幻灯片

观察图7和图11中弹着点位于小加强筋处的靶板的毁伤情况。图7中，数值模拟结果和实验结果处于相同视角。图11中将靶板视角进行转变，从而可以更好地分析和研究目标的毁伤。图8和图12则用相同的方法描述弹着点位于交叉加筋处时靶板的毁伤情况。

图11中包含2个黑色箭头，它们分别指向了已经断裂的小筋两侧。加强筋吸收了弹体穿透过程中损失的动能，并将持续变形直到所有能量都转换为应变能为止，失效模式与实验结果一致。图12中也包含2个黑色箭头，一个指向小筋，另一个指向大筋。大筋已经出现有一个破口，它将沿着裂纹方向延展到上下两端形成断裂，形成类似于小筋的失效模式。到目前为止，已从各个角度验证了物质点方法的有效性。

2.2   弹着点对毁伤模式的影响

弹着点和加强筋之间的相对位置变化是造成毁伤模式产生区别的最主要因素。因此，选取一块方板在上面布置一条加强筋，然后设置弹体可能出现的不同弹着点位置。靶板的长度取为200 mm，其上加筋宽为4 mm，高为10 mm。弹头形状设为半球形，半径为20 mm，圆柱弹筒长为20 mm。背景网格间距为2 mm，其中物质粒子间距则为1 mm，因为网格在每个方向上可以包含两个粒子。材料属性与上文相同，具体形式如图13所示。

图  13  整体布置示意图

Figure  13.  Overall layout diagram

下载: 全尺寸图片 幻灯片

由图13中可以看到，靶板中间为加强筋，红色圆圈表示弹着点从加强筋中心一直向右方偏移。经过上述一系列数值模拟的结果，可以发现靶板产生了不同种类的失效模式，包含侵彻中最常见的冲塞和花瓣型破坏。下文将继续完善不同的毁伤模式，并分析不同弹着点对其的影响。当弹着点位于加强筋中心处时，靶板的毁伤如图14～15所示。

图  14  加强筋毁伤示意图

Figure  14.  Stiffener damage diagram

下载: 全尺寸图片 幻灯片

图  15  靶板整体毁伤示意图

Figure  15.  Target damage diagram

下载: 全尺寸图片 幻灯片

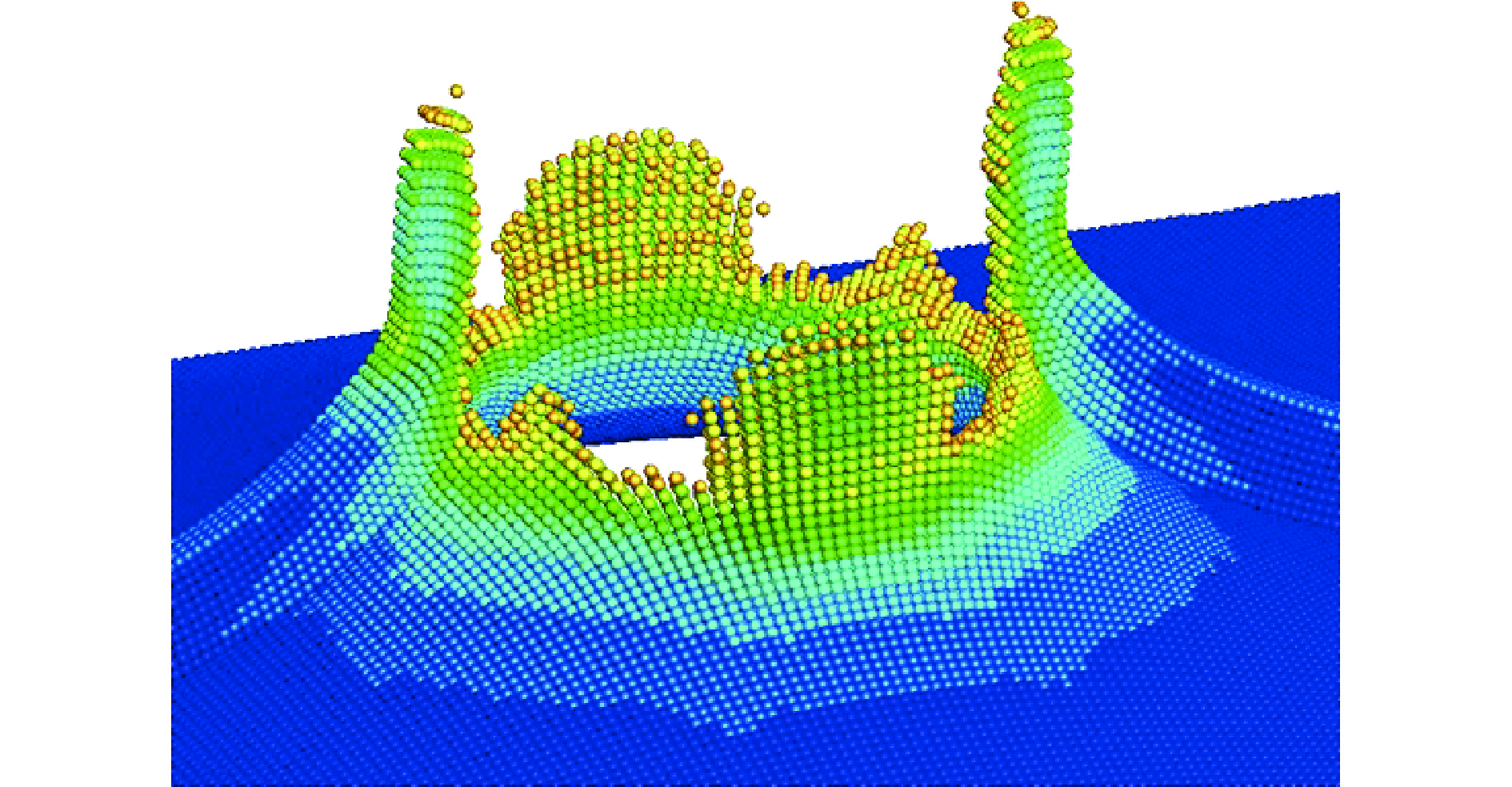
图14中箭头指向加强筋，表明其在弹体作用下弯曲变形，直到应力值达到失效临界值，逐渐发生撕裂破坏。当弹体完全穿透靶板后，靶板的毁伤模式如图15所示，已将失效粒子及飞散粒子隐藏。加强筋将持续发生弯曲变形，直到吸收的弹体动能完全转化形成应变能。而加筋的左右两侧的毁伤几乎完全对称，形成花瓣形失效。随着弹着点位置的向右偏移，偏移距离为1 mm，毁伤模式也开始随之发生变化，如图16～17所示。

图  16  加强筋毁伤示意图

Figure  16.  Stiffener damage diagram

下载: 全尺寸图片 幻灯片

图  17  靶板整体毁伤示意图

Figure  17.  Target damage diagram

下载: 全尺寸图片 幻灯片

在图16中，加强筋向左偏移，顶部出现明显的塑性变形。黑色箭头指向位置出现裂纹，弹体从右侧击穿靶板。从图17中可以看出，裂纹持续扩展一直延伸到加筋顶部的塑性区域形成整体断裂，靶板在加筋两侧不再呈现对称的花瓣破坏。由于弹体的右侧偏移，右侧靶板的变形更加明显，花瓣形状更大。而左侧由于裂纹的扩展效应，形成两个较小的花瓣，并且使得靶板与加强筋连接处发生撕裂。随着弹着点位置的不断偏移，靶板的毁伤模式再次发生变化如图18所示。

图  18  靶板的毁伤模式示意图

Figure  18.  Stiffened target plate failure mode diagram

下载: 全尺寸图片 幻灯片

从图18中可以看出，加强筋此时只发生向左的塑性变形不再出现断裂破坏。靶板右侧主体呈现花瓣型失效，伴随着部分冲塞失效，在图中用黑色圆圈标注。更多细节见图19～20。

图  19  弹着点位置偏移5 mm

Figure  19.  The impact point is offset by 5 mm

下载: 全尺寸图片 幻灯片

图  20  弹着点位置偏移6 mm

Figure  20.  The impact point is offset by 6mm

下载: 全尺寸图片 幻灯片

从图19～20中可以看到，在侵彻过程中（如箭头所指）靶板与加强筋连接处产生破口，加强筋发生明显塑性变形。随着弹着点位置的继续偏移，加强筋的塑性变形程度将不断下降，并且破口会逐渐减小直至消失。因此，之后的研究将主要针对靶板加筋右侧的毁伤模式变化，可以通过下图21～28进行对比分析。

图  21  弹着点位置偏移7 mm

Figure  21.  The impact point is offset by 7 mm

下载: 全尺寸图片 幻灯片

图  22  弹着点位置偏移8 mm

Figure  22.  The impact point is offset by 8 mm

下载: 全尺寸图片 幻灯片

图  23  弹着点位置偏移9 mm

Figure  23.  The impact point is offset by 9 mm

下载: 全尺寸图片 幻灯片

图  24  弹着点位置偏移10 mm

Figure  24.  The impact point is offset by 10 mm

下载: 全尺寸图片 幻灯片

图  25  弹着点位置偏移11 mm

Figure  25.  The impact point is offset by 11 mm

下载: 全尺寸图片 幻灯片

图  26  弹着点位置偏移12 mm

Figure  26.  The impact point is offset by 12 mm

下载: 全尺寸图片 幻灯片

图  27  弹着点位置偏移13 mm

Figure  27.  The impact point is offset by 13 mm

下载: 全尺寸图片 幻灯片

图  28  弹着点位置偏移14 mm

Figure  28.  The impact point is offset by 14 mm

下载: 全尺寸图片 幻灯片

从图21～28中可以发现由箭头所指向的花瓣型毁伤模式一直在由于弹着点位置的变化而发生改变。由图21中完整的一个花瓣变为3个花瓣，随后中间花瓣的弯曲曲率逐渐增加，最后变为图28中的4个花瓣的毁伤形式。这是由于在弹着点不断向右偏移的过程中，使得弹体与靶板的接触形式发生改变。弹体偏转程度发生改变，使得其施加在花瓣上的力持续增加，致使花瓣分裂成3个。中间的花瓣由于获得的初始动能持续增加，因此应变程度不断提高，弯曲曲率增大。当应力高于靶板最大承受力时，花瓣会从中间断裂，形成最终的毁伤模式。并将毁伤模式再次跟实验结果进行比对，如图29～30所示。

图  29  弹着点位置偏移5 mm

Figure  29.  The impact point is offset by 5 mm

下载: 全尺寸图片 幻灯片

图  30  弹着点位置偏移6 mm^[2]

Figure  30.  The impact point is offset by 6 mm^[2]

下载: 全尺寸图片 幻灯片

如图29所示，红色圆圈表明中间花瓣的毁伤模式，花瓣在弹体侵彻作用下形成并产生弯曲变形。与实验结果十分相近，再次验证了数值模拟的准确性。

3.   结　论

本文中采用物质点方法研究船体板架结构高速侵彻毁伤模式。首先，针对板架结构侵彻实验进行数值模拟，验证物质点方法在模拟板架结构高速侵彻问题的有效性。然后，针对半球头弹体正侵彻板架结构不同位置开展数值计算，分析板架结构毁伤模式及出现规律。主要结论如下。

（1）针对板架结构侵彻实验进行数值模拟，发现数值模拟结果与实验吻合良好，剩余速度误差在5%之内，且对靶板撕裂破坏、花瓣破口等模拟良好，说明了物质点法在模拟板架结构高速侵彻破坏模式方面存在优势。

（2）当质点上的应力超过式（18）计算得到的屈服应力时，开始进入塑性应变，板架也因此产生塑性变形。通过与最大拉应力${s_{\max }}$和剪切应力${t_{\max }}$比较，达到相应判据条件后，质点进入脱聚状态。当$\lambda = 1$时，脱聚面两边的物质点完全脱离，因此产生断裂。板上的裂纹径向扩展生成花瓣形破口，加强筋上的裂纹沿垂向扩展使其从中间断开，加强筋与板连接处发生撕裂。当加强筋未发生断裂时，弯曲塑性变形即为塑性应变的具体表现形式。

因此，破坏大体分为两个阶段展开，首先产生塑性应变，然后随着侵彻持续作用挠度增加最终形成断裂。塑性应变积累但未产生断裂时，便表现为弯曲塑性变形。当发生断裂时，裂纹发生位置及延伸方向的不同可能形成靶板的花瓣破坏或撕裂破坏。

（3）发现了板架结构毁伤模式与弹着点位置密切相关，对于正中加强筋、偏心命中加强筋情况，毁伤模式都不同，发现了板架结构3种毁伤模式：①加强筋断裂，加筋两侧板出现对称花瓣破坏；②加强筋弯曲变形，加筋一侧板出现拉伸撕裂，另一侧板出现花瓣开裂；③加强筋弯曲变形、加筋一侧板仅有塑性变形，另一侧板花瓣开裂。毁伤模式与弹着点位置有关，仅当正中加强筋时，发生毁伤模式①；加强筋偏心距离为1～6 mm时（球头半径30%以内）发生毁伤模式②；加强筋偏心距离为6～20 mm时（球头半径30%～100%）发生毁伤模式③。

并且，通过计算结果可以发现：弹着点无偏移时，加强筋从中部断裂；弹着点偏移较小时，加强筋与板子连接处撕裂；弹着点继续偏移，则可能出现板材与加筋连接处的中部裂纹扩展形成花瓣（如图14所示）；偏移继续增加，则靶板与加筋连接处形成小破口，直至破口消失只剩下塑性变形。对于弹着点偏移一侧的靶板，呈现主体花瓣并伴随小部分的充塞失效。花瓣失效模式中，弹着点改变，花瓣个数及形式始终发生变化，花瓣卷曲程度也不尽相同。