通过轧制加工得到的金属板材常表现出各向异性塑性变形行为^[1]。基于单一曲线假定的各项同性金属屈服准则难以准确有效描述金属板材的塑性变形行为，为此，人们发展了各向异性屈服准则。然而，基于标准单轴拉伸实验数据难以对各向异性屈服准则进行有效标定^[2]。除了金属板材，纤维增强复合材料的力学性能也通常表现为各向异性^[3-5]，单向应力状态下复合材料力学行为研究已不能满足结构设计的需要。另外，各种结构在加工和服役期间通常经受多轴载荷条件，这可能导致结构处于复杂的应力状态^[6]。因此，发展多轴加载实验技术研究复杂应力状态下材料力学性能显得尤为重要和迫切。

目前，双轴拉伸实验技术发展相对成熟。早期发展的双轴拉伸实验技术是在单向拉伸下通过改变试样构型设计来实现双轴拉伸应力状态，诸如平滑宽板试样(宽厚比大于30)^[7]、板中央双轴带加固轮缘试样^[8]。后来，人们又发展了薄膜凸胀双轴拉伸实验技术^[9]、内压薄壁圆筒^[10]或球壳双轴拉伸实验技术以及十字形试样双轴拉伸实验技术^[11]等。十字形试样双轴拉伸实验技术，由于十字形试样制作简单，同时容易对试样沿着不同方向施加不同比例加载，受到人们广泛关注。任家陶等^[8]以及Hannon等^[12]都曾对板材平面双轴拉伸实验系统的发展进行过综述。双轴拉伸实验系统主要有两类：独立双轴液压伺服静态试验机和基于连杆机构附加装置的双轴试验机^[13]。Makinde等^[14]在1992年首先发展了独立双轴液压伺服实验系统，在两个垂直方向上分别设置两对液压驱动器，对十字形试样进行双轴加载，在每个方向设置测力传感器和位移传感器。在双轴拉伸实验中，一种重要因素是在整个实验过程中保持试样的中心不动，这对液压伺服同步性控制提出了比较高的要求，同时建立一套独立双轴液压伺服静态拉伸实验系统是非常昂贵的。为此，人们尝试在常用的液压伺服万能试验机上设计一套四连杆或八连杆机构的附加装置^[15-16]，把竖直方向的单轴驱动转化为水平方向的双轴驱动，即当液压万能试验机的压盘向下移动时，通过四连杆或八连杆将运动转化为两个不同方向的水平运动，从而对十字形试样施加双轴加载。针对许多金属板材和纤维增强复合材料，人们已开展一些双轴拉伸实验研究工作，取得了一些重要的研究成果^[17]。除了双轴拉伸加载系统，双轴拉伸试样尺寸合理设计也是至关重要的。尽管十字形试样已被广泛应用，由于缺乏试样几何形状的标准，很难比较不同实验室的实验结果^[18]。在设计试样基本原则是保证试样的中心部分产生大部分变形，尽量避免试样其他区域出现应力集中。由于板材在单轴拉伸下的变形能力远小于双轴拉伸时的变形能力，导致断裂通常发生在试样臂上。十字形试样设计中，人们提出了许多方法来防止这种情况，主要方法有切割型、缩小截面型以及带槽型^[19]。

无论是在双轴加载系统，还是在十字形试样设计方面，都已经取得一些研究成果，但已发展的双轴拉伸实验技术主要局限于准静态加载条件，难以开展动态双轴加载实验^[20]。最近，李玉龙等^[21]发明了一种基于电磁力加载的双轴分离式Hopkinson拉杆实验装置，首先实现十字形试样的高应变率双轴加载。本文中基于HTM-5020液压伺服高速试验机，发展一种的具有宽广加载应变率调节能力的双轴拉伸加载系统，利用锥面接触导向驱动方法，把加载锤头竖直方向的驱动力通过十字形布置的高刚度加载力臂和导向滑轨联合作用转化为水平方向的双轴驱动力。利用超高速相机与数字图像相关性(digital image correlation, DIC)技术相结合^[22]，发展十字形试样全场应变测量技术。借助数值模拟手段，对双轴加载实验装置和十字形试样设计进行优化。最后，开展2024-T351铝合金单轴和双轴拉伸实验，验证双轴拉伸实验技术的可行性和可靠性。

1.   实验技术

1.1   双轴拉伸加载装置设计

1.1.1   结构组成及工作原理

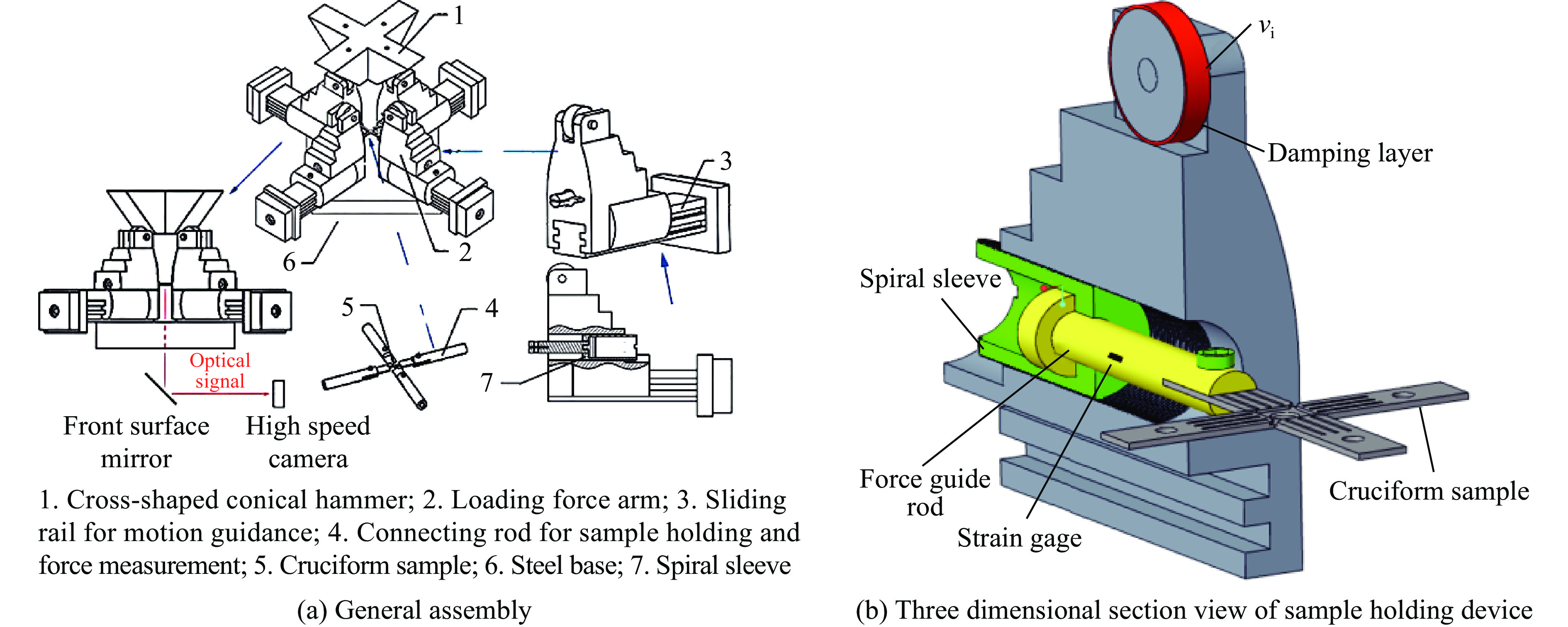
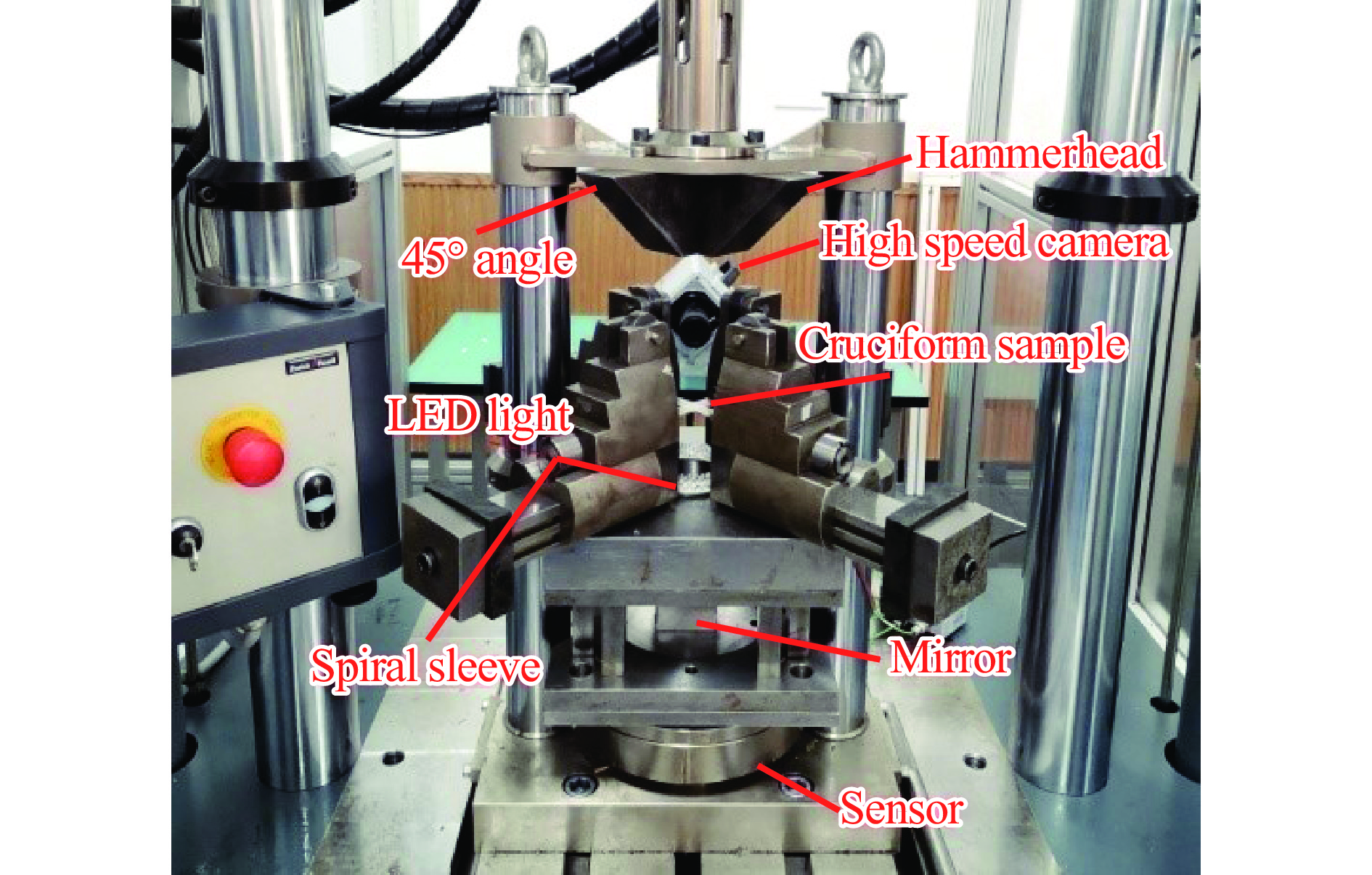
图1(a)给出了锥面驱动滑槽式双轴拉伸加载装置结构组成示意图，主要由十字形锥面锤头、加载力臂、十字形布置的导向滑轨、试样夹持和测力连接杆、十字形试样、钢基座以及螺旋套筒组成，其中加载力臂和导向滑轨必须具有足够的刚度，确保加载稳定性。十字形锥面锤头与Zwick HTM-5020液压伺服高速试验机的加载杆相连接，并沿着钢导杆上下运动，如图2所示。试样夹持连接杆通过加载力臂内部的套筒旋转前后移动，从而调整锤头与滚轮接触间隙，如图1(b)所示，十字形试样与试样夹持连接杆通过自锚式固定螺栓相连接，同时在夹持连接杆上粘贴应变片来实时测量试件受到的加载力时程。当锤头的十字形锥面与高刚度的加载力臂上滚轮相接触时，将驱动十字形布置的加载力臂沿着导向滑轨向外运动，从而把锤头竖直方向的单轴驱动力转化为水平方向的双轴驱动力，实现对十字形试样同步平面双轴加载。Zwick HTM-5020液压伺服高速试验机可通过闭环控制和开环控制实现加载速度变化范围为0.001～20 m/s。改变锤头运动速度，可方便地调节试样的应变速率。改变十字形锥面锤头的锥面倾斜角度，还可以实现不同加载路径比的非等轴双轴拉伸。为了监测十字形试件中心部分应变，在钢基座中心处开孔，在基座下面布置45°反射镜，利用水平布置的高速相机实时获取试样变形过程的散斑图像，再借助数字图像相关性分析技术获得试样中心处全场应变。这里提出的双轴拉伸加载装置具有加载方式灵活多变，结构紧凑且成本低的特点。

图  1  双轴拉伸加载装置结构装配图

Figure  1.  Structural assembly drawing of biaxial tensile loading device

下载: 全尺寸图片 幻灯片

图  2  基于Zwick HTM-5020液压伺服高速试验机的双轴拉伸加载装置

Figure  2.  Biaxial tensile loading device based on Zwick HTM-5020 hydraulic servo high speed machine

下载: 全尺寸图片 幻灯片

1.1.2   结构与优化

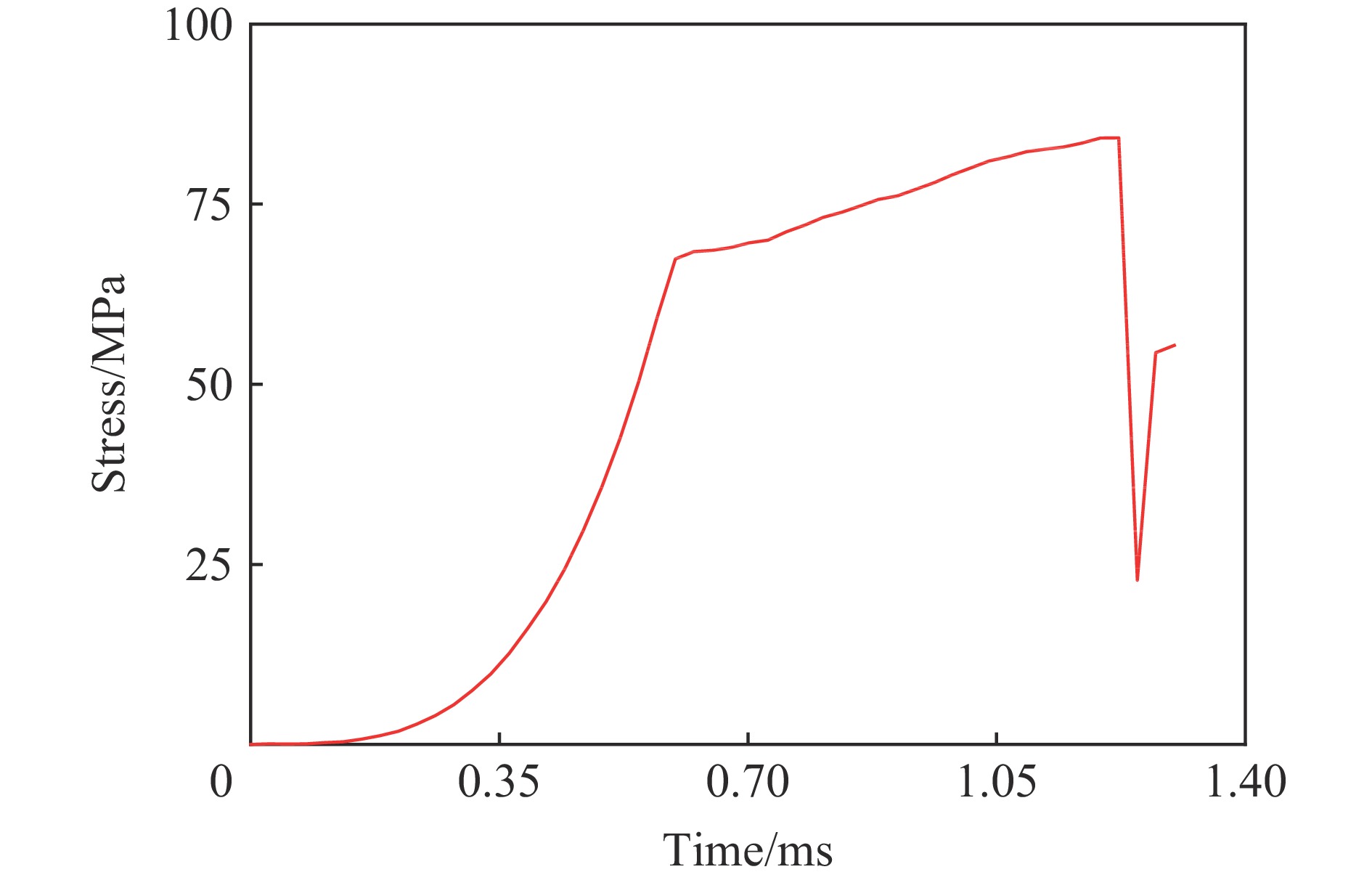
在开展实验之前，采用数值模拟手段，对图1给出的双轴拉伸加载装置进行结构优化，考虑到结构的对称性，为了减少计算量，这里利用ABAQUS软件建立了装置的四分之一对称有限元计算模型，如图3所示。计算中，锤头和接触滚轮材料选取40Cr合金钢，屈服应力为785 MPa；其他结构部件的材料都是45钢，屈服应力355 MPa，材料本构关系采用线弹性模型，计算参数：ρ=7 800 kg/m³，E=210 GPa, ν=0.3；橡胶缓冲层材料采用Ogden^[23]超弹性本构模型，材料模型参数来自文献[24]。十字形试样材料为2024-T351铝合金，材料本构关系使用Johnson-Cook弹塑性本构模型, 本构模型介绍和材料参数详见文献[25]。计算中，锤头竖直方向速度v_y设置为3 m/s，在装置设计中，十字形锤头的锥角α是关键设计参量之一。在15°、30°、45°、60°和75°等5种锥角下开展数值分析，讨论关键结构组件的运动情况和关键接触点的受力情况。在加载速度保持3 m/s恒定条件下，锥角对加载力臂水平速度v_x有明显的影响，如图4所示，锥角越大，加载力臂获得的速度越大，速度转化效率越高。为了降低锤头和滚轮之间接触应力，在接触面添加橡胶缓冲层，图5给出了不同锥角条件下锤头与滚轮接触处单元最大等效应力（定义为接触应力）时程曲线，结果显示：随着锥角增大，接触应力增大；在锥角60°时，接触应力达到2.4 GPa，远大于滚轮材料40Cr合金钢的屈服应力；而锥角45°时，接触应力约500 MPa，低于40Cr合金钢的屈服应力，保证实验中滚轮不发生塑性变形。同时在图5右上角也给出没有橡胶缓冲层时45°锥角条件下接触单元最大应力时程对比曲线，结果显示橡胶缓冲层的缓冲效果非常好，大幅降低了接触等效应力，可以为锤头和滚轮提供一定保护。图6给出了加载力臂中最大应力单元时程曲线，最大应力值为80 MPa，远低于45钢的屈服应力，这表明在加载过程中加载力臂始终处于弹性阶段。图7给出了不同锥角条件下速度和接触应力的拟合曲线，当锥角15°时，加载臂水平方向速度和滚轮接触应力有最小值，而锥角75°时其有最大值；随着锥角增大，加载力臂获得的速度和滚轮接触应力也增大，为了避免滚轮发生塑性变形，锥角不宜过大。基于数值分析结果与讨论，建议十字形锤头的锥角α选择为45°为宜。

图  3  双轴拉伸加载装置的四分之一对称有限元计算模型

Figure  3.  The quarter finite element calculation model for the biaxial tensile loading device

下载: 全尺寸图片 幻灯片

图  4  不同锥角条件下加载力臂速度时程曲线

Figure  4.  Time histories of velocity of the loading force arm at different conical angles

下载: 全尺寸图片 幻灯片

图  5  不同锥角条件下接触单元应力时程曲线

Figure  5.  Time histories of stress of contact elements at different conical angles

下载: 全尺寸图片 幻灯片

图  6  45°锥角下加载力臂上最大单元应力时程曲线

Figure  6.  Time history of the maximum element stress of the loading force arm at the conical angle of 45°

下载: 全尺寸图片 幻灯片

图  7  不同锥角条件下速度和接触应力的拟合曲线

Figure  7.  Fitted curves for velocity and contact stress at different conical angles

下载: 全尺寸图片 幻灯片

1.2   试样设计

1.2.1   几何设计

在双轴拉伸实验中试样设计也是非常重要的^[26]，试样优化目标是在十字形试样的中心区域（简称为标距段）实现均匀应变，并尽量避免标距段之外发生过早失效。原始的十字形试样尺寸如图8(a)所示，这里采用标距段减薄和加载臂上开多条狭缝两种手段来优化试样，优化后的十字形试样几何尺寸如图8(b)所示。中心区域的厚度减小，在外载荷作用下容易在标距段产生大部分变形，同时有助于避免标距段外提早发生失效。在加载臂上开狭缝减少了双轴载荷分配对加载臂的影响，同时这些狭缝也有助于将所施加的载荷均匀地分布到标距段。试样几何设计中的关键参数：狭缝的条数M、狭缝与减薄区边缘长度L和标距段厚度T。下面仍然借助数值模拟手段，对上述3个关键参数进行优化。

图  8  十字形试样几何尺寸

Figure  8.  Geometry sizes of cruciform sample

下载: 全尺寸图片 幻灯片

1.2.2   尺寸优化

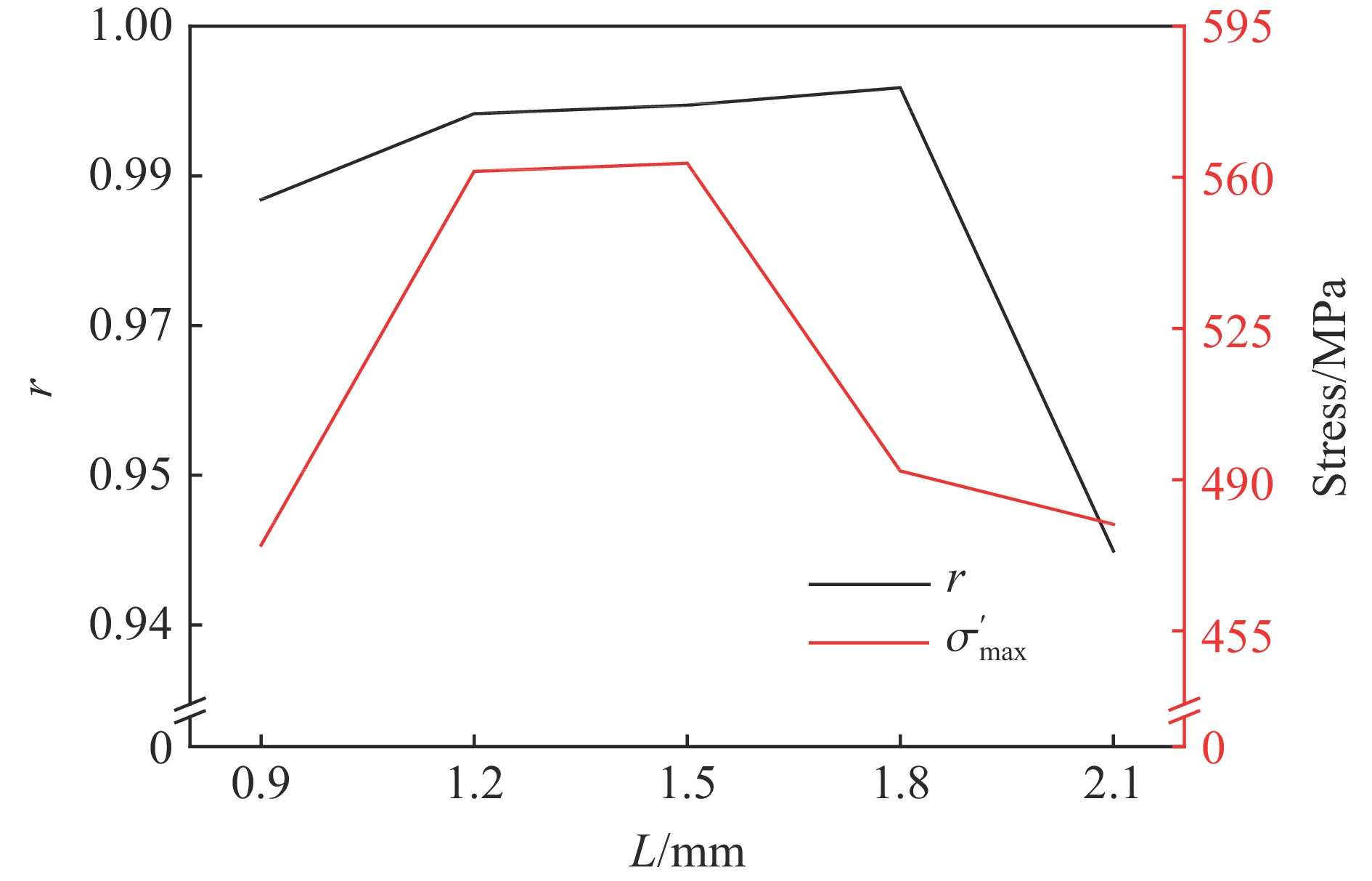
有限元计算模型仍然采用1.1.2节给出的四分之一对称有限元计算模型，材料本构参数和边界条件不变。首先对十字形试样加载臂上狭缝条数M进行优化分析，优化目标是标距段应力均匀分布、中心区和狭缝区存在大变形。这里提出应力集中系数r指标^[27]、标距段平均应力和狭缝区最大应力来定量讨论标距段的应力分布情况，从而确定最优的狭缝条数M、狭缝与减薄区边缘长度L和标距段厚度T。应力集中系数r定义为：

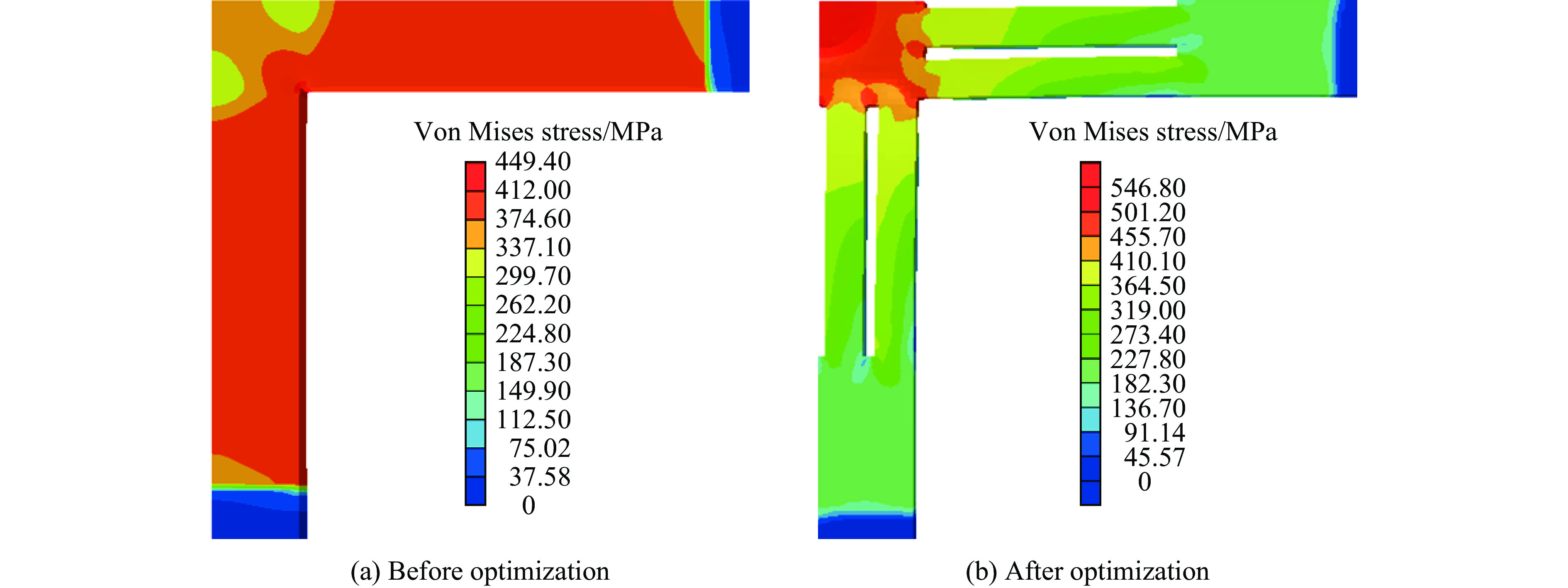
式中：σ_ave为标距段内所选单元平均应力，σ_max为标距段所选单元中最大应力。$\sigma'_{\rm{max}}$为狭缝内单元的最大应力，图9给出了不同M条件下应力在不同空间点的分布曲线，结果显示：随着M增大，应力集中系数减小，系数差值增大，导致应力的均匀性变差，当M=2～3时，应力系数接近极限值1，说明均匀性较好；当M=3时，狭缝区内最大应力$\sigma'_{\rm{max}}$在空间点分布取得最大值，如此狭缝区域表现的应力集中，使得标距段有大变形，同时降低了标距段的横向刚度，其四边获得了最大的拉伸量。因此，M=3是空间点上最合适的值。图10给出了不同L条件下应力在不同空间点的分布曲线，结果显示：随着L增大，应力集中系数先增大后减小；当L=1.5～1.8时，应力系数接近极限值1，应力均匀性最好；当L=1.5时，狭缝内最大应力$\sigma'_{\rm{max}}$为560 MPa优于L为1.8的$\sigma'_{\rm{max}}$为490 MPa，且在空间点分布取得最大值，狭缝区表现出应力集中，从而标距段表现出大变形。因此，L=1.5时标距段内有大变形且应力分布均匀。采用类似方法，对标距段厚度T进行优化，图11不同T条件下应力在不同空间点的分布曲线，当T=0.45 mm，标距段有大变形且应力均匀分布。表1给出了优化后十字形试件的最佳尺寸，而图12给出了优化前后十字形试样的等效应力分布云图对比，结果显示：优化后的十字形试样标距段等效应力分布更均匀，同时加载臂的应力远小于标距段的，这表明优化后的试样满足实验条件。

图  9  不同狭缝条数条件下应力集中系数和狭缝区最大单元应力

Figure  9.  Stress concentration factor of gauge section and maximum element stress of slit under different slit numbers

下载: 全尺寸图片 幻灯片

图  10  不同边缘长度条件下应力集中系数和狭缝区最大单元应

Figure  10.  Stress concentration factor of gauge section and maximum element stress of slit under different lengths of slit edge

下载: 全尺寸图片 幻灯片

图  11  不同标距段厚度条件下应力集中系数和狭缝区最大单元应力

Figure  11.  Stress concentration factor of gauge section and maximum element stress of slit under different thicknesses of gauge section

下载: 全尺寸图片 幻灯片

表  1  优化后十字试件的最佳尺寸参数

Table  1.  The parameters of cruciform samples after optimizing

L/mm	M	T/mm
1.5	3	0.45

下载: 导出CSV 

| 显示表格

图  12  优化前和优化后十字形试样的等效应力分布云图

Figure  12.  Diagrams of equivalent stress distribution of the cruciform sample before and after optimization

下载: 全尺寸图片 幻灯片

1.3   测试技术

在双轴拉伸实验中，采用在夹持导向杆表面合适位置处粘贴普通应变片来直接测量加载力时程曲线，采用非接触光学测量技术来测量试样标距段的应变，测试系统布置如图13所示。实验前需要在试样标距段喷涂黑白散斑，如图14所示，再通过基座下面布置45°反射镜，把散斑图像输入到水平布置的高速相机中，这样就可以获取试样变形过程的系列散斑图像，对获取的散斑图像进行数字图像相关性分析就可以得到试样标距段全场应变。最后，借助数据采集同步器把力时程和基于数字图像相关性分析得到的应变时程同步，消除时间参数后，得到材料的σ-ε实验曲线。

图  13  双轴拉伸实验测试技术示意图

Figure  13.  Schematic diagram of biaxial tensile test technology

下载: 全尺寸图片 幻灯片

图  14  标距段喷涂散斑的试样

Figure  14.  Cruciform sample with speckles pattern on gauge section

下载: 全尺寸图片 幻灯片

2.   双轴拉伸实验装置的可行性检验

2.1   单轴加载检验

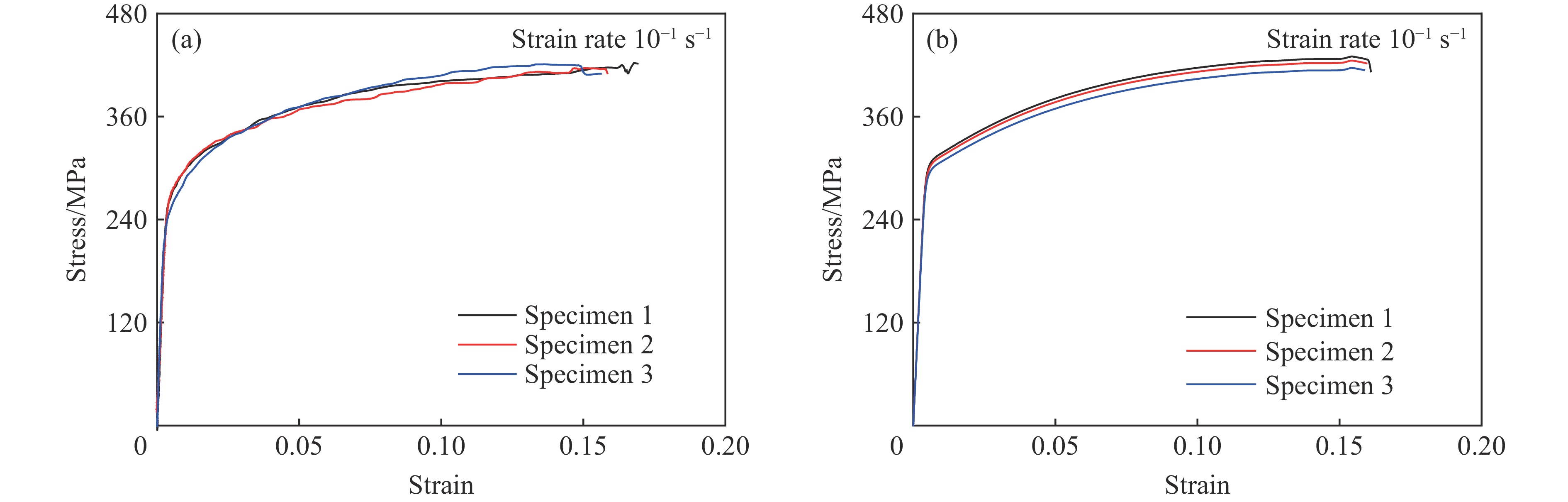
为了进一步验证双轴拉伸实验装置的有效性，这里基于双轴拉伸实验装置来开展准静态单轴拉伸实验，即仅在一对加载力臂上安装普通板条拉伸试样，如图15所示。在准静态加载条件下，HTM-5020液压伺服高速试验机采用闭环控制模式，加载应变率控制为10⁻¹ s⁻¹。利用双轴拉伸加载装置开展单轴拉伸实验，3次重复性实验得到2024-T351铝合金板材的单轴拉伸应力应变曲线，如图16(a)所示。另外，采用Instron5966电子万能材料试验机对同一批2024-T351铝合金板材试样在相同加载应变率条件下也开展3次重复单轴拉伸实验，得到的实验结果也显示在图16(b)中。对比分析两种实验技术得到的应力应变曲线，发现两者之间的一致性是比较好的，由此初步验证了双轴拉伸加载装置的有效性。

图  15  喷涂散斑的铝合金单轴试样

Figure  15.  Aluminum alloy specimen with speckles pattern

下载: 全尺寸图片 幻灯片

图  16  不同实验技术测得铝合金单轴应力应变实验数据对比

Figure  16.  Comparison of stress-strain curves of aluminum alloy specimens by different test techniques

下载: 全尺寸图片 幻灯片

2.2   双轴同步性检验

在双轴拉伸加载过程中保持试样的中心不动是实验有效的必备条件，这就要求在十字形锥面锥头驱动下一条直线上一对加载力臂必须同步相背运动。在上述的利用双轴拉伸装置开展单轴拉伸实验中，同一方向两个测力连接杆测得应力时程曲线对比如图17所示，σ_x1、σ_x2为同一方向应力，两者的一致性很好，验证了同一直线上一对加载力臂相背运动的同步性很好。同时，还需要验证在相互垂直的一对加载力臂必须同步运动，这里，采用双探头的全光纤激光干涉测速仪对加载力臂的运动历程进行监测^[28]，考察其同步性，测试系统布置如图18所示。从两个激光探头中发射的两束激光分别聚焦于一对相邻运动的加载力臂表面，激光在目标表面上发生反射,部分反射光被探头收集,反射信号激光发生多普勒频移，利用多普勒频移f_d和目标运动速度v(t)关系：

图  17  同一方向连接杆上实测的应力时程曲线

Figure  17.  Evolution of stress profiles measured on the connecting rod along the same direction

下载: 全尺寸图片 幻灯片

图  18  双轴拉伸加载装置同步性验证的激光干涉测试系统布置

Figure  18.  Schematic diagram of the laser interference system for verifying synchronism of the biaxial tensile loading device

下载: 全尺寸图片 幻灯片

式中：$\lambda$为激光的波长。对示波器记录的激光干涉信息采用短时傅里叶变换方法进行频域分析，提取出多普勒频移f_d，即可用式(2)计算出被测目标的运动速度。在不夹持十字形试样条件下，锤头以50 mm/s速度撞击4个加载力臂，图19给出一对相邻运动加载力臂的速度和位移时程曲线对比，d_x1、d_y2为相邻方向位移，v_x1、v_y2为相邻方向速度，两者一致性很好，实验验证了相邻加载力臂运动同步性很好。

图  19  相互垂直两个方向实测的位移和速度时程曲线对比

Figure  19.  Comparison of displacement and velocity curves measured along two directions perpendicular to each other

下载: 全尺寸图片 幻灯片

3.   双轴拉伸实验及结果讨论

3.1   2024-T351铝合金板材静态/动态双轴拉伸实验

基于Zwick HTM-5020液压伺服高速试验机搭建的双轴拉伸加载平台如图2所示。Zwick HTM-5020液压伺服高速试验机有闭环控制和开环控制两种模式，闭环控制条件下最大加载速度小于700 mm/s，而开环控制条件下最大加载速度可达20 m/s，因此，这里发展双轴拉伸加载平台既可以施加静态载荷，也可以施加动态载荷，从而研究不同应变率条件下板材双轴拉伸力学性能。这里，以2024-T351铝合金板材为例，双轴拉伸试样几何尺寸见图8(b)，标距段13 mm×13 mm，开展了10⁻¹和10 s⁻¹两种应变率条件下双轴拉伸实验。通过夹持连接杆上粘贴应变片实测试样承受载荷时程信息。采用高速相机来拍摄试样标距段内散斑，10⁻¹ s⁻¹应变率条件下，采集帧率为250 Hz，而10 s⁻¹应变率条件下，采集帧率为3 000 Hz。采用数字图像相关性分析软件VIC-2D对采集的试样散斑图像进行分析，得到试样标距段的全场应变信息。

3.2   实验结果与讨论

3.2.1   双轴拉伸加载力和应变时程曲线

图20给出10⁻¹和10 s⁻¹两种应变率条件下通过夹持连接杆上粘贴的应变片实测力时程曲线，结果显示不同应变率加载下实测的x和y方向加载力实验数据几乎是一致的，这再次验证了4个加载力臂对试样的作用是同步的。另外，两种应变率条件下加载力实测曲线其波前沿和波平面较光滑，不存在振荡，表明了橡胶缓冲层的有效性。

图  20  不同应变率下4个连接杆上应变片实测的力时程曲线

Figure  20.  Evolution of forces measured by strain gauges on four clamping guide rods at different strain rates

下载: 全尺寸图片 幻灯片

3.2.2   双轴拉伸应力-应变曲线

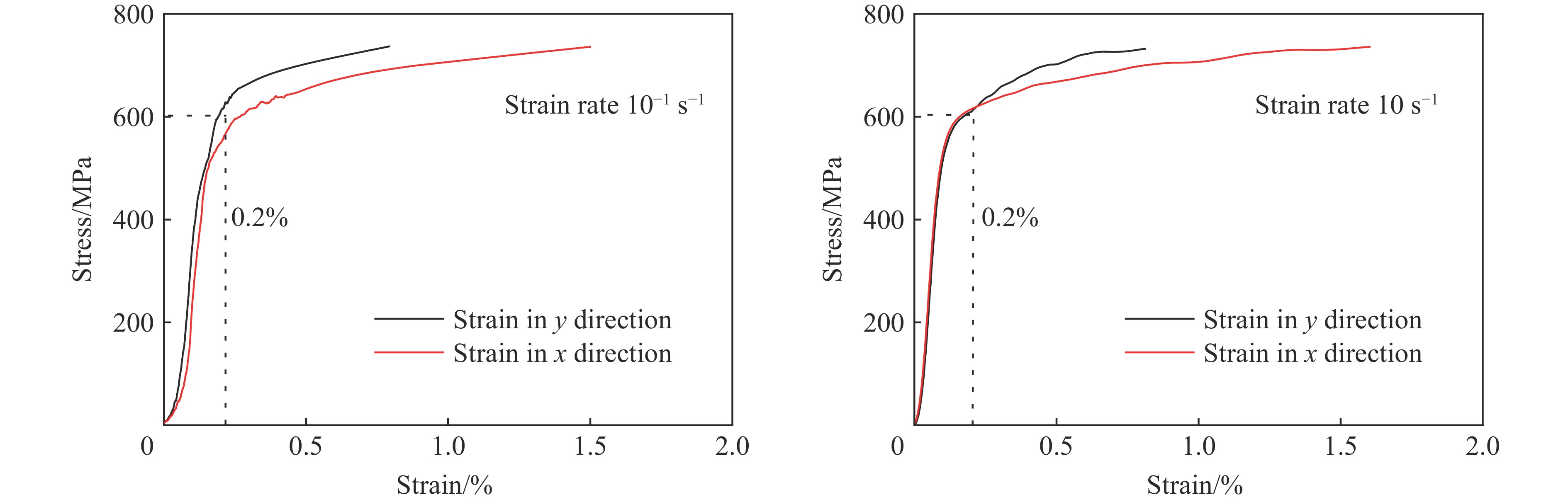
在测力连接杆实测的力时程曲线，见图20和DIC方法实测标距段平均应变时程曲线，见图21，消去时间轴后，得到不同应变率下铝合金双轴拉伸应力应变曲线，如图22所示。结果显示：不同应变率加载下实测的x和y方向应力应变曲线在应变0.2%以内两者具有很好的一致性，这时试样表现为各向同性力学性能，而当应变超过0.2%后两个方向的应力应变曲线出现明显偏差，表明轧制板材力学性能具有一定的各向异性。应变0.2%以内，应力应变之间基本呈线性关系，这时试样基本处于弹性变形阶段，而应变超过0.2%，试样进入塑性变形阶段。同时发现，两种应变率下，双轴加载下铝合金断裂强度约等于单轴加载下的断裂强度的2倍，沿轧制方向(x方向)的断裂应变明显高于垂直轧制方向（y方向）的。图23中给出10⁻¹和10 s⁻¹应变率下铝合金双轴和单轴von Mises等效应力应变曲线对比，结果显示：铝合金在双轴加载下的强化效应非常明显，双轴加载下屈服强度和弹性模量比单轴加载下屈服强度和弹性模量都提高了1倍多。

图  21  不同应变率下DIC实测的标距段平均应变时程曲线

Figure  21.  Average strain measured by the DIC method as a function of time at different strain rates

下载: 全尺寸图片 幻灯片

图  22  不同应变率下铝合金双轴拉伸应力应变曲线

Figure  22.  Stress-strain curves of aluminum alloy at different strain rates under biaxial tensile loading

下载: 全尺寸图片 幻灯片

图  23  不同应变率下铝合金双轴和单轴等效应力应变曲线对比

Figure  23.  Comparison of biaxial and uniaxial equivalent stress-equivalent strain curves of aluminum alloy at different strain rates

下载: 全尺寸图片 幻灯片

4.   结　论

基于Zwick HTM-5020液压伺服高速试验机，发展了一种的具有静态和动态双重功能的双轴拉伸加载系统。采用数值模拟手段对加载系统和试样尺寸进行了设计优化。当锥面接触导向驱动的接触锥角为45°时，既具有较好的驱动转化效率，也容易保证适当的接触应力，实现加载设施重复使用；获得了加载臂狭缝个数M、狭缝与减薄区边缘长度L和标距段厚度T等试样设计关键参数。通过设计单轴加载和双探头激光干涉位移同步性实验，对双轴拉伸加载装置的加载一致性和同步性进行了实验验证，初步证实了双轴拉伸实验装置的可行性和可靠性。利用该双轴拉伸实验装置，开展2024-T351铝合金板双轴拉伸加载实验，获得了10⁻¹ s⁻¹和10 s⁻¹两种应变率条件下材料双轴拉伸应力应变曲线，观察到铝合金板材力学性能存在一定的各向异性，同时与单轴加载下的应力应变数据进行了对比，发现双轴加载下2024-T351铝合金的弹性模量和屈服强度都大幅度提高。