Dynamic responses of metal foam sandwich beams to repeated impacts
-
摘要: 为了研究重复冲击载荷作用下泡沫金属夹芯梁的动态响应,采用Abaqus数值仿真软件,基于可压碎泡沫模型(crushable foam),建立了泡沫金属夹芯梁遭受楔形质量块冲击的有限元模型。通过将仿真获得的夹芯梁上下面板最终挠度与重复冲击实验结果进行对比,验证仿真方法的准确性。在此基础之上,分析了泡沫金属夹芯梁在楔形质量块重复冲击作用下的变形模式、加卸载过程以及能量耗散特性。结果表明,在重复冲击载荷作用下,夹芯梁的变形不断累积,上面板主要出现局部凹陷和整体弯曲,而芯层则是局部压缩,下面板表现为整体弯曲。在重复加卸载过程中,加卸载刚度随着冲击次数的增加而增大。随着冲击次数的增加,上面板和芯层的能量吸收增量不断减小,而下面板的能量吸收增量不断增加,且最终均趋于稳定。泡沫金属夹芯梁的塑性变形能增量不断减小,而回弹系数随着冲击次数逐渐增加,最后趋于稳定值。Abstract: The phenomena of repeated impacts are very common, especial in the field of ship and ocean engineering. When the ship structures suffering from repeated impact loadings, the deformation and damages will accumulate, leading to failure even damage of the structures, which may cause serious accident. In order to study the dynamic behaviors of metal foam sandwich beams (MFSBs) under repeated impact loadings, the nonlinear finite element model was established based on the material model of crushable foam by using Abaqus-Explicit, and the approach to achieve repeated impacts in the software was proposed. The accuracy of the numerical simulation was verified by comparing the permanent deflections of front and back face sheets. Based on the results of the numerical simulations, the deformation modes, loading and unloading process as well as the energy absorption behavior of the MFSBs under repeated impacts were analyzed. Results show that during repeated impacts, the deformation of the MFSBs is accumulated gradually, the front face sheet mainly experiences global bending and local indentation, and the metal foam core suffers from local compression, while the back face sheet is subjected to global bending. During the repeated impacts, the loading and unloading stiffness increases with the impact number. The energy absorption of front face is larger than that of back face and metal foam core in all the impacts. As the impact number increases, the energy absorbed by front face sheet and foam core declines gradually, while that of the back face sheet increases, approaching a constant value. The plastic deformation energy of the MFSBs decreases with the impact number, on the opposite, the rebound energy of the MFSBs increases gradually with the impact number, while both of them trends to be stable. The proposed finite element method can be applied to accurately predict the dynamic responses of the MFSBs suffering from repeated impact loadings, and provide technical supports for the anti-impact design of metal foam sandwich structures.
-
船舶与海洋工程装备在海上航行或作业过程中,经常会遭受重复冲击载荷作用,比如货船在装载货物时船底板受到货物的反复撞击,海洋平台和现代舰船在服役过程中直升机多次降落时对主甲板的反复撞击以及冰区船舶受到浮冰和冰排等的反复撞击等。这些反复碰撞载荷将导致船体结构出现塑性变形累积,最终产生失效破坏,给船舶结构安全带来严重的威胁和极大的挑战。因此,重复冲击问题在船舶与海洋工程领域受到广泛关注,是目前研究的热点。
为了研究船舶重复碰撞问题,Zhu[1]结合理论、数值和实验方法对船体板受质量块重复碰撞响应进行了分析,基于有限差分法开发了数值程序,预测了船体板在重复冲击载荷作用下的最终挠度。为了进一步研究船体板在重复冲击载荷下的动态力学行为,Zhu等[2]对四周刚固矩形板进行了重复碰撞模型实验,获得了船体板最终挠度随冲击次数的变化关系。随后,Zhu等[3-4]对船体结构重复冲击问题展开了系列研究,阐明了重复冲击过程中的船体板的变形损伤累积机理。
高性能船舶正朝着大型化、高速化和多样化的方向发展,将泡沫金属夹芯结构应用于船舶结构设计当中,可以在实现轻量化设计的同时提高船舶结构的安全性。当泡沫金属夹芯结构受到重复冲击载荷时,前面板会率先出现塑性变形,随着冲击次数的增加,芯层逐渐被压缩。在多次冲击作用下,由于大部分冲击能量被前面板和泡沫金属吸收,后面板的变形相对较小,即泡沫金属夹芯结构可以对船体内部结构起到很好的保护作用。因此,泡沫金属夹芯结构在船舶与海洋工程重复冲击防护方面具有重要的应用前景。
由于具有优良的抗冲击性能,泡沫金属夹芯结构已在工程领域得到了广泛的应用。越来越多的学者开展泡沫金属及其夹芯结构的动态力学行为研究[5-6],研究方法主要为实验测试、理论分析和数值仿真。Yu等[7-8]利用Hopkinson杆开展了泡沫铝动态压缩实验,获得了不同应变率下的应力应变曲线,通过对比发现泡沫铝为应变率不敏感材料。在此基础上,进行了静态和动态三点弯曲实验,对泡沫金属夹芯梁的动态响应和失效模式进行了对比分析。结果表明,当加载速率较低(小于5 m/s)时,可以用准静态实验来预测泡沫铝夹芯梁在动态加载下的失效模式。为了进一步探究两端固支夹芯梁在质量块撞击下的动态响应,Tan等[9]设计了相应的实验装置,获得了质量块的加速度时程曲线,并分析了夹芯梁的失效破坏模式。结果显示,在质量块动态冲击作用下,泡沫金属夹芯梁的失效模式以整体弯曲为主。泡沫金属夹芯梁在脉冲载荷作用下和质量块冲击作用下的动态响应存在一定的差异。敬霖等[10]、Jing等[11]利用泡沫铝子弹为撞击体以模拟脉冲载荷加载,研究了泡沫金属夹芯梁在脉冲载荷作用下的失效模式。结果表明,泡沫金属夹芯结构在脉冲载荷作用下的失效模式主要分为非弹性大变形、面板褶皱、芯层剪切和界面失效。
材料本构模型的建立是开展泡沫金属夹芯结构力学行为数值仿真分析的必要条件。为了建立泡沫金属材料的本构模型,Deshpande等[12]开展了泡沫金属材料的准静态单轴压缩实验,在实验中考虑了静水压的影响,获得了初始屈服面,提出了基于各向同性假设的可压缩泡沫金属材料本构模型,即Deshpande-Fleck材料模型。Deshpande-Fleck材料模型已经嵌入到有限元软件当中,在数值仿真中等到广泛使用。Qiu等[13] 基于Deshpande-Fleck材料模型,建立了数值仿真模型,研究了两端固支泡沫金属夹芯梁在冲击波载荷作用下的动态响应,分析了材料弹性和应力强化对结构响应的影响。Tilbrook等[14]在考虑流固耦合作用的情况下,对泡沫金属夹芯梁在水下爆炸载荷作用下的动态响应进行了研究。Jing等[15]建立了数值仿真模型,对梯度泡沫金属夹芯梁的动态冲击响应进行了分析,探讨了夹芯梁的变形及失效模式、能量吸收特性以及边界条件的影响,揭示了梯度泡沫金属夹芯梁动态冲击能量耗散机制。
Qiu等[16]建立了刚塑性理论模型,基于方形屈服面求解了两端固支泡沫金属夹芯梁在局部区域遭受脉冲载荷作用下的塑性动力响应,并将理论模型的分析结果和仿真结果进行了对比,验证了理论模型的可靠性。Qin等[17]基于理想刚塑性假设建立了理论分析模型,对泡沫金属夹芯梁在质量块撞击作用下的塑性动力响应进行了分析,基于方形屈服面获得了无量纲挠度的上下限,理论模型的求解结果与数值仿真结果吻合较好。在此基础上,Qin等[18]在理论建模时,考虑了局部凹陷的影响,探讨了局部凹陷对泡沫金属夹芯梁最终挠度以及能量吸收的影响。结果表明,在理论分析时若不考虑局部凹陷的影响,泡沫金属夹芯梁的承载能力将被高估。
由上述分析可知,目前针对泡沫金属夹芯梁单次冲击动态响应的研究方法相对成熟,理论模型和数值仿真方法均可以较准确地预报夹芯梁的塑性动力响应。然而,由于泡沫金属夹芯梁在遭受重复冲击过程中,其变形和失效模式、加卸载刚度、能量吸收特性等均会随着冲击次数的增加而有所变化,无法简单地用单次冲击的方法来研究重复冲击响应。重复冲击载荷作用下,泡沫金属夹芯结构的变形累积机理、能量耗散机制尚不明确,限制了其在船舶与海洋工程中冲击防护方面的应用。因此,非常有必要开展泡沫金属夹芯结构重复冲击动态力学行为研究。
本文中,将基于Deshpande-Fleck材料模型[12],在Abaqus-Explicit分析模块中建立泡沫金属夹芯梁动态响应弹塑性数值分析模型,利用重启动技术考虑上一次冲击的残余变形对下一次冲击响应的影响。分析泡沫金属夹芯梁的受力状态和变形模式,将数值仿真获得的最终挠度与实验结果进行对比,验证数值仿真模型的有效性。随后,探究重复加卸载过程中的刚度变化以及面板和芯层的能量分配规律。最后,分析泡沫金属夹芯结构的塑性变形能以及回弹能量随冲击次数的变化规律。
1. 数值仿真
1.1 材料属性
在仿真中,低碳钢面板按弹塑性材料模型定义,其中弹性部分定义杨氏模量和泊松比,塑性部分输入拉伸实验获得的塑性应力应变曲线。泡沫金属芯层材料采用可压碎泡沫模型 (crushable foam)[12],该模型也被称为Deshpande-Fleck模型[12],将泡沫金属材料简化为各向同性强化本构模型。依据Deshpande-Fleck模型[12],对于闭孔泡沫金属而言,可以假设弹性泊松比、塑性泊松比、屈服面形状参数以及单轴压缩强度与静水压比值。本文中考虑塑性大变形,而不考虑材料失效。低碳钢和泡沫铝的塑性应力应变曲线如图1所示,其材料力学性能分别为:低碳钢密度为7800 kg/m3,杨氏模量为201 GPa,屈服强度为182 MPa,泊松比为0.3;泡沫金属材料的密度为480 kg/m3,杨氏模量为0.42 GPa,弹性泊松比为0.3,平台应力为10 MPa,塑性泊松比为0,塑性应力比为1.73。
1.2 有限元模型
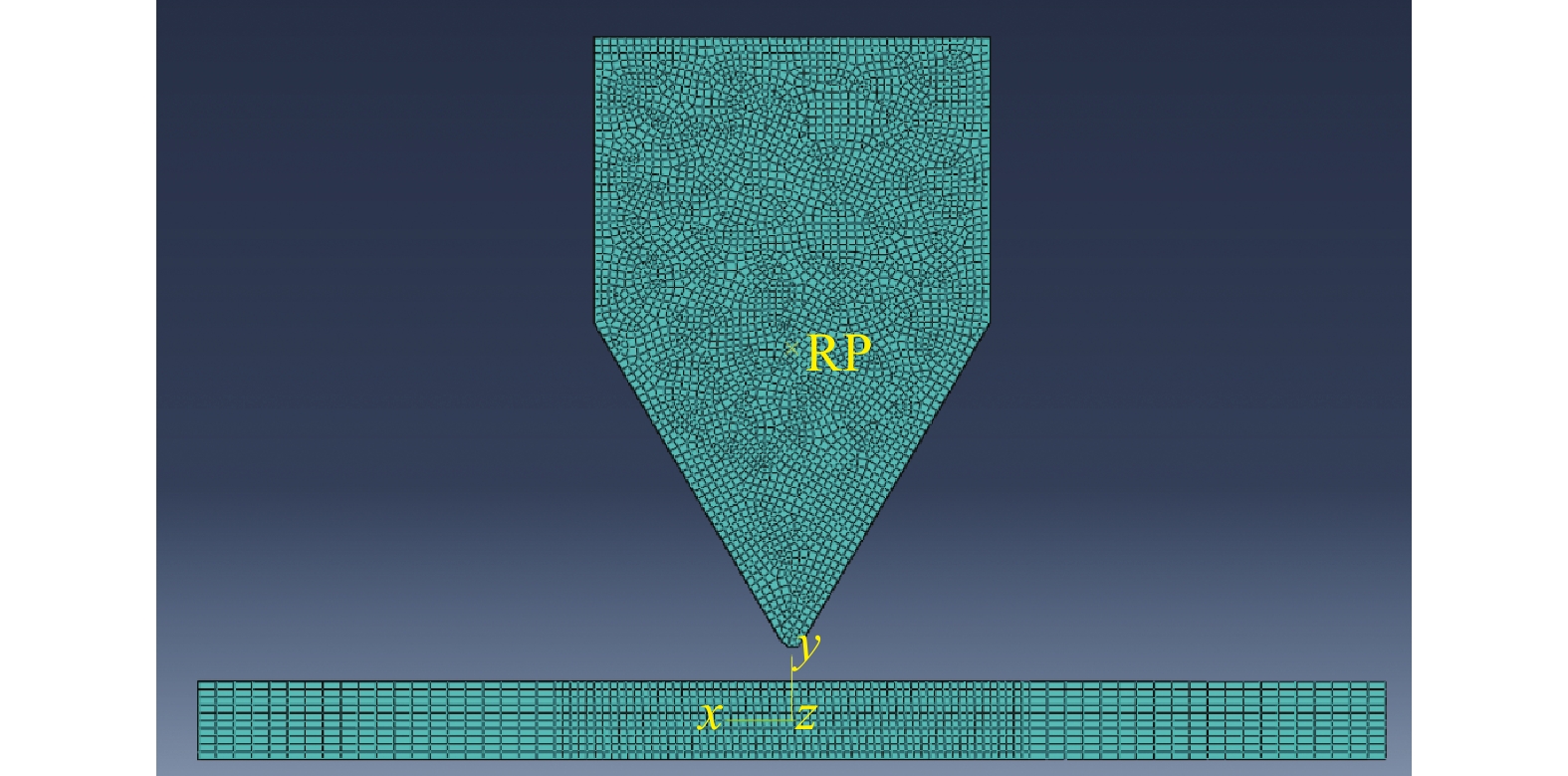
在数值仿真中可仅建立泡沫金属夹芯梁中间区域的几何模型,即夹芯梁的长度L=150 mm,宽度B=30 mm,芯层厚度为10 mm,上、下面板厚度均为1.0 mm。楔形冲头的宽度为40 mm,楔形角为60°,倒角半径R=1.5 mm。
为提高计算效率,并分析在冲击区域产生的局部凹陷情况,需要进行网格局部加密。如图2所示,网格加密范围为梁跨中60 mm区域,网格尺寸为1 mm,非加密区的网格尺寸为2.5 mm。夹芯梁厚度方向的网格大小为1 mm,宽度方向网格大小为1.5 mm。冲头设为离散刚体,采用四边形壳单元(R3D4);芯层采用线性缩减积分六面体单元(C3DR8);上、下面板采用四边形壳单元(S4R)。
在仿真中,夹芯梁边界条件设置为两端固支,限制边界上所有节点的转动和平移。仿真中限制冲头的自由度,只保留竖直方向上的位移。冲头和上面板之间采用面-面接触(surface-to-surface contact),由于摩擦力对能量消耗的贡献非常小,所以在仿真中忽略摩擦力的影响。在仿真中将楔形冲头定义为刚性体并赋予质量7.884 kg,定义冲头竖直向下的初始冲击速度为2.10 m/s,初始冲击能量为17.4 J。
在模拟重复冲击时,需要考虑到泡沫金属夹芯梁的加载和卸载过程,因此需要多次对冲头定义相同的初始冲击速度。为了满足上述条件,需要采用多步分析,通过多个分析步来定义每次冲击的初始冲击速度,同时每个分析步的时间需要设置得足够长,以满足夹芯梁的变形值达到稳定值。每次冲击之后的变形和应力状态都是下一次冲击的初始状态。为了与实验结果进行对比,在仿真当中重复冲击次数设为10次。本文中所选取的冲击能量值适中,在仿真过程中不考虑夹芯梁的断裂破坏。
1.3 数值仿真方法验证
利用数值仿真,可以对泡沫金属夹芯梁重复冲击过程进行可视化分析,而数值仿真的可靠性需要进行对比验证。
实验使用的装置是Instron9350冲击试验机,如图3(a)所示。Instron9350落体冲击试验机,为落地式试验系统,冲击速度为0.75~24 m/s,最大冲击质量为70 kg,冲击能量范围为 0.59~1800 J。与Instron9350冲击试验机连接的数据采集仪是Das 64K,可实时采集数据。力传感器为应变式,其量程为0~90 kN,内置于冲头内部,可以监测冲头受到的瞬态冲击力。
实验所用到的夹具由上夹具和下夹具组成,上、下夹具之间通过螺栓连接,以实现固支边界条件,夹具的下方与基座相连,如图3(b)所示。实验使用楔形冲头,冲头通过螺纹与冲击连杆相连。冲头首部的楔形角为60°,楔形宽度为50 mm。楔形冲头的质量为7.884 kg,冲击速度为2.10 m/s,即冲击能量为17.4 J。
仿真和实验中获得的泡沫铝夹芯梁的变形轮廓对比情况如图4所示。从图4可以看出,仿真和实验中均可以观察到上面板出现整体弯曲和局部凹陷。对于不同冲击次数,仿真中得到的整体变形、局部凹陷的形状和大小与实验中获得的结果基本一致,并且塑性铰的位置也相同。图5是实验和仿真的冲击力时程曲线对比图。从图5(a)可以看出,对于第1次冲击,仿真和实验的冲击力随时间变化趋势基本一致,冲击力大小也基本相同。而从图5(b)可以看出,对于第4次冲击,仿真当中的冲击力峰值大于实验,而仿真中的冲击持续时间小于实验。出现上述现象的主要原因是:在实验当中,夹芯梁两端边界是由螺栓进行固定的,每次冲击过程中,螺栓会产生一定弹性变形,即边界具有一定的弹性;而在仿真当中,边界假设为两端固定。因此,实验当中夹芯梁的刚度小于仿真,即仿真的冲击力峰值大于实验的,且随着冲击次数的增加,两者的差距逐渐增加。
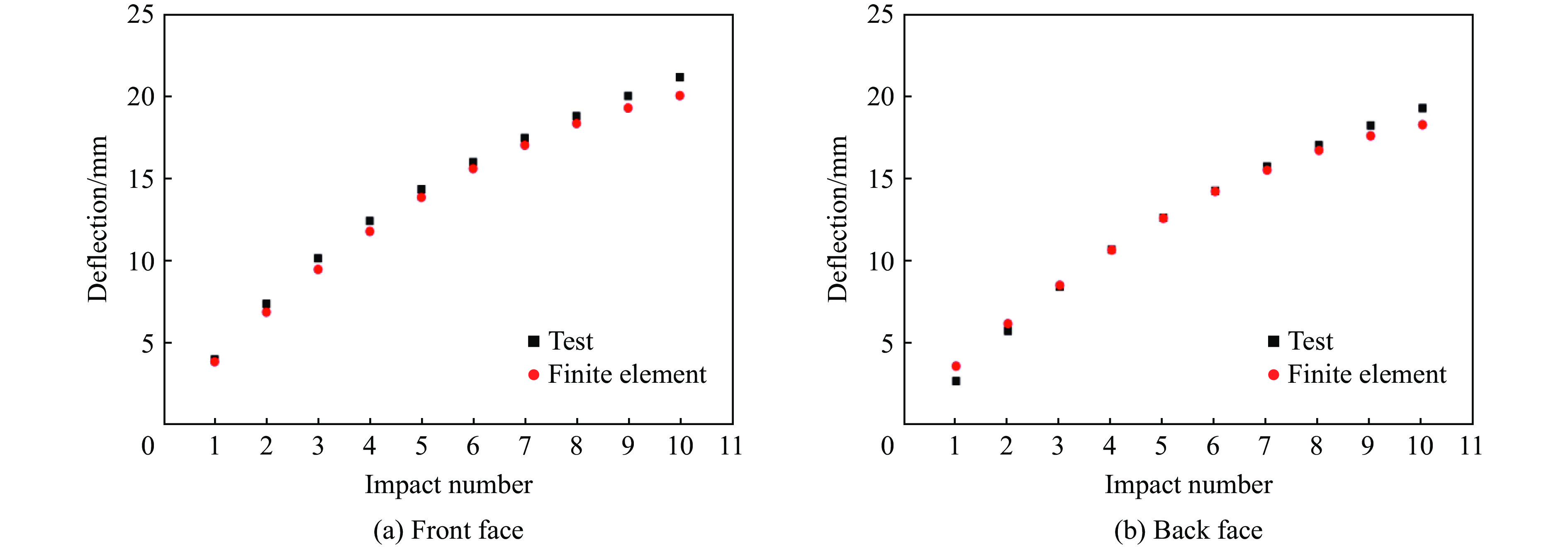
图6是不同冲击次数下夹芯梁的上下面板挠度的仿真结果和实验结果的对比。从图6可以看出,上下面板的最终挠度均随着冲击次数的增加而不断增大,而增长速率不断减小,仿真和实验的变化规律基本一致。同时,仿真和实验的最终挠度值也基本相同,最大偏差仅为5.6%。通过上述分析可知,仿真结果与实验结果吻合较好。因此,可以用非线性有限元方法模拟泡沫金属夹芯梁遭受质量块重复冲击的动态响应过程,并准确的预测夹芯梁上下面板的变形和最终挠度值。
2. 结果与讨论
2.1 重复冲击动态响应
数值仿真中可以获得不同时刻的结构响应,可以对泡沫金属夹芯梁重复冲击过程进行可视化分析。如图7所示,随着冲击次数的增加,上、下面板的变形及最终挠度均不断增大。除了出现整体弯曲变形之外,夹芯梁上面板在冲击处还会出现局部凹陷。
夹芯梁的变形模式和受力状态如图8所示(以第5次冲击为例)。从图8可以看出,夹芯梁跨中至边界间的位移近似呈线性分布。对于上面板而言,分别在冲击处、局部凹陷两端、边界两端出现塑性铰。对于下面板而言,分别在夹芯梁跨中、边界两端出现塑性铰。而对于芯层而言,分别在梁跨中和边界两端出现塑性铰,除了塑性弯曲之外,芯层还出现了局部压缩变形。通过对比可以发现,泡沫金属夹芯梁上面板和下面板的变形模式存在一定的差异,主要原因是二者的受力状态不同。上面板的上表面受到楔形冲头的集中力作用及芯层的支撑,而下表面则受到芯层的分布力的加载。因为泡沫金属的压缩强度比低碳钢面板小很多,所以上面板在受到质量块冲击时,其变形是整体弯曲伴随局部凹陷。而下面板的上表面受到泡沫金属向下的分布力,发生整体弯曲变形。
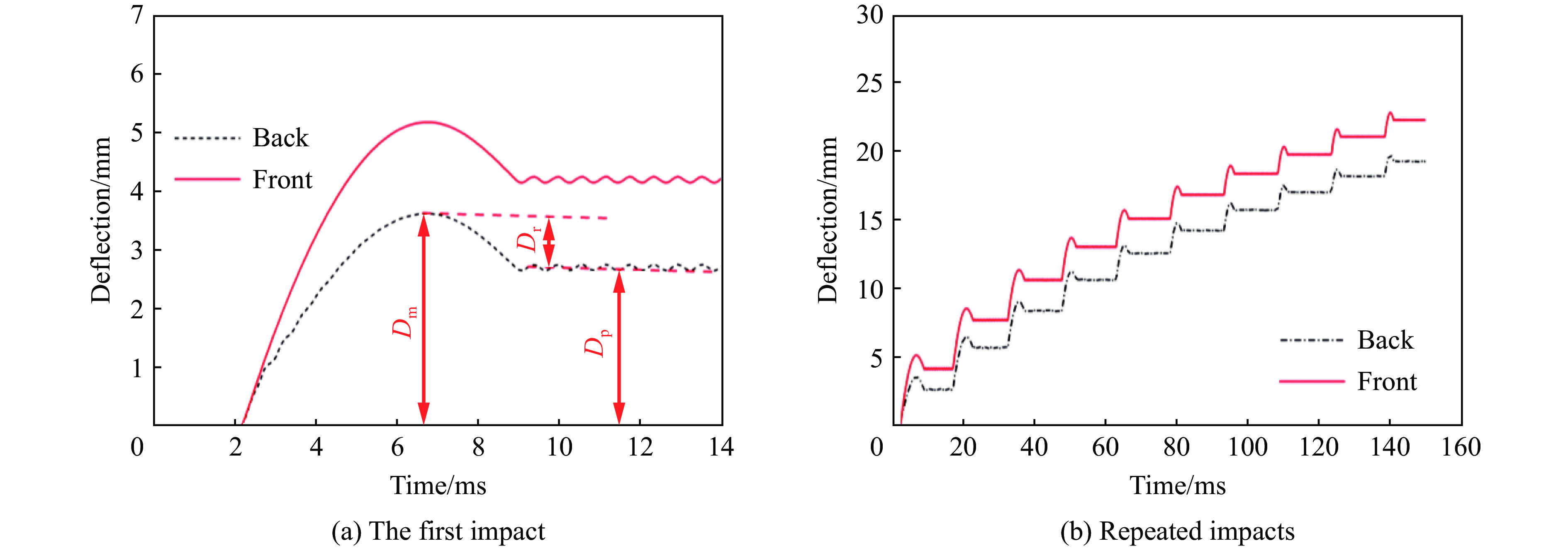
重复冲击作用下泡沫金属夹芯梁面板的挠度时程曲线如图9所示,图中Dm为最大挠度,Dr为反弹挠度,Dp为最终挠度。从图9可以看出,对于每次冲击而言,夹芯梁的变形都可分为3个阶段。第1阶段,随着时间的增长,面板挠度不断增大,直至达到最大值;第2阶段,面板的挠度随着冲击时间的增长而不断减小,直至冲头与上面板分离便不再减小;第3阶段,冲头与上面板分离之后,夹芯梁发生弹性振动,因此上、下面板的挠度值在某一范围内微小波动。由于仿真中没有设置阻尼,因此弹性振动不会衰减。由于第3阶段的时间设置得较长,因此夹芯梁的受力状态基本达到稳定,不会对下一次冲击造成影响。面板挠度增大,夹芯梁处于加载阶段,而挠度减小则对应于卸载阶段。在卸载阶段,夹芯梁弹性能释放,冲头出现回弹现象。
为了研究泡沫金属夹芯梁重复冲击加卸载过程,对力位移曲线进行分析。从图10可以看出,冲击过程可以分为加载阶段和卸载阶段,其中加载阶段又分为弹性加载段和塑性加载段,卸载段为弹性卸载。在加载阶段,弹性加载刚度大于塑性加载刚度。如图10(a)所示,对于第1次冲击和第2次冲击而言,都有塑性加载刚度小于弹性加载刚度,即K12<K11和K22<K21。第1次冲击的弹性卸载刚度等于第2次冲击的弹性加载刚度,即K13=K21;而第2次冲击的卸载刚度大于第1次冲击的卸载刚度,即K23>K13。从图10(b)可以看出,上述规律在重复冲击中普遍存在,即在重复冲击过程中,夹芯梁的刚度不断增加。原因主要有两个方面,一方面是随着梁变形的增大,梁的中性轴与水平面的夹角增大,因此梁的轴向拉力在竖直方向的分量也增大,导致加载刚度随着冲击次数的增加而增大;另一方面,由于面板材料在塑性屈服之后,会出现应变强化,因此塑性加载刚度也会随着冲击次数的增加而增大。
2.2 能量吸收与回弹效应
图11是重复冲击作用下泡沫金属夹芯梁各部分的变形能时程曲线。从图11可以看出,随着冲击次数的增加,外界输入能量不断累积,泡沫金属夹芯梁各部分所储存的变形能也相应的不断增加。而对于每次冲击而言,泡沫金属夹芯梁各个部分耗散的能量并不相同。
图12是上、下面板和芯层吸收能量与冲击次数间的关系。从图12可以看出,随着冲击次数的增加,上面板和芯层单次冲击吸收的能量不断减小,而下面板吸收的能量则不断增大,其变化速率不断减小。对于前几次冲击而言,随着冲击次数的增加,芯层局部凹陷不断增大,整体变形和局部凹陷同时发生且相互耦合,导致各部分吸收的能量随着冲击次数不断变化。但是,当泡沫金属压缩到一定程度之后,夹芯梁的变形模式几乎只有整体弯曲变形,因此随着冲击次数的增加,面板及芯层单次冲击吸收的能量保持稳定。
夹芯梁能量的消耗主要分为两部分,即弹性变形能和塑性变形能。弹性变形能可以恢复,一部分将转化为冲头的动能,使冲头以一定的速度反弹;另一部分则转化为夹芯板的动能,使其在平衡位置附近发生弹性振动。从夹芯梁的变形能时程曲线(见图11)可以看出:当冲头反弹之后,夹芯梁的塑性变形能波动非常小,塑性变形能的值基本趋于稳定,即夹芯板的弹性振动非常小。因此,在本文的分析当中,假设冲头的反弹动能等于夹芯板的弹性变形能:
ΔEk=12mv2i−12mv2r (1) 式中:m为冲头质量,vi为冲击速度,vr为反弹速度,
ΔEk 为夹芯梁吸收的能量。如图13(a)所示,冲头的反弹速度随着冲击次数的增加而增大,且可以发现从第8次冲击开始,反弹速度的增长速率不断减小。利用式(1)所示的动能定理,可以近似地由初始冲击速度和反弹速度计算夹芯梁吸收的能量。如图13(b)所示,夹芯梁吸收的能量随着冲击次数的增加而减小,而其存储的弹性能则随着冲击次数的增大而增大,其增长速率先增大后减小,弹性能的值逐渐趋于稳定。
为了分析泡沫金属夹芯梁的能量吸收特性,在此定义能量吸收效率
Ea 和回弹因数Rc :Ea=(Ei−Er)/Ei,Rc=v2r/v2i (2) 式中:Ei为冲击能量,Er为反弹能量。
如图14所示,夹芯梁的能量吸收效率随着冲击次数的增大而减小,且变化速率先增加后减小,其值在0.91与0.82之间。在多次冲击之后,反弹能量积累量会不断累积,因此在分析过程中不能忽略泡沫金属夹芯梁的回弹效应。从图15可以看出,回弹因数随着冲击次数的增加而增大,但其增长速率先增加后减小,最后逐渐趋于稳定。即当冲击次数足够大时,回弹因数趋于0.18。
3. 结 论
利用非线性有限元软件ABAQUS/Explicit,建立了泡沫金属夹芯梁的数值分析模型,研究了泡沫金属夹芯梁重复冲击动态响应和能量吸收特性,得到的结论如下。
(1)泡沫金属夹芯梁在重复冲击过程中,其变形不断累积。上下面板的最终挠度均随着冲击次数的增加而不断增大,而增长速率不断减小。夹芯梁跨中至边界间的位移近似呈线性分布,夹芯梁产生整体变形的同时伴随着局部凹陷,上面板主要出现局部凹陷和整体弯曲,而芯层则是局部压缩,下面板表现为整体弯曲。
(2)泡沫金属夹芯梁在重复加卸载过程中,弹性加载刚度大于塑性加载刚度。前一次冲击的弹性卸载刚度与下一次冲击的弹性加载刚度几乎相同,且加载刚度和卸载刚度均随着冲击次数的增加而不断增大。
(3)随着冲击次数的增加,上面板和芯层的能量吸收增量不断减小,而下面板的能量吸收增量不断增加,且最终均趋于稳定。此外,泡沫金属夹芯梁的塑性变形能增量逐渐减小,而夹芯梁的弹性变形能逐渐增大,但两者的变化速率均先增加后减小。回弹系数随着冲击次数的增加不断增大,当冲击次数较大时,其值趋于0.18。
-
-
[1] ZHU L. Dynamic inelastic behaviour of ship plates in collision [D]. Glasgow: University of Glasgow, 1990: 45−56. [2] ZHU L. Faulkner D. Damage estimate for plating of ships and platforms under repeated impacts [J]. Marine Structures, 1996, 9(9): 697–720. DOI: 10.1016/0951-8339(95)00018-6. [3] ZHU L, CAI W, CHEN M S, et al. Dynamic analysis of ship plates under repeated ice floes impacts based on a simplified ship-ice collision model [C]//Proceedings of the 28th International Ocean and Polar Engineering Conference. Sapporo, Japan: International Society of Offshore and Polar Engineers, 2018: 1718−1723. [4] ZHU L, SHI S Y, JONES N. Dynamic response of stiffened plates under repeated impacts [J]. International Journal of Impact Engineering, 2018, 117: 113–122. DOI: 10.1016/j.ijimpeng.2018.03.006. [5] 杨宝, 汤立群, 刘逸平, 等. 冲击条件下泡沫铝的细观变形特征分析 [J]. 爆炸与冲击, 2012, 32(4): 399–403. DOI: 10.11883/1001-1455(2012)04-0399-05.YANG B, TANG L Q, LIU Y P, et al. Meso deformation characteristics analysis of aluminum foam under impact [J]. Explosion and Shock Waves, 2012, 32(4): 399–403. DOI: 10.11883/1001-1455(2012)04-0399-05. [6] 王鹏飞, 徐松林, 李志斌, 等. 高温下轻质泡沫铝动态力学性能实验 [J]. 爆炸与冲击, 2014, 34(4): 433–438. DOI: 10.11883/1001-1455(2014)04-0433-06.WANG P F, XU S L, LI Z B, et al. An experimental study on dynamic mechanical property of ultra-light aluminum foam under high temperatures [J]. Explosion and Shock Waves, 2014, 34(4): 433–438. DOI: 10.11883/1001-1455(2014)04-0433-06. [7] YU J L, WANG X, WEI Z G, et al. Deformation and failure mechanism of dynamically loaded sandwich beams with aluminum-foam core [J]. International Journal of Impact Engineering, 2003, 28(3): 331–347. DOI: 10.1016/S0734-743X(02)00053-2. [8] YU J L, WANG E H, LI J R, et al. Static and low-velocity impact behavior of sandwich beams with closed-cell aluminum-foam core in three-point bending [J]. International Journal of Impact Engineering, 2008, 35(8): 885–894. DOI: 10.1016/j.ijimpeng.2008.01.006. [9] TAN Z H, LUO H H, LONG W G, et al. Dynamic response of clamped sandwich beam with aluminum alloy foam core subjected to impact loading [J]. Composites Part B: Engineering, 2013, 46: 39–45. DOI: 10.1016/j.compositesb.2012.10.044. [10] 敬霖, 王志华, 赵隆茂, 等. 撞击载荷下泡沫铝夹芯梁的塑性动力响应 [J]. 爆炸与冲击, 2010, 30(6): 561–568. DOI: 10.11883/1001-1455(2010)06-0561-08.JING L, WANG Z H, ZHAO L M, et al. Dynamic plastic response of foam sandwich beams subjected to impact loading [J]. Explosion and Shock Waves, 2010, 30(6): 561–568. DOI: 10.11883/1001-1455(2010)06-0561-08. [11] JING L, WANG Z H, NING J G, et al. The dynamic response of sandwich beams with open-cell metal foam cores [J]. Composites Part B: Engineering, 2011, 42(1): 1–10. DOI: 10.1016/j.compositesb.2010.09.024. [12] DESHPANDE V S, FLECK N A. Isotropic constitutive models for metallic foams [J]. Journal of the Mechanics and Physics of Solids, 2000, 48(6−7): 1253–1283. DOI: 10.1016/S0022-5096(99)00082-4. [13] QIU X M, DESHPANDE V S, FLECK N A. Finite element analysis of the dynamic response of clamped sandwich beams subject to shock loading [J]. European Journal of Mechanics: A/Solids, 2003, 22(6): 801–814. DOI: 10.1016/j.euromechsol.2003.09.002. [14] TILBROOK M T, DESHPANDE V S, FLECK N A. Underwater blast loading of sandwich beams: regimes of behaviour [J]. International Journal of Solids and Structures, 2009, 46(17): 3209–3221. DOI: 10.1016/j.ijsolstr.2009.04.012. [15] JING L, SU X Y, CHEN D, et al. Experimental and numerical study of sandwich beams with layered-gradient foam cores under low-velocity impact [J]. Thin-Walled Structures, 2019, 135: 227–244. DOI: 10.1016/j.tws.2018.11.011. [16] QIU X M, DESHPANDE V S, FLECK N A. Impulsive loading of clamped monolithic and sandwich beams over a central patch [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(5): 1015–1046. DOI: 10.1016/j.jmps.2004.12.004. [17] QIN Q H, WANG T J. Low-velocity heavy-mass impact response of slender metal foam core sandwich beam [J]. Composite Structures, 2011, 93(6): 1526–1537. DOI: 10.1016/j.compstruct.2010.11.018. [18] QIN Q H, WANG T J. Low-velocity impact response of fully clamped metal foam core sandwich beam incorporating local denting effect [J]. Composite Structures, 2013, 96: 346–356. DOI: 10.1016/j.compstruct.2012.09.024. 期刊类型引用(8)
1. 张晓阳,谭仕锋,刘泽宇,赵飘. 恒定高应变率拉伸条件下泡沫金属力学性能. 爆炸与冲击. 2024(01): 108-116 .  本站查看
本站查看2. 张晓阳,赵飘,刘泽宇,陈国豪,王治樵. 不同侧限及荷载比例工况下泡沫金属冲击性能研究. 南华大学学报(自然科学版). 2024(01): 46-52 .  百度学术
百度学术3. 王飞龙,李强. 泡沫铝填充铣孔加工参数优化及表面质量验证. 机械设计与制造. 2024(08): 166-169+173 .  百度学术
百度学术4. 朱浩霖,张天辉,刘志芳. 重复冲击载荷下泡沫铝夹芯壳的动态响应. 高压物理学报. 2024(05): 228-240 .  百度学术
百度学术5. 郭开岭,张义江,穆梦颖,朱凌. 基于膜力因子法的金属梁低速重复冲击塑性响应研究(英文). 船舶力学. 2024(12): 1891-1907 .  百度学术
百度学术6. 肖珍,吴晓. 夹芯梁在重物低速冲击下的动力计算分析. 工程与试验. 2023(03): 1-3+58 .  百度学术
百度学术7. 郭开岭,穆梦颖,朱凌,丁圆圆. 泡沫金属夹芯梁在低速重复冲击下的塑性响应分析(英文). 船舶力学. 2023(12): 1812-1826 .  百度学术
百度学术8. 陈松,习会峰,黄世清,王博伟,王小刚. 软基体混合胞孔材料的力学性能及抗多次冲击性能. 爆炸与冲击. 2022(06): 62-70 .  本站查看
本站查看其他类型引用(3)
-








 下载:
下载:














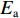





 下载:
下载:














 百度学术
百度学术