Crashworthiness analysis and optimization of bionic corrugated sandwich structures
-
摘要: 为提高薄壁夹层结构耐撞性，以虾螯为仿生原型，设计梯度分布的仿生波纹形夹层结构，包括单层、双层和三层波纹结构。以初始峰值载荷Fp、比吸能Es为耐撞性指标，利用有限元法分析了单元高宽比γ（γ1、γ2和γ3分别为单元第1层、第2层和第3层的高宽比）对波纹夹层结构耐撞性的影响，采用多目标粒子群优化方法得到了夹层结构最优参数。结果表明，单层波纹结构耐撞性随单元高宽比γ的增大逐渐变差，双层波纹结构下层结构单元高宽比γ对耐撞性的影响大于上层结构单元高宽比γ对耐撞性的影响，较小的γ值有利于提高三层波纹结构的比吸能。结构优化结果表明：单层结构最优尺寸γ1为0.8；双层结构最优尺寸为γ1 = 0.5和γ2 = 1.2；三层结构最优组合为γ1 = 0.6，γ2 = 0.6和γ3 = 0.9。上述结果可为薄壁夹层结构轻量化设计提供新思路。Abstract: To improve the crashworthiness of thin-walled sandwich structures, a series of bionic corrugated sandwich structures with triangular elements (the height-to-width ratios of the elements are defined as γ) were designed inspired by the structures of shrimp chelas, including single-layer, double-layer and three-layer corrugated sandwich structures (γ1, γ2 and γ3 denotes the height-to-width ratios of single-layer, double-layer and three-layer elements, respectively). To analyze the deformation and mechanical response of the bionic thin-walled structures, the finite element method was adopted based on LS-DYNA and HyperMesh. By taking the initial peak load Fp and specific energy absorption Es as crashworthiness indexes, the influences of γ on the crashworthiness of the corrugated sandwich structures were discussed. The crashworthiness of the single-layer sandwich structures becomes worse gradually when the parameters γ exceed a certain value. For the double-layer sandwich structures, the influences of the parameters γ2 in the lower layers on the crashworthiness is greater than that of the parameters γ1 in the upper layers.. The Fp decreases by 37.8% with the increase of γ2, which means the greater γ2 of the lower layer is beneficial to improve the crashworthiness of the structure. For the three-layer sandwich structures, the influence of γ on crashworthiness indexes was investigated by range and variance analysis methods. The results show that the γ3 has the most significant influence on Es, and the significance level reaches 0.1. Finally, the optimal parameters of the bionic corrugated sandwich structures were obtained by using the multi-objective particle swarm optimization method based on a polynomial regression (PR) meta model. The optimal results show that the crashworthiness of the single-layer corrugated sandwich structures improves with the increase of γ. For the double-layer corrugated sandwich structures, The γ of the lower layer affects the crashworthiness more significantly than the γ of the upper layer. The three-layer corrugated sandwich structure with lower γ has higher Es. The optimal dimensions of the single-layer, double-layer and three-layer structures are γ = 0.8; γ1 = 0.5 and γ2 = 1.2; γ1 = 0.6, γ2 = 0.6 and γ3 = 0.9, respectively. The above results are helpful for the design of the thin-walled sandwich structures.
-
Key words:
- thin-walled sandwich structure /
- crashworthiness /
- structure optimization /
- engineering bionics /
- impact
-
破甲战斗部是毁伤装甲目标的利器，然而，随着装甲防护由单层化向多层化发展，传统实心聚能装药形成的射流在对抗多层防护目标时的毁伤效果受到了极大限制。为此，有学者提出采用串联战斗部对多层防护目标进行毁伤[1]。串联战斗部通常由2～3个附属战斗部组成，并按作用顺序分为前级、中级和后级。在对抗多层防护目标时，前级战斗部在外层防护装甲上造成大口径通孔，后级战斗部通过通孔对内层装甲进行毁伤[2-3]。因此，前级战斗部的扩孔能力直接决定了串联战斗部的整体毁伤效果。Leidel[4]提出可以采用装药的聚能效应形成环形毁伤元，从而实现大口径、大穿深的侵彻效果。
对环形聚能装药的研究已有很多。根据装药结构的不同，环形聚能装药可以分为实心环形聚能装药和中空环形聚能装药[5]。针对实心环形聚能装药结构，王成等[6]提出了等冲量设计法，通过将药型罩外壁进行逐段变壁厚处理，使炸药作用于药型罩内、外相应微元上的冲量相等。徐文龙等[7-9]设计了一种中心开孔式环形装药结构，研究了不同结构因素及药型罩材料对射流轴向速度和径向速度的影响规律，该结构形成的环形EFP（explosively formed projectile）除了具有良好的飞行稳定性之外，还具有较强的侵彻能力。相较实心环形聚能装药结构，中空环形聚能装药在形成相同口径开孔的同时，所需药量更小。曹涛等[10-11]研究了起爆点数量、药型罩形状、曲率半径等参数对环形聚能装药成型和侵彻效果的影响。王伟力等[12]对环形装药的药型罩、起爆方式和炸高等方案进行了优化设计。段嘉庆等[13]优化了内外罩等动量方法，提出了药型罩同步压垮的设计方法，该方法形成的射流不易偏斜，能够大大改善射流成型效果。何降润等[14]讨论了内外壳体材料相同时，壳体厚度比对环形射流侵彻性能的影响规律。
然而，中空环形聚能装药结构在形成环形侵彻体时，内侧壳体在爆轰产物作用下也会在装药中轴线上汇聚，形成向弹体前、后两个方向运动的中心侵彻体[15-17]。向前运动的毁伤元能够增强对装甲目标的毁伤效果，但向后运动的毁伤元会对后级战斗部的结构造成破坏。为了减小中心侵彻体侵彻威力的同时增加环形射流毁伤威力，本文中对比了四种不同的壳体材料组合下，中空环形聚能装药形成的中心侵彻体对后端靶板的侵彻威力，基于中心侵彻体侵彻深度最小的壳体材料组合，研究药型罩偏心距离和壁厚对环形射流成型和侵彻特性的影响规律，并调整不同炸高，提升环形射流的侵彻威力。基于优化的装药结构及炸高，设计环形聚能装药静破甲试验，验证本文数值模拟结果的准确性。
1. 非对称中空环形聚能装药模型
1.1 几何模型
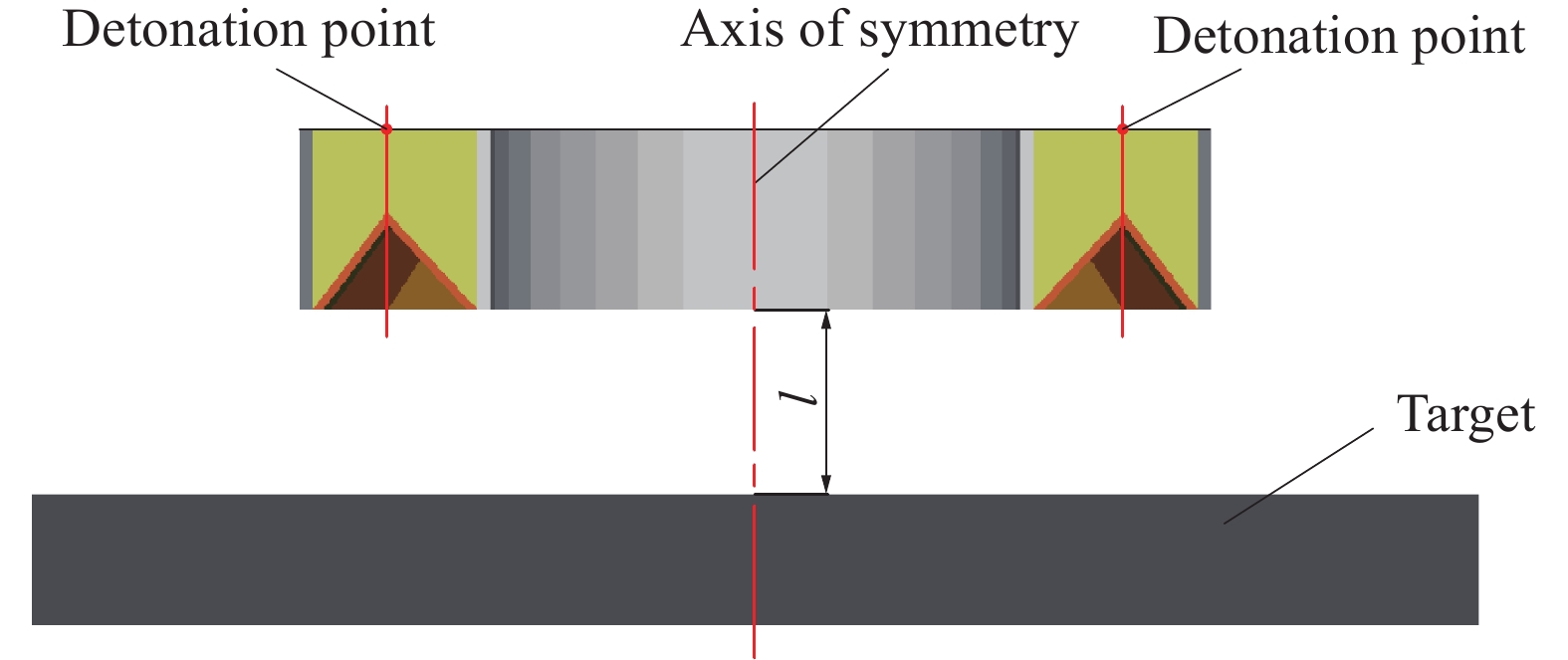
设计的非对称中空环形聚能装药结构如图1所示，非对称指药型罩的横截面形状不关于罩顶呈镜面对称，通过调整锥顶位置改变药型罩和装药的质量分布，通过控制罩顶的移动距离Δr实现对环锥罩结构的调整，将适配于这种偏心环锥罩的装药结构命名为非对称中空环形聚能装药结构。基于此结构，研究环形聚能装药结构对射流成型和侵彻威力的影响规律。
装药的径向厚度为d，装药高度为g；药型罩为等壁厚结构，壁厚为b，研究过程中保持药型罩高度f不变；装药起爆方式为顶部环形起爆，起爆环直径为e，与环锥罩顶直径相同，炸高为l；内壳厚度为ci，外壳厚度为co。
1.2 数值模拟模型
采用AUTODYN软件对环形射流的成型过程进行数值模拟。模型由装药、内外壳体、药型罩和靶板组成，药型罩壁厚b=0.05d，内外壳体壁厚ci=co=0.08d。环形装药部分采用多物质欧拉算法进行计算，欧拉域边界设置流出边界条件，模拟装药在空旷场情景下的爆轰过程。靶板采用拉格朗日算法进行计算。根据射流成型网格收敛性研究[18]，本文网格尺寸选为0.25 mm×0.25 mm。为了提高数值模拟研究的计算效率，建立二维轴对称模型进行计算。为便于对模型进行展示，将二维模型沿对称轴旋转180°，所得中空环形聚能装药的有限元模型如图2所示。
装药选用PBX-9404炸药，采用JWL状态方程描述其爆炸过程，材料参数如表1所示，其中ρ为材料密度，A、B、R1、R2、ω为状态方程参数，vD为炸药爆速，E0为炸药的初始比内能，pCJ为炸药爆压。药型罩材料高导无氧铜和壳体材料4340钢均采用Linear状态方程和Johnson-Cook强度模型来描述材料在高温高压下的变形，材料参数如表2所示，其中K为体积模量，G为剪切模量，A1、B1、n、C、m均为与材料自身性质有关的参数。靶板材料为均质装甲钢，壳体材料为铝合金，靶板和壳体均采用Shock状态方程描述，材料参数如表3所示，其中γ为Grüneisen系数，c1为材料声速，S1为常数。在计算过程中，对靶板材料设置了Johnson-Cook失效模型[19]，模拟靶板的失效现象，失效参数如表4所示，D1、D2、D3、D4、D5为材料断裂毁伤参数。
表 1 装药JWL状态方程参数Table 1. 1JWL equation of state parameters of PBX-9404 ρ/(kg·m−3) A/GPa B/GPa R1 R2 ω vD/(m·s−1) E0/(kJ·m−3) pCJ/GPa 1 840 852.4 18.02 4.6 1.3 0.38 8 800 1.02e7 37 表 2 药型罩和壳体材料参数Table 2. Material parameters of liner and casing材料 ρ/(kg·m−3) K/GPa G/GPa A1/MPa B1/MPa n C m 高导无氧铜 8 960 129 46 90 292 0.31 0.025 1.09 4340钢 7 830 159 81.8 792 510 0.26 0.014 1.03 表 3 靶板和壳体材料参数Table 3. Material parameters of target and casing材料 ρ/(kg·m−3) γ c1/(m·s−1) S1 铝合金 2 785 2.00 5 328 1.338 装甲钢 7 860 1.67 4 610 1.730 材料 D1 D2 D3 D4 D5 装甲钢 −2.2 5.43 −0.47 0.16 0.63 2. 壳体材料对中心侵彻体侵彻特性的影响
为研究内、外壳体材料对内壳形成的中心侵彻体侵彻特性的影响规律，选取钢和铝合金两种不同密度的常用材料，设计了内外壳均为钢、外壳为钢内壳为铝合金、外壳为铝合金内壳为钢和内外壳均为铝合金的四种不同内外壳体材料组合的环形聚能装药结构，由于药型罩结构对中心侵彻体的影响不大，因此仅选取药型罩为罩顶向外侧偏移距离Δr=0.05d的偏心环锥罩进行研究。后端靶板厚度为1.6d，与装药之间的距离为1.12d，有限元模型如图3所示。
图 3 环形聚能装药与后端靶板有限元模型Figure 3. Finite element model of annular shaped charges and rear target通过计算，四种工况下中心侵彻体对后端靶板的侵彻结果如图4所示。
图 4 不同壳体材料组合时后端靶板的侵彻结果Figure 4. Penetration results of rear target with different casing material combinations不同工况下中心侵彻体侵彻后端靶板形成的中心孔径和侵彻深度如表5所示。
表 5 不同壳体材料组合时后端靶板的中心孔尺寸Table 5. Core hole sizes in rear target with different casing material combinations壳体材料 后端靶板中心孔深度/d 后端靶板中心孔直径/d 外钢内钢 1.600（打穿） 0.24 外钢内铝合金 1.008 0.44 外铝合金内钢 1.600（打穿） 0.24 外铝合金内铝合金 1.036 0.44 从上述结果可以看出，当内壳材料为铝时，中心侵彻体对后端靶板侵彻形成的中心孔呈现孔径较大但深度较小的形态；当内壳材料为钢时，形成的中心孔呈现孔径较小但深度较大的形态。考虑到环形聚能装药常常应用于串联战斗部前级结构的用途，其中心侵彻体对后端靶板形成的侵彻深度越小，对后级战斗部的影响越小。对比不同工况下的中心孔侵彻深度，内壳为铝合金时的中心孔平均侵彻深度较内壳为钢时的平均侵彻深度低36.13%，内壳为铝合金、外壳为钢时，中心侵彻体对后端靶板的侵彻深度最小。外壳材料对中心孔的侵彻效果影响较小，当选用波阻抗更大的外壳材料时，爆轰波反射形成的反射冲击波与爆轰波叠加作用更大，可以提高药型罩的压垮速度，进而提高环形射流速度，有利于提升环形射流的毁伤效果[14]。因此本文中选取外壳材料为钢，内壳材料为铝合金的壳体材料组合进行研究。
3. 环形射流侵彻特性影响因素分析
3.1 罩顶偏心距离
设计罩顶偏心距离Δr为−0.10d、−0.05d、0、0.05d和0.10d五种不同的环锥罩结构，其中药型罩顶向远离装药轴线方向偏移时偏心距离Δr为正，向靠近装药轴线方向偏移时偏心距离Δr为负，Δr为0时的药型罩即为非偏心环锥罩。通过计算，五种工况下环形射流成型及侵彻结果如图5所示。
图 5 不同偏心距离时环形射流成型及侵彻结果Figure 5. Formation and penetration results of annular jet at different eccentric distances从图5中可以看出，不同偏心距离的环锥形药型罩形成的射流都有不同程度的径向偏移，原因在于，空心环形装药爆轰后在药型罩内、外侧产生的压力不同，从而使得射流产生径向偏移。为量化环形射流径向偏移的程度，定义单侧射流主体部分宽度为射流径向偏移量k，如图6所示。
不同工况下环形射流的径向偏移量和侵彻深度随偏心距离的变化规律如图7所示。
图 7 环形射流的径向偏移量和侵彻深度随偏心距离的变化Figure 7. Radial offset and penetration depth of annular jet at different eccentric distances从上述结果可以看出，射流的径向偏移量和侵彻深度具有相反的趋势。由于非偏心环锥罩内侧装药量低于外侧装药量，导致形成的射流出现径向偏移。当环锥罩顶向内侧偏移时，内侧装药量进一步减少，导致内外侧装药量差异增加，形成的射流径向偏移量增加，侵彻深度减小。当罩顶向外侧偏移0.05d时，外侧装药量减小，内侧装药量增加，内外侧装药量差异减小，因此此时形成的射流准直性较好，且对靶板的侵彻深度较大。当罩顶进一步向外侧偏移时，内侧装药量大于外侧装药量，射流径向偏移量增加，且偏移方向与非偏心环锥罩相反，对靶板的侵彻威力下降。由此可知，针对本文所研究的装药结构，将环锥罩顶向外侧偏移0.05d有助于提高射流的准直性和侵彻威力。
3.2 药型罩壁厚
为探索药型罩壁厚对中空环形聚能射流成型及侵彻效果的影响规律，在外壳为钢、内壳为铝合金的壳体材料组合和罩顶偏心距离
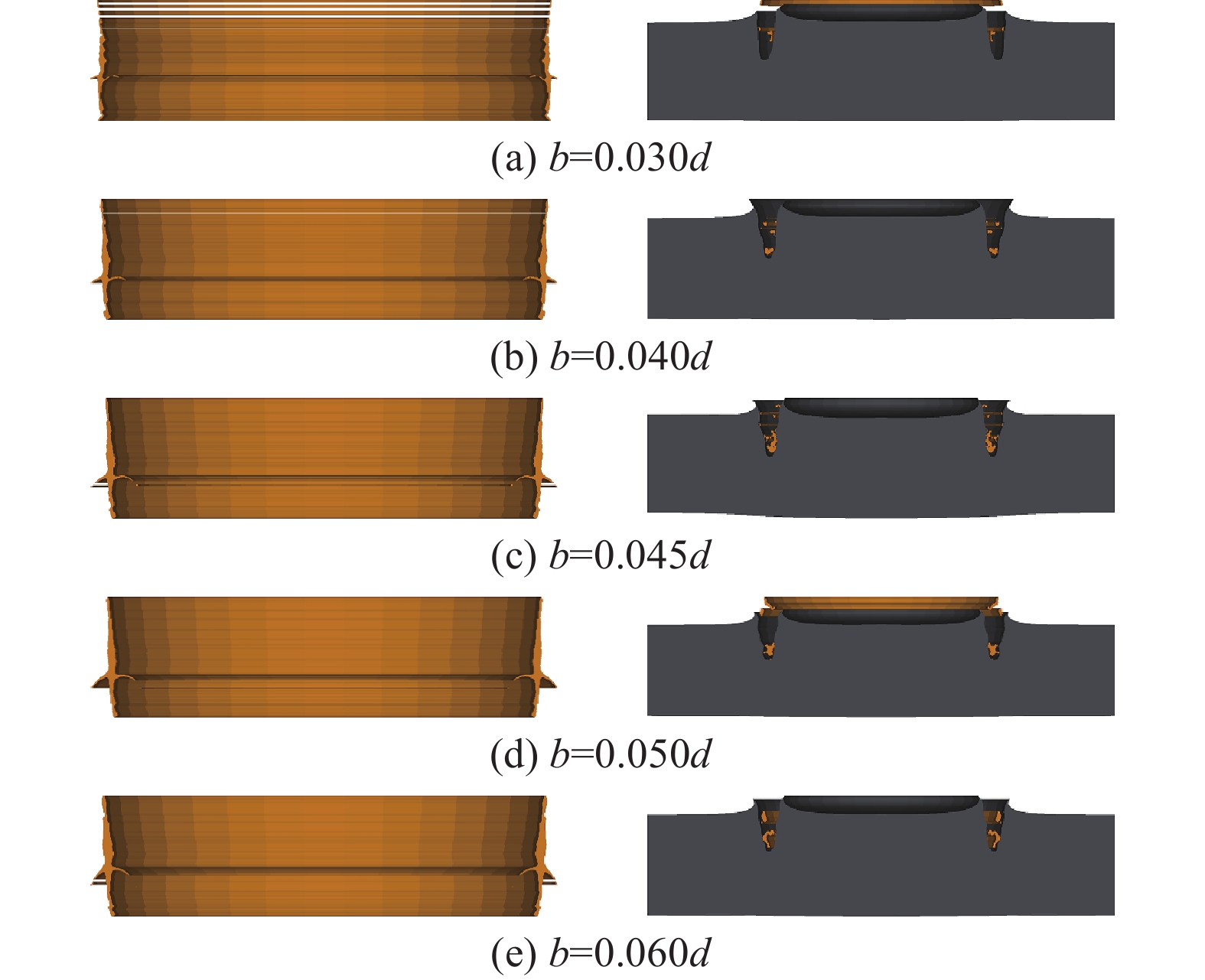
Δr =0.05d的环锥罩基础上，设计了药型罩壁厚b为0.030d、0.040d、0.045d、0.050d和0.060d的五种不同的工况，五种工况下环形射流的成型及侵彻结果如图8所示。
图 8 不同药型罩壁厚时环形射流成型及侵彻结果Figure 8. Formation and penetration results of annular jet at different liner thicknesses观察不同工况下射流的成型状态可知，在射流偏心距离相同的情况下，射流的径向偏移程度相似，不同工况中射流的侵彻深度和头部速度随药型罩壁厚的变化规律如图9所示。
图 9 环形射流的头部速度和侵彻深度随药型罩壁厚变化规律Figure 9. Tip velocity and penetration depth of annular jet at different liner thicknesses从以上计算结果可以看出，在装药结构和药型罩形状不变的情况下，随着药型罩壁厚的增加，药型罩质量增加，驱动药型罩所需的能量增加，因此射流成型后头部速度减小。在毁伤威力方面，随着药型罩壁厚的增加，侵彻深度呈先增加后减小的趋势，其中壁厚b=0.045d的工况下，射流侵彻深度较大。这是因为壁厚过小时药型罩质量小，导致形成的射流过细且有效质量降低，不利于对靶板的侵彻。而药型罩壁厚过大时射流成型后头部速度降低，同样不利于后续的侵彻。因此，针对本文所研究的中空环形聚能装药而言，壁厚为0.045d的偏心环锥罩毁伤效果较好。
3.3 炸高
在偏心距离
Δr 为0.05d、壁厚b为0.045d的偏心环锥罩的基础上，设计了l为0.80d、0.96d、1.12d、1.28d和1.44d五种不同的炸高。通过计算，五种工况下环形射流侵彻结果如图10所示。
图 10 不同炸高时环形射流侵彻结果Figure 10. Penetration results of annular jet at different standoff不同工况下环形射流的侵彻深度随炸高的变化规律如图11所示。从图11可知，随着炸高的增大，射流侵彻深度呈先增大后减小的趋势，这是因为，当药型罩结构和装药结构相同时，射流成型效果也相同，但炸高较小时，射流未完全成型，此时射流的侵彻深度较小。随着飞行距离的增大，射流触靶前已经成型，其侵彻深度有所增大。当炸高进一步增大时，射流飞行距离增大，触靶前射流头部断裂，侵彻深度减小。由此可见，环形射流对炸高敏感性强，当射流成型且未发生断裂时其侵彻效果最好，本文所研究的环形射流在炸高为1.12d时可对靶板形成较好的侵彻效果。
图 11 环形射流的侵彻深度随炸高的变化规律Figure 11. Penetration depth of annular jet at different standoff4. 试验验证
4.1 试验装置
为验证数值模拟的计算结果，采用精车工艺分别制备了非偏心环锥罩和偏心环锥罩两种药型罩，开展了环形聚能装药静破甲试验，其中，偏心环锥罩为本文优化后的最终结构，非偏心环锥罩为对比工况。装药结构参数及炸高如表6所示。
表 6 试验中的环形聚能装药结构参数及炸高Table 6. Structural parameters and standoff of annular shaped charge in the test环锥罩 Δr/d b/d l/d 非偏心环锥罩 0 0.045 1.12 偏心环锥罩 0.05 0.045 1.12 制备出的药型罩如图12～13所示。为降低试验结果的偶然性，对两种罩型分别开展两次重复试验。内、外壳体材料分别选取铝合金和钢。靶板尺寸为12d×12d×0.8d，材料为均质装甲钢，采用12点起爆模拟环形起爆。
试验布置如图14所示，主要由环形聚能装药、支撑板、炸高筒和靶板组成。
4.2 试验结果
靶板侵彻结果如图15所示，非偏心环锥罩形成的环形射流未穿透靶板，仅在靶板表面留下一个圆形凹坑，但装药内壳形成的中心侵彻体击穿靶板，在靶板中央形成一个圆形通孔；偏心环锥罩形成的环形射流击穿靶板，在靶板表面留下一个大直径圆孔和圆形塞块，塞块中心同样存在内壳形成的中心侵彻体击穿的圆形通孔。
为对比两种环形聚能装药形成的射流对靶板的毁伤情况，将试验后的靶板分别沿图15中红色虚线位置剖切为两部分，从截面处观察侵彻结果，非偏心环锥罩和偏心环锥罩试验所得结果与数值模拟结果对比分别如图16和图17所示。
图 16 非偏心环锥罩的试验结果和数值模拟结果对比Figure 16. Comparison between experimental and numerical simulation results of non-eccentric liner
图 17 偏心环锥罩的试验结果和数值模拟结果对比Figure 17. Comparison between experimental and numerical simulation results of eccentric liner由于三维计算模型需要消耗大量的计算资源，而二维轴对称模型的计算精度已足够满足本文的研究需求，因此本文中使用的数值模拟模型为二维模型，只是在展示时进行了三维填充，这就导致在二维模型上出现的失效将会以轴对称的方式进行三维展示，因此，在图16和图17结果中未使用三维模型模拟射流对靶板破坏形态的影响细节。总体来说，试验与数值模拟中靶板侵彻孔径形态相似。为对比二者的破坏参数，定义侵彻后的靶板几何参数如图18所示，图中d1为环形侵彻通道外径，d2为环形侵彻通道内径，d3为中心侵彻孔径。试验和数值模拟中的靶板破坏参数如表7所示。
图 18 侵彻后的靶板几何参数示意图Figure 18. Schematic diagram of geometric parameters of penetrated target表 7 数值模拟结果与试验结果的对比Table 7. Comparison between numerical simulation and experimental results罩型 方法 d1 d2 d3 h 非偏心环锥罩 试验 4.40d 3.44d 1.00d 0.68d 数值模拟 4.48d 3.68d 0.88d 0.64d 误差 1.81% 6.98% 12.00% 5.88% 偏心环锥罩 试验 4.74d 3.84d 0.80d 0.80d（打穿） 数值模拟 4.48d 4.00d 0.88d 0.80d（打穿） 误差 5.08% 4.17% 10.00% − 从表7可知，数值模拟与试验得到的侵彻后靶板的形貌基本一致，几何参数误差在合理范围内，说明了本文研究中采用的数值模拟模型的合理性及所得结论的准确性。
5. 结 论
设计了一种非对称中空环形聚能装药结构。基于钢和铝合金两种常用材料，设计了不同壳体材料组合并研究了壳体材料对中心侵彻体侵彻特性的影响，通过改变环锥罩的偏心距离和壁厚，调整装药和药型罩的质量分布，形成准直环形射流，研究了炸高对环形射流侵彻威力的影响规律，开展了环形聚能装药静破甲试验，得到以下结论。
(1)内壳材料为铝合金时，形成的中心侵彻体侵彻孔洞孔径大、侵彻深度小，内壳材料为钢时，形成的中心孔孔径小、侵彻深度大，为减小环形聚能装药对后级战斗部的影响，选取铝合金作为内壳材料。选用波阻抗较高的钢作为外壳材料有利于提高环形射流的速度。
(2)非偏心环锥罩形成的射流存在径向偏移，且侵彻深度较小；罩顶向内侧移动会导致环形射流的径向偏移现象加剧；罩顶向外侧移动0.05d时，射流准直性较好且侵彻深度较大，但罩顶继续向外侧偏移会导致射流准直性和侵彻威力降低。药型罩壁厚为0.03d～0.06d时，随着壁厚的增加，射流头部速度不断减小，壁厚为0.045d的偏心环锥罩形成射流的毁伤效果较好。环形射流侵彻深度对炸高较为敏感，炸高为0.80d～1.44d时，随着炸高的增加，射流侵彻深度呈现先增大后减小的趋势，炸高为1.12d时射流侵彻深度较大。
(3)设计了环形聚能装药静破甲试验，采用12点起爆模拟环形起爆，试验结果与数值模拟结果误差在12%以内，且试验所得侵彻通道形态与数值模拟结果具有较好的一致性，验证了本文数值模拟模型的合理性和所得结论的准确性。
-
图 1 雀尾螳螂虾及其虾螯宏微观结构
Figure 1. Odontodactylus scyllarus and macro-micro structure of shrimp chela
图 3 仿生波纹夹层结构有限元模型
Figure 3. A finite element model for the bionic corrugated-core sandwich structure
图 4 单层波纹结构峰值载荷和比吸能随高宽比变化关系
Figure 4. The initial peak load and specific energy absorption of single-layer structure versus with height-to-width ratios
图 6 双层波纹结构峰值载荷和比吸能随高宽比变化关系
Figure 6. The initial peak load and specific energy absorption of double-layer structure versus with height-to-width ratios
图 7 不同结构的峰值载荷和比吸能模型预测值变化
Figure 7. The initial peak load and specific energy absorption predicted by the models for different structures
表 1 三层波纹结构耐撞性仿真结果
Table 1. Simulated crashworthiness of three-layer sandwich structures
试验号 γ1 γ2 γ3 Fp/kN Es/（kJ·kg−1） 1 0.5 0.5 0.5 3.88 0.65 2 0.5 1.0 1.0 1.99 0.38 3 0.5 1.5 1.5 2.01 0.32 4 0.5 2.0 2.0 3.06 0.29 5 1.0 0.5 2.0 1.90 0.29 6 1.0 1.0 1.5 1.56 0.35 7 1.0 1.5 1.0 1.53 0.31 8 1.0 2.0 0.5 1.72 0.28 9 1.5 0.5 1.0 3.10 0.39 10 1.5 1.0 0.5 3.46 0.40 11 1.5 1.5 2.0 1.65 0.24 12 1.5 2.0 1.5 1.64 0.26 13 2.0 0.5 1.5 3.25 0.28 14 2.0 1.0 2.0 3.58 0.28 15 2.0 1.5 0.5 3.73 0.34 16 2.0 2.0 1.0 3.58 0.28 
下载: 导出CSV
表 2 极差分析结果
Table 2. Results of range analysis
参数 Fp/kN Es/（kJ·kg−1） γ1 γ2 γ3 γ1 γ2 γ3 ˉyj1 2.732 3.031 3.196 0.410 0.400 0.415 ˉyj2 1.678 2.645 2.548 0.305 0.350 0.341 ˉyj3 2.462 2.233 2.116 0.321 0.302 0.300 ˉyj4 3.536 2.499 2.548 0.293 0.277 0.273 Rj 1.858 0.798 1.080 0.117 0.123 0.142
下载: 导出CSV
表 3 方差分析结果
Table 3. Results of variance analysis
因素 Fp Es Pj 显著性水平α Pj 显著性水平α γ1 8.29 0.05 2.57 0.25 γ2 1.57 ＞0.25 2.69 0.25 γ3 2.80 0.25 3.5 0.1
下载: 导出CSV
表 4 模型误差分析
Table 4. Error analysis of the model
波纹结构 Fp Es εe/% ζ/kN εe /% ζ/（kJ·kg−1） 单层 7.87 1.94 4.68 0.0372 双层 10.27 0.67 0.71 0.0041 三层 8.07 0.25 3.67 0.0172
下载: 导出CSV
表 5 优化结果与验证
Table 5. Optimization results and validation
结构 γ1 γ2 γ3 Fp /kN Es /（kJ·kg−1） 预测值 实际值 误差 /% 预测值 实际值 误差 /% 单层 0.8 − − 18.97 19.67 −3.56 0.78 0.86 −9.30 双层 0.5 1.2 − 4.42 4.91 −9.98 0.54 0.56 −3.57 三层 0.6 0.6 0.9 2.73 3.03 −9.90 0.50 0.46 8.70
下载: 导出CSV
-
[1] 周晓松, 梅志远, 张焱冰. 复合材料夹层结构在舰艇碰撞防护中的研究进展 [J]. 爆炸与冲击, 2018, 38(3): 696–706. DOI: 10.11883/bzycj-2016-0303.ZHOU X S, MEI Z Y, ZHANG Y B. Research progress of composite sandwich structure in ship collision protection [J]. Explosion and Shock Waves, 2018, 38(3): 696–706. DOI: 10.11883/bzycj-2016-0303. [2] 张振华, 钱海峰, 王媛欣, 等. 球头落锤冲击下金字塔点阵夹芯板结构的动态响应实验 [J]. 爆炸与冲击, 2015, 35(6): 888–894. DOI: 10.11883/1001-1455(2015)06-0888-07.ZHANG Z H, QIAN H F, WANG Y X, et al. Experiment of dynamic response of multilayered pyramidal lattices during ball hammer collision loading [J]. Explosion and Shock Waves, 2015, 35(6): 888–894. DOI: 10.11883/1001-1455(2015)06-0888-07. [3] 邓泽华, 郭锐, 周昊, 等. 梯度波纹夹层防护结构超高速碰撞特性仿真研究 [J]. 航天器环境工程, 2018, 35(1): 7–13. DOI: 10.3969/j.issn.1673-1379.2018.01.002.DENG Z H, GUO R, ZHOU H, et al. Simulation of hypervelocity impact characteristics of gradient corrugated-core sandwich plates [J]. Spacecraft Environment Engineering, 2018, 35(1): 7–13. DOI: 10.3969/j.issn.1673-1379.2018.01.002. [4] 潘晋, 黄义飞, 徐荣康, 等. 波纹夹层结构耐撞性影响因素分析 [J]. 武汉理工大学学报(交通科学与工程版), 2019, 43(2): 192–197. DOI: 10.3963/j.issn.2095-3844.2019.02.003.PAN J, HUANG Y F, XU R K, et al. Analysis of influencing factors on crashworthiness of corrugated sandwich structures [J]. Journal of Wuhan University of Technology (Transportation Science & Engineering), 2019, 43(2): 192–197. DOI: 10.3963/j.issn.2095-3844.2019.02.003. [5] 骆伟, 谢伟, 刘敬喜. 芯层几何构形对复合材料波纹夹层结构冲击特性的影响 [J]. 江苏科技大学学报(自然科学版), 2018, 32(1): 21–26. DOI: 10.3969/j.issn.1673-4807.2018.01.004.LUO W, XIE W, LIU J X. Research on dynamic characteristics of a sandwich structures with various core shapes under impact loads [J]. Journal of Jiangsu University of Science and Technology (Natural Science Edition), 2018, 32(1): 21–26. DOI: 10.3969/j.issn.1673-4807.2018.01.004. [6] HE W T, LIU J X, TAO B, et al. Experimental and numerical research on the low velocity impact behavior of hybrid corrugated core sandwich structures [J]. Composite Structures, 2016, 158: 30–43. DOI: 10.1016/j.compstruct.2016.09.009. [7] LIU J X, HE W T, XIE D, et al. The effect of impactor shape on the low-velocity impact behavior of hybrid corrugated core sandwich structures [J]. Composites Part B: Engineering, 2017, 111: 315–331. DOI: 10.1016/J.COMPOSITESB.2016.11.060. [8] HE W T, LIU J X, WANG S Q, et al. Low-velocity impact response and post-impact flexural behaviour of composite sandwich structures with corrugated cores [J]. Composite Structures, 2018, 189: 37–53. DOI: 10.1016/j.compstruct.2018.01.024. [9] 杨欣, 范晓文, 许述财, 等. 仿虾螯结构薄壁管设计及耐撞性分析 [J]. 爆炸与冲击, 2020, 40(4): 043301. DOI: 10.11883/bzycj-2019-0280.YANG X, FAN X W, XU S C, et al. Design and crashworthiness analysis of thin-walled tubes based on a shrimp chela structure [J]. Explosion and Shock Waves, 2020, 40(4): 043301. DOI: 10.11883/bzycj-2019-0280. [10] 秦诗牧, 秦俊奇, 杨玉良, 等. 枪虾夹螯的结构特性、运动特性与射流聚焦机理研究 [J]. 振动与冲击, 2019, 38(21): 202–209. DOI: 10.13465/j.cnki.jvs.2019.21.028.QIN S M, QIN J Q, YANG Y L, et al. Structure and motion characteristics as well as jet focusing mechanism of snapping shrimp claw [J]. Journal of Vibration and Shock, 2019, 38(21): 202–209. DOI: 10.13465/j.cnki.jvs.2019.21.028. [11] 郭婷, 王跃方. 仿甲壳虫芯柱的缓冲吸能结构 [J]. 工程力学, 2011, 28(2): 246–251, 256.GUO T, WANG Y F. Energy absorbing structures imitating trabecular of beetle cuticles [J]. Engineering Mechanics, 2011, 28(2): 246–251, 256. [12] ZHANG X M, XIE J, CHEN J X, et al. The beetle elytron plate: a lightweight, high-strength and buffering functional-structural bionic material [J]. Scientific Reports, 2017, 7(1): 4440. DOI: 10.1038/s41598-017-03767-w. [13] YANG X F, MA J X, SHI Y L, et al. Crashworthiness investigation of the bio-inspired bi-directionally corrugated core sandwich panel under quasi-static crushing load [J]. Materials & Design, 2017, 135: 275–290. DOI: 10.1016/j.matdes.2017.09.040. [14] PATEK S N, KORFF W L, CALDWELL R L. Biomechanics: deadly strike mechanism of a mantis shrimp [J]. Nature, 2004, 428(6985): 819–820. DOI: 10.1038/428819a. [15] PATEK S N, CALDWELL R L. Extreme impact and cavitation forces of a biological hammer: strike forces of the peacock mantis shrimp Odontodactylus scyllarus [J]. Journal of Experimental Biology, 2005, 208(19): 3655–3664. DOI: 10.1242/jeb.01831. [16] WEAVER J C, MILLIRON G W, MISEREZ A, et al. The stomatopod dactyl club: a formidable damage-tolerant biological hammer [J]. Science, 2012, 336(6086): 1275–1280. DOI: 10.1126/science.1218764. [17] CLAVERIE T, CHAN E, PATEK S N. Modularity and scaling in fast movements: power amplification in mantis shrimp [J]. Evolution: International Journal of Organic Evolution, 2011, 65(2): 443–461. DOI: 10.1111/j.1558-5646.2010.01133.x. [18] QIU N, GAO Y K, FANG J G, et al. Crashworthiness analysis and design of multi-cell hexagonal columns under multiple loading cases [J]. Finite Elements in Analysis and Design, 2015, 104: 89–101. DOI: 10.1016/j.finel.2015.06.004. [19] HUANG H, XU S C. Crashworthiness analysis and bionic design of multi-cell tubes under axial and oblique impact loads [J]. Thin-Walled Structures, 2019, 144: 106333. DOI: 10.1016/J.TWS.2019.106333. [20] BARAKAT S, BANI-HANI K, TAHA M Q, et al. Multi-objective reliability-based optimization of prestressed concrete beams [J]. Structural Safety, 2004, 26(3): 311–342. DOI: 10.1016/j.strusafe.2003.09.001. -
下载:
计量
- 文章访问数: 693
- HTML全文浏览量: 500
- PDF下载量: 153
- 被引次数: 0