A review on the improved Whipple shield and related numerical simulations
-
摘要: 基于弹丸在超高速撞击薄板时破碎形成碎片云的机理,Whipple防护结构能够对航天器所面临的空间碎片及微流星体等威胁形成有效防护。通过回顾Whipple防护结构的研究和发展历程,对多层板结构、填充式防护结构、夹芯板结构等进行对比,分析其力学效应和防护性能;总结可应用于含泡沫、蜂窝、梯度和编织等材料的防护结构超高速撞击的数值模拟方法及其改进方法;结合相关材料的超高速撞击试验及数值模拟结果,为防护结构未来的研究方向提出建议。
-
关键词:
- 超高速碰撞 /
- Whipple防护结构 /
- 碎片云 /
- 多层板结构 /
- 填充/夹芯板结构 /
- FEM-SPH自适应耦合算法
Abstract: Based on the formation mechanism of the debris cloud caused by the projectile hypervelocity impacting onto a thin plate, the Whipple shield can effectively protect the spacecraft from space debris and micrometeoroid. By reviewing the research and development of the Whipple shield, and compares the mechanical effects and protective performance of multilayer, stuffed and sandwich shield. The paper also summarizes the application of numerical simulation methods and their improvement for the hypervelocity impact of protective structures containing materials such as foam and honeycomb, etc. By addressing the results of hypervelocity impact tests and numerical simulations of relevant materials, suggestions are made for the future research of the Whipple shield. -
随着重要军事目标的坚固化和地下化,世界各国加速发展钻地武器,使其打击更加精确、侵彻更深、破坏力更大,对地下防护工程带来极大威胁。与空中爆炸或触地爆炸相比,钻入地下的武器再爆炸能使绝大多数爆炸能量耦合至岩土中,使地冲击威力大大增强。同时,钻地爆炸对地下工程产生的破坏效应与其地冲击能量特征密切相关。大当量地下爆炸现场实测数据均表明[1-5],地下爆炸耦合至岩土中的地冲击能量随装药埋深增加而迅速增大,在某一临界深度时增速减缓,而后随埋深增大逐渐趋近于地下封闭爆炸。深埋封闭爆炸的耦合地冲击能量可达同当量触地爆炸的10倍以上,因此对于防护工程设计来讲,必须要建立爆炸深度和耦合地冲击能量间的定量关系。
近几十年来,对于岩土介质中的封闭爆炸和触地爆炸的研究已经较为完善,可获得较丰富试验数据和较为成熟的计算方法。梁霍夫等[6]在土壤(包括饱和土和非饱和土)、砂(包括饱和砂和非饱和砂)、岩石中进行了平面波、球面波和柱面波的地冲击效应研究,综合考虑现场的地质特征和实验所用介质的物理力学参数建立了较为简练的地冲击效应计算方法。在不同岩土介质中地冲击衰减规律方面,学者们也做了大量的研究工作。Yankelevsky等[7]通过对已有实验数据的分析和数值模拟,得到冲击波峰值压力衰减的特点。穆朝民等[8-9]在黄土和砂土中进行了一系列爆炸成坑试验,并结合爆炸宏观特征,确定了黄土及饱和砂土中发生封闭爆炸的临界比例埋深,得到了变埋深条件下应力波在土中传播规律。施鹏等[10]通过模拟手段计算了不同装药比例埋深下土中爆炸能量耦合问题,通过实验得到了耦合系数数据,并给出了公式使用范围和对象。叶亚齐等[11]在砂质黏土中进行了不同装药比例埋深爆炸自由场试验,给出了砂质黏土中不同深度爆炸自由场地冲击参数的衰减规律,建立了砂质黏土中不同深度爆炸自由场地冲击参数的预计公式。赵红玲等[12]研究了石灰岩中常规装药不同埋深爆炸自由场地冲击参数的传播规律,得到了石灰岩介质中变埋深爆炸地冲击参数随比例距离的预计公式。何翔等[13]基于试验手段研究了常规装药爆炸不同深度对自由场直接地冲击参数的影响,建立了石灰岩中爆炸成坑经验公式和地冲击传播特性。但由于土中浅埋爆炸是复杂的耦合效应问题,其理论和实验研究均存在诸多困难,目前尚无可靠的计算方法。目前对于浅埋爆炸地冲击效应的计算,通常采用由美国陆军工程兵水道试验站给出的地冲击耦合系数,将浅埋爆炸转变成等效的封闭或者触地爆炸[14-15]。然而,为验证此方法的有效性,在饱和砂土中共进行了58次爆炸试验[16],发现该计算与试验结果存在较大偏差,特别是在爆炸远区,预测公式不能准确求得地冲击参数,只能定性分析。关于地冲击耦合系数的计算,目前尚无准确的计算方法,给出的实验数据误差较大,难以适应钻地爆炸等效当量及耦合地冲击参数的准确计算。同时,现有文献[17-18]中存在多种“地冲击耦合系数”,如能量耦合系数、等效当量系数、地冲击应力耦合系数等,相互间未建立准确的换算方法,若不仔细区分其物理本质而加以混淆使用,容易造成计算错误。
为了研究黏土中爆炸成坑体积与耦合地冲击能量的关系,本文在已有研究成果的基础上,系统开展不同埋深下成坑地冲击耦合效应实验研究,探索弹坑体积以及地冲击压力随装药埋深增加的变化规律,寻找不同耦合系数间的换算关系以及等效封闭当量计算方法,以期为地下工程的抗爆防护提供设计依据。
1. 实验介绍
1.1 黏土试样
本实验所用的黏土取自南京孟墓地区,首先清除地表覆土,然后将黏土平摊放置地表捡去里面大块杂质,之后倒入爆炸试验容器中,分层夯实。该黏土中主要化学组成及质量分数分别为:SiO2 (61.16 %)、Al2O3 (23.10 %)、Fe2O3 (8.94 %)、K2O (2.65 %)、MgO (1.56 %),等。黏土试样的密度是2.242×103 kg/m3,含水率12.8 %,纵波速度1832 m/s,其波阻抗近似为0.4×107 N∙s/m3。根据国家标准[19]采用筛析法计算绘出黏土的粒径级配曲线如图1所示。
1.2 爆炸容器及球形爆源
采用一种分层可拼装的筒体结构作为爆炸容器,如图2所示。该装置高度是1 490 mm,容器内径1 500 mm,钢板厚度为15 mm,由7个子单元自下而上垂直拼装而成。子单元由Q345钢板经弯、卷、焊等工序加工而成,包括上下两片宽75 mm、厚16 mm的法兰盘,起到提高容器半径法向刚度和连接拼装单元的作用。法兰盘上均匀加工24个内径16 mm的通孔,为增强法兰和圆环之间的强度,在圆环外侧铺设12个肋板。子单元之间通过强度等级为8.8级的高强度M14螺栓连接。按照薄壁圆筒公式计算,容器可承受内壁上强度为5.2 MPa的均匀荷载。
在黏土介质爆炸实验研究中,大多使用块状TNT、雷管和乳化炸药,当测点距离爆心较远时,可以把爆源近似当作点源,但是测点距离爆心较近时,炸药的形状、密度和种类均会给试验结果产生显著的影响,Krauthammer[20]指出在爆炸试验中采用球形装药所采集的试验数据更加科学。为获取爆心距较近范围内黏土中的爆炸应力波衰减规律,本实验选用球形装药作为爆源,如图3所示。药球由三硝基甲苯(TNT)采用一体成型技术压装而成,药球质量为10.5 g,直径为24.4 mm,装药密度1.5 g/cm3。药球顶部预留直径8 mm、深度12 mm的雷管安装孔,其尺寸和标准雷管中猛炸药尺寸相近,安装孔的体积占比约为7.93 %。采用电雷管起爆,实验前将雷管插入装药安装孔中,并使用绝缘胶带固定。
1.3 测点布置
为获取装药埋深对地冲击压力传播衰减的影响规律,共设计7组不同埋深的爆炸实验,每组实验中布置5组测点(记为1#、2#、3#、4#、5#),各实验装药埋深(h)和地冲击压力测点比例爆心距(R)见表1。
表 1 装药埋深(h )及爆心距(R )设计Table 1. Design of burial depth of charge (h ) and burst core distance (R )工况 h/(m·kg−1/3) R/(m·kg−1/3) 1# 2# 3# 4# 5# 1 −0.056 0.799 1.427 2.295 3.274 4.000 2 0 0.799 1.427 2.295 3.274 4.000 3 0.14 0.799 1.427 2.295 3.274 4.000 4 0.37 0.799 1.427 2.295 3.274 4.000 5 0.55 0.799 1.427 2.295 3.274 4.000 6 1.19 0.799 1.427 2.295 3.274 4.000 7 1.46 0.479 1.155 2.068 3.046 3.772 药球位于筒体容器的中轴线上,在试样制备过程中预留装药孔,然后将药球放入装药孔后回填。爆心下方共铺设5层土压力传感器,每层铺设2个,于中轴线两侧对称布置,距离容器轴线50 mm,具体如图4(a)所示。采用DNS123型土压力传感器获取不同测点处爆炸波法向应力的时程曲线,其尺寸为
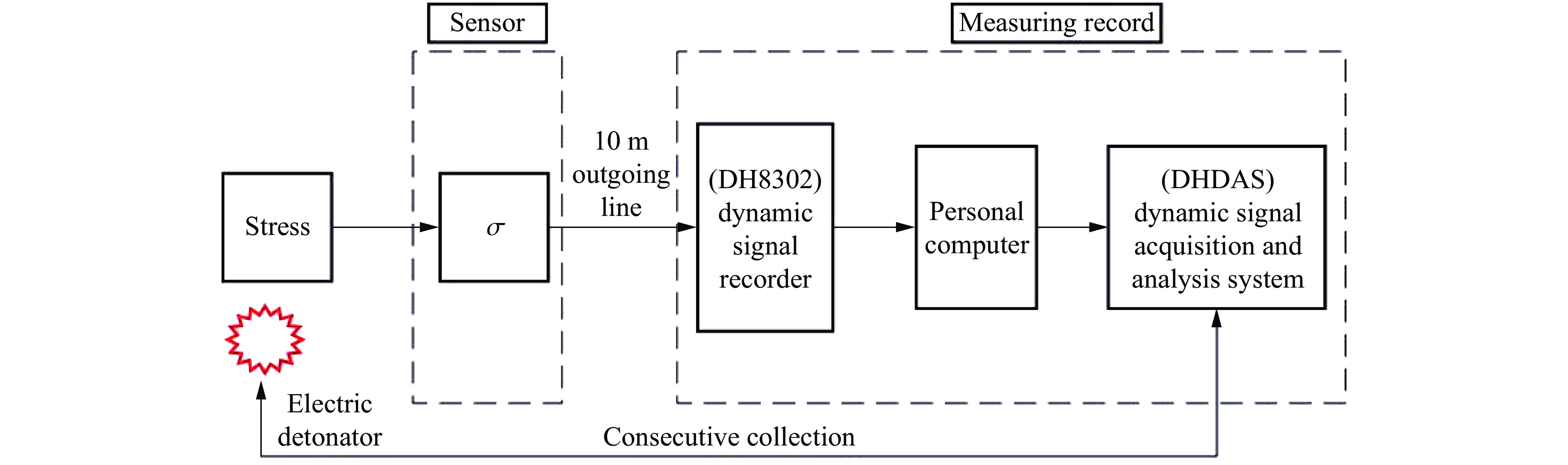
∅ 50 mm×10 mm,如图4(b)所示。为了减少传感器本身对地冲击传播的干扰,采用上下层交叉铺设的方式使传感器位于不同方位,交叉角度约36°,如图4(c)所示(图中编号表示传感器铺设所在的层数)。采用东华DH8302高性能动态信号测试系统进行地冲击压力数据采集,采样频率为100 kHz,如图5所示。为方便对比不同装药埋深对地冲击传播的影响,7次实验中保持各组传感器距离爆心的比例距离不变。每做完一次实验后首先利用3D扫描仪对弹坑进行扫描,然后沿弹坑中轴面将弹坑剖开,观察弹坑周围介质的压缩破坏情况,分析完成后将破坏的黏土铲除而后重新铺设新的黏土层并进行重新夯实。
2. 实验结果分析
2.1 成坑特征
2.1.1 可视弹坑分析
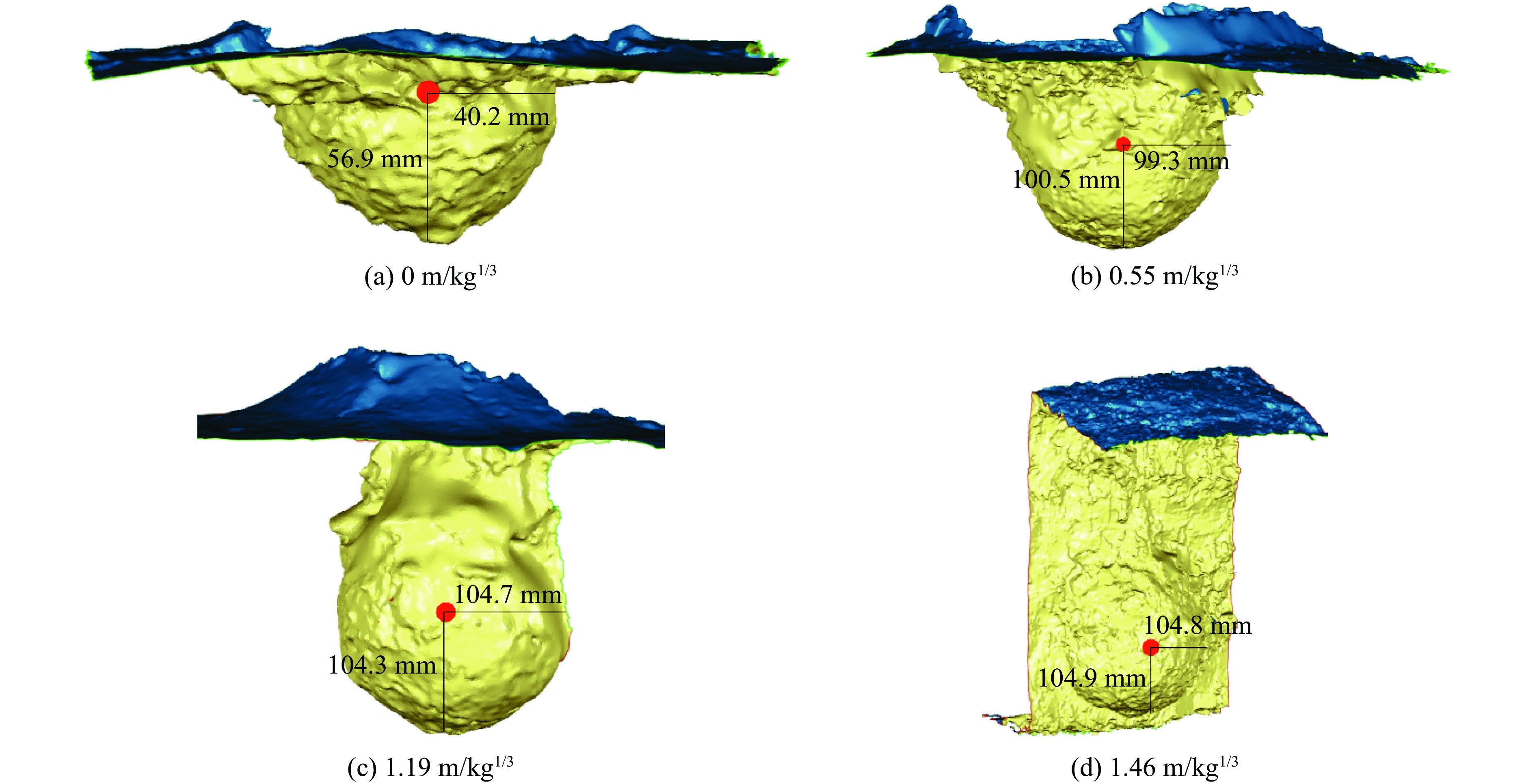
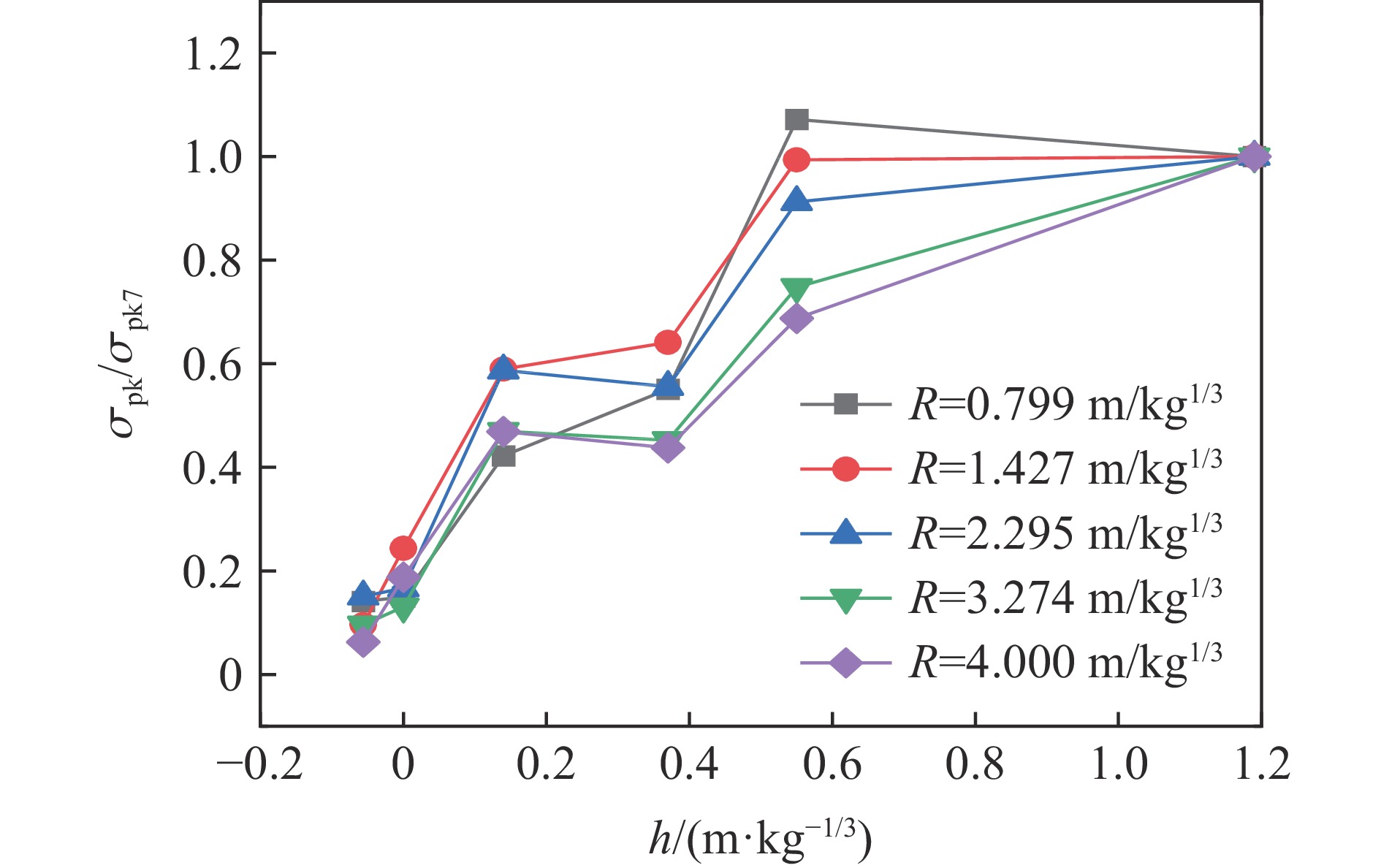
黏土中爆炸时,爆炸冲击波和爆生气体压缩爆炸中心周围黏土介质,并形成冲击波向四周传播,随着传播距离增加,冲击波逐渐衰减为塑性波、弹性波,同时在黏土介质中形成爆炸空腔区、破坏区等区域。当冲击波遇到自由面时,在自由面反射作用下形成反向传播的拉伸波,对黏土介质产生层裂或者剥离。如果爆炸埋深较浅,爆轰产物和爆炸应力波激发近地表土层土颗粒发生飞散形成抛掷弹坑;而随着埋深进一步增大,弹坑体积也进一步增大,直至在某一最佳临界深度处体积达到最大;而后随着埋深进一步增加,地表可视弹坑逐渐消失,地下逐渐形成完整的爆炸空腔,但由于空腔膨胀作用,在地面处形成鼓包和破裂;最后,当埋深超出封闭爆炸临界深度时,地下爆炸破坏效应完全被封闭在地下,地表无反应,一般将地表面上无明显可见变化的深度称为封闭爆炸临界深度。图6和图7给出了不同埋深情况下爆炸弹坑的宏观破坏情况,相关弹坑尺寸数据见表2。从结果可知:可视弹坑,在装药埋深达到0.55 m/kg1/3时,弹坑体积达到最大,在装药比例埋深到1.19 m/kg1/3时,地表弹坑几乎将近消失,同时地表发生明显隆起鼓包(图6(c)和图7(c) ),当比例埋深达到1.46 m/kg1/3时,地表鼓包几近消失,但在土层表面形成不规则裂纹(图6(d)和图7(d) )。对于封闭爆炸临界深度,目前公开文献的外观点基本一致,穆朝民等[8]所做的土中爆炸试验指出在比例埋深到达1.96 m/kg1/3时,地表面接近没有明显可见变化,梁霍夫[6]在归纳整理的资料中,也得出土中集团装药封闭爆炸临界深度为2.0 m/kg1/3。
表 2 不同埋深条件下弹坑尺寸数据Table 2. Size data of craters under different burial depths工况 h/
(m·kg−1/3)rv /
(m·kg−1/3)dv /
(m·kg−1/3)Vv /
(m3·kg−1)ra /
(m·kg−1/3)d/
(m·kg−1/3)V/
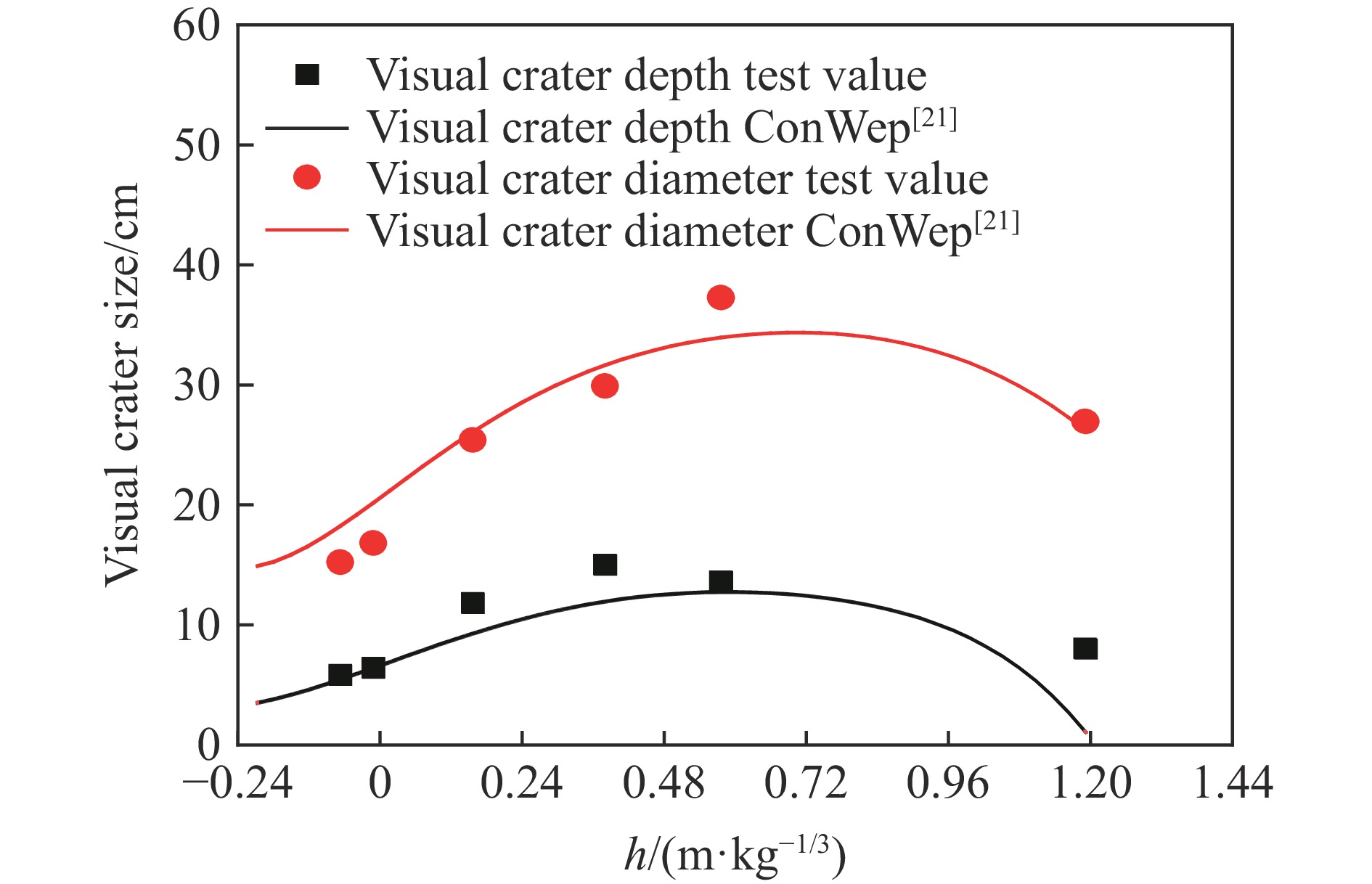
(m3·kg−1)1 −0.056 0.261 0.247 0.035 0.179 0.251 0.017 2 0 0.280 0.292 0.048 0.184 0.260 0.018 3 0.14 0.580 0.539 0.380 0.289 0.379 0.066 4 0.37 0.682 0.685 0.667 0.340 0.416 0.101 5 0.55 0.896 0.776 1.304 0.453 0.459 0.197 6 1.19 0.615 0.502 0.397 0.478 0.478 0.228 7 1.46 0 0 0.000 0.479 0.479 0.230 注:rv、dv、Vv分别为可视弹坑的半径、深度和体积,ra、d、V为有效弹坑的半径、深度和体积。 图8为ConWep爆炸荷载计算程序[21]预测的质量为10.5 g TNT药球在黏土中可视爆坑的深度和直径随装药比例埋深变化曲线,及其与本文实测结果的对比。在装药比例埋深h≤0.55 m/kg1/3时,可视爆坑深度实测值和ConWep计算程序预测的值偏差最高可达20.6 %,而实测的可视爆坑直径与ConWep预测值具有很好的一致性,可视爆坑深度产生的偏差可能由于夯实的黏土起炸后,飞散的黏土粘连并带出爆坑里面黏土,回填变少,现场实测的可视爆坑比ConWep中预测的可视爆坑要大。
2.1.2 有效弹坑分析
装药爆炸过程中,装药爆生产物通过压缩周围介质形成地冲击波,装药中心下方的压缩弹坑对于应力波的形成具有重要作用,将装药中心下方的压缩弹坑称为有效弹坑。理论分析表明[22]:耦合至岩土中的地冲击能量与有效弹坑体积呈正比例关系,在以往历史试验数据中,往往只关注可视弹坑,而忽略了有效弹坑体积的统计。为了便于描述有效弹坑的演化过程,不妨引入球形度和有效弹坑体积比两个量;其中,球形度是有效弹坑半径ra与弹坑深度d的比值。有效弹坑体积比是不同装药比例埋深下的有效弹坑体积V与工况7有效弹坑体积V7
的比值,其比值记为V/V7。可通过表2数据计算得到有效弹坑的球形度和有效弹坑体积比。图9给出了随装药埋深增加有效弹坑球形度和有效弹坑体积比值的变化规律。当装药比例埋深为h=0时,有效弹坑轮廓呈抛物线型(图6(a)),装药中心所在的球形度约为0.708;随装药埋深增大,有效弹坑的球形度和体积比均在增加(图9),同时爆心下方弹坑轮廓线逐渐由抛物线型演化为半球形(图6(b)~(d));当装药比例埋深h≥0.55 m/kg1/3时,装药中心下方有效弹坑体积达到趋近于极限,其形状也接近于完全封闭爆炸。 2.2 地冲击传播规律特征
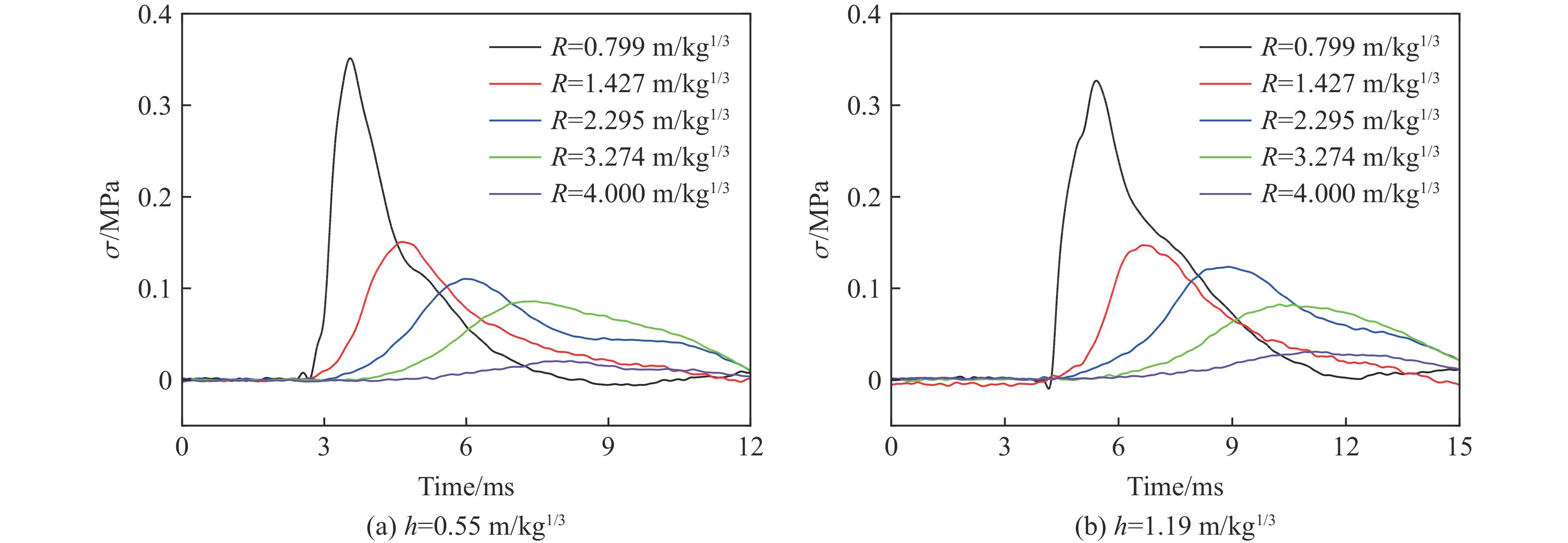
图10分别给出了装药比例埋深为0.55 m/kg1/3 (工况5)和1.19 m/kg1/3 (工况6)时,不同测点处的地冲击应力实测波形,从图10中可以看出,随距离装药比例距离的增加,地冲击应力峰值呈指数衰减特征。
表3给出了不同装药埋深情况下地冲击应力峰值统计数据,图11给出了不同比例爆心距离处地冲击应力峰值比值随装药比例埋深增加的变化情况,地冲击应力峰值比值是不同装药比例埋深下爆炸应力峰值σpk与工况7下爆炸应力峰值σpk7的比值。从表3和图11中可以看出,在相同比例爆心距离处,当装药比例埋深h≤0.55 m/kg1/3时,随装药埋深增加,耦合至黏土中的地冲击压力峰值也急剧增大,而当装药比例埋深h≥0.55 m/kg1/3时,耦合至黏土中的地冲击压力峰值增势趋近平缓,接近于完全封闭爆炸,这一变化趋势与有效弹坑体积随装药埋深的变化趋势(图9(b) )基本一致。
表 3 黏土中各比例埋深下地冲击应力峰值数据Table 3. Subsurface impact stress peak data of each proportion buried depth in clay工况 h/
(m·kg−1/3)σpk/MPa 工况 h/
(m·kg−1/3)σpk/MPa 1# 2# 3# 4# 5# 1# 2# 3# 4# 5# 1 −0.056 0.047 0.015 0.019 0.011 0.002 5 0.55 0.358 0.155 0.115 0.086 0.022 2 0 0.050 0.038 0.021 0.015 0.006 6 1.19 0.334 0.156 0.126 0.115 0.032 3 0.14 0.141 0.092 0.074 0.054 0.015 7 1.46 1.080 0.196 0.124 0.100 0.024 4 0.37 0.184 0.100 0.070 0.052 0.014 (0.389) (0.166) (0.047) (0.023) (0.015) 注:(1)1~6炮次1#、2#、3#、4#、5#测点比例距离分别为0.799、1.427、2.295、3.274、4.000;(2)第7炮次1#、2#、3#、4#、5#测点比例距离分别为0.479、1.155、2.068、3.046、3.772;(3)第7炮括号内数据为将第7炮次数据在比例距离0.799、1.427、2.295、3.274、4.000处换算数据。 将爆炸地冲击应力峰值接近于封闭爆炸时的爆炸比例埋深称为等效封闭爆炸临界埋深,可知等效封闭爆炸临界埋深与封闭爆炸临界埋深为两个不同概念:对于土中化学爆炸,当装药埋深与封闭爆炸空腔半径相当时,漏斗坑位于装药中心以下的部分呈半球型,半径与封闭爆炸半径基本相同,此时地下爆炸辐射至地下的地冲击参数基本不受来自地表的影响,介质中爆炸压缩波的幅值与完全封闭爆炸产生的幅值几乎接近,因此可以将封闭爆炸空腔半径作为等效封闭爆炸临界埋深。
对于岩土体介质中爆炸,目前一致认为可以利用公式
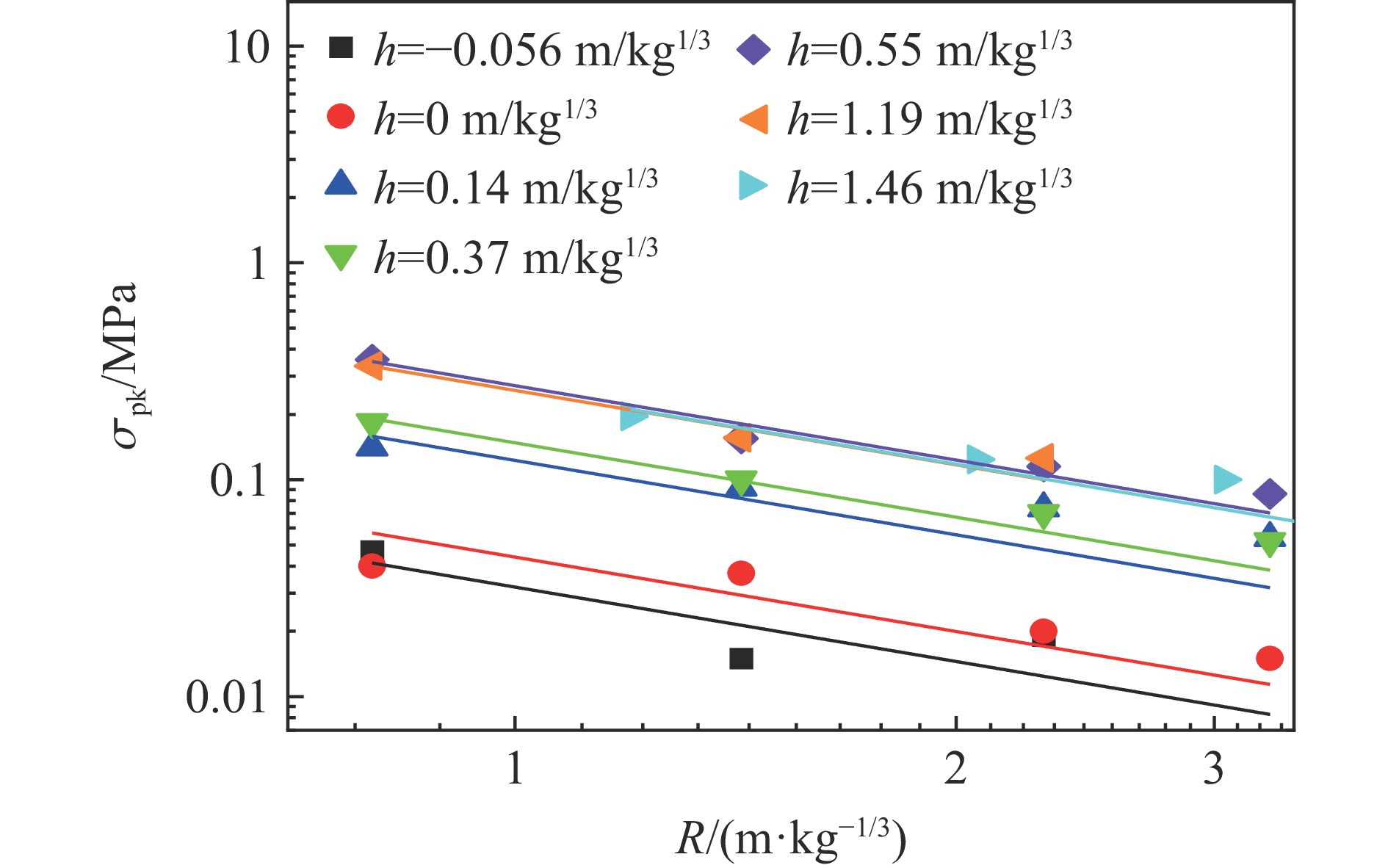
σ=A(r/Q1/3)−n 描述地冲击应力传播的衰减规律;其中:A和n分别为应力经验表达式的衰减系数和衰减指数;r为爆心距,单位m;Q为炸药质量,单位kg。图12给出了不同装药比例埋深条件下,地冲击压力传播衰减曲线,表4给出了对于的A、n值。衰减指数n反映了随爆心比例距离的增加地冲击传播的衰减规律,只与岩土体介质的物理力学性质相关,与装药埋深无关。为了上述公式简洁性,对不同装药比例埋深条件下衰减指数n求平均值,其平均值记为ˉn ,可得实验所用黏土的地冲击传播平均衰减指数ˉn 为1.14。衰减系数A则反映了随装药埋深增加耦合至岩土中的地冲击应力的变化规律;将平均衰减指数为1.14条件下的不同比例埋深的衰减系数记为A',从表4可以看出,当装药比例埋深h≥0.55 m/kg1/3时,随装药埋深增加,A和A'值变化均趋近平缓,进一步说明对于黏土,可将h≈0.55 m/kg1/3作为等效封闭爆炸临界埋深。表 4 不同装药比例埋深条件下拟合参数Table 4. Fitting parameters with different scaled buried depths of chargeh/(m·kg−1/3) n A ˉn A' −0.056 1.16 0.035 1.14 0.031 0 1.05 0.038 0.041 0.14 1.09 0.120 0.123 0.37 1.07 0.124 0.148 0.55 1.23 0.261 0.258 1.19 1.16 0.267 0.258 1.46 1.19 0.269 0.260 3. 成坑地冲击耦合规律分析与等效当量计算
3.1 成坑与地冲击耦合规律理论分析
岩土介质中发生爆炸时,爆炸空腔中的爆轰产物挤压四周的岩土介质扩张,并形成冲击波向外传播,耦合进介质中的能量可以通过压缩边界(非弹性变形区边界)径向力沿边界位移所做的功进行计算,有约一半的能量变为动能[23]:
W=12Sr∫ur(∞)0σrdur (1) 式中:
Sr 为半径为r的球形表面积,ur 为r处岩土的径向位移,ur(∞) 时间趋于无穷的最终位移大小,σr 为r处的径向应力。文献[24-25]给出了封闭爆炸和触地爆炸时从弹性边界传播出的能量,推导发现对于浅埋爆炸而言,弹性区边界处传播出的能量Wfailure依然由弹性边界所包围的破坏区岩体体积V*所控制,即Wfailure与V*呈线性关系:
Wfailure=B12τs2Gc2pc2sV∗ (2) 式中:
cp 为介质中纵波速度,cs 为介质中剪切波速,τs 为剪切强度,G 为剪切模量,B=[5+3(1+24υ)2]/64 取决于爆源周围岩石介质的性质,υ 为岩土介质的泊松比。图13给出了依据爆炸成坑最终形态岩土介质中爆坑常见的三种形式。对于地下爆炸,从装药中心传播出的地冲击能量向四周传播,但对防护工程而言一般均处于装药中心下方。对于有效的地冲击能量,通常只考虑装药中心以下的半空间范围,因此在本文中所述的地冲击能量均指有效地冲击能量。
耦合入岩土介质中的地冲击能量源自于爆炸空腔扩张压缩做功,当爆炸空腔扩张停止后,爆炸产生的能量耦合入介质的过程基本结束。根据已有的数据发现,可以用Boltzmann函数来表达爆炸地冲击能量耦合系数ηe(h)变化规律,结合函数的特点,只要分别求出触地爆炸和封闭爆炸的能量耦合系数(ηe(0)和ηe(∞)),即可描绘能量耦合系数的发展规律:
ηe(h)=1−2(1−D1)eh/D2+1 (3) 式中:D1为触地爆炸时的能量耦合系数,即
ηe(0)=D1 ;理论上,埋深为无限大时,h→∞ ,ηe→1 ,通过本实验发现,存在着最小封闭爆炸的最小比例埋深,该值与D2相关联,决定着曲线的发展轨迹,可取ηe=0.99 时,当作封闭爆炸能量完全耦合。当装药比例埋深大于等效封闭爆炸临界埋深时,传入岩土介质中下方的有效地冲击能量已达到饱和,本文中选取h = 1.19 m/kg1/3作为参考点,代入式(3)中可得:D2=1.19ln(199−200D1) (4) 根据表2数据得:
{D1=ηe(0)=(2/3)π×0.1842×0.260(2/3)π×0.4783=0.081D2=1.19ln(199−200×0.081)=0.228 (5) 代入式(3),得到地冲击能量耦合系数
ηe(h) 曲线如图14所示。对于相等的爆炸当量地下爆炸,在装药比例埋深
h=1.19m/kg1/3 ,爆炸地冲击能量W超出触地爆炸地冲击能量W0的倍数为:WW0=(2/3)π×0.4783(2/3)π×0.1842×0.260≈12.4 (6) 3.2 浅埋爆炸等效当量耦合系数与地冲击参数应力/粒子速度耦合系数
对于封闭爆炸,质点峰值速度计算公式为:
vpk(r)=Kv(rQ1/3)−n,σpk(r)=ρcpvpk(r) (7) 式中:
Kv 为地冲击质点峰值速度经验表达式的衰减系数,Kv 与n 均可由实验得到;vpk 为质点峰值速度;σpk 为峰值应力;ρ为介质密度;Q 为药球当量;cp 为纵波波速。对于浅埋爆炸,实际计算时采用当量耦合系数或地冲击应力耦合系数将浅埋爆炸变为等效的封闭爆炸或者触地爆炸,即利用式(8)和(9)进行求解。
当量耦合系数
ηQ 为在同种介质中,比例爆心距相等时产生相同地冲击参数(应力、速度、加速度等)大小的封闭爆炸与浅埋爆炸的当量比值,即为:ηQ=QeffQ (8) 式中:
Q 为装药比例埋深为h 的爆炸当量;Qeff 为等效的封闭爆炸当量。地冲击参数应力耦合系数
ησ 为在同种介质中,爆炸当量和比例爆心距均相等时浅埋爆炸地冲击参数与封闭爆炸地冲击参数比值,即为:ησ=σσclose=vvclose=aaclose=uuclose (9) 式中:σ、v、a、u分别为地冲击应力、粒子速度、加速度以以及介质位移,下标close表示封闭爆炸。
由此,对于浅埋爆炸,地冲击质点峰值速度可表达为
vpk(r)=Kv(rQ1/3eff)−n=ησKv(rQ1/3)−n (10) 于是得到
ησ 与ηQ 的对应关系:ησ=ηQn/3 (11) 为准确计算浅埋爆炸等效封闭当量,还需要建立当量耦合系数与能量耦合系数间的关系。对于地下爆炸,距离装药中心为
r 处介质最大质点径向速度的表达式为:vr,max=v∗(rr*)−n (12) 式中:
v∗ 为破坏边界处粒子速度,r∗ 为破坏边界与装药中心距离。对于封闭爆炸,装药中心下方破坏区呈半球形,且有
r∗=kQ1/133 ,式(12)可改写为:vr,max=v∗(rr∗)−n=Kv(rQ1/3)−n (13) 式中:
Kv=v∗kn ,k为 破坏区半径比例系数,和岩土介质的力学性质有关。就岩土介质中的浅埋爆炸,装药中心下方破坏区截面边界为抛物线型,如图13(a)和图13(b)所示,可定义装药中心下方破坏区竖直高度为破坏区水平半径的
λ(h) 倍,随着h变大,λ(h) 接近于1,即随着装药比例埋深的变大,装药中心下方破坏区的轮廓由抛物线形渐变为半球形,如图13(c)。如果按破坏区体积进行等效,即将浅埋爆炸破坏区体积等效为封闭爆炸破坏区体积:23π r∗[r∗/λ(h)]2≈23π r∗3eff ,利用式(13),进行地冲击粒子速度计算,则对于装药中心正下方:vr,max=v∗(rr∗)−n=v∗(rλ2/233r*eff)−n=Kv(rQ1/3eff)−n=v∗(rkQ1/133eff)−n (14) 通过式(14)得,
λ2/233r∗eff=kQ1/3eff ,依据式(2)爆炸冲击波能量与破坏区体积间关系,可以建立三种耦合系数间的关系:ηe=ηQλ2=η3/nσλ2 (15) 3.3 黏土中化学爆炸地冲击应力参数耦合系数和当量耦合系数
由计算而来的
ηe ,依据式(15)计算地冲击参数应力耦合系数ησ ,结果如图15(a)所示,其中n取1.14。计算得到ησ 并与实测值对比,如图15(a)所示。从图15(a)中可以看出,地冲击参数应力耦合系数ησ 计算值与地冲击粒子速度实测值整体上吻合度较好。与TM5-855-1[2] 地冲击参数应力耦合曲线和施鹏[10]根据数值模拟得到的曲线进行比较,可以看出,本实验黏土的地冲击参数应力耦合系数ησ 起点较施鹏[10]数值计算的数据较相近,当装药比例埋深增加到0.40~0.60 m/kg1/3之间时,地冲击参数应力耦合系数ησ 和施鹏[10]数值计算曲线符合度高,之后随着装药比例埋深的增加,地冲击参数应力耦合系数ησ 逐渐趋近1,从而也验证了利用有效弹坑体积计算地冲击耦合系数的可行性与可信性,在工程方面偏向安全。图15(b)中当量耦合系数
ηQ 同样是由式(15)换算而得,随着装药比例埋深的增加,当量耦合系数ηQ 先快速增加,装药比例埋深增加到0.55 m/kg1/3后,当量耦合系数ηQ 增加变得缓慢,最后逐渐趋近于1。为便于实际工程应用,将图15(b)中黏土当量耦合系数表达成如下关系:ηQ=1−0.91e−h/0.33 (16) 4. 结 论
通过在
∅ 1500 mm×1490 mm分层式爆炸装置开展了变埋深黏土中爆炸实验,利用3D扫描仪和预埋土压力传感器分别测得不同埋深的弹坑尺寸和爆炸冲击应力,从现场实验和理论分析给出了弹坑压缩体积与耦合地冲击能量之间关系,得到如下结论:(1) 地下爆炸发生后,随着埋深增加,有效弹坑轮廓逐渐由抛物线型发展为半球型,其形状演化过程与地冲击耦合过程同步;黏土的等效封闭爆炸临界埋深约为0.55 m/kg1/3,数值上略大于地下封闭爆炸空腔半径,与Conwep计算程序预测的值基本一致;通过黏土中可视弹坑演化过程可知,黏土的封闭爆炸临界埋深约为1.46 m/kg1/3;
(2) 对于本实验中的黏土,当−0.056 m/kg1/3≤h≤0.37 m/kg1/3,埋深增加对爆炸效应有加强的作用;在h≥0.55 m/kg1/3时,埋深增加,衰减系数处于稳定状态,爆炸地冲击基本完全耦合,埋深再增加爆炸耦合地冲击能量此时可忽略;
(3) 爆炸耦合进介质中能量正比于有效弹坑的体积,此结论适用于浅埋爆炸和封闭爆炸;对于浅埋爆炸,可以把不规则轮廓通过等效面积转化为规则的球体进行计算;黏土爆炸实验证实了通过有效弹坑体积方式计算地冲击耦合系数的可行性与可信性;
(4) 建立了三种地冲击耦合系数的关系,最终都可与有效弹坑体积建立联系,引入Boltzmann函数给出了黏土耦合系数与比例埋深的关系;为了便于实际工程应用,文中直接给出了黏土的当量耦合系数与比例埋深的函数关系,此公式具有较好的预估精度。
-
-
[1] ANZ-MEADOR P D, OPIELA J N, SHOOTS D, et al. History of on-orbit satellite fragmentations: NASA/TM-2018-220037 [R]. Houston, Texas: Lyndon B. Johnson Space Center, NASA, 2018. [2] 龚自正, 徐坤博, 牟永强, 等. 空间碎片环境现状与主动移除技术 [J]. 航天器环境工程, 2014, 31(2): 129–135. DOI: 10.12126/see.2014.02.003.GONG Z Z, XU K B, MU Y Q, et al. The space debris environment and the active debris removal techniques [J]. Spacecraft Environment Engineering, 2014, 31(2): 129–135. DOI: 10.12126/see.2014.02.003. [3] 郑世贵, 闫军. 空间碎片防护需求与防护材料进展 [J]. 国际太空, 2014(6): 49–53.ZHENG S G, YAN J. A review on the space debris protection and protective materials [J]. Space International, 2014(6): 49–53. [4] 龚自正, 杨继运, 张文兵, 等. 航天器空间碎片超高速撞击防护的若干问题 [J]. 航天器环境工程, 2007, 24(3): 125–130. DOI: 10.3969/j.issn.1673-1379.2007.03.001.GONG Z Z, YANG J Y, ZHANG W B, et al. Spacecraft protection from the hypervelocity impact of space meteoroid and orbital debris [J]. Spacecraft Environment Engineering, 2007, 24(3): 125–130. DOI: 10.3969/j.issn.1673-1379.2007.03.001. [5] WHIPPLE F L. Meteorites and space travel [J]. The Astronomical Journal, 1947, 52: 131. DOI: 10.1086/106009. [6] PIEKUTOWSKI A J. Formation and description of debris clouds produced by hypervelocity impact [R]. Huntsville, Alabama: Marshall Space Flight Center, NASA, 1996. [7] ANDERSON JR C E, TRUCANO T G, MULLIN SA. Debris cloud dynamics [J]. International Journal of Impact Engineering, 1990, 9(1): 89–113. DOI: 10.1016/0734-743X(90)90024-P. [8] PIEKUTOWSKI A J. Debris clouds generated by hypervelocity impact of cylindrical projectiles with thin aluminum plates [J]. International Journal of Impact Engineering, 1987, 5(1–4): 509–518. DOI: 10.1016/0734-743X(87)90066-2. [9] MORRISON R H. A preliminary investigation of projectile shape effects in hypervelocity impact of a double-sheet structure: TN-D-6944 [R]. Washington: National Aeronautics and Space Administration, 1972. [10] MACLAY T D, CULP R D, BAREISS L, et al. Topographically modified bumper concepts for spacecraft shielding [J]. International Journal of Impact Engineering, 1993, 14(1–4): 479–489. DOI: 10.1016/0734-743X(93)90044-8. [11] COUR-PALAIS B G, CREWS J L. A multi-shock concept for spacecraft shielding [J]. International Journal of Impact Engineering, 1990, 10(1–4): 135–146. DOI: 10.1016/0734-743X(90)90054-Y. [12] CHRISTIANSEN E L. Performance equations for advanced orbital debris shields [C]// Space Programs and Technologies Conference. Huntsivolle: AIAA, 1992. [13] CREWS J L, CHRISTIANSEN E L. The NASA JSC hypervelocity impact test facility (HIT-F) [C]// Space Programs and Technologies Conference. Huntsivolle: AIAA, 1992. DOI: 10.2514/6.1992-1640. [14] CHRISTIANSEN E L, CREWS J L, WILLIAMSEN J E, et al. Enhanced meteoroid and orbital debris shielding [J]. International Journal of Impact Engineering, 1995, 17(1–3): 217–228. DOI: 10.1016/0734-743X(95)99848-L. [15] DESTEFANIS R, SCHÄFER F, LAMBERT M, et al. Enhanced space debris shields for manned spacecraft [J]. International Journal of Impact Engineering, 2003, 29(1–10): 215–226. DOI: 10.1016/j.ijimpeng.2003.09.019. [16] RYAN S, HEDMAN T, CHRISTIANSEN E L. Honeycomb vs. foam: evaluating potential upgrades to ISS module shielding [J]. Acta Astronautica, 2010, 67: 818–825. DOI: 10.1016/j.actaastro.2010.05.021. [17] 周昊, 郭锐, 南博华, 等. 填充式波纹夹层结构超高速撞击特性仿真 [J]. 国防科技大学学报, 2017, 39(2): 57–63. DOI: 10.11887/j.cn.201702008.ZHOU H, GUO R, NAN B H, et al. Simulation on hypervelocity impact characteristics of stuffed corrugation-cored sandwiches [J]. Journal of National University of Defense Technology, 2017, 39(2): 57–63. DOI: 10.11887/j.cn.201702008. [18] SCHONBERG W P, TULLOS R J. Spacecraft wall design for increased protection against penetration by orbital debris impacts [J]. AIAA Journal, 1991, 29(12): 2207–2214. DOI: 10.2514/6.1990-3663. [19] KAWAI N, KURODA Y, NAGANO M, et al. Stress-wave propagation and damage formation associated with hypervelocity penetration into polycarbonate [J]. Procedia Engineering, 2017, 204: 255–261. DOI: 10.1016/j.proeng.2017.09.733. [20] KUMAR S K S, JURADO-MANRIQUEZ E A, KIM Y H, et al. Polybenzimidazole (PBI) film coating for improved hypervelocity impact energy absorption for space applications [J]. Composite Structures, 2018, 188: 72–77. DOI: 10.1016/j.compstruct.2017.12.052. [21] LI T, YU X, LIU H F, et al. Tensile behavior of C/SiC composites plate after hypervelocity penetration: residual strength and fracture mechanism [J]. Composite Structures, 2018, 189: 378–385. DOI: 10.1016/j.compstruct.2018.01.058. [22] WU Q, ZHANG Q M, LONG R R, et al. Potential space debris shield structure using impact-initiated energetic materials composed of polytetrafluoroethylene and aluminum [J]. Applied Physics Letters, 2016, 108: 135–183. DOI: 10.1063/1.4943584. [23] COUR-PALAIS B G. Meteorid environment model: NASA/SP-8013 [R]. Washington: National Aeronautics and Space Administration, 1969. DOI: CDSTIC.GRA.00159492. [24] CHRISTIANSEN E L. Meteoroid/debris shielding: NASA/TP-2003-210788 [R]. Houston, Texas: NASA Johnson Space Center, 2003. [25] HAYASHIDA K B, ROBINSON J H. Double-plate penetration equations: NASA/TM-2000-209907 [R]. Alabama: Marshall Space Flight Center, NASA, 2000. [26] 郑建东, 龚自正, 童靖宇, 等. 一种新的Whipple防护结构弹道极限方程准确率分析 [J]. 航天器环境工程, 2012, 29(2): 134–138. DOI: 10.3969/j.issn.1673-1379.2012.02.004.ZHENG J D, GONG Z Z, TONG J Y, et al. Accuracy analysis of a new Whipple shield ballistic limit equations [J]. Spacecraft Environment Engineering, 2012, 29(2): 134–138. DOI: 10.3969/j.issn.1673-1379.2012.02.004. [27] CHRISTIANSEN E L, KERR J H. Mesh double-bumper shield: a low-weight alternative for spacecraft meteoroid and orbital debris protection [J]. International Journal of Impact Engineering, 1993, 14(1 –4): 169–180. DOI: 10.1016/0734-743X(93)90018-3. [28] CHRISTIANSEN E L. Advanced meteoroid and debris shielding concepts [C]// Orbital Debris Conference: Technical Issues and Future Directions, AIAA. 1990. DOI: 10.2514/6.1990-1336. [29] CHRISTIANSEN E L, KERR J H, DE LA FUENTE H M, et al. Flexible and deployable meteoroid/debris shielding for spacecraft [J]. International Journal of Impact Engineering, 1999, 23: 125–136. DOI: 10.1016/S0734-743X(99)00068-8. [30] RYAN S, CHRISTIANSEN E L. Hypervelocity impact testing of advanced materials and structures for micrometeoroid and orbital debris shielding [J]. Acta Astronautica, 2013, 83: 216–231. DOI: 10.1016/j.actaastro.2012.09.012. [31] 贾斌, 马志涛, 庞宝君. 填充泡沫铝防护结构的超高速撞击数值模拟 [J]. 哈尔滨工业大学学报, 2011, 43(1): 16–20. DOI: 10.11918/j.issn.0367-6234.2011.01.004.JIA B, MA Z T, PANG B J. Numerical simulation investigation in hypervelocity impact on Al-foam stuffed shields [J]. Journal of Harbin Institute of Technology, 2011, 43(1): 16–20. DOI: 10.11918/j.issn.0367-6234.2011.01.004. [32] 贾斌, 马志涛, 庞宝君. 含泡沫铝防护结构的超高速撞击数值模拟研究 [J]. 高压物理学报, 2009, 23(6): 453–459. DOI: 10.11858/gywlxb.2009.06.009.JIA B, MA Z T, PANG B J. Numerical simulation investigation in hypervelocity impact on shield structure containing aluminum foam [J]. Chinese Journal of High Pressure Physics, 2009, 23(6): 453–459. DOI: 10.11858/gywlxb.2009.06.009. [33] 刘文祥, 张德志, 张向荣, 等. 填充式泡沫铝防护结构的弹道极限 [J]. 爆炸与冲击, 2012, 32(1): 43–46. DOI: 10.11883/1001-1455(2012)01-0043-04.LIU W X, ZHANG D Z, ZHANG X R, et al. Ballistic limit of an aluminum foam-filledshield [J]. Explosion and Shock Waves, 2012, 32(1): 43–46. DOI: 10.11883/1001-1455(2012)01-0043-04. [34] DESTEFANIS R, SCHÄFER F, LAMBERT M, et al. Selecting enhanced space debris shields for manned spacecraft [J]. International Journal of Impact Engineering, 2006, 33(1–12): 219–230. DOI: 10.1016/j.ijimpeng.2006.09.065. [35] TAYLOR E A, GLANVILLE J P, CLEGG R A, et al. Hypervelocity impact on spacecraft honeycomb: hydrocode simulation and damage laws [J]. International Journal of Impact Engineering, 2003, 29: 691–702. DOI: 10.1016/j.ijimpeng.2003.10.016. [36] TAYLOR E A. Computational study of hypervelocity impact onto Whipple bumpers and sandwich plates with honeycomb core [R]. European Space Agency/European Space Research and Technology Centre, 1999. [37] RYAN S, SCHÄFER F, DESTEFANIS R, et al. A ballistic limit equation for hypervelocity impacts on composite honeycomb sandwich panel satellite structures [J]. Advances in Space Research, 2008, 41(7): 1152–1166. DOI: 10.1016/j.asr.2007.02.032. [38] SIBEAUD J-M, PRIEUR C, PUILLET C. Hypervelocity impact on honeycomb target structures: experimental part [C]// The 4th European Conference on Space Debris. Darmstadt, Germany: The European Space Agency, 2005. [39] SIBEAUD J-M, THAMIE L, PUILLET C. Hypervelocity impact on honeycomb target structures: experiments and modeling [J]. International Journal of Impact Engineering, 2008, 35(12): 1799–1807. DOI: 10.1016/j.ijimpeng.2008.07.037. [40] JEX D W, MAC KAY C, MILLER A. The characteristics of penetration for a double-sheet structure with honeycomb: NASA/TM-X-53974 [R]. Huntsville: Marshall Space Flight Center, NASA, 1970. [41] DECONINCK P, ABDULHAMID H, HÉREIL P L, et al. Experimental and numerical study of submillimeter-sized hypervelocity impacts on honeycomb sandwich structures [J]. Procedia engineering, 2017, 204: 452–459. DOI: 10.1016/j.proeng.2017.09.740. [42] NITTA K, HIGASHIDE M, KITAZAWA Y, et al. Response of a aluminum honeycomb subjected to hypervelocity impacts [J]. Procedia Engineering, 2013, 58: 709–714. DOI: 10.1016/j.proeng.2013.05.082. [43] SCHONBERG W, SCHÄFER F, PUTZAR R. Hypervelocity impact response of honeycomb sandwich panels [J]. Acta Astronautica, 2010, 66(3–4): 455–466. DOI: 10.1016/j.actaastro.2009.06.018. [44] ANON. Effectiveness of aluminum honeycomb shields in preventing meteoroid damage to liquid-filled spacecraft tanks: NASA/CR-65261 [R]. Salt Lake City: Utah Research and Development Co. Inc., NASA, 1964. [45] LATHROP B L, SENNETT R E. Effects of hypervelocity impact on honeycomb structures [J]. Journal of Spacecraft and Rockets, 1968, 5(12): 1496–1497. DOI: 10.2514/3.29514. [46] LAMBERT M, SCHÄFER F K, GEYER T. Impact damage on sandwich panels and multi-layer insulation [J]. International Journal of Impact Engineering, 2001, 26(1–10): 369–380. DOI: 10.1016/S0734-743X(01)00108-7. [47] KANG P, YOUN S K, LIM J H. Modification of the critical projectile diameter of honeycomb sandwich panel considering the channeling effect in hypervelocity impact [J]. Aerospace Science and Technology, 2013, 29: 413–425. DOI: 10.1016/j.ast.2013.04.011. [48] RYAN S, ORDONEZ E, CHRISTIANSEN E L, et al. Hypervelocity impact performance of open cell foam core sandwich panel structures [C]// The 11th Hypervelocity Impact Symposium. Freiburg, Germany, 2010. [49] YASENSKY J, CHRISTIANSEN E L. Hypervelocity impact evaluation of metal foam core sandwich structures: NASA/JSC63945 [R]. NASA, 2007. [50] RYAN S, HEDMAN T, CHRISTIANSEN E L. Honeycomb vs. foam: evaluating a potential upgrade to international space station module shielding for micrometeoroids and orbital debris: NASA/TM-2009-214793 [R]. Arizona: USRA Lunar and Planetary Institute, NASA, 2009. [51] VOILLAT R, GALLIEN F, MORTENSEN A, et al. Hypervelocity impact testing on stochastic and structured open porosity cast Al-Si cellular structures for space applications [J]. International Journal of Impact Engineering, 2018, 120: 126–137. DOI: 10.1016/j.ijimpeng.2018.05.002. [52] GAITANAROS S, KYRIAKIDES S. On the effect of relative density on the crushing and energy absorption of open-cell foams under impact [J]. International Journal of Impact Engineering, 2015, 82: 3–13. DOI: 10.1016/j.ijimpeng.2015.03.011. [53] SHI X P, LIU S Y, NIE H L, et al. Study of cell irregularity effects on the compression of closed-cell foams [J]. International Journal of Mechanical Sciences, 2018, 135: 215–225. DOI: 10.1016/j.ijmecsci.2017.11.026. [54] SANCHEZ G A, CHRISTIANSEN E L. FGB energy block meteoroid and orbital (M/OD) debris shield test report: NASA/JSC-27460 [R]. Washington: National Aeronautics and Space Administration, 1996. [55] THOMA K, SCHÄFER F, HIERMAIER S, et al. An approach to achieve progress in spacecraft shielding [J]. Advances in Space research, 2004, 34(5): 1063–1075. DOI: 10.1016/j.asr.2003.03.034. [56] 张雄, 刘岩, 马上. 无网格法的理论及应用 [J]. 力学进展, 2009, 39(1): 1–36. DOI: 10.3321/j.issn:1000-0992.2009.01.001.ZHANG X, LIU Y, MA S. Meshfree methods and their applications [J]. Advances in Mechanics, 2009, 39(1): 1–36. DOI: 10.3321/j.issn:1000-0992.2009.01.001. [57] 张雄, 宋康祖, 陆明万. 无网格法研究进展及其应用 [J]. 计算力学学报, 2003, 20(6): 730–742. DOI: 10.7511/jslx20036138.ZHANG X, SONG K Z, LU M W. Research progress and application of meshless method [J]. Chinese Journal of Computational Mechanics, 2003, 20(6): 730–742. DOI: 10.7511/jslx20036138. [58] 胡德安, 韩旭, 肖毅华, 等. 光滑粒子法及其与有限元耦合算法的研究进展 [J]. 力学学报, 2013, 45(5): 639–652. DOI: 10.6052/0459-1879-13-092.HU D A, HAN X, XIAO Y H, et al. Research developments of smoothed particle hydrodynamicsmethod and its coupling with finite element method [J]. Chinese Journal of Theoretical and Applied Mechanics, 2013, 45(5): 639–652. DOI: 10.6052/0459-1879-13-092. [59] GINGOLD R A, MONAGHAN J J. Smoothed particle hydrodynamics: theory and application to non-spherical stars [J]. Monthly Notices of the Royal Astronomical Society, 1977, 181(3): 375–389. DOI: 10.1093/mnras/181.3.375. [60] LUCY L B. A numerical approach to the testing of the fission hypothesis [J]. The Astronomical Journal, 1977, 82(12): 1013–1024. DOI: 10.1086/112164. [61] LIBERSKY L D, PETSCHEK A G. Smooth particle hydrodynamics with strength of materials [J]. Advances in the Free Lagrange Method, 1990, 248: 248–257. DOI: 10.1007/3-540-54960-9_58. [62] LIBERSKY L D, RANDLES P W, CARNEY T C, et al. Recent improvements in SPH modeling of hypervelocity impact [J]. International Journal of Impact Engineering, 1997, 20(6–10): 525–532. DOI: 10.1016/S0734-743X(97)87441-6. [63] LIBERSKY L D, PETSCHEK A G, CARNEY T C, et al. High strain Lagrangian hydrodynamics: a three-dimensional SPH code for dynamic material response [J]. Journal of Computational Physics, 1993, 109(1): 67–75. DOI: 10.1006/jcph.1993.1199. [64] RANDLES P W, LIBERSKY L D. Smoothed particle hydrodynamics: some recent improvements and applications [J]. Computer Methods in Applied Mechanics and Engineering, 1996, 139(1–4): 375–408. DOI: 10.1016/S0045-7825(96)01090-0. [65] HAYHURST C J, CLEGG R A. Cylindrically symmetric SPH simulations of hypervelocity impacts on thin plates [J]. International Journal of Impact Engineering, 1997, 20(1–5): 337–348. DOI: 10.1016/S0734-743X(97)87505-7. [66] 崔伟峰, 曾新吾. SPH算法在超高速碰撞数值模拟中的应用 [J]. 国防科技大学学报, 2007, 29(2): 43–46. DOI: 10.3969/j.issn.1001-2486.2007.02.010.CUI W F, ZENG X W. Smoothed particle hydrodynamics algorithm applied in numerical simulation of hypervelocity impact [J]. Journal of National University of Defense Technology, 2007, 29(2): 43–46. DOI: 10.3969/j.issn.1001-2486.2007.02.010. [67] 徐志宏, 汤文辉, 罗永. SPH 算法在高速侵彻问题中的应用 [J]. 国防科技大学学报, 2005, 27(4): 41–44. DOI: 10.3969/j.issn.1001-2486.2005.04.010.XU Z H, TANG W H, LUO Y. Smoothed particle hydrodynamics algorithm applied in penetration problem [J]. Journal of National University of Defense Technology, 2005, 27(4): 41–44. DOI: 10.3969/j.issn.1001-2486.2005.04.010. [68] LIU G R, LIU M B, LI S F. Smoothed particle hydrodynamics: a meshfree method [J]. Computational Mechanics, 2004, 33: 491–491. DOI: 10.1007/s00466-004-0573-1. [69] DYKA C T, INGEL R P, FLIPPEN L D. A new approach to dynamic condensation for FEM [J]. Computers & Structures, 1995, 61(4): 763–773. DOI: 10.1016/0045-7949(96)00017-X. [70] SWEGLE J W, HICKS D L, ATTAWAY S W. Smoothed particle hydrodynamics stability analysis [J]. Journal of Computational Physics, 1995, 116(1): 123–134. DOI: 10.1006/jcph.1995.1010. [71] 傅学金, 强洪夫, 杨月诚. 固体介质中SPH方法的拉伸不稳定性问题研究进展 [J]. 力学进展, 2007, 37(3): 375–388. DOI: 10.3321/j.issn:1000-0992.2007.03.005.FU X J, QIANG H F, YANG Y C. Advances in the tensile instability of smoothed particle hydrodynamics applied to solid dynamics [J]. Advances in Mechanics, 2007, 37(3): 375–388. DOI: 10.3321/j.issn:1000-0992.2007.03.005. [72] MORRIS J P. A study of the stability properties of smooth particle hydrodynamics [J]. Publications of the Astronomical Society of Australia, 1996, 13(1): 97–102. DOI: 10.1017/S1323358000020610. [73] 刘谋斌, 常建忠. 光滑粒子动力学方法中粒子分布与数值稳定性分析 [J]. 物理学报, 2010, 59(6): 3654–3662. DOI: 10.7498/aps.59.3654.LIU M B, CHANG J Z. Particle distribution and numerical stability in smoothed particle hydrodynamics method [J]. Acta Physica Sinica, 2010, 59(6): 3654–3662. DOI: 10.7498/aps.59.3654. [74] 卞梁, 王肖钧, 章杰, 等. 高速碰撞数值计算中的 SPH 分区算法 [J]. 计算物理, 2011, 28(2): 207–212. DOI: 10.3969/j.issn.1001-246X.2011.02.007.BIAN L, WANG X J, ZHANG J, et al. Numerical simulation of hypervelocity impact with subdomains in SPH computation [J]. Chinese Journal of Computational Physics, 2011, 28(2): 207–212. DOI: 10.3969/j.issn.1001-246X.2011.02.007. [75] 倪国喜, 王瑞利, 林忠, 等. 任意区域上的粒子均匀分布方法 [J]. 计算力学学报, 2007, 24(4): 408–413. DOI: 10.3969/j.issn.1007-4708.2007.04.005.NI G X, WANG R L, LIN Z, et al. Equi-distribution of particles in arbitrary domain [J]. Chinese Journal of Computational Mechanics, 2007, 24(4): 408–413. DOI: 10.3969/j.issn.1007-4708.2007.04.005. [76] 董晃晃. SPH 的粒子生成方法及其在弹体侵彻金属靶中的应用[D]. 南昌: 华东交通大学, 2017.DONG H H. Particle generation method for SPH and the application of SPH to penetration of metal targets by projectiles [D]. Nanchang: East China Jiaotong University, 2017. [77] DYKA C T, RANDLES P W, INGEL R P. Stress point for tension instability in SPH [J]. International Journal for Numerical Methods in Engineering, 1997, 40(13): 2325–2341. DOI: 10.1002/(SICI)1097-0207(19970715)40:13<2325::AID-NME161>3.0.CO,2. [78] MONAGHAN J J. SPH without a tensile instability [J]. Journal of Computational Physics, 2000, 159(2): 290–311. DOI: 10.1006/jcph.2000.6439. [79] LIU M B, LIU G R, ZONG Z. An overview on smoothed particle hydrodynamics [J]. International Journal of Computational Methods, 2008, 5(1): 135–188. DOI: 10.1142/S021987620800142X. [80] LIU M B, XIE W P, LIU G R. Modeling incompressible flows using a finite particle method [J]. Applied Mathematical Modelling, 2005, 29(12): 1252–1270. DOI: 10.1016/j.apm.2005.05.003. [81] LIU M B, LIU G R. Restoring particle consistency in smoothed particle hydrodynamics [J]. Applied Numerical Mathematics, 2006, 56(1): 19–36. DOI: 10.1016/j.apnum.2005.02.012. [82] 杨秀峰, 刘谋斌. 光滑粒子动力学 SPH 方法应力不稳定性的一种改进方案 [J]. 物理学报, 2012, 61(22): 224701. DOI: 10.7498/aps.61.224701.YANG X F, LIU M B. Improvement on stress instability in smoothed particle hydrodynamics [J]. Acta Physica Sinica, 2012, 61(22): 224701. DOI: 10.7498/aps.61.224701. [83] GRADY D E, WINFREE N A. Impact fragmentation of high-velocity compact projectiles on thin plates: a physical and statistical characterization of fragment debris [J]. International Journal of Impact Engineering, 2001, 26(1 –10): 249–262. DOI: 10.1016/S0734-743X(01)00085-9. [84] NAKAMURA A, FUJIWARA A. Velocity distribution of fragments formed in a simulated collisional disruption [J]. Icarus, 1991, 92(1): 132–146. DOI: 10.1016/0019-1035(91)90040-Z. [85] HOCKNEY R W, EASTWOOD J W. Computer simulation using particles [M]. New York: McGraw-Hill, 1981. [86] HOCKNEY R W, EASTWOOD J W. Computer simulation using particles [M]. Boca Raton: CRC Press, 1988. [87] BENZ W. Smooth particle hydrodynamics: a review [C] // The Numerical Modelling of Nonlinear Stellar Pulsations. Dordrecht: NATO ASI Series, 1990: 269–288. DOI: 10.1007/978-94-009-0519-1_16. [88] BENZ W, ASPHAUG E. Impact simulations with fracture: I: method and tests [J]. Icarus, 1994, 107(1): 98–116. DOI: 10.1006/icar.1994.1009. [89] BENZ W, ASPHAUG E. Simulations of brittle solids using smooth particle hydrodynamics [J]. Computer Physics Communications, 1995, 87(1–2): 253–265. DOI: 10.1016/0010-4655(94)00176-3. [90] 徐金中, 汤文辉, 徐志宏. 超高速碰撞碎片云特征的SPH方法数值分析 [J]. 高压物理学报, 2008, 22(4): 377–383. DOI: 10.11858/gywlxb.2008.04.007.XU J Z, TANG W H, XU Z H. Numerical analysis of the characteristics of debris clouds produced by hypervelocity impacts using SPH method [J]. Chinese Journal of High Pressure Physics, 2008, 22(4): 377–383. DOI: 10.11858/gywlxb.2008.04.007. [91] LIANG S C, LI Y, CHEN H, et al. Research on the technique of identifying debris and obtaining characteristic parameters of large-scale 3D point set [J]. International Journal of Impact Engineering, 2013, 56: 27–31. DOI: 10.1016/j.ijimpeng.2012.07.004. [92] SAKONG J, WOO S C, KIM T W. Determination of impact fragments from particle analysis via smoothed particle hydrodynamics and k-means clustering [J]. International Journal of Impact Engineering, 2019, 134: 103387. DOI: 10.1016/j.ijimpeng.2019.103387. [93] ZHANG X T, JIA G H, HUANG H. Fragment identification and statistics method of hypervelocity impact SPH simulation [J]. Chinese Journal of Aeronautics, 2011, 24: 18–24. DOI: 10.1016/S1000-9361(11)60003-4. [94] 张晓天, 贾光辉, 黄海. 基于 FE 重构方法的冲击破碎仿真 [J]. 计算力学学报, 2011, 28(5): 792–797. DOI: 10.7511/jslx201105024.ZHANG X T, JIA G H, HUANG H. Combination of FE and SPH method for impact fragmentation [J]. Chinese Journal of Computational Mechanics, 2011, 28(5): 792–797. DOI: 10.7511/jslx201105024. [95] ZHANG X T, JIA G H, HUANG H. Finite element reconstruction approach for on-orbit spacecraft breakup dynamics simulation and fragment analysis [J]. Advances in Space Research, 2013, 51(3): 423–433. DOI: 10.1016/j.asr.2012.09.023. [96] 张晓天, 贾光辉, 黄海. 基于超高速碰撞仿真的卫星碰撞解体碎片分析 [J]. 航空学报, 2011, 32(7): 1224–1230. DOI: CNKI:11-1929/V.20110330.1305.004.ZHANG X T, JIA G H, HUANG H. Debris analysis of on-orbit satellite collision based on hypervelocity impact simulation [J]. Acta Aeronautica Astronautica Sinica, 2011, 32(7): 1224–1230. DOI: CNKI:11-1929/V.20110330.1305.004. [97] ATTAAWAY S W, HEINSTEIN M W, SWEGLE J W. Coupling of smooth particle hydrodynamics with the finite element method [J]. Nuclear Engineering and Design, 1994, 150(2–3): 199–205. DOI: 10.1016/0029-5493(94)90136-8. [98] VUYST TD, VIGNJEVIC R, CAMPBELL J C. Coupling between meshless and finite element methods [J]. International Journal of Impact Engineering, 2005, 31(8): 1054–1064. DOI: 10.1016/j.ijimpeng.2004.04.017. [99] JOHNSON G R. Linking of Lagrangian particle methods to standard finite element methods for high velocity impact computations [J]. Nuclear Engineering and Design, 1994, 150(2–3): 265–274. DOI: 10.1016/0029-5493(94)90143-0. [100] 冷冰林, 许金余, 邵宁, 等. 刚性弹丸侵彻金属靶体的 FEM-SPH 耦合计算 [J]. 弹箭与制导学报, 2008, 28(5): 105–108. DOI: 10.3969/j.issn.1673-9728.2008.05.032.LENG B L, XU J Y, SHAO N, et al. Computation of steel penetrated by rigid projectile with coupled FEM-SPH methods [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2008, 28(5): 105–108. DOI: 10.3969/j.issn.1673-9728.2008.05.032. [101] 肖毅华, 胡德安, 韩旭, 等. 一种自适应轴对称FEM-SPH耦合算法及其在高速冲击模拟中的应用 [J]. 爆炸与冲击, 2012, 32(4): 51–59. DOI: 10.11883/1001-1455(2012)04-0384-09.XIAO Y H, HU D A, HAN X, et al. An adaptive axisymmetric FEM-SPH coupling algorithm and its application to high velocity impact simulation [J]. Explosion and Shock Waves, 2012, 32(4): 51–59. DOI: 10.11883/1001-1455(2012)04-0384-09. [102] 纪冲, 龙源, 方向. 基于FEM-SPH耦合法的弹丸侵彻钢纤维混凝土数值模拟 [J]. 振动与冲击, 2010, 29(7): 69–74. DOI: 10.3969/j.issn.1000-3835.2010.07.015.JI C, LONG Y, FANG X. Numerical simulation for projectile penetrating steel fiber reinforced concrete with FEM-SPH coupling algorithm [J]. Journal of Vibration and Shock, 2010, 29(7): 69–74. DOI: 10.3969/j.issn.1000-3835.2010.07.015. [103] 武玉玉, 何远航, 李金柱. 耦合方法在超高速碰撞数值模拟中的应用 [J]. 高压物理学报, 2005, 19(4): 385–389. DOI: 10.11858/gywlxb.2005.04.019.WU Y Y, HE Y H, LI J Z. Application of the coupling method in simulating the hypervelocity impact [J]. Chinese Journal of High Pressure Physics, 2005, 19(4): 385–389. DOI: 10.11858/gywlxb.2005.04.019. [104] 何远航, 武玉玉, 张庆明. 碰撞倾角对碎片云分布影响的数值模拟 [J]. 北京理工大学学报, 2007, 27(10): 851–854. DOI: 10.3969/j.issn.1001-0645.2007.10.002.HE Y H, WU Y Y, ZHANG Q M. Numerical simulation for the influence of impact angle on debris clouds distribution [J]. Transactions of Beijing Institute of Technology, 2007, 27(10): 851–854. DOI: 10.3969/j.issn.1001-0645.2007.10.002. [105] SAKONG J, WOO S C, KIM J Y, et al. Study on material fracture and debris dispersion behavior via high velocity impact [J]. Transactions of the Korean Society of Mechanical Engineers A, 2017, 41(11): 1065–1075. DOI: 10.3795/KSME-A.2017.41.11.1065. [106] BECKER M, SEIDL M, MEHL M, et al. Numerical and experimental investigation of SPH, SPG, and FEM for high-velocity impact applications [C]// The 12th European LS-DYNA Conference. Koblenz: DYNAmore GmbH, 2019. [107] JOHNSON G R, BEISSEL S R, STRYK R A. An improved generalized particle algorithm that includes boundaries and interfaces [J]. International Journal for Numerical Methods in Engineering, 2002, 53(4): 875–904. DOI: 10.1002/nme.316. [108] JOHNSON G R, STRYK R A. Conversion of 3D distorted elements into meshless particles during dynamic deformation [J]. International Journal of Impact Engineering, 2003, 28(9): 947–966. DOI: 10.1016/S0734-743X(03)00012-5. [109] JOHNSON G R. Numerical algorithms and material models for high-velocity impact computations [J]. International Journal of Impact Engineering, 2011, 38(6): 456–472. DOI: 10.1016/j.ijimpeng.2010.10.017. [110] JOHNSON G R, STRYK R A, BEISSEL S R, et al. An algorithm to automatically convert distorted finite elements into meshless particles during dynamic deformation [J]. International Journal of Impact Engineering, 2002, 27(10): 997–1013. DOI: 10.1016/S0734-743X(02)00030-1. [111] BEISSEL S R, GERLACH C A, JOHNSON G R. Hypervelocity impact computations with finite elements and meshfree particles [J]. International Journal of Impact Engineering, 2006, 33(1–12): 80–90. DOI: 10.1016/j.ijimpeng.2006.09.047. [112] BEISSEL S R, GERLACH C A, JOHNSON G R. A quantitative analysis of computed hypervelocity debris clouds [J]. Cement Technology, 2008, 35(12): 1410–1418. DOI: 10.1016/j.ijimpeng.2008.07.059. [113] JOHNSON G R, BEISSEL S R, GERLACH C A. A 3D combined particle-element method for intense impulsive loading computations involving severe distortions [J]. International Journal of Impact Engineering, 2015, 84: 171–180. DOI: 10.1016/j.ijimpeng.2015.06.006. [114] JOHNSON G R, BEISSEL S R, STRYK R A. A generalized particle algorithm for high velocity impact computations [J]. Computational Mechanics, 2000, 25: 245–256. DOI: 10.1007/s004660050473. [115] GERLACH C A, JOHNSON G R. A contact and sliding interface algorithm for the combined particle-element method [J]. International Journal of Impact Engineering, 2018, 113: 21–28. DOI: 10.1016/j.ijimpeng.2017.11.003. [116] SAUER M. Adaptive kopplung des netzfreien SPH-verfahrens mit finiten elementen zur berechnung von impaktvorgängen [D]. Munich: Universität der Bundeswehr München, 2000. [117] 王吉, 王肖钧, 卞梁. 光滑粒子法与有限元的耦合算法及其在冲击动力学中的应用 [J]. 爆炸与冲击, 2007, 27(6): 44–50. DOI: 10.11883/1001-1455(2007)06-0522-07.WANG J, WANG X J, BIAN L. Linking of smoothed particle hydronamics method to standard finite element method and its application in impact dynamics [J]. Explosion and Shock Waves, 2007, 27(6): 44–50. DOI: 10.11883/1001-1455(2007)06-0522-07. [118] 张志春, 强洪夫, 高巍然. 一种新型SPH-FEM耦合算法及其在冲击动力学问题中的应用 [J]. 爆炸与冲击, 2011, 31(3): 243–249. DOI: 10.11883/1001-1455(2011)03-0243-07.ZHANG Z C, QIANG H F, GAO W R. A new coupled SPH-FEM algorithm and its application to impact dynamics [J]. Explosion and Shock Waves, 2011, 31(3): 243–249. DOI: 10.11883/1001-1455(2011)03-0243-07. [119] HE Q G, CHEN X W, CHEN J F. Finite element-smoothed particle hydrodynamics adaptive method in simulating debris cloud [J]. Acta Astronautica, 2020, 175: 99–117. DOI: 10.1016/j.actaastro.2020.05.056. [120] PIEKUTOWSKI A J. Characteristics of debris clouds produced by hypervelocity impact of aluminum spheres with thin aluminum plates [J]. International Journal of Impact Engineering, 1993, 14(1–4): 573–586. DOI: 10.1016/0734-743X(93)90053-A. [121] PIEKUTOWSKI A J. Fragmentation initiation threshold for spheres impacting at hypervelocity [J]. International Journal of Impact Engineering, 2003, 29(1–10): 563–574. DOI: 10.1016/j.ijimpeng.2003.10.005. [122] HE Y, BAYLY A E, HASSANPOUR A, et al. A GPU-based coupled SPH-DEM method for particle-fluid flow with free surfaces [J]. Powder Technology, 2018, 338: 548–562. DOI: 10.1016/j.powtec.2018.07.043. [123] ZAITSEV Y B, WITTMANN F H. Simulation of crack propagation and failure of concrete [J]. Matériaux et Construction, 1981, 14: 357–365. DOI: 10.1007/BF02478729. [124] GIBSON L J, ASHBY M F. Cellular solids: structure and properties [M]. Cambridge: Cambridge University Press, 1997. [125] SANTOSA S, WIERZBICKI T. On the modeling of crush behavior of a closed-cell aluminum foam structure [J]. Journal of the Mechanics and Physics of Solids, 1998, 46(4): 645–669. DOI: 10.1016/S0022-5096(97)00082-3. [126] LI K, GAO X L, ROY A K. Micromechanics model for three-dimensional open-cell foams using a tetrakaidecahedral unit cell and Castigliano’s second theorem [J]. Composites Science and Technology, 2003, 63(12): 1769–1781. DOI: 10.1016/S0266-3538(03)00117-9. [127] CHEON S S, MEGUID S A. Crush behavior of metallic foams for passenger car design [J]. International Journal of Automotive Technology, 2004, 5(1): 17–22. DOI: 10.1109/TVT.2004.823505. [128] TUNVIR K, KIM A, CHEON S. Analytical solution for crushing behavior of closed cell al-alloy foam [J]. Mechanics of Advanced Materials and Structures, 2007, 14: 321–327. DOI: 10.1080/15376490600845660. [129] 冯阳, 梁增友, 吴鸿超, 等. 基于渗流法的泡沫铝细观结构模型研究 [J]. 中北大学学报(自然科学版), 2016, 37(1): 90–96. DOI: 10.3969/j.issn.1673-3193.2016.01.017.FENG Y, LIANG Z Y, WU H C, et al. Research on micro structural model of open-cell aluminum foam based on infiltration casting methods [J]. Journal of North University of China (Natural Science Edition), 2016, 37(1): 90–96. DOI: 10.3969/j.issn.1673-3193.2016.01.017. [130] LI L, XUE P, CHEN Y, et al. Insight into cell size effects on quasi-static and dynamic compressive properties of 3D foams [J]. Materials Science & Engineering A, 2015, 636: 60–69. DOI: 10.1016/j.msea.2015.03.052. [131] TEKOĞLU C, GIBSON L, PARDOEN T, et al. Size effects in foams: experiments and modeling [J]. Progress in Materials Science, 2011, 56(2): 109–138. DOI: 10.1016/j.pmatsci.2010.06.001. [132] 王长峰, 郑志军, 虞吉林. 泡沫杆撞击刚性壁的动态压溃模型 [J]. 爆炸与冲击, 2013, 33(6): 587–593. DOI: 10.11883/1001-1455(2013)06-0587-07.WANG C F, ZHENG Z J, YU J L. Dynamic crushing models for a foam rod striking a rigid wall [J]. Explosion and Shock Waves, 2013, 33(6): 587–593. DOI: 10.11883/1001-1455(2013)06-0587-07. [133] LI Z Q, ZHANG J J, FAN J H, et al. On crushing response of the three-dimensional closed-cell foam based on Voronoi model [J]. Mechanics of Materials, 2014, 68: 85–94. DOI: 10.1016/j.mechmat.2013.08.009. [134] TANG L Q, SHI X P, ZHANG L, et al. Effects of statistics of cell’s size and shape irregularity on mechanical properties of 2D and 3D Voronoi foams [J]. Acta Mechanica, 2014, 225: 1361–1372. DOI: 10.1007/s00707-013-1054-4. [135] ZHANG X T, WANG R Q, LIU J X, et al. A numerical method for the ballistic performance prediction of the sandwiched open cell aluminum foam under hypervelocity impact [J]. Aerospace Science and Technology, 2018, 75: 254–260. DOI: 10.1016/j.ast.2017.12.034. [136] WEJRZANOWSKI T, SKIBINSKI J, SZUMBARSKI J, et al. Structure of foams modeled by Laguerre–Voronoi tessellations [J]. Computational Materials Science, 2013, 67: 216–221. DOI: 10.1016/j.commatsci.2012.08.046. [137] FAN Z G, WU Y G, ZHAO X H, et al. Simulation of polycrystalline structure with Voronoi diagram in Laguerre geometry based on random closed packing of spheres [J]. Computational materials science, 2004, 29(3): 301–308. DOI: 10.1016/j.commatsci.2003.10.006. [138] REDENBACH C. Microstructure models for cellular materials [J]. Computational Materials Science, 2009, 44(4): 1397–1407. DOI: 10.1016/j.commatsci.2008.09.018. [139] FANG Q, ZHANG J H, ZHANG Y D, et al. A 3D mesoscopic model for the closed-cell metallic foams subjected to static and dynamic loadings [J]. International Journal of Impact Engineering, 2015, 82: 103–112. DOI: 10.1016/j.ijimpeng.2014.10.009. [140] FANG Q, ZHANG J H, ZHANG Y D, et al. Mesoscopic investigation of closed-cell aluminum foams on energy absorption capability under impact [J]. Composite Structures, 2015, 124: 409–420. DOI: 10.1016/j.compstruct.2015.01.001. [141] ZHENG Z J, WANG C F, YU J L, et al. Dynamic stress-strain states for metal foams using a 3D cellular model [J]. Journal of the Mechanics and Physics of Solids, 2014, 72: 93–114. DOI: 10.1016/j.jmps.2014.07.013. [142] ZHANG C Y, TANG L Q, YANG B, et al. Meso-mechanical study of collapse and fracture behaviors of closed-cell metallic foams [J]. Computational Materials Science, 2013, 79: 45–51. DOI: 10.1016/j.commatsci.2013.05.046. [143] GREENBERGER M. An a priori determination of serial correlation in computer generated random numbers [J]. Mathematics of Computation, 1961, 15(76): 383–389. DOI: 10.1090/S0025-5718-1961-0144489-8. [144] FANG Q, ZHANG J H, CHEN L, et al. An algorithm for the grain-level modelling of a dry sand particulate system [J]. Modelling and Simulation in Materials Science and Engineering, 2014, 22(5): 055021. DOI: 10.1088/0965-0393/22/5/055021. [145] MAIRE E, FAZEKAS A, SALVO L, et al. X-ray tomography applied to the characterization of cellular materials. Related finite element modeling problems [J]. Composites Science and Technology, 2003, 63(16): 2431–2443. DOI: 10.1016/S0266-3538(03)00276-8. [146] MCDONALD S A, MUMMERY P M, JOHNSON G, et al. Characterization of the three-dimensional structure of a metallic foam during compressive deformation [J]. Journal of Microscopy, 2006, 223(2): 150–158. DOI: 10.1111/j.1365-2818.2006.01607.x. [147] JEON I, ASAHINA T, KANG K-J, et al. Finite element simulation of the plastic collapse of closed-cell aluminum foams with X-ray computed tomography [J]. Mechanics of Materials, 2010, 42(3): 227–236. DOI: 10.1016/j.mechmat.2010.01.003. [148] SAENGER E H, URIBE D, JÄNICKE R, et al. Digital material laboratory: wave propagation effects in open-cell aluminium foams [J]. International Journal of Engineering Science, 2012, 58: 115–123. DOI: 10.1016/j.ijengsci.2012.03.030. [149] 陈鹏. 泡沫铝夹芯结构力学性能研究[D]. 哈尔滨: 哈尔滨工业大学, 2013.CHEN P. Research on mechanical properties of aluminum foam sandwich structure [D]. Harbin: Harbin Institute of Technology, 2013. [150] SUN Y L, LI Q M, LOWE T, et al. Investigation of strain-rate effect on the compressive behaviour of closed-cell aluminium foam by 3D image-based modelling [J]. Materials and Design, 2016, 89: 215–224. DOI: 10.1016/j.matdes.2015.09.109. [151] 程振, 方秦, 张锦华, 等. 闭孔泡沫金属三维细观模型建模方法 [J]. 工程力学, 2017, 34(8): 212–221. DOI: 10.6052/j.issn.1000-4750.2016.02.0098.CHENG Z, FANG Q, ZHANG J H, et al. Mesoscopic methodology for the three-dimensional modelling of closed-cell metallic foam [J]. Engineering Mechanics, 2017, 34(8): 212–221. DOI: 10.6052/j.issn.1000-4750.2016.02.0098. [152] 李侯贞强, 张亚栋, 张锦华, 等. 基于CT的泡沫铝三维细观模型重建及应用 [J]. 北京航空航天大学学报, 2018, 44(1): 160–168. DOI: 10.13700/j.bh.1001-5965.2016.0959.LI H Z Q, ZHANG Y D, ZHANG J H, et al. Reconstruction and application of three-dimensional mesoscopic model of aluminum foam based on CT [J]. Journal of Beijing University of Aeronautics and Astronautics, 2018, 44(1): 160–168. DOI: 10.13700/j.bh.1001-5965.2016.0959. 期刊类型引用(1)
1. 刘蕊, 于渤, 邓龙胜, 范文, 汤冬, 杜中东, 宋方述, 邹煜. 爆炸作用下黄土介质损伤规律试验. 地球科学与环境学报. 2025(04)  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:









































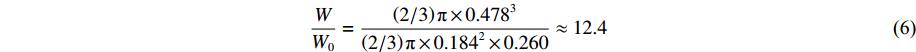















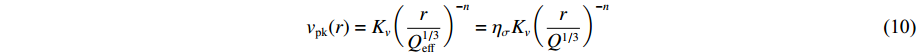




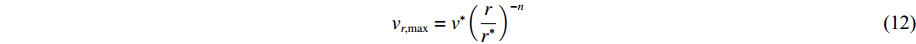

























 下载:
下载:





















 百度学术
百度学术