Shock wave characteristics in a conical water explosion shock tube under cylindrical charge condition
-
摘要: 锥形水中爆炸激波管是进行水中爆炸实验的一种装置,该装置能够通过较小装药量在相同距离处实现自由场水中较大装药量爆炸的冲击波峰值。为了获得柱形装药条件下锥形水中爆炸激波管内的冲击波特性,本文通过数值计算的方式,对不同圆锥角和不同柱形装药质量下锥形激波管内的冲击波传播过程进行了模拟,通过对不同工况下激波管内冲击波特性进行分析,发现其初始冲击波的衰减规律符合自由场水中的指数衰减形式,并拟合得到了与自由场水中爆炸相容的冲击波峰值、比冲量和能流密度经验公式;发现其二次脉动压力周期与炸药质量呈反常规的变化规律,并引入等效静水压深度解释了这一现象;发现其二次脉动压力幅值与初始冲击波幅值之比比自由场水中更大,而二次脉动压力的比冲量与初始冲击波冲量之比与自由场水中相当。Abstract: A conical shock tube is a kind of underwater explosive devices which uses small conical explosive charge to form high intensity shock pressure. Theoretically, the shock wave pressure in the conical shock tube is the same as that generated by a virtual spherical explosive charge in free field water. However, considering the effect of practical factors, the characteristics of shock wave in the actual device and the theoretical device are different to some extent. In order to investigate the shock wave characteristics in the conical water explosion shock tube under a cylindrical charge condition, and to obtain the variation rules of the peak pressure value, the specific impulse and the energy flux density, a series of numerical calculations with different cone angles and different quality of cylindrical charges were conducted. The reliability of the simulation methods was verified by comparing with the published experimental data. Through the analysis of the pressure data obtained by the validated simulation method, it is found that the shock wave in the tube follows the same scaling law as it is in the free field underwater explosion. The constants k and n of the empirical expressions for peak pressure, the impulse and the energy flux density for the shock wave in shock tube are obtained by data fitting. Furthermore, the relationships among the coefficient k, index n and cone angle α were deduced, and the result shows that the coefficients k have well linear relationship with constructed angle coefficient β, and the indexes n can be quantitatively expressed by cone angle α. Regarding the free field as a special case with a cone angle of 360°, it’s constants k and n also conform to the obtained relationships. It is also found that the secondary pulsation pressure period shows an anomalous change rule with explosive mass, which can be well explained by the significant increasement of the equivalent hydrostatic pressure depth. The ratio between the secondary impulse pressure peak and initial pressure peak is bigger than that in free field while the ratio between the secondary impulse pressure’s impulse to the initial pressure impulse is almost the same. These results can provide support for the application of conical shock tubes.
-
Key words:
- underwater explosion /
- conical shock tube /
- shock wave /
- secondary impulse
-
水中爆炸实验是进行舰船材料和结构抗水中爆炸特性研究的重要手段。经过几十年的发展,各式各样的水中爆炸实验方式不断涌现,根据水中爆炸实验所处环境的不同,分为开放水域实验和密闭水域实验。开放水域实验包括在水箱[1-3]、爆炸水池[4-5]和自然水域中的水中爆炸实验等,这类实验较为简单直接,但对实验场所条件的有较高要求,而且每次实验涉及到的各方面协调准备工作较为繁杂[6]。密闭水域实验包括采用封闭增压水池和激波管进行的水中爆炸实验等,其中密闭增压水池主要为了满足水中爆炸气泡的缩比准则,适用于个别特殊的情况[7];激波管则为各种新型结构材料的抗爆特性研究[8-10]提供了较为方便的实验途径。激波管根据是否采用炸药进行加载,又可分为装药和非装药两种类型。非装药激波管水中爆炸实验通过飞片撞击的方式在激波管水体中产生类似于水中爆炸形成的冲击波[11-12],该方式的优点是不需要使用炸药,能够在大多数实验室内使用,但由于没有爆轰产物气泡的形成,使其无法考虑脉动载荷的作用。装药激波管主要是指锥形水中爆炸激波管,它采用炸药在充满水的锥形激波管尖端起爆,对放置另一端的试件进行加载,能够采用较小药量实现较大冲击波幅值的加载,且能够实现二次脉动载荷的加载,也能够在大多数实验室内使用。本文将针对采用炸药加载的锥形水中爆炸激波管进行讨论。
锥形水中爆炸激波管装置的原理最早由Filler[13]提出,如图1所示,圆锥角为α的锥形激波管内装填有球锥形炸药,如果管壁材料为刚性,则该锥形炸药在管内产生的冲击波压力与具有相同半径的球形装药在自由场水中相同距离处产生的冲击波压力相同。Zalesak 等[14]在该原理基础上,设计了图2所示的锥形激波管,列装于美国海军研究实验室开展相关试验。
根据上述原理,可以采用较小质量m的锥形装药实现较大质量W的球形装药下的冲击波幅值加载。根据几何关系,锥形装药质量m与虚拟球形装药质量W的关系为[13]
W=ηm=1sin2(α/α44)m (1) 式中:α为激波管的圆锥角,η为理论质量放大系数:
η=1sin2(α/α44) (2) 但在实际装置中,会存在各种与理论预设条件不一致的情况,例如实际装药并非理想的球锥,管壁材料并非刚性等,这些实际因素的存在会使得激波管中的冲击波与理论存在差别。例如,Heshmati等[15]设计锥形激波管的装药端并未完全收缩到尖端,并采用球形装药,如图3(b)所示。Heshmati等通过数值计算得到该装药类型下的质量放大系数约为理论质量放大系数的1/5。
考虑加工工艺和装填炸药的可操作性等方面因素,柱形装药是一种更为可取的方式,如图3(c)所示,但与此种装药方式对应的激波管中的水中爆炸冲击波载荷特性必然也会与理论情况存在一定的差别。因此,本文采用数值计算的方式,对采用柱形装药的锥形水中爆炸激波管的冲击波传播过程进行模拟,通过对不同圆锥角和不同装药质量下的压力进行分析,以获得柱形装药锥形水中爆炸击波管中冲击波峰值、比冲量、能流密度的表达式以及二次脉动压力幅值和周期的变化规律,为锥形激波管的实际应用提供支撑。
1. 锥形激波管的计算模型和验证
1.1 计算模型的基本参数
为了获得不同圆锥角和不同炸药质量下激波管内的压力,采用AUTODYN软件对锥形激波管内的冲击波传播过程进行模拟。激波管的结构示意如图4所示。激波管的长度L=200 cm,管体厚度为10 cm,圆锥角为α,考虑α=4, 6, 8, 10°等4种情况;柱形炸药的半径为r=0.5 cm,柱形炸药的长度为l,考虑l=1, 2, 3 cm等3种情况,选用传统TNT作为炸药,密度为1.63 g/cm3,因此3种炸药长度l对应的炸药质量m分别为1.28、2.56和3.84 g。
进行数值计算时,对材料模型和状态方程的选取很大程度上决定了计算结果的有效性。在本文的计算中,TNT采用JWL状态方程的形式:
p=A(1−ω/ωR1VR1V)e−R1V+B(1−ω/ωR2VR2V)e−R2V+ωe0/ωe0VV (3) 式中:p为爆轰压力;V为爆轰产物体积和炸药初始体积之比;e0为炸药的单位体积初始内能;A、B、R1、R2、ω为特征参数,各参数取值如表1所示。
表 1 TNT的JWL状态方程参数Table 1. The JWL EOS parameters for TNT参数 A/GPa B/Pa R1 R1 ω 取值 373.8 374.7 4.15 0.9 0.35 水的状态方程采用线性多项式方程进行描述,其形式由根据压缩状态的不同而定。
当水压缩时(μ>0时),其状态方程为:
p=A1μ+A2μ2+A3μ3+(B0+B1μ)ρ0u (4) 当水膨胀时(μ<0时),其状态方程为:
p=T1μ+T2μ2+B0ρ0u (5) 式中:p为水中的压力;μ为压缩比,μ=ρ/ρ0−1,ρ和ρ0为初始密度;u为水的比内能;A1、A2、A3、B0、B1、T1和T2是由AUTODYN 材料库赋值的常数。各参数取值如表2所示。
表 2 水的状态方程参数Table 2. The EOS parameters for waterρ0/(kg·m−3) A1/GPa A2/GPa A3/GPa B0 B1 T1/GPa T2/Pa 1×103 2.2 9.54 14.57 0.28 0.28 2.2 0 激波管的管壁的材料为4340钢,其材料模型采用Johnson-Cook的形式
σ=[As+Bsεnsp][1+Cln(˙ε∗)][1−T∗ms] (6) 式中:εp为有效塑性应变,
˙ε∗ = ˙εp/˙εp˙ε0˙ε0 为无量纲的塑性应变率,˙ε0 =1 s−1;T*=(T−Tr)/(Tm−Tr)∈ [0,1] ,为无量纲温度,T、Tr和Tm分别为温度、室温和材料的熔化温度;As、Bs、ns、C和ms为材料常数。4340钢的材料基本参数见表3。表 3 4340钢的基本参数Table 3. Basic parameters for 4340 steel参数 ρ/(kg·m−3) E/Pa ν As/Pa Bs/Pa ns C ms 取值 7.83×103 2.0×1011 0.29 7.92×108 5.10×108 0.26 0.014 1.03 注:ν为泊松比,E为弹性模量. 由于所计算的问题具有较好的对称性,因此在采用AUTODYN进行计算时,可以采用二维轴对称模型进行简化计算,划分均匀网格的大小为0.25 cm,其中激波管采用拉格朗日网格,水和炸药采用欧拉网格,在炸药的远离水的端面设置起爆点。
1.2 计算模型的可靠性验证
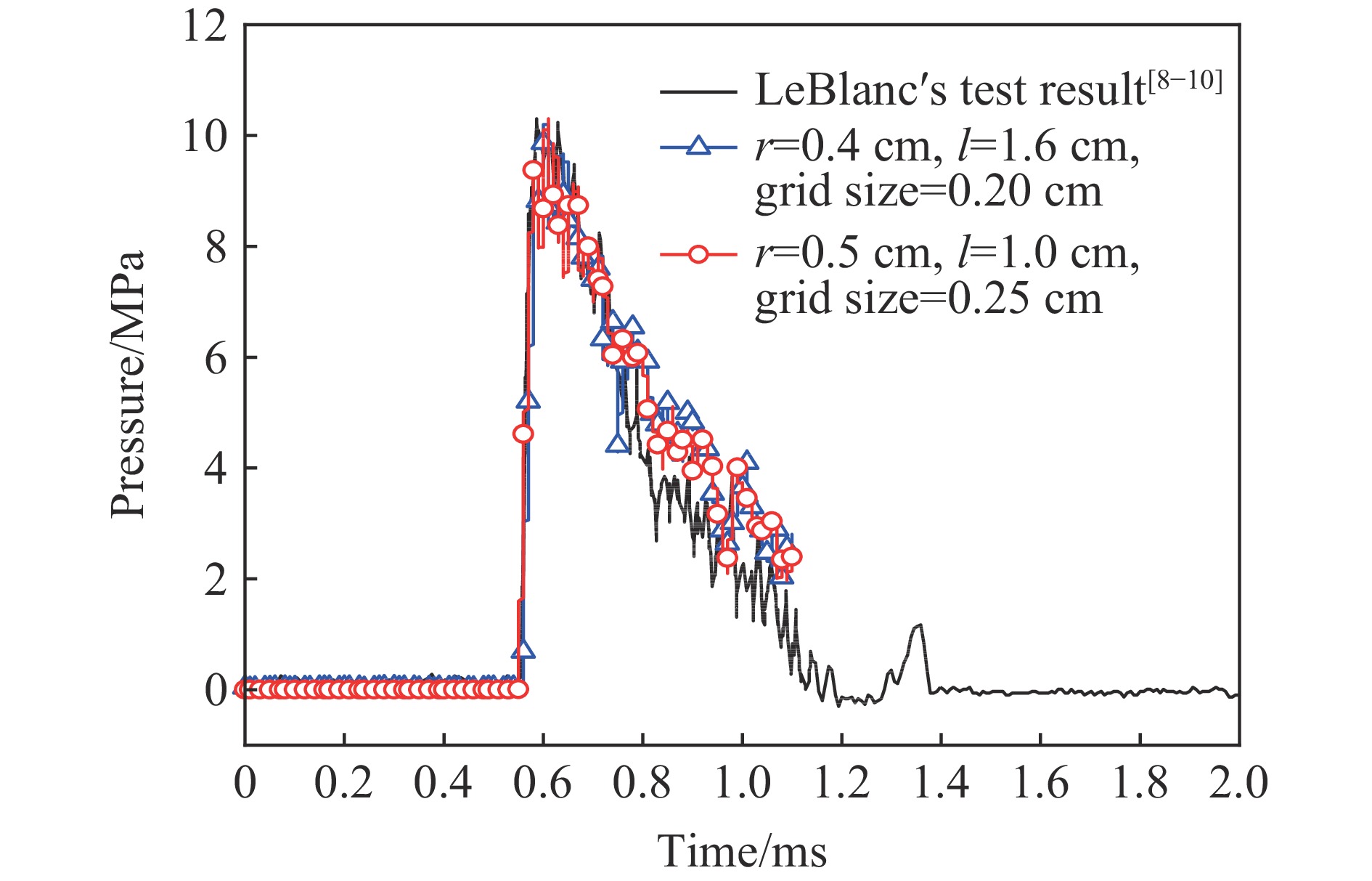
为了验证上述计算方法的可靠性,以LeBlanc等[8-10]的实验数据为基础,对数值模拟的结果进行了对比分析。根据文献[8-10]中的实验数据,其采用的激波管锥角α=2.6°,激波管的长度L=525 cm,爆炸源为6号雷管,等效TNT当量为1.32 g,在距离管端50.8 cm(距离起爆点474.2 cm)处测量得到的冲击波幅值约为10.3 MPa,其压力时程信号如图5中所示。
根据上述信息按第1.1节中的方法进行建模计算时,还需确定装药尺寸。文献[8-10]中未给出雷管的尺寸信息,但可以确定雷管为圆柱形,为了能进行有效比较,计算时采用了两种装药尺寸。工况1:柱形装药的半径为r=0.4 cm,柱形炸药的长度为l=1.6 cm,实际装药质量为m=1.31 g,划分均匀网格的大小为0.20 cm;工况2:柱形装药的半径为r=0.5 cm,柱形炸药的长度为l=1.0 cm,实际装药质量为m=1.28 g,划分均匀网格的大小为0.25 cm。两种工况的其他计算模型参数一致:管体长度L=525 cm,管体厚度为10 cm,圆锥角α=2.6度,在474.2 cm处设置压力监测点。
模拟计算时,监测点从起爆的时刻开始记录。如果按水中声速进行估算,冲击波波头从起爆点传到474.2 cm处所需的时间大约为3 ms。根据文献[8-10]给出的冲击波时程曲线,其冲击波达到时间约为0.55 ms,如果其传感器记录时间也是从起爆时开始,则显然是不合理的,因此可以判定其传感器触发时间是在起爆之后。故为了方便进行对比,将计算得到的压力时程曲线向左平移,使其峰值到达时间一致。模型计算得到的压力时程曲线如图5所示。
根据图5所示的计算结果,工况1和工况2中,距离起爆点474.2 cm处的冲击波幅值分别为10.2和10.3 MPa,该冲击波幅值的计算结果与文献[8-10]的结果几乎一致,且冲击波衰减趋势也基本一致。因此,可以认为本文所采用的计算方法是有效的,所得结果也是可靠的。
2. 锥形激波管模拟结果的分析和讨论
2.1 锥形激波管中的冲击波传播过程
采用1.1节的计算模型和参数对锥形激波管水中爆炸过程的12个工况进行了模拟,得到了冲击波在管体内部的传播变化过程。图6所示为α=4°的激波管,在炸药质量m=1.28 g条件下不同时刻的管内冲击波传播情况,为了更为清晰地观察冲击波的传播情况,仅对管内流体中的压力云图进行了输出。从图6可知,炸药起爆后,向管内的水中传入冲击波,冲击波波阵面的宽度随着管内径的变大而逐渐变大,管内压力的最大值逐渐降低,t=400 μs时最大压力为96.52 MPa,t=900 μs时最大压力为41.39 MPa。
爆轰产物的膨胀速度要远慢于冲击波的传播速度,图7所示为爆轰产物与水的界面在不同时刻的发展情况。在膨胀阶段(0 μs≤t<2500 μs),爆轰产物与水的界面保持为圆弧面;在收缩初始阶段(2500 μs≤t<3600 μs),靠近管中轴的爆轰产物先收缩,靠近管壁的爆轰产物仍沿管壁缓慢扩展;在收缩中间阶段(3600 μs≤t<5100 μs),靠近管壁的爆轰产物收缩进度逐渐超过管中轴上的收缩进度;在收缩最后阶段(5100 μs≤t<6900μs),爆轰产物与水的界面恢复为圆弧面;在t≈6900 μs时,爆轰产物收缩到最小,此后,爆轰产物再次膨胀,形成二次脉动压力。
对锥形激波管中的压力进行监测,可以得到不同位置处的压力历史曲线,图8所示为质量m=1.28 g的炸药在不同圆锥角下的典型压力历史曲线。从曲线中可以明显看到初始冲击波峰值、压力衰减和二次脉动压力的存在。初始冲击波、二次脉动压力是对水中爆炸压力进行衡量的主要参数,接下来从这两个方面对锥形激波管内的水中爆炸压力进行讨论。
2.2 锥形激波管中的初始冲击波
2.2.1 初始冲击波的峰值
对于水中爆炸冲击波,其初始压力峰值随着距离的增大而呈指数衰减,满足[16]:
pm=kp(m1/133R)np (7) 式中:pm为压力峰值,MPa;R为到爆炸中心的距离,m;R0为药包等效半径,m,R0=0.053m1/3;m为炸药的质量,kg;kp为峰值压力系数,np为指数,对于自由场水中的爆炸,当12<R/R0<240时,kp=52.16,np=1.13。
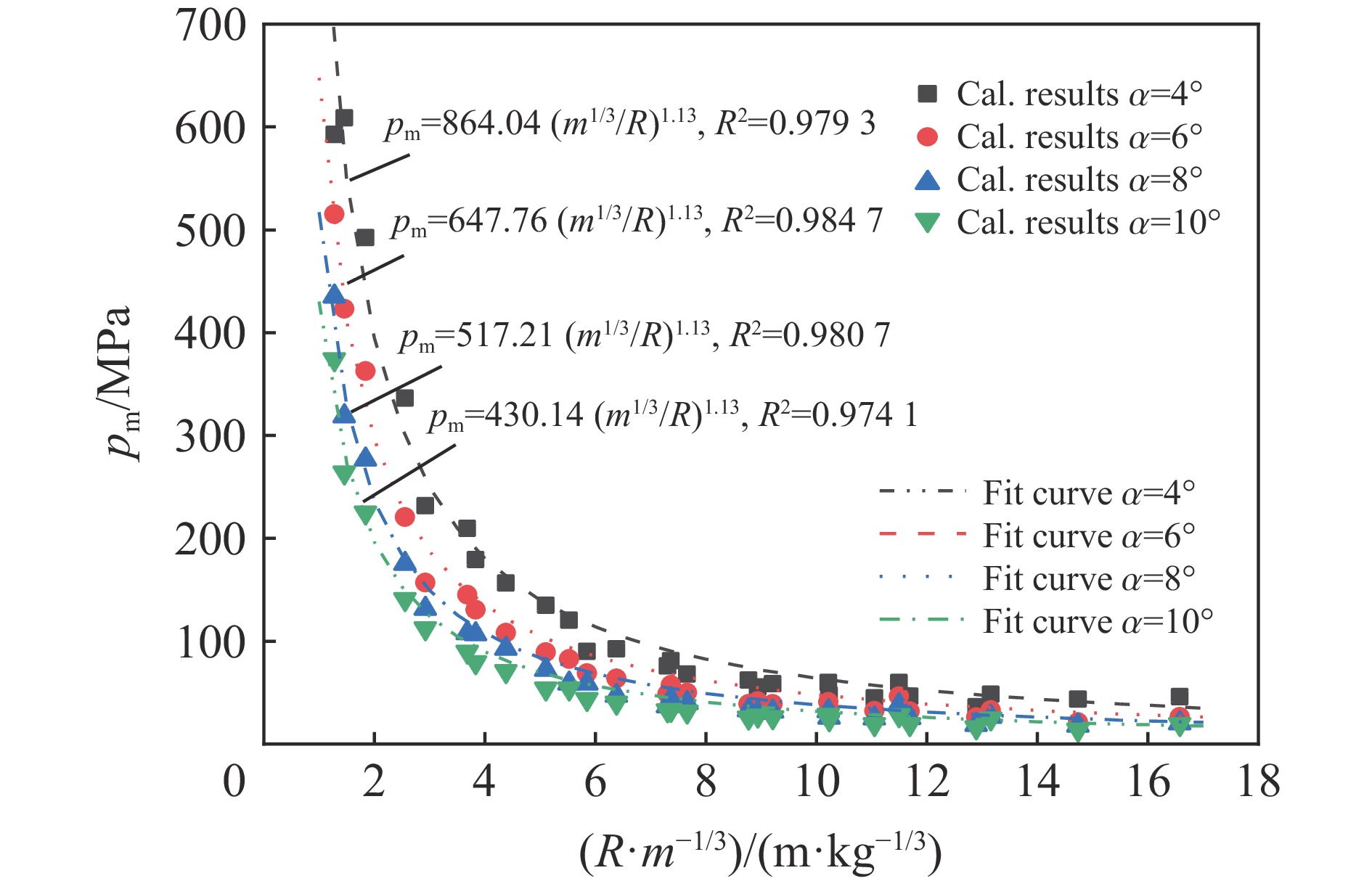
在锥形激波管的尖端采用柱形装药会对靠近炸药有限区域的相似性产生影响,但随着距离的增加,这种影响可以忽略不计。当R/R0>12时,可以忽略这种影响。对不同质量装药条件下激波管中不同位置(R/R0>12)处的初始冲击波峰值进行统计,可以得到冲击波幅值在锥形激波管内随比例距离衰减的变化情况,如图9所示。采用式(7)对数据点进行拟合,拟合时保持指数np不变,针对不同峰值压力系数kp进行拟合,得到图9中的各条拟合曲线。
从拟合结果可以观察到,峰值压力系数kp随着圆锥角α的增大而减小,α=4°时,kp=864.04;α=10°时,kp=430.14,均远大于自由场水中爆炸对应的峰值压力系数(kp =52.16)。由此可以明显看出,锥形激波管的确能通过较小质量的炸药实现较大冲击波幅值的水中爆炸载荷。
将自由场水中爆炸的情形视为时的kp=52.16视为α=360°对应的系数,进一步探讨kp与α之间的关系。引入角度系数βp:
βp=[1sin2(α/α44)]np/np33 (8) 式中: np=1.13。
用角度系数βp表征α,对kp与α之间的关系进行刻画,可以得到角度系数βp与峰值压力系数kp之间的关系,如图10所示。通过对α=4, 6, 8, 10, 360°通所对应的5组kp和βp进行拟合,可以得到两者之间较好的线性关系:
kp=apβp+bp (9) 式中:系数ap=38.35,系数bp=13.81。
结合式(1)、式(7)、式(8)和式(9),可以得到适用于锥形激波管的峰值压力衰减公式:
pm=ap(W1/133R)np+bp(m1/133R)np (10) 式中:等号右边第1项可以理解为虚拟球形装药引起的压力峰值项,第2项为实际装药引起的压力峰值项。
2.2.2 初始冲击波的比冲量和能流密度
初始冲击波的比冲量和能流密度可以近似按下式进行计算[16]:
i=∫10θ0p(t)dt (11) e=1ρ0c0∫5θ0[p(t)]2dt (12) 式中:i为冲击波的比冲量;e为冲击波的能流密度;θ为时间常数,即冲击波幅值从pm衰减到pm/e所需的时间;p(t)为监测点处得到的压力时间曲线;c0为未扰动介质中的声速。
根据水中爆炸的相似准则,冲击波的比冲量和能流密度的经验表达式可以写为[16]:
im1/133=ki(m1/133R)ni (13) em1/133=ke(m1/133R)ne (14) 式中:ki和ke分别为比冲量系数和能流密度系数;ni和ne为指数,当i的单位取Pa·s,e的单位取Pa·m时,对于自由场水中的爆炸,ki=5760,ni=0.89;ke=9.8×104,ne=2.10。
对不同质量装药条件下激波管中不同位置处的时间常数θ进行统计,并分别按式(11)和式(12)对对应的压力时间曲线进行数值积分,可以得到冲击波的比冲量和能流密度在锥形激波管内随比例距离衰减的变化情况,结果如图11和图12中的数据点所示。
采用式(7)、(13)和(14)的形式分别对数据点进行拟合,得到图9、图11和图12中的各条拟合曲线,各拟合曲线的系数和指数汇总于表4。表4中还给出了自由场水中的系数值,也即α=360°所对应的列。
表 4 基于数值模拟结果拟合得到的初始冲击波压力峰值、比冲量和能流密度曲线方程的参数及决定系数Table 4. Parameters and determination coefficient of fitting curve Eq. for simulational results of maximum pressure, specific impulse and energy flow densityα kp np R2 (pm) ki/105 ni R2 (i) ke/105 ne R2 (e) 4° 864.04 1.13 0.9793 2.99 0.464 0.9567 581 1.62 0.9978 6° 647.76 1.13 0.9847 2.22 0.476 0.9183 349 1.69 0.9980 8° 517.21 1.13 0.9807 1.73 0.490 0.9380 242 1.79 0.9988 10° 430.14 1.13 0.9741 1.59 0.583 0.9474 181 1.86 0.9992 360°[16] 52.16 1.13 0.0058 0.891 0.98 2.10 按照2.2.1节的思路,将自由场水中爆炸时的ki和ke视为α=360°场对应的系数,进一步探讨ki和ke与α之间的关系,引入角度系数βi和βe:
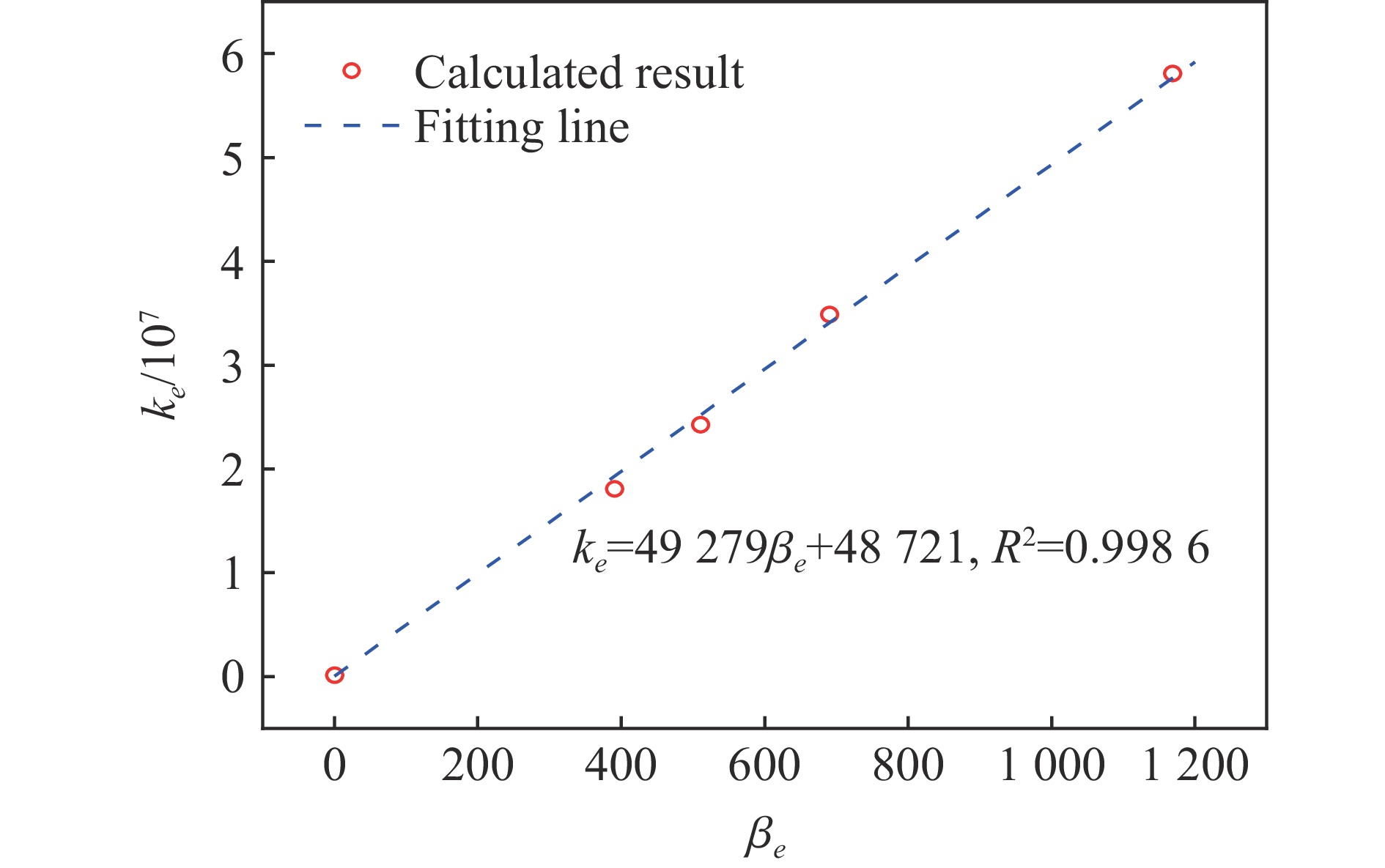
βi=[1sin2(α/α44)](1+ni)/(1+ni)33 (15) βe=[1sin2(α/α44)](1+ne)/(1+ne)33 (16) 图13和图14给出了角度系数βi和βe与ki和ke的关系。通过拟合,发现两者之间也呈现较好的线性关系:
ki=aiβi+bi (17) ke=aeβe+be (18) 式中:ai=5923.5,bi=−163.5;ae=49279,be=48721。
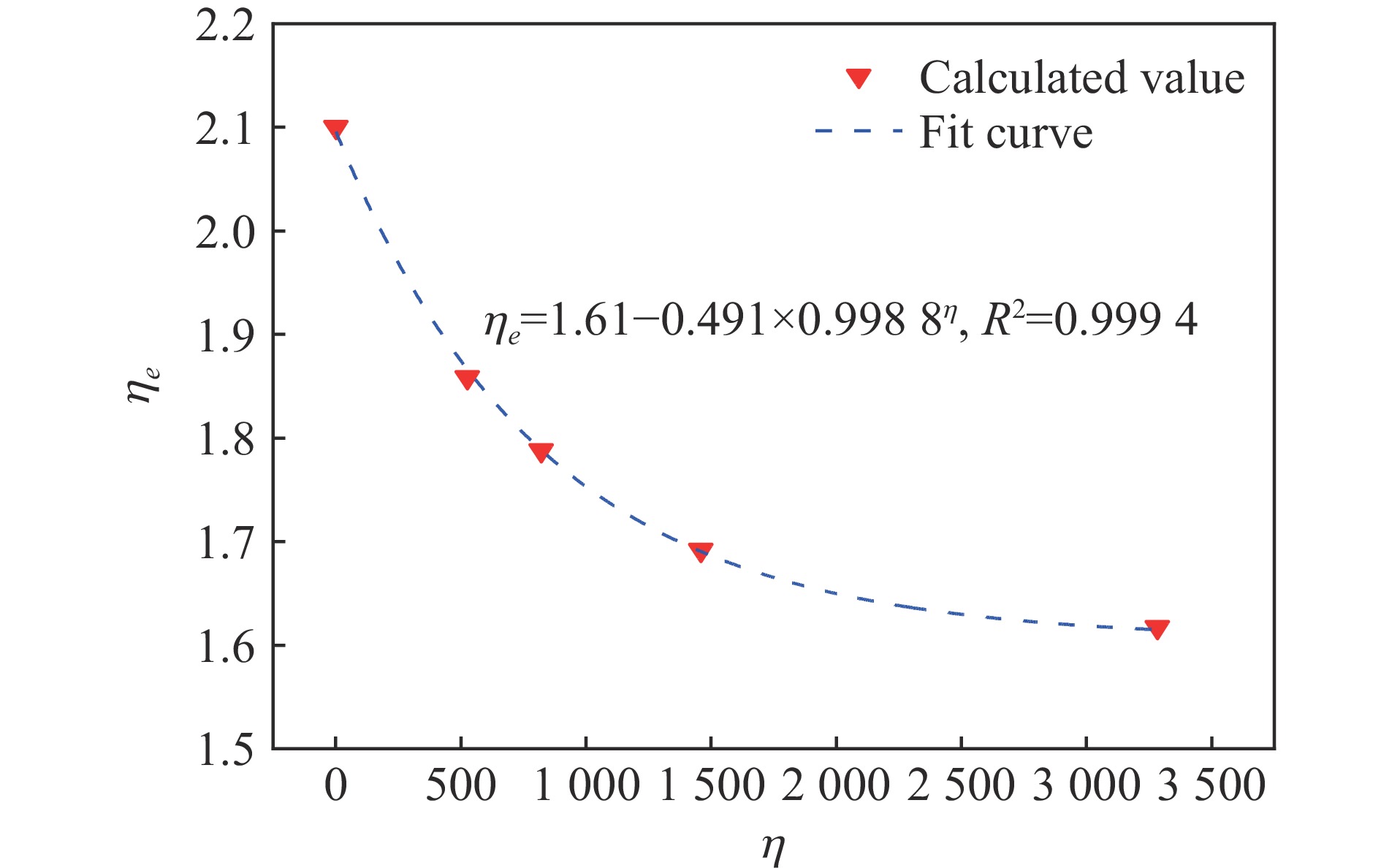
在2.2.1节中对冲击波峰值压力进行拟合时,保持np为常数,只对kp进行拟合就达到了很好的拟合效果,当激波管的α确定后,根据式(9)就能确定适用于该激波管中冲击波峰值经验公式的系数。但在对比冲量和能流密度进行拟合时,发现只有同时对两个系数进行拟合才能达到较好的拟合效果,此时,ni和ne不是常数,由于βi和βe与ni和ne相关,因此还需要找到ni和ne与α的关系,才能通过α确定激波管比冲量和能流密度经验公式的系数。为此,根据表4中数据,用理论质量放大系数η表征α,对ni和ne与α的关系式进行了探索,图15和图16给出了对应的数据及其拟合曲线,曲线方程为
ni=0.46−0.432×0.9974η (19) ne=1.61−0.491×0.9988η (20) 式(19)和式(20)的函数形式仅是为了保证拟合效果而选取,这里不对其物理含义进行分析。
2.3 锥形激波管中的二次脉动压力
二次脉动压力是水中爆炸载荷的重要成分,一般主要从二次脉动压力的周期、压力幅值和比冲量3个方面来进行描述。在自由场水中,二次脉动压力的周期满足[17]:
τ=kTm1/133(d+10.3)5/566 (21) 式中:τ为二次脉动压力的周期,s;kT为炸药特征常数,TNT对应的特征常数约为kT=2.11;d表示炸药所处的深度,m;(d+10.3)用来等效表征爆炸深度上的流体静压力,m。
对锥形激波管中不同工况下的二次脉动压力周期进行统计,得到了图17所示的结果。由图17中曲线的变化趋势可知,在锥形激波管中二次脉动压力周期随着炸药质量的增加而减小,这与式(21)所表示的含义相反。由式(21)可知,在自由场水中,二次脉动压力周期是随炸药质量增大而增大的。为了解释这一反常规现象,可以从等效静水压的角度进行分析。
注意到式(21)的分母,可知二次脉动压力周期随静水压增大而减小。在锥形激波管中,管内流体处于封闭空间内,由于水的可压缩性远小于爆轰产物的可压缩性,因此,在爆轰产物的发展过程中,爆轰产物周围的水处于一个较高的压力状态,这将为爆轰产物提供一个较高的等效静水压。为此,引入等效静水压深度参数D描述炸药所处的深度d(满足D=d)。利用图17中的数据,根据式(21)可以计算得到不同工况下的等效静水压深度,如图18所示。可以看出,炸药质量越大,所对应的等效静水压深度越大,且D与m1/3近似为线性关系,其斜率远远大于1000,虽然在式(18)中(d+10.3)的指数为5/6,但该分母的变化速率仍大于m1/3的变化速率,因此随着炸药质量的增大,锥形激波管中的二次脉动周期反而减小。
一般用二次脉动压力幅值p2m与初始冲击波幅值pm的比值来对二次脉动压力幅值进行衡量。对所有工况的二次脉动压力幅值进行了统计,并计算得到了冲击波幅值之比的结果,如表5所示。由表5可知,在锥形激波管中,二次脉动压力的幅值为初始冲击波幅值的1/7~1/3;而在自由场水中,二次脉动压力幅值约为初始冲击波幅值的1/15~1/10[18]。由此可见,激波管中的二次脉动压力幅值相比自由场水中更大。根据文献[17]的论述可知,二次脉动压力幅值的大小与爆轰产物气体的收缩到最小时的径向速度有关:
表 5 二次脉动压力幅值与初始冲击波幅值之比Table 5. The secondary impulse pressure peak to the initial shock pressure peak ratio(R·m−1/3)/(m·kg−1/3) p2m/pm α=4° α=6° α=8° α=10° 1.84 0.14 0.18 0.22 0.19 1.46 0.21 0.24 0.29 0.24 1.28 0.26 0.33 0.21 0.19 平均 0.20 0.25 0.24 0.21 p2m=p0+ρ0Rddt(a2dadt)a=amin (22) 式中:p2m为二次脉动压力的幅值,p0为环境流体静压力,R为到气泡中心的距离,a为气泡半径,amin为气泡最小半径。对于激波管内的二次脉动压力而言,尽管式(22)不一定适用,但仍可以从定性上参考来进行分析。激波管内二次脉动压力的显著提高有2方面原因:(1)等效静水压深度的存在,导致环境流体压力的提高;(2)环境流体压力的提高导致气泡收缩时径向速度和径向速度变化率的显著提高。定量上的分析,还有待深入讨论。
二次脉动压力的比冲量i2与初始冲击波的比冲量i1的比值也是衡量二次脉动压力的重要参数。对所有工况的压力历史曲线按时间进行积分,其中,对初始冲击波的比冲量i1按式(11)进行计算时,积分的时间上限取为5θ;对二次脉动的比冲量i2进行计算时,积分的时间间隔为周期的22%[17],即:
i2=∫t2+0.11Tt2−0.11Tp(t)dt (23) 式中:t2为压力历史曲线中二次脉动压力峰值所在的时刻。得到各工况中的I2和I1后,计算两者的比值,见表6。由表6可知,在锥形激波管中,二次脉动压力的冲量约为初始冲击波冲量的4倍。在自由场水中,二次脉动压力的冲量也约为初始冲击波冲量(时间间隔取5θ)的4倍[17]。
表 6 二次脉动压力的正冲量与初始冲击波冲量之比Table 6. The secondary impulse pressure’s impulse to the initial shock pressure impulse ratio(R·m−1/3)/(m·kg−1/3) i2/i1 α=4° α=6° α=8° α=10° 1.84 3.57 3.75 3.45 3.29 1.46 3.80 3.74 4.05 3.38 1.28 3.57 4.03 4.00 3.69 平均 3.65 3.84 3.83 3.45 2.4 结果汇总
根据前文结果汇总,得到锥形激波管中初始冲击波的峰值压力、比冲量和能流密度的经验表达式,以及其中的参数,见表7。
表 7 冲击波峰值、比冲量和能流密度经验公式及其系数Table 7. Constants of empirical expressions for peak pressure, the impulse and the energy flux density目标物理量 表达式 系数表达式 指数表达式 角度系数 pm (MPa) pm=kp(m1/3/R)np kp =38.35βp+13.81 np=1.13 βp=ηnp/3 I (Pa·s) I/m1/3=ki(m1/3/R)ni ki =5923.5βi−163.5 ni=0.46−0.432×0.9974η βi=η(1+ni)/3 E (Pa·m) E/m1/3=ke(m1/3/R)ne ke =49279βe+48721 ne=1.61−0.491×0.9988η βe=η(1+ne)/3 3. 结 论
通过对不同锥角和不同装药量情况下锥形水中爆炸激波管的爆炸冲击波传播过程进行模拟,及对管内冲击波的定量分析,得到了柱形装药条件下,关于锥形水中爆炸激波管内冲击波特性的以下结论:
(1)锥形激波管中初始冲击波的峰值压力pm、比冲量I和能流密度E均符合水中爆炸的相似规律;冲击波峰值、比冲量(用i/m1/3表征)和能流密度(用e/m1/3表征)均可用x=k(m1/3/R)n的关系来表达(x分别对应pm, i/m1/3或e/m1/3);系数k与构建的角度系数β呈现较好的线性关系,冲击波峰值压力指数np为常数,比冲量和能流密度的指数ni和ne与激波管的放大系数η相关;
(2)锥形激波管中二次脉动压力周期随着炸药质量的增大而减小,与自由场水中的变化规律相反,这一反常规的现象主要是由于炸药质量增大引起的管内等效静水压深度增大导致的;
(3)锥形激波管中二次脉动压力的幅值为初始冲击波的1/7~1/3,这一比例比自由场水中的更大;锥形激波管中二次脉动压力的比冲量约为初始冲击波的4倍,这一比值与自由场水中基本一致。
-
表 1 TNT的JWL状态方程参数
Table 1. The JWL EOS parameters for TNT
参数 A/GPa B/Pa R1 R1 ω 取值 373.8 374.7 4.15 0.9 0.35 表 2 水的状态方程参数
Table 2. The EOS parameters for water
ρ0/(kg·m−3) A1/GPa A2/GPa A3/GPa B0 B1 T1/GPa T2/Pa 1×103 2.2 9.54 14.57 0.28 0.28 2.2 0 表 3 4340钢的基本参数
Table 3. Basic parameters for 4340 steel
参数 ρ/(kg·m−3) E/Pa ν As/Pa Bs/Pa ns C ms 取值 7.83×103 2.0×1011 0.29 7.92×108 5.10×108 0.26 0.014 1.03 注:ν为泊松比,E为弹性模量. 表 4 基于数值模拟结果拟合得到的初始冲击波压力峰值、比冲量和能流密度曲线方程的参数及决定系数
Table 4. Parameters and determination coefficient of fitting curve Eq. for simulational results of maximum pressure, specific impulse and energy flow density
α kp np R2 (pm) ki/105 ni R2 (i) ke/105 ne R2 (e) 4° 864.04 1.13 0.9793 2.99 0.464 0.9567 581 1.62 0.9978 6° 647.76 1.13 0.9847 2.22 0.476 0.9183 349 1.69 0.9980 8° 517.21 1.13 0.9807 1.73 0.490 0.9380 242 1.79 0.9988 10° 430.14 1.13 0.9741 1.59 0.583 0.9474 181 1.86 0.9992 360°[16] 52.16 1.13 0.0058 0.891 0.98 2.10 表 5 二次脉动压力幅值与初始冲击波幅值之比
Table 5. The secondary impulse pressure peak to the initial shock pressure peak ratio
(R·m−1/3)/(m·kg−1/3) p2m/pm α=4° α=6° α=8° α=10° 1.84 0.14 0.18 0.22 0.19 1.46 0.21 0.24 0.29 0.24 1.28 0.26 0.33 0.21 0.19 平均 0.20 0.25 0.24 0.21 表 6 二次脉动压力的正冲量与初始冲击波冲量之比
Table 6. The secondary impulse pressure’s impulse to the initial shock pressure impulse ratio
(R·m−1/3)/(m·kg−1/3) i2/i1 α=4° α=6° α=8° α=10° 1.84 3.57 3.75 3.45 3.29 1.46 3.80 3.74 4.05 3.38 1.28 3.57 4.03 4.00 3.69 平均 3.65 3.84 3.83 3.45 表 7 冲击波峰值、比冲量和能流密度经验公式及其系数
Table 7. Constants of empirical expressions for peak pressure, the impulse and the energy flux density
目标物理量 表达式 系数表达式 指数表达式 角度系数 pm (MPa) pm=kp(m1/3/R)np kp =38.35βp+13.81 np=1.13 βp=ηnp/3 I (Pa·s) I/m1/3=ki(m1/3/R)ni ki =5923.5βi−163.5 ni=0.46−0.432×0.9974η βi=η(1+ni)/3 E (Pa·m) E/m1/3=ke(m1/3/R)ne ke =49279βe+48721 ne=1.61−0.491×0.9988η βe=η(1+ne)/3 -
[1] RAMAJEYATHILAGAM K, VENDHAN C P. Deformation and rupture of thin rectangular plates subjected to underwater shock [J]. International Journal of Impact Engineering, 2004, 30(6): 699–719. DOI: 10.1016/j.ijimpeng.2003.01.001. [2] HUNG C F, HSU P Y, HWANG-FUU J J. Elastic shock response of an air-backed plate to underwater explosion [J]. International Journal of Impact Engineering, 2005, 31(2): 151–168. DOI: 10.1016/j.ijimpeng.2003.10.039. [3] 汪斌, 张远平, 王彦平. 水中爆炸气泡脉动现象的实验研究 [J]. 爆炸与冲击, 2008, 28(6): 572–576. DOI: 10.11883/1001-1455(2008)06-0572-05.WANG B, ZHANG Y P, WANG Y P. Experimental study on bubble oscillation formed during underwater explosions [J]. Explosion and Shock Waves, 2008, 28(6): 572–576. DOI: 10.11883/1001-1455(2008)06-0572-05. [4] 朱凌, 段沐德, 黄骏德. 固支方板对水下爆炸的塑性动力响应 [J]. 海军工程大学学报, 1987(3): 1009–3486. [5] LEE J J, SMITH M J, HUANG J, et al. Deformation and rupture of thin steel plates due to cumulative loading from underwater shock and bubble collapse [J]. Shock and Vibration, 2011, 18(3): 459–470. DOI: 10.3233/SAV-2010-0526. [6] 郑监, 卢芳云, 李翔宇. 金属板在水下爆炸加载下的动态响应研究进展 [J]. 中国测试, 2018, 44(10): 20–30. DOI: 10.11857/j.issn.1674-5124.2018.10.004.ZHENG J, LU F Y, LI X Y. Research progress on dynamic response of metal plate in underwater explosion loading [J]. China Measurement & Test, 2018, 44(10): 20–30. DOI: 10.11857/j.issn.1674-5124.2018.10.004. [7] 张效慈. 水下爆炸试验相似准则 [J]. 船舶力学, 2007, 11(1): 108–118. DOI: 10.3969/j.issn.1007-7294.2007.01.014.ZHANG X C. Similarity criteria for experiment of underwater explosion [J]. Journal of Ship Mechanics, 2007, 11(1): 108–118. DOI: 10.3969/j.issn.1007-7294.2007.01.014. [8] LEBLANC J, GARDNER N, SHUKLA A. Effect of polyurea coatings on the response of curved E-Glass/Vinyl ester composite panels to underwater explosive loading [J]. Composites Part B: Engineering, 2013, 44(1): 565–574. DOI: 10.1016/j.compositesb.2012.02.038. [9] LEBLANC J, SHUKLA A. Dynamic response of curved composite panels to underwater explosive loading: experimental and computational comparisons [J]. Composite Structures, 2011, 93(11): 3072–3081. DOI: 10.1016/j.compstruct.2011.04.017. [10] LEBLANC J, SHUKLA A. Response of E-glass/vinyl ester composite panels to underwater explosive loading: effects of laminate modifications [J]. International Journal of Impact Engineering, 2011, 38(10): 796–803. DOI: 10.1016/j.ijimpeng.2011.05.004. [11] DESHPANDE V S, HEAVER A, FLECK N A. An underwater shock simulator [J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2006, 462(2067): 1021–1041. DOI: 10.1098/rspa.2005.1604. [12] ESPINOSA H D, LEE S, MOLDOVAN N. A novel fluid structure interaction experiment to investigate deformation of structural elements subjected to impulsive loading [J]. Experimental Mechanics, 2006, 46(6): 805–824. DOI: 10.1007/s11340-006-0296-7. [13] FILLER W S. Propagation of shock waves in a hydrodynamic conical shock tube [J]. The Physics of Fluids, 1964, 7(5): 664–667. DOI: 10.1063/1.1711266. [14] ZALESAK J F, POCHÉ JR L B. The shock test facility: an explosive-driven, water-filled conical shock tube [C]// Proceedings of a Conference Sponsored by the Department of Defense, the National Aeronautics and Space Administration, and the Department of Energy. Virginia Beach, 1989: 73−76. [15] HESHMATI M, ZAMANI J, MOZAFARI A. The experimental and numerical impacts of geometrical parameters of conical shock tube on the function, maximum pressure and generative impulses to expose equivalent mass and behavioral equation [J]. Materials Science & Engineering Technology, 2016, 47(7): 623–634. DOI: 10.1002/mawe.201600510. [16] BJØRNØ L, LEVIN P. Underwater explosion research using small amounts of chemical explosives [J]. Ultrasonics, 1976, 14(6): 263–267. DOI: 10.1016/0041-624X(76)90033-0. [17] 库尔 P. 水下爆炸[M]. 罗耀杰, 韩润泽, 官信, 等, 译. 北京: 国防工业出版社, 1960: 167−168; 219. [18] KEIL A H. The response of ships to underwater explosions [R]. New York: Society of Naval Architects and Marine Engineers, 1961: 43. 期刊类型引用(5)
1. 刘芳,郝慧敏,卢熹,郭策安. 基于AGEP-DNN的水下聚能装药比冲量预测模型. 沈阳理工大学学报. 2024(02): 15-21+28 .  百度学术
百度学术2. 程建华,孟兵兵,王辉,顿志林,张新生,郭帅房. 大型激波管产生的长持时冲击波的特性研究. 爆破器材. 2024(05): 13-20 .  百度学术
百度学术3. 王振宁,尹建平,伊建亚,李旭东. 柱形装药近地动爆冲击波周向传播规律研究. 爆炸与冲击. 2023(06): 90-107 .  本站查看
本站查看4. 张军,黄含军,毛勇建,黄海莹,郑星,杨茂. 爆炸激波管出口流场特征的纹影诊断与分析. 光学精密工程. 2023(19): 2789-2798 .  百度学术
百度学术5. 周岳兰,裴鲁,龙仁荣,张庆明,刘博文,任健康. 激波管内压力脉冲演化特性及模拟空爆冲击波的方法研究. 兵工学报. 2023(12): 3815-3825 .  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:
























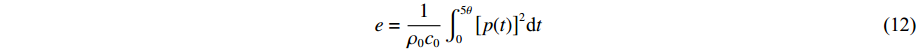








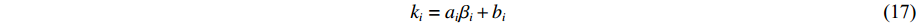











 下载:
下载:

















 百度学术
百度学术