Dynamic failure mechanism of gas pipeline with flange joint under blasting seismic wave
-
摘要: 基于典型城市燃气管道直埋地层特点，通过全尺寸直埋燃气管道爆破地震实验，并结合LS-DYNA动力有限元数值计算软件建立不同爆源距离的无接口和法兰接口的燃气管道模型，分析研究了爆破地震波作用下法兰接口燃气管道动力响应特征及其失效机制。研究结果表明：管道截面应变以轴向拉伸应变为主，环向应变为辅；不同爆破工况下，无接口管道和法兰接口管道及地表峰值振动速度随爆源距离减小而增大；沿管道轴线方向，无接口管道、地表峰值振动速度以管道中心截面为对称面沿两端不断减小，法兰接口管道峰值振速由两侧向中间逐渐增大，在法兰接口处突然减小；法兰接口处出现明显的应力集中现象；管道法兰接口处是爆破地震作用下研究的关键点，螺栓的峰值有效应力、垫片轴向压力、法兰峰值有效应力、法兰偏转角随爆源距离增大而减小；法兰管道偏转角与地表峰值振动速度具有对应关系，法兰接口燃气管道中心正上方地表的控制振速（13.82 cm/s）可作为邻近燃气管道爆破工程地表的安全控制值。Abstract: In the process of blasting and excavation of urban subways, controlling the impact of blasting vibration on adjacent pipelines is critical. Based on the characteristics of directly buried gas pipelines in Wuhan and the full-scale direct-buried gas pipeline blasting seismicexperiment, the dynamic finite element numerical calculation software LS-DYNA was used to establish gas pipeline without joints and flange gas pipeline models under different blasting source distances. The effects of blasting seismic wave’s dynamic response characteristics of flanged gas pipeline were analyzed. The research results show that the strain of pipeline section is mainly axial tensile strain, supplemented by circumferential strain. The peak particle velocity of pipeline without joints and flange pipes and the ground surface increase with the decrease of the distance from the blasting source under different blasting conditions. Along the pipeline axis, the peak vibration velocity of the pipeline without joints and the ground surface decreases along the two ends with the central section of the pipe as the symmetry plane. The peak particle velocity of the flange pipeline gradually increases from two sides to the middle but suddenly decreases at the flange joint. There is an obvious stress concentration at the flange interface. The flange joint is the key point of pipeline under blasting earthquake. The peak effective stress of the bolt, the axial pressure of the gasket, the peak effective stress of the flange, and the flange deflection angle decrease with the increase of the explosion source distance. The deflection angle of the flanged pipeline has a corresponding relationship with the peak vibration velocity of the ground surface. The control vibration speed of 13.82 cm/s on the surface directly above the center of the flanged gas pipeline is used as the safety control value of the adjacent gas pipeline under blasting engineering.
-
Key words:
- blasting vibration /
- dynamic response /
- vibration speed /
- flange interface /
- control vibration speed
-
炸药库、火药库、油气库、弹药库等危险品仓库在雷电、火灾、武器打击等外界作用下，库内储存的危险品易发生爆炸，引发严重的安全事故。“8•12天津滨海新区爆炸事故”导致165人死亡，798人受伤，直接经济损失达70亿元[1]；俄乌冲突中，俄军新卡霍夫卡军火库被乌军击中并发生爆炸，导致严重的人员伤亡[2]。为确保库房发生爆炸时周围人员和环境的安全，充分利用建设场地的地形地貌并预留充足的安全距离，是建设危险品仓库的首要考量[3]。
根据不同的结构形式，典型的危险品仓库可分为地面库、地下库和覆土库[4-8]3类。对各类型危险品仓库的安全距离已有广泛研究，并取得了丰富的成果。文献[4]中指出，地面库强度低，需与防护土堤等防护设施配合建设。地面库不能有效限制爆炸冲击波的传播和爆炸破片的飞散[4]，且不得存储有整体性爆炸风险的爆炸物[5]。Park等[9]基于理论分析和数值计算比较了地面库和地下库的安全距离，指出地下库的最小库间安全距离比地面库小62.8%。
地下库发生爆炸时会引发强烈地震动，导致相邻库室墙壁剥落并产生破片抛掷，引发殉爆[5]。云庆[10]、刘桂英等[11]以相邻库室墙壁不发生剥落为准则，对地下库覆盖层的临界厚度进行了研究，得到了相邻库室的最小间距。Sugiyama等[12-18]通过缩尺试验和数值计算的方法对地下库爆炸产生的冲击波进行了研究，分析了库房洞室长径比、口部尺寸及装药形状对冲击波的传播和安全距离的影响。Wu等[19]通过数值计算的方法对不同围岩条件下的地下库内爆炸进行了研究，提出了估算地下库周围损伤区域、相邻库室之间安全间距和安全埋置深度的经验公式。
由于建设方便、费效比优良，覆土库在危险品仓库建设中广泛使用。Weals[20]对覆土库开展了4组大比例爆炸试验，得到了防止两侧及后方库房相邻库房殉爆的最小库间距离和结构破片的飞散范围。Oswald[21]认为，覆土库爆炸破片的飞散范围与药室比和库房的材料有关。李铮等[22]通过缩尺试验，研究了丘陵地区覆土危险品仓库爆炸冲击波的传播规律，指出了该型库房爆炸时冲击波流场分布的方向性。Kim等[23]、荣凯等[24]分别通过缩尺试验和数值计算研究了覆土库爆炸产生的冲击波的衰减规律，表明覆土可在一定程度上限制爆炸冲击波向库房两侧及后方传播，库房两侧及后方的超压峰值、冲量小于地面库爆炸。渡辺萌奈等[25]通过1∶10和1∶20的小比例缩尺爆炸试验对覆土库的爆炸破片进行研究，发现覆土越厚爆炸破片的飞散范围越小。
现有研究结果表明，危险品仓库的安全距离受其结构形式的影响很大：一般情况下，地面库安全距离大[5]；地下库多修建于山体中，可有效减小安全距离，但其建设受到地形地貌的限制大[4-5]；覆土库适用范围广，其爆炸冲击波的流场分布具有方向性，与地面库相比能在一定程度上减小两侧及后方的安全距离，但减小程度有限，且破片的方向性不强[5]。虽然上述3种形式的库房已被广泛研究和应用，但仍难以满足在相对平坦开阔场地且安全距离受限环境中的建设需求。
为解决此问题，本文中借鉴地下库和覆土库的结构特征和研究成果，针对一种新的结构形式的危险品仓库，开展3组缩尺模型野外爆炸试验，研究仓库各组成部分对爆炸冲击波传播和爆炸破片飞散的影响，拟合冲击波超压峰值随比例距离的衰减公式，给出新型危险品仓库的爆炸冲击波安全距离，以期为平坦场地、安全距离受限的条件下修建危险品仓库提供解决方案。
1. 新型危险品仓库的结构形式
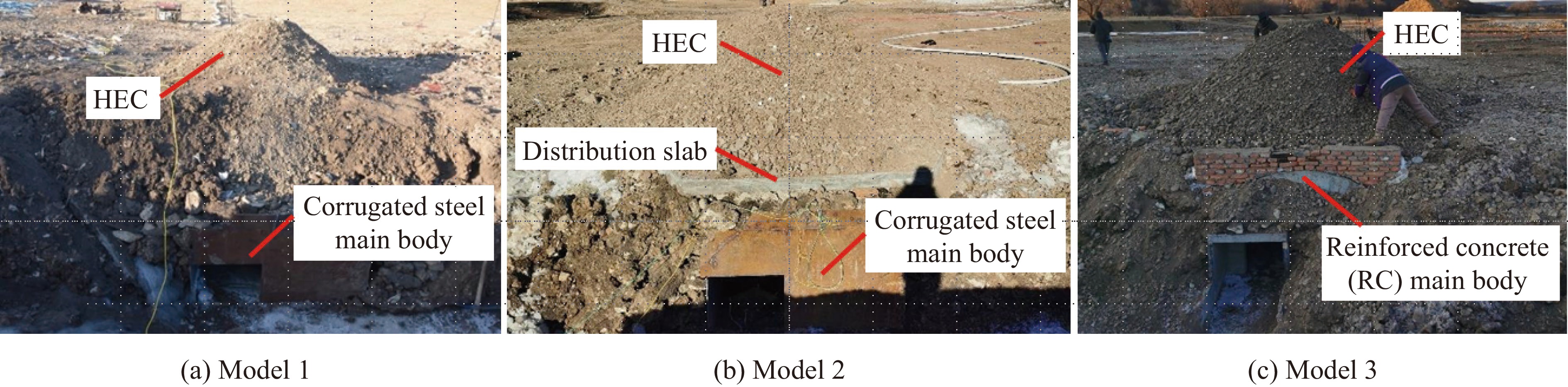
借鉴地下库和覆土库爆炸冲击波和破片具有方向性的特点，新型危险品仓库的基本设想是：修建一种地下浅埋式危险品仓库，库房前端朝向允许泄爆方向，库房主体上方两侧和后方一定范围内堆填适当高度的土壤，以限制库房爆炸对两侧及后方的影响，减小库房两侧及后方的安全距离。进一步在主体上方堆土中设置一块钢筋混凝土板（称为分配板），使更多的堆土能够参与抑制爆炸冲击波传播，以期分散库房爆炸产生的冲击波荷载。由此，新型危险品仓库主要由浅埋式库房主体、顶部堆土（heaped-up earth cover, HEC）和钢筋混凝土分配板3部分组成，如图1所示。
库房主体的断面形式为直墙拱，口部朝向开阔方向（泄爆方向），前墙外侧少量堆土或不堆土。顶部堆土前低后高，截面形状为梯形，梯形上底位于装药中心后侧。分配板前小后大呈梯形，底面与地面平齐，前端在装药中心以前，后端在库房主体之后，左右两端完全覆盖库房主体。前墙外侧设置运输通道，用于库内危险品的运输。
2. 试验设计
2.1 试验模型
为验证新型库房设计的合理性，研究分配板和库房主体强度对爆炸冲击波、爆炸破片的影响，制作了3组缩尺模型。缩尺模型各组成部分如图2所示。库房主体强度分为“强”“弱”两种。“强库房”为钢筋混凝土结构，采用C30混凝土和HRB 400级钢筋；库房主体墙壁厚0.10 m，配筋率0.8%，前墙为砌体结构；“弱库房”为波纹钢结构。分配板为钢筋混凝土结构，尺寸为3.1 m×2.5 m/5.5 m×0.15 m（长×宽（前/后）×厚），采用C80混凝土和HRB 400级钢筋，配筋率1.0%。
3组试验模型分别编号为模型1、2、3。3组试验模型顶部堆土的高度、范围、土质等情况均一致，模型1为“弱库房”结构，无分配板；模型2设置分配板，其余情况同模型1；模型3为“强库房”结构，设置分配板。库房主体、分配板及顶部堆土的尺寸及相对位置如图3所示。图4为库房模型现场照片，模型3分配板埋在土中，分配板不可见。
图 3 试验模型及顶部堆土尺寸（单位：m）Figure 3. Size of the test models and heaped-up earth cover (unit: m)2.2 试验工况
根据新型危险品仓库的设计储药量和爆炸相似律[26]，各缩尺试验模型的装药量均为156 kg，采用柱状TNT装药，装药底面中心与库房主体的地面中心重合，如图5所示。采用8#电雷管起爆及120 g传爆药柱传爆。
对比试验模型1~2，研究分配板对爆炸冲击波传播、爆炸破片飞散的影响；对比试验模型2和模型3，研究库房主体强度对爆炸冲击波传播、爆炸破片飞散的影响。试验工况如表1所示。
表 1 缩尺试验模型情况Table 1. Cases of scaled test model模型 库房主体材料 分配板 装药量/kg 1 波纹钢 无 156 2 波纹钢 有 156 3 钢筋混凝土 有 156 2.3 测点布置
试验测量了库房周围的地面冲击波压力分布，并记录了3组模型试验的爆炸过程。
地面冲击波压力测点布置如图6所示，以模型的开口朝向为0°方向，沿逆时针在0°、30°、60°、90°、135°、180°方向分别布置冲击波压力测点。压力传感器采用PCB 113型，如图7所示，传感器测量面与地面平齐，量程范围均为0 ～ 0.69 MPa。
采用IX黑白高速摄像机记录爆炸过程，拍摄速度为10000 s−1。使用徕卡FlexLine plus全站仪统计爆炸破片的飞散角度和飞散距离。
3. 试验结果
3.1 爆炸过程
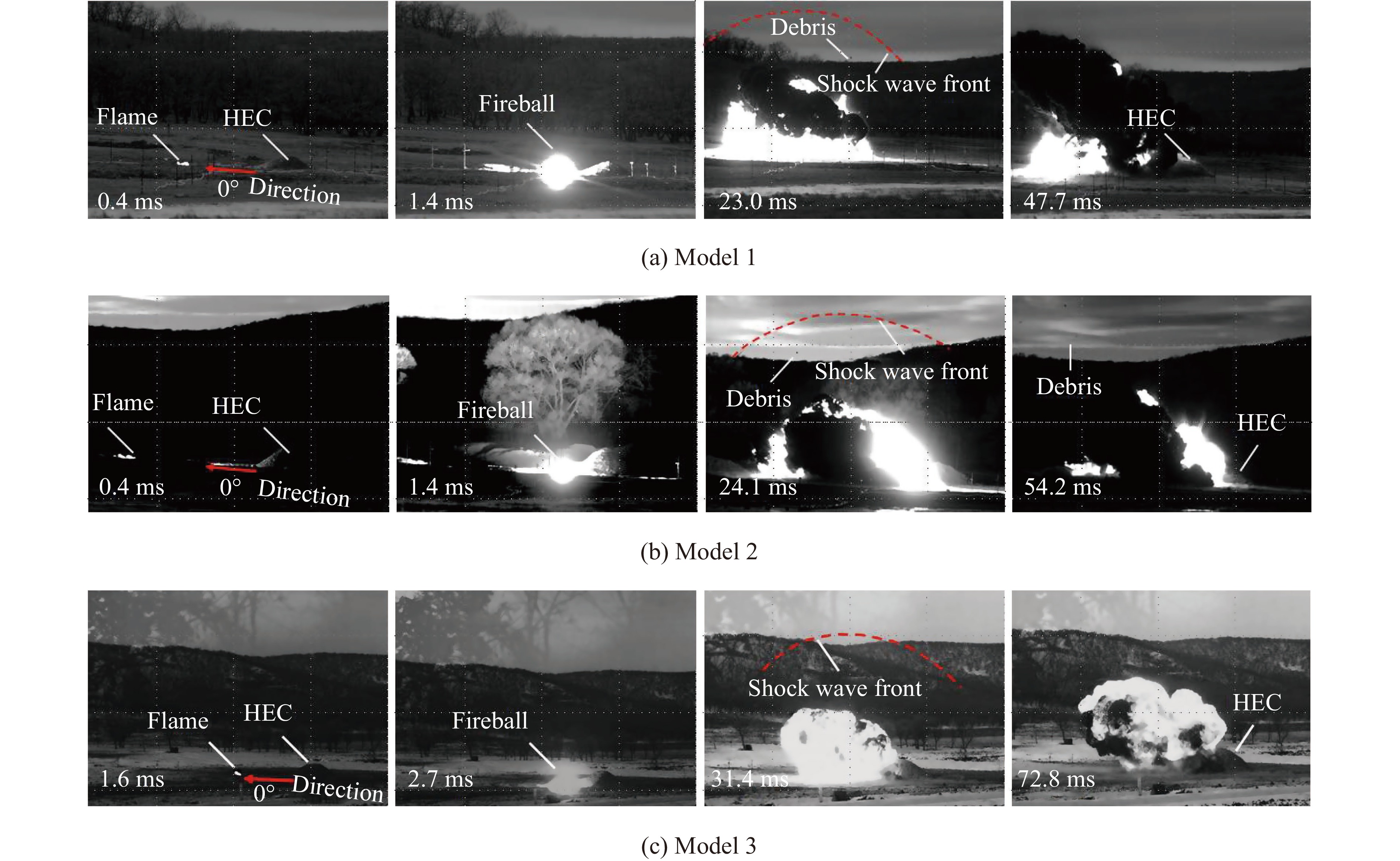
图8给出了高速摄像机记录的试验模型爆炸过程。可将爆炸过程分为4个阶段。（1）起爆阶段，即炸药起爆至爆轰产物冲出库房口部阶段。炸药起爆后，爆轰产物从库房前端冲出，受运输通道阻挡向上传播，并出现火光。以炸药起爆时刻为0 ms，模型1、2、3出现火光的时刻分别为0.4、0.4、1.6 ms。（2）前墙破坏阶段，即爆轰产物冲出库房口部至前墙破坏阶段。在炸药的爆炸作用下，库房前墙迅速破坏，爆轰产物泄出并形成火球，伴有冲击波从库房前墙处泄出。模型1、2、3出现火球的时刻分别为1.4、1.4、2.7 ms。（3）结构破坏阶段，即前墙破坏至顶部堆土开始掀起阶段。在爆炸荷载作用下，库房主体发生破坏并产生结构破片，顶部堆土向上掀起，堆土和破片向前、向上飞散。此阶段可以清晰地观察到冲击波呈椭球形向外传播，椭球球心位于前墙处，冲击波从顶部堆土上方、两侧绕射至库房后侧。试验模型1、2、3顶部堆土开始掀起的时刻分别为47.7、54.2、72.8 ms，顶部堆土开始掀起时，冲击波波阵面已在高速摄像机画面外。（4）破片、堆土飞散阶段，即顶部堆土掀起至堆土、破片落地阶段。此阶段结构破片向前飞散，顶部堆土从前至后被掀起、抛洒。
爆炸过程中，3组试验模型的爆炸冲击波均以库房前墙处为中心向外传播，爆炸破片向前、向上飞散，说明本文中提出的库房形式改变了爆炸冲击波传播和破片飞散的方向，达到了定向泄爆的目的。
3.2 超压峰值
提取3组试验模型中各测点的冲击波超压峰值，并与TNT在软质地面爆炸的经验公式[27]计算结果进行对比。经验公式[27]为：
p=0.102Z+0.399Z2+1.26Z3 (1) 式中：p为超压峰值，MPa；Z为比例距离，m/kg1/3。
试验数据与对比结果如图9所示。由图9可知，试验测得的超压峰值随比例距离的增大呈对数衰减，这与经验公式计算结果趋势一致，主要由TNT爆炸产生的冲击波在空气中扩散衰减造成的。对比不同方向的超压峰值发现，同一比例距离处超压峰值随角度增加而减小。例如，比例距离为1.39 m/kg1/3处，模型1、2、3在180°方向的超压峰值分别为0°方向的23%、12%、9%，比例距离为13.23 m/kg1/3处，模型1、2、3的超压峰值分别为0°方向的59%、43%、25%，说明爆炸冲击波传播过程具有明显的方向性。
3.3 爆炸破片
3组试验模型的爆炸破片均分布在库房前侧−90°～90°方向范围内，如图10所示。模型1的典型破片为4块波纹钢破片，其中3块分布在−30°～30°范围内，飞出较远的一块在45°方向；模型2的爆炸破片主要分布在−30°～30°范围内；模型3的爆炸破片集中分布在−60°～60°范围内。由此说明，3组试验模型爆炸破片的分布具有明显的方向性，符合定向泄爆的预期。
对比各组试验模型的破片数量及分布范围发现，混凝土库房的破片数量和飞散距离远大于波纹钢库房，最大飞散比例距离达32.8 m/kg1/3，是模型1的2.5倍。这表明波纹钢材料延展性好，在库房内爆炸作用下形成的破片体积大、数量少，而钢筋混凝土材料延展性差，在爆炸荷载作用下，易形成大量破片。
4. 冲击波安全距离
4.1 冲击波超压分布规律
为定量研究新型危险品仓库爆炸冲击波的方向性及分配板、库房主体强度对冲击波传播的影响，对比3组试验模型在同一方向上的超压峰值，如图11所示。
图 11 冲击波超压峰值、地面爆炸经验公式及拟合公式Figure 11. Peak pressures, empirical formula and fitting functions of shock waves由图11可知，在0° ～ 180°方向上，模型1测得的冲击波超压峰值均小于地面爆炸经验公式[27]的计算结果，且在135°和180°方向上更明显。如比例距离为2.78 m/kg1/3处，相较于地面爆炸经验公式[27]计算结果，模型1的超压峰值降低了54% ～ 76%。
对比试验模型1、2各方向的冲击波超压峰值发现，在顶部堆土、库房强度等条件相同的情况下，分配板提高了模型2在0°、30°方向的超压峰值。比例距离为2.78 m/kg1/3处，模型2的超压峰值分别为64.42、72.04 kPa，相较于模型1的60.15、62.13 kPa分别高出了7%和16%；60°方向上，模型2与模型1的超压峰值相差不大，平均相差0.5%；90°方向上，比例距离大于2.04 m/kg1/3时，模型1、2的超压峰值平均相差不足2%；135°、180°方向上，模型2各测点的超压峰值相较于模型1明显更低，如比例距离为3.25 m/kg1/3处，模型2的超压峰值分别为19.55、17.65 kPa，分别是模型1的73%（26.68 kPa）和68%（26.11 kPa）。这表明分配板能够进一步增强库房的定向泄爆性能，显著降低了库房侧后方、正后方的超压峰值，减小了该方向的安全距离。分配板在库房爆炸作用下的运动变形是与爆炸冲击波传播强耦合的过程[28]：在爆炸作用下分配板向上运动并向后翻转，期间发生变形、破坏，但其结构响应与破坏远滞后于冲击波传播；当冲击波作用于分配板时，由于钢筋混凝土材料强度高、波阻抗大[27]，分配板使更多的堆土能够参与抑制爆炸冲击波向四周扩散，起到了分散爆炸荷载的作用，阻挡冲击波向库房后方传播。
对比试验模型2、3的超压峰值发现，钢筋混凝土库房主体可减小库房两侧、后方的冲击波超压峰值，135°和180°方向尤为明显。以180°方向为例，比例距离为2.78 m/kg1/3处，模型3的超压峰值为13.83 kPa，相较于模型2的23.04 kPa减小了40%；比例距离为7.19 m/kg1/3处，模型3的超压峰值为5.67 kPa，不足模型2超压峰值的50%（11.51 kPa）。这表明库房主体的结构和强度会影响冲击波传播，可归结于两方面因素：（1）相较于模型2，模型3库房主体强度更高，前墙（砌体）强度与库房主体其他部分（钢筋混凝土）强度相差较大，爆炸冲击波作用于库房主体时，前墙迅速破坏并发生泄爆，冲击波与爆轰产物从前墙泄出，从而减小了库房两侧及后方的超压峰值；（2）钢筋混凝土库房主体在破坏过程中能够消耗更多的爆炸冲击波能量，迟滞了库房前部上方覆土掀开的过程，从而降低了库房外侧的爆炸荷载。
4.2 冲击波安全距离
对图11中各方向冲击波超压峰值随比例距离的衰减规律进行公式拟合，以超压峰值0.10、0.05和0.02 MPa作为冲击波对人员的重伤、轻伤和安全的毁伤阈值[29-30]，绘制冲击波超压对人员的毁伤范围，如图12所示。
图 12 各试验模型冲击波超压峰值等值线及对比Figure 12. Isolines and comparison of peak pressures of the test models由图12可知，模型1后方的冲击波安全距离显著小于地面爆炸经验公式[27]的计算结果。135°、180°方向上，冲击波对人员的安全距离分别为4.16和4.37 m/kg1/3，相较于地面爆炸经验公式[26]的计算值（8.38 m/kg1/3）分别减小50%和48%。这与浅埋式库房主体和顶部堆土形状有关：浅埋式库房主体限制了爆炸冲击波向两侧及后方传播；前低后高的堆土形式致使堆土前端强度弱、后端强度高，有利于冲击向前泄出，促进库房内爆炸的定向泄爆，显著减小库房90° ～ 180°方向的冲击波安全距离。
对比试验模型1、2的冲击波安全距离，发现135°、180°方向上，模型2的冲击安全距离分别为3.38、3.07 m/kg1/3，相较于模型1的4.16和4.37 m/kg1/3分别减小了19%和30%；与地面爆炸经验公式[27]相比分别减小了60%和63%。由此说明，分配板能有效减小库房后方的冲击波安全距离。对比试验模型2、3的冲击波安全距离，发现135°、180°方向上，模型3的冲击波安全距离分别为2.40、1.91 m/kg1/3，较模型2的3.38和3.07 m/kg1/3分别减小了29%和38%；与地面爆炸经验公式[27]相比，分别减了71%和77%。由此说明，相较于波纹钢库房主体，钢筋混凝土结构显著减小了库房后方的冲击波安全距离。
对比试验模型3与覆土库[24]的冲击波安全距离，发现135°、180°方向上，覆土库[24]的冲击安全距离分别为4.13、4.27 m/kg1/3，分别为模型3的1.72倍和2.24倍。由此说明，相较于覆土库[24]，新型危险品仓库可使库房后方的冲击波安全距离减小约50%。
5. 结 论
针对一种新的结构形式的危险品仓库开展了缩尺模型试验，验证了新型结构形式的合理性，并分析了主体结构强度和钢筋混凝土分配板的影响，得到以下主要结论。
(1)新型危险品仓库可有效实现定向泄爆，减小爆炸冲击波和破片对库房两侧及后方的影响范围，与地面爆炸经验公式[27]相比，库房两侧及后方的安全距离最大可减小77%；与覆土库[24]相比，库房两侧及后方的安全距离可减小约50%。
(2)顶部堆土能有效限制爆炸冲击波的传播和爆炸破片的飞散，使爆炸破片集中分布在库房前侧，并减小冲击波在库房后方的安全距离。
(3)分配板是新型仓库结构的重要组成部分，能够加强定向泄爆效果、减小库房前侧爆炸破片的飞散角度，并使库房后侧的安全距离进一步减小30%。
(4)与波纹钢库房主体相比，钢筋混凝土库房主体后侧的安全距离最大可减小38%，但产生的破片数量更多，且向库房前端的飞散距离更远、分布范围更大。
-
图 5 实验和数值模拟的波形和频谱图
Figure 5. Waveform and spectrogram of experiment and numerical simulation
图 7 法兰接口管道数值模型示意图
Figure 7. Schematic diagram of numerical model of flange interface pipe
图 13 各个工况的螺栓的有效应力分布图
Figure 13. Effective stress distribution diagram of bolts in various working conditions
图 19 法兰偏转角与地表振速关系
Figure 19. Relationship between flange deflection angle and ground surface vibration velocity
表 1 模型材料参数
Table 1. Model material parameters
材料 密度/(g·cm−3) 弹性模量/GPa 剪切模量/GPa 泊松比 黏聚力/MPa 内摩擦角/（°） 抗拉强度/MPa 管道、法兰 7.85 205.000 1.2 0.33 420.000 螺栓 7.82 210.000 1.0 0.30 660.000 粉质黏土 1.98 0.012 4.3 0.28 0.035 15 0.028 砂岩 2.40 3.000 11.2 0.28 5.500 43 2.580 
下载: 导出CSV
表 2 爆轰产物状态方程参数
Table 2. Detonation product state equation parameters
ρ/（g·cm−3） A/GPa B/GPa R1 R2 ω E0/GPa V/cm3 1.25 214 18.2 4.2 0.9 0.1 4.19 1
下载: 导出CSV
表 3 数值模拟结果与实测数据对比分析
Table 3. Comparative analysis of numerical simulation results and measured data
工况 监测点 合振动速度、应变 误差率/% 现场实验 数值模拟 Ⅰ D3 1.65 cm/s 1.72 cm/s 4.2 D4 1.17 cm/s 1.26 cm/s 7.6 D6 0.76 cm/s 0.72 cm/s 5.3 D7 1.45 cm/s 1.54 cm/s 6.2 S1 28.65×10−6 34.23×10−6 19.4 S2 13.54×10−6 8.56×10−6 3.7 Ⅱ D3 2.84 cm/s 2.76 cm/s 8.0 D4 1.99 cm/s 2.06 cm/s 3.5 D6 2.64 cm/s 2.73 cm/s 9.0 D7 1.32 cm/s 1.46 cm/s 10.6 S1 36.71×10−6 41.23×10−6 12.3 S2 16.12×10−6 13.15×10−6 18.4 Ⅲ D3 6.57 cm/s 6.98 cm/s 6.2 D4 4.18 cm/s 4.45 cm/s 6.4 D6 5.47 cm/s 5.78 cm/s 5.6 D7 3.98 cm/s 4.15 cm/s 4.3 S1 37.15×10−6 43.23×10−6 16.3 S2 15.96×10−6 18.56×10−6 16.2 Ⅳ D3 15.19 cm/s 15.32 cm/s 0.8 D4 11.21 cm/s 12.54 cm/s 1.3 D6 13.18 cm/s 14.25 cm/s 8.1 D7 7.34 cm/s 8.32 cm/s 13.4 S1 187.06×10−6 198.09×10−6 5.9 S2 19.23×10−6 22.63×10−6 17.7 Ⅴ D3 30.45 cm/s 31.56 cm/s 3.6 D4 21.19 cm/s 23.23 cm/s 9.6 D6 28.45 cm/s 29.56 cm/s 3.9 D7 12.15 cm/s 13.21 cm/s 8.7 S1 209.50×10−6 225.61×10−6 7.6 S2 35.62×10−6 42.66×10−6 19.8
下载: 导出CSV
表 4 垫片的各项参数
Table 4. The parameters of the gasket
密度/（g·cm−3） Ex/MPa Ey/MPa Ez/MPa μxy μyz μxz Gxy/MPa Gyz/MPa Gxz/MPa 7.85 232.17 434.51 19089.64 0.44 0.008 0.005 115.88 32770.11 103.59
下载: 导出CSV
-
[1] 管晓明, 张良, 王利民, 等. 隧道近距下穿管线的爆破振动特征及安全标准 [J]. 中南大学学报(自然科学版), 2019, 50(11): 2870–2885. DOI: 10.11817/j.issn.1672-7207.2019.11.026.GUAN X M, ZHANG L, WANG L M, et al. Blasting vibration characteristics and safety standard of pipeline passed down by tunnel in short distance [J]. Journal of Central South University (Science and Technology), 2019, 50(11): 2870–2885. DOI: 10.11817/j.issn.1672-7207.2019.11.026. [2] 夏宇磬, 蒋楠, 姚颖康, 等. 粉质黏土层预埋承插式混凝土管道对爆破振动的动力响应 [J]. 爆炸与冲击, 2020, 40(4): 043302. DOI: 10.11883/bzycj-2019-0207.XIA Y Q, JIANG N, YAO Y K, et al. Dynamic responses of a concrete pipeline with bell-and-spigot joints buried in a silty clay layer to blasting seismic waves [J]. Explosion and Shock Waves, 2020, 40(4): 043302. DOI: 10.11883/bzycj-2019-0207. [3] ASHFORD S A, JUIRNARONGRIT T. Response of single piles and pipelines in liquefaction–induced lateral spreads using controlled blasting [J]. Earthquake Engineering and Engineering Vibration, 2002, 1(2): 181–193. DOI: 10.1007/s11803-002-0064-3. [4] ABEDI A S, HATAF N, GHAHRAMANI A. Analytical solution of the dynamic response of buried pipelines under blast wave [J]. International Journal of Rock Mechanics and Mining Sciences, 2016, 88: 301–306. DOI: 10.1016/j.ijrmms.2016.07.014. [5] KOURETZIS G P, BOUCKOVALAS G D, GANTES C J. Analytical calculation of blast-induced strains to buried pipelines [J]. International Journal of Impact Engineering, 2007, 34(10): 1683–1704. DOI: 10.1016/j.ijimpeng.2006.08.008. [6] LIU X B, ZHANG H, XIA M Y, et al. Mechanical response of buried polyethylene pipelines under excavation load during pavement construction [J]. Engineering Failure Analysis, 2018, 90: 355–370. DOI: 10.1016/j.engfailanal.2018.03.027. [7] 张震, 周传波, 路世伟, 等. 爆破振动作用下邻近埋地混凝土管道动力响应特性 [J]. 哈尔滨工业大学学报, 2017, 46(9): 79–84. DOI: 10.11918/j.issn.0367-6234.201611089.ZHANG Z, ZHOU C B, LU S W, et al. Dynamic response characteristic of adjacent buried concrete pipeline subjected to blasting vibration [J]. Journal of Harbin Institute of Technology, 2017, 46(9): 79–84. DOI: 10.11918/j.issn.0367-6234.201611089. [8] JIANG N, GAO T, ZHOU C B, et al. Effect of excavation blasting vibration on adjacent buried gas pipeline in a metro tunnel [J]. Tunnelling and Underground Space Technology, 2018, 81: 590–601. DOI: 10.1016/j.tust.2018.08.022. [9] 高坛, 周传波, 蒋楠, 等. 基坑开挖爆破下邻近管道振动速度安全阈值研究 [J]. 安全与环境学报, 2017, 17(6): 2191–2195. DOI: 10.13637/j.issn.1009-6094.2017.06.029.GAO T, ZHOU C B, JIANG N, et al. Study on the vibration velocity threshold of the adjacent pipeline under the blasting excavation of the foundation pit [J]. Journal of Safety and Environment, 2017, 17(6): 2191–2195. DOI: 10.13637/j.issn.1009-6094.2017.06.029. [10] 屈若枫, 徐光黎, 王金峰, 等. 武汉地区典型软土物理力学指标间的相关性研究 [J]. 岩土工程学报, 2014, 36(S2): 113–119. DOI: 10.11779/CJGE2014S2019.QU R F, XU G L, WANG J F, et al. Correlations of physical and mechanical properties of typical soft soils in Wuhan [J]. Chinese Journal of Geotechnical Engineering, 2014, 36(S2): 113–119. DOI: 10.11779/CJGE2014S2019. [11] 时党勇, 李裕春, 张胜民. 基于ANSYS/LS-DYNA 8.1进行显式动力分析[M]. 北京: 清华大学出版社, 2005: 156−158. [12] 王先军, 陈明祥, 常晓林, 等. Drucker-Prager系列屈服准则在稳定分析中的应用研究 [J]. 岩土力学, 2009, 30(12): 3733–3738. DOI: 10.3969/j.issn.1000-7598.2009.12.030.WANG X J, CHEN M X, CHANG X L, et al. Studies of application of Drucker-Prager yield criteria to stability analysis [J]. Rock and Soil Mechanics, 2009, 30(12): 3733–3738. DOI: 10.3969/j.issn.1000-7598.2009.12.030. [13] MOKHTARI M, NIA A A. A parametric study on the mechanical performance of buried X65 steel pipelines under subsurface detonation [J]. Archives of Civil and Mechanical Engineering, 2015, 15(3): 668–679. DOI: 10.1016/j.acme.2014.12.013. [14] 郑爽英, 杨立中. 隧道爆破地震下输气管道动力响应数值试验 [J]. 西南交通大学学报, 2017, 52(2): 264–271. DOI: 10.3969/j.issn.0258-2724.2017.02.008.ZHENG S Y, YANG L Z. Numerical experiments of dynamic response of buried gas pipeline under the action of seismic waves induced by tunnel blasting [J]. Journal of Southwest Jiaotong University, 2017, 52(2): 264–271. DOI: 10.3969/j.issn.0258-2724.2017.02.008. [15] 朱斌, 蒋楠, 贾永胜, 等. 下穿燃气管道爆破振动效应现场试验研究 [J]. 岩石力学与工程学报, 2019, 38(12): 2582–2592. DOI: 10.13722/j.cnki.jrme.2019.0183.ZHU B, JIANG N, JIA Y S, et al. Field experiment on blasting vibration effect of underpass gas pipeline [J]. Chinese Journal of Rock Mechanics and Engineering, 2019, 38(12): 2582–2592. DOI: 10.13722/j.cnki.jrme.2019.0183. [16] 中国机械工业联合会. 整体铸铁法兰: GB/T 17241.6-2008 [S]. 北京: 中国标准出版社, 2009. [17] 王志文, 蔡仁良. 化工容器设计[M]. 北京: 化学工业出版社, 2005: 42−45. [18] 中华人民共和国国家市场监督管理总局, 中国国家标准化管理委员会. 水及燃气用球墨铸铁管、管件和附件: GB/T 13295−2019 [S]. 北京: 中国标准出版社, 2019. [19] MATHAN G, PRASAD N S. Evaluation of effective material properties of spiral wound gasket through homogenization [J]. International Journal of Pressure Vessels and Piping, 2010, 87(12): 704–713. DOI: 10.1016/j.ijpvp.2010.10.003. [20] 蒋国庆, 马斌, 陈万华. 螺栓法兰连接结构有限元模型参数确定方法 [J]. 国防科技大学学报, 2020, 42(4): 51–56. DOI: 10.11887/j.cn.202004009.JIANG G Q, MA B, CHEN W H. Parameter determination method for bolted flange’s finite element model [J]. Journal of National University of Defense Technology, 2020, 42(4): 51–56. DOI: 10.11887/j.cn.202004009. [21] 蔡仁良, 顾伯勤, 宋鹏云. 过程装备密封技术[M]. 北京: 化学工业出版社, 2006: 51−52. [22] ASME锅炉及压力容器委员会压力容器分委员会. ASME锅炉及压力容器规范: 2010版. 第8卷. 第1册, 压力容器建造规则[M]. 北京: 中国石化出版社, 2011: 415. [23] 陆晓峰, 沈轶. 高温法兰密封接头的可靠性分析 [J]. 压力容器, 2007, 24(9): 20–24. DOI: 10.3969/j.issn.1001-4837.2007.09.005.LU X F, SHEN Y. Reliability analysis on bolted flanged joints at elevated temperature [J]. Pressure Vessel Technology, 2007, 24(9): 20–24. DOI: 10.3969/j.issn.1001-4837.2007.09.005. [24] 王和慧, 卢均臣, 关凯书, 等. 带接管组合法兰的强度和密封有限元分析 [J]. 压力容器, 2012, 29(2): 22–29. DOI: 10.3969/j.issn.1001-4837.2012.02.005.WANG H H, LU J C, GUAN K S, et al. Strength and seal FE analysis of combined flanges with a pipe [J]. Pressure Vessel Technology, 2012, 29(2): 22–29. DOI: 10.3969/j.issn.1001-4837.2012.02.005. 期刊类型引用(1)
1. 解江，潘汉源，蒋逸伦，杨祥，李漩，郭德龙，冯振宇. 带剪切销抗爆容器定向泄压特性研究. 爆炸与冲击. 2024(07): 139-156 . 
本站查看其他类型引用(0)
-
下载:
计量
- 文章访问数: 495
- HTML全文浏览量: 318
- PDF下载量: 88
- 被引次数: 1