On impact response of a prestressed metal beam
-
摘要: 工程结构在使用过程中,大部分构件处于预应力状态。为了理清预应力对金属梁在冲击载荷作用下响应的影响机理,对不同轴向预应力条件和不同冲击强度下金属梁的塑性变形规律进行了研究。通过自主设计的预应力加载装置和落锤试验机,实现对金属梁的预应力控制和冲击加载;借助商用软件建立数值模型,对相关工况进行模拟。数值模拟结果与试验结果有较好的一致性。通过对梁的剩余挠度进行对比发现,压预应力状态下的梁受冲击载荷作用所产生的中点剩余挠度会比无预应力时更大;而拉预应力状态下的梁,挠度的变化量与预应力之间没有较一致的规律。从能量角度进行分析发现,梁的塑性变形能来自外加动能和初始内能,外加动能的能量比越高,梁的能量吸收率就越高,且在低能量比时,压预应力下的能量吸收率相对较高,拉预应力下的相对较低;高能量比时,预应力对能量吸收率几乎无影响。压预应力下,梁的极限弯矩增大,长度缩小,增大了的塑性变形能分布在长度缩小了的梁内,必然会导致更大的剩余挠度;拉预应力下,梁的极限弯矩减小,长度增大,增大了的塑性变形能分布在长度增大了的梁内,剩余挠度则没有显而易见的规律。这在一定程度上解释了预应力对冲击载荷作用下金属梁变形的影响机理。Abstract: During the service time of engineering structure, most structural members are under prestress conditions. In order to clarify the effect mechanism of prestress on the response of metal beams subjected to impulsive loading, the plastic deformations of metal beams under different axial prestress conditions and different impact strength were studied. The prestress conditions were controlled by a self-designed prestress loading device while the impact loadings were realized by the drop-hammer method. Numerical models were also established to simulate the related test conditions. The numerical results are in good agreement with the test results. By comparing the residual deflections of the beams, it is found that the middle-point residual deflection under compressive prestress is larger than that without prestress, and there is no regular rule between the deflection and prestress under the condition of tensile prestress. From the perspective of energy, it is found that the plastic deformation energy of the beam comes from the external dynamic energy and the initial internal energy. The higher the external kinetic energy ratio is, the higher the energy absorption rate of the beam will be. At a lower external kinetic energy ratio, the energy absorption rate of the beam is relatively higher under compressive prestress, and relatively lower under tensile prestress. While at a higher external kinetic energy ratio, the prestress has little effect on the energy absorption rate. Under compressive prestress, the limit moment increases while the length decreases, and the increased plastic deformation energy is distributed in the beam with reduced length, which will inevitably lead to larger residual deflection. Under the tensile prestress, the limit moment decreases while the length increases, and the increased plastic deformation energy is distributed in the beam with the increased length, for which the residual deflection has no obvious rule. This explains to a certain extent the effect mechanism of prestress on the deformation of the metal beam subjected to impact loading.
-
Key words:
- metal beam /
- prestress /
- impact response /
- residual deflection
-
金属梁是结构的基本构件,研究其在冲击载荷作用下的损伤机理是工程中的一个重要课题。自1973年Menkes等[1]发表了关于梁的损伤模式的研究成果以来,关于冲击加载下梁板的变形预测和损伤模式分类的研究有很多。例如:Jones[2]对产生梁的不同失效模式的临界速度进行了推导;Liu等[3]对拉伸撕裂和剪切破坏的机理进行了研究,找出了决定这些损伤模式的关键因素;Yakov[4]讨论了轴向压应力对极限弯矩的影响;此外,还有很多研究侧重于梁结构的弹性响应问题[5-7]以及梁结构的疲劳破坏问题[8-9]。这些研究很少涉及预应力对金属梁冲击响应的影响。但在实际使用过程中,金属梁在受到冲击载荷作用时,大多处于预应力状态。为了理清预应力对金属梁在冲击载荷作用下损伤的影响机理,本文中对轴向预应力条件下的金属梁进行一系列研究,且仅考虑损伤模式为塑性变形的情况。
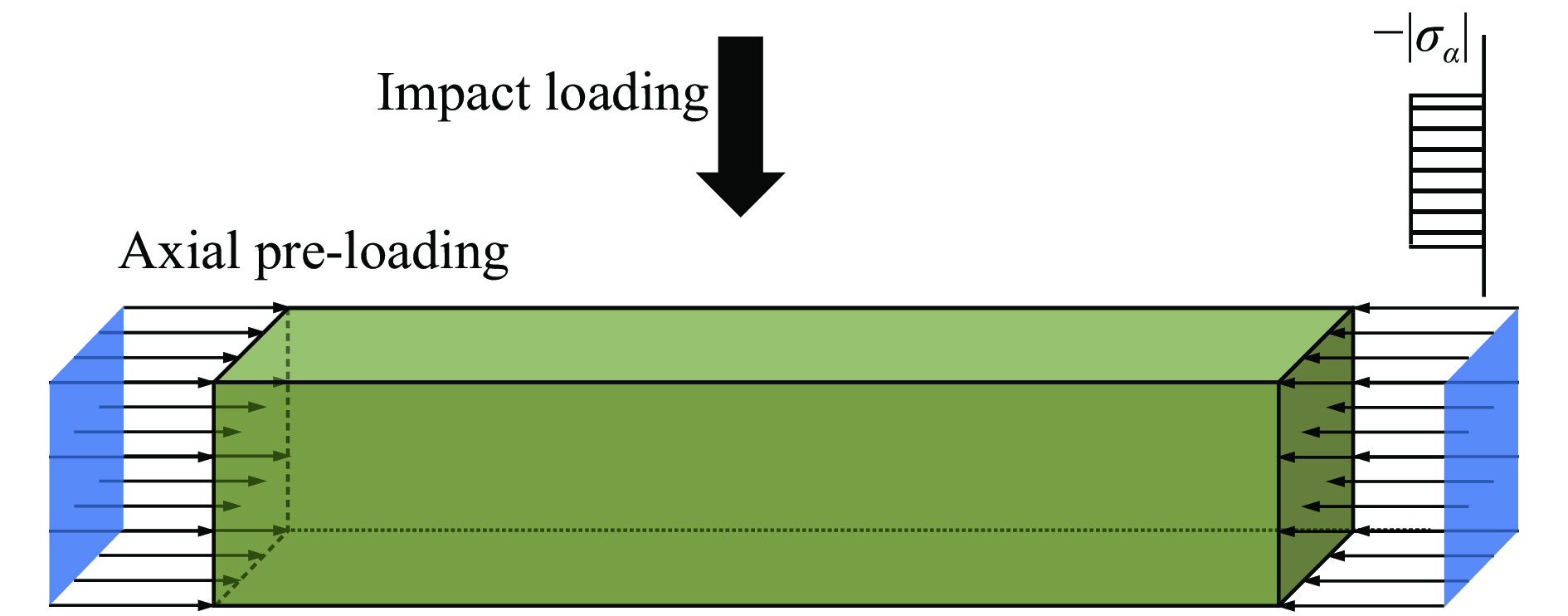
图1为轴向预应力条件下的梁受到横向冲击载荷的示意图。一般情况下,梁所受到的轴向预应力
σα 在材料的屈服应力σ0范围内。通过自主设计的预应力加载装置和落锤试验机,实现了对试件的预应力控制和冲击加载;借助商用软件AUTODYN建立数值模型,对相关工况进行了模拟,两者得到的结果具有较好的一致性。从能量角度对梁塑性变形能的来源、变化和分配进行了分析,有效地解释了预应力对冲击载荷作用下金属梁变形的影响机理。1. 落锤加载下预应力金属梁的试验研究
1.1 试验装置和原理
预应力条件下对金属梁的冲击加载试验主要包括两方面内容:一是对金属梁进行预应力加载和控制,二是对金属梁进行冲击加载和测试。预应力加载原理如图2所示,通过机械控制可移动端的左右移动,使梁在受冲击载荷之前处于一定的预应力状态。梁表面的应变片用于实时监测加载过程中的预应力状态。采用惠斯通电桥,对梁轴向的应变进行监测,采用单臂测量,因此预应力
σα 的值可以表示为:σα=E4ΔUK1K2U0 (1) 式中:E为梁构件材料的弹性模量,且E=73.3 GPa;
ΔU 为测得的电平高度;K1 为应变片的灵敏度因数,且K1=1.93 ;K2 为应变仪的放大因数,且K2=100 ;U0 为供电电压,且U0=8V 。落锤加载试验如图3所示,其中落锤质量为10.4 kg,可调节高度H为0~300 cm。
通过调节落锤高度,对处于一定预应力状态的梁进行冲击加载,获得梁在不同预应力状态下受不同冲击载荷作用产生的响应变化。试验用的梁构件为6061铝,其结构尺寸如图4所示。梁的宽b、厚h、长L分别为10、10、100 mm。
1.2 试验方案和结果
借助上述试验装置,设计了8种试验工况,其中每种工况都进行了多次重复性试验以消除不确定因素的影响。具体工况如表1所示。
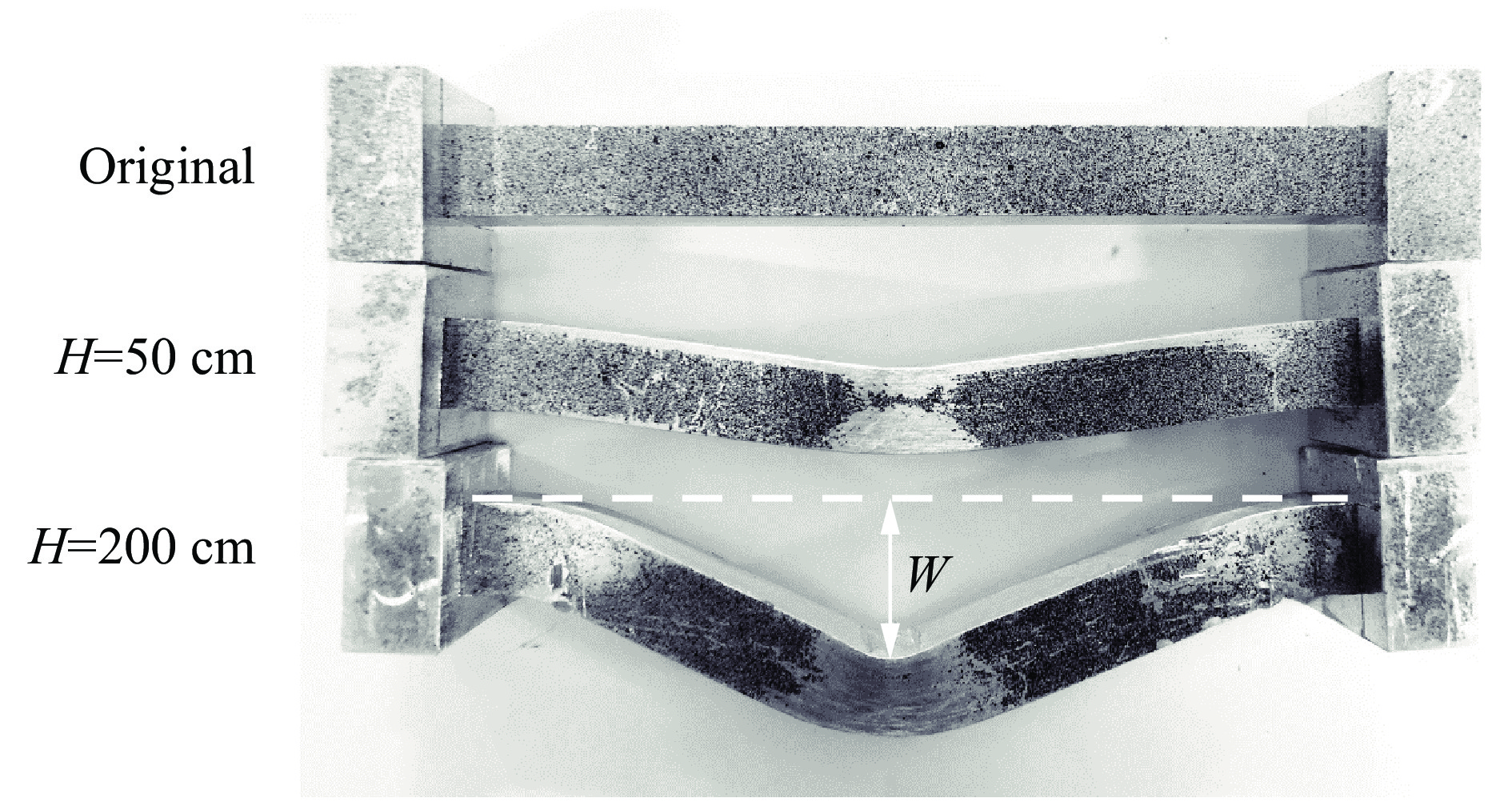
表 1 落锤试验的工况Table 1. Conditions of drop-weight tests工况 H/cm ΔU/mV 1 50 0 2 100 0 3 150 0 4 200 0 5 100 −310 6 100 −410 7 100 350 8 100 450 试验结束后的梁试件发生变形,部分试件的变形效果如图5所示,W为梁中点的剩余挠度。
对试验后梁的中点剩余挠度进行测量,并对每组试验的结果取平均值,最终得到梁的塑性变形结果如表2所示,表中
v0 为落锤对梁的撞击速度。表 2 不同试验工况下梁中点的剩余挠度Table 2. Residual deflections at the middle points of the beams under different test conditions工况 H/cm v0/(m·s−1) ΔU/mV σα/MPa W/mm 1 50 3.13 0 0 5.06 2 100 4.43 0 0 9.71 3 150 5.42 0 0 12.66 4 200 6.26 0 0 16.96 5 100 4.43 −310 −44 9.78 6 100 4.43 −410 −58 10.48 7 100 4.43 350 36 9.70 8 100 4.43 450 63 8.90 2. 落锤加载下预应力金属梁的数值模拟
2.1 模拟模型和方法
为了获得更多数据,借助商用软件AUTODYN对相关工况进行数值模拟。数值模拟同样主要包括两方面内容:一是预应力的加载,二是冲击载荷的加载。
根据图3,构建模拟模型。为了简化相关过程和结构件,通过赋予落锤初速度来简化落锤下落过程;通过赋予梁两端固定边界条件来简化夹持装置与梁的相互作用。最终的简化模拟模型如图6(a)所示。
落锤的材料为钢,对其采用Johnson-Cook本构模型:
σ=(As+Bsεnsp)(1+Cln˙ε∗)(1−T∗ms) (2) 式中:
εp 为有效塑性应变,˙ε∗=˙εp/˙ε0 ,˙ε0=1s−1 ;T∗=(T−Tr)/(Tm−Tr)∈[0,1] ,T、Tr和Tm分别为温度、室温和材料的熔化温度;As、Bs、ns、C和ms为材料常数。材料基本参数见表3。落锤的锤体为长方体,其宽、厚、高分别为120、50和200 mm。落锤的锤头为梯形体,其下底宽、上底宽、厚、高分别为4、40、10、38 mm。模型中整个落锤的质量(约9.54 kg)与试验中落锤质量(10.4 kg)较接近。由于落锤不是所研究的对象,且变形较小,所以对其进行网格划分时,采用较大尺寸网格以节约计算资源,网格划分情况如图6(b)所示,锤体的网格尺寸为10 mm×1 mm×10 mm。表 3 材料的基本参数Table 3. Basic parameters for selected materials材料 ρ0/(kg·m−3) E/GPa µ As/MPa Bs/MPa ns C ms 钢 7.896×103 211 0.29 350 275 0.36 0.022 1.00 铝 2.77×103 73 0.33 337 343 0.41 0.01 1.00 梁的材料为铝,由于落锤试验不涉及非常高的应变率和较大的温度变化,对该材料也采用Johnson-Cook本构模型,对应的基本材料参数见表3,ρ0为材料初始密度,µ为泊松比。梁为长方体,尺寸b×h×L为10 mm×10 mm×100 mm。由于更关注的是梁的变形,因此对梁进行网格划分时,采用1 mm×1 mm×1 mm的均匀网格,如图6(b)所示。
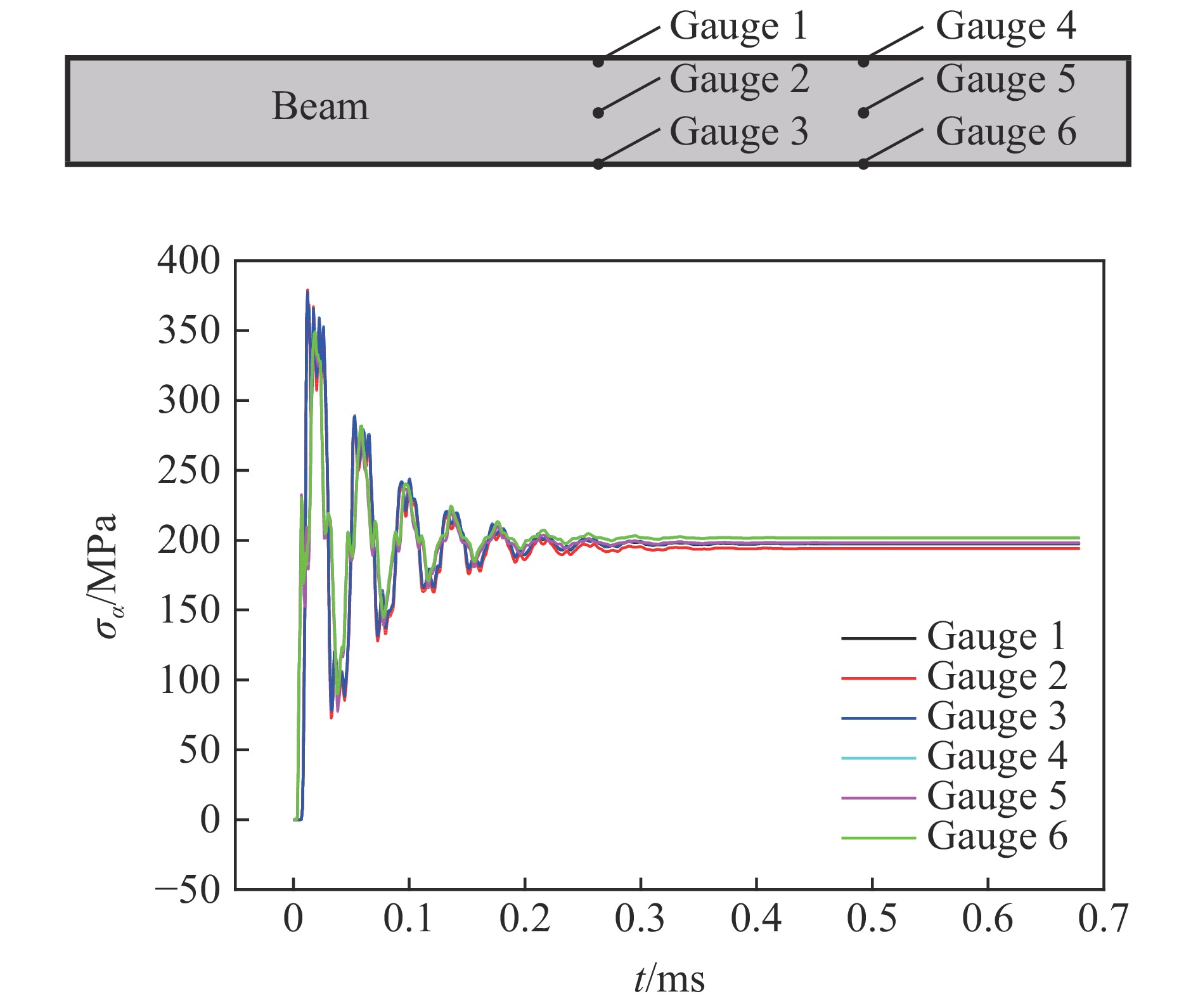
为了使金属梁在受到撞击前处于稳定的轴向预应力状态,先在梁构件的两个端面赋予常应力边界条件。图7展示了在常应力边界条件下,梁内部不同位置轴向应力的稳定过程,该示例工况所设计的常应力边界条件为200 MPa。由图7可知,约0.3 ms后,梁内部各点轴向应力基本保持稳定,约为200 MPa,达到了预设的应力状态。在梁的轴向应力稳定后,再赋予落锤初速度,实现落锤对梁的冲击加载。计算时设计的落锤初速度为2~8 m/s。
2.2 模拟有效性的验证
为了验证所建模型的有效性,从落锤与梁的相互作用过程、梁响应结果的定性和定量分析3个方面对数值模拟结果和试验结果进行了对比。首先是落锤与梁的相互作用过程,主要通过落锤在撞击过程中的速度和位移变化来体现。图8(a)所示为通过试验获取的高速摄影图片得到的落锤位移变化曲线和从数值模拟结果提取的落锤位移曲线,图8(b)为通过差分计算得到的落锤速度变化曲线,其中试验落锤下落高度H=50 cm(对应落锤撞击梁的理论初始速度v0=3.13 m/s),数值模拟中落锤撞击梁的初始速度v0=3.5 m/s。由于试验中和数值模拟中的落锤质量存在一定差别,因此选用的落锤初速也存在一定差别,以保证落锤动量基本相等。由图8可以看出,落锤以一定初速撞击梁后,会以一定速度回弹,回弹速度约为−1 m/s,且数值模拟中的落锤速度和位移的变化过程与试验中的较一致。这说明数值模拟得到的落锤与梁相互作用的整个过程与试验结果较一致。
在梁响应结果的定性方面,图9所示为数值模拟中落锤初速度v0=6 m/s、初始预应力
σα=0 MPa时梁的最终变形形态以及试验工况2(H=100 cm)中某一梁的最终变形形态。由图9(a)中模拟模型的屈服应力分布可知,在梁中主要有3块塑性变形区,分别为固定端的2个塑性变形区和梁跨中的塑性变形区,在3块塑性变形区之间的蓝色区域塑性变形较小;图9(a)中试验试件表面的栅格变形情况也同样显示梁在落锤加载下主要出现3块塑性变形区。图9(b)所示为梁跨中底部的颈缩现象,模拟结果和试验试件中均呈现出了这一现象。由此可知,定性上所采用的计算模型能够较好地模拟梁受撞击后的响应现象。为了从定量上验证数值模拟模型的有效性,对比初始预应力
σα=0 MPa时不同落锤初始速度的数值模拟结果和不同落锤初始高度的试验结果,如图10所示,横坐标为落锤的初始动量,纵坐标为梁中点的剩余挠度。由两者的对比可知,尽管数值模拟中采用的落锤质量与实际落锤质量有一定差距,但相同的落锤动量所引起的梁中点挠度基本一致。因此,可以认为所建立的数值模型能够有效模拟相关试验工况。2.3 数值模拟结果
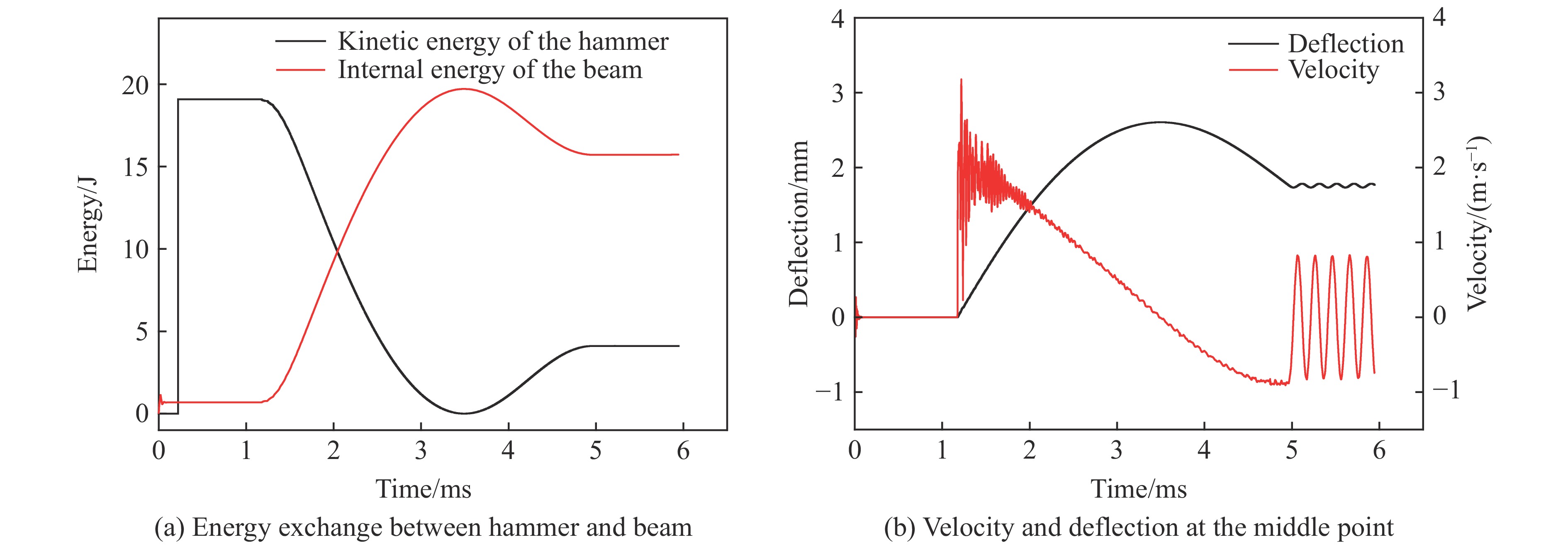
采用2.2节验证后的计算模型,对不同初始预应力的梁在不同落锤初速度撞击下的动态响应过程进行模拟,得到了一系列的结果。图11所示为预应力梁在落锤撞击下的响应过程。图11(a)从能量角度给出了梁的内能Ei和落锤的动能Ek之间的对应关系,t=0~0.3 ms为预应力加载阶段,预应力的作用使梁具有一定的初始内能Ei0;t=0.3 ms时赋予了落锤初速度;t=1.3 ms时落锤与梁之间发生撞击,落锤动能向梁内能、梁动能以及落锤内能转变,但从图11(a)中能量的数值对比可以看出,梁内能的增加量几乎等于落锤动能的减少量,即梁动能和落锤内能的增加量较小;t=3.5 ms时,落锤动能为零,梁内能达到最大;t=3.5~4.9 ms,梁内能向落锤动能转变,此过程发生了梁与落锤的同步回弹;t=4.9 ms之后,梁变形结束,内能维持不变,落锤以一定速度回弹离开梁。
图11(b)从梁中点的运动过程给出了梁的响应过程,与图11(a)所反映的落锤与梁的相互作用过程一致,主要分为3个阶段。第1个阶段为梁的正向运动和变形阶段,在t=1.3 ms时梁受到落锤撞击,突然获得一定的速度,此后这个速度不断减小,直到t=3.5 ms时,速度为零,位移达到最大。第2阶段为回弹阶段,t=3.5~4.9 ms,梁速度反向,位移逐渐减小,恢复一定的变形。第3阶段为周期振动阶段,t=4.9 ms之后,梁在平衡位置周期振动。
图12给出了不同预应力和不同落锤动量条件下,梁中点剩余挠度W的统计结果。由图12可知,W随着落锤动量的增加而增大;在落锤动量一致时,压预应力(
σα <0)状态下的梁,其剩余挠度W比无预应力时更大,且σα 的绝对值越大,W越大;而在拉预应力(σα >0)状态下,W与预应力σα 之间未发现较一致的变化规律。3. 结果分析与讨论
对于重物撞击固支梁的问题,Jones[10]将刚性理想塑性梁的变形简化成3个塑性铰的形式,通过能量守恒得到梁中点的剩余挠度:
W=mv2016M0L (3) 式中:m为落锤质量,
L 为梁的跨长,M0为梁的极限弯矩。对于矩形截面梁,M0的表达式为:M0=14bh2σ0 (4) 式中:σ0为材料的准静态屈服应力,σ0=337 MPa。尽管由于简化,式(3)计算得到的梁中点剩余挠度会偏大,但是该式所代表的物理含义是值得借鉴的。由式(3)可知,落锤动能
mv20/2 越大,梁的极限弯矩M0越小,梁的跨长L越大,梁的最终挠度W就会越大。如果将受到预应力作用后的梁仍视为刚性理想塑性,则也可采用式(3)进行分析,只是梁的初始尺寸参数(b、h、L)会由于受到预应力的作用而发生改变。梁在受预应力作用时仍为弹性,则预应力的作用使式(3)变为:
Wα=mv2016M0(1−μεα)3L(1+εα) (5) 式中:
Wα 为预应力状态下梁中点的剩余挠度,εα 为梁长度方向的预应力σα 引起的应变。由式(5)可知,当预应力为拉应力时,
εα >0,Wα >W,可以理解为拉预应力使梁的长度增大,截面积变小,实际极限弯矩减小,在相同的落锤动能作用下,挠度会增大;当预应力为压应力时,εα <0,Wα <W,可以理解为压预应力使梁的长度减小,截面积变大,实际极限弯矩增大,在相同的落锤动能作用下,挠度会减小。然而,由图12和表2可知,在拉压预应力作用下,梁中点剩余挠度的改变并非与式(5)所预测的一致。下面从能量的角度对图12和表2中的结果进行分析。金属梁在落锤撞击下发生变形的过程,实际上是一个能量吸收的过程,金属梁吸收的能量以塑性变形能的形式留在梁内。在梁的变形模式一致的前提下,梁吸收的能量越多,塑性变形能就越大,对应的特征变形量也会越大。图13给出了不同外加动能的情况下,梁吸收能量的情况。图13中,外加动能Ek0为落锤的初始动能:
Ek0=12mv20 (6) 梁以完全弹性方式可以吸收的最大应变能Ee为:
Ee=σ202EbhL (7) 能量比Ek0/Ee表示外加动能的相对大小。如果忽略落锤因自身变形而损耗的能量,那么可以用落锤撞击梁前后的动能变化量(Ek0−Ekl)来表示梁吸收的能量,能量吸收率(Ek0−Ekl)/Ek0表示梁吸收能量的相对大小。为了便于分析,图13中还引入预应力因数
α :σα=ασ0 (8) 式中:α满足−1≤α≤1,拉应力状态下α为正,压应力状态下α为负。
由图13可知,能量比越大,梁的能量吸收率越高,在能量比Ek0/Ee>1.5时,梁的能量吸收率达到了90%以上。预应力的存在(α≠0)会使梁的能量吸收率产生一些差别,在小能量比(Ek0/Ee<1)时,这一差别较为明显,压预应力状态下的能量吸收率相对较大,拉预应力状态下的能量吸收率相对较小;而在较大能量比(Ek0/Ee>2)时,这一差别较细微,可以认为几乎不变。
预应力使梁储存有初始内能:
Ei0=∫σ2α2EdV=α2Ee (9) 式中:V为梁的体积。
初始内能Ei0不会随着变形的发生而消失,这一部分能量与来自外加动能Ek0一起,共同构成了梁总变形能Et的来源。在能量吸收率一致的情况下,拉压预应力贡献的Ei0都会使梁的总变形能Et变大,见图14。对于压预应力状态(α<0),增大的总变形能Et分布在缩小了的长度L内,必然会导致更大的W,且α的绝对值越大,Et的增量越大,W越大。这解释了压预应力下挠度增大的现象。对于拉预应力状态(α>0),增大的总变形能Et分布在伸长了的长度L内,则不一定会导致W的增大。这在一定程度上解释了拉预应力下挠度变化不规律的现象。
综合对式(5)和从能量角度的分析可以看出:压预应力能够增大梁的极限弯矩,但在冲击载荷下,一旦梁产生塑性变形,预应力储存在梁构件内部的能量反而会增大其塑性变形量;拉预应力能够减小梁构件的极限弯矩,但其对梁构件在冲击载荷下发生塑性变形的影响则较复杂,需要更深入的分析。
4. 结 论
采用落锤加载试验和数值模拟两种方法,对处于轴向预应力下的金属梁在落锤冲击加载下的响应进行了研究。两种方法均能有效准确地控制预应力和冲击载荷的加载,且在落锤与梁的相互作用过程和梁的响应结果方面得到了较一致的结果。通过对梁的剩余挠度进行对比发现,压预应力状态下的梁受冲击载荷作用所产生的中点挠度会比无预应力时更大,且预应力的幅值越大,挠度的增大越显著;而拉预应力状态下的梁,挠度的变化量与预应力之间则没有较一致的规律。从能量角度对这一现象进行了分析和讨论,发现外加动能的能量比越大,梁的能量吸收率就越高,预应力的存在会对能量吸收率产生一定影响,表现为:小能量比时,压预应力下的能量吸收率较高,拉预应力下的能量吸收率较低;大能量比时,预应力对能量吸收率几乎无影响。另外,预应力所引入的初始内能也是梁塑性变形能的来源。压预应力下增大了的塑性变形能分布在缩小了的梁长度内,必然会导致更大的挠度;而拉预应力的各种变化则更复杂,相关机理需要更深入的研究。
-
表 1 落锤试验的工况
Table 1. Conditions of drop-weight tests
工况 H/cm ΔU/mV 1 50 0 2 100 0 3 150 0 4 200 0 5 100 −310 6 100 −410 7 100 350 8 100 450 表 2 不同试验工况下梁中点的剩余挠度
Table 2. Residual deflections at the middle points of the beams under different test conditions
工况 H/cm v0/(m·s−1) ΔU/mV σα/MPa W/mm 1 50 3.13 0 0 5.06 2 100 4.43 0 0 9.71 3 150 5.42 0 0 12.66 4 200 6.26 0 0 16.96 5 100 4.43 −310 −44 9.78 6 100 4.43 −410 −58 10.48 7 100 4.43 350 36 9.70 8 100 4.43 450 63 8.90 表 3 材料的基本参数
Table 3. Basic parameters for selected materials
材料 ρ0/(kg·m−3) E/GPa µ As/MPa Bs/MPa ns C ms 钢 7.896×103 211 0.29 350 275 0.36 0.022 1.00 铝 2.77×103 73 0.33 337 343 0.41 0.01 1.00 -
[1] MENKES S B, OPAT H J. Broken beams: tearing and shear failures in explosively loaded clamped beams [J]. Experimental Mechanics, 1973, 13(11): 480–486. DOI: 10.1007/BF02322734. [2] JONES N. Plastic failure of ductile beams loaded dynamically [J]. Journal of Engineering for Industry, 1976, 98(1): 131–136. DOI: 10.1115/1.3438805. [3] LIU J H, JONES N. Experimental investigation of clamped beams struck transversely by a mass [J]. International Journal of Impact Engineering, 1987, 6(4): 303–335. DOI: 10.1016/0734-743X(87)90097-2. [4] YAKOV B H. Failure of an axially compressed beam with uncertain initial deflection of bounded strain energy [J]. International Journal of Engineering Science, 1993, 31(7): 989–1001. DOI: 10.1016/0020-7225(93)90107-6. [5] ORYNYAK I V, KRASOWSKY A J. The modelling of elastic response of a three-point bend specimen under impact loading [J]. Engineering Fracture Mechanics, 1998, 60(5−6): 563–575. DOI: 10.1016/S0013-7944(98)00034-4. [6] XING Y F, QIAO Y S, ZHU D C, et al. Elastic impact on finite Timoshenko beam [J]. Acta Mechanica Sinica, 2002, 18(3): 252–263. DOI: 10.1007/BF02487953. [7] KURT M, CHEN H, LEE Y S, et al. Nonlinear system identification of the dynamics of a vibro-impact beam: numerical results [J]. Archive of Applied Mechanics, 2012, 82(10−11): 1461–1479. DOI: 10.1007/s00419-012-0678-5. [8] IVANOV L D. Change of steel’s yield stress over ship’s service life and its effect on the first yield hull girder bending moment [J]. Transactions of the Royal Institution of Naval Architects Part A: International Journal of Maritime Engineering, 2013, 155(3): 153–159. [9] CHENG B, CAO X E, YE X H, et al. Stringer longitudinal bending-induced fatigue failure of stringer-to-floor beam welded connections in orthotropic steel railway bridge decks [J]. Journal of Bridge Engineering, 2019, 24(6): 04019055. DOI: 10.1061/(ASCE)BE.1943-5592.0001428. [10] JONES N. Structural impact [M]. 2nd ed. New York: Cambridge University Press, 2012: 86−102. 期刊类型引用(1)
1. 苗春贺,徐松林,马昊,袁良柱,陆建华,王鹏飞. 递进式凸轮加载的中等应变率实验技术. 爆炸与冲击. 2023(03): 128-137 .  本站查看
本站查看其他类型引用(0)
-









 下载:
下载:
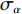



















































 下载:
下载: