An improved visco-hyperelastic constitutive behaviour of NEPE propellant at low and high strain rates
-
摘要: 为研究低高应变率条件下NEPE推进剂的力学特性,通过电子万能试验机和分离式霍普金森杆装置,对NEPE推进剂进行了准静态和冲击实验,得到了不同应变率下(1.667×10−4~4 500 s−1)的应力-应变曲线。实验结果表明NEPE推进剂具有明显的非线性弹性和应变率敏感性,随着应变率的增加,材料的强度、屈服应力和弹性模量显著增加,与低应变率相比,高应变率条件下材料的应变率敏感性更高。在高速冲击下材料内部瞬间产生大量热量无法及时散发出去,使得材料内部温度升高,导致材料出现软化效应,力学性能降低。本文建立了一个非线性黏超弹本构模型,其中采用Rivlin应变能函数来描述稳态超弹响应部分,采用积分型本构模型来描述材料的动态黏弹性响应部分,考虑到松弛时间具有应变率相关性,本文采用了一个率相关松弛函数来替代传统的Prony级数形式。使用极慢速压缩实验数据对本构模型中的超弹部分进行拟合获得超弹参数,然后用准静态和动态实验数据对本构模型进行拟合得出其他参数。不同应变率下的预测曲线与实验曲线具有较好的重合度,证明了该模型可以很好地描述低高应变率下NEPE推进剂的力学特性。Abstract: In order to study the mechanical properties of NEPE propellant at low and high strain rates, the quasi-static and impact eperiments of NEPE propellant were carried out by the electronic universal testing machine and split Hopkinson bar, and the stress-strain curves of NEPE propellant under different strain rates (1.667×10−4−4 500 s−1) were obtained by processing the experiment data. By analyzing the stress-strain curve of low and high strain rates experiment, it can be found that NEPE propellant has obvious nonlinear elasticity and strain rate sensitivity. With the increase of strain rate, the strength, yield stress and elastic modulus of the material increase significantly. Compared with low strain rate, the strain rate sensitivity of the material at high strain rate is higher. Under the high speed impact, a large amount of heat is generated inside the material and cannot be released in time, which makes the internal temperature of the material rise, leading to softening effect of the material and reduction of mechanical properties. In this paper, a nonlinear visco-hyperelastic constitutive model is established to describe the mechanical properties of NEPE propellant at low and high strain rates, in which the Rivlin strain energy function is used to describe the static hyperelastic behaviour, and an integral constitutive model is used to characterize the dynamic response of the material. Considering that the relaxation time has strain rate correlation, a rate-dependent relaxation function is adopted in this paper to replace the traditional Prony series. The hyperelastic parameters were obtained by fitting the hyperelastic part of the constitutive model with extremely slow compression experiment data, and then the other parameters were obtained by fitting the constitutive model with quasi-static and dynamic experiment data. It was proved that the model could well describe the mechanical properties of NEPE propellant at low and high strain rates by the good coincidence degree between the prediction curve and the experiment curve under different strain rates.
-
Key words:
- NEPE propellant /
- low and high strain rates /
- constitutive model /
- relaxation function /
- nonlinear
-
固体推进剂在生产、运输、存储和发射过程中常受高应变率载荷作用,因此研究不同应变率对推进剂力学特性的影响在推进剂装药结构完整性分析中具有重要意义。硝酸酯增塑的聚醚聚氨酯推进剂(NEPE推进剂)是一种由黏弹性基体和铝粉组成的高填充固体黏弹性复合材料[1]。作为一种新型推进剂,NEPE推进剂综合了双基推进剂和复合推进剂的优点,具有高能量的特点和优异的力学性能,近年来得到了广泛的应用。NEPE推进剂具有复杂的非线性黏弹性力学性能,因此必须要考虑有限变形下的加载历史、温度和应变率对材料力学性能的影响。
目前,在宏观研究领域常采用唯象学的方法来建立固体推进剂的本构模型。由于固体推进剂具有的超弹性和黏弹性力学特性,其本构模型通常包括多项式形式、Mooney-Rivlin模型[2-3]、Ogden模型[4]、Yeoh模型[5]的超弹部分,以及Maxwell模型、Duncan模型[6]、Schapery模型[7]、朱王唐模型[8]的黏弹部分。常新龙等[9]在Burke模型的基础上,考虑了温度和应变率效应,采用Mooney-Rivlin应变能函数来描述稳态超弹响应部分,采用一个Maxwell单元来描述动态黏弹响应部分,同时引入温度效应关系式,建立了一个黏超弹本构模型,该模型可以较好地描述HTPB推进剂在低中应变率下的压缩力学响应,但在应变率较高时误差会随着应变增大。杨龙等[10]通过低中应变率实验发现1 s−1为应力和应变率双线性关系的转折点,他在Mohotti模型的基础上,将应变能函数与应变率对数线性联系起来,再结合应变依赖性建立了应变率相关的超弹本构模型。Wang等[11]发现随着温度的不断降低和应变速率的增大,HTPB推进剂的应力-应变曲线和损伤特征更加复杂;考虑到温度对材料超弹性、黏弹性和损伤的影响,他基于HTPB推进剂的压缩行为和非线性黏弹性本构理论,提出了一种新的含损伤热黏超弹本构模型。Guo等[12]基于Lubliner等提出的变形梯度的乘法分解和自由能的加法分裂,采用Attard应变能函数,得到了有限变形下聚脲的非线性黏超弹本构模型,能够较好地描述宽泛应变率下聚脲的拉伸和压缩力学行为。
为了研究推进剂在受载时导致宏观变化的内在因素,近年来,许多学者使用细观方法,根据推进剂外部的受载情况,基于计算机颗粒堆积算法,构建出满足所需条件的细观数值模型,通过数值模拟得到细观结构对宏观力学行为的影响。韩龙等[13]将宏观研究方法与细观研究方法结合起来,基于黏弹性脱湿准则将获得的细观数值代入到宏观本构模型中,提出了一个考虑NEPE推进剂细观颗粒脱湿因素的非线性黏弹性本构模型。
分离式Hopkinson杆(SHB)技术是目前对复合材料进行高应变率测试的主要方法。Davies[14]和Kolsky[15]首先提出了用两个Hopkinson杆夹住试样来测量材料的动态响应的方法,后来经过众多学者的改进,分离式Hopkinson杆技术已经可以用来对材料进行压缩、拉伸和扭转等多种加载形式的测试。
本文通过使用分离式Hopkinson压杆(SHPB)和电子万能试验机,对NEPE推进剂进行高低应变率单轴压缩实验,在Yang等[16]提出的NLHV模型的基础上,把松弛时间与应变率联系起来,构建一个非线性黏超弹本构模型。
1. 本构模型
考虑到NEPE推进剂不仅具有卸载后应变可以基本恢复的超弹特性,同时还具有率相关性、加载历史相关性和松弛等黏弹特性,本文在Yang等[16]提出的NLHV模型基础上,采用Rivlin应变能函数,并根据松弛时间和应变率之间的关系,将松弛时间替换成应变率相关的函数,建立了一个宽泛应变率非线性黏超弹本构模型,模型表达式如下:
σij=σeij(t)+σvij(t) (1) 式中:
σeij 为Rivlin超弹性应力张量,σvij 为黏弹性柯西应力张量。1.1 超弹性本构方程
在黏超弹本构模型中,需要一个超弹公式来表示低应变率过程。根据Sligtenhorst等[17]和Mooney[2]的研究,Rivlin超弹公式在静态实验中对多聚物材料拟合较好,因此本文选用Rivlin超弹公式[18]作为本构模型中的超弹项。在低应变率过程中,不考虑应变率对本构模型的影响,对Rivlin超弹模型中的应力应变关系进行推导。
当一个质点从
t=0 时的参考位置Xi 到t时刻的即时位置xi ,经历了位移ui 后的位置xi 可以表示为xi=Xi+ui 。此时与这个新位置相关的变形梯度由Fij=∂xi/∂xj 表示,变形由左柯西-格林张量Bij=FikFkj 来描述,应变不变量由I1=Bkk ,I2=(B2kk−BijBji)/2 和I3=|Bij| 给出。Rivlin[3]证明超弹性材料中任意点处的柯西超弹性应力σeij 和应变能函数W之间的关系为σeij=2I1/23[(∂W∂I1+I1∂W∂I2)Bij−BikBkj∂W∂I2+I3∂W∂I3δij]−pδij (2) 式中:
p 为考虑了位置与应力状态的相关性以保证平衡的任意压力,δij 为单位张量。假设NEPE推进剂为有限变形下的不可压缩材料,则W=N∑a,b,c=0Aabc(I1−3)a(I2−3)b(I3−1)c (3) 式中:Aabc为系数。
式(2)常写成简化形式
σeij=α1Bij+α2BikBkj+α0δij−pδij (4) 其中
α1=2I1/23(∂W∂I1+I1∂W∂I2)α2=−2I1/23∂W∂I2α0=2I1/23∂W∂I3 (5) 对于单轴加载条件下的不可压缩材料,第三应变不变量
I3=1 ,伸长比λ11=λ ,λ22=λ33=λ−1/2 (λ为压缩方向上的伸长比),对应的变形梯度Fij 和左柯西-格林变形张量Bij 分别为(Fij)=[λ000λ−1/2000λ−1/2],(Bij)=[λ2000λ−1000λ−1] (6) 根据Rivlin的假设,应变能函数中
N=1 ,应变能函数可以写成如下形式W=A10(I1−3)+A01(I2−3)+A11(I1−3)(I2−3) (7) 将式(4)和式(6)代入到式(9)中,可以得出单轴加载条件下的Rivlin超弹性模型如下:
σe11=A10(2λ2−2λ−1)+A01(2λ−2λ−2)+A11(6λ−6λ−2)(λ−2+λ−1−λ−1) (8) 1.2 黏弹性本构模型
由于NEPE推进剂具有率相关性和加载历史相关性,在准静态和动态加载条件下都表现出明显的非线性黏弹特性,因此采用Truesdell等[19]证明的非线性黏弹性本构方程:
σvij(t)=−pvδij+FiktΩτ→−∞{Ckl(τ)}Fjl (9) 式中:
pv 为静水压力;δij 为单位张量;Cij 为右柯西-格林变形张量,可以表示为Cij=FkiFkj (10) 其相关的不变量为
I1=CkkI2=12(C2kk−CijCji)I3=|Cij| (11) Ω 是一个包含了黏弹性效应的张量泛函,反映了加载历史对应力的影响,可以用如下关系式表示tΩτ→−∞{Cij(τ)}=∫t−∞Φ(I1,I2)G(t−τ)˙Eij(τ)dτ (12) 其中
Φ(τ)=M1+M2[I2(τ)−3] (13) 式中:M1和M2为拟合系数。
松弛函数
G(t) 可以表示成如下的Prony级数形式G(t−τ)=N∑a=1αaexp(−t−τθa) (14) 式中:αa为Prony的拟合系数;θa为松弛时间;
˙E 为应变率,是格林应变张量的时间导数,可以表示为˙Eij(t)=12[˙Fki(t)Fkj(t)+Fki(t)˙Fkj(t)] (15) 利用式(6)的单轴载荷简化和不可压缩性假设,可以将单轴加载的一维本构NLHV模型表示为
σ11(t)=σe11(t)+12λ−1∫t0λ(τ)−2{M1+M2[λ(τ)−2+2λ(τ)−3]}[N∑a=1αaexp(−t−τθa)]˙λ(τ)dτ+λ2∫t0λ(τ){M1+M2[λ(τ)−2+2λ(τ)−3]}[N∑a=1αaexp(−t−τθa)]˙λ(τ)dτ (16) 目前,不同应变率范围内的加载过程通常使用不同阶数的Prony级数来描述。从微观结构的角度看,弹性体在变形过程中,由于化学键的可逆断裂和交换发生了分子间滑移,从而产生了松弛现象。从宏观的角度来看,不同的应变水平下都存在一个整体的松弛时间(θ),Khajehsaeid等[20]提出该松弛时间最终取决于变形率,通过如下关系将材料的整体松弛时间和变形速率联系起来:
θ(˙ˉε)=θref(˙ˉεref˙ˉε)η (17) 式中:
˙ˉε 为等效应变率;˙ˉεref 和θref 分别为参考路径中的等效应变率和等效松弛时间;η 是一个非负材料参数,当η=0 时松弛时间是一个定值。在有限变形中,等效应变率可以由右柯西-格林变形张量定义:
˙ˉε=‖˙C‖=√˙Cij˙Cij (18) 参考路径中的等效松弛时间可以定义为如下形式:
θref=φref‖˙C‖−ηref (19) 式中:
φref 为一个材料参数。将式(18)和式(19)代入式(17)可得
θ=θ(‖˙C‖)=θref(‖˙C‖ref‖˙C‖)η=φref(1‖˙C‖)η (20) 对于恒应变率的单轴加载情况,
‖˙C‖ 具有如下形式:‖˙C‖=˙ε11√4λ2+2λ−4 (21) 式中:
˙ε11 为应变率张量的分量。利用定义的材料松弛时间函数取代松弛函数
G(t) 的Prony级数形式,可以得到松弛函数G(t−τ)=exp[−t−τφref(˙ε√4λ(τ)2+2λ(τ)−4)η] (22) 由此可以将单轴加载的一维本构NLHV模型转化为如下形式
σ11(t)=σe11(t)+12λ−1∫t0λ(τ)−2{M1+M2[λ(τ)−2+2λ(τ)−3]}[exp(−t−τφref(˙ε√4λ(τ)2+2λ(τ)−4)η)]˙λ(τ)dτ+λ2∫t0λ(τ){M1+M2[λ(τ)−2+2λ(τ)−3]}[exp(−t−τφref(˙ε√4λ(τ)2+2λ(τ)−4)η)]˙λ(τ)dτ (23) 2. 实 验
2.1 实验材料
由于NEPE推进剂是一种多组分的复合材料,不同批次的NEPE推进剂的力学性能也存在偏差,因此本文选择同一批次的NEPE推进剂来进行实验,并将浇筑成型的管状药切割为两种尺寸。准静态实验使用公称尺寸为
∅ 10 mm×10 mm的试件,动态实验使用公称尺寸为∅ 10 mm×5 mm的试件。2.2 低应变率压缩实验
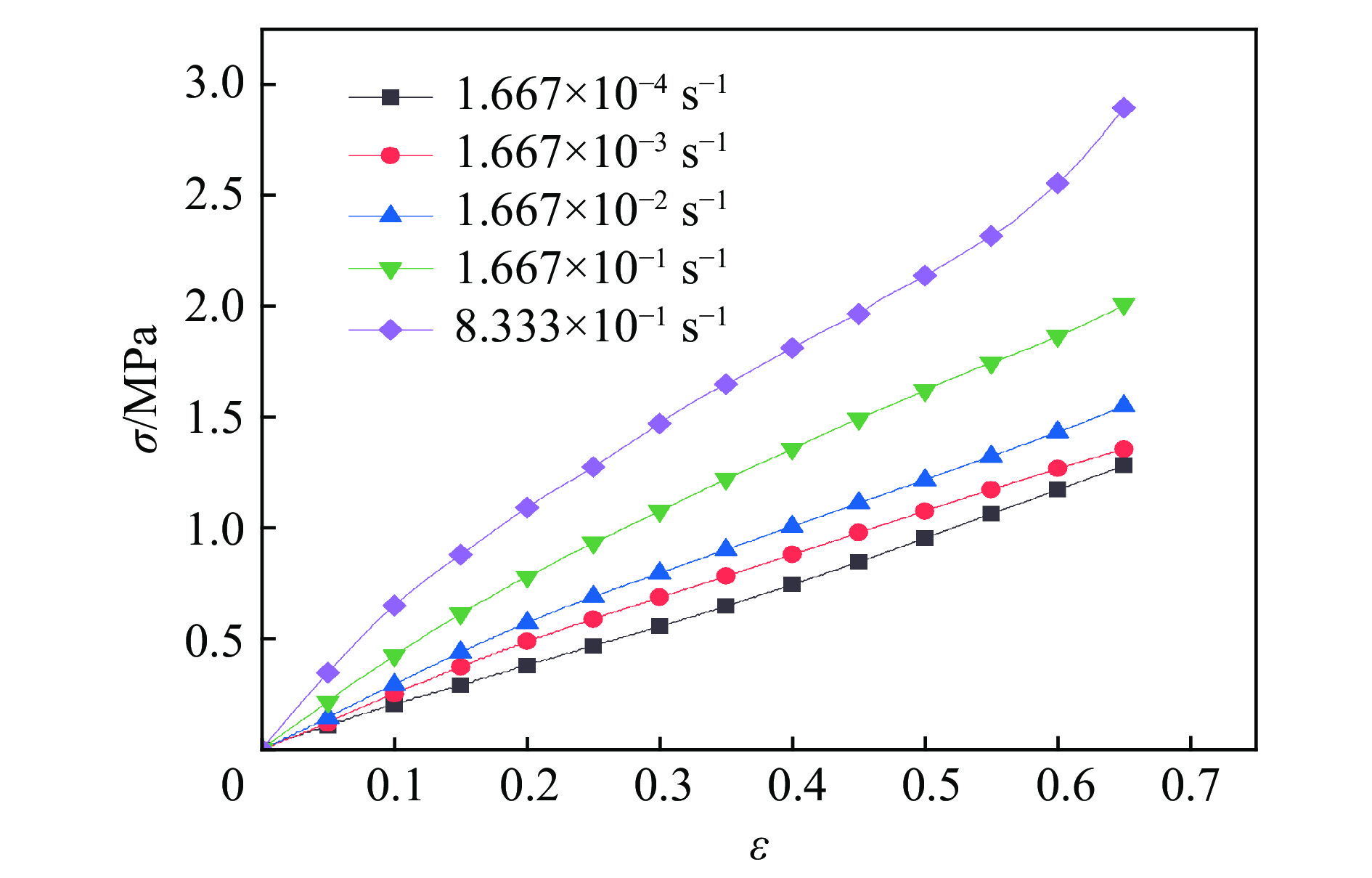
低应变率单轴压缩实验在电子万能试验机上进行,实验前将NPEN推进剂放在保温箱中存放24 h来消除残余应力,分别对试件进行5种速率的压缩实验:0.1、1、10、100和500 mm/min;对应的应变率分别为:1.667×10−4、1.667×10−3、1.667×10−2、1.667×10−1和8.333×10−1 s−1。同一工况下至少进行5组实验以保证实验数据的有效性,通过压杆上连接的应力传感器得到实验过程中材料实时轴向应力
Φ ,将数据采集系统上获得的有效标距上的位移、时间和轴向应力通过下式转化为应力应变曲线:εeng=v⋅tl0,σtrue=ΦA0(1+εeng) (24) 式中:
l0 为圆柱形试件的初始长度,A0 为NEPE推进剂试件初始横截面积,v 为实验机压缩速率,εeng 和σtrue 分别为材料的工程应变和真实应力。2.3 高应变率压缩实验
2.3.1 试件尺寸设计
在高应变率动态压缩实验中,根据材料的性质和实验要求,试件需要设计成不同的形状和尺寸,通常采用短圆柱形试件。为了保证实验数据的可靠性,试件内必须满足一维应力假设,即忽略横向惯性效应,只考虑试件的轴向变形。Forrestal等[21]通过实验发现,当软材料的试件较厚时,试件内部不满足应力均匀性假设。Davies等[22]采用能量法分析了试件内应力波的横向惯性效应,提出了当长径比满足:
Ls/Ds=√3μ/2 (Ls和Ds为试件的长度和直径,μ为泊松比)时,可以忽略试件内的横向惯性效应,使试件内满足一维应力假设。考虑到材料的不可压缩性,试件尺寸的设计应尽量满足长径比为0.6左右,同时还要保证试件变形后直径不得大于杆直径,因此本实验采用尺寸为∅ 10 mm×5 mm的圆柱形NEPE试件,长径比为0.5。实验前将NPEN推进剂放在保温箱中存放24 h来消除残余应力。2.3.2 实验方法及过程
高应变率动态压缩实验在分离式Hopkinson实验台上进行。由于NEPE推进剂较软,波阻抗较低,为了获得较为准确的透射信号,选择LC4铝制作了本实验的子弹、入射杆、透射杆和吸收杆。在实验过程中,为了减少试验杆与试件之间摩擦带来的影响,在试件端部涂上适量的润滑脂。为了保证实验数据的可靠性,试件内必须满足恒应变率变形和应力均匀条件,使用脉冲整形技术,将厚纸片置于入射杆端部,提升入射脉冲上升沿时间,达到恒应变率变形。在室温下分别进行5种不同应变率下的实验,每个应变率下重复5组实验以获得较为准确的数据。
使用二波法对数据采集系统获得的信号进行处理,获得试件的应力应变曲线和应变率:
˙εtrue(t)=2c0l0εR(t) (25) εtrue(t)=2c0l0∫t0εR(t)dt (26) σtrue(t)=E0S0SsεT(t) (27) 式中:
c0 为杆中的弹性波波速;l0为试件的初始长度;E0 为杆的杨氏模量;S0 为杆的横截面积;Ss 为试件的初始横截面积,εR 和εT 分别为反射应变和透射应变。2.4 实验结果分析
2.4.1 高应变率实验可靠性分析
为保证实验结果具有有效性和可信性,需要对每次的实验数据进行应力均匀性和恒应变率加载校核。图1为0.12 MPa炮压下的SHPB实验波形。图1(a)为应变片测得的原始波形,由式(25)可知试件的应变率与反射波强度成正比,图中可以看出反射波呈平台状,因此满足恒应变率加载要求。图1(b)为实验透射波和计算透射波的对比波形,通过将入射波和反射波进行平移相加获得计算透射波,对比发现实验透射波和计算透射波基本重合,说明实验满足应力均匀性假设。
2.4.2 应力应变曲线分析
通过对万能试验机上采集到的数据进行处理,可以获得室温下试件不同应变率的应力应变曲线,如图2所示。由图2可知,随着应变率的增加,NEPE推进剂的弹性模量明显增大,硬度增加;在曲线中并未找到明显的屈服点;在应变率较低时,曲线接近于直线,当应变率达到8.333×10−1 s−1时,表现出明显的非线性且存在应力强化段。
对满足恒应变率变形和应力均匀性的高应变率实验数据进行处理,可以得到高应变率下试件的应力应变曲线,如图3所示。通过观察可以发现,在加载过程中,试件经历了初始弹性上升段、屈服阶段和屈服后应力下降阶段,应力达到峰值后的下降段可能是由于子弹长度有限,产生的应力波作用时间有限,导致了试件内应力被卸载,并不一定是材料结构破坏造成的;材料的性质具有明显的率相关性,随着应变率的增加,材料的强度、屈服应力和弹性模量显著增加,与低应变率相比,材料的应变率敏感性更高;由于高速冲击下材料内部瞬间产生大量热量无法及时散发出去,使得材料内部温度升高,导致了材料出现软化效应,力学性能降低,同时在高速冲击下材料内部结构遭到破坏,颗粒发生了破碎,因此材料在屈服后出现了短暂的应变软化段,然后出现了应力下降段。
准静态实验后获得的恢复试件如图4和图5所示,通过观察试件可以发现,对试件进行70%应变的压缩后卸载,卸载后与初始长度相差0.81 mm,静置24 h后试件几乎可以恢复初始长度,与初始长度相差0.47 mm,基体未产生裂痕和发生断裂;当应变提升至95%时,卸载后与初始长度相差2.1 mm,静置24 h试件长度与初始长度相差1.3 mm,基体仍未产生裂痕和发生断裂,试件呈现出鼓状,说明材料较软且具有较好的弹性和延展性。在准静态压缩实验中,试件内部颗粒与基体的黏合处会产生应力集中,颗粒脱湿形成横向的孔洞并产生微裂纹,随着应变增大,微裂纹增多,但并未进一步发展成断裂破坏。高应变率实验获得的试件则不同,如图6所示,试件主要分为3种形态,当应变率较低时呈鼓状,此时颗粒受到冲击发生破碎,试件侧面出现裂痕,但试件基体还未发生完全断裂;当应变率升高时,此时裂痕由试件边缘向内扩展,造成基体内部发生破坏,试件断裂;随着应变率继续升高,试件被完全打散,颗粒破碎程度增加,基体被压成片状,试件内部被完全破坏,产生了大量裂痕和空隙,基体发生解体现象。在冲击实验中,由于颗粒的模量远大于基体的模量,大部分的冲击载荷会作用在颗粒上,导致部分颗粒还未脱湿就发生破碎,微裂纹从颗粒内部产生并向基体延伸,推进剂发生穿晶断裂,随着应变率增加,颗粒破碎程度增大,基体解体现象更加明显。
3. 模型拟合与预测
采用最小二乘法对准静态实验中速率为0.1 mm/min的数据与Rivlin超弹模型进行拟合,获得的Rivlin超弹模型参数和相关系数见表1。图7是应变率为1.667×10−4 s−1时的实验曲线和拟合曲线,可以看出拟合曲线与实验数据基本重合,超弹参数能较好地反映材料的非线性弹性响应。
表 1 Rivlin超弹模型参数Table 1. Rivlin fitted parametersA10 A01 A11 R 0.814 −0.442 0.079 0.999 对于恒应变率变形,伸长率为关于时间的函数
λ=t˙ε+1 (28) 松弛函数
G(t) 可以化为G(λ)=exp[−λ−ξφref˙ε(˙ε√4ξ2+2ξ−4)η] (29) 当进行宽泛应变率拟合时,式(23)可化为
σ11(λ)=σe11(λ)+12λ−1∫λ1ξ−2{M1+M2[ξ−2+2ξ−3]}{exp[−λ−ξφref˙ε(˙ε√4ξ2+2ξ−4)η]}dξ+λ2∫λ1ξ{M1+M2[ξ−2+2ξ−3]}{exp[−λ−ξφref˙ε(˙ε√4ξ2+2ξ−4)η]}dξ (30) 分别对准静态压缩实验和分离式Hopkinson杆实验获得的数据进行处理,获得应力应变曲线,转化成应力和伸长比代入式(30),采用最小二乘法进行拟合,获得黏弹参数如表2所示。图8是包含了准静态压缩实验和分离式Hopkinson杆实验的实验曲线和拟合曲线。由图中可以看出,在低/高应变率范围内,拟合曲线与实验数据具有较好的重合度,能够较为准确地描述低/高应变率下NEPE推进剂的应力应变关系。
表 2 黏弹参数Table 2. Viscoelastic parametersM1 M1 φref η R 25.9 0.1 0.021 0.648 0.997 利用本文建立的黏超弹本构模型对单轴压缩速率为500 mm/min的准静态实验和应变率为4 500 s−1的动态压缩实验进行预测,预测曲线和实验曲线如图9所示。从图9中可以看出,实验曲线与预测曲线具有较好的一致性,可以较为准确地描述NEPE推进剂在低高应变率下的力学响应;在高应变率情况下,当应变大于0.45时实验曲线逐渐偏离预测曲线,出现应变软化现象,这是由于在冲击实验中响应时间十分短暂,实验过程中的产热速率远大于热扩散速率,此时材料内部的温度急剧升高造成了材料的软化。本文没有考虑在高速冲击下材料内部温升对材料的软化效应,因此造成了高应变率下当应变大于0.45时,预测曲线高于实验曲线的现象。
4. 结 论
本文建立的黏超弹本构方程由超弹部分和黏弹部分组成,首先用极慢速压缩实验数据拟合出超弹参数,然后使用准静态和动态实验结果拟合出其他参数。通过预测曲线和实验曲线对比可以看出,本文提出的本构模型能够较为准确地描述NEPE推进剂在低应变率和高应变率下的力学行为。
考虑到松弛时间是具有率相关性的,本文采用了一个率相关松弛函数来替代传统的Prony级数形式。与Prony级数形式相比,该函数减少了材料黏弹参数的数量,同时说明了松弛时间与应变水平有关联。
与准静态实验相比,材料在冲击实验中具有更高的应变率敏感性。在高速冲击下材料内部瞬间产生大量热量无法及时散发出去,使得材料内部温度升高,导致了材料出现软化效应,力学性能降低,同时在高速冲击下部分颗粒还未脱湿就发生破碎,微裂纹从颗粒内部产生并向基体延伸,推进剂发生穿晶断裂,随着应变率增加颗粒破碎程度增大,基体解体现象更加明显,破坏形式通常表现为鼓形变形、试件断裂和完全破坏三种。
-
表 1 Rivlin超弹模型参数
Table 1. Rivlin fitted parameters
A10 A01 A11 R 0.814 −0.442 0.079 0.999 表 2 黏弹参数
Table 2. Viscoelastic parameters
M1 M1 φref η R 25.9 0.1 0.021 0.648 0.997 -
[1] LIU X, AO W, LIU H, et al. Aluminum agglomeration on burning surface of NEPE propellants at 3−5 MPa [J]. Propellants, Explosives, Pyrotechnics, 2017, 42(3): 260–268. DOI: 10.1002/prep.201600131. [2] MOONEY M. A theory of large elastic deformation [J]. Journal of Applied Physics, 1940, 11(9): 582–592. DOI: 10.1063/1.1712836. [3] RIVLIN R S. Large elastic deformations of isotropic materials: II: some uniqueness theorems for pure, homogeneous deformation [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1948, 240(822): 491–508. DOI: 10.1098/rsta.1948.0003. [4] OGDEN R W. Large deformation isotropic elasticity: on the correlation of theory and experiment for incompressible rubberlike solids [J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1972, 326(1567): 565–584. DOI: 10.1098/rspa.1972.0026. [5] YEOH O H. Some forms of the strain energy function for rubber [J]. Rubber Chemistry and Technology, 1993, 66(5): 754–771. DOI: 10.5254/1.3538343. [6] DUNCAN E J S, MARGETSON J. A nonlinear viscoelastic theory for solid rocket propellants based on a cumulative damage approach [J]. Propellants, Explosives, Pyrotechnics, 1998, 23(2): 94–104. DOI: 10.1002/(SICI)1521-4087(199804)23:2<94::AID-PREP94>3.0.CO;2-C. [7] HINTERHOELZL R M, SCHAPERY R A. FEM implementation of a three-dimensional viscoelastic constitutive model for particulate composites with damage growth [J]. Mechanics of Time-Dependent Materials, 2004, 8(1): 65–94. DOI: 10.1023/B:MTDM.0000027683.06097.76. [8] 朱兆祥, 徐大本, 王礼立. 环氧树脂在高应变率下的热粘弹性本构方程和时温等效性 [J]. 宁波大学学报, 1988(1): 58–67.ZHU Z X, XU D B, WANG L L. Thermoviscoelastic constitutive equation and time-temperature equivalence of epoxy resin at high strain rates [J]. Journal of Ningbo University, 1988(1): 58–67. [9] 常新龙, 赖建伟, 张晓军, 等. HTPB推进剂高应变率粘弹性本构模型研究 [J]. 推进技术, 2014, 35(1): 123–127. DOI: 10.13675/j.cnki.tjjs.2014.01.001.CHANG X L, LAI J W, ZHANG X J, et al. High strain-rate viscoelastic constitutive model for HTPB propellant [J]. Journal of Propulsion Technology, 2014, 35(1): 123–127. DOI: 10.13675/j.cnki.tjjs.2014.01.001. [10] 杨龙, 谢侃, 裴江峰, 等. HTPB推进剂拉伸力学行为的应变速率相关超弹本构模型 [J]. 推进技术, 2017, 38(3): 687–694. DOI: 10.13675/j.cnki.tjjs.2017.03.027.YANG L, XIE K, PEI J F, et al. A strain-rate-dependent hyperelastic constitutive model for tensile mechanical behaviour of HTPB propellant [J]. Journal of Propulsion Technology, 2017, 38(3): 687–694. DOI: 10.13675/j.cnki.tjjs.2017.03.027. [11] WANG Z J, QIANG H F, WANG T J, et al. A thermovisco-hyperelastic constitutive model of HTPB propellant with damage at intermediate strain rates [J]. Mechanics of Time-Dependent Materials, 2018, 22(3): 291–314. DOI: 10.1007/s11043-017-9357-9. [12] GUO H, GUO W G, AMIRKHIZI A V. Constitutive modeling of the tensile and compressive deformation behavior of polyurea over a wide range of strain rates [J]. Construction and Building Materials, 2017, 150: 851–859. DOI: 10.1016/j.conbuildmat.2017.06.055. [13] 韩龙, 许进升, 封涛, 等. 考虑细观脱湿损伤的NEPE推进剂粘弹性本构模型研究 [J]. 推进技术, 2017, 38(8): 1885–1892. DOI: 10.13675/j.cnki.tjjs.2017.08.027.HAN L, XU J S, FENG T, et al. Research on viscoelastic constitutive model for NEPE composite propellant with meso-mechanics damage due to particle dewetting [J]. Journal of Propulsion Technology, 2017, 38(8): 1885–1892. DOI: 10.13675/j.cnki.tjjs.2017.08.027. [14] DAVIES R M. A critical study of the Hopkinson pressure bar [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1948, 240(821): 375–457. DOI: 10.1098/rsta.1948.0001. [15] KOLSKY H. An investigation of the mechanical properties of materials at very high rates of loading [J]. Proceedings of the Physical Society. Section B, 1949, 62(11): 676. DOI: 10.1088/0370-1301/62/11/302. [16] YANG L M, SHIM V P W, LIM C T. A visco-hyperelastic approach to modelling the constitutive behaviour of rubber [J]. International Journal of Impact Engineering, 2000, 24(6−7): 545–560. DOI: 10.1016/S0734-743X(99)00044-5. [17] Van SLIGTENHORST C, CRONIN D S, BRODLAND G W. High strain rate compressive properties of bovine muscle tissue determined using a split Hopkinson bar apparatus [J]. Journal of Biomechanics, 2006, 39(10): 1852–1858. DOI: 10.1016/j.jbiomech.2005.05.015. [18] RIVLIN R S. Some topics in finite elasticity [C] // Proceedings of the First Symposium on Naval Structural Mechanics, 1960: 169–198. DOI: 10.1007/978-1-4612-2416-7_25. [19] TRUESDELL C, NOLL W. The non-linear field theories of mechanics [M]. Berlin: Springer, 1992. DOI: 10.1007/978-3-662-13183-1. [20] KHAJEHSAEID H, ARGHAVANI J, NAGHDABADI R, et al. A visco-hyperelastic constitutive model for rubber-like materials: a rate-dependent relaxation time scheme [J]. International Journal of Engineering Science, 2014, 79: 44–58. DOI: 10.1016/j.ijengsci.2014.03.001. [21] FORRESTAL M J, WRIGHT T W, CHEN W. The effect of radial inertia on brittle samples during the split Hopkinson pressure bar test [J]. International Journal of Impact Engineering, 2007, 34(3): 405–411. DOI: 10.1016/j.ijimpeng.2005.12.001. [22] DAVIES E D H, HUNTER S C. The dynamic compression testing of solids by the method of the split Hopkinson pressure bar [J]. Journal of the Mechanics and Physics of Solids, 1963, 11(3): 155–179. DOI: 10.1016/0022-5096(63)90050-4. 期刊类型引用(7)
1. 徐一航,李道奎,周仕明. 复合固体推进剂本构模型研究进展及发展趋势. 国防科技大学学报. 2025(01): 1-22 .  百度学术
百度学术2. 雷经发,沈俊杰,刘涛,王璐,赵晨霞. 宽温域下Talc/NR复合材料的力学性能及本构分析. 塑料. 2025(01): 52-57 .  百度学术
百度学术3. 刘俊,梁爽,刘向阳,郜婕. 基于分子链演化的NEPE推进剂基体超弹本构模型. 含能材料. 2024(05): 518-527 .  百度学术
百度学术4. 韩若寒,付小龙,蔚红建,石拉,刘瀚文. 复合固体推进剂细观力学性能模拟研究进展. 兵器装备工程学报. 2024(08): 290-300 .  百度学术
百度学术5. 申志彬,邓旷威,李海阳,张慧慧. 长期贮存发动机中HTPB推进剂老化特性及蠕变本构模型. 推进技术. 2024(09): 171-178 .  百度学术
百度学术6. 徐跃跃,张向荣,高家乐,刘战伟,苗飞超,刘攀,周霖. DNP基熔铸炸药的力学性能和失效准则. 兵工学报. 2024(09): 3114-3124 .  百度学术
百度学术7. 夏剑冬,徐滨,廖昕. 双基发射药黏弹特性与本构模型研究. 含能材料. 2023(11): 1124-1133 .  百度学术
百度学术其他类型引用(3)
-







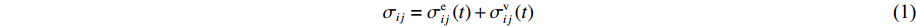

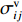


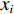














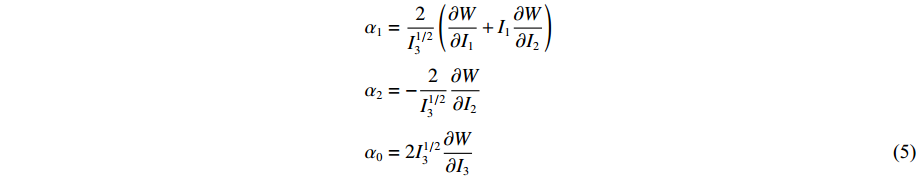
































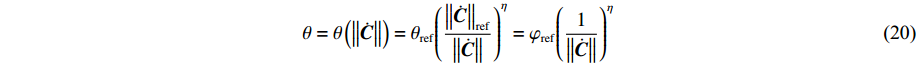





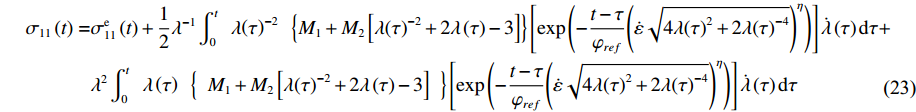












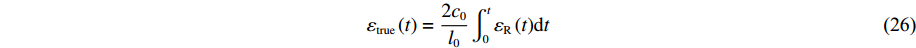








 下载:
下载:













 下载:
下载:








 百度学术
百度学术