Effect of loading rate on the modeⅡ dynamic fracture characteristics of 40Cr steel
-
摘要: 采用新型Ⅱ型动态断裂测试技术,对高强钢40Cr在高加载速率下的Ⅱ型动态断裂特性进行了测试研究。基于新设计的Ⅱ型动态断裂试样和分离式霍普金森压杆(split Hopkinson pressure bar, SHPB)技术,通过实验-数值方法确定了裂尖在加载过程中的应力强度因子曲线。采用应变片法确定了试样的起裂时间,最终得到40Cr的Ⅱ型动态断裂韧性值,并对其加载速率相关性和材料的失效机理进行了研究。结果表明,在1.08~5.53 TPa·m1/2/s的加载速率范围内,40Cr的Ⅱ型动态断裂韧性基本表现为与加载速率成正相关的变化趋势。通过对试样断口形貌的分析,确定了材料的失效模式及机理,发现随着加载速率的增加,存在拉伸型失效向绝热剪切型失效模式转变的现象。Abstract: 40Cr high strength steel is often used in aerospace and national defense fields due to its excellent mechanical properties. Therefore, the research on the mode Ⅱ dynamic fracture characteristics and failure mechanism of 40Cr high strength steel under high-speed impact has important scientific significance and engineering value. Experiments and numerical simulations were carried out to study the mode Ⅱ dynamic fracture properties of 40Cr material under high loading rates. Based on the newly designed specimen and the novel test technique for mode Ⅱ dynamic fracture, the experimental-numerical method was used to determine the dynamic stress intensity factor curve of the crack tip during the loading process. The crack initiation time of the specimen was obtained by the strain gauge pasted on the specimen, and the mode Ⅱ dynamic fracture toughness of 40Cr was finally determined. The effect of loading rate and the failure mechanism of the material were also studied. The results show that within the present loading rate range of this work (1.08−5.53 TPa·m1/2/s), the mode Ⅱ dynamic fracture toughness of 40Cr presents a positive correlation with the loading rate. Through the analysis of the fracture morphology of the recovered specimen, it is found that there exists a transition from tensile failure mode to adiabatic shear failure mode with the increase of loading rate, and the critical loading rate is about 2.92 TPa·m1/2/s. When KⅡd≤1.70 TPa·m1/2/s, the 40Cr steel mainly exhibits brittle fracture; when KⅡd is in the range of 2.12−2.92 TPa·m1/2/s, the fracture mainly presents the morphological characteristics of ductile fracture; when KⅡd ≥ 3.19 TPa·m1/2/s, the material failure is mainly caused by adiabatic shear bands. Brittle fracture is dominated by strain rate hardening mechanism, ductile fracture is dominated by strain/strain rate hardening and thermal softening mechanisms, and adiabatic shear fracture is dominated by thermal softening mechanism.
-
40Cr钢是航空航天以及国防领域中使用最广泛的高强钢之一,因其淬透性良好,经热处理后有良好的综合力学性能和低温冲击韧性,常被用于制作机翼大梁、坦克变速器扭轴以及装甲车辆的轴承类零件等[1],但这些构件在服役过程中容易受到冲击剪切载荷的作用而发生Ⅱ型断裂失效。因此,工程材料在Ⅱ型加载下的动态断裂问题日益受到人们的关注,Ⅱ型裂纹在动态载荷下的起裂及扩展行为也成为力学和材料学科的交叉前沿课题。20世纪80年代,Kalthoff等[2-5]发现42CrMo4钢在低加载速率下Ⅱ型裂纹发生拉伸型失效,裂纹扩展方向与韧带方向成−70°夹角,而在高加载速率下裂纹沿韧带方向以绝热剪切带形式发生失效。Zhou等[6-8]的研究表明,材料的失效模式转变行为更多地依赖于材料特性,而不是试样的几何形状。Ravi-Chandar等[9-10]发现,随着冲击速度的增大,聚碳酸酯(PC)的失效模式存在从延性到脆性再到延性的转变,且第二次模式转变会导致裂纹尖端附近形成剪切带。Mason等[11]对C-300钢单缺口试件进行动态剪切试验,研究了不同失效形式之间的转换。Chu等[12]对金属材料的韧脆模式转变进行了相场损伤演化,发现应力波的传播在脆性-韧性破坏模式转变中起着重要作用。在实验测试方面,莫尔云纹法、全息法和光弹性法等光测力学方法[13-15]已被应用到断裂试验中[16-20],并取得了满意的效果。但是,目前对于高强钢Ⅱ型裂纹在冲击载荷下失效行为的研究大多仍采用类似Kalthoff的加载方法或单杆加载方法对单边裂纹试样进行测试[21],这类加载方法不易于对断口形貌特征的转变行为进行定量控制和精确测量。近期,许泽建等[22-24]提出了一种新型Ⅱ型动态断裂实验方法,该方法可以实现对加载速率的精确控制,从而可以对材料的失效模式转变行为进行系统的量化研究。他们通过对TC4材料的研究,发现失效模式转变(FMT)不是突变的结果,而是受不同微观机制主导的渐变过程。随着加载速率的增加,材料的失效模式及断口特征均发生改变,是多种失效机制协同作用的结果。以上结果为Ⅱ断裂的失效行为研究提供了新的方法和思路。
本文中采用该新型Ⅱ型动态断裂实验方法,基于分离式霍普金森压杆(SHPB)技术[25],对40Cr高强钢开展不同加载速率下的动态断裂实验;采用实验-数值方法确定材料的动态断裂韧性值,并通过高速摄影观察裂纹的起裂及扩展过程;结合试样断口的宏、微观形貌特征分析,对材料的失效模式及微观机理进行研究。
1. 实验方法
采用SHPB系统[26]对Ⅱ型裂纹进行动态加载和测量,并采用实验-数值方法对裂尖的应力强度因子和断裂韧性进行确定。实验时,通过入射杆对特殊设计的Ⅱ型断裂试样进行加载,并在裂尖形成Ⅱ型载荷,试样的几何外形及尺寸如图1所示。入射杆、透射杆和试样上均贴有应变片,分别记录入射波
εi(t) 、透射波εt(t) 信号和试样的起裂信号。为避开裂尖的塑性区,试样上应变片的粘贴位置位于裂尖的一侧,应变片中心点位于距离裂尖约3 mm处(见图1)。根据一维应力波理论,可获得压杆与试样接触面处的载荷和位移曲线,如下式:{Fin=AE[εi(t)+εr(t)]Fout=AEεt(t) (1) {Uin=c0∫t0[εi(t)−εr(t)]dtUout=c0∫t0εt(t)dt (2) 把实验所测入射杆的应力波波形与实际位移边界条件等作为输入量,对试样的受载过程进行数值模拟,并通过位移法[22]计算裂尖的应力强度因子曲线KⅡ(t),如下式:
KⅡ(t)=√π 2rG1−μu(r,t) (3) 式中:
Fin 、Fout 为入射杆与透射杆端部的载荷,Uin 、Uout 为入射杆与透射杆端部的位移,A为横截面积,E为杨氏模量,c0 为杆的纵波波速,r为距裂尖的距离,G为剪切模量,μ 为泊松比,u(r, t)为裂尖位移场。在此基础上,结合试样的起裂信号可以确定材料的Ⅱ型动态断裂韧性。
另外,裂尖的加载速率
˙KⅡd 可由下式确定:˙KⅡd=KⅡ(tf)tf=KⅡdtf (4) 式中:
tf 为试样的起裂时间,KⅡd为动态断裂韧性。实验材料为40Cr高强钢,元素成分见表1,热处理工艺为:40Cr正火850 ℃,冷却方式为空冷,40Cr淬火温度为780 ℃,冷却方式为水冷,回火温度为200 ℃,冷却方式为空冷。针对同批次、同炉热处理的材料进行了力学性能测试,得到40Cr材料的力学性能测试参数,见表2,表中ρ为密度,E为弹性模量,μ为泊松比,
σb 为抗拉强度。实验中通过控制气压大小和子弹长度来控制加载速率。表 1 高强钢40Cr的元素成分及质量分数Table 1. Composition and mass fraction of high-strength steel 40Crw(C)/% w(Mn)/% w(Si)/% w(Cr)/% w(Ni)/% 0.37~0.45 0.50~0.80 0.20~0.40 0.80~1.10 − 表 2 高强钢40Cr的力学性能参数Table 2. Mechanical properties of high strength steel 40Cr材料 ρ/(kg·m−3) E/GPa μ σb/MPa 40Cr 7820 199 0.3 1987 在1.08~5.53 TPa·m1/2/s加载速率范围内对40Cr试样进行动态加载,为避免压杆中反射的应力波对试样造成重复加载,实验中采用了单脉冲加载技术[27]。图2为在4.86 TPa·m1/2/s加载速率下获得的原始波形。由图2可知,反射波在入射杆端部经单脉冲系统吸收后,剩余的反射波成分很少,幅值接近于零,因此可以实现对试样的单次加载。通过该技术,可以避免压杆中因应力波的反射给试样造成重复加载,有利于对材料的失效模式进行确定和研究。另外,试样的起裂时间采用应变片法[22]进行测定,原理为当应力波作用于试样时,试样先发生弹性变形,此时应变信号从零开始线性增加;当裂尖出现塑性区并开始起裂时,模拟中试样应变信号与实测信号发生分离,其分离点即为起裂时间。这部分时间包括了裂尖起裂时的应力波传到应变片的时间[23],见下式:
Δt=√D2+(B/2)2c0 (5) 式中:D为应变片中心到裂尖的距离,B为试样的厚度,c0为卸载波的波速, 一般为弹性波纵波波速。
2. 实验结果
在1.25 TPa·m1/2/s加载速率下的实验波形如图3所示,试样发生了拉伸型起裂。由图3可知,反射波电压先上升到0.2 V,然后骤降;与此同时,透射波信号也不断降低。在138 μs左右,反射波恢复到0.2 V并保持稳定,透射波对应的信号上升至−0.1 V。结合高速摄影观察分析知,反射波与透射波信号下降阶段对应于应力波对试样的加载过程,此过程中试样始终与压杆保持接触,且试样发生起裂但尚未完全断裂。当反射波信号上升至0.2 V、透射波信号上升至−0.1 V时,试样完全起裂,且通过高速摄影观察可知,试样单边支撑端先发生断裂。此后透射端载荷未降至0 V,说明该阶段透射杆与试样支撑端仍保持接触状态。
在4.86 TPa·m1/2/s加载速率下的实验波形见图4,试样发生绝热剪切型断裂。由图4可知,反射波电压在上升到0.45 V后开始缓慢下降;与此同时,透射波信号也较为平缓地下降。在38 μs左右,反射波恢复到0.5 V并保持稳定,透射波对应的信号上升到0 V。结合高速摄影(见图5)观察分析知,反射波与透射波信号下降阶段对应于应力波对试样的加载过程,此过程中试样始终与压杆保持接触,且试样发生起裂但尚未完全断裂。当反射波电压值保持为0.5 V、透射波电压保持为0 V时,试样完全起裂,且通过高速摄影观察可知试样双边支撑端同时发生断裂。此后透射波电压降为0 V,说明该阶段透射杆与试样两支撑端已脱离接触。
以上两次实验的加载过程如图5所示,其中图5(a)、(e)分别为试样初始受载时刻;图5(b)、(f)为试样裂尖开始起裂的时刻,可以观察到此时裂尖局部出现白亮区域,说明已经发生了小范围屈服现象;图5(c)、(g)为不同加载速率下试样完全断裂的失效形态。根据以上分析可将加载过程分为2个阶段:第1阶段为从试样开始受载到临界起裂(即出现白点)的阶段,此阶段表现为试样吸收能量发生弹性变形,同时在裂尖区域形成小范围塑性变形和微裂纹;第2阶段是从裂尖处的微裂纹集聚形成新的界面(即发生起裂)至试样以拉伸型或绝热剪切型失效模式发生完全断裂。在第2阶段,随着加载速率的增加,裂纹扩展角及扩展路径也随之发生变化。该现象说明裂纹扩展过程中裂尖的应力状态和材料特性会发生改变并决定着裂纹面的发展趋势。
3. 分析及讨论
3.1 有限元模拟及验证
有限元模拟采用ABAQUS/Explicit,此软件的显示动力学模块在计算高速冲击加载和接触性问题时具有显著的优势。根据实际工况和尺寸进行1∶1建模,并将实测的入射杆应力波作为输入量。入射杆、透射杆和试样的材料均定义为线弹性模型,参数见表3;入射杆、透射杆与试样的接触端面均设为法向硬接触,并在试样支撑端的侧面施加横向位移约束以模拟实际工况中夹具的限位作用。入射杆与透射杆均采用八节点六面体单元;为提高计算精度,在裂尖区域采用六面体-楔形体-四面体的过渡方式划分网格,并进行网格细化,使裂纹端部与裂纹半长处的网格尺寸比值控制在0.001~0.02之间,此时裂尖弹性应变场的计算误差约为1%。模型装配图如图6所示。
表 3 入射杆、透射杆的力学性能参数Table 3. Mechanical properties of the incident and transmission bars材料 ρ/(kg·m−3) E/GPa μ 18Ni 8000 190 0.3 将模拟结果中试样粘贴应变片区域的应变信号与实测的应变信号进行对比,如图7所示。当材料处于线弹性阶段时,试样的模拟真实应变与实测的试样应变信号基本吻合,佐证了材料参数的准确性以及有限元模拟的可靠性。当时材料处于塑性变形阶段,且实测应变为0.005时,模拟信号与实测信号发生分离,该分离点作为起裂时刻。由图8可知,随着子弹速度的增加,试样的起裂时间明显提前,且基本保持线性关系。采用位移法确定的应力强度因子(dynamic stress intensity factor, DSIF)曲线如图9所示。根据起裂时间,在DSIF曲线上确定了试样Ⅱ型断裂韧性,结果见表4。图10中还给出了文献[21]采用单杆加载单边裂纹试样方法所测出的40Cr钢动态断裂韧性结果。从图10中可看出,新型Ⅱ型加载方式测得的动态断裂韧性DFT(dynamic fracture toughness)明显高于文献结果,这可能是由于文献中的单杆加载方式在对试样加载的过程中,单边裂纹在弯矩作用下出现了Ⅰ型断裂现象(即张开型断裂),因此裂尖起裂时受到Ⅰ型断裂的影响,导致所测得的Ⅱ型动态断裂韧性值偏低。
表 4 高强钢40Cr的动态断裂韧性值Table 4. Dynamic fracture toughness values of high strength steel 40CrL/m p/MPa tf/µs KⅡd/(MPa·m1/2) ˙KⅡd/(TPa·m1/2/s) 0.380 0.18 27 29.2 1.08 0.380 0.19 26 32.6 1.25 0.380 0.22 22 37.5 1.70 0.203 0.18 19 40.2 2.12 0.203 0.20 17 49.4 2.92 0.203 0.22 16 51.0 3.19 0.100 0.14 13 50.3 3.87 0.100 0.16 11 53.5 4.86 0.100 0.18 10 55.3 5.53 注: L为子弹长度,p为气压,tf为起裂时间,KⅡd为Ⅱ型断裂韧性,˙KⅡd为加载速率 综上所述,当加载速率在1.08~5.53 TMPa·m1/2/s范围时,40Cr材料Ⅱ型断裂韧性与加载速率呈现正相关趋势。其中当
˙KⅡd ≤2.92 TPa·m1/2/s时,40Cr试样均属于拉伸型失效模式,裂纹扩展方向与韧带方向夹角由−60°逐渐减小至−45°,动态断裂韧性(DFT)与加载速率的拟合表达式为y=10.45x+18.83 ;当˙KⅡd ≥3.19 TPa·m1/2/s时,试样均表现为绝热剪切型失效模式,裂纹扩展与韧带方向夹角约为−16°,拟合表达式为y=2.09x+43.39 。3.2 断口形貌分析
采用Phenom Pro扫描电镜对试样断口形貌进行观察,根据形貌特征分为脆性、韧性以及绝热剪切型3种断口。由图11可知,拉伸型失效模式下存在脆性、韧性两种断口形貌,而绝热剪切型失效模式下仅存在绝热剪切型断口形貌特征。在不同的断口形貌下,DFT与
˙KⅡd 的线性拟合参数见表5。表 5 不同断口形貌下DFT与加载速率的线性拟合参数Table 5. Linear fitting parameters of DFT and the loading rates under different fracture morphology材料 断口类型 A b 40Cr 脆性 12.88 15.80 韧性 9.98 19.94 绝热剪切型 2.09 43.39 在低加载速率(
˙KⅡd ≤1.70 TPa·m1/2/s)下,在裂尖起裂区观察到小范围的韧窝,而整个断口形貌如图12所示。在图12的左中可以观察到晶粒呈现出多面体外形的冰糖状花样,而且有较为明显的晶粒以及清晰的晶界,因此断口有沿晶断裂的特征。这通常是由于晶界内存在夹杂物使得晶界结合力降低,从而导致微裂纹在该处萌生并沿晶界扩展,最终形成沿晶断口。此外,从A区放大图中不难发现试样断口存在二次裂纹,且具有穿晶断裂特征,说明在该条件下试样具有沿晶和穿晶的混合断裂特征。随着加载速率的增加,断裂韧性的增加表征着材料起裂的能耗增大,这可能是由于断口韧窝数目的增加造成的。因此在低加载速率下,40Cr材料断口属于由应变率硬化机制主导且以脆性断裂为主的断裂模式。在中加载速率(2.12 TPa·m1/2/s≤
˙KⅡd ≤2.92 TPa·m1/2/s)下,40Cr试样断口宏观上呈亮灰色,断面有平滑的剪切滑移带且有金属光泽。将图13局部区域放大后可观察到明显的韧性断口形貌特征:抛物线型的剪切韧窝以及平滑的长条状形貌。其原因一般为微孔洞在剪切应力作用下萌生、成核、长大、集聚形成剪切韧窝,随着加载速率的增加,形变不断加剧,由此产生的绝热温升使剪切韧窝软化,同时在剪切应力作用下被快速剪切为平滑的长条状形貌,甚至形成无特征的平坦面,该区域面积占比伴随着加载速率的增大而不断增大。因此,在中加载速率下,40Cr材料断口属于由应变/应变率硬化以及热软化机制共同主导的韧性断裂模式。在高加载速率(
˙KⅡd ≥3.19 TPa·m1/2/s)下,40Cr试样断口宏观上呈亮色,断面有较为平滑的剪切滑移带且有金属光泽。从图14中观察到绝热剪切型失效特征:断口存在连续的熔融区,并附有光亮的、球状的和不规则形状的金属液滴。在较高加载速率下,微裂纹的形成机理与在脆韧性下相同,但在更高加载速率下,断裂面之间错动会引起更剧烈的绝热温升并在局部形成绝热剪切带,表现为熔融的金属液滴以及连续的熔融区。此后由于断面分离,冷却速度均匀的液滴由于表面张力会凝固成形状规则的白亮球体,反之则形成不规则形状的金属液滴。因此,在高加载速率下,40Cr材料断口属于由热软化机制主导的绝热剪切型断裂模式。由以上分析可知,不同加载速率下,材料的断口形貌特征也不同。低加载速率下,40Cr材料断口属于由应变率硬化机制主导的脆性断裂模式;中加载速率下,属于由应变/应变率硬化以及热软化机制共同主导的韧性断裂模式;高加载速率下,属于由热软化机制主导的绝热剪切型断裂模式。
4. 结 论
采用新型Ⅱ型动态断裂测试技术,对40Cr钢进行了不同加载速率下的Ⅱ型动态断裂特性研究,得到如下结论:
(1)在加载速率范围(1.08~5.53 TPa·m1/2/s)内,40Cr材料的Ⅱ型断裂韧性值与加载速率基本呈正相关趋势。
(2)在1.08~5.53 TPa·m1/2/s的加载速率范围内,40Cr存在拉伸型失效模式向绝热剪切型失效模式转变,且临界加载速率为2.92 TPa·m1/2/s。
(3)当
˙KⅡd ≤1.70 TPa·m1/2/s时,40Cr材料的断口表现为脆性断裂;当˙KⅡd 介于2.12~2.92 TPa·m1/2/s之间时,断口表现为韧性断裂;当˙KⅡd ≥3.19 TPa·m1/2/s时,断口表现为绝热剪切型形貌特征。(4)脆性断裂由应变率硬化机制主导,韧性断裂由应变/应变率硬化和热软化机制共同主导,绝热剪切型断裂由热软化机制主导。
-
表 1 高强钢40Cr的元素成分及质量分数
Table 1. Composition and mass fraction of high-strength steel 40Cr
w(C)/% w(Mn)/% w(Si)/% w(Cr)/% w(Ni)/% 0.37~0.45 0.50~0.80 0.20~0.40 0.80~1.10 − 表 2 高强钢40Cr的力学性能参数
Table 2. Mechanical properties of high strength steel 40Cr
材料 ρ/(kg·m−3) E/GPa μ σb/MPa 40Cr 7820 199 0.3 1987 表 3 入射杆、透射杆的力学性能参数
Table 3. Mechanical properties of the incident and transmission bars
材料 ρ/(kg·m−3) E/GPa μ 18Ni 8000 190 0.3 表 4 高强钢40Cr的动态断裂韧性值
Table 4. Dynamic fracture toughness values of high strength steel 40Cr
L/m p/MPa tf/µs KⅡd/(MPa·m1/2) ˙KⅡd/(TPa·m1/2/s) 0.380 0.18 27 29.2 1.08 0.380 0.19 26 32.6 1.25 0.380 0.22 22 37.5 1.70 0.203 0.18 19 40.2 2.12 0.203 0.20 17 49.4 2.92 0.203 0.22 16 51.0 3.19 0.100 0.14 13 50.3 3.87 0.100 0.16 11 53.5 4.86 0.100 0.18 10 55.3 5.53 注: L为子弹长度,p为气压,tf为起裂时间,KⅡd为Ⅱ型断裂韧性,˙KⅡd为加载速率 表 5 不同断口形貌下DFT与加载速率的线性拟合参数
Table 5. Linear fitting parameters of DFT and the loading rates under different fracture morphology
材料 断口类型 A b 40Cr 脆性 12.88 15.80 韧性 9.98 19.94 绝热剪切型 2.09 43.39 -
[1] 黄浩. 40Cr表面激光熔覆硬质涂层及其应用研究[D]. 河北秦皇岛: 燕山大学, 2016. [2] KALTHOFF J F. Shadow optical analysis of dynamic shear fracture [J]. Optical Engineering, 1988, 27(10): 271035. DOI: 10.1117/12.7976772. [3] KALTHOFF J F. Transition in the failure behavior of dynamically shear loaded cracks [J]. Applied Mechanics Reviews, 1990, 43(S5): 47–50. DOI: 10.1115/1.3120818. [4] KALTHOFF J F. Modes of dynamic shear failure in solids [J]. International Journal of Fracture, 2000, 101(1): 1–31. DOI: 10.1023/A: 1007647800529. [5] KALTHOFF J F, BÜRGEL A. Influence of loading rate on shear fracture toughness for failure mode transition [J]. International Journal of Impact Engineering, 2004, 30(8): 957–971. DOI: 10.1016/j.ijimpeng.2004.05.004. [6] ZHOU M, ROSAKIS A J, RAVICHANDRAN G. Dynamically propagating shear bands in impact-loaded prenotched plates—Ⅰ. experimental investigations of temperature signatures and propagation speed [J]. Journal of the Mechanics and Physics of Solids, 1996, 44(6): 981–1006. DOI: 10.1016/0022-5096(96)00003-8. [7] ZHOU M, RAVICHANDRAN G, ROSAKIS A J. Dynamically propagating shear bands in impact-loaded prenotched plates—Ⅱ. numerical simulations [J]. Journal of the Mechanics and Physics of Solids, 1996, 44(6): 1007–1021, 1023-1032. DOI: 10.1016/0022-5096(96)00004-X. [8] ZHOU M, ROSAKIS A J, RAVICHANDRAN G. On the growth of shear bands and failure-mode transition in prenotched plates: a comparison of singly and doubly notched specimens [J]. International Journal of Plasticity, 1998, 14(4): 435–451. DOI: 10.1016/S0749-6419(98)00003-5. [9] RAVI-CHANDAR K. On the failure mode transitions in polycarbonate under dynamic mixed-mode loading [J]. International Journal of Solids and Structures, 1995, 32(6): 925–938. DOI: 10.1016/0020-7683(94)00169-W. [10] RAVI-CHANDAR K, LU J, YANG B, et al. Failure mode transitions in polymers under high strain rate loading [J]. International Journal of Fracture, 2000, 101(1): 33–72. DOI: 10.1023/A: 1007581101315. [11] MASON J J, ROSAKIS A J, RAVICHANDRAN G. On the strain and strain rate dependence of the fraction of plastic work converted to heat: an experimental study using high speed infrared detectors and the Kolsky bar [J]. Mechanics of Materials, 1994, 17(2): 135–145. DOI: 10.1016/0167-6636(94)90054-X. [12] CHU D Y, LI X, LIU Z L, et al. A unified phase field damage model for modeling the brittle-ductile dynamic failure mode transition in metals [J]. Engineering Fracture Mechanics, 2019, 212: 197–209. DOI: 10.1016/j.engfracmech.2019.03.031. [13] CHIANG F. Moiré and speckle methods applied to elastic-plastic fracture studies[C]//Experimental Techniques in Fracture Mechanics. New York: VCH, 1993: 291-325. [14] SANFORD R J. Determining fracture parameters with full-field optical methods [J]. Experimental Mechanics, 1989, 29(3): 241–247. DOI: 10.1007/BF02321401. [15] PATTERSON E A, OLDEN E J. Optical analysis of crack tip stress fields: a comparative study [J]. Fatigue & Fracture of Engineering Materials & Structures, 2004, 27(7): 623–635. DOI: 10.1111/j.1460-2695.2004.00774.X. [16] MA L, KOBAYASHI A S, ATLURI S N, et al. Crack linkup: an experimental analysis [J]. Experimental Mechanics, 2002, 42(2): 147–152. DOI: 10.1007/BF02410876. [17] GOECKE K E, MOSHIER M A. A technique to measure fatigue crack growth threshold [J]. Experimental Mechanics, 2002, 42(2): 182–185. DOI: 10.1007/BF02410881. [18] 王洪山. 几种实用的断裂试验方法 [J]. 理化检验-物理分册, 2001, 37(7): 292–294. DOI: 10.3969/j.issn.1001-4012.2001.07.005.WANG H S. Some kinds of practical fracture test methods [J]. Physical Testing and Chemical Analysis Part A: Physical Testing, 2001, 37(7): 292–294. DOI: 10.3969/j.issn.1001-4012.2001.07.005. [19] 姜风春, 刘瑞堂. 动态断裂韧性测试方法的有效性分析 [J]. 哈尔滨工程大学学报, 1999, 20(3): 97–101. DOI: 10.3969/j.issn.1006-7043.1999.03.017.JIANG F C, LIU R T. Analysis of validity of dynamic fracture toughness measurement [J]. Journal of Harbin Engineering University, 1999, 20(3): 97–101. DOI: 10.3969/j.issn.1006-7043.1999.03.017. [20] 郑坚, 王泽平, 段祝平. 动态断裂的加载和测试技术 [J]. 力学进展, 1994, 24(4): 459–475. DOI: 10.6052/1000-0992-1994-4-j1994-042.ZHENG J, WANG Z P, DUAN Z P. Loading and measuring techniques indynamic fracture testing [J]. Advances in Mechanics, 1994, 24(4): 459–475. DOI: 10.6052/1000-0992-1994-4-j1994-042. [21] 许泽建, 李玉龙, 刘元镛, 等. 两种高强钢在高加载速率下的Ⅱ型动态断裂韧性 [J]. 金属学报, 2006, 42(6): 635–640. DOI: 10.3321/j.issn: 0412-1961.2006.06.013.XU Z J, LI Y L, LIU Y Y, et al. Mode Ⅱ dynamic fracture toughness of two high strength steels under high loading rate [J]. Acta Metallurgica Sinica, 2006, 42(6): 635–640. DOI: 10.3321/j.issn: 0412-1961.2006.06.013. [22] 许泽建, 黄风雷, 何晓东. 一种用于纯Ⅱ型动态断裂的试验件: CN201820669843.4 [P]. 2018-12-11. [23] XU Z J, HE X D, HAN Y, et al. A different viewpoint on mechanism of fracture to shear-banding failure mode transition [J]. Journal of the Mechanics and Physics of Solids, 2020, 145: 104165. DOI: 10.1016/j.jmps.2020.104165. [24] XU Z J, HAN Y, FAN C Z, et al. Dynamic shear fracture toughness and failure characteristics of Ti-6Al-4V alloy under high loading rates [J]. Mechanics of Materials, 2021, 154: 103718. DOI: 10.1016/j.mechmat.2020.103718. [25] JIANG F C, VECCHIO K S. Hopkinson bar loaded fracture experimental technique: a critical review of dynamic fracture toughness tests [J]. Applied Mechanics Reviews, 2009, 62(6): 060802. DOI: 10.1115/1.3124647. [26] BAI Y L, DODD B. Adiabatic shear localization: occurrence, theories, and applications [M]. Oxford: Pergamon Press, 1992: 73−75. [27] NEMAT-NASSER S, ISAACS J B, STARRETT J E. Hopkinson techniques for dynamic recovery experiments [J]. Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1991, 435(1894): 371–379. DOI: 10.1098/rspa.1991.0150. 期刊类型引用(3)
1. 范瑞伯. 腐蚀性环境下石油钻修井机井架强度可靠性分析模型. 能源与环保. 2023(03): 99-104 .  百度学术
百度学术2. 李志刚,李淑欣,余丰,鲁思渊,王永刚. 试样形状对轴承钢绝热剪切带微观组织的影响. 爆炸与冲击. 2023(04): 56-66 .  本站查看
本站查看3. 徐恒威,梁斌,刘俊新,卢永刚. 高应变速率下无铅锡基焊料的力学性能. 有色金属工程. 2022(11): 30-37 .  百度学术
百度学术其他类型引用(1)
-










 下载:
下载:






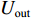
































 下载:
下载:













 百度学术
百度学术












