Effect of damping on equivalent static load dynamic factor of air blast load
-
摘要: 为考查阻尼参数对空爆荷载等效静载动力系数的影响，理论推导了空爆荷载下结构等效单自由体系弹塑性位移解及延性比解，设计并计算了阻尼比0.000 1～0.1、延性比1～4的20种典型工况的动力系数，并与现行抗爆设计规范动力系数公式结果进行了对比。结果表明：阻尼比小于0.000 1时可基本代表无阻尼状态，阻尼比0.01的动力系数比无阻尼的最大降低幅度为2.08%，数值差异很小，因此阻尼比为0.01以内时，可忽略阻尼对动力系数的影响；阻尼比0.05的动力系数比无阻尼的降低幅度约9.92%，数值差异较大，认为阻尼比0.05以上时将具有明显的经济效益；现行设计规范动力系数更适用于柔性结构体系，运用于刚性结构抗爆设计时，计算误差较大，对阻尼比较小的结构设计更不利。Abstract: In order to examine the effect of damping on the equivalent static load dynamic factor of the air blast loading, the solutions of the elastoplastic displacement and ductility ratio were derived by the structural equivalent single degree of freedom (SDOF) method for the air blast loading. According to the relationship between the duration of the air blast loading and the duration required for the structural members to complete elastic vibration, the members are divided into two types: rigid members and flexible members. Twenty typical calculation cases, including damping ratios from 0.000 1 to 0.1 and ductility ratios from 1 to 4, were completed and compared with the dynamic factor formula results of the current blast resistant design code. The results show as follow. A ductility ratio less than 0.000 1 can be regarded as a state without damping. The relative error of the dynamic factor between the calculation results with a damping ratio of 0.01 and without damping is less than 2.08%. This relative error is so small that the damping effect with a damping ratio less than 0.01 can be ignored. The dynamic factor with a damping ratio of 0.05 is about 9.92% lower than the one without damping. This relative error is so great that considering the damping ratio will have obvious economic benefits for the blast resistant design when its value is greater than 0.05. Based on the elastic design, the calculation results from the current blast resistant code formula are in good agreement with those from the derived formula in this paper, and the value of the dynamic factor calculated from the code is between the results of damping ratios of 0.01 and 0.05. Furthermore, the current air blast resistant design code formula is more suitable for flexible structure systems. When the code formula is applied to calculate the dynamic factor of rigid members, there will be a large calculation error, which is more unfavorable for members with small damping.
-
易爆物品储存运输不当、燃气爆炸及暴恐袭击等时有发生，建筑结构在其服役期内遭受空爆荷载的概率逐渐增大。在进行结构抗爆分析时，抗爆设计规范普遍推荐采用无阻尼结构动力学体系[1-4]，实现基于动力系数的等效静载抗爆分析。空爆荷载作用时长很短，可近似简化为三角形衰减荷载，这种简化可使不熟悉空爆荷载的结构设计人员完成抗爆计算。目前，民用建筑抗爆设计采用延性比等参数完成弹塑性抗爆分析，延性比为结构振动弹塑性变形最大值与弹性变形最大值的比[4]。无阻尼自由振动体系没有能量耗散，是一种无休止的简谐振动。忽略阻尼作用，将放大结构振动各个阶段的位移幅值，而对延性比、动力系数等防爆设计参数的影响，还没有明确的理论结论。
认识到阻尼对结构振动响应存在影响，已开展了一些研究。在理论方面：Biggs[5]采用等效单自由度（single degree of freedom, SDOF）进行抗爆分析时，提及阻尼对塑性阶段结构振动存在一定影响；Gantes等[6]分析空爆作用结构弹塑性振动时，指出阻尼对结构响应前几个振动周期存在一些影响；Riedel等[7]认为对于空爆作用下结构失效的情况，阻尼可忽略不计；方秦等[8-9]建立并求解了端部有阻尼支承的梁体空爆作用下的振动方程，表明空爆荷载作用时长越短，端部的阻尼支撑对梁体的抗力提高作用越显著；郭东等[10]采用杜哈梅积分方法，求解了空爆作用下弹性阶段含阻尼体系的等效单自由度动力方程，表明阻尼对反弹阶段的弹性位移振动影响显著，建议按30%进行位移折减，但未解决阻尼参数对塑性阶段响应的影响；陈万祥等[11]求解了含阻尼的柔性边界支承下浅梁的振动方程，认为边界阻尼对结构的破坏模式不会发生变化；董彬等[12]通过数值方法分析了含阻尼体系的梁体振动，表明加设阻尼器能有效控制空爆作用下的动力响应；杜志鹏等[13]将船体结构简化为梁模型，分析了水下爆炸船体鞭状运动的阻尼效应，结果表明不考虑阻尼效应将高估运动响应幅值。由空爆作用结构试验可知，阻尼对冲击波荷载结束后的自由振动阶段确实存在影响：Liu等[14]分析了尺寸0.15 m×0.15 m×1.7 m的钢筋混凝土梁在近场空爆作用下的破坏特征，实测位移显示，阻尼影响下结构在4～5个振动周期后静止；Nassr等[15]完成了5组工字型钢梁在远场空爆下的动力响应，4～6个振动周期后结构静止；Zhang等[16]完成了50 kg炸药近场空爆作用下长2.5 m钢管混凝土梁构件的振动效应，结果显示3～7个振动周期后构件静止；Liu等[17]进行了尺寸0.22 m×0.3 m×2 m的钢筋-玻璃纤维-混凝土梁构件在0.3～4 kg当量炸药下近场空爆试验，发现梁体弹性振动、塑性振动、截面断裂3种响应类型均在2～4个振动周期后静止，塑性振动及截面断裂对应的阻尼明显偏大；Nagata等[18]、Syed等 [19]、Ritchie等[20]、Shi等[21]和Foglar等[22]完成的结构空爆试验也充分说明阻尼对结构振动的显著影响。阻尼对空爆作用结构弹性阶段和塑性阶段强迫振动的影响、影响动力系数的程度、进而对抗爆设计的影响，试验上无法直接判别，也缺乏理论上的研究探索。
本文中，建立含阻尼的等效自由度体系振动模型，考虑空爆荷载作用时长与结构进入塑性振动时长的关系，将结构分为柔性结构、刚性结构、临界结构等3种类型，进行各种类型下结构弹塑性振动方程的求解，结合延性比、动力系数等抗爆设计参数定义，以典型的阻尼比设计验算工况，对比现行抗爆设计规范的推荐公式，考查阻尼对动力系数的影响。
1. 柔性结构空爆作用动力响应求解
1.1 弹塑性阶段动力方程
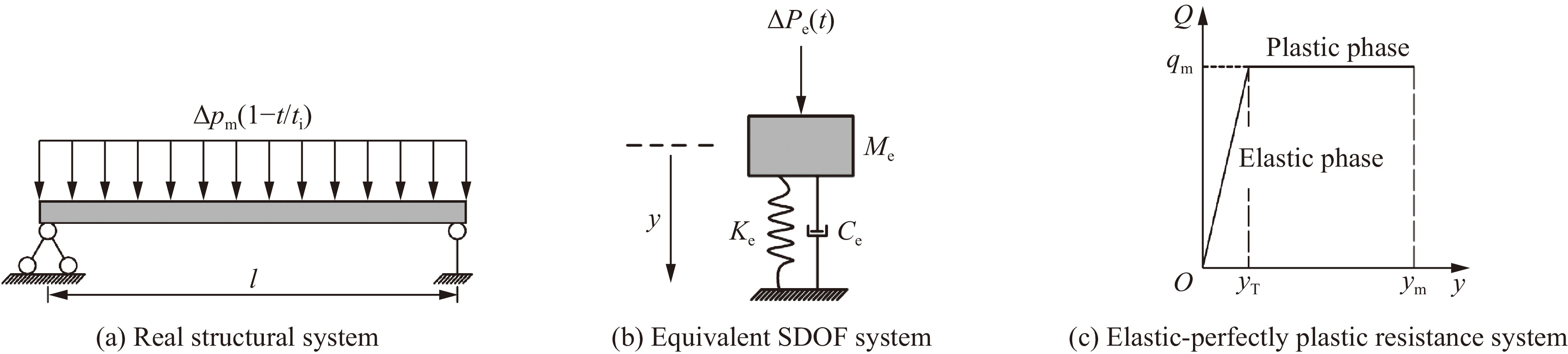
采用延性比的概念进行梁式及单向板结构空爆作用动力分析，且结构响应以弯曲振动分析为主时，等效单自由度方法具有良好的计算精度、简易的计算流程，被广泛采用，如图1所示。
图 1 理想弹塑性的含阻尼等效单自由度体系Figure 1. Elastic-perfectly plastic SDOF vibration system with damping考虑阻尼参数的弹性阶段等效单自由度振动方程为[5]：
Me¨y+Ce˙y+Key=ΔPe(t) (1) 式中：Me为弹性阶段等效结构质量，Ce为弹性阶段等效结构阻尼，Ke为等效结构刚度，y为与等效结构相等的、真实结构在典型位置处的振动位移，ΔPe(t)为等效结构承受的随时间t变化的等效空爆荷载。各等效系数分别为[5]：
Me=kMml,Ce=2ξ√kMmlkLK,Ke=kLK (2) 式中：m为结构每延米的质量，l为结构跨长，ξ为结构阻尼比，K为真实结构刚度， kM为弹性阶段质量变换系数，kL为弹性阶段荷载变换系数。空爆荷载持续时间非常短，可简化为等冲量的线性荷载，GB 50038–2005《人民防空地下室设计规范》[1]中，推荐采用等效空爆荷载：
ΔPe(t)={lkL(l)(1−t/ti)Δpm0≤t≤ti0t＞ti (3) 式中：ti为空爆荷载作用时长，∆pm为冲击波超压峰值，kL(l)为弹性阶段荷载变换系数kL或塑性阶段荷载变换系数kl。
设结构弹性位移最大值yT对应的时刻为tT，对应的振动速度为vT，此为结构进入塑性振动的区分点，将弹性参数替换为塑性参数后，结构塑性阶段方程为：
me¨y+ce˙y+qm=ΔPe(t) (4) 式中：me为塑性阶段等效结构质量，ce为塑性阶段等效结构阻尼，qm为结构最大抗力。各等效系数分别为：
me=kmml,ce=2ξ√kmmlklK,qm=klKyT (5) 式中：km为塑性阶段质量变换系数。
1.2 结构分类及柔性结构弹性阶段动力响应求解
柔性结构指的是空爆荷载作用时长ti小于该结构振动从0至最大弹性位移的时长tT，即ti＜tT；类似地，刚性结构指的是ti＞tT对应的结构，临界结构指的是ti=tT对应的结构。
求解空爆作用结构动力方程时，常采用杜哈梅积分方法，在求解过程中，会出现多次分部积分，计算过程复杂。根据微分方程理论：任意微分方程解答均可表示为通解与特解之和的形式，这个方法可一定程度简化本文动力方程的求解。
对于柔性结构，在弹性阶段且在荷载作用时长范围0≤t≤ti，由初始位移和初始速度均为0，结合式(1)、(3)可求该强迫振动阶段解：
y=yste−ξωt(−(1+2ξωti)cos(ωdt)+(1ωdti−ξωωd(1+2ξωti))sin(ωdt) + 1−tti+2ξωti) (6) v=yste−ξωt(((1+2ξωti)(ξ2ω2ωd+ωd)−ξωωdti)sin(ωdt) + 1ticos(ωdt)−1ti) (7) 式中：ω为无阻尼振动等效频率，ωd为含阻尼振动等效频率，yst为超压峰值为静载时的结构位移。各参数计算公式为：
ω=√Ke/Me,ωd=ω√1−ξ2,yst=Δpml/K (8) 将ti代替t代入式(6)～(7)，即可得到强迫振动结束时的位移yi、速度vi。当空爆荷载消去，这个阶段结构外荷载为0、以位移yi及速度vi为初始条件的含阻尼弹性自由振动（即当ti＜t≤tT），可由式(1)、(3)求解：
y=(yicos(ωd(t−ti))+vi+ξωyiωdsin(ωd(t−ti)))e−ξω(t−ti) (9) v=(vicosωd(t−ti)−(ωd+ξωvi+ξ2ω2yiωd)sinωd(t−ti))e−ξω(t−ti) (10) 在tT时，结构达到弹性振动位移最大值yT，此时结构振动速度vT，其显式表达式为：
yT=yst((e−ξθi(−(1+2ξγθi)cos(γθi)+(1γθi−ξγ(1+2ξγθi))sin(γθi))+2ξθi)cos(γ(θT−θi))+(e−ξθi((1γθi−ξγ−2ξ2γ2θi)cos(γθi)+(1+2ξγθi)sin(γθi))+2ξ2γθi−1γθi)sin(γ(θT−θi)))e−ξ(θT−θi) (11) vT=ystω((e−ξθi(cos(γθi)θi+(γ+ξ2γ+2ξ3γ2θi+2ξθi−ξγθi)sin(γθi))−1θi)cos(γ(θT−θi)) + e−ξθi((γ+ξ2γ+2ξ3γ2θi+2ξθi−ξγθi)cos(γθi)−sin(γθi)θi)sin(γ(θT−θi))+(2ξθi−ξγθi+2ξ3γθi)sin(γ(θT−θi)))e−ξ(θT−θi) (12) 式中：γ为阻尼综合降低系数，θT、θi为结构时长参数。计算公式为：
γ=√1−ξ2,θT=ωtT,θi=ωti (13) 1.3 柔性结构塑性阶段动力响应求解
根据理想弹塑性理论假设，结构完成弹性振动后结构达到最大的抗力，在塑性阶段该抗力保持不变，此时为外荷载为0、以位移yT及速度vT为初始条件的含阻尼塑性阶段自由振动。由式(4)可得：
y=−mece(vT+qmce)e−ceme(t−tT)−qmce(t−tT)+yT+mecevT+meqmce2 (14) v=−mece(vT+qmce)e−ceme(t−tT)−qmce (15) 在tm达到弹塑性位移最大值ym时，此时振动速度vm=0。令式（15）右为0，得出：
tm=meceln(1+cevTqm)+tT (16) 将式(16)对应的时刻tm代入式(14)，得出结构弹塑性振动最大位移为：
ym=yT−meqmc2eln(1+cevTqm)+mecevT (17) 代入等效单自由度体系对应参数，即将式(5)中me、ce及qm代入(17)，可得：
ym=yT−yT4ξ2ln(1+2ξvTωyT√km-lkM-L)+vT2ξω√km-lkM-L (18) 式中：kM-L、km-l分别为弹性、塑性阶段质量变换系数与荷载变换系数的比。即：
kM-L=kMkL,km −l=kmkl (19) 由结构弹塑性理论及抗爆设计规范，弹塑性阶段抗力动力系数kh和延性比β分别为：
kh=yTyst,β=ymyT (20) 将式(11)、(18)代入式(20)，且令：
f∗=(e−ξθi(1θicos(γθi)+(γ+ξ2γ+2ξ3γ2θi+2ξθi−ξγθi)sin(γθi))−1θi)cos(γ(θT−θi))−(e−ξθi((ξγθi−ξ2γ−2ξ3γ2θi−γ−2ξθi)cos(γθi)+1θisin(γθi))+2ξθi−ξγθi+2ξ3γθi)sin(γ(θT−θi)) (21) 则得到柔性结构延性比β关于抗力动力系数kh的表达式：
β=1−14ξ2ln(1+2ξf∗khe−ξ(θT−θi)√km-lkM-L)+f∗2ξkhe−ξ(θT−θi)√km-lkM-L (22) 2. 刚性及临界结构空爆作用动力响应求解
2.1 刚性结构弹性阶段动力响应求解
根据定义及理论分析可知，刚性结构弹性阶段（0＜t≤tT）与柔性结构0＜t≤ti时段的振动方程相同，将tT代入式(6)～(7)，可得tT时刻的位移和速度：
yT=yst(−(1+2ξωti)cos(ωdtT)+(1ωdti−ξωωd(1+2ξωti))sin(ωdtT))e−ξωtT−tTtiyst+yst(1+2ξωti) (23) vT=yst(1ticos(ωdtT)+((1+2ξωti)(ξ2ω2ωd+ωd)−ξωωdti)sin(ωdtT))e−ξωtT−ystti (24) 2.2 刚性结构塑性阶段动力响应求解
由定义可知，刚性结构从弹性振动进入塑性振动后，第1个塑性响应阶段为外荷载不为0、以位移yT和速度vT为初始条件的含阻尼塑性阶段强迫振动，即在tT＜t＜ti内，由式(4)求出其动力响应解答后，可得到ti时刻结构振动位移和速度分别为：
yi=yT+(vT−ΔPece−mΔPec2e+qmce)(mece(1−e−ceme(ti−tT)))+(ΔPece+meΔPec2e−qmce)(ti−tT)−ΔPe2ceti(ti−tT)2 (25) vi=(vT−ΔPece−mΔPec2e+qmce)e−ceme(ti−tT)−ΔPeceti(ti−tT)+ΔPece+meΔPec2e−qmce (26) 刚性结构振动t＞ti时，为无外荷载、位移yi和速度vi为初始条件的含阻尼塑性阶段自由振动，即当ti＜t≤tm时，该振动方程与柔性结构塑性阶段响应方程求解一致，将式(14)～(16)中用tT替换为ti后，便得出其解答，其中ym为：
ym=yi−meqmc2eln(1+ceviqm)+mecevi (27) 同理，将式(5)中me、ce和qm代入式(27)后，可将等效单自由体系转变为原结构参数。为精简篇幅、清晰显示延性比对应的参数关系，本文中省略该化简过程。令：
f∗1=1−kh2ξ√kM - Lkm - l+14ξ2θi (28) f∗2=(e−ξθT(1θicos(γθT)+((1+2ξγθi)(ξ2γ+γ)−ξγθi)sinγθT)−1θi)−f∗1 (29) f∗3=e−2ξ(θi−θT)√kM−Lkm−l,f∗4=θi−θT2ξθi√kM−Lkm−l (30) 由式(20)、(23)和(27)可知，基于动力系数的延性比为：
β=1−14ξ2ln(1+2ξkh√km-lkM-L(f∗3f∗2−f∗4+f∗2))+12ξkh(f∗1f∗3−f∗4+f∗1)+1kh(f∗2(1−f∗3)2ξ√km-lkM-L+f∗2(θi−θT)−14ξθi√kM-Lkm-l(θi2−θT)2) (31) 2.3 临界状态结构动力响应求解
根据临界状态定义可知，空爆荷载作用时长ti与结构完成弹性振动时长tT恰好相等，即ti=tT。在弹性阶段且在荷载作用时长范围0＜t＜ti，由弹性振动式(23)～(24)可得：
yi=yT=yst(−(1+2ξωti)cos(ωdti)+(1ωdti−ξωωd(1+2ξωti))sin(ωdti))e−ξωti+yst2ξωti (32) vi=vT=yst(1ticos(ωdti)+((1+2ξωti)(ξ2ω2ωd+ωd)−ξωωdti)sin(ωdti))e−ξωti−ystti (33) 振动时刻大于ti时，外荷载为0、以yi和vi为初始条件的含阻尼塑性阶段自由振动，即当ti＜t＜tm时，与柔性结构塑性阶段振动一样，即利用式(18)、(20)、(23)～(24)，且令：
f∗5=e−ξθi(1θicos(γθi)+(γ+ξ2γ+2ξ3γ2θi+2ξθi−ξγθi)sin(γθi))−1θi (34) 可得临界结构基于动力系数的延性比：
β=1−14ξ2ln(1+2ξkh√km−lkM−Lf∗5)+12ξkh√km−lkM−Lf∗5 (35) 由式(22)、(31)、(35)可以看出，将动力系数化解为关于阻尼比、延性比等参数的显性表达式难度很大，几乎不可能。因此，采用本文公式进行考虑阻尼的动力系数kh分析时，可根据结构类型的界定条件迭代计算。
3. 算例验证
3.1 算例工况遴选
GB 50038–2005《人民防空地下室设计规范》[1]中，推荐采用等效单自由振动动力系数计算方法。无阻尼、不含塑性参数的简化公式为：
kh=(2θi√2β−1+2β−12β(1+4/4θiθi))−1 (36) 为了校核本文公式的精准性，以式（36）为对比前提，以简支梁为结构选型，以文献[1]中推荐的钢筋混凝土构件允许延性比1～4为计算范围，以文献[23]中推荐的空爆结构-荷载参数θi≤2.2为参数范围；为了独立观察阻尼对动力系数的影响程度，选用典型的塑性参数，即弹性、塑性阶段等效质量系数与等效荷载系数之比kM-L、km-l，由文献[5]取常数值0.78、0.66；在阻尼比为0.0001～0.1时，选择5种典型阻尼比，即0.000 1（接近无阻尼）、0.001（极小阻尼）、0.01（小阻尼，建筑钢构件可采用数值）、0.05（常用阻尼比，钢筋混凝土及砌体构件常采用数值）、0.1（较大阻尼，塑性阶段可能性数值）作为建筑物构件典型的阻尼比。共计20种典型计算工况，见表1。
表 1 典型工况Table 1. Typical calculation cases工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β C1 0.0001 1 C6 0.0001 2 C11 0.0001 3 C16 0.0001 4 C2 0.001 1 C7 0.001 2 C12 0.001 3 C17 0.001 4 C3 0.01 1 C8 0.01 2 C13 0.01 3 C18 0.01 4 C4 0.05 1 C9 0.05 2 C14 0.05 3 C19 0.05 4 C5 0.1 1 C10 0.1 2 C15 0.1 3 C20 0.1 4 为了查看动力系数与延性比、阻尼比的关系，考虑阻尼比后的20种工况计算结果，与文献[1]中公式在延性比为1～4时计算结果的比较，如图2所示；各工况计算结果与文献[1]中公式计算结果的相对误差如图3(a)所示，与阻尼比为0.000 1工况计算结果的相对误差如图3(b)所示。
图 2 本文工况计算结果与文献公式的比较Figure 2. Comparison of the results from the calculation cases and from the code formula延性比β=1表示弹塑性抗爆设计退化为弹性设计，此时各阻尼比的动力系数曲线均为光滑曲线。延性比增加表示结构的塑性变形比例增加，随着β从2递增到4，动力系数kh对参数θi的斜率从0.8至0.6附近发生转折。相同延性比数值下，阻尼比越大，动力系数越小，阻尼比为0.000 1（接近无阻尼）、0.001（极小阻尼）、0.01（小阻尼）的差异非常小。相同阻尼比情况下，随着延性比β的增加，相同θi对应的动力系数降低。规范计算公式与柔性结构、临界结构计算结果差异很小，与刚性结构动力系数计算结果差异较大。
3.2 结果分析与讨论
由图2可知，延性比β为1时：文献[1]中公式计算结果与阻尼比为0.0001～0.1的计算结果差异均较小，低于阻尼比0.0001的计算结果，最小为0.06%，最大为4.23%，平均为2.43%；文献[1]中公式计算结果位于阻尼比0.01与0.05（常用阻尼比）之间，相差最小值为0.07%，最大值为2.63%，平均值为0.86%；阻尼比为0.1时，差异变得显著，平均值为11.73%。可见，用文献[1]中公式进行弹性阶段抗爆设计具有很高计算精度和优势，可观察到增加5%以上的阻尼比，对抗爆设计具有明显的经济效益。
由图3(a)可知：延性比β为2～4时，文献[1]中公式计算结果与柔性结构、临界结构计算公式的计算结果差异很小，略低于阻尼比0.0001、0.001的，略高于阻尼比0.01～0.1的，其差异为0～4%，用文献[1]中公式进行柔性结构抗爆设计仍具有较高精度；而较大幅度低于本文刚性结构的计算结果，在阻尼比0.0001～0.1下，延性比β=2时最大差异为31.88%～14.52%，延性比β=3时最大差异为38.83%～18.25 %，延性比β=4时最大差异为41.49%～18.09%。这种差异与文献[1]中忽略阻尼比及塑性参数有关，也与文献[1]中拟合公式时选择的类型有关。
由图3(b)（以阻尼比0.0001对应的动力系数为基准）可知：阻尼比为0.001时，动力系数结果降低有限，其差异为−0.11%～−0.20%，基本可以忽略，说明结构阻尼比在0.001以下，可按无阻尼进行结构抗爆设计；阻尼比为0.01时，动力系数值略有降低，其差异为−1.49%～−2.08%，数值较小，在结构设计允许范围内，可忽略该阻尼比对结构的影响；阻尼比为0.05时，动力系数值降低明显，平均值为−7.33%～−9.92%；阻尼比为0.1时，对应的动力系数降低非常明显，动力系数值降低平均值为−13.42%～−19.43%；阻尼比大于0.05时，临界结构的动力系数差异性明显低于柔性结构、刚性结构数值，即对于大多数抗爆结构，不应忽略此时的阻尼影响，可增设阻尼构造来降低抗爆设计的工程造价。
4. 结 论
推导了空爆作用下柔性结构、刚性结构和临界结构等效静载动力系数的隐式函数表达式，通过典型计算工况，分析了阻尼比对动力系数的影响，得到以下结论。
（1） 阻尼比0.001、0.01较阻尼比0.0001的动力系数值降低幅度约0.20%、2.08%，即阻尼比小于0.01时，数值差异性很小，即可忽略阻尼比小于0.01对抗爆设计动力系数影响作用。
（2） 阻尼比0.05、0.1较阻尼比0.0001的动力系数值降低幅度约9.92%、19.43%，说明将抗空爆结构的阻尼比提高至0.05以上，将产生有较大的经济效益，是一种良好的抗空爆设计手段。
（3） GB 50038–2005《人民防空地下室设计规范》[1]中，空爆动力系数计算公式忽略阻尼比、塑性参数，对完成弹性阶段抗爆设计具有很高计算精度和优势。当进行弹塑性抗爆设计时，规范中公式较适用于柔性结构、临界结构抗爆设计，且误差在4%以内。运用于刚性结构时，规范中公式均小于本文中计算公式计算结果，且阻尼比越小误差越大，延性比β=4时相对差异值可达18.09%～41.49%。
-
图 1 理想弹塑性的含阻尼等效单自由度体系
Figure 1. Elastic-perfectly plastic SDOF vibration system with damping
图 2 本文工况计算结果与文献公式的比较
Figure 2. Comparison of the results from the calculation cases and from the code formula
表 1 典型工况
Table 1. Typical calculation cases
工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β 工况 阻尼比 ξ 延性比 β C1 0.0001 1 C6 0.0001 2 C11 0.0001 3 C16 0.0001 4 C2 0.001 1 C7 0.001 2 C12 0.001 3 C17 0.001 4 C3 0.01 1 C8 0.01 2 C13 0.01 3 C18 0.01 4 C4 0.05 1 C9 0.05 2 C14 0.05 3 C19 0.05 4 C5 0.1 1 C10 0.1 2 C15 0.1 3 C20 0.1 4 
下载: 导出CSV
-
[1] 中华人民共和国建设部, 中华人民共和国国家质量监督检验检疫总局. 人民防空地下室设计规范: GB 50038–2005 [S]. 北京: 中国标准出版社, 2006. [2] US Army Corps of Engineers. Structures to resist the effects of accidental explosions: TM5–1300 [S]. Washington: Department of the Army, 1990. [3] Canadian Standards Association. Design and assessment of buildings subjects to blast loads: CSA/S 850–12 [S]. Ontario: CSA, 2012. [4] 中华人民共和国住房和城乡建设部. 建筑结构荷载规范: GB 50009–2012 [S]. 北京: 中国建筑工业出版社, 2012. [5] BIGGS J M. Introduction to structural dynamics [M]. New York: McGraw-Hill Book Company, 1964: 315−327. [6] GANTES C J, PNEVMATIKOS N G. Elastic-plastic response spectra for exponential blast loading [J]. International Journal of Impact Engineering, 2004, 30(3): 323–343. DOI: 10.1016/S0734-743X(03)00077-0. [7] RIEDEL W, FISCHER K, KRANZER C, et al. Modeling and validation of a wall-window retrofit system under blast loading [J]. Engineering Structures, 2012, 37: 235–245. DOI: 10.1016/j.engstruct.2011.12.015. [8] 方秦, 杜茂林. 爆炸荷载作用下弹性与阻尼支承梁的动力响应 [J]. 力学与实践, 2006, 28(2): 53–56. DOI: 10.3969/j.issn.1000-0879.2006.02.012.FANG Q, DU M L. Dynamic responses of an elastically supported beams with damping subjected to blast loads [J]. Mechanics in Engineering, 2006, 28(2): 53–56. DOI: 10.3969/j.issn.1000-0879.2006.02.012. [9] 方秦, 陈力, 杜茂林. 端部设置弹簧和阻尼器提高防护门抗力的理论与数值分析 [J]. 工程力学, 2008, 25(3): 194–199,221.FANG Q, CHEN L, DU M L. Theoretical and numerical investigations in effects of end-supported springs and dampers on increasing resistance of blast doors [J]. Engineering Mechanics, 2008, 25(3): 194–199,221. [10] 郭东, 刘晶波, 闫秋实. 爆炸荷载作用下梁板结构反弹机理分析 [J]. 建筑结构学报, 2012, 33(2): 64–71. DOI: 10.14006/j.jzjgxb.2012.02.009.GUO D, LIU J B, YAN Q S. Rebound mechanism analysis in beams and slabs subjected to blast loading [J]. Journal of Building Structures, 2012, 33(2): 64–71. DOI: 10.14006/j.jzjgxb.2012.02.009. [11] 陈万祥, 郭志昆, 叶均华. 爆炸荷载作用下柔性边界钢筋混凝土梁的动力响应与破坏模式分析 [J]. 兵工学报, 2011, 32(10): 1271–1277.CHEN W X, GUO Z K, YE J H. Dynamic responses and failure modes of reinforced concrete beams with flexible supports under blast loading [J]. Acta Armamentarii, 2011, 32(10): 1271–1277. [12] 董彬, 李志军, 魏同. 爆炸荷载下建筑结构的振动控制及分析 [J]. 西安工业大学学报, 2020, 40(4): 404–409. DOI: 10.16185/j.jxatu.edu.cn.2020.04.006.DONG B, LI Z J, WEI T. Vibration control and analysis of structure under explosion load [J]. Journal of Xi’an Technological University, 2020, 40(4): 404–409. DOI: 10.16185/j.jxatu.edu.cn.2020.04.006. [13] 杜志鹏, 汪玉, 杜俭业, 等. 舰船水下爆炸鞭状运动中的阻尼效应 [J]. 船海工程, 2008, 37(5): 95–98. DOI: 10.3963/j.issn.1671-7953.2008.05.027.DU Z P, WANG Y, DU J Y, et al. Damping effects on the whipping response of warship subjected to an underwater explosion [J]. Ship and Ocean Engineering, 2008, 37(5): 95–98. DOI: 10.3963/j.issn.1671-7953.2008.05.027. [14] LIU Y, YAN J B, HUANG F L. Behavior of reinforced concrete beams and columns subjected to blast loading [J]. Defence Technology, 2018, 14(5): 550–559. DOI: 10.1016/j.dt.2018.07.026. [15] NASSR A A, YAGI T, MARUYAMA T, et al. Damage and wave propagation characteristics in thin GFRP panels subjected to impact by steel balls at relatively low-velocities [J]. International Journal of Impact Engineering, 2018, 111: 21–33. DOI: 10.1016/j.ijimpeng.2017.08.007. [16] ZHANG F R, WU C Q, ZHAO X L, et al. Experimental study of CFDST columns infilled with UHPC under close-range blast loading [J]. International Journal of Impact Engineering, 2016, 93: 184–195. DOI: 10.1016/j.ijimpeng.2016.01.011. [17] LIU S F, ZHOU Y Z, ZHOU J N, et al. Blast responses of concrete beams reinforced with GFRP bars: Experimental research and equivalent static analysis [J]. Composite Structures, 2019, 226(10): 111271. DOI: 10.1016/j.compstruct.2019.111271. [18] NAGATA M, BEPPU M, ICHINO H, et al. Method for evaluating the displacement response of RC beams subjected to close-in explosion using modified SDOF model [J]. Engineering Structures, 2018, 157: 105–118. DOI: 10.1016/j.engstruct.2017.11.067. [19] SYED Z I, RAMAN S N, NGO T, et al. The failure behavior of reinforced concrete panels under far-field and near-field blast effects [J]. Structures, 2018, 14: 220–229. DOI: 10.1016/j.istruc.2018.03.009. [20] RITCHIE C B, PACKER J A, SEICA M V, et al. Behaviour and analysis of concrete-filled rectangular hollow sections subject to blast loading [J]. Journal of Constructional Steel Research, 2018, 147: 340–359. DOI: 10.1016/j.jcsr.2018.04.027. [21] SHI Y C, XIONG W, LI Z X, et al. Experimental studies on the local damage and fragments of unreinforced masonry walls under close-in explosions [J]. International Journal of Impact Engineering, 2016, 90: 122–131. DOI: 10.1016/j.ijimpeng.2015.12.002. [22] FOGLAR M, HAJEK R, KOVAR M, et al. Blast performance of RC panels with waste steel fibers [J]. Construction and Building Materials, 2015, 94: 536–546. DOI: 10.1016/j.conbuildmat.2015.07.082. [23] 耿少波, 李洪, 葛培杰. 考虑跃迁的指数型炸药空爆荷载等效静载动力系数 [J]. 爆炸与冲击, 2019, 39(3): 032201. DOI: 10.11883/bzycj-2018-0048.GENG S B, LI H, GE P J. Equivalent static load dynamical coefficient for exponential air blast loading with transition [J]. Explosion and Shock Waves, 2019, 39(3): 032201. DOI: 10.11883/bzycj-2018-0048. 期刊类型引用(4)
1. 胡志坚，张周煜，宋周滢，张永涛，耿波，刘辉. 混凝土梁桥实桥爆炸试验. 中国公路学报. 2025(05): 78-89 . 
百度学术2. 耿少波，洪欣，郑毅，沈新月. 质量参数对空爆荷载梁构件振动位移的影响. 爆炸与冲击. 2024(05): 110-120 . 
本站查看3. 耿少波，韩晓丹，牛艳伟，韩云山，马林林. 爆炸荷载线性强化抗力模型梁构件动力系数研究. 计算力学学报. 2024(05): 886-893 . 百度学术4. 耿少波，陈佳龙，赵洲，牛艳伟. 基于双折线抗力模型的空爆荷载梁式构件振动位移研究. 爆炸与冲击. 2022(10): 139-151 . 本站查看其他类型引用(4)
-
下载:
百度学术
计量
- 文章访问数: 599
- HTML全文浏览量: 336
- PDF下载量: 76
- 被引次数: 8