Optimal design of ballistic performance of fiber-metal laminates based on the response surface method
-
摘要: 纤维金属层合板因其复合材料的各向异性和层合结构特征而具有较好的可设计性,开展金属纤维层合板的优化设计研究对其力学性能的增强和轻量化具有重要意义。为提高纤维金属层合板的抗弹性能,基于响应面分析法对纤维金属层合板的铺层方向和铺层厚度进行了优化设计。采用Box-Behnken方法进行方案设计,以纤维金属层合板各铺层相对厚度比为设计变量,以结构的比吸能为设计目标,根据设计的方案进行参数化建模获取样本点,在对设计样本进行方差分析和参数估计的基础上,建立了结构比吸能的响应面模型并验证了其精确度。采用遗传算法对响应面方程进行寻优分析,通过显式动力学计算程序ABAQUS/Explicit验证优化效果。最终,在最优的铺层方案下,层合板的质量减小了11.70%,能量吸收增加了19.40%,抗弹性能显著提升。Abstract: Fiber-metal laminates are highly designable due to the characteristics of their constituent materials and laminate structure. They have the characteristics of anisotropy, large interface differences, and flexible design. Optimizing the design of fiber-metal laminates is of great significance to the enhancement of its mechanical properties and weight reduction. In order to improve the ballistic performance of fiber-metal laminates, of which the layer direction and layer thickness are optimized based on the response surface analysis method. For layup direction optimization, several layup directions are designed based on the corresponding principles according to the composite material layup optimization design requirements, and the energy absorptions of the corresponding structures are calculated, respectively, then the design plan for the better layup direction is screened out. For the optimization of ply thickness, the relative thickness ratio of each ply of the fiber-metal laminate is used as the design variable, and the specific energy absorption of the structure is the design goal. The Box-Behnken method is used to design the experiment. According to the test plan, the explicit dynamic calculation program ABAQUS/Explicit is used for parametric modeling to obtain test sample points, and the design test samples are analyzed by using variance analysis and parameter estimation, and the response surface model of structural specific energy absorption (SEA) is established. The errors between the experimental values and the predicted values are compared, and the model can be used for prediction; the genetic algorithm is used to optimize the obtained response surface equation, and the optimization effect is verified by ABAQUS/Explicit. The optimization result shows that the accuracy of the obtained response surface model is high. Under the premise of not increasing the thickness and weight of the laminate, the best layup plan is finally obtained, which improves the energy absorption capacity of the laminate. Finally, the mass of laminates decreases by 11.70% and the energy absorption increases by 19.40% under the optimal lamination scheme.
-
Key words:
- laminate /
- response surface method /
- genetic algorithm /
- composite material
-
纤维金属层合板是由纤维增强复合材料与金属或金属合金交替层铺而成的新型复合结构,由于其具有优异的强度、刚度、耐腐蚀、耐疲劳以及冲击吸能等性能,在航天航空、汽车以及船舶等行业都存在巨大的应用潜力[1-3]。纤维增强复合材料本身具有各项异性、界面差异性较大、设计灵活多变等特点,且金属层和纤维层的厚度以及排列顺序也会对层合板的抗弹吸能特性产生一定影响。因此,开展纤维金属层合板优化设计研究对其力学性能增强和轻量化具有重要意义。
近年来,学者们对于复合材料结构的设计和优化开展了大量研究工作,通过对复合材料的铺层方案进行优化设计,显著减小了结构质量,且制造工艺和结构强度也可以满足要求[4-6];通过采用遗传算法对复合材料层间黏结参数进行优化设计,可显著提升其拉伸和剪切强度[7-8]。复合材料结构动态响应和吸能等特性优化也受到了研究人员的关注,Mostofi等[9]采用响应面法对聚脲-铝层合板结构进行优化设计,得到了冲击载荷作用下层合板中心永久变形最小时的铝板厚度、聚脲层厚度和总预爆压力,并验证了优化方案的合理性;王振等[10]基于响应面模型开展了复合材料锥形圆管结构的优化设计,优化后的单向碳纤维复合材料圆锥管的比吸能提高了15.6%,初始峰值载荷降低了51.8%,质量减小了22.4%;顾杰斐等[11]基于变刚度层合板的抗屈曲机制建立了一种铺层优化设计方法,通过遗传算法得到了初步最优的铺层方案,通过与原方案对比,最优铺层方案的屈曲载荷提高了25.7%;冯振宇等[12]确定了不同复合材料薄壁结构设计参数相关的响应面方程,采用序列二次规划算法对模型进行优化求解并验证了该方法的适用性。Cutolo等[13]结合理论分析和实验测试,以每层纤维方向为设计变量,以特定边界条件下的应变能为优化目标,得到了多层纤维的最佳排列,改善了结构的应力分布,提高了抗屈曲能力。Ghashochi-Bargh等[14]采用粒子群算法和有限条法对3种不同边界条件的纤维金属层合板的固有频率响应进行了优化,以铺层角度、层数、面板长宽比、金属板厚为设计变量,得到了使纤维金属层合板固有频率最大的设计方案。Arhore等[15]利用多层损伤有限元模型,基于遗传算法优化的方法研究了多层结构对纤维金属层合板冲击性能的影响,以每一铺层的厚度和纤维方向为设计变量对结构的吸能效果进行优化,得到了吸能效果良好的设计方案。
从目前已开展的研究工作来看,纤维金属层合板的优化目标多以减小结构质量为主。层合板在受到弹体高速冲击时的抗弹吸能效果需给予考虑和关注。响应面分析法(response surface methodology,RSM)在对结构耐撞性、结构可靠性等方面的优化应用研究较多,而该方法在高速冲击下结构吸能优化方面开展的研究较少。本文中,以提升纤维金属层合板的抗弹吸能性能为目标,采用响应面分析法,分别以纤维铺层方向、金属层和复合材料层厚度为设计变量,对纤维金属层合板进行优化设计,以期为纤维金属层合板的抗弹设计提供一定参考。
1. 弹体冲击纤维金属层合板过程的数值模拟
1.1 有限元模型参数
采用平面几何尺寸为
100 mm×100 mm 的纤维金属层合板作为研究对象,纤维增强材料为玻璃纤维增强聚丙烯(glass fiber reinforced polypropylene, GFPP),如图1所示。分别定义下层Al、中间层Al、上层Al(靠近弹体一侧)的厚度为t1 、t2 、t3 以及纤维层厚度为t4 。材料的各项性能参数[16]见表1~2。表中:ρ为密度,E1、E2、E3分别为纤维方向弹性模量、横向弹性模量和厚度方向弹性模量,μ12、μ13、μ23分别为纤维面内泊松比、纤维和厚度方向面内泊松比、横向和厚度方向面内的泊松比,G12、G13、G23分别为纤维面内剪切模量、纤维和厚度方向面内剪切模量、横向和厚度方向面内的剪切模量,XT、XC分别为纤维方向的拉伸强度和压缩强度,YT、YC分别为横向拉伸强度和横向压缩强度,S12、S13、S23分别为纤维面内剪切强度、纤维和厚度方向面内剪切强度、横向和厚度方向面内的剪切强度。铺层形式为A3G23,即铝合金薄板的数量为3,每层厚为0.5 mm,纤维增强材料叠合块的数量为2,每块厚度为1 mm,每个纤维增强材料叠合块中的GFPP层数为3。优化前的层合板总质量为78.70 g。弹头直径为10 mm,弹体长15 mm,初始速度为200 m/s。表 1 热塑性纤维增强材料的弹性参数Table 1. Elastic parameters of thermoplastic fiber reinforced materialsρ/(kg∙m−3) E1/GPa E2/GPa E3/GPa μ12 μ13 μ23 G12/GPa G13/GPa G23/GPa 1 800 13 13 4.8 0.1 0.3 0.3 1.72 1.69 1.69 表 2 热塑性纤维增强材料的强度参数Table 2. Strength parameters of thermoplastic fiber reinforced materialsXT/MPa XC/MPa YT/MPa YC/MPa S12/MPa S13/MPa S23/MPa 300 200 300 200 120 120 120 纤维增强材料的损伤模式主要有纤维拉伸变形、断裂、纤维压缩破坏以及纤维层间分层失效等。为合理描述纤维层合板的破坏,采用改进的3D-Hashin失效准则,具体形式如下。
(1)纤维拉伸破坏(
σ11≥0 ):FTf=(σ11XT)2+αS2C(σ212+σ213)≥1 (1) (2)纤维压缩破坏(
σ11<0 ):FCf=(σ11XC)2≥1 (2) (3)基体拉伸破坏(
σ22+σ33≥0 ):FTm=(σ22+σ33YT)2+(σ223−σ22σ33)S2T+(σ212+σ213)S2C≥1 (3) (4)基体压缩破坏(
σ22+σ33<0 ):FCm=(σ22+σ332S12)2+[(YC2S12)2−1]σ22+σ33YC+(σ223−σ22σ33)S223+σ212S212+σ213S213≥1 (4) 式中:
FTf 、FCf 、FTm 、FCm 分别为相应损伤模式下的损伤阈值;σ 下标中1、2、3分别表示纤维方向、横向和厚度方向,σ11 、σ12 、σ13 、σ22 、σ23 、σ33 为对应方向下的应力; α 为剪切修正因子; ST、 SC分别为横向剪切强度和纤维方向剪切强度。将以上改进的3D-Hashin失效准则通过用户子程序编译实现,接入ABAQUS/Explicit的计算程序中。网格收敛性分析如表3所示,随着网格尺寸减小,吸能逐渐收敛,但计算耗时也随之增加。因此,在保证模型计算精度的前提下,采用渐变网格进行划分,尺寸为1.0 mm×1.0 mm×0.3 mm,层合板与弹体接触区域进行细化,最小网格尺寸为0.4 mm×0.4 mm×0.3 mm。纤维金属层合板的金属层和纤维层均采用八节点线性六面体单元进行离散,单元类型为C3D8R,各材料层的应力应变数据可以通过该单元准确获取;GFPP材料层在厚度方向上采用3个单元进行离散;界面间的连接与分层采用八节点的内聚力cohesive单元模拟,cohesive单元损伤失效的临界条件用二次名义应力准则来描述,cohesive材料参数[16]如表4所示。表中:ρc为cohesive单元的材料密度,
En 、Es 、Et 分别为cohesive单元法向和2个切向的弹性模量,s(0)n 、s(0)s 、s(0)t 分别为cohesive单元的法向和2个切向的应力,G(0)n 、G(0)s 、G(0)t 分别为cohesive单元的法向和2个切向失效的临界断裂能量。弹体使用八节点线性六面体实体单元建模,单元类型为C3D8R,网格尺寸为1.0 mm×1.0 mm×1.0 mm,弹体设置为刚性材料。弹体和靶体之间采用通用接触,层合板四周完全固定,弹头冲击方向与层合板垂直。优化前的层合板有限元模型如图2所示。表 3 网格收敛性分析Table 3. Analysis of grid convergence网格尺寸/mm 网格数量 吸能/J 相对偏差/% 2.0 43 908 90.72 34.70 1.0 171 408 69.42 3.07 0.5 680 000 67.35 0 1.2 实验验证及结果分析
为验证所采用的数值模拟方法的准确性,以纤维金属层合板的高速冲击实验为研究对象[2],对3Al(6-O)/2G型纤维金属层合板的穿甲实验进行模拟分析,实验中采用高压气枪对弹体加速,层合板前设置测速示波器,层合板后设置2台高速摄影仪,分别用于测量弹体的初速度和剩余速度,进而得到层合板的吸能情况,实验布置如图3所示。将实验与数值模拟结果进行对比分析,如表5所示。可以看到,数值模拟所得弹体剩余速度与实验结果吻合较好,各工况模拟结果与实验结果的相对误差均在5%以内。对于3Al(6-O)/2G型纤维金属层合板,实验及数值模拟中弹体侵彻后的剩余速度与初速度的拟合公式为:
表 5 弹体剩余速度实验结果与数值模拟结果对比Table 5. Comparison of projectile residual velocities between experimental and numerical results实验工况 实验初速/(m·s−1) 实验剩余速度/(m·s−1) 模拟剩余速度/(m·s−1) 剩余速度相对误差/% 3Al(6-O)/2G-1 143 109 107.9 –1.0 3Al(6-O)/2G-2 172 125 127.6 2.1 3Al(6-O)/2G-3 195 150 151.1 0.7 3Al(6-O)/2G-4 214 181 172.1 –4.9 3Al(6-O)/2G-5 252 216 211.5 –2.1 vr=a(vbi−vbbl)1/b (5) 式中:vr为弹体剩余速度,vi为弹体初始入射速度,vbl为层合板弹道极限速度,a和b为拟合参数。
所得弹道极限速度为118 m/s,如图4所示。以弹体入射速度为172 m/s的工况为例,实验与数值模拟所得纤维金属层合板破坏形貌如图5~6所示,纤维金属层合板中部分纤维发生拉伸断裂,铝合金背板产生大范围的塑性变形,进一步撕裂形成花瓣状并造成穿孔,铝合金与纤维之间的分层主要分布在冲击附近区域。实验以及数值模拟中层合板的变形模式和穿孔形貌均吻合较好。通过以上对比验证,说明本文中的数值模拟方法具有较高的准确性,可进一步用于层合板优化方案的计算分析。
2. 纤维金属层合板吸能优化分析
纤维金属层合板的纤维层具有设计灵活多变、材料各向异性等特点,因而可以通过改变材料相应的结构参数来调整其性能。层合板结构的性能设计可通过选择合适的铺设角度、各铺层的叠放顺序、各铺层厚度等方面展开。在铺层设计时,通常还需要综合考虑强度、刚度以及工艺制备等方面的要求。
2.1 纤维铺层方向优化分析
根据复合材料铺层优化设计方案的计算要求,优化后的铺层应当满足以下基本原则[17]。
(1)考虑设计和生产制造的工作量,在满足受力的前提下,铺层方向数应该尽量少。工程上常采用0°、±45°、90°的标准铺层,以降低制造加工的复杂性。
(2)取向相同的铺层叠放不能超过2层。
(3)上下两部分复合层铺设方向关于中间金属层均衡对称,防止拉-剪、拉-弯耦合引起固化后的翘曲变形。
(4)铺层取向按承载选取原则,铺层轴线应与内力拉压方向一致,最大限度地利用纤维轴向具有高强度和高刚度的特性。其中,0°为主方向,主要承受纵向载荷;90°有利于调节泊松比和改善横向强度;
±45∘ 用来承受剪切载荷。(5)对于冲击载荷区的设计原则,需配置一定数量的与载荷方向成
±45∘ 的单层,以便将集中载荷扩散。表面纤维应均布于各个方向,使相邻层夹角尽可能小,目的是防止基体受载和减少分层。基于上述原则,对纤维铺层方向进行方案设计,如表6所示。分别对12组铺层方案进行参数化建模计算。后续处理中分别提取各铺层方案中弹体的动能时程曲线,如图7所示。
表 6 纤维金属层合板铺层方案Table 6. Layer schemes of fiber metal laminates铺层方案 铺层方向 铺层方案 铺层方向 1 Al/45°/90°/0°/Al/0°/90°/45°/Al 7 Al/90°/0°/–45°/Al/–45°/0°/90°/Al 2 Al/45°/90°/–45°/Al/–45°/90°/45°/Al 8 Al/90°/45°/0°/Al/0°/45°/90°/Al 3 Al/45°/0°/90°/Al/90°/0°/45°/Al 9 Al/90°/45°/–45°/Al/–45°/45°/90°/Al 4 Al/45°/0°/–45°/Al/–45°/0°/45°/Al 10 Al/90°/–45°/0°/Al/0°/–45°/90°/Al 5 Al/45°/–45°/0°/Al/0°/–45°/45°/Al 11 Al/0°/45°/–45°/Al/–45°/45°/0°/Al 6 Al/45°/–45°/90°/Al/90°/–45°/45°/Al 12 Al/0°/90°/–45°/Al/–45°/90°/0°/Al 图8中直观展示了不同铺层方案的层合板吸能效果。其中铺层方案0为优化前的层合板铺层方案。在上述12种铺层方案下,铺层方案1的抗弹吸能效果最好,因此在该铺层方案的基础上继续优化。
2.2 层合板铺层厚度优化分析
根据2.1节中层合板铺层方向Al/45°/90°/0°/Al/0°/90°/45°/Al,采用响应面分析法对结构进行优化设计。在响应面最优点附近,用二阶模型来逼近响应面,二阶模型表示为:
Y=α0+k∑i=1αixi+k∑i=1k∑j≥iαijxixj+δ (6) 式中:
Y 为目标响应,xi 为主要设计因子,α0 、αi 、αij 为系数,δ 为误差。二阶响应面设计过程中,常采用中心复合设计(central composite design, CCD)和Box-Behnken设计(Box-Behnken design, BBD)方法进行实验设计,利用数值计算模型进行模拟采样得到若干样本点。通过方差分析建立响应面模型并进行误差分析和参数检验,验证模型的准确性,若模型精度不佳,可新增设计点,通过数值计算模型获得更多样本点,再次验证模型的准确性,直至响应面的模型和优化设计结果达到理想。响应面优化步骤如图9所示。如图1所示,根据复合材料铺层优化设计要求,2层纤维增强材料叠合块的厚度取值相同。结合工程实际,铝薄板厚度取值在0.10~0.70 mm,优化前层合板总厚度
t0 为3.50 mm,纤维层厚度取值范围为0.70~1.60 mm。定义相对厚度比αi=ti/t0(i=1,2,3,4) ,比吸能Q表示为:Q=E0−Erm (7) 式中:
E0 为弹体初始动能,Er 为弹体剩余动能,m 为层合板质量。本文中,以α1 、α2 、α3 、α4 为设计因子,比吸能Q为目标响应进行响应面设计。各因子设计水平如表7所示,对目标层合板进行BBD实验设计,编码后实验设计方案,因子数为4,实验样本数为27。使用ABAQUS分别对27个样本进行参数化建模并计算,后处理中采集各样本的吸能和质量计算比吸能Q。实验设计方案和比吸能数据如表8所示。使用MATLAB对实验样本进行响应面设计,根据方差分析对模型进行优化,方差分析结果如表9所示。其中,模型P<0.000 1,模型极显著,决定系数
R2 接近1,与调整决定系数R2adj 相差不大,模型精度较高,可用于预测。4个因子的P均小于0.05,说明4个因子对模型影响显著,即对比吸能Q的影响明显。在各因子交互项中,α1×α4 、α3×α4 之间影响显著,相互作用明显,响应面曲线和等高线分别如图10~11所示。α1×α2 、α1×α3 、α2×α4 之间相互作用不显著。因此,得到的二阶响应面模型为:表 7 设计因子水平Table 7. Design factor levels设计因子 低水平 高水平 α1 0.029 0.200 α2 0.029 0.200 α3 0.029 0.200 α4 0.200 0.457 表 8 BBD实验设计Table 8. Experimental schemes designed by the BBD method实验方案 α1 α2 α3 α4 Q/(J⋅kg−1) Qpre/(J⋅kg−1) 1 –1 0 0 –1 982.58 983.58 2 0 0 –1 1 820.35 815.75 3 1 0 0 –1 1 032.25 1 041.37 4 0 0 0 0 799.74 799.74 5 0 –1 0 –1 979.84 965.03 6 –1 0 0 1 675.37 671.43 7 –1 1 0 0 836.94 847.07 8 1 –1 0 0 846.44 859.65 9 0 0 –1 –1 1 135.93 1 143.95 10 –1 0 1 0 780.02 754.93 11 1 0 –1 0 1 015.36 1 002.85 12 0 –1 –1 0 914.80 912.54 13 –1 –1 0 0 730.39 746.48 14 0 1 0 1 808.02 808.85 15 1 1 0 0 960.88 960.23 16 1 0 1 0 881.44 868.10 17 0 0 0 0 799.74 799.74 18 0 0 1 –1 913.77 937.77 19 0 0 0 0 799.74 799.74 20 0 1 –1 0 976.15 985.71 21 0 0 1 1 741.05 752.42 22 1 0 0 1 835.80 839.97 23 –1 0 –1 0 887.89 889.69 24 0 1 0 –1 1 092.94 1 065.62 25 0 –1 0 1 716.09 708.26 26 0 –1 1 0 754.74 750.36 27 0 1 1 0 870.92 878.37 表 9 方差分析和参数估计Table 9. Analysis of variance and parameter estimation方差来源 平方和 自由度 均方 F P 模型 366 000.00 11 33 247.56 156.55 < 0.000 1 α1 38 416.01 1 38 416.01 180.89 < 0.000 1 α2 30 354.44 1 30 354.44 142.93 < 0.000 1 α3 54 478.75 1 54 478.75 256.52 < 0.000 1 α4 198 000.00 1 198 000.00 931.36 < 0.000 1 α1×α4 3 066.78 1 3 066.78 14.44 0.001 4 α2×α3 751.72 1 751.72 3.54 0.077 2 α3×α4 5 101.74 1 4 179.89 24.02 0.000 1 α1×α1 4 179.89 1 5 170.59 19.68 0.000 4 α2×α2 5 170.59 1 18 752.36 24.35 0.000 1 α3×α3 18 752.36 1 22 551.08 88.30 < 0.000 1 α4×α4 22 551.08 1 3 066.78 106.19 < 0.000 1 残差 3 610.39 17 212.38 总和 370 000.00 28 R2 0.99 R2adj 0.98 表 10 遗传算法优化结果Table 10. Genetic algorithm optimization results实验方案 α1 α2 α3 α4 Q/(J⋅kg−1) 优化 0.183 0.198 0.041 0.217 1186.0 Q=1891.58−955.20α1−504.85α2−3736.90α3−4000.03α4+2512.54α1α4+1865.91α2α3+3240.65α3α4+3455.19α21+3842.90α22+7318.41α23+3566.89α24 (8) 本文中,利用响应面分析法分析了4个因子之间的相互作用,所得到的响应面方程一定程度上可用于预测模型的比吸能,27个样本点的预测值
Qpre 如表8所示。4个因子对模型比吸能Q的影响程度最高为α4 ,其次为α3 ,再次为α1 ,影响程度最低为α2 。2.3 遗传算法寻优分析
根据2.2中所得的响应面方程(式(8)),采用遗传算法进行寻优分析。在保证优化后层合板的总厚度
t 和总质量m 不超过优化前层合板的前提下,尽可能改善层合板的吸能效果。因此,建立层合板吸能优化数学模型和约束条件:f=min(−Q){0.029≤α1≤0.2000.029≤α2≤0.2000.029≤α3≤0.2000.200≤α4≤0.457t≤3.50mmm≤78.70g (9) 采用遗传算法接力进化,通过将上次进化结束后得到的最终种群作为本次输入的初始种群来实现,初始种群随机性降低,趋向稳定,所得结果准确性更高。初次进化代数100,种群规模100,最优个体系数为0.3,停止代数300,适应度函数偏差为
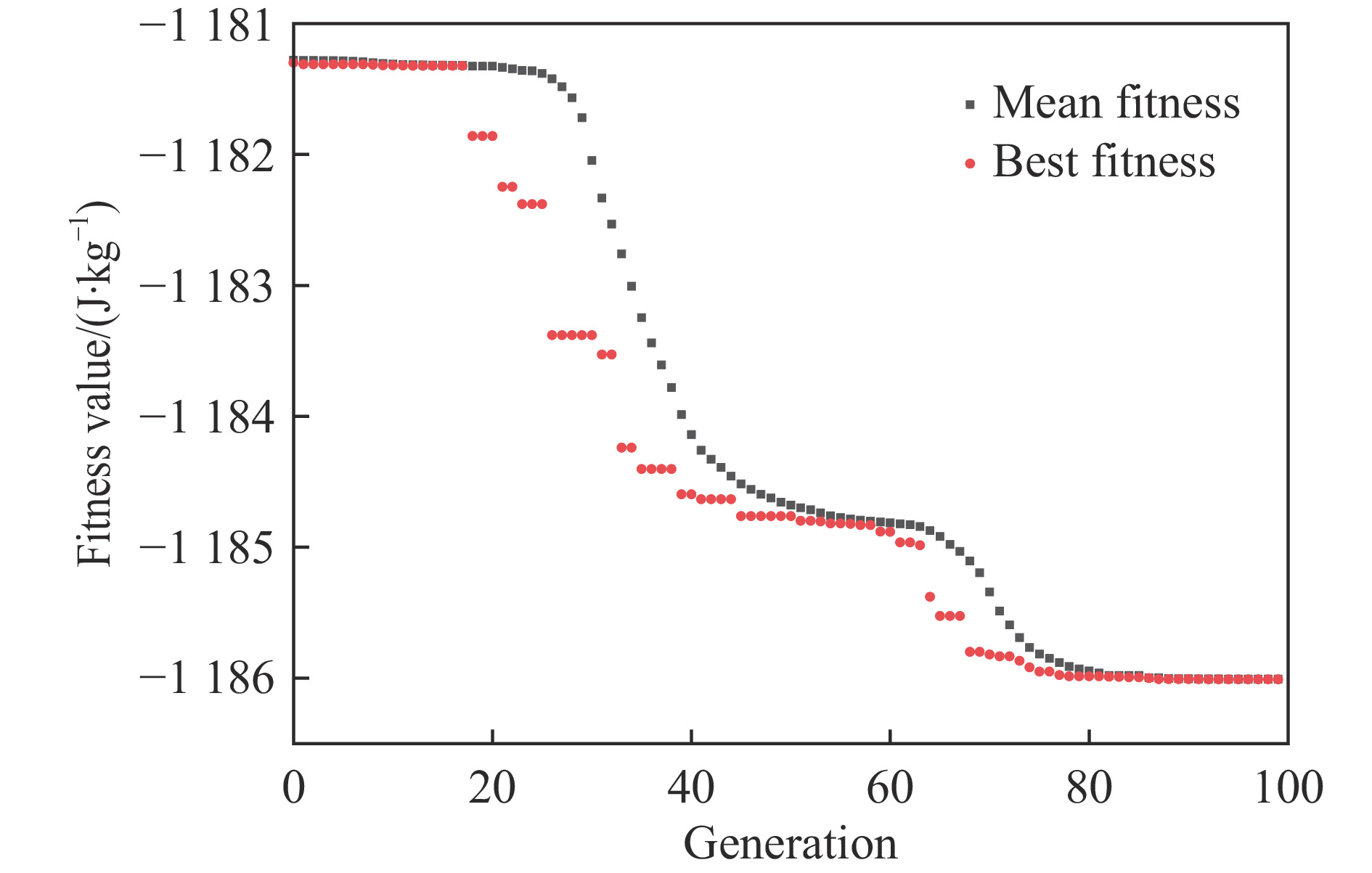
1×10−100 ;初次进化的最终种群作为第2次接力进化初始种群输入,接力进化代数100。遗传算法寻优过程如图12所示,最终所得优化方案如表10所示。当层合板下层Al厚度t1 =0.642 mm,中间层Al厚度t2 =0.693 mm,下层Al厚度t3 =0.144 mm,纤维增强材料厚度t4 =0.760 mm时,模型比吸能Q最大,为1 186.0 J/kg。2.4 优化结果验证
根据2.3节中的优化结果,建立层合板优化方案模型并开展计算分析,优化后的层合板有限元模型如图13所示。层合板背面板和前面板的最终破坏形式分别如图14~15所示,层合板产生由弹体圆周向四周扩展的裂纹,为花瓣状。图16为弹体侵彻层合板过程中各时刻层合板的形态。0.05 ms时,弹体穿透上层铝薄板,中间层铝板和下层铝板被高速运动的弹体挤压而向外膨胀,发生大变形,纤维层和金属层间发生分层现象。0.10 ms时,层合板背面板受到拉应力作用,下层铝板出现裂口,随着应力的不断升高,单元不断发生失效和脱落,脱落的单元碎片被弹体的剩余动能冲出靶板背面,随之一起运动。下层铝和纤维层分层范围进一步扩大。0.15 ms时,弹体即将穿透整个层合板,随着侵彻的深入,层合板裂口不断增大,部分纤维被拔出,层间分层现象更明显。0.20 ms时,随着靶板材料的破坏和失效,靶板对弹体冲击的抵抗力逐渐减小。最终弹体以一定的剩余速度完全穿透层合板,弹体速度逐渐趋于平稳,惯性力作用后层合板破口面积趋于稳定。
计算得到优化后的层合板比吸能
Qopt 为1 192.66 J/kg,与遗传算法求得的优化解误差为0.56%,说明响应面设计方法是可行的。如表11所示,优化后的层合板总厚度t =3 mm,总质量为69.50 g,抗弹吸能为82.89 J。与初始方案层合板相比,厚度减小了14.30 %,质量减小了11.70 %,吸能增加了19.40 %,优化前后层合板吸能效果对比如图17所示。表 11 优化前后层合板主要参数Table 11. The main parameters of original and optimized laminates层合板方案 t1/mm t2/mm t3/mm t4/mm t0/mm m/g Q/(J⋅kg−1) 原方案 0.50 0.50 0.50 1.00 3.50 78.70 882.12 优化后 0.64 0.69 0.14 0.76 3.00 69.50 1192.66 3. 结 论
利用ABAQUS进行参数化建模分析,结合多组有限元分析样本,通过响应面算法对铺层形式为A3G23的纤维金属层合板进行优化设计,得到以下结论。
(1)A3G23层合板在不同纤维铺层方向下的吸能效果略微存在差异。其中,当采取的铺层方向为Al/45°/90°/0°/Al/0°/90°/45°/Al时,层合板吸能效果最佳。比较12种不同铺层顺序方案下的层合板吸能效果,不难发现,层合板最外层铺层方向优先采取45°,层合板最内层铺层方向优先采取0°。
(2)A3G23层合板中金属层和纤维层的厚度分布对吸能效果有显著影响。根据响应面分析结果可知,4个因子中,纤维层厚度
α4 对比吸能Q的影响程度最高,上层铝厚度α3 影响程度较其他因子低。就因子间相互作用而言,上层铝厚度α3 和下层铝厚度α1 分别与纤维层厚度α4 之间的相互作用显著。当纤维厚度α4 取值在中、低、高任一水平时,下层铝厚度α1 增大会提高层合板的比吸能Q,上层铝厚度α3 增大会显著降低层合板的比吸能Q。(3)较薄的上层铝板、较厚的中间层和下层铝板可以提高抗弹性能,优化后的层合板在弹体的冲击下,下层铝板和纤维间分层更明显,也进一步体现更大吸能的结果。
(4)优化后的层合板比吸能为1 192.66 J/kg,较优化前的抗弹性能有明显提高。层合板厚度减小了14.30 %,质量减小了11.70 %,吸能增加了19.40 %。响应面优化模型比较理想,可为A3G23层合板的铺层设计提供一定依据。
-
表 1 热塑性纤维增强材料的弹性参数
Table 1. Elastic parameters of thermoplastic fiber reinforced materials
ρ/(kg∙m−3) E1/GPa E2/GPa E3/GPa μ12 μ13 μ23 G12/GPa G13/GPa G23/GPa 1 800 13 13 4.8 0.1 0.3 0.3 1.72 1.69 1.69 表 2 热塑性纤维增强材料的强度参数
Table 2. Strength parameters of thermoplastic fiber reinforced materials
XT/MPa XC/MPa YT/MPa YC/MPa S12/MPa S13/MPa S23/MPa 300 200 300 200 120 120 120 表 3 网格收敛性分析
Table 3. Analysis of grid convergence
网格尺寸/mm 网格数量 吸能/J 相对偏差/% 2.0 43 908 90.72 34.70 1.0 171 408 69.42 3.07 0.5 680 000 67.35 0 ρc/(kg∙m−3) En/GPa Es/GPa Et/GPa s(0)n/MPa s(0)s/MPa s(0)t/MPa G(0)n/(J∙m−2) G(0)s/(J∙m−2) G(0)t/(J∙m−2) 900 2.05 0.72 0.72 70 100 100 300 700 700 表 5 弹体剩余速度实验结果与数值模拟结果对比
Table 5. Comparison of projectile residual velocities between experimental and numerical results
实验工况 实验初速/(m·s−1) 实验剩余速度/(m·s−1) 模拟剩余速度/(m·s−1) 剩余速度相对误差/% 3Al(6-O)/2G-1 143 109 107.9 –1.0 3Al(6-O)/2G-2 172 125 127.6 2.1 3Al(6-O)/2G-3 195 150 151.1 0.7 3Al(6-O)/2G-4 214 181 172.1 –4.9 3Al(6-O)/2G-5 252 216 211.5 –2.1 表 6 纤维金属层合板铺层方案
Table 6. Layer schemes of fiber metal laminates
铺层方案 铺层方向 铺层方案 铺层方向 1 Al/45°/90°/0°/Al/0°/90°/45°/Al 7 Al/90°/0°/–45°/Al/–45°/0°/90°/Al 2 Al/45°/90°/–45°/Al/–45°/90°/45°/Al 8 Al/90°/45°/0°/Al/0°/45°/90°/Al 3 Al/45°/0°/90°/Al/90°/0°/45°/Al 9 Al/90°/45°/–45°/Al/–45°/45°/90°/Al 4 Al/45°/0°/–45°/Al/–45°/0°/45°/Al 10 Al/90°/–45°/0°/Al/0°/–45°/90°/Al 5 Al/45°/–45°/0°/Al/0°/–45°/45°/Al 11 Al/0°/45°/–45°/Al/–45°/45°/0°/Al 6 Al/45°/–45°/90°/Al/90°/–45°/45°/Al 12 Al/0°/90°/–45°/Al/–45°/90°/0°/Al 表 7 设计因子水平
Table 7. Design factor levels
设计因子 低水平 高水平 α1 0.029 0.200 α2 0.029 0.200 α3 0.029 0.200 α4 0.200 0.457 表 8 BBD实验设计
Table 8. Experimental schemes designed by the BBD method
实验方案 α1 α2 α3 α4 Q/(J⋅kg−1) Qpre/(J⋅kg−1) 1 –1 0 0 –1 982.58 983.58 2 0 0 –1 1 820.35 815.75 3 1 0 0 –1 1 032.25 1 041.37 4 0 0 0 0 799.74 799.74 5 0 –1 0 –1 979.84 965.03 6 –1 0 0 1 675.37 671.43 7 –1 1 0 0 836.94 847.07 8 1 –1 0 0 846.44 859.65 9 0 0 –1 –1 1 135.93 1 143.95 10 –1 0 1 0 780.02 754.93 11 1 0 –1 0 1 015.36 1 002.85 12 0 –1 –1 0 914.80 912.54 13 –1 –1 0 0 730.39 746.48 14 0 1 0 1 808.02 808.85 15 1 1 0 0 960.88 960.23 16 1 0 1 0 881.44 868.10 17 0 0 0 0 799.74 799.74 18 0 0 1 –1 913.77 937.77 19 0 0 0 0 799.74 799.74 20 0 1 –1 0 976.15 985.71 21 0 0 1 1 741.05 752.42 22 1 0 0 1 835.80 839.97 23 –1 0 –1 0 887.89 889.69 24 0 1 0 –1 1 092.94 1 065.62 25 0 –1 0 1 716.09 708.26 26 0 –1 1 0 754.74 750.36 27 0 1 1 0 870.92 878.37 表 9 方差分析和参数估计
Table 9. Analysis of variance and parameter estimation
方差来源 平方和 自由度 均方 F P 模型 366 000.00 11 33 247.56 156.55 < 0.000 1 α1 38 416.01 1 38 416.01 180.89 < 0.000 1 α2 30 354.44 1 30 354.44 142.93 < 0.000 1 α3 54 478.75 1 54 478.75 256.52 < 0.000 1 α4 198 000.00 1 198 000.00 931.36 < 0.000 1 α1×α4 3 066.78 1 3 066.78 14.44 0.001 4 α2×α3 751.72 1 751.72 3.54 0.077 2 α3×α4 5 101.74 1 4 179.89 24.02 0.000 1 α1×α1 4 179.89 1 5 170.59 19.68 0.000 4 α2×α2 5 170.59 1 18 752.36 24.35 0.000 1 α3×α3 18 752.36 1 22 551.08 88.30 < 0.000 1 α4×α4 22 551.08 1 3 066.78 106.19 < 0.000 1 残差 3 610.39 17 212.38 总和 370 000.00 28 R2 0.99 R2adj 0.98 表 10 遗传算法优化结果
Table 10. Genetic algorithm optimization results
实验方案 α1 α2 α3 α4 Q/(J⋅kg−1) 优化 0.183 0.198 0.041 0.217 1186.0 表 11 优化前后层合板主要参数
Table 11. The main parameters of original and optimized laminates
层合板方案 t1/mm t2/mm t3/mm t4/mm t0/mm m/g Q/(J⋅kg−1) 原方案 0.50 0.50 0.50 1.00 3.50 78.70 882.12 优化后 0.64 0.69 0.14 0.76 3.00 69.50 1192.66 -
[1] REYES VILLANUEVA G, CANTWELL W J. The high velocity impact response of composite and FML-reinforced sandwich structures [J]. Composites Science and Technology, 2004, 64(1): 35–54. DOI: 10.1016/S0266-3538(03)00197-0. [2] KABOGLU C, MOHAGHEGHIAN I, ZHOU J, et al. High-velocity impact deformation and perforation of fibre metal laminates [J]. Journal of Materials Science, 2018, 53: 4209–4228. DOI: 10.1007/s10853-017-1871-2. [3] SANTIAGO R, CANTWELL W, ALVES M. Impact on thermoplastic fibre-metal laminates: experimental observations [J]. Composite Structures, 2017, 159: 800–817. DOI: 10.1006/j.compstruct.2016.10.011. [4] 曾漾, 周俊, 沈志远, 等. 基于响应面法的复合材料舱壁结构优化设计 [J]. 重庆大学学报, 2020, 43(6): 82–89. DOI: 10.11835/j.issn.1000-582X.2020.292.ZENG Y, ZHOU J, SHEN Z Y, et al. Optimization design of composite bulkhead structure based on response surface method [J]. Journal of Chongqing University, 2020, 43(6): 82–89. DOI: 10.11835/j.issn.1000-582X.2020.292. [5] 周晓松, 梅志远. 舰船复合材料夹层板架结构的分级递进优化设计方法 [J]. 中国舰船研究, 2014, 9(4): 63–69. DOI: 10.3969/j.issn.1673-3185.2014.04.010.ZHOU X S, MEI Z Y. Hierarchical progressive optimum design method for composite stiffened panels of warships [J]. Chinese Journal of Ship Research, 2014, 9(4): 63–69. DOI: 10.3969/j.issn.1673-3185.2014.04.010. [6] 刘伟先, 穆雪峰, 曾果. 复合材料蜂窝夹层进气道结构优化设计方法 [J]. 南京航空航天大学学报, 2018, 50(1): 86–90. DOI: 10.16356/j.1005-2615.2018.01.012.LIU W X, MU X F, ZENG G. Optimization design of composite honeycomb sandlayer inlet structure [J]. Journal of Nanjing University of Aeronautics and Astronautics, 2018, 50(1): 86–90. DOI: 10.16356/j.1005-2615.2018.01.012. [7] 胡春幸, 侯玉亮, 铁瑛, 等. 基于遗传算法的碳纤维增强树脂复合材料层合板单搭胶接结构的多目标优化 [J]. 复合材料学报, 2021, 38(6): 1847–1858. DOI: 10.13801/j.cnki.fhclxb.20200824.001.HU C X, HOU Y L, TIE Y, et al. Multi-objective optimization of adhesively bonded single-lap joints of carbon fiber reinforced polymer laminates based on genetic algorithm [J]. Acta Materiae Compositae Sinica, 2021, 38(6): 1847–1858. DOI: 10.13801/j.cnki.fhclxb.20200824.001. [8] 胡春幸, 侯玉亮, 铁瑛, 等. 不同胶接参数对CFRP层合板单搭胶接结构强度的影响及优化设计 [J]. 机械工程学报, 2021, 57(8): 154–165. DOI: 10.3901/JME.2021.08.154.HU C X, HOU Y L, TIE Y, et al. Influence of different bonding parameters on the strength of CFRP laminates with single lap bonding structure and optimization [J]. Journal of Mechanical Engineering, 2021, 57(8): 154–165. DOI: 10.3901/JME.2021.08.154. [9] MOSTOFI T M, SAYAH-BADKHOR M, REZASEFAT M, et al. Gas mixture detonation load on polyurea-coated aluminum plates [J]. Thin-Walled Structures, 2020, 155: 106851. DOI: 10.1016/j.tws.2020.106851. [10] 王振, 宋凯, 朱国华, 等. 单向碳纤维复合材料锥管轴向吸能特性研究 [J]. 振动与冲击, 2018, 37(7): 172–178. DOI: 10.13465/j.cnki.jvs.2018.07.026.WANG Z, SONG K, ZHU G H, et al. Axial energy absorption characteristics of unidirectional carbon-fiber composite cone tubes [J]. Journal of Vibration and Shock, 2018, 37(7): 172–178. DOI: 10.13465/j.cnki.jvs.2018.07.026. [11] 顾杰斐, 陈普会, 孔斌, 等. 考虑制造因素的变刚度层合板的抗屈曲铺层优化设计 [J]. 复合材料学报, 2018, 35(4): 866–875. DOI: 10.13801/j.cnki.fhclxb.20170705.001.GU J F, CHEN P H, KONG B, et al. Layup optimization for maximum buckling load of variable-stiffness laminates considering manufacturing factors [J]. Acta Materiae Compositae Sinica, 2018, 35(4): 866–875. DOI: 10.13801/j.cnki.fhclxb.20170705.001. [12] 冯振宇, 苏璇, 赵彦强, 等. 含概率不确定性的复合材料吸能结构优化设计方法研究 [J]. 振动与冲击, 2019, 38(11): 101–109. DOI: 10.13465/j.cnki.jvs.2019.11.016.FENG Z Y, SU X, ZHAO Y Q, et al. Optimization design method for energy-absorbing composite structure with probabilistic uncertainty [J]. Journal of Vibration and Shock, 2019, 38(11): 101–109. DOI: 10.13465/j.cnki.jvs.2019.11.016. [13] CUTOLO A, CAROTENUTO A R, PALUMBO S, et al. Stacking sequences in composite laminates through design optimization [J]. Meccanica, 2021, 56: 1555–1574. DOI: 10.1007/s11012-020-01233-y. [14] GHASHOCHI-BARGH H, SADR M H. PSO algorithm for fundamental frequency optimization of fiber metal laminated panels [J]. Structural Engineering and Mechanics, 2013, 47(5): 713–727. DOI: 10.12989/sem.2013.47.5.713. [15] ARHORE E G, YASAEE M, et al. Lay-up optimisation of fibre-metal laminates panels for maximum impact absorption [J]. Journal of Composite Materials, 2020, 54(29): 4591–4609. DOI: 10.1177/0021998320937396. [16] SITNIKOVA E, GUAN Z W, CANTWELL W J. The analysis of the ultimate blast failure modes in fibre metal laminates [J]. Composites Science and Technology, 2016, 135: 1–12. DOI: 10.1016/j.compscitech.2016.09.006. [17] 王耀先. 复合材料结构设计 [M]. 北京: 化学工业出版社, 2001. 期刊类型引用(6)
1. 高建村,王乐,胡守涛,孙谞,周尚勇,王涛. 不同磁性金属丝对丙烷爆炸反应抑制机理研究. 中国安全生产科学技术. 2020(07): 125-130 .  百度学术
百度学术2. 王连聪,梁运涛,罗海珠. 我国矿井热动力灾害理论研究进展与展望. 煤炭科学技术. 2018(07): 1-9 .  百度学术
百度学术3. 路长,刘洋,于子凯,潘荣锟,刘磊,滕飞. 四氟乙烷对甲烷/空气爆炸特性的影响. 化工进展. 2017(10): 3596-3603 .  百度学术
百度学术4. 徐永亮,王兰云,余明高,万少杰,梁栋林. 感应式荷电细水雾对瓦斯爆炸传播速度的影响. 中南大学学报(自然科学版). 2016(08): 2884-2890 .  百度学术
百度学术5. 余明高,梁栋林,徐永亮,郑凯,纪文涛. 荷电细水雾抑制瓦斯爆炸实验研究. 煤炭学报. 2014(11): 2232-2238 .  百度学术
百度学术6. 丁小勇,谭迎新,李媛,秦涧,许航. 管道倾斜角对瓦斯爆炸影响的实验研究. 消防科学与技术. 2012(12): 1269-1271 .  百度学术
百度学术其他类型引用(14)
-













 下载:
下载:

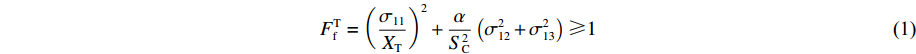









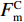













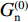


















































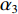



























 下载:
下载:
























 百度学术
百度学术