Modified discrete numerical model for reinforced concrete structures
-
摘要: 提出一种考虑粘结滑移效应的钢筋混凝土改进型分离式数值模型。在混合物理论基础上,该模型兼顾混凝土基体和钢筋的力学行为,且基于钢筋混凝土界面粘结滑移模型,获得了钢筋等效模型。改进型分离式数值模型由于对钢筋及其界面无显式离散要求,使得钢筋的运用完全独立于其几何形状,同时对混凝土网格没有约束,并且不增加计算成本,因此该模型可适用于钢筋混凝土宏观结构层面分析。通过钢筋混凝土构件-结构的爆炸实验,对改进型分离式数值模型进行层次化验证。对比结果表明,考虑粘结滑移效应的有限元模型能够更好地预测钢筋混凝土结构的宏观力学行为。Abstract: The interaction between rebar and concrete must always be considered to well describe and predict the mechanical behavior of reinforced concrete (RC) structures. A common way to model RC structures is by discrete reinforcements in finite element models where the discrete reinforcements imply that bond conditions between rebar and concrete are perfect. In order to take into account the bond-slip phenomenon, a modified discrete numerical model for RC structures is presented in this paper. The model is formulated within the framework of the mixture theory, considering two phases corresponding to the matrix concrete and the reinforcement bars and incorporating bond-slip effects to the stress-strain relation of the latter by considering the bond-slip model recommended by CEB-FIB. A nonlinear equivalent stress-strain relation for discrete steel bars is generated by incorporating the bond-slip strain to the strain of bars. Based on this model, a comprehensive parametric study is accomplished to obtain the influence of the parameters, including the strengths of the concrete and steel bars as well as the diameter of bars on the modified stress-strain relation of the discrete steel bars. In comparison to the traditional discrete numerical model where interface elements are generated via connecting the degrees of freedom of the bars and concrete meshing, the new model allows for the slipping of the steel bars without explicit discretization of the steel bars and the steel/concrete interfaces. This fact makes it attractive for numerical simulation of concrete structures at the macrostructural level. Using a code JUST-PANDA that is developed in-house, the model is verified by the explosion experiments at the component level and the structural level respectively. The comparisons with experimental results show that the new model can provide a more reliable prediction of the concrete structural behavior due to the consideration of the bond-slip effects in the stress-strain relation of the discrete steel bars by means of a simple procedure.
-
Key words:
- concrete /
- reinforcement bar /
- bond-slip /
- explosion load
-
钢筋混凝土作为结构材料广泛应用于土木工程中,其力学性能非常复杂,与混凝土基体、钢筋及它们之间相互作用等力学行为紧密相关。当钢筋混凝土结构承受爆炸荷载时,钢筋与混凝土间的粘结应力上升,当超过一定的限制值后,钢筋与混凝土间发生滑移。尽管界面的粘结应力-滑移关系建立在微观尺度层面,却显著影响结构在爆炸荷载下的力学性能。
在钢筋混凝土结构的有限元分析方法中,围绕钢筋的数值建模,常用有限元模型有3种:(1) 弥散式模型[1];(2) 嵌入式模型[2-3];(3) 分离式模型[4-12]。
弥散式钢筋模型常用于由均匀分布的钢筋组成的墙体、楼板等表面型结构。该模型将钢筋混凝土简化为均质材料,易于使用。但是对于梁柱结构中箍筋和纵筋等复杂的钢筋几何问题,弥散式模型由于离散精度问题而不被采纳[1]。
在嵌入式模型中,钢筋混凝土构件横截面分为混凝土部分和钢筋部分,依据平截面假定计算截面应变,再根据钢筋和混凝土应力-应变关系和力平衡条件得到单元刚度矩阵,其中钢筋强化效果等效为嵌入在混凝土单元中的附加轴向分量。嵌入式模型使得钢筋的运用完全独立于其几何形状,且不增加数值模型的自由度。该模型假定嵌入的钢筋与混凝土完美粘结,即钢筋节点位移与混凝土节点位移一致[2,3]。
在分离式模型中,分别对钢筋和混凝土精细建模[4-12],混凝土采用实体单元离散,钢筋采用一维杆或者梁单元离散。在数值精度方面,该模型优于嵌入式模型,且通过大量的钢筋混凝土构件爆炸实验验证,故而在构件一级的抗爆分析中常为工程师所青睐。由于用于离散钢筋的单元需布置于混凝土单元的边界,这导致混凝土的网格严重受限于钢筋几何形状。
同时,在分离式模型中通过显式构造界面单元,可以考虑界面的粘结滑移效应。例如,师燕超等[5]在混凝土网格节点和钢筋节点间构造一维的虚拟弹簧单元,通过一维滑动接触模型考虑二者间的粘结滑移效应。Kwak等[13]通过混凝土网格节点与钢筋网格节点共点方式,构造了无物理尺寸的一维连接单元。De Groot等[14]在混凝土与钢筋之间定义二维粘结带,考虑平移、旋转等不同类型的相互作用。基于钢筋、混凝土网格节点建立的界面模型能较好地捕捉粘结滑移效应。但是界面模型给计算带来额外的开销。更重要的一点是,基于节点的界面几何建模的复杂度随着工程结构几何复杂度的增加而急剧增长,将耗费巨大的时间人力成本,这会极大地制约该类模型在钢筋混凝土结构级的工程应用[15-16]。因此针对大型钢筋混凝土建筑结构,如何高效高精度地建立钢筋混凝土数值模型依旧是一个开放性问题。
根据混合物理论,钢筋混凝土的力学行为可由混凝土基体和强化的钢筋两项组分的本构关系来描述,并且无需对钢筋进行显式离散化。该理论具有不同组分间应变相容性的局限性假定,可以通过修正钢筋本构关系,对混合物理论进行修正。本文构建一种基于修正混合物理论的改进型分离式数值模型,以期给出在钢筋本构方程中如何考虑界面粘结滑移机制的简单有效的修正方法,并避免显式构造界面即可考虑钢筋尺度的局部效应;对改进型分离式数值模型进行钢筋混凝土构件级、结构级的实验验证,以探索该模型在宏观大型钢筋混凝土结构有限元分析中的实用性和可靠性。
1. 改进型分离式数值模型
参照《混凝土结构设计规范(GB50010—2010)》,本文中以6层钢筋混凝土框架结构为例详细阐述改进型分离式数值模型的离散步骤:(1) 混凝土结构几何建模;(2) 钢筋骨架线几何建模;(3) 采用实体单元对框架结构进行网格离散;(4) 根据钢筋骨架线几何位置及钢筋特征尺寸信息,对包含钢筋骨架线的网格定义成钢筋混凝土网格,未包含钢筋骨架线的网格定义成素混凝土网格(这种离散方式巧妙地避免了传统分离式建模中混凝土网格节点与钢筋网格节点的复杂拓扑约束关系,极大缩短建模时间);(5) 定义两类网格的材料本构模型,素混凝土网格采用经典Holmquist-Johnson-Cook (HJC)模型,钢筋混凝土网格采用本文中提出的基于修正混合物理论的钢筋混凝土复合材料本构模型。
图1为网格尺寸为25 mm六面体单元的有限元模型,网格数为1.08×108。图1(a)为整体框架结构有限元模型,1~3层为混凝土部分,4~6层为钢筋骨架线,其中图1(b)为局部放大图。图1(c)展示了构件级混凝土网格和钢筋混凝土网格。对于大型混凝土结构而言,如采用实体单元—结构单元的传统分离式数值模型建模,以25 mm的网格为例,需处理千万级钢筋混凝土节点与混凝土节点的约束关系,前处理在网格剖分过程中将耗费大量的时间人力成本,不利于工程实用化。基于改进型分离式数值模型,可快速实现工程级有限元模拟,且网格分辨率达到钢筋尺寸。
1.1 修正型混合物理论模型
根据经典混合物理论[17],每个组分变形满足应变协调假定,即:
ε=ε1=ε2 (1) 式中:
ε 和εi (i=1,2⋯ )分别为应变和第i个组分的应变张量。应变协调假定在复合材料理论中具有一定的局限性。对于由混凝土基体和钢筋组成的复合材料而言,当钢筋和混凝土间的界面发生变形或者滑移时,界面滑移将导致复合材料应变场不连续,并影响混凝土与钢筋间的应力传递,从而导致钢筋中应力下降。通过考虑界面变形或者滑移,应变协调方程(式(1))可写成:εf=εm−εs (2) 式中:下标f和m分别代表钢筋和混凝土,
εs 为界面变形或者滑移的应变张量。不用显式构造界面,而将界面的粘结滑移效应引入到钢筋应力应变关系中。等效钢筋弹塑性本构关系可表达为:σeqf=Ceqf:εeqe=Ceqf:(εf+εs−εeqp) (3) 式中:上标eq表示考虑界面粘结滑移效应的等效物理量;
σeqf 、Ceqf 、εeqe 、εeqp 分别对应应力张量和弹性张量,弹性应变张量、塑性应变张量。基于修正混合物理论,兼顾混凝土基体和具有粘结滑移效应的钢筋力学行为,复合材料本构方程可表达为:
σ=∂ψ/∂ψ∂ε∂ε=Vm∂ψm/∂ψm∂ε∂ε+Vf∂ψf/∂ψf∂ε∂ε=Vmσm+Vfσeqf (4) 式中:
σ 和ψ 分别为钢筋混凝土复合材料的应力张量和自由能密度,Vm和Vf分别为混凝土和钢筋的体积分数,ψm 和ψf 分别为混凝土自由能密度及钢筋等效自由能密度,σm 和σeqf 混凝土应力张量和钢筋等效应力张量。1.2 钢筋等效模型
混凝土与钢筋间相对位移可以增加复合材料韧性。实际上,粘结力学机制控制钢筋混凝土的变形。相对高的粘结应力导致复合材料在早期阶段发生失效,而粘结应力较低的情况反而形成较大的破坏位移。因此,在钢筋混凝土力学性能的有限元模拟过程中,需要建立一个考虑粘结滑移效应的钢筋等效模型。通常采用等向强化弹塑性本构方程描述钢筋应力应变关系,即
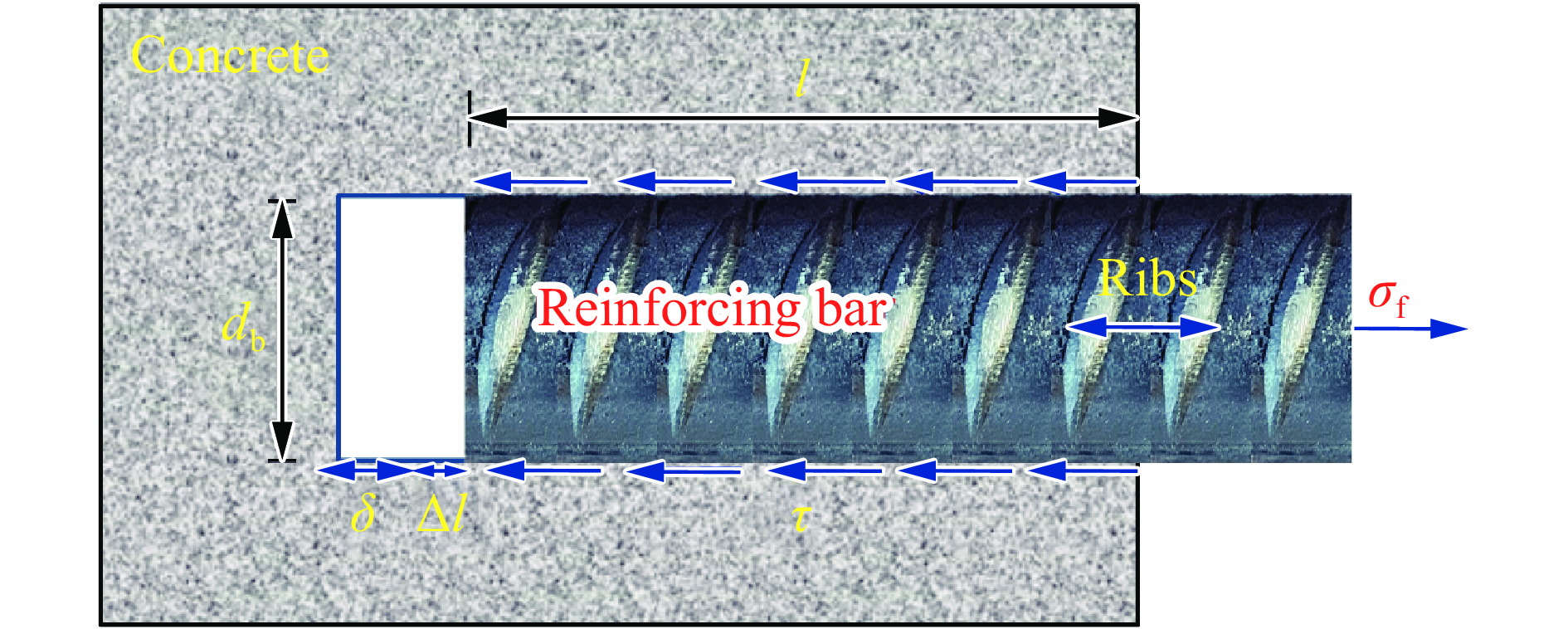
σf=(fy+Hfεp)[1+Cfln(˙εp/˙ε0)] ,其中σf 为钢筋应力,εp 为塑性应变,Cf 为应变率系数,˙εp 为塑性应变率,˙ε0 为参考应变率。通过修正钢筋模型参数(屈服强度fy 、弹性模量Ef 、塑性硬化模量Hf 等),建立考虑界面粘结滑移效应的钢筋等效本构关系。图2为钢筋尺度模型。假定钢筋总应变与它自身应变和由界面滑移引起的位移
δ 相关,张量形式应变协调方程(式(2))进一步简化成一维形式,即εeqf=εf+δ/l ,其中:εeqf 和εf 分别为一维形式的钢筋总应变与它的自身应变,l 为界面粘结区域长度,εf 与钢筋的形变位移Δl 相关。借鉴Dehestani等[3]的思想,在钢筋应力由弹性阶段进入屈服阶段过程中,钢筋等效弹性模量为:Eeqf=∫δy0feqy/feqy(εf+δ/δll)(εf+δ/δll)dδδy (5) 式中:
feqy 为钢筋等效屈服强度,δy 为钢筋屈服时所对应的界面滑移位移。Belarbi等[18]根据实验结果获取了嵌入在混凝土中钢筋的实际屈服强度满足如下关系式:
feqy=fy[0.93−2(fmr/fy)1.5ρ] (6) 式中:
ρ 为有效配筋率,fmr为混凝土在开裂应变为8×10−5时的拉伸断裂应力。界面滑移位移
δy 与钢筋物性参数、应力状态及界面本构关系均相关。在图2中,钢筋应力与界面剪应力满足力平衡关系,即τdblπ =π σfd2b/4 ,其中:τ 为钢筋混凝土界面剪应力,db为钢筋直径。剪应力τ 可采用CEB-FIB的四段式粘结滑移的本构模型[19],其剪应力-滑移位移关系如下:(1) 上升段,0≤δ≤δ1 ,τ=τmax(δ/δδ1δ1)α ;(2) 应力平台段,δ1≤δ≤δ2 ,τ=τmax ;(3) 下降段,δ2≤δ≤δ3 ,τ=τmax−(τmax−τf)(δ−δ2)/(δ3−δ2) ;(4) 残余应力平台段,δ3≤δ ,τ=τr 。该模型中各参数定义如下[19]:1.0 mm≤δ1≤1.8 mm ,2.0mm≤δ2≤3.6mm ,δ3 等于钢筋筋条间距12 mm;α=0.4 ;粘结强度max的范围为 2.5√fm∼1.25√fm ,fm 为混凝土抗压强度;残余应力τr=0.4τmax 。四段式本构关系对应的物理机制为:上升段对应局部混凝土压溃和微裂纹萌生的时候,钢筋筋条侵入到混凝土基体的过程;平台段表示筋条间受约束的混凝土发生剪切变形;下降段表示由于劈裂裂纹导致的粘结强度的下降,残余平台对应着横向钢筋效应残余的剩余粘结力。在粘结滑移模型中,钢筋混凝土粘结破坏有3种形式:
(1) 混凝土劈裂破坏,当由混凝土保护层或者箍筋提供的环向约束较弱时,钢筋周边环向拉伸应力将造成混凝土劈裂失效。
(2) 拉拔式破坏,当环向约束较强时,避免了混凝土劈裂破坏模式;当界面粘结强度较小时候,界面剪应力容易达到粘结强度,从而诱发拉拔式破坏模式。
(3) 钢筋断裂失效,当界面粘结长度较大、粘结强度较高时,钢筋应力优先达到最大拉伸应力,从而诱发钢筋断裂失效。
在爆炸荷载钢筋混凝土构件毁伤破坏过程中,通常表现为钢筋屈服、混凝土剥落等失效形式。即钢筋屈服时,界面剪应力满足上升段本构关系,根据图2中力平衡关系给出滑移位移计算公式:
δy=δ1[fydb/(fydbτmax4lτmax4l)]1/α (7) 钢筋等效弹性模量可进一步由式(5)、(6)和(7)计算。
钢筋等效模型中,除了修正屈服强度、弹性模量等参数,同时需对塑性硬化模量进行修正。等效塑性模量为:
Heqf=∫δcδy(σf−fy)/(σf−fy)(εp+δ/δll)(εp+δ/δll)dδδc−δy (8) 其中钢筋应力可由图2中力平衡关系和界面最大剪应力给出,δc=δ3。
因此,通过式(5)、(6)、(8),可获得考虑界面粘结滑移的钢筋等效应力-应变关系,其具体表达式为:
σeqf=(feqy+Heqfεp)[1+Cfln(˙εp/˙εp˙ε0˙ε0)] (9) 该本构关系与混凝土抗压强度、钢筋直径、界面粘结区长度等物理量相关。界面粘结区长度
l 可通过拉拔实验获取。参考CEB-FIB[19],粘结区长度可由钢筋直径和配筋率给出,即l=db/(7.2ρ) 。一些简单分析可用于评估粘结区长度的参数影响效果:对于钢筋与混凝土粘结一般情况,改变钢筋直径(db=12, 16, 20 mm)、屈服强度(fy=400, 500, 550 MPa)、混凝土强度(fc=40, 50, 60 MPa),研究粘结区长度l 对弹性模量缩放因子Eeqf/Ef 、塑性硬化模量缩放因子Heqf/Hf 的影响(图3和图4)。从图3和图4中可以得出:钢筋等效弹性、塑性硬化模量与粘结区长度呈正相关性,且均收敛到稳定值。塑性模量的收敛速度大于弹性模量的收敛速度。钢筋直径越粗、屈服强度越高、混凝土强度越高,则钢筋等效模量越大,规律趋势与Dehestani等[3]的结果一致。当粘结区长度较小时,钢筋混凝土粘结容易发生拉拔式破坏,界面变形受钢筋直径、屈服强度、混凝土强度等因素影响较大。此时考虑界面粘结滑移的等效模量对钢筋直径、屈服强度、混凝土强度等因素较为敏感。当粘结区长度较大时,容易诱发钢筋断裂的破坏模式,其等效模量主要与钢筋模型相关。
1.3 混凝土HJC本构模型
混凝土材料在爆炸荷载作用下将处于大应变、高围压及高应变率的状态。在众多混凝土动态本构模型中,HJC本构模型因其简明合理的描述和计算程序的适应性,在混凝土爆炸问题中获得了广泛应用。因此,复合材料中混凝土基体部分采用HJC本构模型,其应力-应变关系为:
σm=fm[Am(1−D)+Bm(p/pfmfm)Nm][1+Cmln(˙ε/˙ε˙ε0˙ε0)] (10) 式中:
σm 为混凝土应力,fm为混凝土抗压强度,Am为内聚力参数,D为损伤变量,Bm为压力硬化系数,p为压力,Nm为压力硬化指数,Cm为应变率参数。1.4 钢筋混凝土复合材料本构模型
结合钢筋等效模型及混凝土HJC模型,复合材料本构方程(式(4))可表达为:
σ=Vmσm+Vfσeqf (11) 式中:
σm 和σeqf 可分别由不考虑压力敏感的无损混凝土基体本构方程(式(10))和考虑界面粘结滑移效应的钢筋等效本构方程(式(9))给出。进一步,式(11)具体形式为:σ=(A+Bεp)[1+Cln(˙εp/˙εp˙ε0˙ε0)] (12) 式中:
A=Vffeqy+VmfmAm ,B=VfHeqf ,C=Cf-VffmAm(Cf-Cm)/A 。2. 层次化验证与确认
2.1 构件级精度验证
改进型数值模型的功能模块已在冲击波结构毁伤程序中实现[20],该程序是一款大规模流固耦合并行程序,能够高效高精度地模拟爆炸冲击类问题,其中流场分析模块是基于JASMIN框架[21]研发,结构动力学响应分析模块是基于JAUMIN框架[22]研发,数值模拟结果采用大规模可视分析平台TeraVAP进行后处理分析[23]。为验证数值模型在钢筋混凝土结构爆炸毁伤方面的计算精度以及粘结滑移模型的有效性,选取了轴压作用下钢筋混凝土柱[24]及板爆炸实验。
2.1.1 轴压作用柱爆炸实验
采用18.2 kg岩石乳化炸药(等效11.1 kg TNT),爆高为1.5 m。钢筋混凝土柱长2500 mm,截面为边长200 mm的正方形。主筋选用4根直径为20 mm的HRB335级钢筋,对称布置,配筋率为3.14%,钢筋抗拉屈服强度为466.7 MPa,极限强度为601.3 MPa,弹性模量为200 GPa,塑性硬化模量为1.12 GPa。箍筋选用直径为8 mm HRB235级钢筋,间距为150 mm,配筋率为0.34%,钢筋抗拉屈服强度为483.5 MPa,极限强度为582.4 MPa,弹性模量为200 GPa,塑性硬化模量为0.94 GPa。混凝土为C40级商品混凝土,保护层厚度为20 mm。爆炸实验前在柱端部施加轴向压力12 MPa,爆炸过程中柱构件下表面通过LVDT位移计测量位移时程,位移计布置于柱跨中位置。
基于冲击波结构毁伤程序分别建立考虑钢筋与混凝土间粘结滑移的改进型分离式数值模型和不考虑粘结滑移效应的分离式数值模型,网格尺寸均为10 mm。素混凝土网格采用HJC混凝土本构模型,模型参数为:初始密度为2.4 g/cm3,剪切模量为14.4 GPa,泊松比为0.20,fm=50.16 MPa, Am=0.28,Bm=1.84,Nm=0.84,Cm=0.007。对于钢筋混凝土网格,在考虑界面粘结滑移的数值模型中,参照Dehestani等[3]的研究,界面粘结区长度
l =300 mm,通过纵筋、箍筋等效材料参数(表1),及混凝土参数,再结合式(12),给出钢筋混凝土网格中复合材料模型参数。在不考虑粘结滑移数值模型中,纵筋、箍筋材料参数为原始参数。表 1 钢筋等效材料参数Table 1. Equivalent material parameters of rebar钢筋 feqy/MPa Eeqf/GPa Heqf/MPa Vf/% 纵筋 425.0 162.2 788.8 78.5 箍筋 368.1 150.2 656.5 50.0 对于爆炸荷载的模拟,根据药量大小和目标距离,采用ConWep爆炸荷载模型[25],通过结合反射超压pr和入射超压pi,并把入射角θ的影响考虑进来,计算得到目标上压力p,直接作用在结构上,计算模型为:
p=prcos2θ+pi(1+cos2θ−cosθ) (13) 在近场爆炸实验中,通过预备性爆炸实验,标定ConWep模型有效性。图5为爆心距离1.5 m位置处反射超压时程曲线的ConWep模拟结果与实验结果对比情况。从图5可以看出,通过修正ConWep模型中药量参数,获取与实验测量结果相当的超压时程曲线模拟结果,因此该模型可作为有效的构件荷载输入。
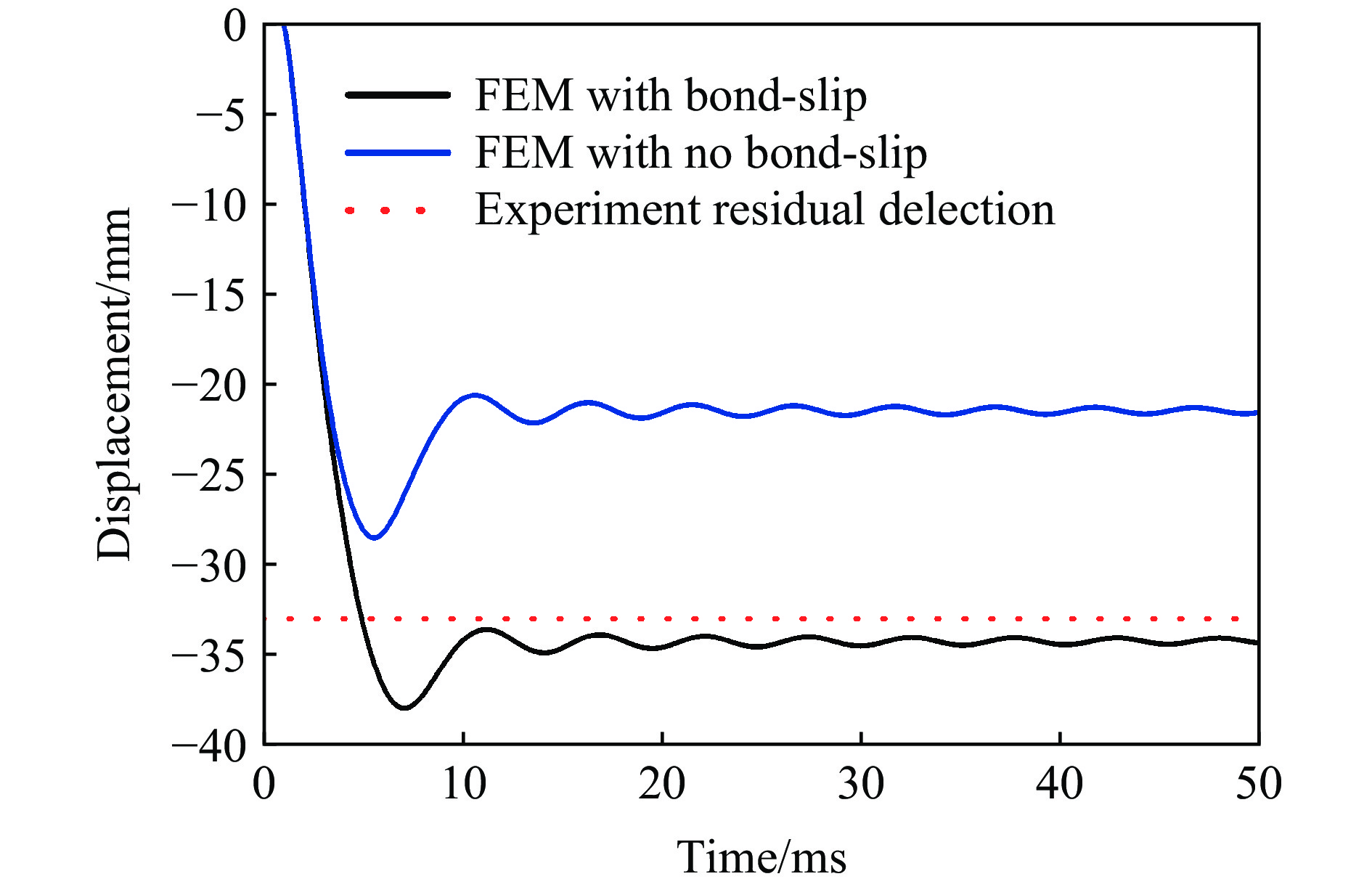
图6为柱跨中竖向位移及破坏形貌的模拟结果与实验结果的对比。从对比结果可以看出:考虑钢筋与混凝土间粘结滑移效应时,数值模拟的峰值位移和残余位移与实验值误差分别为6.28%和8.54%,试验件损伤破坏形貌与模拟的损伤破坏形貌吻合较好。相比于不考虑粘结滑移效应有限元模型,改进型分离式模型的模拟结果与实验结果更接近,这说明在爆炸荷载作用下钢筋混凝土结构动力学响应有限元模拟中,应该考虑钢筋与混凝土间粘结滑移。
2.1.2 板爆炸实验
实验中药量为10 kg TNT,爆高2.0 m。钢筋混凝土板几何尺寸为1200 mm×500 mm×50 mm,钢筋型号为直径8 mm的HRB235级钢筋,采用双层双向配筋,主方向间距100 mm,次方向按构造配筋间距200 mm,保护层厚度10 mm,配筋率为0.96%,钢筋参数详见2.1.1节。混凝土为C60级商品混凝土,保护层厚度为10 mm。
类似地,基于冲击波结构毁伤程序分别建立考虑粘结滑移的改进型分离式数值模型和不考虑粘结滑移效应的分离式数值模型,网格尺寸均为10 mm。素混凝土单元采用HJC混凝土本构模型,混凝土强度为39.2 MPa,模型参数参照2.1.1节内容。钢筋等效材料参数为:等效强度为420.3 MPa,等效弹性模量为170.1 GPa,等效塑性硬化模量657.0 MPa,钢筋混凝土单元中钢筋体积分数为50%。
图7为C30板跨中竖向位移的模拟结果与实验结果的对比。从对比结果可以看出:考虑粘结滑移效应时,数值模拟的残余位移与实验结果更为接近。进一步说明在爆炸荷载作用下钢筋混凝土结构动力学响应有限元模拟中,钢筋与混凝土间粘结滑移的必要性。
研究有限元网格尺寸对爆炸荷载下板变形的影响,选取10、5、2.5 mm 等3种网格尺寸,其中对应网格数分别为30000、240000、1920000。图8为3种网格尺寸的数值模拟结果:数值模拟结果与网格尺寸的大小相关,如10 mm 与5 mm的模拟结果略有差异;随着网格尺寸逐渐减小,跨中位移时程曲线差异减少并逐步收敛(5 mm和2.5 mm模拟结果);进一步的细化网格尺寸可有限地提高计算精度,但是计算成本成倍增加,因此10 mm的网格尺寸能够兼顾计算精度及计算成本。
2.2 结构级精度验证
通过结构级爆炸实验,进一步验证改进型分离式数值模型计算精度。Woodson等[26]和Baylot等[27]开展了1/4缩尺的两层框架结构外场爆炸实验。为了避免爆炸冲击波与框架结构强耦合相互作用的干扰,即爆炸冲击波在结构内外表面复杂入射、反射、绕射等现象,选取了文献[26-27]中含砖墙的框架结构爆炸实验用于验证模型。该框架结构宽3.2 m,深1.53 m,高2 m。框架结构由6根柱支撑,结构中柱截面尺寸为89 mm×89 mm,柱高为2 m,背面中柱截面尺寸为76 mm×76 mm,其余的柱截面尺寸为152 mm×152 mm。底层和顶层楼板厚度均为41 mm,层高0.91 m。立柱高于顶层楼板0.178 m。边梁位于中柱和边柱间,截面尺寸为81 mm×54 mm。四块填充墙均由13×15块砖墙堆砌而成,砖墙尺寸为宽99 mm,厚48 mm,高48 mm。实验中采用7.1 kg C4半球形炸药(1 kg C4炸药等效为1.19 kg TNT),爆点位置离地面高度为305 mm,距离底层中柱1.07 m。爆炸前,第2层中柱顶部施加1.7 t重物,模拟2.1 MPa预应力荷载。图9为改进型分离式数值模型,网格尺寸为5 mm,总计814万单元,其中暴露在外部分为钢筋混凝土网格。图9中插图为爆炸前实验设置。素混凝土网格采用HJC混凝土本构模型,其基本参数参照2.1.1节。钢筋混凝土网格采用复合材料模型,参数由混凝土强度和钢筋基本参数[26-27]给出。在炸药起爆前,在目标柱顶部施加2.1 MPa预应力分析,当结构所有节点速度低于0.1 m/s时,便认为结构达到新的静力平衡。随后开展爆炸模拟,总模拟时间为0.3 s。
图10为框架结构破坏形貌的模拟结果与实验结果的定性对比,其中图10(a)为爆炸荷载作用刚结束时框架结构损伤云图,图10(b)为结构最终状态损伤破坏云图,图10(c)为钢筋骨架的塑性区云图,图10(d)为实验结果。模拟结果表明:(1) 迎爆面砖墙均失效;(2) 低层中柱损伤区域最早出现在柱上下两端,随后发展至柱跨中背爆面处,由此可见该柱失效模式为剪切为主,弯曲为辅的耦合失效(图10(a)和10(b));(3) 低层中柱钢筋骨架的塑性区域主要集中在柱两端部及跨中背面位置;(4) 低层中柱失效后,失去支撑的边梁通过其抗弯承载力提供框架的抗倒塌能力,在“梁机制”作用下框架结构未发生垮塌。
图11为中柱位移时程曲线的定量对比结果。低层中柱弯曲变形,水平方向永久性位移为0.103 m,与实验测量永久性位移0.114 m基本一致。与不考虑粘结滑移相比,改进型数值模型计算结果与试验结果更接近。综合上述定性与定量验证,可进一步验证改进型分离式数值模型的有效性。
3. 结 论
(1)提出一种考虑钢筋与混凝土间粘结滑移机制的改进型分离式数值模型。将界面粘结滑移本构模型引入钢筋弹塑性本构关系中,对钢筋应力-应变曲线进行了修正。修正后的弹性、塑性硬化模量与界面粘结区长度、钢筋直径、混凝土强度等物性参数呈正相关性。
(2)改进型数值模型通过了钢筋混凝土构件级、结构级爆炸实验的精度验证,与不考虑界面粘结滑移数值模型相比,改进型模型的模拟结果与实验结果更接近。进一步说明界面效应在爆炸荷载作用下钢筋混凝土结构动力学响应分析中的重要性。
(3)改进型分离式数值模型由于没有对钢筋及其界面的显式离散要求,使得钢筋的运用完全独立于其几何形状,同时对混凝土网格没有约束,并且不增加计算成本,因此该模型可适用于钢筋混凝土宏观结构层面分析。
-
表 1 钢筋等效材料参数
Table 1. Equivalent material parameters of rebar
钢筋 feqy/MPa Eeqf/GPa Heqf/MPa Vf/% 纵筋 425.0 162.2 788.8 78.5 箍筋 368.1 150.2 656.5 50.0 -
[1] MARKOU G, PAPADRAKAKIS M. An efficient generation method of embedded reinforcement in hexahedral elements for reinforced concrete simulations [J]. Advances in Engineering Software, 2012, 45(1): 175–187. DOI: 10.1016/j.advengsoft.2011.09.025. [2] 李易, 陆新征, 任爱珠, 等. 某八层混凝土框架结构火灾连续倒塌模拟 [J]. 工程力学, 2011, 28(S1): 53–59.LI Y, LU X Z, REN A Z, et al. Simulation for fire-induced progressive collapse of an 8-storey rc frame structure [J]. Engineering Mechanics, 2011, 28(S1): 53–59. [3] DEHESTANI M, MOUSAVI S S. Modified steel bar model incorporating bond-slip effects for embedded element method [J]. Construction and Building Materials, 2015, 81: 284–290. DOI: 10.1016/j.conbuildmat.2015.02.027. [4] 武海军, 黄风雷, 付跃升, 等. 钢筋混凝土中爆炸破坏效应数值模拟分析 [J]. 北京理工大学学报, 2007, 27(3): 200–204. DOI: 10.3969/j.issn.1001-0645.2007.03.004.WU H J, HUANG F L, FU Y S, et al. Numerical simulation of reinforced concrete breakage under internal blast loading [J]. Transactions of Beijing Institute of Technology, 2007, 27(3): 200–204. DOI: 10.3969/j.issn.1001-0645.2007.03.004. [5] 师燕超, 李忠献. 爆炸荷载作用下钢筋混凝土柱的动力响应与破坏模式 [J]. 建筑结构学报, 2008, 29(4): 112–117. DOI: 10.3321/j.issn:1000-6869.2008.04.015.SHI Y C, LI Z X. Dynamic responses and failure modes of RC columns under blast loading [J]. Journal of Building Structures, 2008, 29(4): 112–117. DOI: 10.3321/j.issn:1000-6869.2008.04.015. [6] 汪维, 张舵, 卢芳云, 等. 方形钢筋混凝土板的近场抗爆性能 [J]. 爆炸与冲击, 2012, 32(3): 251–258. DOI: 10.11883/1001-1455(2012)03-0251-08.WANG W, ZHANG D, LU F Y, et al. Anti-explosion performances of square reinforced concrete slabs under close-in explosions [J]. Explosion and Shock Waves, 2012, 32(3): 251–258. DOI: 10.11883/1001-1455(2012)03-0251-08. [7] WANG W, YANG J C, WANG J H, et al. Experimental investigation of polyisocyanate-oxazodone coated square reinforced concrete slab under contact explosions [J]. International Journal of Impact Engineering, 2021, 149: 103777. DOI: 10.1016/j.ijimpeng.2020.103777. [8] YU X, ZHOU B K, HU F, et al. Experimental investigation of basalt fiber-reinforced polymer (BFRP) bar reinforced concrete slabs under contact explosions [J]. International Journal of Impact Engineering, 2020, 144: 103632. DOI: 10.1016/j.ijimpeng.2020.103632. [9] NING J G, MENG F L, MA T B, et al. Failure analysis of reinforced concrete slab under impact loading using a novel numerical method [J]. International Journal of Impact Engineering, 2020, 144: 103647. DOI: 10.1016/j.ijimpeng.2020.103647. [10] SHI Y C, WANG J, CUI J. Experimental studies on fragments of reinforced concrete slabs under close-in explosions [J]. International Journal of Impact Engineering, 2020, 144: 103630. DOI: 10.1016/j.ijimpeng.2020.103630. [11] LI Y, CHEN Z Y, REN X B, et al. Experimental and numerical study on damage mode of RC slabs under combined blast and fragment loading [J]. International Journal of Impact Engineering, 2020, 142: 103579. DOI: 10.1016/j.ijimpeng.2020.103579. [12] SU Q, WU H, SUN H S, et al. Experimental and numerical studies on dynamic behavior of reinforced UHPC panel under medium-range explosions [J]. International Journal of Impact Engineering, 2021, 148: 103761. DOI: 10.1016/j.ijimpeng.2020.103761. [13] KWAK H G, FILIPPOU F C. A new reinforcing steel model with bond-slip [J]. Structural Engineering and Mechanics, 1995, 3(4): 299–312. DOI: 10.12989/sem.1995.3.4.299. [14] DE GROOT A K, KUSTERS G M A, MONNIER T. Numerical modeling of bond-slip behavior [M]// Heron Concrete Mechanics, 1981. [15] LUCCIONI B M, AMBROSINI R D, DANESI R F. Analysis of building collapse under blast loads [J]. Engineering Structures, 2004, 26(1): 63–71. DOI: 10.1016/j.engstruct.2003.08.011. [16] KWASNIEWSKI L. Nonlinear dynamic simulations of progressive collapse for a multistory building [J]. Engineering Structures, 2010, 32(5): 1223–1235. DOI: 10.1016/j.engstruct.2009.12.048. [17] TRUESDELL C, TOUPIN R. The classical field theories [M]// FLÜGGE S. Principles of Classical Mechanics and Field Theory/Prinzipien der Klassischen Mechanik und Feldtheorie. Berlin: Springer, 1960: 226–858. DOI: 10.1007/978-3-642-45943-6_2. [18] BELARBI A, HSU T T C. Constitutive laws of concrete in tension and reinforcing bars stiffened by concrete [J]. Structural Journal, 1994, 91(4): 465–474. [19] FIB (International Federation For Structural Concrete). Bulletin 55: model code for concrete structures 2010 [S]. Lausanne: International Federation for Structural Concrete, 2010. [20] 曾繁, 肖桂仲, 田荣. 强冲击波结构毁伤等级评估软件JUST-PANDA及应用 [C]// 2018第十二届全国爆炸力学学术会议论文集. 桐乡: 中国力学学会, 2018. [21] MO Z Y, ZHANG A Q, CAO X L, et al. JASMIN: a parallel software infrastructure for scientific computing [J]. Frontiers of Computer Science in China, 2010, 4(4): 480–488. DOI: 10.1007/s11704-010-0120-5. [22] LIU Q K, MO Z Y, ZHANG A Q, et al. JAUMIN: a programming framework for large-scale numerical simulation on unstructured meshes [J]. CCF Transactions on High Performance Computing, 2019, 1(1): 35–48. DOI: 10.1007/s42514-019-00001-z. [23] 肖丽, 曹小林, 王华维, 等. 激光聚变数值模拟中的大规模数据可视分析 [J]. 计算机辅助设计与图形学学报, 2014, 26(5): 675–686.XIAO L, CAO X L, WANG H W, et al. Large-scale data visual analysis for numerical simulation of laser fusion [J]. Journal of Computer-Aided Design & Computer Graphics, 2014, 26(5): 675–686. [24] CHEN L, HU Y, REN H Q, et al. Performances of the RC column under close-in explosion induced by the double-end-initiation explosive cylinder [J]. International Journal of Impact Engineering, 2019, 132: 103326. DOI: 10.1016/j.ijimpeng.2019.103326. [25] RANDERS-PEHRSON G, BANNISTER K A. Airblast loading model for DYNA2D and DYNA3D: ARL-TR-1310 [R]. Maryland: U. S. Army Research Laboratory, 1997. [26] WOODSON S C, BAYLOT J T. Quarter-scale building/column experiments [C]// Structures Congress. Philadelphia: ASCE, 2000: 1–8. [27] BAYLOT J T, BEVINS T L. Effect of responding and failing structural components on the airblast pressures and loads on and inside of the structure [J]. Computers & Structures, 2007, 85(11): 891–910. DOI: 10.1016/j.compstruc.2007.01.001. 期刊类型引用(0)
其他类型引用(2)
-








 下载:
下载:

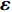







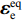


















































































 下载:
下载: