Oblique penetration of elliptical cross-section projectile into metal target
-
摘要: 为研究椭圆截面弹体对半无限金属靶体的侵彻弹道规律,基于14.5 mm弹道枪平台,开展了椭圆截面弹体在0°~20°倾角、850~950 m/s撞击速度下对2A12铝合金的斜侵彻试验。基于空腔膨胀理论及局部相互作用模型,建立了椭圆截面弹体侵彻弹道模型,并结合试验数据验证了模型的准确性。在此基础上,进一步分析了椭圆截面弹体长短轴之比、绕弹轴旋转角度、弹体撞击速度对侵彻弹道的影响规律。弹体长短轴之比为1.0时,弹体退化为尖卵形圆截面弹体,且椭圆截面弹体侵彻弹道稳定性随长短轴之比的增大而变弱,最优长短轴之比为1.0,即尖卵形圆截面弹体。椭圆截面弹体绕弹轴旋转一定角度后,侵彻弹道在平面曲线与空间曲线之间变化,当旋转角度为0°、90°时,侵彻弹道为二维平面弹道,当旋转角度在0°~90°之间时,侵彻弹道为三维空间弹道。当弹体撞击速度由800 m/s提升至1000 m/s时,椭圆截面弹体姿态角增量由18.6°降至17.8°。Abstract: In order to study the penetrating trajectories of elliptical cross-section projectiles into semi-infinite metal targets, a penetration trajectory model was established based on the dynamic cavity expansion theory and local interaction model. The shape function of the elliptical cross-section projectile was developed based on the local interaction model, and the resistance model derived from the dynamic cavity expansion theory was used to calculate the forces and moments acting on the elliptical cross-section projectile under the local Cartesian coordinate system. Thus, the factors affecting the projectile penetration trajectory were considered, including the major axis to minor axis ratio of the cross-section, the angle around the projectile axis and the striking velocity. Then, oblique penetrating experiments were carried out at a striking velocity ranging from 850 to950 m/s and an oblique angle ranging from 0° to 20°. Furthermore, the model was validated by experimental results. Finally, the influence of the major axis to minor axis ratio of the cross-section, the angle around the projectile axis and the striking velocity on the penetration trajectory was analyzed. When the major axis to minor axis ratio is 1.0, the projectile is degenerated into an ogive-nosed one. With the increase of this ratio, the stability of the elliptical cross-section projectile reduces. The optimal value of the major axis to minor axis ratio is 1.0, and the penetration trajectory is the most stable at this time. The penetration trajectory will change from a two-dimensional plane curve to a three-dimensional space curve when the angle around the projectile axis varies. When the angle around the projectile axis is 0° or 90°, the penetration trajectory is in a two-dimensional plane. Otherwise, the penetration trajectory is a three-dimensional space curve. The increasement of the attitude angle of the elliptical cross-section projectile decreases from 18.6° to 17.8° when the striking velocity increases from 800 m/s to 1000 m/s.
-
相较于圆截面弹体,异型截面弹体具有平台适应性好、装填比大、抗弯强度高及可提高平台内部腔体空间利用率等优点。近年来,异型截面弹体侵彻问题已成为武器研发领域的研究热点。Woo[1]研究了任意截面形状的空腔膨胀过程中的边界阻力分布特性,并由此得到了非圆截面弹体结构能够有效降低靶体侵彻阻力的结论。Bless[2]开展了长方形截面杆体及圆截面杆体高速侵彻试验,对比分析了二者的侵彻效率,得出了在临界速度以下非对称截面弹体侵彻性能更优的结论。杜忠华等[3-4]开展了圆截面、矩形截面、三角形截面3类侵彻体侵彻半无限金属板的试验与理论研究,发现在3类弹体截面比动能相等的情况下,侵彻效率由高到低分别为三角形截面弹体、矩形截面弹体及圆截面弹体。高光发等[5]基于杜忠华等[3-4]的试验结果,开展了相应的数值模拟工作,进一步验证了该结论。Partom等[6-7]开展了不同速度下旋转对称圆截面弹体和平面对称非圆截面弹体侵彻金属板的数值模拟研究。荣光等[8]开展了正方形和正三角形截面结构弹体的高速垂直和斜侵彻金属靶板的试验研究,结果表明,相同速度下,两种变截面结构弹体的垂直侵彻深度相差不大,但正方形截面结构弹体斜侵彻的深度略大于三角形截面结构弹体。王文杰等[9]开展了椭圆截面弹体侵彻半无限厚混凝土试验,基于局部相互作用理论及空腔膨胀理论,分析了椭圆截面弹体的侵彻阻力,建立了椭圆截面弹体侵彻深度计算模型。Dong等[10]、Zhang等[11]、刘子豪等[12]、王浩等[13-14]开展了椭圆截面弹体侵彻半无限厚混凝土试验,通过引入椭圆截面弹体头部形状因子对空腔膨胀理论进行修正,分析了弹体头部阻力变化规律,建立了椭圆截面弹体正侵彻深度模型,随后开展了椭圆截面弹体对多层钢板的侵彻破坏模式研究,分析了弹体侵彻过程中的弹道特性。
弹体在侵彻过程中偏离初始的速度方向而使得侵彻弹道弯曲、偏转是一个普遍的物理现象[15-16]。试验结果[17-20]表明,弹体侵彻混凝土、岩石、土壤、金属等靶体过程中均会受到多种因素的作用而发生弹体偏转现象。当前,Bernard等[21-22]、Warren等[19-20]、Fang等[23-24]、Kong等[25]、孔祥振等[26]、Wei等[27]对侵彻弹道的研究多以圆截面弹体为主,且主要采用数值模拟以及试验研究手段,而针对侵彻弹道的理论研究工作开展较少。同时,关于椭圆截面弹体侵彻弹道研究工作的公开报道较少。
目前,关于异型截面弹体的设计包括多种截面形状,且以异型截面弹体对典型靶体的正侵彻研究为主。侵彻弹道研究则关注圆截面卵形弹体侵彻问题,尚未涉及椭圆截面弹体的侵彻弹道研究。本文中,以圆截面卵形弹体形状函数为基准设计椭圆截面弹体,开展椭圆截面弹体以不同撞击速度及倾角侵彻半无限2A12铝合金靶体试验研究;同时,基于局部相互作用模型及空腔膨胀理论,建立弹体形状表征函数,发展椭圆截面弹体对半无限金属靶体的侵彻弹道模型,并结合试验数据验证模型的可靠性,分析椭圆长短轴之比、弹体绕轴旋转角度、弹体撞击速度对侵彻弹道的影响规律。
1. 椭圆截面弹体侵彻弹道模型
弹体侵彻问题是一个复杂的动力学问题。为简化这一过程,本文中,假设弹体为刚性弹体,结合空腔膨胀阻力模型及局部相互作用理论,分析椭圆截面弹体斜侵彻过程中的阻力特性,建立椭圆截面弹体对半无限金属靶体的侵彻弹道模型。
1.1 椭圆截面弹体形状函数
基于局部相互作用理论,引入局部笛卡尔坐标系描述椭圆截面弹体形状,如图1所示。图中r为椭圆截面的短半轴长,a为椭圆截面的长短轴之比(b=1),Lp为弹体头部长度,L为弹体长度。图1中以短半轴长r为有效半径,其所对应的头部曲率半径为R,以此获得椭圆截面弹体的等效曲径比为R/2r,同时,对椭圆截面长半轴进行放缩,使得椭圆截面弹体头部任意椭圆截面的长短轴之比恒定,其所对应的椭圆截面弹体头部曲线为椭圆弧,见图1。因此,椭圆截面弹体的头部形状可表示为:
√x2a2+y2b2+(R−r)=√R2−(Lp−z)2 (1) 考虑弹体绕z轴逆时针(从z轴正向)旋转γ角,且椭圆截面弹体为非旋转弹体。为便于分析计算,将弹体形状函数转换至柱坐标系下,因此,式(1)可改写为:
ρ=f(z,θ)={√R2−(Lp−z)2−(R−r)√1+ξsin(2θ)0<z<Lpr√1+ξsin(2θ)Lp<z<L (2) 式中:f (z, θ)为椭圆截面弹体形状函数,ξ为与弹体绕z轴旋转角度γ相关的因子,可表示为:
ξ=(ba−ab)sinγcosγ√1+(sinγcosγ)2(b2a2+a2b2) (3) 1.2 弹体侵彻阻力模型
Forrestal等[28]的研究表明,弹体在侵彻理想弹塑性应变硬化不可压缩材料过程中,其空腔膨胀阻力模型可表示为:
σnYt=a0+a2(vn√ρt/Yt)2 (4) a0=23[1+(2E3Yt)nI] (5) I=∫1−3Yt/2E0(−lnδ)n1−δdδ (6) a0=3/2 (7) 式中:σn为弹体表面的法向应力,vn为弹体表面微元法向速度,ρt、Yt、E、n分别为靶体密度、静态屈服强度、杨氏模量及应变硬化指数,δ为积分变量,
I 为积分常数,a0、a2为无量纲系数。图2为弹体侵彻初始条件分析示意图。图中v0为弹体初始撞击速度,θ0为弹体在YOZ平面内的弹轴与速度方向夹角,即攻角;ψ0为弹体在XOZ平面内的弹轴与速度方向夹角,即偏航角;vx0、vy0、vz0为初始撞击速度在局部坐标下沿x、y、z轴方向的速度分量;α0为弹体在YOZ平面内的初始倾角;β0为弹体在XOZ平面内的初始倾角。为便于分析弹体侵彻弹道,在局部坐标系之外引入整体坐标系。其中,笛卡尔局部坐标系与整体坐标系间的转换关系可以表示为:
(XYZ)=(xyz)(cosβ0sinβ−sinαsinβcosαsinαcosβ−cosαsinβ−sinαcosαcosβ) (8) 由空腔膨胀理论及式(4)可知,弹体侵彻过程中所受偏转力及力矩与弹体表面微元法向速度有关。弹体表面微元法向速度由弹体形状、弹体偏转角速度、弹体瞬时侵彻速度等因素决定。图3为局部坐标系下弹体表面微元法向速度分析示意图。图3(a)中,c为弹体质心,p为弹体表面微元,ωx、ωy、ωz为弹体绕x、y、z轴旋转角速度;图3(b)中,弹体表面微元至弹体质心距离为
\boldsymbol{l} (lx,ly,lz),lx、ly、lz分别为\boldsymbol{l} 沿x、y、z方向的距离分量。因此,考虑弹体偏转角速度的表面微元法向速度可表示为:{{v}}_{\mathrm{n}}=\left[{{v}}_{x}+\left({{\omega } }_{y}{l}_{{\textit{z}}}-{{\omega } }_{{\textit{z}}}{l}_{y}\right)\right]{{n}}_{x}+\left[{{v}}_{y}+\left({{\omega } }_{{\textit{z}}}{l}_{x}-{m{\omega }}_{x}{l}_{{\textit{z}}}\right)\right]{{n}}_{y}+\left[{{v}}_{{\textit{z}}}+\left({{\omega } }_{x}{l}_{y}-{{\omega } }_{y}{l}_{x}\right)\right]{{n}}_{{\textit{z}}} (9) 式中:vx、vy、vz分别为弹体在局部坐标系沿x、y、z方向的平移速度分量,nx、ny、nz分别表示弹体表面外法向向量在局部坐标系下沿x、y、z方向的分量。
图4为局部坐标系下弹体斜侵彻过程中的阻力分析示意图。由图4可知,弹体表面所受偏转力及力矩可表示为:
\left\{\begin{array}{l}{{F}}_{x}={{F}}_{\rm{n}}\cdot {{n}}_{x}\\ {{F}}_{y}={{F}}_{\rm{n}}\cdot {{n}}_{y}\\ {{F}}_{{\textit{z}}}={{F}}_{\rm{n}}\cdot {{n}}_{{\textit{z}}}\end{array}\right. (10) \left\{\begin{array}{l}{{M}}_{x}={{F}}_{y}\cdot {l}_{{\textit{z}}}-{{F}}_{{\textit{z}}}\cdot {l}_{y}\\ {{M}}_{y}={{F}}_{{\textit{z}}}\cdot {l}_{x}-{{F}}_{x}\cdot {l}_{{\textit{z}}}\\ {{M}}_{{\textit{z}}}={{F}}_{x}\cdot {l}_{y}-{{F}}_{y}\cdot {l}_{x}\end{array}\right. (11) 式中:Fn为弹体表面微元法向受力。有:
{{F}}_{\rm{n}}=\underset{{\varSigma }}{\overset{}{\iint }}{\sigma }_{\rm{n}}\mathrm{d}A (12) 式中:Σ为弹靶接触面积,dA为弹体表面微元的微分形式。有:
\mathrm{d}A=f\sqrt{1+{{f}_{{\textit{z}}}^{2}}}\,\mathrm{d}\theta\, \mathrm{d}{\textit{z}} (13) 因此,结合式(8),即可将弹体在整体坐标系下的偏转力及力矩表示出来。
1.3 椭圆截面弹体侵彻半无限厚金属靶弹道模型
基于刚性弹体假设,弹体在局部坐标系下的空间运动方程为:
\left\{\begin{array}{l}{F}_{x}=m{a}_{x}\\ {F}_{y}=m{a}_{y}\\ {F}_{{\textit{z}}}=m{a}_{{\textit{z}}}\end{array}\right. (14) \left\{\begin{array}{l}{M}_{x}={J}_{x}{\eta }_{x}\\ {M}_{y}={J}_{y}{\eta }_{y}\\ {M}_{{\textit{z}}}={J}_{{\textit{z}}}{\eta }_{{\textit{z}}}\end{array}\right. (15) 式中:m为弹体质量,ax、ay、az分别为弹体沿x、y、z方向的过载,Jx、Jy、Jz分别为弹体绕穿过质心的x、y、z轴的转动惯量,ηx、ηy、ηz为弹体偏转角加速度。弹体在局部坐标系下的初始侵彻条件为:
\left\{\begin{array}{l}{v}_{x}|_{t=0}={v}_{0}\mathrm{sin}{\psi }_{0}\\ {v}_{y}|_{t=0}={v}_{0}\mathrm{cos}{\psi }_{0}\,\mathrm{sin}{\theta }_{0}\\ {v}_{{\textit{z}}}|_{t=0}={-v}_{0}\mathrm{cos}{\psi }_{0}\,\mathrm{cos}{\theta }_{0}\end{array}\right. (16) \left\{\begin{array}{l}{\omega }_{x}|_{t=0}={\omega }_{x0}\\ {\omega }_{y}|_{t=0}={\omega }_{y0}\\ {\omega }_{{\textit{z}}}|_{t=0}={\omega }_{{\textit{z}}0}\end{array}\right. (17) 式中:ωx|t=0、ωy|t=0、ωz|t=0为弹体偏转角速度分量初始条件,ωx0、ωy0、ωz0为弹体着靶前绕局部坐标系下x、y、z轴的旋转速度。弹体整体坐标系下的侵彻初始条件可结合式(8)得到,同时弹体侵彻弹道亦可结合式(8)、式(14)~(17)得到。
2. 椭圆截面弹体侵彻铝合金靶弹道试验
为研究椭圆截面弹体对半无限金属靶的侵彻弹道规律及验证侵彻弹道模型,基于14.5 mm弹道枪平台,开展了椭圆截面弹体以850~950 m/s撞击速度斜侵彻半无限金属靶试验。
2.1 试验弹体及靶体
图5为试验椭圆截面弹体实物。图中,弹身处椭圆截面长轴长14.5 mm,短轴长9.0 mm,等效头部曲径比为5.6。弹体全长43.5 mm,质量为22.2 g。试验弹体由弹芯、底推组成。弹芯材料为30CrMnSiNi2A,密度为7.85 g/cm3,热处理后HRC硬度为42~45。为达到良好的闭气效果,底推材料选用铝合金,使弹芯在发射过程中与枪管配合紧密,保证弹体发射的稳定性,弹体结构质量参数见表1。
表 1 弹体结构质量参数Table 1. Parameters of the projectile弹体 等效曲径比 2ar/mm 2br/mm L/mm m/g HRC硬度 30CrMnSiNi2A 5.6 14.5 9.0 43.5 22.2 42~45 为保证侵彻弹道的完整以验证侵彻弹道模型,本文中,选用强度较低的金属材料2A12铝合金作为靶体材料。靶体尺寸设计为
\varnothing 150 mm×180 mm,以忽略靶体背部自由面对侵彻弹道的影响。同时,靶体由\varnothing 150 mm铝合金棒料切削加工而成,以保证靶体材料的均匀性。图6为试验现场布局。以14.5 mm弹道枪枪管为水平基准,靶体置于靶架上,通过水平仪测量靶面倾角确定弹体着靶倾角,并通过高速摄像机观察弹体着靶前的飞行姿态。表2为2A12铝合金力学性能参数。表 2 2A12铝合金力学性能参数Table 2. Parameters of the target material aluminum alloy 2A12材料 ρt/(kg∙m−3) E/GPa Yt/MPa n 2A12 2730 69.3 326.7 0.069 2.2 试验结果
共计开展3发试验,弹体倾角分别为5°、10°、20°。图7为弹体飞行姿态分析示意图。由图7可知,弹体外弹道飞行特性较好。
弹体侵彻前和侵彻后的长度及质量分别为43.5 mm、22.2 g和42.3 mm、21.2 g。因此,椭圆截面弹体斜侵彻过程可视为刚体侵彻。图8为椭圆截面弹体在不同倾角及撞击速度下靶体的破坏结果。由图8可知,当弹体以较小角度侵彻靶体时,靶体表面变形呈花瓣状。同时,由于弹体截面为椭圆形,在着靶前弹体绕弹轴旋转一定角度,导致靶体表面孔洞与水平方向存在一定角度。当弹体斜侵彻时,由于弹靶相互作用而产生的非对称力,使得靶体表面孔洞一侧受到的挤压较另一侧更严重。随着弹体着靶倾角的增大,与弹体上表面接触的靶体材料逐渐由挤压转向崩落。
图9为不同倾角及撞击速度下弹体的侵彻弹道。由图9可知,椭圆截面弹体侵彻弹道随着倾角的增大而更加不稳定,随着撞击速度的提升则趋向于稳定。同时,由图9(a)可知,尽管在试验前预设的倾角为0°,但是弹体的侵彻弹道仍然出现了弯曲偏转,通过高速摄像对弹体着靶姿态进行校核,弹体着靶角度为5°,使得弹道发生偏转。通过高速摄像系统观察及记录侵彻后靶体破坏结果,弹体侵彻试验结果见表3。表中:SX、SY、SZ分别为弹体在整体坐标系下沿X、Y、Z方向的位移,αend为弹体最终姿态角。
表 3 弹体侵彻试验结果Table 3. Test results of the penetration trajectories试验 v0/(m∙s−1) α0/(°) γ/(°) θ0/(°) 试验结果 SX/mm SY/mm SZ/mm αend/(°) T2-8 873 5.0 14 –1.0 3.7 14.8 –55.4 22.0 T2-13 898 11.1 –54 1.1 10.0 13.8 –53.3 25.1 T2-12 920 20.8 –7 0.8 5.3 43.5 –57.5 43.3 3. 椭圆截面弹体侵彻弹道模型验证与讨论
3.1 模型验证
基于第2节的理论公式,通过编写程序代入弹体形状函数及初始侵彻条件,求解出不同侵彻条件下椭圆截面弹体对半无限2A12铝合金靶体的侵彻弹道,并与第2节试验结果进行对比验证。图10为不同侵彻条件下椭圆截面弹体侵彻弹道的计算结果与试验结果的对比。由于本模型未考虑靶体表面破坏如材料挤压、裂纹扩展等对侵彻弹道的影响,同时,弹体在XOZ平面内的攻角并未观测,因此,当弹体在YOZ平面的倾角为5°时,弹尖终点坐标SY与试验结果相差较大,但是弹体在Z向的侵彻深度与试验结果接近。当弹体在YOZ平面的倾角为11.1°及20.8°时,弹尖终点坐标与试验结果吻合较好。表4为弹体终点坐标计算结果与试验结果的对比。
表 4 椭圆截面弹体终点坐标对比Table 4. Comparison of the final coordinates of the elliptic cross section projectiles试验 v0/(m∙s–1) 试验结果 计算结果 误差 SX/mm SY/mm SZ/mm αend/(°) SX/mm SY/mm SZ/mm αend/(°) ΔSX/mm ΔSY/mm ΔSZ/mm Δαend/(°) T2-8 873 3.7 14.8 –55.4 22.0 0.2 8.5 –60.0 15.4 3.5 6.3 –4.6 6.6 T2-13 898 10.0 13.8 –53.3 25.1 0.9 17.8 –57.5 14.6 9.1 –4.0 –4.2 7.3 T2-12 920 5.3 42.7 –57.5 43.3 1.4 37.2 –59.1 36.1 3.9 5.5 –1.6 –15.8 3.2 椭圆截面弹体侵彻弹道影响因素分析
基于椭圆截面弹体侵彻弹道模型,对影响弹体侵彻弹道的主要因素进行分析,包括弹体的长短轴之比a,弹体绕弹轴旋转角度γ以及弹体撞击速度v0。
3.2.1 弹体长短轴之比对侵彻弹道的影响分析
为研究椭圆截面弹体长短轴之比对侵彻弹道的影响规律,长短轴之比a(b=1)分别取1.0、1.2、1.4、1.6、1.8、2.0,并计算相应弹体的侵彻弹道。其中,当长短轴之比为1.0时,弹体退化为尖卵形圆截面弹体。计算弹体结构质量参数如表5所示。表中:1#弹体为尖卵形圆截面弹体,2#~6#弹体为椭圆截面弹体,rCRH为弹体头部等效曲径比,loc为弹体质心至弹尖的距离。各弹体质量、弹体头部长度、弹体等效曲径比均保持一致。
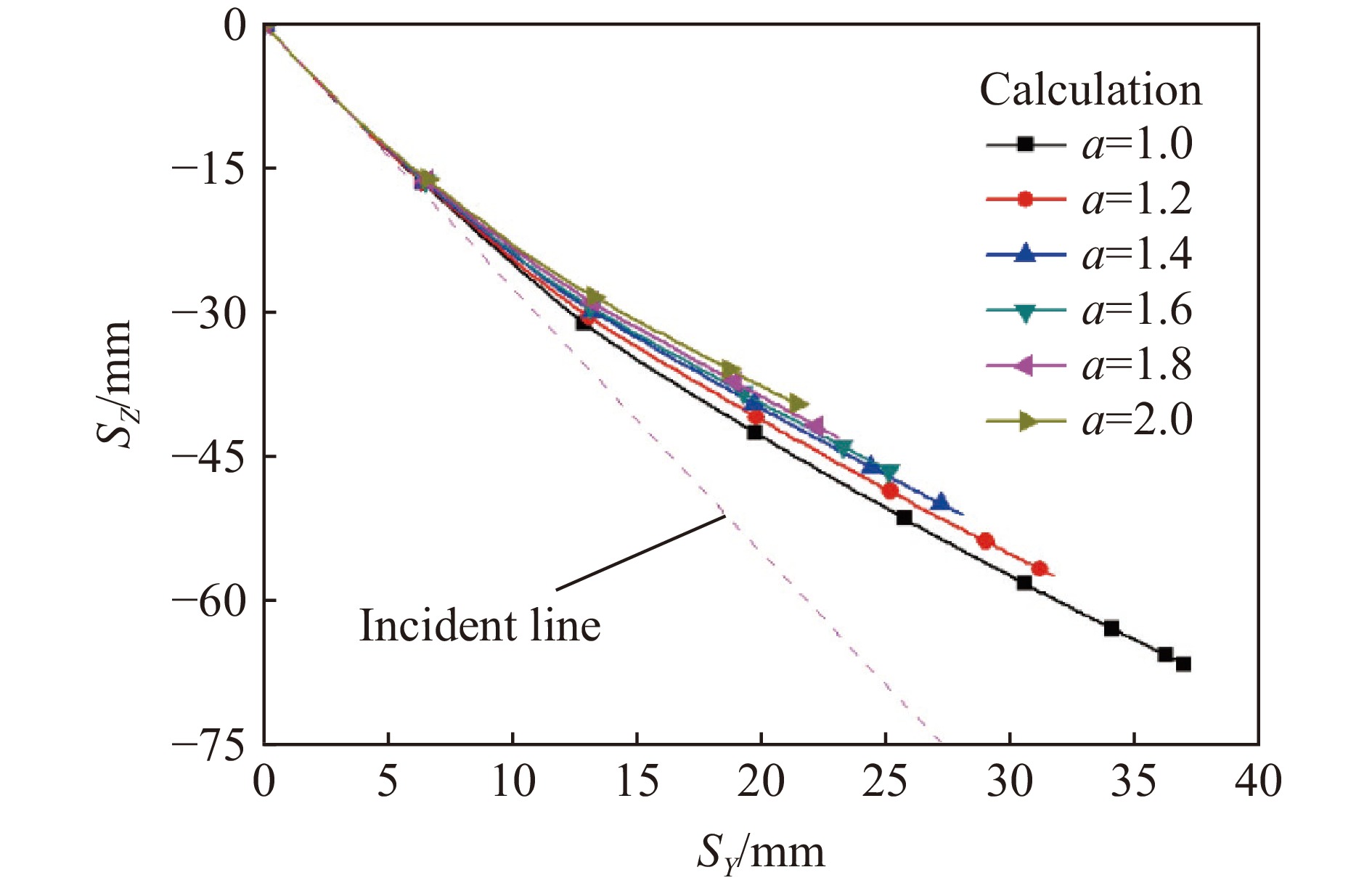
表 5 计算弹体结构质量参数Table 5. Computational parameters of the projectiles弹体 2ar/mm 2br/mm a rCRH Lp/mm L/mm m/g Jx/(g·mm2) Jy/(g·mm2) Jz/(g·mm2) loc/mm 1# 9.0 9.0 1.0 5.6 20.9 43.5 16.9 1876 1876 158 26.2 2# 10.8 9.0 1.2 5.6 20.9 43.5 17.0 1839 1877 217 24.3 3# 12.6 9.0 1.4 5.6 20.9 43.5 17.0 1847 1930 274 23.1 4# 14.5 9.0 1.6 5.6 20.9 43.5 17.1 1967 2108 344 23.4 5# 16.2 9.0 1.8 5.6 20.9 43.5 17.1 2091 2289 414 22.2 6# 18.0 9.0 2.0 5.6 20.9 43.5 17.0 2010 2266 468 21.2 同时,为对比分析弹体的侵彻弹道,弹体的撞击速度均为900 m/s,YOZ平面内初始倾角为20°,其他初始侵彻条件均设为零。图11~12分别为不同长短轴之比的椭圆截面弹体侵彻弹道、弹体姿态变化计算结果。从图中可以看出,当弹体绕轴旋转角度为0°时,椭圆截面弹体侵彻弹道稳定性随着长短轴之比的增大越来越差,同时,弹体长短轴之比的最优值为1.0。
3.2.2 弹体绕弹轴旋转角度对侵彻弹道的影响分析
为分析椭圆截面弹体绕轴旋转角度对侵彻弹道的影响规律,以表5中的4#弹体为弹体计算模型,计算弹体在900 m/s撞击速度、20°倾角时的侵彻弹道。其中,弹体绕弹轴旋转角度γ分别取0°、30°、60°、90°,如图13所示。图14为不同γ角度下椭圆截面弹体侵彻弹道计算结果。由图14可知,椭圆截面弹体侵彻弹道稳定性随着绕轴旋转角度的增大而变差。同时,根据计算结果,弹体在绕弹轴旋转角度γ分别取0°、30°、60°、90°时,在X方向上终点坐标分别为0、2.7、3.0、0 mm。由此可知,当椭圆截面弹体以一定的绕轴旋转角度侵彻靶体时,弹体的受力不再局限于YOZ平面,在XOZ平面内亦有分量。
图15为不同γ角度下弹体姿态角的变化曲线。由图15(a)可知,当β0为0°时,侵彻初始条件α0成为影响弹体侵彻弹道的主控因素。当γ分别为0°、90°时,弹体在YOZ平面内的姿态角αx的变化规律相同,但是γ为0°时弹体的侵彻弹道比γ为90°时的侵彻弹道稳定;当γ分别为30°、60°时,弹体姿态角α的变化说明弹体受力及力矩已变成空间向量,使得弹体侵彻弹道成为空间曲线。由图15(b)可知,当γ分别为0°、90°时,弹体侵彻弹道落在YOZ平面内,而当γ分别为30°、60°时,弹体侵彻弹道不再局限于YOZ平面内,而是成为复杂的空间曲线。
3.2.3 弹体撞击速度对侵彻弹道的影响分析
为分析椭圆截面弹体撞击速度对侵彻弹道的影响规律,以4#弹体为计算模型,分别计算弹体以800、900、1000 m/s的撞击速度、20°倾角下对铝合金靶体的侵彻弹道。其中,倾角位于YOZ平面内,弹体绕弹轴旋转角度γ为0°。图16和17分别为不同撞击速度下弹体的侵彻弹道计算结果及弹体姿态角变化。从图中可知,随着弹体撞击速度的提升,弹体偏转角度变小,弹体侵彻弹道越趋于稳定。
4. 结 论
开展了0°、10°、20°倾角椭圆截面弹体在850~950 m/s撞击速度范围内斜侵彻铝合金靶体试验,获得了椭圆截面弹体对半无限金属靶体的侵彻弹道。在此基础上,建立了椭圆截面弹体对半无限金属靶体的侵彻弹道模型,并结合试验结果验证了模型的有效性,主要结论如下。
(1)椭圆截面弹体长短轴之比对侵彻弹道有较显著影响。当长短轴之比为1.0时,弹体退化为尖卵形圆截面弹体。椭圆截面弹体侵彻弹道稳定性随着长短轴之比的增大而变弱,最优长短轴之比为1.0,即尖卵形圆截面弹体。
(2)椭圆截面弹体绕弹轴旋转角度对侵彻弹道影响非常显著。当弹体绕弹轴旋转角度为0°、90°时,侵彻弹道可视为平面曲线,且旋转角度为0°时的侵彻弹道稳定性优于旋转角度为90°时的侵彻弹道;当旋转角度为30°、60°时,侵彻弹道则成为空间曲线,弹体受力及力矩变得更复杂,且在侵彻过程中弹体边侵彻边绕轴旋转,使得侵彻弹道更加不稳定。
(3)椭圆截面弹体撞击速度对侵彻弹道影响较小。当绕轴旋转角度为0°,椭圆弹体撞击速度由800 m/s提升至1000 m/s时,弹体姿态角增量由18.6°降至17.8°。
-
表 1 弹体结构质量参数
Table 1. Parameters of the projectile
弹体 等效曲径比 2ar/mm 2br/mm L/mm m/g HRC硬度 30CrMnSiNi2A 5.6 14.5 9.0 43.5 22.2 42~45 表 2 2A12铝合金力学性能参数
Table 2. Parameters of the target material aluminum alloy 2A12
材料 ρt/(kg∙m−3) E/GPa Yt/MPa n 2A12 2730 69.3 326.7 0.069 表 3 弹体侵彻试验结果
Table 3. Test results of the penetration trajectories
试验 v0/(m∙s−1) α0/(°) γ/(°) θ0/(°) 试验结果 SX/mm SY/mm SZ/mm αend/(°) T2-8 873 5.0 14 –1.0 3.7 14.8 –55.4 22.0 T2-13 898 11.1 –54 1.1 10.0 13.8 –53.3 25.1 T2-12 920 20.8 –7 0.8 5.3 43.5 –57.5 43.3 表 4 椭圆截面弹体终点坐标对比
Table 4. Comparison of the final coordinates of the elliptic cross section projectiles
试验 v0/(m∙s–1) 试验结果 计算结果 误差 SX/mm SY/mm SZ/mm αend/(°) SX/mm SY/mm SZ/mm αend/(°) ΔSX/mm ΔSY/mm ΔSZ/mm Δαend/(°) T2-8 873 3.7 14.8 –55.4 22.0 0.2 8.5 –60.0 15.4 3.5 6.3 –4.6 6.6 T2-13 898 10.0 13.8 –53.3 25.1 0.9 17.8 –57.5 14.6 9.1 –4.0 –4.2 7.3 T2-12 920 5.3 42.7 –57.5 43.3 1.4 37.2 –59.1 36.1 3.9 5.5 –1.6 –15.8 表 5 计算弹体结构质量参数
Table 5. Computational parameters of the projectiles
弹体 2ar/mm 2br/mm a rCRH Lp/mm L/mm m/g Jx/(g·mm2) Jy/(g·mm2) Jz/(g·mm2) loc/mm 1# 9.0 9.0 1.0 5.6 20.9 43.5 16.9 1876 1876 158 26.2 2# 10.8 9.0 1.2 5.6 20.9 43.5 17.0 1839 1877 217 24.3 3# 12.6 9.0 1.4 5.6 20.9 43.5 17.0 1847 1930 274 23.1 4# 14.5 9.0 1.6 5.6 20.9 43.5 17.1 1967 2108 344 23.4 5# 16.2 9.0 1.8 5.6 20.9 43.5 17.1 2091 2289 414 22.2 6# 18.0 9.0 2.0 5.6 20.9 43.5 17.0 2010 2266 468 21.2 -
[1] WOO H J. Cavity expansion analysis of non-circular cross-sectional penetration problems [D]. Austin, USA: The University of Texas, 1997. [2] BLESS S J. Penetration mechanics of non-circular rods [J]. American Institute of Physics, 1996, 370(1): 1119–1122. DOI: 10.1063/1.50861. [3] 杜忠华, 曾国强, 余春祥, 等. 异型侵彻体垂直侵彻半无限靶板试验研究 [J]. 弹道学报, 2008, 20(1): 19–21.DU Z H, ZENG G Q, YU C X, et al. Experimental research of novel penetrator vertically penetrating semi-infinite target [J]. Journal of Ballistics, 2008, 20(1): 19–21. [4] 杜忠华, 朱建生, 王贤治, 等. 异型侵彻体垂直侵彻半无限靶板的分析模型 [J]. 兵工学报, 2009, 30(4): 403–407. DOI: 10.3321/j.issn:1000-1093.2009.04.005.DU Z H, ZHU J S, WANG X Z, et al. Analytical model on non-circular penetrator impacting semi-infinite target perpendicularly [J]. Acta Armamentarii, 2009, 30(4): 403–407. DOI: 10.3321/j.issn:1000-1093.2009.04.005. [5] 高光发, 李永池, 刘卫国, 等. 长杆弹截面形状对垂直侵彻深度的影响 [J]. 兵器材料科学与工程, 2011, 34(3): 5–8. DOI: 10.3969/j.issn.1004-244X.2011.03.002.GAO G F, LI Y C, LIU W G, et al. Influence of the cross-section shapes of long rod projectile on the vertical penetration depth [J]. Ordnace Marerial Science and Engineering, 2011, 34(3): 5–8. DOI: 10.3969/j.issn.1004-244X.2011.03.002. [6] PARTOM Y. The optimal velocity of constant kinetic energy constant L/D long rod projectiles [J]. International Journal of Impact Engineering, 1995, 17(4): 605–614. DOI: 10.1016/0734-743X(95)99884-T. [7] PARTOM Y, YAZIV D. Penetration of L/D=10 and 20 tungsten alloy projectiles into RHA targets [J]. American Institute of Physics, 1994, 309: 1801–1804. DOI: 10.1063/1.46345. [8] 荣光, 薛晓中, 孙传杰, 等. 异型弹芯斜侵彻靶板的数值分析 [J]. 弹道学报, 2009, 21(1): 9–12.RONG G, XUE X Z, SUN C J, et al. Numerical analysis of a non-circular cross-sectional projectile oblique penetrating into target [J]. Journal of Ballistics, 2009, 21(1): 9–12. [9] 王文杰, 张先锋, 邓佳杰, 等. 椭圆截面弹体侵彻砂浆靶规律分析 [J]. 爆炸与冲击, 2018, 38(1): 164–173. DOI: 10.11883/bzycj-2017-0020.WANG W J, ZHANG X F, DENG J J, et al. Analysis of projectile penetrating into mortar target with elliptical cross-section [J]. Explosion and Shock Waves, 2018, 38(1): 164–173. DOI: 10.11883/bzycj-2017-0020. [10] DONG H, WU H J, LIU Z H, et al. Penetration characteristics of pyramidal projectile into concrete target [J]. International Journal of Impact Engineering, 2020, 143: 103583. DOI: 10.1016/j.ijimpeng.2020.103583. [11] ZHANG S, WU H J, ZHANG X X, et al. High-velocity penetration of concrete targets with three types of projectiles: experiments and analysis [J]. Latin American Journal of Solids and Structures, 2017, 14(9): 1614–1628. DOI: 10.1590/1679-78253753. [12] 刘子豪, 武海军, 高旭东, 等. 椭圆截面弹体侵彻混凝土阻力特性研究 [J]. 北京理工大学学报, 2019, 39(2): 135–141; 146. DOI: 10.15918/j.tbit1001-0645.2019.02.005.LIU Z H, WU H J, GAO X D, et al. Study on the resistance characteristics of elliptical cross-section projectile penetrating concrete [J]. Transactions of Beijing Institute of Technology, 2019, 39(2): 135–141; 146. DOI: 10.15918/j.tbit1001-0645.2019.02.005. [13] 王浩, 武海军, 闫雷, 等. 椭圆横截面弹体斜贯穿双层间隔薄钢板失效模式 [J]. 兵工学报, 2020, 41(S2): 1–12. DOI: 10.3969/j.issn.1000-1093.2020.S2.001.WANG H, WU H J, YAN L, et al. Failure mode of oblique perforation of truncated ogive-nosed projectiles with elliptic cross-section into double-layered thin steel plate with gap space [J]. Acta Armamentarii, 2020, 41(S2): 1–12. DOI: 10.3969/j.issn.1000-1093.2020.S2.001. [14] 王浩, 潘鑫, 武海军, 等. 椭圆截面截卵形刚性弹体正贯穿加筋板能量耗散分析 [J]. 爆炸与冲击, 2019, 39(10): 103203. DOI: 10.11883/bzycj-2018-0350.WANG H, PAN X, WU H J, et al. Energy dissipation analysis of elliptical truncated oval rigid projectile penetrating stiffened plate [J]. Explosion and Shock Waves, 2019, 39(10): 103203. DOI: 10.11883/bzycj-2018-0350. [15] GAO X D, LI Q M. Trajectory instability and convergence of the curvilinear motion of a hard projectile in deep penetration [J]. International Journal of Mechanical Sciences, 2017, 121: 123–142. DOI: 10.1016/j.ijmecsci.2016.12.021. [16] 高旭东, 李庆明. 带攻角斜侵彻混凝土的弹道偏转分析 [J]. 兵工学报, 2014, 35(S2): 33–39.GAO X D, LI Q M. Trajectory analysis of projectile obliquely penetrating into concrete target at attack angle [J]. Acta Armamentarii, 2014, 35(S2): 33–39. [17] 马兆芳, 段卓平, 欧卓成, 等. 弹体斜侵彻贯穿薄混凝土靶姿态变化实验和理论研究 [J]. 兵工学报, 2015, 36(S1): 248–254.MA Z F, DUAN Z P, OU Z C, et al. The experimental and theoretical research on attitude of projectile obliquely penetrating into thin concrete target [J]. Acta Armamentarii, 2015, 36(S1): 248–254. [18] CHEN X G, LU F Y, ZHANG D. Penetration trajectory of concrete targets by ogived steel projectiles−experiments and simulations [J]. International Journal of Impact Engineering, 2018, 120: 202–213. DOI: 10.1016/j.ijimpeng.2018.06.004. [19] WARREN T L, POORMON K L. Penetration of 6061-T6511 aluminum targets by ogive-nosed VAR 4340 steel projectiles at oblique angles: experiments and simulations [J]. International Journal of Impact Engineering, 2001, 25(10): 993–1022. DOI: 10.1016/S0734-743X(01)00024-0. [20] WARREN T L, HANCHAK S J, POORMON K L. Penetration of limestone targets by ogive-nosed VAR 4340 steel projectiles at oblique angles: experiments and simulations [J]. International Journal of Impact Engineering, 2004, 30(10): 1307–1331. DOI: 10.1016/j.ijimpeng.2003.09.047. [21] BERNARD R S, CREIGHTON D C. Projectile penetration in soil and rock: analysis for non-normal impact: SL-79-15 [R]. Vicksbury, USA: U. S. Army Engineer Waterways Experimental Station Soils and Pavements Laboratory, 1979. [22] BERNARD R S, CREIGHTON D C. Non-normal impact and penetration. analysis for hard targets and small angles of attack: SL-78-14 [R]. Vicksbury, USA: U. S. Army Engineer Waterways Experimental Station Soils and Pavements Laboratory, 1978. [23] FANG Q, KONG X Z, WU H, et al. Predictions of projectile penetration and perforation by DAFL with the free surface effect [C] // 12th International Conference on Structures Under Shock and Impact. Greece, 2012. DOI: 10.2495/SU120221. [24] FANG Q, KONG X Z, HONG J, et al. Prediction of projectile penetration and perforation by finite cavity expansion method with the free-surface effect [J]. Acta Mechanica Solida Sinica, 2014, 27(6): 597–611. DOI: 10.1016/S0894-9166(15)60005-2. [25] KONG X Z, FANG Q, HONG J, et al. Numerical study of the wake separation and reattachment effect on the trajectory of a hard projectile [J]. International Journal of Protective Structures, 2014, 5(1): 97–117. DOI: 10.1260/2041-4196.5.1.97. [26] 孔祥振, 方秦, 吴昊. 考虑靶体自由表面和开裂区影响的可变形弹体斜侵彻脆性材料的终点弹道分析 [J]. 兵工学报, 2014, 35(6): 814–821. DOI: 10.3969/j.issn.1000-1093.2014.06.010.KONG X Z, FANG Q, WU H. Terminal ballistics study of deformable projectile penetrating brittle material targets for free-surface and crack region effects [J]. Acta Armamentarii, 2014, 35(6): 814–821. DOI: 10.3969/j.issn.1000-1093.2014.06.010. [27] WEI H Y, ZHANG X F, LIU C, et al. Oblique penetration of ogive-nosed projectile into aluminum alloy targets [J]. International Journal of Impact Engineering, 2021, 148: 103745. DOI: 10.1016/j.ijimpeng.2020.103745. [28] FORRESTAL M J, WARREN T L. Penetration equations for ogive-nose rods into aluminum targets [J]. International Journal of Impact Engineering, 2008, 35(8): 727–730. DOI: 10.1016/j.ijimpeng.2007.11.002. 期刊类型引用(9)
1. 薛颖杰,陈智刚,杨芮,崔晋,康彦姝,付建平. 椭圆截面弹体侵彻多层混凝土靶的数值仿真. 中北大学学报(自然科学版). 2025(01): 35-44 .  百度学术
百度学术2. 杨士林,高旭东,张先锋,王晓锋. 椭圆类截面弹体侵彻多层间隔钢靶的弹道特性. 爆炸与冲击. 2025(03): 122-138 .  本站查看
本站查看3. 董恒,黄风雷,武海军,邓希旻,李萌,刘龙龙. 异形弹体高速侵彻/穿甲机理研究进展. 兵工学报. 2024(09): 2863-2887 .  百度学术
百度学术4. 丁华,解文辉,乔立刚,李峰梅,许通通. 非圆截面战斗部侵彻双层钢板数值模拟. 兵工自动化. 2024(11): 10-15 .  百度学术
百度学术5. 戴湘晖,王可慧,周刚,李明,沈子楷,李鹏杰,段建,吴海军,杨慧. 刚性椭圆截面弹体侵彻性能理论研究. 现代应用物理. 2024(06): 132-141 .  百度学术
百度学术6. 戴湘晖,王可慧,周刚,李明,沈子楷,段建,李鹏杰,杨慧,吴海军. 椭圆截面侵彻弹体爆炸特性试验研究. 爆炸与冲击. 2023(05): 112-124 .  本站查看
本站查看7. 邓希旻,武海军,董恒,田泽,黄风雷. 椭圆截面截锥弹体的高速穿甲特性及阻力模型. 爆炸与冲击. 2023(09): 106-122 .  本站查看
本站查看8. 刘均伟,张先锋,刘闯,王佳敏,熊玮,谈梦婷,肖川. 椭圆截面弹体侵彻性能的影响因素分析. 爆炸与冲击. 2023(09): 155-168 .  本站查看
本站查看9. 解文辉,乔立刚,徐全振,魏许昌. 椭圆截面战斗部破片飞散特性研究. 兵器装备工程学报. 2023(11): 196-202 .  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:




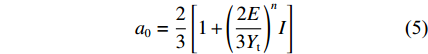













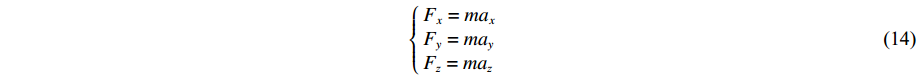
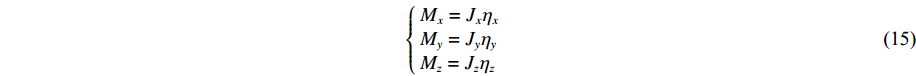


















 下载:
下载:
















 百度学术
百度学术