Numerical simulation and test study on ground shock subzones in soil produced by ground and buried explosion
-
摘要: 为研究爆炸条件下土中应力波的时空分布，基于黄土中接触爆炸和半埋爆炸试验，验证了ANSYS/AUTODYN软件建立的计算模型，并在此基础上开展了土中爆炸地冲击效应研究。结果表明：随着土介质深度的增加，感生地冲击峰值减小，而直接地冲击峰值增大，最终，压力和竖向应力时程曲线中的2个峰值减少为1个峰值，据此特征可将土中应力波场分为3个区域，即地表区、近地表区和中心区；当装药比例埋深为−0.05～0.075 m/kg1/3时，随着装药比例埋深的增大，中心区迅速扩大，地表区迅速缩小，近地表区逐渐扩大；当装药比例埋深为0.1～0.4 m/kg1/3时，地冲击作用区的分布趋于稳定；爆炸耦合进入空气和土介质中的动能受炸药类型影响，但在一定范围内，地冲击作用区角度与地面空气冲击波超压冲量和直接地冲击应力冲量之比呈线性相关关系。Abstract: In order to investigate the temporal and spatial distribution of the stress wave in the soil produced by buried explosion, the ANSYS/AUTODYN software was employed for modelling and simulation, and the ground shock effect of explosion in soil was analyzed. Based on the relationship between the pressure and volumetric strain of Luoyang loess obtained by predecessors, the relationship between the pressure and density of the impact compaction in the SAND model was modified. The numerical model was validated by the test data, which were measured from the contact explosion and semi-buried explosion test in loess. Then, a total of 22 numerical simulation conditions were examined to study the influence of the scaled buried depth of the charge and the type of the explosive on the ground shock subzones. The results show that as the depth of the soil medium increases, the peak of induced ground shock decreases, while the peak of direct ground shock increases, until the peak of the pressure-time curve and the peak in the vertical stress-time curve finally merge into a single peak. According to the characteristics of the pressure and vertical stress at various depths, the stress wave field in soil can be divided into three subzones consisting of surface subzone, near-surface subzone and central subzone. With the increase of the scaled buried depth of the charge, the central subzone rapidly increases, the surface subzone rapidly decreases, and the near-surface subzone gradually increases from zero, when the scaled buried depth of the charge ranges from −0.05 m/kg1/3 to 0.075 m/kg1/3. The distribution of the ground shock subzones tends to be stable, when the scaled buried depth of the charge ranges from 0.1 m/kg1/3 to 0.4 m/kg1/3. The energy of the explosive coupling into the air and soil mediums is affected by the type of the explosive. In certain extent, the angle of the ground shock subzones is linearly related to the ratio of the air-blast overpressure impulse to the impulse of the direct ground shock stress.
-
Key words:
- ground explosion /
- buried explosion /
- direct ground shock /
- induced ground shock /
- ground shock subzone
-
地表或浅埋爆炸产生的能量一方面用于破碎爆炸附近的岩土介质形成飞散的抛掷体，另一方面与地表上面的空气发生相互作用，在空气中形成冲击波。爆炸时，通过直接压缩地介质并在其中形成的波称为直接地冲击，而空气中的冲击波掠过地表，通过压缩空气和地介质相互作用形成的在地介质中传播的波称为感生地冲击。所以爆心附近土中浅层地下介质所受地冲击荷载比较复杂，既有直接地冲击，也有感生地冲击。地冲击和空气冲击波到达地表观测点的时间相对关系由岩土介质中的地冲击传播速度和空气冲击波传播速度差异决定，不同介质、不同埋置深度的爆炸造成地冲击应力、加速度场的不同分布。
近年来，对于岩土介质中直接地冲击方面的研究已经较为完善。Wu等[1]通过现场大型地下爆炸试验，研究了介质内部、岩土界面和地表的爆炸应力波特性；给出并分析了实测应力波时程及其特征参数，如质点速度峰值和质点加速度峰值以及不同位置的主频率。何翔等[2]通过石灰岩中不同装药深度的爆炸试验，得到了石灰岩中爆炸成坑经验公式以及地冲击传播规律。Leong等[3]根据新加坡残积土的小规模现场爆破试验，检验了TM5-855-1对应力峰值的估算。吴祥云等[4]通过现场试验研究了常规装药爆炸埋深对自由场直接地冲击参数的影响，得到了不同岩土介质、不同地冲击参数等效当量埋深系数的计算方法。叶亚齐等[5]运用等效当量埋深系数，给出了砂质黏土中不同深度爆炸自由场地冲击参数的预估方法。Yankelevsky等[6]通过现有经验数据的分析和数值模拟，研究了土中爆炸冲击波峰值压力衰减特性。Jayasinghe等[7]对不同埋深爆炸条件下饱和砂土中桩的动力响应进行了数值分析，给出了爆炸波在土体中的传播和桩的水平变形。Song等[8]对聚能装药爆炸过程进行了数值模拟，得到了土介质的动力响应、爆炸空腔的形成和发展规律以及爆炸波在土中的传播规律。
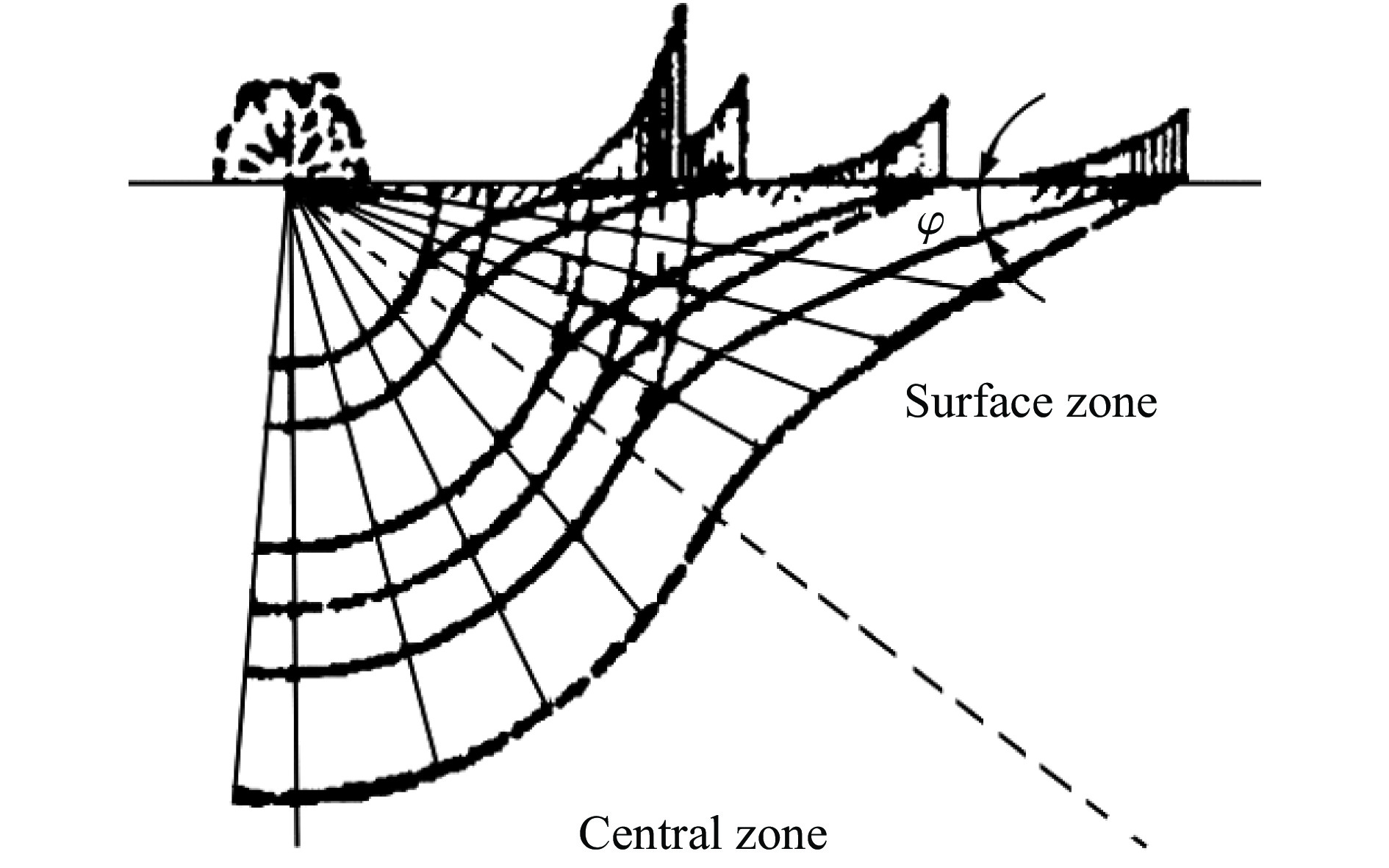
然而，感生地冲击方面的研究则十分有限，且大多是研究触地爆炸条件下的感生地冲击效应。埋置爆炸条件下土中感生地冲击的影响范围尚不明确。Alekseenko等[9]基于相似理论进行了相关试验研究，试验测量结果如图1所示，得到了土中压缩波的大致分布情况以及表面区域地冲击有2个峰值等结论。Beshara[10]基于拟静态特性，给出了包括烈性炸药、混合气体和粉尘悬浮物在内的多种内爆气体压力的半经验关系式和预测方法，在忽略土-结构相互作用的情况下，给出了一种感生地冲击和直接地冲击的简化计算形式。Wu等[11]基于对地面爆炸的一系列参数化数值模拟，确定了可以应用于结构响应分析的地冲击和空气冲击波荷载。然后，他们以单层砌体填充的钢筋混凝土框架为例，计算了结构在地冲击和空气冲击波共同作用下，或单独在地冲击和空气冲击波作用下的动力响应和损伤[12]。范俊余等[13]结合Alekseenko等[9]的试验现象，对炸药地面爆炸条件下浅地表波场的分布以及作用到土中浅埋结构上的荷载进行了数值模拟研究，发现典型土中浅埋结构的顶板主要承受感生地冲击的作用，外墙主要承受直接地冲击的作用。柳锦春等[14]基于数值模拟结果和试验数据，对根据一维平面波理论和特征线解法得到的空气冲击波为突加三角形荷载的感生地冲击的理论计算公式进行修正，提出了炸药地面接触爆炸下土中感生地冲击的实用计算方法。杨仁华等[15]采用平面装药方法进行了核弹空爆试验模拟研究，给出了间接地冲击在黄土中的衰减规律。吴祥云等[16]通过石灰岩中不同装药深度的爆炸试验，研究了石灰岩中装药埋深对地表空气冲击波超压的影响，给出了不同埋深爆炸地表空气冲击波超压峰值的预计方法。Krauthammer等[17-18]和Chee[19]基于高能炸药模拟技术（high explosive simulation technique，HEST）试验，提出了一种改进的单自由度（single-degree-of-freedom，SDOF）方法，并对浅埋钢筋混凝土箱形结构在空气冲击波荷载下的受力性能进行了一系列研究。荣吉利等[20]提出了考虑感生冲击波与直接冲击波叠加效应的核爆地冲击描述方法，并以美军核爆地冲击试验为例研究了核爆地冲击作用下土体运动特性。
为了研究爆炸条件下土中直接地冲击和感生地冲击的影响范围，本文中利用ANSYS/AUTODYN软件[21]建立二维轴对称有限元模型，开展土中爆炸地冲击效应数值模拟分析；通过黄土中爆炸试验，测得不同比例爆距上地面空气冲击波超压和土中直接地冲击竖向应力，并以此进行计算模型的验证；利用验证后的计算模型，对装药不同比例埋深和不同类型炸药的土中爆炸进行数值模拟；根据不同工况的计算结果，获得不同爆炸条件下土中感生地冲击和直接地冲击荷载的作用特征，并以此对地冲击作用区域进行划分；最后分析影响地冲击作用区的关键参数。
1. 计算模型
1.1 几何模型
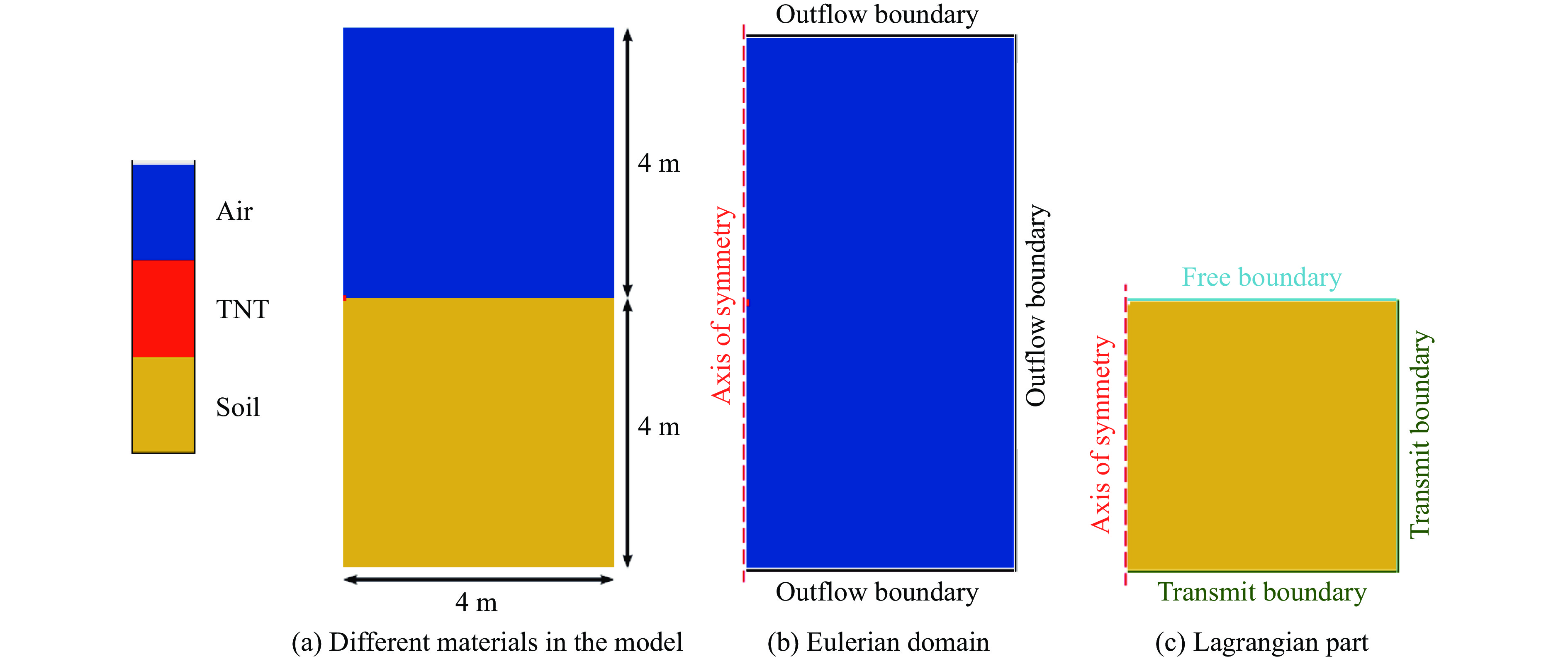
建立了如图2所示的模型，该模型由炸药、空气和黄土3部分组成，其中，对炸药和空气采用欧拉算法，对黄土采用拉格朗日算法。数值模拟中采用ANSYS/AUTODYN软件的自动流固耦合算法。炸药装药为圆柱体，起爆点为炸药的几何中心。
计算工况如表1所示。B1～B10为TNT炸药不同比例埋深的工况，装药量为1 kg，比例埋深分别为−0.05、−0.025、0、0.025、0.05、0.075、0.1、0.2、0.3和0.4 m/kg1/3。C1～C12为不同类型炸药的工况，不同工况以炸药的初始比内能E0为依据进行等效处理。炸药类型分别为ANFO、C4、EXPLOS.D、HMX、HNS 1.65、NM、PBX9407、PBX9502、PETN 1.77、SEISMOPLAS、TETRYL、TNT。
表 1 计算工况Table 1. Calculation conditions工况 炸药类型 装药量/kg 装药比例埋深/(m·kg−1/3) 装药半径/mm 装药高度/mm B1 TNT 1 −0.05 44.2 100 B2 TNT 1 −0.025 44.2 100 B3 TNT 1 0 44.2 100 B4 TNT 1 0.025 44.2 100 B5 TNT 1 0.05 44.2 100 B6 TNT 1 0.075 44.2 100 B7 TNT 1 0.1 44.2 100 B8 TNT 1 0.2 44.2 100 B9 TNT 1 0.3 44.2 100 B10 TNT 1 0.4 44.2 100 C1 ANFO 1.380 0 61.8 123.6 C2 C4 0.655 0 40.2 80.4 C3 EXPLOS.D 0.968 0 47.7 95.4 C4 HMX 0.663 0 38.2 76.4 C5 HNS 1.65 0.815 0 42.8 85.6 C6 NM 0.814 0 48.6 97.2 C7 PBX9407 0.685 0 40.8 81.6 C8 PBX9502 0.987 0 43.6 87.2 C9 PETN 1.77 0.645 0 38.7 77.4 C10 SEISMOPLAS 0.835 0 43.7 87.4 C11 TETRYL 0.777 0 41.5 83.0 C12 TNT 1 0 46.0 92.0 1.2 有限元模型
考虑到计算模型的对称性，建立了二维轴对称有限元模型[22]，计算域尺寸为4 m×8 m，如图3(a)所示。其中，空气尺寸为4 m×8 m，炸药通过Fill命令填充在空气中。如图3(b)所示，欧拉域的左边界为对称边界，其他3条边界为流出边界。如图3(c)所示，拉格朗日域的左边界为对称边界，下边界和右边界为传输边界，其余边界为自由边界。
如表1所示，对于计算工况B1～B10，炸药尺寸为
∅ 88.4 mm×100 mm。黄土的宽度为4 m，随装药比例埋深的增大，黄土的高度略有差别。炸药附近区域的局部放大如图4所示，炸药放置位置以上为空气，以此模拟钻地武器的钻地效果。对于计算工况C1～C12，炸药密度和装药量的不同导致装药尺寸略有差别，黄土的宽度为4 m，高度为4 m。以工况B3为例，图5给出了有限元模型的网格划分情况。炸药网格的径向长度为4.42 mm，高度方向长度为5 mm。对空气和黄土以炸药网格的尺寸为基础采用渐变网格，空气网格的最小尺寸为4.42 mm×5.0 mm，黄土网格的最小尺寸为8.84 mm×10.0 mm。整个有限元模型中，欧拉单元为165000个，拉格朗日单元为36000个，共201000个单元。
1.3 材料模型
1.3.1 炸药
炸药采用ANSYS/AUTODYN内置材料库中的炸药模型[23]。Jones-Wilkins-Lee （JWL）状态方程常用来描述炸药的爆炸和膨胀，其表达式如下[24]：
p=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωE0V (1) 式中：V为相对体积，V=1/ρ；E0为初始比内能；A、B、R1、R2、ω为特征参数。炸药模型参数取值如表2所示[23]，表中ρ0为炸药的初始密度，Dd为炸药的爆速，pd为炸药的爆压。
工况 炸药类型 ρ0/(g·cm−3) A/GPa B/GPa R1 R2 ω Dd/(m·s−1) pd/GPa E0/(GJ·m−3) C1 ANFO 0.931 49.46 1.891 3.907 1.118 0.333 4160 5.15 2.484 C2 C4 1.601 609.77 12.95 4.5 1.4 0.25 8193 28 9.0 C3 EXPLOS.D 1.42 300.7 3.94 4.3 1.2 0.35 6500 16 5.4 C4 HMX 1.891 778.28 7.0714 4.2 1.0 0.30 9110 42 10.5 C5 HNS 1.65 1.65 463.1 8.873 4.55 1.35 0.35 7030 21.5 7.45 C6 NM 1.128 209.25 5.689 4.4 1.2 0.30 6280 12.5 5.1 C7 PBX9407 1.60 573.2 14.64 4.6 1.4 0.32 7910 26.5 8.6 C8 PBX9502 1.895 460.3 9.544 4.0 1.7 0.48 7710 30.2 7.07 C9 PETN 1.77 1.77 617.05 16.926 4.4 1.2 0.25 8300 33.5 10.1 C10 SEISMOPLAS 1.588 620.60 23.27 5.399 1.651 0.282 7200 20.5 7.0 C11 TETRYL 1.73 586.83 10.671 4.4 1.2 0.275 7910 28.5 8.2 C12 TNT 1.63 373.77 3.7471 4.15 0.9 0.35 6930 21.0 6.0 B1～B10 TNT 1.63 373.77 3.7471 4.15 0.9 0.35 6930 21.0 6.0 1.3.2 空气
对空气采用ANSYS/AUTODYN内置材料库中的AIR模型[25]，其状态方程为：
p=(γ−1)ρE0 (2) 式中：γ为理想气体等熵绝热指数，ρ为气体密度，E0为初始比内能。本文中空气的初始密度为1.225 kg/m3，γ=1.4，E0=206.8 J/g，比热容为717.6 J/(kg·K)，参考温度为288.2 K，这些参数均取自文献[25]。
1.3.3 黄土
对黄土采用ANSYS/AUTODYN软件内置材料库中的SAND模型[26]。Laine等[27]根据砂土在动态加载条件下的响应提出了SAND模型，并基于该模型进行了一系列数值模拟研究[28-30]。SAND模型主要包括状态方程与颗粒强度模型2个部分。ANSYS/AUTODYN软件中的压实态状态方程分为线性和非线性2类，SAND模型选用的是线性压实态状态方程。压实态状态方程还可以与多种强度模型、失效模型共同描述材料的力学行为。颗粒强度模型是Drucker-Prager强度模型[31]的拓展，考虑了包括粉末、黏土和砂土等颗粒介质的强度。除了压力强化效应，SAND模型还描述了密度强化效应以及剪切模量与密度之间的函数关系。表3为SAND模型输入的参数值[32-33]。
冲击压实方程（参考密度ρ=2.641 g/cm3） 颗粒强度模型 压力/MPa 密度1/(g·cm−3) 声速/(km·s−1) 密度2/(g·cm−3) 压力/MPa 强度/MPa 密度/(g·cm−3) 剪切模量/GPa 0 1.674 0.2652 1.674 0 0 1.674 0.077 4.577 1.739 0.8521 1.745 3.40 4.23 1.746 0.869 14.98 1.874 1.722 2.086 3.49 44.7 2.086 4.03 29.15 1.997 1.875 2.147 101 124 2.147 4.91 59.17 2.144 2.265 2.300 185 226 2.300 7.77 98.09 2.250 2.956 2.572 500 226 2.572 14.8 179.4 2.380 3.112 2.598 2.598 16.6 289.4 2.485 4.600 2.635 静水拉力极限
pmin= −1.00 kPa2.635 36.7 450.2 2.585 4.634 2.641 2.641 37.3 650.7 2.670 4.634 2.800 2.800 37.3 注：(1)利用压力与密度1的分段线性函数来描述砂土的塑性压实过程；(2)利用声速与密度2的分段线性函数来描述砂土的弹性加载和卸载过程。 试验时，土介质为洛阳地区的黄土[34-36]，该黄土比重为2.54～2.84，干密度为1200～1790 kg/m3，天然含水率为10%～15%，黏聚力为30～60 kPa，内摩擦角为15°～25°。洛阳地区黄土的压力p（Pa）与体积应变
ε 的关系为[37-38]：p=8.626×105exp(18.93ε) (3) 因此，基于式(3)对SAND模型中冲击压实方程的压力与密度1的对应关系进行修正，如表4所示。
表 4 线性压实状态方程中压力与密度1的关系（基于式(3)）Table 4. Relationship between pressure and density 1 in the compaction linear equation of state (based on formula (3))密度1/(g·cm−3) 体应变εi = 1−ρi/−ρiρi+1ρi+1 累积体应变ε 修正后压力/MPa 1.674 0 0 0 1.739 0.03738 0.03738 1.750 1.874 0.07204 0.10942 6.844 1.997 0.06159 0.17101 21.96 2.144 0.06856 0.23957 80.42 2.250 0.04711 0.28668 196.2 2.380 0.05462 0.34131 551.8 2.485 0.04225 0.38356 1227.8 2.585 0.03868 0.42224 2553.6 2.670 0.03184 0.45408 4665.3 2. 计算模型的试验验证
2.1 试验概况
图6为土中接触爆炸和半埋爆炸的布置图，TNT炸药的比例埋深分别为−0.05和0.0 m/kg1/3。地面布置空气冲击波超压测点A1～A4，土中爆心正下方布置直接地冲击竖向应力测点S1～S4。
试验中采用PCB压力传感器（见图7(a)）测试地面空气冲击波超压，采用电荷式压力传感器（见图7(b)）测试土中应力。图6给出了8个传感器的位置，4个地面空气冲击波超压测点距爆心水平距离分别为0.50、0.75、1.00和1.25 m，4个土中直接地冲击竖向应力测点的爆心距分别为0.5、0.8、1.2和1.7 m。回填黄土的密度控制在1600～1700 kg/m3范围内。数据采集仪选用了东华测试公司生产的DH5960超动态信号测试分析仪（见图7(c)），采样频率高达1 MHz。
2.2 试验方案
试验共进行2炮次，工况见表5，试验工况E1和E2分别对应数值模拟中的工况B1和B3。图8为试验准备完成后的现场布置。炸药采用电雷管起爆，将电雷管插入炸药中间，使得起爆点接近炸药中心位置。
表 5 试验工况Table 5. Test conditions工况 装药量/kg 装药比例埋深/(m·kg−1/3) 地面空气冲击波超压测点 土中直接地冲击竖向应力测点 E1 1 −0.05 A1～A4 S1～S4 E2 1 0.00 2.3 试验结果与计算模型验证
图9为试验后的破坏情况。将坑底回落的土进行清理后，测得试验E1的真实爆坑直径约为69.0 cm，真实爆坑深度约为23.5 cm；试验E2的真实爆坑直径约为87.0 cm，真实爆坑深度约为27.5 cm。根据图9中传感器的位置判断，试验E1的爆坑唇缘直径约为140 cm，试验E2的爆坑唇缘直径约为160 cm。
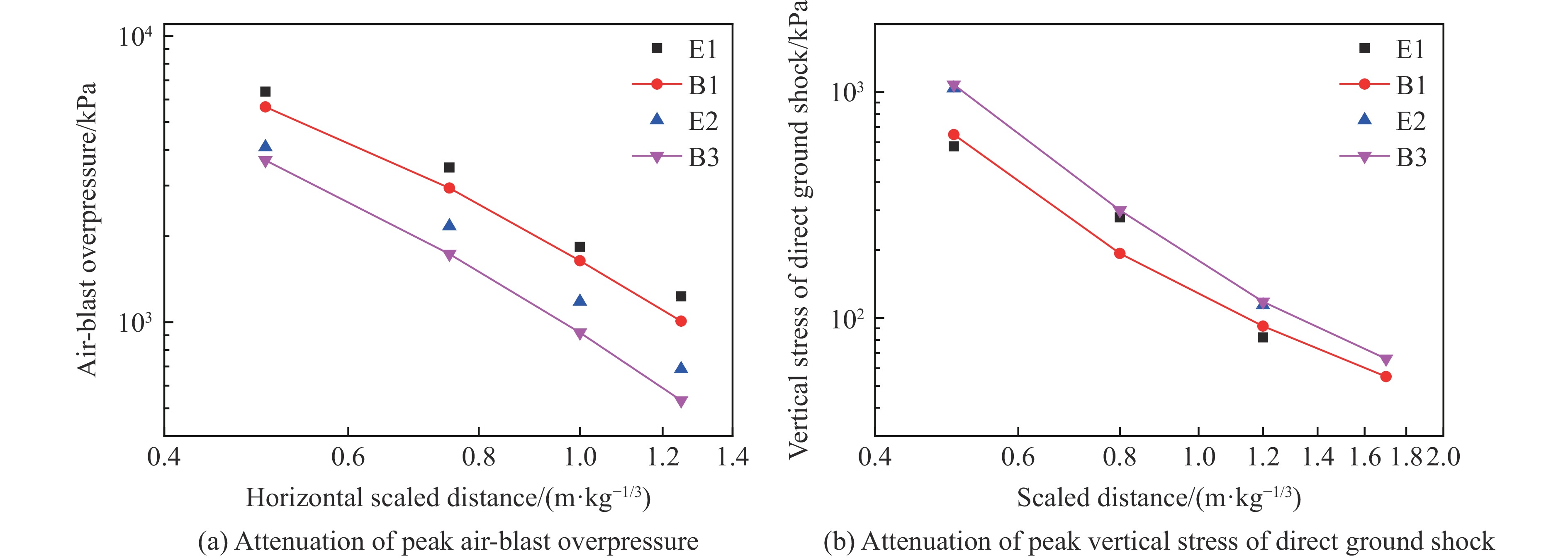
采用截止频率为2000 Hz的低通滤波方法处理试验数据，这2次试验中各测点的时程曲线如图10所示，由于数据异常，部分测点未给出曲线。对比这2次试验数据，随着装药比例埋深的增大，地面空气冲击波超压峰值减小，而土中直接地冲击应力峰值增大。图10～12和表6给出了试验与数值模拟结果的荷载峰值对比情况。其中，图10测得的地下应力，在不同距离的传感器上测得波形在相同的时间均出现1个类似的负向跳动，这是由于试验时传感器距离爆心较近，受到爆炸产生的电磁干扰。经计算，试验数据与数值模拟结果的平均偏差约为14.8%。由此表明，试验与模拟结果的一致性较好，可利用该计算模型做进一步分析研究。
图 10 地面空气冲击波超压和土中直接地冲击竖向应力时程曲线试验结果Figure 10. Tested time history curves of air-blast overpressure and vertical stress of direct ground shock
图 11 地面空气冲击波超压和土中直接地冲击竖向应力时程曲线数值模拟结果Figure 11. Numerically-simulated time history curves of air-blast overpressure and vertical stress of direct ground shock表 6 试验与数值模拟荷载峰值Table 6. Peak loads obtained by tests and simulations工况 测点 荷载峰值 工况 测点 荷载峰值 模拟值/kPa 试验值/kPa 偏差/% 模拟值/kPa 试验值/kPa 偏差/% E1（B1） A1 5650 6394 11.6 E2（B3） A1 3678 4092 10.1 A2 2943 3470 15.2 A2 1731 2167 20.1 E1（B1） A3 1640 1832 10.5 E2（B3） A3 920 1180 22.0 A4 1008 1231 18.1 A4 533 686 22.3 S1 649 575 12.9 S1 1079 1041 3.7 S2 193 279 30.8 S2 300 S3 92 82 12.2 S3 118 114 3.5 S4 55 S4 66 3. 地冲击作用分区
3.1 感生地冲击影响范围
基于已验证的计算模型，对土中爆炸地冲击效应作进一步数值模拟研究。以工况B3为例，其压力云图如图13所示，从图中发现土中应力波的时空分布与图1[9]相符，可将地冲击作用区域划分为表面区（即区域A和B）和中心区（区域C）。
图14(a)为表面区中地下5 cm处压力时程曲线，该时程曲线存在2个明显的峰值（即感生地冲击峰值和直接地冲击峰值）。图14(b)为中心区中地下40 cm处压力时程曲线，该时程曲线只存在1个峰值（即直接地冲击峰值）。由地下5 cm至地下40 cm处的压力时程曲线从2个峰值减少为1个峰值，表明随着深度的增加，地冲击作用区域的受力特征从感生地冲击与直接地冲击共同作用演变为主要受直接地冲击作用。进一步分析表面区的受力特征，如图15(a)所示，在区域A中地下20 cm处的竖向应力时程曲线存在2个峰值，且感生地冲击应力峰值明显大于直接地冲击应力峰值，此时感生地冲击起主导作用。随着深度增加，在区域B中地下35 cm处的竖向应力时程曲线如图15(b)所示，此时同样存在2个峰值，但感生地冲击应力峰值与直接地冲击应力峰值相当，说明该区域受感生地冲击和直接地冲击联合作用。根据表面区中不同深度竖向应力的特征，将表面区划分为地表区（区域A）和近地表区（区域B）。图14和图15中地下测点具体位置在图13中用红色点进行标注。
图 14 工况B3下爆心水平距离0.5 m处的压力时程曲线Figure 14. Pressure-time curves at the horizontal distance of 0.5 m from the detonation point under condition B3
图 15 工况B3下爆心水平距离0.5 m处竖向应力时程曲线Figure 15. Vertical stress time curves at the horizontal distance of 0.5 m from the detonation point under condition B33.2 装药比例埋深的影响
图16是工况B1～B10下1.0 ms时的压力云图，为了方便对比，压力云图截取了炸药周围2.5 m×3.5 m的区域。图16的压力云图采用了相同的标尺，可以发现相同时刻下，随着装药比例埋深的增加，黄土中直接地冲击的作用范围和压力值逐渐增大，而接近地面处空气冲击波的作用范围和压力值逐渐减小。
图 16 工况B1～B10下1.0 ms时的压力云图Figure 16. Pressure contours at 1.0 ms under conditions B1−B10图17以工况B3为例，给出了数值模拟的总能量时程曲线和计算模型中空气、黄土和TNT的动能时程曲线。由图17(a)可知，在6.4 ms左右，空气冲击波到达流出边界，随后空气冲击波溢出流出边界，导致计算模型的能量逐渐降低。在6.4 ms时，能量守恒误差为2.479×105 J，计算模型能量为5.377×107 J，能量守恒误差约为计算模型能量的0.46%；在计算终止时刻10 ms时，能量守恒误差为2.532×105 J，计算模型能量为4.898×107 J，能量守恒误差约为计算模型能量的0.52%。由图17(b)可知，计算模型中空气、黄土和TNT炸药的动能达到峰值的时间均在1 ms以内，此时空气冲击波尚未溢出流出边界。
图18给出了空气和黄土的动能峰值随装药比例埋深的变化情况。随装药比例埋深的增加，空气的动能峰值迅速减小，在装药比例埋深达到0.1 m/kg1/3后趋于稳定；黄土的动能峰值迅速增大，在装药比例埋深达到0.05 m/kg1/3后趋于稳定。这说明随着装药比例埋深的增加，空气冲击波获得的能量越来越少，更多的能量耦合进入黄土介质，这将导致感生地冲击影响范围减小。
图 18 工况B1～B10下空气和黄土的动能峰值随装药比例埋深的变化Figure 18. Peak kinetic energy of air and loess varying with charge scaled depth of burial under conditions B1−B10如图19(a)所示，以地平线为角的一条边，以对称轴和地平线的交点O为顶点；从距离点O水平距离500 mm处的点H向下选取数据测点。通过对比分析H点正下方不同深度处数据测点的压力时程曲线，同时根据压力云图中波阵面经过数据测点的大致时间，可以发现，随着深度的加深，感生地冲击压力峰值迅速减小。在一定深度后，从压力时程曲线上只能观察到直接地冲击峰值，观察不到感生地冲击峰值。图19(b)仅展示部分数据测点，实际为了判断准确，所取数据测点间隔更小。存在某一深度处测点的压力时程曲线从2个明显的峰值转变为1个明显的峰值，取这一点为点N，用90°减去∠HON即为γ的大小。然后，对比点H和点N之间的数据测点的竖向应力时程曲线（见图19(c)），可以发现，随着深度的增加，感生地冲击竖向应力峰值减小，直接地冲击竖向应力峰值增大。存在某一深度处测点的竖向应力时程曲线中，感生地冲击和直接地冲击的竖向应力峰值相等，取这一点为点M，则∠HOM=α，∠HON−∠HOM=β。
图20给出了工况B1～B10下的数值模拟结果。其中，α、β和γ分别表示图13中地表区、近地表区和中心区的角度，且α、β和γ三者之和为90°。随装药比例埋深的增大，α、β和γ的变化呈较强的规律性。
图 20 工况B1～B10下地冲击作用区的角度随装药比例埋深的变化Figure 20. Angles of ground shock subzones varying with charge scaled depth of burial under conditions B1−B10(1)当装药比例埋深为−0.05～0.075 m/kg1/3时，地冲击作用区角度的变化较剧烈。随着装药比例埋深的增加，中心区的角度γ迅速增大，地表区的角度α迅速减小，近地表区的角度β逐渐增大。
(2)当装药比例埋深为0.1～0.4 m/kg1/3时，地冲击作用区的角度趋于稳定。
3.3 炸药类型的影响
图21为工况C1～C12下1.0 ms时的压力云图，同样截取了炸药周围2.5 m×3.5 m的区域。图22给出了不同工况下空气和黄土的动能峰值的变化情况。不同工况下，直接地冲击和感生地冲击压力值不同，爆炸耦合进入空气和黄土介质动能存在差异，这将引起不同工况地冲击作用区角度的变化。
图 21 工况C1～C12下1.0 ms时的压力云图Figure 21. Pressure contours at 1.0 ms under conditions C1−C12
图 22 工况C1～C12下空气和黄土的动能峰值Figure 22. Peak kinetic energy of air and loess under conditions C1−C12表7给出了工况C1～C12下的数值模拟结果。表7中：p0.5为水平爆心距0.5 m处地面空气冲击波超压峰值，σ0.5为爆心正下方0.5 m处直接地冲击应力峰值，其位置可参见图6(b)）中A1和S1测点；Ia为水平爆心距0.5 m处地面空气冲击波超压的冲量，Is为爆心正下方0.5 m处直接地冲击应力的冲量。由图23可知，Ia/Is随炸药爆速和爆压的升高呈增大的趋势，即随着炸药爆速和爆压的升高，地面空气冲击波超压的冲量增大，而直接地冲击应力的冲量减小。
表 7 工况C1～C12下的数值模拟结果Table 7. Numerically simulated results under conditions C1−C12工况 炸药类型 α/(°) β/(°) γ/(°) p0.5/kPa σ0.5/kPa Ia/(kPa·ms) Is/(kPa·ms) Ia/Is C1 ANFO 23.12 6.99 59.89 2835.50 1636.93 137.3128 2336.691 0.05876 C2 C4 42.18 0.43 47.39 3733.34 893.77 159.3808 1138.626 0.13998 C3 EXPLOS.D 30.67 4.32 55.01 3451.48 1164.38 147.5805 1575.046 0.09370 C4 HMX 44.51 0.00 45.49 4125.33 849.82 161.8497 1077.647 0.15019 C5 HNS1.65 36.01 1.94 52.05 3794.17 1043.78 153.7076 1364.579 0.11264 C6 NM 34.75 1.75 53.50 3535.13 1121.93 154.8051 1491.994 0.10376 C7 PBX9407 42.09 0.00 47.91 3990.68 894.76 162.5825 1153.090 0.14100 C8 PBX9502 32.66 3.10 54.24 3932.76 1064.81 150.5989 1415.379 0.10640 C9 PETN1.77 45.35 0.00 44.65 3907.44 843.95 162.0005 1058.838 0.15300 C10 SEISMOPLAS 34.59 3.36 52.05 3592.10 983.94 150.9218 1301.707 0.11594 C11 TETRYL 38.58 1.45 49.97 3758.19 963.50 152.3302 1240.691 0.12278 C12 TNT 32.90 3.60 53.50 3562.00 1133.18 146.1541 1515.783 0.09642 
图 23 Ia/Is随炸药爆速和爆压的变化Figure 23. Variation of Ia/Is with detonation velocity and detonation pressure of explosive经分析，发现当Ia/Is的取值范围为0.05876～0.15300时，地冲击作用区角度与地面空气冲击波超压冲量和直接地冲击应力冲量之比呈线性相关关系（见图24），拟合后可得到：
{α=9.25517+234.01963Ia/IaIsIsβ=10.89993−74.47072Ia/IaIsIsγ=69.84490−159.54891Ia/IaIsIs (4) 式中：α、β和γ的单位均为(°)。
4. 结 论
针对土中爆炸应力波的时空分布问题，以数值模拟分析为主要手段，并辅以土中爆炸试验，得到以下主要结论。
(1)通过黄土中接触爆炸和半埋爆炸试验，得到了不同比例爆距上地面空气冲击波超压和土中直接地冲击竖向应力，并以此验证了所建立计算模型的有效性。
(2)根据土中不同深度压力和竖向应力的特征，将土中应力波场划分为地表区、近地表区和中心区。地表区的时程曲线出现2个峰值，且感生地冲击起主导作用；近地表区的时程曲线同样出现2个峰值，且受感生地冲击和直接地冲击的联合作用；中心区的时程曲线仅出现1个峰值，主要受直接地冲击的作用。
(3)通过研究不同装药比例埋深爆炸工况，发现当装药比例埋深为−0.05～0.075 m/kg1/3时，随着装药比例埋深的增加，土中应力波场的中心区迅速增大，地表区迅速减小，近地表区逐渐增大；当装药比例埋深为0.1～0.4 m/kg1/3时，地冲击作用区的分布趋于稳定。
(4)通过研究装药比例埋深为0.0 m/kg1/3时，不同类型炸药爆炸工况，发现随着炸药爆速和爆压的增加，地面空气冲击波超压的冲量增大，而直接地冲击应力的冲量减小，在一定范围内，地冲击作用区角度与地面空气冲击波超压冲量和直接地冲击应力冲量之比呈线性相关关系。
-
图 10 地面空气冲击波超压和土中直接地冲击竖向应力时程曲线试验结果
Figure 10. Tested time history curves of air-blast overpressure and vertical stress of direct ground shock
图 11 地面空气冲击波超压和土中直接地冲击竖向应力时程曲线数值模拟结果
Figure 11. Numerically-simulated time history curves of air-blast overpressure and vertical stress of direct ground shock
图 14 工况B3下爆心水平距离0.5 m处的压力时程曲线
Figure 14. Pressure-time curves at the horizontal distance of 0.5 m from the detonation point under condition B3
图 15 工况B3下爆心水平距离0.5 m处竖向应力时程曲线
Figure 15. Vertical stress time curves at the horizontal distance of 0.5 m from the detonation point under condition B3
图 16 工况B1～B10下1.0 ms时的压力云图
Figure 16. Pressure contours at 1.0 ms under conditions B1−B10
图 18 工况B1～B10下空气和黄土的动能峰值随装药比例埋深的变化
Figure 18. Peak kinetic energy of air and loess varying with charge scaled depth of burial under conditions B1−B10
图 20 工况B1～B10下地冲击作用区的角度随装药比例埋深的变化
Figure 20. Angles of ground shock subzones varying with charge scaled depth of burial under conditions B1−B10
图 21 工况C1～C12下1.0 ms时的压力云图
Figure 21. Pressure contours at 1.0 ms under conditions C1−C12
图 22 工况C1～C12下空气和黄土的动能峰值
Figure 22. Peak kinetic energy of air and loess under conditions C1−C12
图 23 Ia/Is随炸药爆速和爆压的变化
Figure 23. Variation of Ia/Is with detonation velocity and detonation pressure of explosive
表 1 计算工况
Table 1. Calculation conditions
工况 炸药类型 装药量/kg 装药比例埋深/(m·kg−1/3) 装药半径/mm 装药高度/mm B1 TNT 1 −0.05 44.2 100 B2 TNT 1 −0.025 44.2 100 B3 TNT 1 0 44.2 100 B4 TNT 1 0.025 44.2 100 B5 TNT 1 0.05 44.2 100 B6 TNT 1 0.075 44.2 100 B7 TNT 1 0.1 44.2 100 B8 TNT 1 0.2 44.2 100 B9 TNT 1 0.3 44.2 100 B10 TNT 1 0.4 44.2 100 C1 ANFO 1.380 0 61.8 123.6 C2 C4 0.655 0 40.2 80.4 C3 EXPLOS.D 0.968 0 47.7 95.4 C4 HMX 0.663 0 38.2 76.4 C5 HNS 1.65 0.815 0 42.8 85.6 C6 NM 0.814 0 48.6 97.2 C7 PBX9407 0.685 0 40.8 81.6 C8 PBX9502 0.987 0 43.6 87.2 C9 PETN 1.77 0.645 0 38.7 77.4 C10 SEISMOPLAS 0.835 0 43.7 87.4 C11 TETRYL 0.777 0 41.5 83.0 C12 TNT 1 0 46.0 92.0 
下载: 导出CSV
工况 炸药类型 ρ0/(g·cm−3) A/GPa B/GPa R1 R2 ω Dd/(m·s−1) pd/GPa E0/(GJ·m−3) C1 ANFO 0.931 49.46 1.891 3.907 1.118 0.333 4160 5.15 2.484 C2 C4 1.601 609.77 12.95 4.5 1.4 0.25 8193 28 9.0 C3 EXPLOS.D 1.42 300.7 3.94 4.3 1.2 0.35 6500 16 5.4 C4 HMX 1.891 778.28 7.0714 4.2 1.0 0.30 9110 42 10.5 C5 HNS 1.65 1.65 463.1 8.873 4.55 1.35 0.35 7030 21.5 7.45 C6 NM 1.128 209.25 5.689 4.4 1.2 0.30 6280 12.5 5.1 C7 PBX9407 1.60 573.2 14.64 4.6 1.4 0.32 7910 26.5 8.6 C8 PBX9502 1.895 460.3 9.544 4.0 1.7 0.48 7710 30.2 7.07 C9 PETN 1.77 1.77 617.05 16.926 4.4 1.2 0.25 8300 33.5 10.1 C10 SEISMOPLAS 1.588 620.60 23.27 5.399 1.651 0.282 7200 20.5 7.0 C11 TETRYL 1.73 586.83 10.671 4.4 1.2 0.275 7910 28.5 8.2 C12 TNT 1.63 373.77 3.7471 4.15 0.9 0.35 6930 21.0 6.0 B1～B10 TNT 1.63 373.77 3.7471 4.15 0.9 0.35 6930 21.0 6.0
下载: 导出CSV
冲击压实方程（参考密度ρ=2.641 g/cm3） 颗粒强度模型 压力/MPa 密度1/(g·cm−3) 声速/(km·s−1) 密度2/(g·cm−3) 压力/MPa 强度/MPa 密度/(g·cm−3) 剪切模量/GPa 0 1.674 0.2652 1.674 0 0 1.674 0.077 4.577 1.739 0.8521 1.745 3.40 4.23 1.746 0.869 14.98 1.874 1.722 2.086 3.49 44.7 2.086 4.03 29.15 1.997 1.875 2.147 101 124 2.147 4.91 59.17 2.144 2.265 2.300 185 226 2.300 7.77 98.09 2.250 2.956 2.572 500 226 2.572 14.8 179.4 2.380 3.112 2.598 2.598 16.6 289.4 2.485 4.600 2.635 静水拉力极限
pmin= −1.00 kPa2.635 36.7 450.2 2.585 4.634 2.641 2.641 37.3 650.7 2.670 4.634 2.800 2.800 37.3 注：(1)利用压力与密度1的分段线性函数来描述砂土的塑性压实过程；(2)利用声速与密度2的分段线性函数来描述砂土的弹性加载和卸载过程。
下载: 导出CSV
表 4 线性压实状态方程中压力与密度1的关系（基于式(3)）
Table 4. Relationship between pressure and density 1 in the compaction linear equation of state (based on formula (3))
密度1/(g·cm−3) 体应变εi = 1−ρi/−ρiρi+1ρi+1 累积体应变ε 修正后压力/MPa 1.674 0 0 0 1.739 0.03738 0.03738 1.750 1.874 0.07204 0.10942 6.844 1.997 0.06159 0.17101 21.96 2.144 0.06856 0.23957 80.42 2.250 0.04711 0.28668 196.2 2.380 0.05462 0.34131 551.8 2.485 0.04225 0.38356 1227.8 2.585 0.03868 0.42224 2553.6 2.670 0.03184 0.45408 4665.3
下载: 导出CSV
表 5 试验工况
Table 5. Test conditions
工况 装药量/kg 装药比例埋深/(m·kg−1/3) 地面空气冲击波超压测点 土中直接地冲击竖向应力测点 E1 1 −0.05 A1～A4 S1～S4 E2 1 0.00
下载: 导出CSV
表 6 试验与数值模拟荷载峰值
Table 6. Peak loads obtained by tests and simulations
工况 测点 荷载峰值 工况 测点 荷载峰值 模拟值/kPa 试验值/kPa 偏差/% 模拟值/kPa 试验值/kPa 偏差/% E1（B1） A1 5650 6394 11.6 E2（B3） A1 3678 4092 10.1 A2 2943 3470 15.2 A2 1731 2167 20.1 E1（B1） A3 1640 1832 10.5 E2（B3） A3 920 1180 22.0 A4 1008 1231 18.1 A4 533 686 22.3 S1 649 575 12.9 S1 1079 1041 3.7 S2 193 279 30.8 S2 300 S3 92 82 12.2 S3 118 114 3.5 S4 55 S4 66
下载: 导出CSV
表 7 工况C1～C12下的数值模拟结果
Table 7. Numerically simulated results under conditions C1−C12
工况 炸药类型 α/(°) β/(°) γ/(°) p0.5/kPa σ0.5/kPa Ia/(kPa·ms) Is/(kPa·ms) Ia/Is C1 ANFO 23.12 6.99 59.89 2835.50 1636.93 137.3128 2336.691 0.05876 C2 C4 42.18 0.43 47.39 3733.34 893.77 159.3808 1138.626 0.13998 C3 EXPLOS.D 30.67 4.32 55.01 3451.48 1164.38 147.5805 1575.046 0.09370 C4 HMX 44.51 0.00 45.49 4125.33 849.82 161.8497 1077.647 0.15019 C5 HNS1.65 36.01 1.94 52.05 3794.17 1043.78 153.7076 1364.579 0.11264 C6 NM 34.75 1.75 53.50 3535.13 1121.93 154.8051 1491.994 0.10376 C7 PBX9407 42.09 0.00 47.91 3990.68 894.76 162.5825 1153.090 0.14100 C8 PBX9502 32.66 3.10 54.24 3932.76 1064.81 150.5989 1415.379 0.10640 C9 PETN1.77 45.35 0.00 44.65 3907.44 843.95 162.0005 1058.838 0.15300 C10 SEISMOPLAS 34.59 3.36 52.05 3592.10 983.94 150.9218 1301.707 0.11594 C11 TETRYL 38.58 1.45 49.97 3758.19 963.50 152.3302 1240.691 0.12278 C12 TNT 32.90 3.60 53.50 3562.00 1133.18 146.1541 1515.783 0.09642
下载: 导出CSV
-
[1] WU C, LU Y, HAO H, et al. Characterisation of underground blast-induced ground motions from large-scale field tests [J]. Shock Waves, 2003, 13(3): 237–252. DOI: 10.1007/s00193-003-0212-3. [2] 何翔, 吴祥云, 李永池, 等. 石灰岩中爆炸成坑和地冲击传播规律的试验研究 [J]. 岩石力学与工程学报, 2004, 23(5): 725–729. DOI: 10.3321/j.issn:1000-6915.2004.05.004.HE X, WU X Y, LI Y C, et al. Testing study on crater formed by explosion and propagation laws of ground shock in limestone [J]. Chinese Journal of Rock Mechanics and Engineering, 2004, 23(5): 725–729. DOI: 10.3321/j.issn:1000-6915.2004.05.004. [3] LEONG E C, ANAND S, CHEONG H K, et al. Re-examination of peak stress and scaled distance due to ground shock [J]. International Journal of Impact Engineering, 2007, 34(9): 1487–1499. DOI: 10.1016/j.ijimpeng.2006.10.009. [4] 吴祥云, 刘国军, 杨仁华, 等. 常规装药爆炸埋深对自由场直接地冲击参数的影响 [J]. 防护工程, 2009, 31(5): 26–30.WU X Y, LIU G J, YANG R H, et al. The influence of buried depth of conventional charge on free field direct ground shock parameters [J]. Protective Engineering, 2009, 31(5): 26–30. [5] 叶亚齐, 任辉启, 李永池, 等. 砂质黏土中不同深度爆炸自由场地冲击参数预计方法研究 [J]. 岩石力学与工程学报, 2011, 30(9): 1918–1923. DOI: CNKI:SUN:YSLX.0.2011-09-024.YE Y Q, REN H Q, LI Y C, et al. Study of prediction of ground shock parameters in free field at different depths of burst in sandy clay [J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(9): 1918–1923. DOI: CNKI:SUN:YSLX.0.2011-09-024. [6] YANKELEVSKY D Z, KARINSKI Y S, FELDGUN V R. Re-examination of the shock wave’s peak pressure attenuation in soils [J]. International Journal of Impact Engineering, 2011, 38(11): 864–881. DOI: 10.1016/j.ijimpeng.2011.05.011. [7] JAYASINGHE L B, THAMBIRATNAM D P, PERERA N, et al. Blast induced ground shock effects on pile foundations [J]. International Journal of Civil, Environmental, Structural, Construction and Architectural Engineering, 2013, 7(4): 176–180. DOI: 10.5281/zenodo.1073675. [8] SONG J, LI S C. Study on numerical simulation of explosion in soil based on fluid-solid coupling arithmetic [J]. Applied Mechanics and Materials, 2014, 580-583: 2916–2919. DOI: 10.4028/www.scientific.net/AMM.580-583.2916. [9] ALEKSEYENKO V D, GRIGORYAN S S, KOSHELEV L I, et al. Measurement of stress waves in soft soil [R]. Hanover, NH, USA: U. S. Army Cold Regions Research and Engineering Laboratory, 1970: 12–19. [10] BESHARA F B A. Modelling of blast loading on aboveground structures: Ⅱ. internal blast and ground shock [J]. Computers & Structures, 1994, 51(5): 597–606. DOI: 10.1016/0045-7949(94)90067-1. [11] WU C Q, HAO H. Modeling of simultaneous ground shock and airblast pressure on nearby structures from surface explosions [J]. International Journal of Impact Engineering, 2005, 31(6): 699–717. DOI: 10.1016/j.ijimpeng.2004.03.002. [12] WU C Q, HAO H. Numerical simulation of structural response and damage to simultaneous ground shock and airblast loads [J]. International Journal of Impact Engineering, 2007, 34(3): 556–572. DOI: 10.1016/j.ijimpeng.2005.11.003. [13] 范俊余, 方秦, 柳锦春. 炸药地面爆炸条件下土中浅埋结构上荷载的作用特点 [J]. 解放军理工大学学报(自然科学版), 2008, 9(6): 676–680. DOI: 10.3969/j.issn.1009-3443.2008.06.026.FAN J Y, FANG Q, LIU J C. Characteristics of loads on shallow-buried structures under the ground explosions [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2008, 9(6): 676–680. DOI: 10.3969/j.issn.1009-3443.2008.06.026. [14] 柳锦春, 方秦, 还毅, 等. 炸药地面接触爆炸下土中感生地冲击的实用计算方法 [J]. 解放军理工大学学报(自然科学版), 2010, 11(2): 121–124. DOI: 10.3969/j.issn.1009-3443.2010.02.005.LIU J C, FANG Q, HUAN Y, et al. Practicable calculating method of indirect ground shock in soil at surface contact explosion [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2010, 11(2): 121–124. DOI: 10.3969/j.issn.1009-3443.2010.02.005. [15] 杨仁华, 张新乐, 吴祥云, 等. 间接地冲击在黄土中衰减规律的试验研究 [J]. 防护工程, 2010, 32(5): 6–9.YANG R H, ZHANG X L, WU X Y, et al. Experimental research on attenuation laws of airblast-induced ground shock in loess [J]. Protective Engineering, 2010, 32(5): 6–9. [16] 吴祥云, 曲建波, 李宝宝, 等. 岩石中装药埋深对地表空气冲击波超压的影响 [J]. 防护工程, 2013, 35(4): 23–26.WU X Y, QU J B, LI B B, et al. The influence of buried depth of a conventional charge on the direct ground shock parameters [J]. Protective Engineering, 2013, 35(4): 23–26. [17] KRAUTHAMMER T, BAZEOS N, HOLMQUIST T J. Modified SDOF analysis of RC box-type structures [J]. Journal of Structural Engineering, 1986, 112(4): 726–744. DOI: 10.1061/(ASCE)0733-9445(1986)112:4(726). [18] KRAUTHAMMER T, ASTARLIOGLU S. Direct shear resistance models for simulating buried RC roof slabs under airblast-induced ground shock [J]. Engineering Structures, 2017, 140: 308–316. DOI: 10.1016/j.engstruct.2017.02.056. [19] CHEE K H. Analysis of shallow buried reinforced concrete box structures subjected to airblast loads [D]. Gainesville, Florida, USA: University of Florida, 2008: 19–53. [20] 荣吉利, 宋逸博, 王玺, 等. 核爆炸对地冲击作用下土体运动特性等效模拟 [J]. 兵工学报, 2021, 42(1): 56–64. DOI: 10.3969/j.issn.1000-1093.2021.01.006.RONG J L, SONG Y B, WANG X, et al. Equivalent simulation of soil motion characteristics under the action of ground shock induced by nuclear explosion [J]. Acta Armamentarii, 2021, 42(1): 56–64. DOI: 10.3969/j.issn.1000-1093.2021.01.006. [21] 刘峥, 程怡豪, 邱艳宇, 等. 成层式防护结构抗超高速侵彻的数值分析 [J]. 爆炸与冲击, 2018, 38(6): 1317–1324. DOI: 10.11883/bzycj-2017-0181.LIU Z, CHENG Y H, QIU Y Y, et al. Numerical analysis on hypervelocity penetration into layered protective structure [J]. Explosion and Shock Waves, 2018, 38(6): 1317–1324. DOI: 10.11883/bzycj-2017-0181. [22] 陈材, 石全, 尤志锋, 等. 圆柱形弹药空气中爆炸相似性规律 [J]. 爆炸与冲击, 2019, 39(9): 092202. DOI: 10.11883/bzycj-2018-0255.CHEN C, SHI Q, YOU Z F, et al. Similarity law of cylindrical ammunition explosions in air [J]. Explosion and Shock Waves, 2019, 39(9): 092202. DOI: 10.11883/bzycj-2018-0255. [23] Century Dynamics Inc. Ansys/Autodyn Version 11.0, User Documentation [Z]. Pennsylvania, USA: Century Dynamics Inc, 2007: 89–112. [24] LEE E L, TARVER C M. Phenomenological model of shock initiation in heterogeneous explosives [J]. The Physics Fluids, 1980, 23(12): 2362. DOI: 10.1063/1.862940. [25] LUCCIONI B, AMBROSINI D, NURICK G, et al. Craters produced by underground explosions [J]. Computers & Structures, 2009, 87(21/22): 1366–1373. DOI: 10.1016/j.compstruc.2009.06.002. [26] FISEROVA D. Numerical analysis of buried mine explosions with emphasis on effect of soil properties on loading [D]. Cranfield, England: Cranfield University, 2006: 37–51. [27] LAINE L, SANDVIK A. Derivation of mechanical properties for sand [C]//Proceedings of the 4th Asia-Pacific Conference on Shock and Impact Loads on Structures. Singapore: CI-Premier PTE LTD, 2001: 361–368. [28] LAINE L. Study of planar ground shock in different soils and its propagation around a rigid block [C]//Proceedings of the 77th Shock and Vibration Symposium. Monterey, CA, USA: Shock and Vibration Information Analysis Center, 2006: 1–10. [29] LAINE L, LARSEN O P. Proposal on how to model the unloading in a compaction equation of state based upon tri-axial tests on dry sand [C]//Proceedings of the 80th Shock and Vibration Symposium. San Diego, USA: Shock and Vibration Information Analysis Center, 2009: 1–14. [30] LAINE L, LARSEN O P. Implementation of equation of state for dry sand in Autodyn [C]//Proceedings of the 83rd Shock and Vibration Symposium. New Orleans, USA: Shock and Vibration Exchange, 2012: 1–15. [31] 肖诗云, 林皋, 王哲. Drucker-Prager材料一致率型本构模型 [J]. 工程力学, 2003, 20(4): 147–151. DOI: 10.3969/j.issn.1000-4750.2003.04.026.XIAO S Y, LIN G, WANG Z. A Drucker-Prager consistent rate-dependent model [J]. Engineering Mechanics, 2003, 20(4): 147–151. DOI: 10.3969/j.issn.1000-4750.2003.04.026. [32] 张坤, 郑全平, 李四伟, 等. 土中爆炸对埋地管线冲击作用的数值模拟分析 [J]. 后勤工程学院学报, 2013, 29(3): 12–17; 23. DOI: 10.3969/j.issn.1672-7843.2013.03.003.ZHANG K, ZHENG Q P, LI S W, et al. Numerical simulation and analysis for impact of explosion under ground on buried pipelines [J]. Journal of Logistical Engineering University, 2013, 29(3): 12–17; 23. DOI: 10.3969/j.issn.1672-7843.2013.03.003. [33] 金辉, 张庆明, 高春生, 等. 装药水下沉底爆炸压力场特性研究 [J]. 科技导报, 2009, 27(14): 32–37. DOI: 10.3321/j.issn:1000-7857.2009.14.007.JIN H, ZHANG Q M, GAO C S, et al. Characteristics of pressure field in ground explosion [J]. Science & Technology Review, 2009, 27(14): 32–37. DOI: 10.3321/j.issn:1000-7857.2009.14.007. [34] 扶涛涛. 黄土湿陷和塌陷机理研究 [J]. 河南科技, 2018(23): 104–105. DOI: 10.3969/j.issn.1003-5168.2018.23.058.FU T T. Discussion on collapsibility and collapse mechanism of loess [J]. Henan Science and Technology, 2018(23): 104–105. DOI: 10.3969/j.issn.1003-5168.2018.23.058. [35] 王志良. 河南郑州-洛阳地区黄土湿陷机理研究 [D]. 北京: 中国地质科学院, 2013: 7–14.WANG Z L. The study on collapsible mechanism of loess between Zhengzhou with Luoyang of Henan region [D]. Beijing, China: Chinese Academy of Geological Sciences, 2013: 7-14. [36] 申永庆. 洛阳地区黄土湿陷性及其影响因素研究 [D]. 石家庄: 河北地质大学, 2015: 21–23.SHEN Y Q. Research on the collapsibility and influential factors of loess in Luoyang [D]. Shijiazhuang, Hebei, China: Hebei GEO University, 2015: 21–23. [37] 顾文彬, 叶序双, 詹发民, 等. 球形装药半无限土介质中爆炸动力学分析 [J]. 工程爆破, 1999, 5(1): 5–10. DOI: 10.3969/j.issn.1006-7051.1999.01.002.GU W B, YE X S, ZHAN F M, et al. Dynamic analysis on spherical charges exploding in semi-infinite soil medium [J]. Engineering Blasting, 1999, 5(1): 5–10. DOI: 10.3969/j.issn.1006-7051.1999.01.002. [38] 陈亚娟, 王利. 土介质中TNT炸药爆炸波传播特性的数值模拟 [J]. 河南理工大学学报(自然科学版), 2010, 29(1): 88–91. DOI: 10.3969/j.issn.1673-9787.2010.01.018.CHEN Y J, WANG L. Numerical study on the propagation and damage behavior of the blasting wave with TNT in soil medium [J]. Journal of Henan Polytechnic University (Natural Science), 2010, 29(1): 88–91. DOI: 10.3969/j.issn.1673-9787.2010.01.018. 期刊类型引用(9)
1. 董雨承. 柱形装药爆源条件对土介质爆炸痕迹影响的研究. 山东化工. 2025(02): 117-119 . 
百度学术2. 李岩巍，纪晓琳，于海，闫鸿浩. 侧穿桥桩的隧道爆破设计与模拟. 爆破. 2025(01): 97-106+174 . 百度学术3. 吕中杰，赵开元，王乐阳，刘彦，黄风雷，黄宁，杨剑波. 两点串联装药爆炸区域增效效果分析. 兵器装备工程学报. 2024(01): 27-34 . 百度学术4. 杨峰，翟红波，苏健军，李尚青，肖洋，刘伟. TNT和温压炸药浅埋爆炸效应差异性研究. 兵器装备工程学报. 2024(05): 160-166 . 百度学术5. 杨峰，翟红波，苏健军，李尚青，肖洋，刘伟. 浅埋爆炸空气冲击波峰值压力计算方法研究. 火工品. 2023(01): 42-45 . 百度学术6. 杨峰，翟红波，苏健军，李尚青，肖洋，刘伟. 装药埋深对地表空气冲击波影响的试验研究. 火炸药学报. 2023(04): 327-334 . 百度学术7. 位需贝，卢文波，王高辉. 饱和砂土分层地基爆炸液化引起的地表沉降特征分析. 爆破. 2023(02): 9-18 . 百度学术8. 崔莹，赵梦婷，李章剑，方军，赵奔. 基于SPH-FEM方法的土中浅埋爆炸成坑效应研究. 长江大学学报(自然科学版). 2023(06): 134-140 . 百度学术9. 胡智航，刘万通，孟强，白爽，何茂林. 无人机爆炸覆土灭火试验与仿真研究. 消防界(电子版). 2022(21): 51-54 . 百度学术其他类型引用(0)
-
下载:
百度学术
计量
- 文章访问数: 764
- HTML全文浏览量: 250
- PDF下载量: 152
- 被引次数: 9