Study on mechanical properties of the kinked rebar under high speed dynamic tension
-
摘要: 针对提高混凝土梁抗冲击爆炸性能的起波配筋新技术,采用理论分析与动态冲击拉伸试验相结合的方法,揭示了起波钢筋的快速拉伸变形作用机理,分析了拉伸速度、起波矢高等因素对起波钢筋抗拉强度的影响规律,确定了起波钢筋静态弹性极限强度计算方法。提出了起波钢筋等效拉伸应变率新概念,建立了弹性极限强度动力放大系数(dynamic increase factors,DIF)计算模型。研究结果表明,预先弯折起波使得钢筋在受力拉直过程中产生截面弯矩,起波钢筋的力学性能存在明显的应变率效应;起波钢筋弹性抗拉极限强度DIF随起波矢高的增高先增大后减小,存在一个最优起波设计矢高,可以使起波钢筋抗拉强度动态放大系数达到最大。研究成果可为进一步推动起波配筋技术在防护工程中的应用提供依据。Abstract: Aiming at new technology of the kinked rebar which can improve the resistance of concrete beams to impact and blast loading, the mechanism of rapid tensile deformation of the kinked rebar was revealed through theoretical analysis combined with dynamic impact tensile tests. The influences of the tensile velocity and the bending height of the kinked rebar on its tensile strength were analyzed. According to the mechanism of tensile deformation, the calculation method of the static elastic ultimate strength of the kinked rebar was determined by using the classical plastic mechanics theory, and the proposed calculation method was modified based on the existing literature data to consider the error caused by the Bauschinger effect. A new concept of equivalent tensile strain rate of the kinked rebar was put forward, the kinked rebar was regarded as an equivalent material, and the average strain rate of the bending part of the rebar was defined as the equivalent tensile strain rate of the kinked rebar. Considering the influence of the tensile velocity and the bending height of the kinked rebar, the calculation model of dynamic increase factors (DIF) of the elastic ultimate strength was established, referring to the form of the Johnson-Cook material constitutive model. The results show that the pre-bending kink results in the section bending moment of the steel bar during force straightening and the mechanical properties of the kinked rebar have an obvious strain rate effect. The tensile yield strength first increases and then decreases with the increase of the bending height of the kinked rebar. There is an optimal bending height for the kinked rebar at high strain rates, which can maximize the dynamic amplification factor of the tensile strength of the kinked rebar. The research results can provide a basis for further promotion of the application of the kinked rebar technology in protection engineering.
-
烤燃实验是分析和评估弹药易损性的重要方法,考核弹药在意外热刺激下的响应剧烈程度,评估弹药的不敏感性能[1-2]。复合推进剂是目前固体导弹或固体火箭的常用燃料,在遭受意外热刺激时,可能会发生点火,进而导致燃烧,甚至爆炸或爆轰等严重的事故[3]。
慢速烤燃特性是复合推进剂和发动机热安全性研究中的重点,也是目前研究的难点。针对含能材料在慢速烤燃条件下的安全性,国内外学者们开展了广泛的研究,探讨了升温速率、约束条件、结构尺寸等对烤燃响应结果的影响,建立了慢速烤燃的实验方法和理论模型,形成了考核标准[4-11]。陈朗等[12]、智小琦等[13]、周霖等[14]对DNAN基炸药在慢速烤燃条件下的安全性开展了研究,分析了炸药相变及流变特性对烤燃响应结果的影响。Foureur等[15]研究了高氯酸铵/端羟基聚丁二烯(AP/HTPB)推进剂受装药特性和升温速率的影响,发现在快速升温时反应剧烈程度低于慢速升温时。李文凤[16]研究了装药尺寸对AP/HTPB底排药烤燃特性的影响,发现内孔大小、装药长度对响应结果都有影响。宋柳芳等[17]通过研究不同结构端羟基聚醚(HTPE)推进剂装药的发动机慢速烤燃过程,发现结构和尺寸对发动机慢速烤燃结构影响很大。
在发动机的慢速烤燃低易损设计中,主控提前点火是一种工程上有效降低反应等级的途径。在提前点火设计中,主控点火温度的选择是设计的关键,然而,针对主控点火温度对复合推进剂慢速烤燃响应特性影响的研究较少。因此,研究主控点火温度对推进剂慢速烤燃响应特性的影响规律,了解缓慢加热条件下复合推进剂的热反应过程,对发动机慢速烤燃主控提前点火技术设计中主控点火温度的选择具有重要意义。
本文中针对主控点火温度对含AP复合推进剂的慢速烤燃过程的影响,设计了在不同温度下点火的慢速烤燃实验,并研究缓慢加热条件下推进剂热的分解失重及细观损伤,结合数值计算,探讨点火前推进剂内的温度分布及推进剂细观结构热损伤过程,并给出主控点火温度选择的建议,以期为发动机慢速烤燃主控点火温度的设计提供指导。
1. 实 验
1.1 实验装置
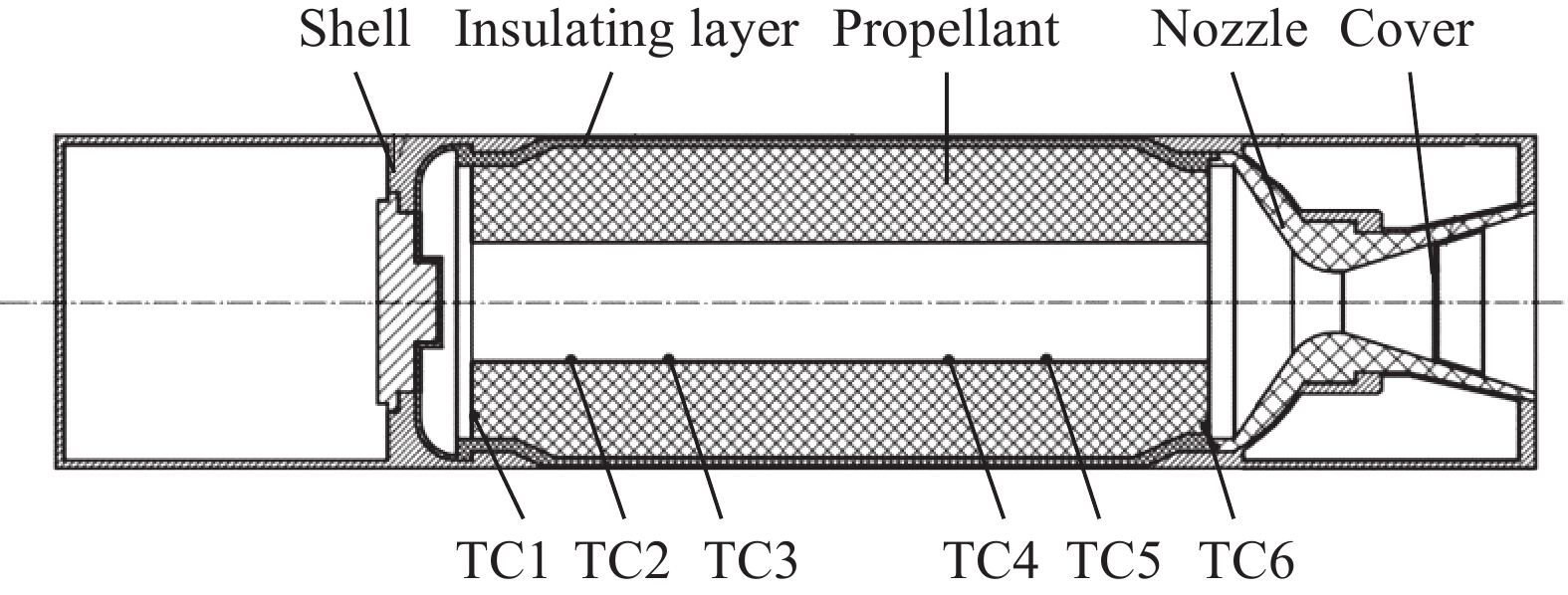
慢速烤燃实验系统包括烤燃件、烤燃箱、热电偶、压力传感器、温控系统及数据采集系统,图1~2分别为实验系统示意图和现场布置图。加热系统由温控系统、烤燃箱及热电偶组成,温控系统由控温热电偶和温控箱组成。热电偶测量加热过程中推进剂装药所处的环境温度,并将温度数据实时反馈到温控系统,温控系统通过调整加热功率实现对烤燃温度的控制,按照既定的升温程序对推进剂装药进行加热。在推进剂装药中预置多个热电偶监测烤燃过程中推进剂内部的温度变化。在距离烤燃件中心3.0和3.5 m处分别布置10个超压传感器,监测烤燃件发生响应时的冲击波超压。
烤燃件通过支架和固定工装固定在烤燃箱内,保证烤燃件可靠固定,如图3所示。烤燃件直径为200 mm,壳体厚度为3 mm,整体长度为900 mm,推进剂装药长度为450 mm,推进剂装药质量为17 kg,装药为HTPE复合推进剂。冲击波超压传感器采用PCB生产的137B24自由场传感器,采集器采集频率不低于2 MHz,采集时间为3 s。温度传感器采用铠装热电偶,测温范围为0~1 200 ℃,采样频率不低于2 Hz,持续采集温度信号,直至烤燃件发生反应。热电偶布置如图4所示,在烤燃件内部布置6路温度测试点,用于记录烤燃件内部推进剂的温度变化。
1.2 实验条件
实验条件参照MIL-2105D《美国非核弹药危险性评估试验》[18]标准中的规定:从室温以5 ℃/min的升温速率升温至50 ℃,保温8 h,再以3.3 ℃/h的升温速率对烤燃件进行烤燃,直至发生反应。为研究主控点火温度对烤燃响应剧烈程度的影响,进行2发实验:实验1按照升温条件持续加热,直至发生反应;实验2先按照升温条件进行加热,当温度到达120 ℃时,通过点火装置进行主动提前点火。
2. 数值计算
推进剂受热发生点火的过程通常可以表示为:(1)环境中热量传入反应系统;(2)反应系统分解放热;(3)分解产生的热量向环境散失。当反应系统产生的热量不能及时散失到环境时,系统温度上升,使分解加速,系统形成自热,使系统的温度更快地上升,最终导致燃烧或爆炸。
为建立计算模型,对烤燃过程作出如下假设[12]:
(1)推进剂与绝热层及绝热层与壳体间无间隙,且忽略接触热阻;
(2)推进剂装药在整个烤燃过程中为固态,相变的影响可忽略;
(3)推进剂装药的自热反应过程采用Arrhenius定律描述,化学反应为零级放热反应;
(4)各材料的物性参数及化学动力学参数为常量,不随温度变化。
在推进剂烤燃过程中,系统中固相的能量输运方程可以用下面的形式表达:
ρici∂T∂t=λi(∂2T∂r2+1r∂T∂r+∂2T∂x2)+Si (1) 式中:i表示烤燃件的不同结构材料;
ρi、ci 、λi 和Si 分别对应材料的密度、比热容、热导率和内热源;T为温度,r为圆柱坐标系中的径向距离;x为轴向距离。烤燃件中惰性材料内热源均为零,推进剂装药的内热源为其自热反应放热率。假设推进剂自热反应放热过程满足Arrhenius方程,按照如下所示的零级反应模型,反应速率表达式为:
dαdt=vf(α)=Aexp[−E/(RT)]f(α) (2) 式中:α为推进剂反应度;v为化学反应速率常数;
f(α) 为反应机理函数;A为指前因子,E为活化能,R为气体常数。则自热源项S可以表示为S=ρQAexp[−E/(RT)]f(α) (3) 式中:ρ为推进剂密度;Q为推进剂反应热;
f(α)=(1−α)n ,n为反应级数。由于假设化学反应为零级放热反应,因此n=0,f(α)=1 。烤燃件材料参数如表1所示,建立的数值计算模型如图5所示,在进行网格无关性验证后,发现当网格尺寸小于1 mm时,计算结果不受网格大小的影响。为更好地捕捉反应位置,对推进剂部分采用加密网格,网格尺寸为0.1 mm,空气域部分网格尺寸为0.5 mm,壳体和绝热层网格尺寸为0.2 mm,网格数量为148万。
3. 结果及讨论
3.1 实验结果
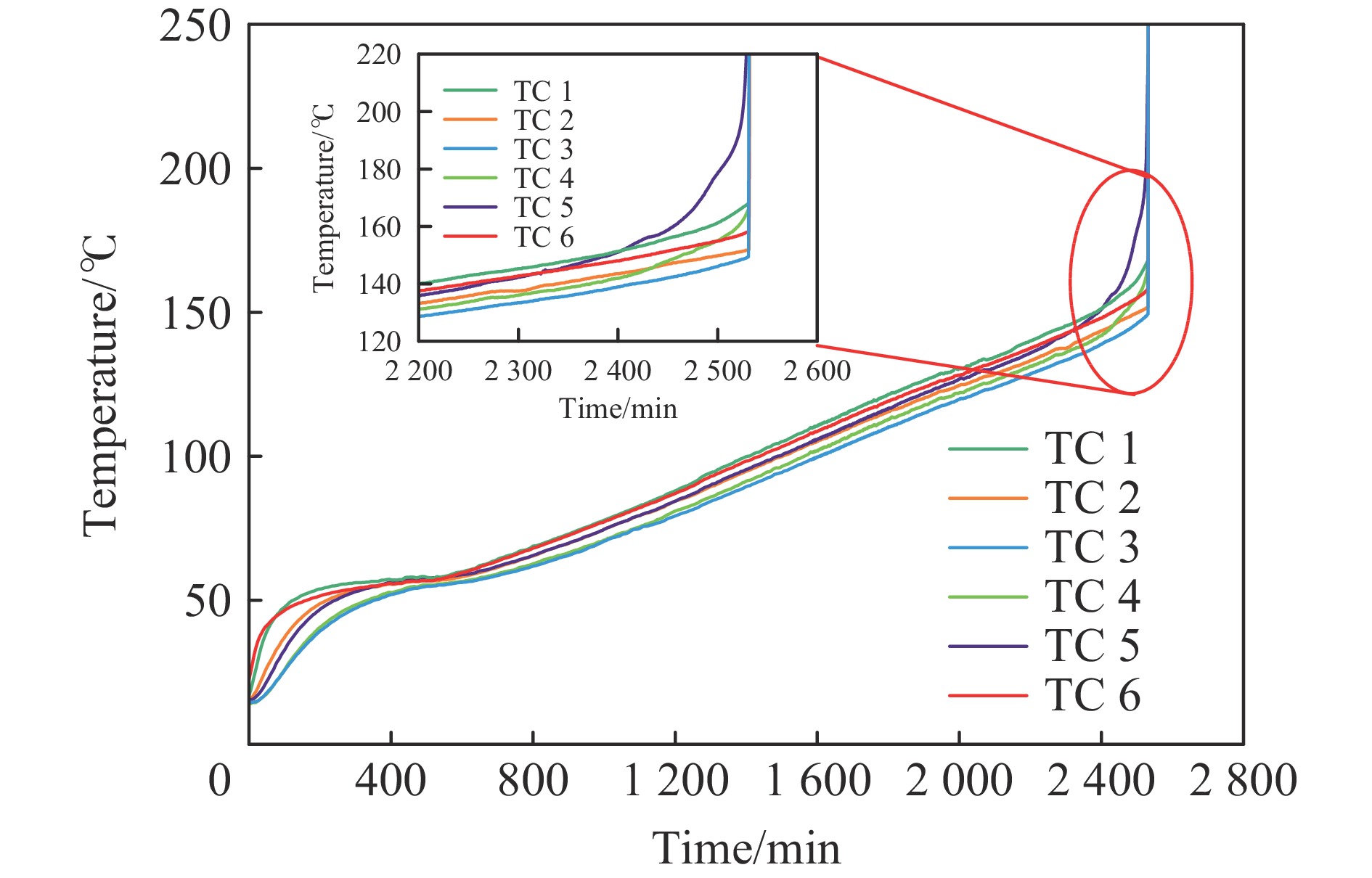
图6为实验1中热电偶测量得到的温度随时间变化曲线,可以看出,在加热的初始阶段,升温速率较高,靠近壳体表面的温度传感器TC1和TC6温度较高,内部靠近两端的温度传感器TC2和TC5温度次之,TC3和TC4温度最低。这是由于在慢速烤燃过程中,热量从壳体表面向装药内部传递,受传热速率的影响,温度从壳体表面到装药内部形成逐渐递减的温度梯度。经过8 h保温后,基本达到热平衡,各监测点温度相差很小。随后以3.3 K/h的升温速率加热时,温度分布从外向内有一个较小的温度差,直到达到推进剂的分解温度,推进剂组分发生分解放出热量。在推进剂热分解、外界加热及热传导的相互作用下,温度最高点逐渐向推进剂内部移动,最终高温区域移动到传感器TC5位置时达到点火温度,发生点火,初始反应位置在监测点5附近。
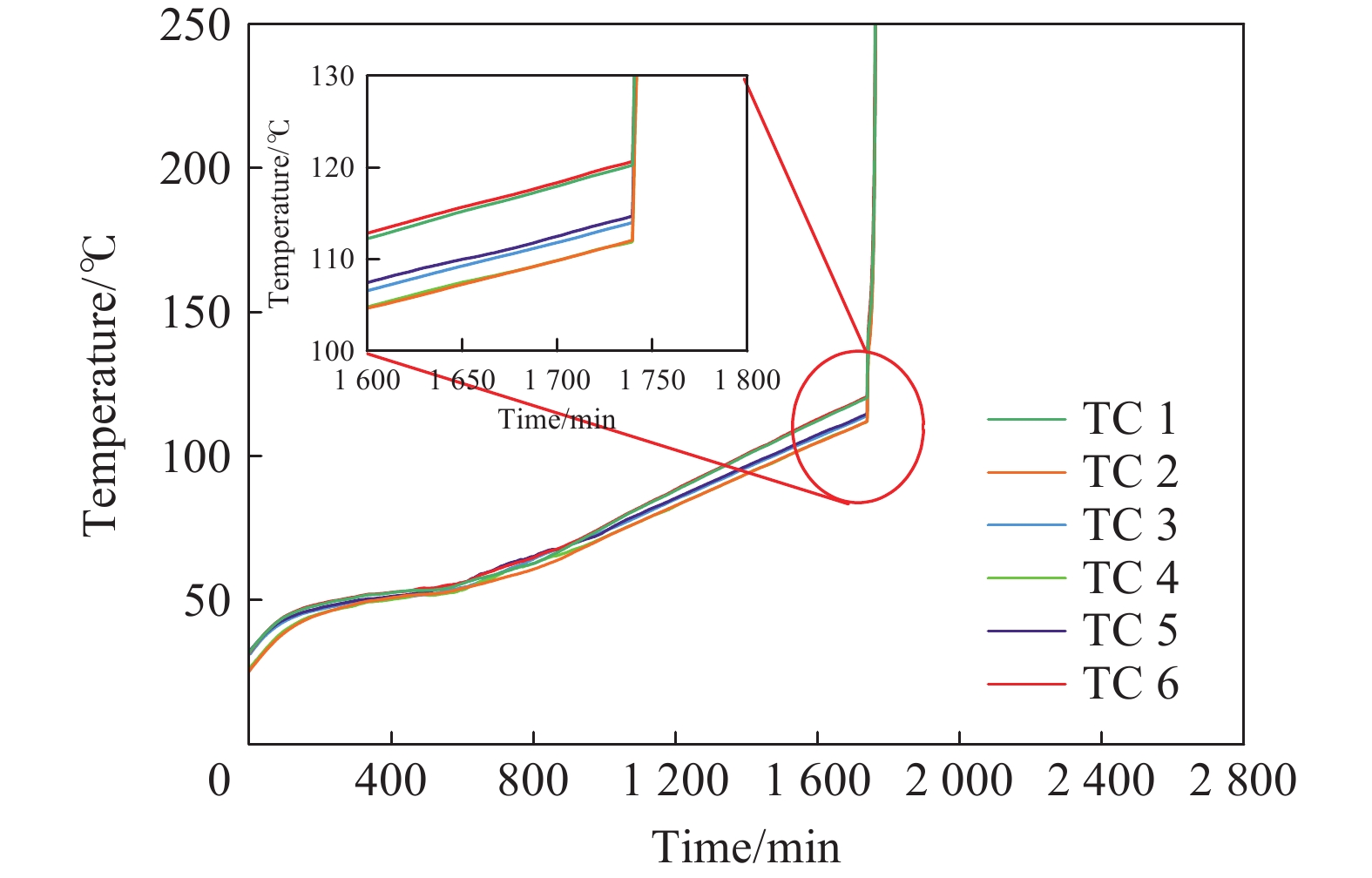
图7为实验2中监测点温度,实验2中选择在120 ℃时进行了人为干预,通过远程点火装置进行提前点火,点火位置位于靠近尾部的燃烧室内(TC5附近)。从监测点的温度分布上看,靠近壳体的推进剂温度更高,与实验1中前1768 min的数据基本一致,从温度分布和变化趋势上来看此时推进剂尚未发生明显的分解。
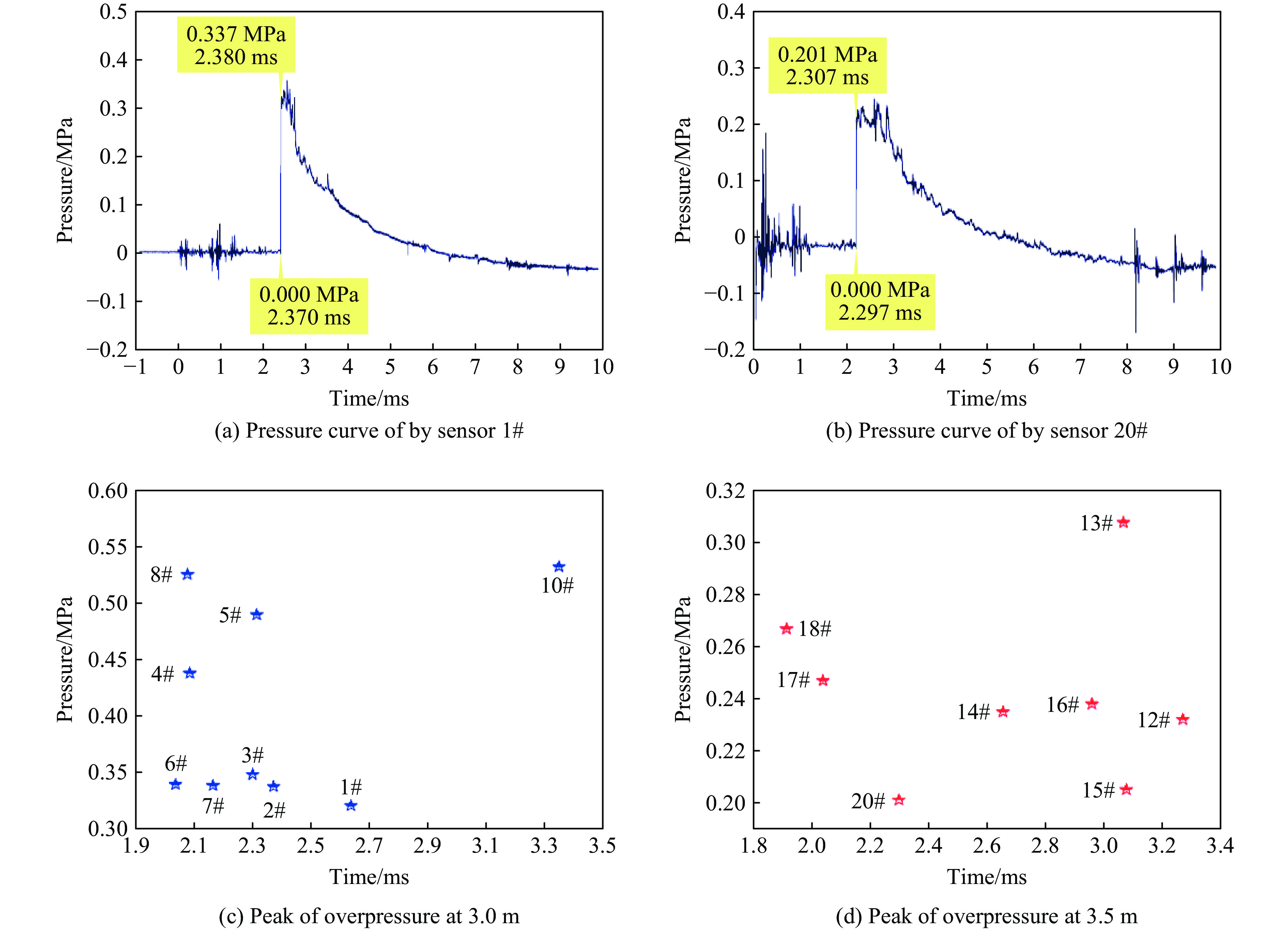
实验1反应后现场破坏情况如图8所示,反应后无残留推进剂,未找到壳体碎片和见证板碎片,烤燃箱体严重破坏,原地只留下少量变形严重金属残片,判断这些残片属于固定工装及烤燃箱,在距离烤燃箱30~100 m的区域找到了少量破坏严重的烤燃箱体碎片。图9为反应后压力传感器收集到的压力数据,测到有效数据17 个,9、11及19传感器损坏未测到有效数据。从压力曲线上可以看出,形成了典型的超压曲线,说明发生了爆炸及以上反应,对相同距离处的冲击波超压峰值求平均值,通过萨道夫斯基经验公式对TNT当量进行计算,TNT当量为10.35 kg,发生剧烈爆炸。
图10为实验2反应后现场破坏情况:烤燃件壳体两端冲开,侧壁完整无明显变形,烤燃箱两端烧穿,主体完整;未测到有效冲击波超压,最终反应等级判定为燃烧。对比两发实验,除点火温度不同外,其他条件相同,而实验2反应剧烈程度远低于实验1。这说明,在较低的温度下通过主动控制提前点火可以有效地缓解反应剧烈程度。
3.2 数值计算结果
根据实验条件建立数值模型,对烤燃过程进行数值模拟,图11所示为实验1中监测点5温度监测结果与计算结果的对比,可以看出计算结果与实验结果吻合良好,数值计算能较好地再现烤燃件的慢速烤燃过程。
为分析主控点火温度影响反应剧烈程度的原因,首先对点火前的推进剂内部的温度分布进行计算。图12所示为烤燃件在实验条件下进行慢速烤燃时的温度变化云图。图12(a)为烤燃1 min时的温度分布,开始加热后热量从壳体表面向推进剂内部传递。图12(b)对应实验2中点火时刻,可以看出,在实验2中开始点火时,温度最高区域位于壳体。这说明,此时推进剂未发生明显的分解,温度分布因从外部加热而呈现由外向内递减的趋势。继续加热,当靠近壳体表面的推进剂达到分解温度时,部分组分发生分解放出热量,高温区域逐渐由壳体转移到该部分推进剂,但是由于温度不是很高,分解速率较慢,放热不多,不足以引发热点积累导致整体点火。推进剂的整体温度在外部加热和推进剂缓慢分解的影响下逐渐升高,温度最高区域从壳体逐渐向装药内部转移,最终在监测点5附近发生点火,如图12(c)所示,与实验测试得到的温度数据吻合。可以看出,在发生点火前,推进剂温度在175 ℃以上,高温区域甚至在210 ℃以上,远高于壳体温度164 ℃。这说明,推进剂整体均发生了明显的分解,尤其是高温区域分解更为明显。
3.3 结果分析与讨论
对比实验1和实验2的结果,可以看出:实验1的点火是由于在加热过程中推进剂发生热分解,放出热量,随着温度的升高,分解速率加快,释放热量增加,最终热量的积累导致了推进剂的整体反应;而实验2在120 ℃时通过远程点火装置进行人为干预点火,此时推进剂本身尚未发生明显的分解。实验1发生剧烈爆炸,而实验2发生燃烧反应,这说明通过主动控制提前点火可以有效缓解发动机慢速烤燃响应的剧烈程度。影响因素中,温度越高,推进剂的燃烧速度越快[19];当点火温度较高时,推进剂中组分的分解导致的细观结构损伤对燃烧过程的影响。
为了分析在自热点火前推进剂的热分解过程,制备了相同配方的推进剂药片,按照1 ℃/min的升温速率,采用如图13所示的加热装置进行加热,并监测加热过程中推进剂药片的质量变化和形貌变化,推进剂初始质量为3.85 g。推进剂的质量和外观形貌变化如图14所示,在80 min前,推进剂的质量变化很小,继续加热,推进剂开始分解失重(由于控温热电偶在氧化铝片下方,测量温度为环境温度,热电偶采集温度低于推进剂表面实际温度),且随着温度的升高,质量损失速率加快,在发生点火前失重16.1%。推进剂在加热过程中颜色也有所加深,当加热到接近160 min时,推进剂从温度较高的四周开始发生明显分解,粘结剂体系的分解导致推进剂颜色变成白灰色,继续加热,整块推进剂都因粘结剂分解而变成白色,并在边缘形成了较大的裂纹,随后发生燃烧。
进一步分析由于推进剂组分分解导致的细观结构变化,重复上述实验,为保证样品加热环境相同,在同一氧化铝片上放置了多块推进剂药块,待加热到80、160 min时在高温条件下分别取出一块样品,待临近点火前停止加热,获得临界点火状态的推进剂样品。待样品冷却后,通过SEM分析加热导致的结构损伤,如图15所示。从图15中可以看出:在80 min以前没有明显的变化;但是加热到160 min时,粘结剂发生了明显分解,同时AP表面可以观察到少量分解导致的孔隙结构;当临近点火前,粘结剂分解严重,AP及铝粉已经几乎完全裸漏出来,同时AP也发生了明显的分解,在表面可以观察到大量孔状结构。在缓慢加热条件下推进剂中粘结剂及AP的分解导致推进剂装药的细观结构遭到严重破坏,这在点火后可能造成燃面增大,燃烧加速,导致剧烈的反应。
为分析提前点火温度对慢速烤燃响应特性的影响,先分析烤燃件慢速烤燃点火前推进剂高温区域分布特征。为避免推进剂中粘结剂及AP发生明显分解进而导致点火后的反应烈度增大,计算大于150 ℃时的推进剂区域随主控点火温度的变化,如图16所示,为主控点火温度为120、130 、140、150、160和164 ℃时的高温区域推进剂分布情况。
从图16中可以看出,在120和130 ℃点火时,推进剂内部温度没有达到150 ℃以上的区域;当点火温度达到140 ℃时,靠近喷管附近的少量推进剂温度已经达到了150 ℃以上,说明此时推进剂已经发生了部分分解并形成了热量积累,导致推进剂内部温度高于壳体表面温度。当点火温度达到150 ℃时,已经有较高比例的推进剂温度高于150 ℃,当点火温度达到160 ℃或自然点火时,全部推进剂温度都在150 ℃以上。
通过图像处理,进一步分析高于150 ℃的推进剂占总推进剂的比例。根据文献[20]中的方法,采用ImageJ软件和Matlab软件,计算不同温度下高温推进剂的占比,结果如图17所示,图中横坐标为壳体温度,纵坐标为对应时刻150 ℃以上推进剂占总推进剂的质量比。从图中可以看出,当壳体温度高于138 ℃时,出现超过150 ℃的推进剂占总推进剂的质量比出现快速增加,当壳体温度达到156 ℃时,全部的推进剂都达到150 ℃以上。因此,针对该烤燃件的慢速烤燃,可以认为在138 ℃以下提前点火,可以避免推进剂中AP发生分解,以达到降低点火后的燃烧演化速率及反应烈度的目的。
4. 结 论
针对点火温度对复合推进剂装药在慢速烤燃条件下的影响,开展了实验和数值计算研究,并对影响机制开展了分析,得到如下结论。
(1)针对复合推进剂的慢速烤燃,点火温度会对反应剧烈程度造成较大的影响,点火温度越低响应剧烈程度越低。以本文中研究的典型复合推进剂为例,在164 ℃发生点火时发生剧烈爆炸,在120 ℃点火时,发生燃烧反应,在推进剂达到分解温度之前提前点火,可以有效降低慢速烤燃响应等级。
(2)针对推进剂缓慢加热条件下的分解过程研究发现,推进剂在缓慢加热过程中粘结剂组分和AP会发生明显分解,产生裂纹和孔隙。数值计算结果表明,当壳体温度大于138 ℃,出现超过150 ℃的推进剂,随着壳体温度的升高,高温推进剂的占比快速提升,当壳体温度达到156 ℃时,全部推进剂都达到150 ℃以上,考虑到AP分解导致的孔隙结构对反应剧烈程度的影响,提前点火温度的设定应低于138 ℃。
(3)为了避免在慢速烤燃过程中推进剂中AP发生分解产生多孔结构进而导致点火后反应剧烈,通过一定的措施提前点火,降低点火时推进剂的温度,可以有效降低反应剧烈程度,技术上可行,降低反应烈度效果明显。
-
表 1 试验工况
Table 1. Test parameters
试件 起波矢高 h/mm 起波间距 s/mm 拉伸速度 v/(m·s−1) 试件数量 W1-2.5 40 150 2.5 3 W1-5 40 150 5.0 3 W1-10 40 150 10.0 3 W1-15 40 150 15.0 3 W2-2.5 50 150 2.5 3 W2-5 50 150 5.0 3 W2-10 50 150 10.0 3 W2-15 50 150 15.0 3 W3-2.5 60 150 2.5 3 W3-5 60 150 5.0 3 W3-10 60 150 10.0 3 W3-15 60 150 15.0 3 注:试件编号中W表示起波钢筋,字母后第1个数字表示不同起波矢高的钢筋组号,第2个数字表示拉伸速度值。 表 2 起波钢筋的静态弹性极限强度和抗拉极限
Table 2. Elastic ultimate strength and tensile capacity of the kinked rebar under static loading
钢筋参数 σ/MPa F/KN d=10 mm(直筋) 400.00 31.40 h=40 mm 127.66 32.50 h=50 mm 92.49 23.54 h=60 mm 71.99 18.32 表 3 各组起波钢筋的弹性极限强度
Table 3. Elastic ultimate strengths of the kinked rebars
拉伸速率
v/(m·s−1)弹性极限强度σ/MPa h=40 mm h=50 mm h=60 mm 2.5 159.46 105.78 93.50 5.0 193.99 140.19 111.03 10.0 201.13 198.50 137.56 15.0 255.40 261.14 175.61 表 4 三组DIF拟合公式中的
a 、b 值Table 4. Values of a and b of the three groups of the kinked rebars
起波失高h/mm a b 40 0.85 0.029700 50 1550 0.000033 60 6.086 0.013100 表 5 起波钢筋静态弹性极限强度理论值与试验值对比
Table 5. Comparison between the theoretical and experimental values of the static elastic ultimate strength
试件 极限强度σ/MPa 误差/
%修正后强度
σr/MPa修正后的
误差/%试验[1] 理论 F10-30 81.917 119.260 31.3 95.41 14.1 F10-45 67.486 61.586 9.6 49.27 37.0 F10-60 96.457 46.027 109.6 − − S10-30 34.127 46.167 26.1 36.93 7.6 S10-45 35.903 43.825 18.1 35.06 2.4 S10-60 17.850 24.140 26.1 19.31 7.6 -
[1] FENG P, QIANG H L, QIN W H, et al. A novel kinked rebar configuration for simultaneously improving the seismic performance and progressive collapse resistance of RC frame structures [J]. Engineering Structures, 2017, 147: 752–767. DOI: 10.1016/j.engstruct.2017.06.042. [2] 杨健翔. 起波钢筋RC梁柱子结构抗连续倒塌性能研究 [D]. 南京: 东南大学, 2019: 1−121. DOI: 10.27014/d.cnki.gdnau.2019.001378.YANG J X. Study on progressive collapse behavior of RC beam-column substructure with a kinked rebar configuration [D]. Nanjing: Southeast University, 2019: 1−121. DOI: 10.27014/d.cnki.gdnau.2019.001378. [3] 樊源, 陈力, 任辉启, 等. 起波配筋RC梁抗爆作用机理及抗力动力系数的理论计算方法 [J]. 爆炸与冲击, 2019, 39(3): 035102. DOI: 10.11883/bzycj-2018-0181.FAN Y, CHEN L, REN H Q, et al. Blast-resistant mechanism of RC beam with kinked rebar and calculation method of dynamic resistance coefficient [J]. Explosion and Shock Waves, 2019, 39(3): 035102. DOI: 10.11883/bzycj-2018-0181. [4] 陈力, 任辉启, 樊源, 等. 强动载作用下起波配筋梁抗力性能的试验研究 [J]. 土木工程学报, 2021, 54(10): 1–8;19. DOI: 10.15951/j.tmgcxb.2021.10.001.CHEN L, REN H Q, FAN Y, et al. Experimental study on the resistance of RC beam with kinked rebar under severe dynamic loading [J]. China Civil Engineering Journal, 2021, 54(10): 1–8;19. DOI: 10.15951/j.tmgcxb.2021.10.001. [5] 陈肇元, 王志浩, 方秦. 第十一篇 防护工程 [M] // 中国土木工程指南. 2版. 北京: 科学出版社, 2000: 1528−1529.CHEN Z Y, WANG Z H, FANG Q. Chapter eleven. Protection engineering [M] // Guide to Civil Engineering in China. 2nd ed. Beijing: Science Press, 2000: 1528−1529. [6] 方秦, 柳锦春. 地下防护结构 [M]. 北京: 中国水利水电出版社, 2010: 228−234.FANG Q, LIU J C. Underground protective structure [M]. Beijing: China Water and Power Press, 2010: 228−234. [7] SOROUSHIAN P, CHOI K B. Steel mechanical properties at different strain rates [J]. Journal of Structural Engineering, 1987, 113(4): 663–672. DOI: 10.1061/(ASCE)0733-9445(1987)113:4(663). [8] CEB. Concrete structures under impact and impulsive loading [R]. Lausanne: Comite Euro-International du Beton, 1988. [9] ROHR I, NAHME H, THOMA K. Material characterization and constitutive modelling of ductile high strength steel for a wide range of strain rates [J]. International Journal of Impact Engineering, 2005, 31(4): 401–433. DOI: 10.1016/j.ijimpeng.2004.02.005. [10] 林峰, 顾祥林, 匡昕昕, 等. 高应变率下建筑钢筋的本构模型 [J]. 建筑材料学报, 2008, 11(1): 14–20. DOI: 10.3969/j.issn.1007-9629.2008.01.003.LIN F, GU X L, KUANG X X, et al. Constitutive models for reinforcing steel bars under high strain rates [J]. Journal of Building Materials, 2008, 11(1): 14–20. DOI: 10.3969/j.issn.1007-9629.2008.01.003. [11] 李敏, 李宏男. 建筑钢筋动态试验及本构模型 [J]. 土木工程学报, 2010, 43(4): 70–75. DOI: 10.15951/j.tmgcxb.2010.04.012.LI M, LI H N. Dynamic test and constitutive model for reinforcing steel [J]. China Civil Engineering Journal, 2010, 43(4): 70–75. DOI: 10.15951/j.tmgcxb.2010.04.012. [12] 黄晓莹, 陶俊林. 三种建筑钢筋材料高应变率下拉伸力学性能研究 [J]. 工程力学, 2016, 33(7): 184–189. DOI: 10.6052/j.issn.1000-4750.2014.12.1064.HUANG X Y, TAO J L. Tensile mechanical properties research of three construction steel bars in high strain rate [J]. Engineering Mechanics, 2016, 33(7): 184–189. DOI: 10.6052/j.issn.1000-4750.2014.12.1064. [13] YU T X, JOHNSON W. Influence of axial force on the elastic-plastic bending and springback of a beam [J]. Journal of Mechanical Working Technology, 1982, 6(1): 5–21. DOI: 10.1016/0378-3804(82)90016-X. 期刊类型引用(3)
1. 边桂珍,曾江保,殷传传. 推进剂装药中的新材料与技术对安全性的影响. 山东化工. 2024(02): 212-214 .  百度学术
百度学术2. 许礼吉,白志玲,段卓平,黄风雷. 基于慢烤实验的混合炸药热传导系数计算方法. 含能材料. 2024(11): 1174-1181 .  百度学术
百度学术3. 王琦,张磊,冀功祥,黄开兴. 某专用炸药慢速烤燃数值模拟与试验研究. 数字海洋与水下攻防. 2024(06): 678-685 .  百度学术
百度学术其他类型引用(0)
-








 下载:
下载:

























 下载:
下载:













 百度学术
百度学术